Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T17:24:15.627Z Has data issue: false hasContentIssue false
  • English
  • Français

Approximation via Hausdorff operators

Part of: Harmonic analysis in one variable Approximations and expansions Integral transforms, operational calculus

Published online by Cambridge University Press:  13 August 2020

Alberto Debernardi  and
Elijah Liflyand
Alberto Debernardi
Affiliation:
Department of Mathematics, Bar-Ilan University, Ramat-Gan, Israel, 52900 e-mail: [email protected]
Elijah Liflyand*
Affiliation:
Department of Mathematics, Bar-Ilan University, Ramat-Gan, Israel, 52900 and Regional Mathematical Center of Southern Federal University, Bolshaya Sadovaya Str. 105/42, Rostov-on-Don, Russia, 344006
*
e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Truncating the Fourier transform averaged by means of a generalized Hausdorff operator, we approximate functions and the adjoint to that Hausdorff operator of the given function. We find estimates for the rate of approximation in various metrics in terms of the parameter of truncation and the components of the Hausdorff operator. Explicit rates of approximation of functions and comparison with approximate identities are given in the case of continuous functions from the class 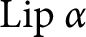$\text {Lip }\alpha $ .

Keywords

Hausdorff operatorsapproximation in Lebesgue spacesmoduli of continuity

MSC classification

Primary: 41A25: Rate of convergence, degree of approximation
Secondary: 42A38: Fourier and Fourier-Stieltjes transforms and other transforms of Fourier type 44A15: Special transforms (Legendre, Hilbert, etc.)
Type
Article
Information
Canadian Mathematical Bulletin , Volume 64 , Issue 3 , September 2021 , pp. 512 - 529
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© Canadian Mathematical Society 2020

Footnotes

Alberto Debernardi was supported by the ERC starting grant No. 713927 and the ISF grant No. 447/16. Elijah Liflyand is the corresponding author.

References

Andersen, K., Boundedness of Hausdorff operators on ${\textsf{L}}^p\left({\textsf{R}}^n\right),{\textsf{H}}^1\left({\textsf{R}}^n\right)$ , and $\mathrm{BMO}\left({\textsf{R}}^n\right)$ . Acta Sci. Math. (Szeged) 69(2003), 409–418.Google Scholar
Aizenberg, L. and Liflyand, E., Hardy spaces in Reinhardt domains, and Hausdorff operators. Illinois J. Math. 53(2009), 1033–1049.CrossRefGoogle Scholar
Brown, G. and Móricz, F., Multivariate Hausdorff operators on the spaces ${L}^p\left({\mathbb{R}}^n\right)$ , J. Math. Anal. Appl. 271(2002), 443–454.CrossRefGoogle Scholar
Burenkov, V. and Liflyand, E., Hausdorff operators on Morrey-type spaces. Kyoto J. Math. 60(2020), 93–106. http://dx.doi.org/10.1215/21562261-2019-0035CrossRefGoogle Scholar
Butzer, P. L. and Nessel, R. J., Fourier analysis and approximation. Volume 1: One-dimensional theory. Pure and Applied Mathematics, 40, Academic Press, New York-London, 1971.Google Scholar
Chen, J., Fan, D., and Wang, S., Hausdorff operators on Euclidean spaces. Appl. Math. J. Chinese Univ. (Ser. B) 28(2014), 548–564. http://dx.doi.org/10.1007/s11766-013-3228-1CrossRefGoogle Scholar
Dyachenko, M., Nursultanov, E., and Tikhonov, S., Hardy-Littlewood and Pitt’s inequalities for Hausdorff operators. Bull. Sci. Math. 147(2018), 40–57. http://dx.doi.org/10.1016/j.bulsci.2018.06.003CrossRefGoogle Scholar
Kanjin, Y., The Hausdorff operators on the real Hardy spaces ${H}^p\left(\mathbb{R}\right)$ . Studia Math. 148(2001), 37–45. http://dx.doi.org/10.4064/sm148-1-4CrossRefGoogle Scholar
Kuang, J. C., Generalized Hausdorff operators on weighted Morrey-Herz spaces. Acta Math. Sinica (Chin. Ser.) 55(2012), 895–902.Google Scholar
Kuang, J. C., Generalized Hausdorff operators on weighted Herz spaces. Mat. Vesnik 66(2014), 19–32.Google Scholar
Georgakis, C., The Hausdorff mean of a Fourier-Stieltjes transform, Proc. Am. Math. Soc. 116(1992), 465–471. http://dx.doi.org/10.2307/2159753CrossRefGoogle Scholar
Lerner, A. and Liflyand, E., Multidimensional Hausdorff operator on the real Hardy space. J. Austr. Math. Soc. 83(2007), 79–86. http://dx.doi.org/10.1017/S1446788700036399CrossRefGoogle Scholar
Liflyand, E., Hausdorff operators on Hardy spaces. Eurasian Math. J. 4(2013), 101–141.Google Scholar
Liflyand, E., Open problems on Hausdorff operators. In: Complex analysis and potential theory, World. Sci. Publ., Hackensack, NJ, 2007, pp. 280–285. http://dx.doi.org/10.1142/9789812778833_0030CrossRefGoogle Scholar
Liflyand, E. and Miyachi, A., Boundedness of the Hausdorff operators in ${H}^p$ spaces, $0<p<1$ . Studia Math. 194(2009), 279–292. http://dx.doi.org/10.4064/sm194-3-4CrossRefGoogle Scholar
Liflyand, E. and Miyachi, A., Boundedness of multidimensional Hausdorff operators in ${H}^p$ spaces, $0<p<1$ , Trans. Amer. Math. Soc. 371(2019), 4793–4814. http://dx.doi.org/10.1090/tran/7572CrossRefGoogle Scholar
Liflyand, E. and Móricz, F., The Hausdorff operator is bounded on the real Hardy space ${H}^1\left(\mathbb{R}\right)$ . Proc. Am. Math. Soc. 128(2000), 1391–1396. http://dx.doi.org/10.1090/S0002-9939-99-05159-XCrossRefGoogle Scholar
Mirotin, A. R., Boundedness of Hausdorff operators on real Hardy spaces ${H}^1$ over locally compact groups. J. Math. Anal. Appl. 473(2019), 519–533. http://dx.doi.org/10.1016/j.jmaa.2018.12.065CrossRefGoogle Scholar
Triebel, H., Theory of function spaces. Monographs in Mathematics, 78, Birkhäuser, Basel, 1983. http://dx.doi.org/10.1007/978-3-0346-0416-1Google Scholar