Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-14T01:29:53.843Z Has data issue: false hasContentIssue false
  • English
  • Français

The Weak Ideal Property and Topological Dimension Zero

Published online by Cambridge University Press:  20 November 2018

Cornel Pasnicu  and
N. Christopher Phillips
Cornel Pasnicu
Affiliation:
Department of Mathematics, The University of Texas at San Antonio, San Antonio TX 78249, USA e-mail: [email protected]
N. Christopher Phillips
Affiliation:
Department of Mathematics, University of Oregon, Eugene OR 97403-1222, USA
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Following up on previous work, we prove a number of results for 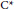${{\text{C}}^{*}}$-algebras with the weak ideal property or topological dimension zero, and some results for 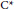${{\text{C}}^{*}}$-algebras with related properties. Some of the more important results include the following:

• The weak ideal property implies topological dimension zero.

• For a separable 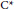${{\text{C}}^{*}}$-algebra $A$, topological dimension zero is equivalent to 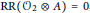$\text{RR}\left( {{\mathcal{O}}_{2}}\otimes A \right)=0$, to 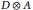$D\,\otimes \,A$ having the ideal property for some (or any) Kirchberg algebra $D$, and to $A$ being residually hereditarily in the class of all 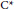${{\text{C}}^{*}}$-algebras $B$ such that 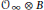${{\mathcal{O}}_{\infty }}\otimes B$ contains a nonzero projection.

• Extending the known result for 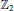${{\mathbb{Z}}_{2}}$, the classes of 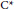${{\text{C}}^{*}}$-algebras with residual $\left( \text{SP} \right)$, which are residually hereditarily (properly) infinite, or which are purely infinite and have the ideal property, are closed under crossed products by arbitrary actions of abelian 2-groups.

• If $A$ and $B$ are separable, one of them is exact, $A$ has the ideal property, and $B$ has the weak ideal property, then 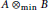$A\,{{\otimes }_{\min }}\,B$ has the weak ideal property.

• If 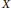$X$ is a totally disconnected locally compact Hausdorff space and $A$ is a ${{C}_{0}}\left( X \right)$-algebra all of whose fibers have one of the weak ideal property, topological dimension zero, residual $\left( \text{SP} \right)$, or the combination of pure infiniteness and the ideal property, then $A$ also has the corresponding property (for topological dimension zero, provided $A$ is separable).

• Topological dimension zero, the weak ideal property, and the ideal property are all equivalent for a substantial class of separable 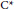${{\text{C}}^{*}}$-algebras, including all separable locally $\text{AH}$ algebras.

• The weak ideal property does not imply the ideal property for separable $Z$-stable 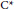${{\text{C}}^{*}}$-algebras.

We give other related results, as well as counterexamples to several other statements one might conjecture.

Keywords

46L05ideal propertyweak ideal propertytopological dimension zero C0(X)-algebrapurely infinite C* -algebra
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 69 , Issue 6 , 01 December 2017 , pp. 1385 - 1421
Copyright
Copyright © Canadian Mathematical Society 2017

References

[1] Atiyah, M. F., K-Theory.W. A. Benjamin, New York, 1967.Google Scholar
[2] Blackadar, B., Matricial and ultramatricial topology. In: Operator algebras, mathematical physics and low dimensional topology (Istanbul, 1991), R. Herman and B. Tanbay (eds.), A. K. Peters, Wellesley, MA, 1993, pp. 11–38.Google Scholar
[3] Blanchard, E. and Kirchberg, E., Non-simple purely infinite C' -algebras: The Hausdorff case. J. Funct. Anal. 207(2004), 461–513. http://dx.doi.Org/10.1016/j.jfa.2003.06.008 Google Scholar
[4] Bratteli, O. and Elliott, G. A., Structure spaces of approximately finite-dimensional C' -algebras, II. J. Funct. Anal. 30(1978), 74–82. http://dx.doi.Org/10.1016/0022-1236(78)90056-3 Google Scholar
[5] Brown, L. G. and Pedersen, G. K., C' -algebras of real rank zero. J. Funct. Anal. 99(1991), 131–149.http://dx.doi.Org/10.1016/0022-1236(91)90056-B Google Scholar
[6] Brown, L. G., Limits and C' -algebras of low rank or dimension. J. Operator Theory 61(2009), 381–417.Google Scholar
[7] Carrion, J. R. and Pasnicu, C., Approximations of C' -algebras and the ideal property. J. Math. Anal. Appl. 338(2008), 925–945.http://dx.doi.Org/10.1016/j.jmaa.2007.05.074 Google Scholar
[8] Dadarlat, M. and Gong, G.,A classification result for approximately homogeneous C' -algebras of real rank zero. Geom. Funct. Anal. 7(1997), 646–711.http://dx.doi.Org/10.1007/s000390050023 Google Scholar
[9] Dixmier, J., C' -Algebras. North-Holland, Amsterdam, 1977.Google Scholar
[10] Elliott, G. A., A classification of certain simple C' -algebras. In: Quantum and non-commutative analysis. H. Araki et al., eds. Kluwer, Dordrecht, 1993, pp. 373–385.Google Scholar
[11] Elliott, G. A., Gong, G., and Li, L., Injectivity of the connecting maps in AH inductive limit systems. Canad. Math. Bull. 48(2005), 50–68. http://dx.doi.org/10.4153/CMB-2005-005-9 Google Scholar
[12] Elliott, G. A., On the classification of simple inductive limit C' -algebras, II: The isomorphism theorem. Invent. Math. 168(2007), 249–320. http://dx.doi.org/10.1007/s00222-006-0033-y Google Scholar
[13] Fell, J. M. G., The structure of algebras of operator fields. Acta Math. 106(1961), 233–280. http://dx.doi.org/10.1007/BF02545788 Google Scholar
[14] Gong, G., Jiang, C., Li, L., and Pasnicu, C., structure of AH algebras with the ideal property and torsion free K-theory. J. Funct. Anal. 258(2010), 2119–2143. http://dx.doi.Org/10.1016/j.jfa.2009.11.016 Google Scholar
[15] Izumi, M., Finite group actions on C' -algebras with the Rohlin property. I. Duke Math. J. 122(2004), 233–280.http://dx.doi.org/10.1215/S0012-7094-04-12221-3 Google Scholar
[16] Jeong, J. A. and Osaka, H., Extremally rich C' -crossed products and the cancellation property. J. Austral. Math. Soc. (Series A) 64(1998), 285–301.http://dx.doi.Org/10.1017/S1446788700039161 Google Scholar
[17] Kirchberg, E., On permanence properties of strongly purely infinite C' -algebras. Preprint, 2003. (Preprintreihe SFB 478-Geometrische Strukturen in der Mathematik, Westfälische Wilhelmsuniversität Munster, Heft 284; ISSN 1435-1188). Google Scholar
[18] Kirchberg, E., The classification of purely infinite C' -algebras using Kasparov's theory. Preprint (3rd draft). Google Scholar
[19] Kirchberg, E. and Phillips, N. C., Embedding of exact C' -algebras in the Cuntz algebra . J. Reine Angew. Math. 525(2000), 17–53.http://dx.doi.org/10.1515/crll.2000.065 Google Scholar
[20] Kirchberg, E. and Rordam, M., Non-simple purely infinite C' -algebras. Amer. J. Math. 122(2000), 637–666.http://dx.doi.org/10.1353/ajm.2000.0021 Google Scholar
[21] Kirchberg, E., Infinite non-simple C' -algebras: absorbing the Cuntz algebra . Adv. Math. 167(2002), 195–264.http://dx.doi.Org/10.1OO6/aima.2OO1.2041 Google Scholar
[22] Kirchberg, E. and Wassermann, S., Operations on continuous bundles ofC' -algebras. Math.Annalen 303(1995), 677–697. http://dx.doi.org/10.1007/BF01461011 Google Scholar
[23] Nagisa, M., Osaka, H., and Phillips, N. C., Ranks of algebras of continuous C' -algebra valued functions. Canad. J. Math. 53(2001), 979–1030.http://dx.doi.org/10.4153/CJM-2001-039-8 Google Scholar
[24] Nilsen, M., C' -bundles and C0(X)-algebras. Indiana Univ. Math. J. 45(1996), 463–477.Google Scholar
[25] Pasnicu, C., Shape equivalence, nonstable K-theory and AH algebras. Pacific J. Math. 192(2000),159–182.http://dx.doi.Org/10.2140/pjm.2000.192.159 Google Scholar
[26] Pasnicu, C., On the AH algebras with the ideal property. J. Operator Theory 43(2000), 389–407.Google Scholar
[27] Pasnicu, C., The projection property. Glasgow Math. J. 44(2002), 293–300. http://dx.doi.Org/10.1017/S0017089502020104 Google Scholar
[28] Pasnicu, C., The ideal property and tensor products of C' -algebras. Rev. Roumaine Math. Pures Appl. 49(2004), 153–162.Google Scholar
[29] Pasnicu, C., Real rank zero and continuous fields of C' -algebras. Bull. Math. Soc. Sci. Math. Roumanie (N.S.) 48(96)(2005), 319–325.Google Scholar
[30] Pasnicu, C., The ideal property, the projection property, continuous fields and crossed products. J. Math. Anal. Appl. 323(2006), 1213–1224.http://dx.doi.Org/10.1016/j.jmaa.2005.11.040 Google Scholar
[31] Pasnicu, C., D-stable C' -algebras, the ideal property and real rank zero. Bull. Math. Soc. Sci. Math. Roumanie 52(2009), 177–192.Google Scholar
[32] Pasnicu, C. and Phillips, N. C., Permanence properties for crossed products and fixed point algebras of finite groups. Trans. Amer. Math. Soc. 366(2014), 4625–4648.http://dx.doi.org/10.1090/S0002-9947-2014-06036-4 Google Scholar
[33] Pasnicu, C., Crossed products by spectrally free actions. J. Funct. Anal. 269(2015), 915–967.http://dx.doi.Org/10.1016/j.jfa.2O15.04.020 Google Scholar
[34] Pasnicu, C. and Rordam, M., Tensor products of C' -algebras with the ideal property. J. Funct. Anal. 177(2000), 130–137.http://dx.doi.org/10.1006/jfan.2000.3630 Google Scholar
[35] Pasnicu, C., Purely infinite C' -algebras of real rank zero. J. Reine Angew. Math. 613(2007), 51–73. http://dx.doi.org/10.1515/CRELLE.2007.091 Google Scholar
[36] Pedersen, G. K., C' -Algebras and their automorphism groups. Academic Press, London, 1979.Google Scholar
[37] Phillips, N. C., Equivariant K-theory and freeness of group actions on C' -algebras. Lecture Notes in Mathematics, 1274. Springer-Verlag, Berlin, 1987.Google Scholar
[38] Phillips, N. C., A classification theorem for nuclear purely infinite simple C' -algebras. Documenta Math. 5(2000), 49–114 (electronic).Google Scholar
[39] Phillips, N. C., Freeness of actions of finite groups on C' -algebras. In: Operator structures and dynamical systems. M. de Jeu, S. Silvestrov, C. Skau, and J. Tomiyama, eds. Contemporary Mathematics, 503. American Mathematical Society, Providence RI, 2009, pp. 217–257.Google Scholar
[40] Razak, S., On the classification of simple stably projectionless C' -algebras. Canad. J. Math. 54(2002), 138–224.http://dx.doi.org/10.4153/CJM-2002-006-7 Google Scholar
[41] Rordam, M., Classification of extensions of certain C' -algebras by their six term exact sequences in K-theory. Math. Ann. 308(1997), 93–117.http://dx.doi.Org/10.1007/s002080050067 Google Scholar
[42] Rosenberg, J., Appendix to O. Bratteli's paper on “Crossed products of UHF algebras”. DukeMath. J. 46(1979), 25–26. http://dx.doi.org/10.1215/S0012-7094-79-04602-7 Google Scholar
[43] Schochet, C., Topological methods for C*-algebras II: geometric resolutions and the Künneth formula. Pacific J. Math. 98(1982), 443–458. http://dx.doi.org/10.1016/j.jfa.2015.05.013 Google Scholar
[44] Tikuisis, A., High-dimensional Z-stable AH algebras. J. Funct. Anal. 269(2015), 2171–2186. http://dx.doi.org/10.1016/j.jfa.2015.05.013 Google Scholar
[45] Wassermann, S., Tensor products of *-automorphisms of C*-algebras. Bull. London Math. Soc. 7(1975), 65–70. http://dx.doi.org/10.1112/blms/7.1.65 Google Scholar