Article contents
Distribution Algebras on p-adic Groups and Lie Algebras
Published online by Cambridge University Press: 20 November 2018
Abstract
When 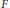 $F$ is a
$F$ is a 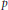 $p$-adic field, and
$p$-adic field, and  $G\,=\,\mathbb{G}\left( F \right)$ is the group of
$G\,=\,\mathbb{G}\left( F \right)$ is the group of 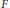 $F$-rational points of a connected algebraic
$F$-rational points of a connected algebraic 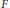 $F$-group, the complex vector space
$F$-group, the complex vector space 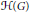 $\mathcal{H}\left( G \right)$ of compactly supported locally constant distributions on
$\mathcal{H}\left( G \right)$ of compactly supported locally constant distributions on  $G$ has a natural convolution product that makes it into a
$G$ has a natural convolution product that makes it into a  $\mathbb{C}$-algebra (without an identity) called the Hecke algebra. The Hecke algebra is a partial analogue for
$\mathbb{C}$-algebra (without an identity) called the Hecke algebra. The Hecke algebra is a partial analogue for 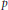 $p$-adic groups of the enveloping algebra of a Lie group. However,
$p$-adic groups of the enveloping algebra of a Lie group. However, 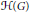 $\mathcal{H}\left( G \right)$ has drawbacks such as the lack of an identity element, and the process
$\mathcal{H}\left( G \right)$ has drawbacks such as the lack of an identity element, and the process 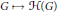 $G\,\mapsto \,\mathcal{H}\left( G \right)$ is not a functor. Bernstein introduced an enlargement
$G\,\mapsto \,\mathcal{H}\left( G \right)$ is not a functor. Bernstein introduced an enlargement 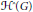 ${{\mathcal{H}}^{\hat{\ }}}\left( G \right)$ of
${{\mathcal{H}}^{\hat{\ }}}\left( G \right)$ of 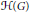 $\mathcal{H}\left( G \right)$. The algebra
$\mathcal{H}\left( G \right)$. The algebra 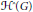 ${{\mathcal{H}}^{\hat{\ }}}\left( G \right)$ consists of the distributions that are left essentially compact. We show that the process
${{\mathcal{H}}^{\hat{\ }}}\left( G \right)$ consists of the distributions that are left essentially compact. We show that the process 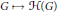 $G\,\mapsto \,{{\mathcal{H}}^{\hat{\ }}}\left( G \right)$ is a functor. If
$G\,\mapsto \,{{\mathcal{H}}^{\hat{\ }}}\left( G \right)$ is a functor. If 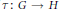 $\tau \,:\,G\,\to \,H$ is a morphism of
$\tau \,:\,G\,\to \,H$ is a morphism of 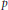 $p$-adic groups, let
$p$-adic groups, let  $F\left( \tau \right):\,{{\mathcal{H}}^{\hat{\ }}}\left( G \right)\,\to \,{{\mathcal{H}}^{\hat{\ }}}\left( H \right)$ be the morphism of
$F\left( \tau \right):\,{{\mathcal{H}}^{\hat{\ }}}\left( G \right)\,\to \,{{\mathcal{H}}^{\hat{\ }}}\left( H \right)$ be the morphism of  $\mathbb{C}$-algebras. We identify the kernel of
$\mathbb{C}$-algebras. We identify the kernel of 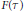 $F\left( \tau \right)$ in terms of
$F\left( \tau \right)$ in terms of 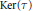 $\text{Ker}\left( \tau \right)$. In the setting of
$\text{Ker}\left( \tau \right)$. In the setting of 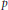 $p$-adic Lie algebras, with
$p$-adic Lie algebras, with  $\mathfrak{g}$ a reductive Lie algebra,
$\mathfrak{g}$ a reductive Lie algebra, 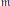 $\mathfrak{m}$ a Levi, and
$\mathfrak{m}$ a Levi, and 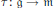 $\tau \,:\,\mathfrak{g}\,\to \,\mathfrak{m}$ the natural projection, we show that
$\tau \,:\,\mathfrak{g}\,\to \,\mathfrak{m}$ the natural projection, we show that 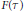 $F\left( \tau \right)$ maps
$F\left( \tau \right)$ maps  $G$-invariant distributions on
$G$-invariant distributions on 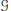 $\mathcal{G}$ to
$\mathcal{G}$ to 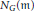 ${{N}_{G}}\left( \mathfrak{m} \right)$-invariant distributions on
${{N}_{G}}\left( \mathfrak{m} \right)$-invariant distributions on 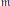 $\mathfrak{m}$. Finally, we exhibit a natural family of
$\mathfrak{m}$. Finally, we exhibit a natural family of  $G$-invariant essentially compact distributions on
$G$-invariant essentially compact distributions on  $\mathfrak{g}$ associated with a
$\mathfrak{g}$ associated with a  $G$-invariant non-degenerate symmetric bilinear form on
$G$-invariant non-degenerate symmetric bilinear form on  $\mathfrak{g}$ and in the case of
$\mathfrak{g}$ and in the case of 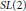 $SL\left( 2 \right)$ show how certain members of the family can be moved to the group.
$SL\left( 2 \right)$ show how certain members of the family can be moved to the group.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2011
References
- 2
- Cited by


