Research Article
A note on coverings and Kervaire complexes
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 1-21
-
- Article
-
- You have access
- Export citation
Isometric immersion of a compact Riemannian manifold into a Euclidean space
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 177-178
-
- Article
-
- You have access
- Export citation
On orders of directly indecomposable finite rings
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 353-359
-
- Article
-
- You have access
- Export citation
The number of factors in a paperfolding sequence
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 23-32
-
- Article
-
- You have access
- Export citation
A certain fixed point theorem and its applications to integral-functional equations
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 179-186
-
- Article
-
- You have access
- Export citation
A note on analytic capacity
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 361-365
-
- Article
-
- You have access
- Export citation
Stability of weak normal structure in James quasi reflexive space
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 367-372
-
- Article
-
- You have access
- Export citation
A remark on Gelfand duality
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 187-197
-
- Article
-
- You have access
- Export citation
Polar locally convex topologies and Attouch-Wets convergence
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 33-46
-
- Article
-
- You have access
- Export citation
Multipliers in continuous vector-valued function spaces
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 199-204
-
- Article
-
- You have access
- Export citation
Invex optimisation problems
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 47-66
-
- Article
-
- You have access
- Export citation
Oscillations of certain partial differential equations with deviating arguments
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 373-380
-
- Article
-
- You have access
- Export citation
A selection theorem and its applications
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 205-212
-
- Article
-
- You have access
- Export citation
On strongly right bounded finite rings II
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 381-384
-
- Article
-
- You have access
- Export citation
A continuity property related to an index of non-separability and its applications
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 67-79
-
- Article
-
- You have access
- Export citation
On sufficiency of the Kuhn-Tucker conditions in nondifferentiable programming
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 385-389
-
- Article
-
- You have access
- Export citation
Right alternative algebras with commutators in a nucleus
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 81-90
-
- Article
-
- You have access
- Export citation
Small embeddings of partial directed cycle systems
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 213-224
-
- Article
-
- You have access
- Export citation
Representations of finite groups and Cuntz-Krieger algebras
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 225-243
-
- Article
-
- You have access
- Export citation
On the Schwarzian coefficients of univalent functions
-
- Published online by Cambridge University Press:
- 17 April 2009, pp. 391-400
-
- Article
-
- You have access
- Export citation

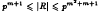 . As a consequence, we have that, for a given finite nilpotent ring
. As a consequence, we have that, for a given finite nilpotent ring  .
.