Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T12:03:00.239Z Has data issue: false hasContentIssue false
  • English
  • Français

EXTENDABLE TEMPERATURES

Part of: Parabolic equations and systems Higher-dimensional theory

Published online by Cambridge University Press:  27 February 2019

NEIL A. WATSON Open the ORCID record for NEIL A. WATSON [Opens in a new window]
NEIL A. WATSON*
Affiliation:
School of Mathematics and Statistics, University of Canterbury, Private Bag, Christchurch, New Zealand email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

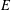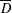Let $E$ and $D$ be open subsets of $\mathbb{R}^{n+1}$ such that $\overline{D}$ is a compact subset of 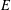$E$, and let $v$ be a supertemperature on 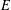$E$. We call a temperature $u$ on 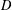$D$extendable by$v$ if there is a supertemperature 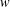$w$ on 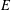$E$ such that 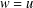$w=u$ on 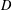$D$ and 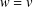$w=v$ on $E\backslash \overline{D}$. Such a temperature need not be a thermic minorant of $v$ on 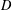$D$. We show that either there is a unique temperature extendable by $v$, or there are infinitely many. Examples of temperatures extendable by $v$ include the greatest thermic minorant 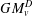$GM_{v}^{D}$ of $v$ on 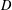$D$, and the Perron–Wiener–Brelot solution of the Dirichlet problem 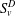$S\!_{v}^{D}$ on 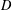$D$ with boundary values the restriction of $v$ to 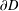$\unicode[STIX]{x2202}D$. In the case where these two examples are distinct, we give a formula for producing infinitely many more. Clearly 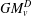$GM_{v}^{D}$ is the greatest extendable thermic minorant, but we also prove that there is a least one, which is not necessarily equal to $S\!_{v}^{D}$.

Keywords

temperaturesupertemperaturethermic minorant

MSC classification

Primary: 35K05: Heat equation
Secondary: 31B05: Harmonic, subharmonic, superharmonic functions 31B10: Integral representations, integral operators, integral equations methods 31B20: Boundary value and inverse problems
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 100 , Issue 2 , October 2019 , pp. 297 - 303
Copyright
© 2019 Australian Mathematical Publishing Association Inc. 

References

Chow, B., Chu, S.-C., Glickenstein, D., Guenther, C., Isenberg, J., Ivey, T., Knoph, D., Lu, P., Luo, F. and Ni, L., The Ricci Flow: Techniques and Applications: Part III: Geometric-Analytic Aspects, Mathematical Surveys and Monographs, 163 (American Mathematical Society, Providence, RI, 2010).Google Scholar
Doob, J. L., Classical Potential Theory and its Probabilistic Counterpart, Grundlehren der mathematischen Wissenschaften, 262 (Springer, New York, 1984).10.1007/978-1-4612-5208-5Google Scholar
Ecker, K., Regularity Theory for Mean Curvature Flow, Progress in Nonlinear Differential Equations and their Applications, 57 (Birkhäuser, Basel, 2004).10.1007/978-0-8176-8210-1Google Scholar
Evans, L. C., Partial Differential Equations, Graduate Studies in Mathematics, 19 (American Mathematical Society, Providence, RI, 1998).Google Scholar
Watson, N. A., ‘Mean values of subtemperatures over level surfaces of Green functions’, Ark. Mat. 30 (1992), 165–185.Google Scholar
Watson, N. A., Introduction to Heat Potential Theory, Mathematical Surveys and Monographs, 182 (American Mathematical Society, Providence, RI, 2012).Google Scholar
Watson, N. A., ‘Thermic minorants and reductions of supertemperatures’, J. Aust. Math. Soc. 99 (2015), 128–144.Google Scholar
Watson, N. A., ‘Extensions of Green functions and the representation of greatest thermic minorants’, New Zealand J. Math. 47 (2017), 97–110.Google Scholar