We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let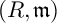 
$(R,\mathfrak {m})$ be a Noetherian local ring and I an ideal of R. We study how local cohomology modules with support in 
$\mathfrak {m}$ change for small perturbations J of I, that is, for ideals J such that 
$I\equiv J\bmod \mathfrak {m}^N$ for large N, under the hypothesis that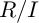 
$R/I$ and 
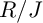$R/J$ share the same Hilbert function. As one of our main results, we show that if 
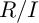$R/I$ is generalized Cohen–Macaulay, then the local cohomology modules of 
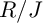$R/J$ are isomorphic to the corresponding local cohomology modules of 
$R/I$, except possibly the top one. In particular, this answers a question raised by Quy and V. D. Trung. Our approach also allows us to prove that if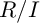 
$R/I$ is Buchsbaum, then so is 
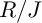$R/J$. Finally, under some additional assumptions, we show that if 
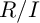$R/I$ satisfies Serre’s property 
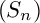$(S_n)$, then so does 
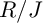$R/J$.
