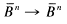Search

Let Ω be an irreducible bounded symmetric domain and $\Gamma \subset {\rm Aut}(\Omega)$ be a torsion-free discrete group of automorphisms, $X: = \Omega/\Gamma$. We study the problem of algebro-geometric and differential-geometric characterizations of certain compact holomorphic geodesic cycles $S \subset X$. We treat special cases of the problem, pertaining to a situation in which S is a compact holomorphic curve, and to the case where Ω is a classical domain dual to the hyperquadric. In both cases we consider algebro-geometric characterizations in terms of tangent subspaces. As a consequence we derive effective pinching theorems where certain complex submanifolds $S \subset X$ are proven to be totally geodesic whenever their scalar curvatures are pinched between certain computed universal constants, independent of the volume of the submanifold S, giving new examples of the gap phenomenon for the characterization of compact holomorphic geodesic cycles.

Search Results

Refine search

Refine search

Actions for selected content:

2 results

Characterization of Certain Holomorphic Geodesic Cycles on Quotients of Bounded Symmetric Domains in terms of Tangent Subspaces

Fixed Points of Holomorphic Mappings in the Cartesian Product of n Unit Hilbert Balls

Search Results

Refine search

Refine search

Actions for selected content:

Save Search

2 results

Characterization of Certain Holomorphic Geodesic Cycles on Quotients of Bounded Symmetric Domains in terms of Tangent Subspaces

Fixed Points of Holomorphic Mappings in the Cartesian Product of n Unit Hilbert Balls