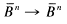Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kuczumow, Tadeusz
1986.
Holomorphic retracts of polyballs.
Proceedings of the American Mathematical Society,
Vol. 98,
Issue. 2,
p.
374.
Kuczumow, Tadeusz
and
Ray, William O.
1988.
Isometers in the Cartesian product ofn unit open Hilbert balls with a hyperbolic metric.
Annali di Matematica Pura ed Applicata,
Vol. 152,
Issue. 1,
p.
359.
Kuczumow, T.
and
Stachura, A.
1989.
Common fixed points of commuting holomorphic mappings.
Kodai Mathematical Journal,
Vol. 12,
Issue. 3,
Reich, Simeon
and
Shafrir, Itai
1990.
Nonexpansive iterations in hyperbolic spaces.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 15,
Issue. 6,
p.
537.
Shafrir, Itai
1990.
The approximate fixed point property in Banach and hyperbolic spaces.
Israel Journal of Mathematics,
Vol. 71,
Issue. 2,
p.
211.
Włodarczyk, Kazimierz
1991.
On the existence and uniqueness of fixed points for holomorphic maps in complex Banach spaces.
Proceedings of the American Mathematical Society,
Vol. 112,
Issue. 4,
p.
983.
Shafrir, Itai
1992.
Coaccretive operators and firmly nonexpansive mappings in the Hilbert ball.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 18,
Issue. 7,
p.
637.
Kuczumow, Tadeusz
Reich, Simeon
and
Schmidt, Malgorzata
1993.
A fixed point property of 𝑙₁-product spaces.
Proceedings of the American Mathematical Society,
Vol. 119,
Issue. 2,
p.
457.
Kuczumow, Tadeusz
Reich, Simeon
Schmidt, Malgorzata
and
Stachura, Adam
1993.
Strong asymptotic normal structure and fixed points in product spaces.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 21,
Issue. 7,
p.
501.
Kuczumow, Tadeusz
Reich, Simeon
and
Stachura, Adam
1994.
Minimal displacement of points under holomorphic mappings and fixed point properties for unions of convex sets.
Transactions of the American Mathematical Society,
Vol. 343,
Issue. 2,
p.
575.
Reich, Simeon
and
Shoikhet, David
1997.
Results and conjectures in holomorphic fixed point theory.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 30,
Issue. 6,
p.
3529.
Kuczumow, Tadeusz
Reich, Simeon
and
Shoikhet, David
2001.
Handbook of Metric Fixed Point Theory.
p.
437.
Budzyńska, Monika
2003.
Existence of a holomorphic retraction onto a common fixed point set of a family of commuting holomorphic self-mappings of BHn.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 53,
Issue. 1,
p.
139.
Ben Amar, Afif
and
O’Regan, Donal
2022.
Topology and Approximate Fixed Points.
Vol. 71,
Issue. ,
p.
99.