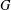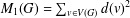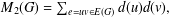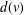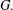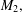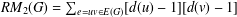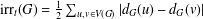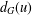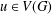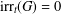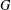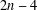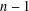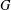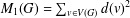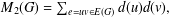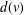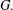The classical first and second Zagreb indices of a graph $G$ are defined as $M_{1}(G)=\sum _{v\in V(G)}d(v)^{2}$ and $M_{2}(G)=\sum _{e=uv\in E(G)}d(u)d(v),$ where $d(v)$ is the degree of the vertex $v$ of $G.$ Recently, Furtula et al. [‘On difference of Zagreb indices’, Discrete Appl. Math.178 (2014), 83–88] studied the difference of $M_{1}$ and 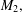$M_{2},$ and showed that this difference is closely related to the vertex-degree-based invariant 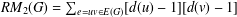$RM_{2}(G)=\sum _{e=uv\in E(G)}[d(u)-1][d(v)-1]$, the reduced second Zagreb index. In this paper, we present sharp bounds for the reduced second Zagreb index, given the matching number, independence number and vertex connectivity, and we also completely determine the extremal graphs.

The total irregularity of a simple undirected graph $G$ is defined as $\text{irr}_{t}(G)=\frac{1}{2}\sum _{u,v\in V(G)}|d_{G}(u)-d_{G}(v)|$, where $d_{G}(u)$ denotes the degree of a vertex $u\in V(G)$. Obviously, $\text{irr}_{t}(G)=0$ if and only if $G$ is regular. Here, we characterise the nonregular graphs with minimal total irregularity and thereby resolve the recent conjecture by Zhu et al. [‘The minimal total irregularity of graphs’, Preprint, 2014, arXiv:1404.0931v1 ] about the lower bound on the minimal total irregularity of nonregular connected graphs. We show that the conjectured lower bound of $2n-4$ is attained only if nonregular connected graphs of even order are considered, while the sharp lower bound of $n-1$ is attained by graphs of odd order. We also characterise the nonregular graphs with the second and the third smallest total irregularity.