1 results
The closure of a local subexponential distribution class under convolution roots, with applications to the compound Poisson process
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 42 / Issue 4 / December 2005
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1194-1203
- Print publication:
- December 2005
-
- Article
-
- You have access
- Export citation
-
Let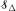
denote the class of local subexponential distributions and F∗ν the ν-fold convolution of distribution F, where ν belongs to one of the following three cases: ν is a random variable taking only a finite number of values, in particular ν ≡ n for some n ≥ 2; ν is a Poisson random variable; or ν is a geometric random variable. Along the lines of Embrechts, Goldie, and Veraverbeke (1979), the following assertion is proved under certain conditions: 
This result is applied to the infinitely divisible laws and some new results are established. The results obtained extend the corresponding findings of Asmussen, Foss, and Korshunov (2003).
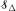denote the class of local subexponential distributions and 
This result is applied to the infinitely divisible laws and some new results are established. The results obtained extend the corresponding findings of Asmussen, Foss, and Korshunov (2003).