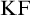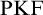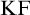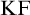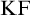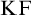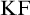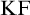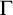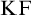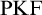1 results
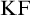 $\mathrm {KF}$
,
$\mathrm {KF}$
,
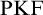 $\mathrm {PKF}$
, AND REINHARDT’S PROGRAM
$\mathrm {PKF}$
, AND REINHARDT’S PROGRAM
- Part of
-
- Journal:
- The Review of Symbolic Logic / Volume 16 / Issue 1 / March 2023
- Published online by Cambridge University Press:
- 08 February 2022, pp. 33-58
- Print publication:
- March 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation