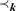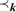We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Theorem. Suppose that k = (K,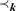 
$$\prec_k$$) is an ℵ0-presentable abstract elementary class with Löwenheim–Skolem number ℵ0, satisfying the joint embedding and amalgamation properties in ℵ0. If K has only countably many models in ℵ1, then all are small. If, in addition, k is almost Galois ω-stable then k is Galois ω-stable. Suppose that k = (K, 
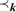$$\prec_k$$) is an ℵ0-presented almost Galois ω-stable AEC satisfying amalgamation for countable models, and having a model of cardinality ℵ1. The assertion that K is ℵ1-categorical is then absolute.
