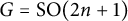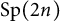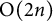Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
  1. Home
  2. Search

Refine search

Refine search

Access:
Content type:
Publication date:
Apply   From and To filters
Subject: Show more
Journals Show more
Publishers: Show more
Societies Show more
Series: Show more
Collections: Show more

Actions for selected content:

Select all | Deselect all
  • View selected items
  • Export citations
  • Download PDF (zip)
  • Save to Kindle
  • Save to Dropbox
  • Save to Google Drive

Save Search

You can save your searches here and later view and run them again in "My saved searches".

Please provide a title, maximum of 40 characters.
Cancel
×

2 results

  • 1 - Topics in representation theory of finite groups

  • Edited by R. A. Bailey, University of St Andrews, Scotland, Peter J. Cameron, University of St Andrews, Scotland, Yaokun Wu, Shanghai Jiao Tong University, China
  • Book:
    Groups and Graphs, Designs and Dynamics
    Published online:
    11 May 2024
    Print publication:
    30 May 2024, pp 1-86

  • Summary

    This is an introduction to representation theory and harmonic analysis on finite groups. This includes, in particular, Gelfand pairs (with applications to diffusion processes à la Diaconis) and induced representations (focusing on the little group method of Mackey and Wigner). We also discuss Laplace operators and spectral theory of finite regular graphs. In the last part, we present the representation theory of GL(2, Fq), the general linear group of invertible 2 × 2 matrices with coefficients in a finite field with q elements. More precisely, we revisit the classical Gelfand–Graev representation of GL(2, Fq) in terms of the so-called multiplicity-free triples and their associated Hecke algebras. The presentation is not fully self-contained: most of the basic and elementary facts are proved in detail, some others are left as exercises, while, for more advanced results with no proof, precise references are provided.

  • The Gelfand–Graev representation of classical groups in terms of Hecke algebras

  • Part of
  • Petar Bakić, Gordan Savin
  • Journal:
    Canadian Journal of Mathematics / Volume 75 / Issue 4 / August 2023
    Published online by Cambridge University Press:
    24 June 2022, pp. 1343-1368
    Print publication:
    August 2023
    • Article
      • You have access
      • Open access
    • PDF
    • HTML
    • Export citation

  • Let G be a p-adic classical group. The representations in a given Bernstein component can be viewed as modules for the corresponding Hecke algebra—the endomorphism algebra of a pro-generator of the given component. Using Heiermann’s construction of these algebras, we describe the Bernstein components of the Gelfand–Graev representation for $G=\mathrm {SO}(2n+1)$, $\mathrm {Sp}(2n)$, and $\mathrm {O}(2n)$.