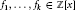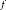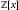1 results
Linear Forms in Monic Integer Polynomials
-
- Journal:
- Canadian Mathematical Bulletin / Volume 56 / Issue 3 / 01 September 2013
- Published online by Cambridge University Press:
- 20 November 2018, pp. 510-519
- Print publication:
- 01 September 2013
-
- Article
-
- You have access
- Export citation
-
We prove a necessary and sufficient condition on the list of nonzero integers
${{u}_{1}},...,{{u}_{k}}$, 
$k\,\ge \,2$, under which a monic polynomial 
$f\,\in \,\mathbb{Z}\left| x \right|$ is expressible by a linear form 
${{u}_{1}}\,{{f}_{1}}\,+\,\cdot \cdot \cdot \,+\,{{u}_{k}}\,{{f}_{k}}$ in monic polynomials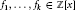 
${{f}_{1}},...,\,{{f}_{k}}\,\in \,\mathbb{Z}\left| x \right|$. This condition is independent of 
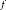$f$. We also show that if this condition holds, then the monic polynomials 
${{f}_{1}},...,\,{{f}_{k}}$ can be chosen to be irreducible in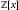 
$\mathbb{Z}\left[ x \right]$.
