1. Introduction
Ternary InxGa1-xN alloys are used as the active layer in GaN-based light emitting diodes (LEDs) and lasers Reference Nakamura and Fasol[1]. It is thus important to understand and control the growth of InGaN. In our earlier work Reference Chen, Smith, Feenstra, Greve and Northrup[2] we studied the dependence of In incorporation on growth parameters for InGaN with (000
The basic InGaN surface structures discussed here are illustrated in Figure 1. Figure 1(a) shows the previously determined structure for the InGaN(000

Figure 1. Basic structure of InGaN surfaces: (a)
2. Experiment
The studies described here were performed in a combined molecular beam epitaxy (MBE)/surface analysis system, as previously described Reference Chen, Smith, Feenstra, Greve and Northrup[2] Reference Chen, Feenstra, Northrup, Zywietz, Neugebauer and Greve[4]. GaN(0001) was grown on Si-face 6H-SiC(0001) substrates, with the polish damage removed by H-etching Reference Ramachandran, Brady, Smith, Feenstra and Greve[5]. After the H-etching, the substrate was introduced into the growth chamber and outgassed up to the temperature of 800°C. A few monolayers of Si were deposited onto the surface and the substrate was then annealed to about 1000°C until a √3×√3 reconstruction was obtained Reference Ramachandran, Smith and Feenstra[6]. GaN was directly grown on this surface at growth temperature of 670°C. Following the GaN growth with typical thickness of 200 nm, the substrate temperature is lowered to 580−620°C for the InGaN deposition. GaN(000
After growth, samples were quenched to room temperature, and transferred under vacuum to the analysis chamber for scanning tunneling microscopy (STM) and Auger spectroscopy study. Auger spectroscopy was measured with a Perkin-Elmer 15-255G system. STM measurements were performed as previously described Reference Chen, Smith, Feenstra, Greve and Northrup[2]. X-ray diffraction (XRD) observations are performed on a Philips Materials Research Diffractometer, with x-ray source using a Bartels monochromator in four crystal mode.
3. Results and Discussion
3.1 Surface Structures
Figure 2 shows an STM image of the InGaN(0001) surface, illustrating two types of surface structures. On the left side of the image there appears a structure with small pits (vacancy islands) and bright double rows, identical to that described in our previous work Reference Chen, Feenstra, Northrup, Zywietz, Neugebauer and Greve[4] Reference Chen, Feenstra, Northrup, Zywietz and Neugebauer[7]. The top atomic layer of this structure consists entirely of In atoms, and partial occupation of In in the second layer also occurs. The vacancy islands and double rows arise as a form of strain relief for the second layer In atoms.
On the right side of Figure 2 is a new structure which has not been previously discussed. This structure is observed on surfaces of InGaN films which have been grown with relatively high In-flux. In this structure we do not observe the vacancy islands or double rows (except for the arrows in Figure 2, discussed below). Rather, the surface is relatively uniform, with 1×1 symmetry, and displays a number of raised features as shown in Figure 3. The raised features are triangular, with their orientation reversing as one moves across a bilayer step of this high In-content structure.

Figure 2. STM image of an InGaN(0001) surface with relatively high In-content, acquired with sample bias voltage of +0.8 V and tunneling current of 0.075 nA. Some local background subtraction has been applied to the image, to permit viewing of the features on both terraces. The grey scale range on a given terrace is 1.1 Å. Sample was grown at 600°C with Ga and In fluxes of 8.3×1013 and 1.3×1014 cm−2s−1 respectively.

Figure 3. STM image of InGaN(0001) surface, from the same film as pictured in Figure 2. Image acquired with sample bias voltage of −0.1 V and tunneling current of 0.075 nA. The grey scale range is 0.35 Å.
An important characteristic of this high In-content structure is its height, measured in the STM constant-current images, relative to the vacancy island type structure. For the terraces in Figure 1 we find a height difference of 3.5 Å, i.e. 0.9 Å larger than an expected bilayer step of 2.6 Å. This height difference is, however, found to be voltage dependent; for relatively low bias voltages such as in Figure 1 we observe a height difference of 3.3–3.5 Å whereas for larger bias voltage of 2–3 V (empty or filled states) we find a height difference of 2.8–2.9 Å. Based on these structural characteristics, we propose that the high In-content structure consists of two adlayers on indium (i.e. one more adlayer than shown in Figure 1(b)), together with sparse occupation of In in the third atomic layer. The top In adlayer would be out of registry with the underlying adlayer (i.e. with atoms in the threefold hollow sites of the underlying layer) such that the triangular shaped features seen in Figure 3 are produced from the third layer In atoms. This structure is supported by theoretical calculations described below.
First principles total energy calculations were performed for an In bilayer structure containing 2 ML of In. We refer to this structure as a T1+T4 indium bilayer since the topmost layer has T4 registry and the second layer has T1 registry relative to their underlying layers, using the notation of Ref. Reference Northrup and Neugebauer[3]. The calculated equilibrium height of the T4 layer is 2.68 Å above the T1 layer, close to that observed experimentally. The slightly larger experimental height is attributed to electronic effects in the tunnel current, indicating that this T1+T4 structure is somewhat more metallic than the vacancy island type structure. In the In-rich limit, where μIn=μIn (bulk), the calculations indicate that the T1+T4 bilayer is lower in energy than the T1 adlayer structure by 0.16 eV/1×1 unit cell. Thus, the surface energies of the two structures are equal when μIn=μIn (bulk) − 0.16 eV. Put another way, the T1+T4 bilayer structure is stable with respect to agglomeration into bulk In droplets residing on the T1 adlayer structure. This result is in agreement with the image shown in Figure 2. This conclusion for the In adlayer energetics is somewhat analogous to a result obtained earlier for Ga adlayers on the GaN(0001) surface. In that case, the laterally contracted Ga bilayer structure was found to be equal in energy to the laterally contracted Ga monolayer structure for μGa=μGa (bulk) − 0.17 eV Reference Northrup, Neugebauer, Feenstra and Smith[8].
A final feature of Figure 2 which we comment upon are the surface depressions indicated by the arrows. The large pit indicated by the black arrow is not so typical of the surface, and we believe it is associated with a defect of some sort. We occasionally see such pits on both the high In-content structure and the vacancy island structure; they have diameter and depth significantly larger than the typical pits which occur on the vacancy island type structure and their origin is unknown. The smaller depressions indicated by white arrows in Figure 2 are seen consistently on the high In-content regions of the surface. Their depth is about 0.6 Å, and their diameter of about 3 nm is comparable to the regular pits which occur on the vacancy island portion of the surface. We speculate that these depressions may arise from an original vacancy island which was not completely filled in during the transition to high In-content structure. In other words, this depression may contain N-vacancies in the fourth atomic layer. While it is likely that these N-vacancies are filled in during a later stage of the growth, it is possible that some part of the associated In compositional variation will remain. This situation is expected to have significant consequences on the optical properties of InGaN Reference Chen, Feenstra, Northrup, Zywietz and Neugebauer[7].
Based on XRD measurements, we find a bulk indium concentration of about 10% for the film pictured in Figure 2 and Figure 3. Since there are two monolayers of In on the surface, it is clear that strong surface segregation of the In occurs. A similar situation occurs for the other InGaN(0001) films we have studied, which contained one monolayer of In on the surface and 0−5% In in the bulk Reference Chen, Feenstra, Northrup, Zywietz, Neugebauer and Greve[4] Reference Chen, Feenstra, Northrup, Zywietz and Neugebauer[7]. The surface segregation arises from the fact that the InN bond is much weaker than the GaN bond (1.93 eV for InN and 2.24 eV for GaN Reference Harrison[9]) so that it is energetically favorable for Ga atoms in the surface layer to exchange with underlying In atoms. Strain considerations also favor the placement of In atoms in the top surface layer Reference Chen, Feenstra, Northrup, Zywietz, Neugebauer and Greve[4].
Indium surface segregation also occurs on InGaN(000

Figure 4. STM image of InGaN(000
We observe relatively few of the type E adsorbates, which, if they arise from indium atoms, is consistent with the theoretical expectation that such In adatoms are only weakly bound to the surface. First principles total energy calculations were performed for structures containing 1/4 ML In adatoms above the 1×1 In adlayer surface. These calculations were performed using a 2×2 unit cell and indicate that the formation energy of such an adatom is 1.6 eV in the In-rich limit, i.e. for the case of μIn=μIn (bulk). In other words, the energy cost to move an In atom from a bulk In reservoir (such as an In droplet) to an adatom site on a terrace is approximately 1.6 eV. Since the cohesive energy of bulk In is 2.5 eV, the binding energy of such an In adatom is therefore quite small, 0.9 eV. Such In adatoms will thus either agglomerate into droplets or evaporate. We therefore expect the In adatom-on-adlayer density to be extremely low, in agreement with the experimental results. This situation is in contrast to the clean GaN(000
3.2 Incorporation Kinetics
Determination of the dependence of indium incorporation on growth parameters is important for controllably growing InGaN with desired indium concentration. It also provides valuable information on growth kinetics, as will be shown below. Similar to what we have done for (000
In this study two growth parameters were varied, the substrate temperature and group III/V ratio. The N2 flux is kept constant to maintain the growth chamber pressure of 1.8×10−5 Torr, and the In/(In+Ga) flux ratio is kept at 33% when In and Ga fluxes are both varied. The results are shown in Figure 5(a). At a given In and Ga flux, when the substrate temperature is increased, the indium incorporation decreases. More interestingly, when temperature is kept constant, and both In and Ga fluxes are increased while keeping their ratio constant, it is found the indium incorporation increases at low metal flux, but decreases at high metal flux. It is well known that GaN growth goes through a smooth/rough transition when Ga/N ratio is unity Reference Tarsa, Heying, Wu, Fini, DenBaars and Speck[12], so by measuring the Ga flux at the transition point, the active N flux can be determined. An active N flux of 2.6×1014 cm−2s−1 is thus found experimentally (with an uncertainty of about 10%). The results of Figure 5(a) are similar to what we observed for (000

Figure 5. (a) Indium incorporation dependence on (In+Ga) flux for (0001) polarity InGaN. The In/(In+Ga) flux ratio was kept constant at 33%. (b) Theoretical curves based on Eqs. (3) and (4) in the text.

Figure 6. (a) Indium incorporation dependence on (In+Ga) flux for
Figure 7 illustrates the near surface region of an InGaN(0001) film, showing a typical distribution of metal atoms (indium and gallium) on the surface and in the bulk. We assume for ease of illustration the situation with a single In adlayer, although the results are the same if we have two In adlayers (i.e. the high In-content structure). Our surface is then terminated with two monolayers of metal atoms (layer 2 and 3 in Figure 7), and during growth a few additional metal atoms (layer 1) may reside on top of this monolayer. The population of metal atoms in layer 1 depends on how metal rich the growth is. For the present case of InGaN growth, the observed surface segregation reveals that layer 2 (and layer 1) contain mainly In atoms.
In general, the In composition x in the alloy is given by a ratio of incorporation rates for In atoms to the total incorporation rate for metal (i.e. In+Ga) atoms. The former we write as f In- R In, where f In is the incident flux of In and R In is the rate of In loss from the film. Loss may occur through either evaporation or droplet formation, and one can further distinguish losses from the different surface layers of the film (i.e. layers 1 or 2 for In, as shown in Figure 7). The total metal incorporation rate is similarly written as f In − R In + f Ga − R Ga where f Ga is the incident flux of Ga atoms and R Ga is the loss rate for Ga. For growth of a stoichiometric film, we have

Figure 7. Schematic view of the InGaN(0001) surface layers: layer 1, indium adatoms residing on top of the indium adlayer; layer 2, indium adlayer; layer 3, metal (In or Ga) atoms; layers 4 and 6, nitrogen atoms; layer 5, metal atoms.
where f N is the incident flux of active N and R N is the loss rate of N atoms. Thus for the indium composition we have in general
Since there is a strong surface segregation of indium atoms, the gallium atom population on the surface is small, under the condition that the growth is not overly metal rich (we consider in this work cases where f Ga < f N). Then, the gallium evaporation will also be small, and most of the incident gallium flux will incorporate into the bulk. We thus take R Ga = 0 for both the metal rich and nitrogen rich situations discussed below.
Let us consider indium incorporation in the metal rich regime. In that case, the maximum amount of In+Ga which can be incorporated into the bulk is limited by the active nitrogen flux. Since the surface is metal rich, we expect minimal loss of N atoms, so that R N = 0. Thus, the rate of metal incorporation is simply equal to the flux of active nitrogen, f N. When both indium and gallium fluxes are increased, those additional gallium atoms will compete to go into bulk. Since there is strong indium surface segregation, those additional gallium atoms will mostly go into the bulk and kick out indium atoms, so that the indium incorporation will decrease. From Eq. (1) we have for the rate of In incorporation f In-R In = f N − f Ga. Therefore, the indium concentration incorporated into bulk is given by
Excess indium atoms, formed from the increased metal flux as well as the decreased bulk incorporation, will tend to increase the concentration of In atoms in layer 1, and so loss from that layer will also increase. The excess indium will evaporate, or alternatively, it will form droplets if there is too much indium. This result is consistent with that of Böttcher et al.'s Reference Boettcher, Einfeldt, Kirchner, Figge, Heinke, Hommel, Selke and Ryder[13], although the latter didn't distinguish the case of metal rich and N rich conditions.
In the nitrogen rich region, the number of metal atoms in layer 1 is minimal. Thus, metal evaporation mainly proceeds via layer 2, for which the loss rate is constant for a given temperature since the number of In atoms in layers 2 is constant (close to 1 monolayer) Footnote [a]
The rate of In incorporation is thus given by f In- R In , and the rate of total metal incorporation is given by f Ga +f In- R In. Therefore, the indium concentration in the bulk can be described by
where R In is treated as a parameter. With the loss of indium being constant, an increase of both indium and gallium flux while keeping their ratio constant will thus lead to an increase in the indium incorporation.
Theoretical curves based on equation 3 and equation 4 are shown in Figure 5(b). Parameters used are: f N = 2.6×1014 cm−2s−1; R In = 0.6×1014 cm−2s−1 for T = 620°C; R In = 0.3×1014 cm−2s−1 for T = 600°C; R In = 0.04×1014 cm−2s−1 for T = 580°C. All these parameters were adjusted to get a close fit to the experimental results. It should be pointed out that the active N flux obtained from the incorporation curve fitting is the same as that determined from smooth/rough transition experiment described above. The deduced variation of R In with growth temperature is consistent with a thermal activation barrier of about 4 eV, close to the result of Averbeck et al. of 3.5–3.8 eV Reference Averback and Riechert[14]. Comparing Figure 5(a) and (b), we find the theoretical curves give a good fit to the experimental results.
The model described here can also be used to fit the (000
4. Conclusion
InGaN (0001) and
ACKNOWLEDGMENTS
This work was supported by the National Science Foundation (grant DMR-9985898), the Office of Naval Research (grant N00014-96-1-0214, monitored by Colin Wood), and the German Science Foundation (focused project "Group-III Nitrides").

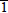 ) polarities are grown by plasma-assisted molecular beam epitaxy. Scanning tunneling microscopy images, interpreted using first-principles theoretical calculations, show that there is strong indium surface segregation on InGaN for both (0001) and (000
) polarities are grown by plasma-assisted molecular beam epitaxy. Scanning tunneling microscopy images, interpreted using first-principles theoretical calculations, show that there is strong indium surface segregation on InGaN for both (0001) and (000




