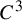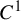Hyperbolicity of renormalization for dissipative gap mappings

Abstract

A gap mapping is a discontinuous interval mapping with two strictly increasing branches that have a gap between their ranges. They are one-dimensional dynamical systems, which arise in the study of certain higher dimensional flows, for example the Lorenz flow and the Cherry flow. In this paper, we prove hyperbolicity of renormalization acting on $C^3$ dissipative gap mappings, and show that the topological conjugacy classes of infinitely renormalizable gap mappings are $C^1$ manifolds.

1 Introduction

Higher dimensional, physically relevant, dynamical systems often possess features that can be studied using techniques from one-dimensional dynamical systems. Indeed, often a one-dimensional discrete dynamical system captures essential features of a higher dimensional flow. For example, for the Lorenz flow [22], one may study the return mapping to a plane transverse to its stable manifold, the stable manifold intersects the plane in a curve, and the return mapping to this curve is a (discontinuous) one-dimensional dynamical system known as a Lorenz mapping, see paper [47]. This approach has been very fruitful in the study of the Lorenz flow. It would be difficult to cite all the papers studying this famous dynamical system, but for example see papers [1, 3, 15, 18, 39, 49]. The success of the use of the one-dimensional Lorenz mapping in studying the flow has led to an extensive study of these interval mappings, see papers [6, 14, 19, 20, 26, 29, 30, 43, 50] among many others. Great progress in understanding the Cherry flow on a two-torus has followed from a similar approach [2, 8, 11, 28, 33–38, 40].

In this paper, we study a class of Lorenz mappings, which have ‘gaps’ in their ranges. These mappings arise as return mappings for the Lorenz flow and for certain Cherry flows. They are also among the first examples of mappings with a wandering interval – the gap. This phenomenon is ruled out for $\mathcal C^{1+\mathrm {Zygmund}}$ mappings with a non-flat critical point by van Strien and Vargas [48]. In fact, Berry and Mestel [5] proved that Lorenz mappings satisfying a certain bounded nonlinearity condition have a wandering interval if and only if they have a renormalization which is a gap mapping. See the introduction of paper [17] for a detailed history of gap mappings.

The main result of this paper concerns the structure of the topological conjugacy classes of $\mathcal C^4$ dissipative gap mappings. Roughly, these are discontinuous mappings with two orientation preserving branches, whose derivatives are bounded between zero and one. They are defined in Definition 2.1.

Theorem 1.1. The topological conjugacy class of an infinitely renormalizable $\mathcal C^4$ dissipative gap mapping is a $\mathcal C^1$ -manifold of codimension-one in the space of dissipative gap maps.

To obtain this result, we prove the hyperbolicity of renormalization for dissipative gap mappings. In the usual approach to renormalization, one considers renormalization as a restriction of a high iterate of a mapping. While this is conceptually straightforward, it is technically challenging as the composition operator acting on the space of, say, $\mathcal {C}^4$ functions is not differentiable. Nevertheless, we are able to show that the tangent space admits a hyperbolic splitting. To do this, we work in the decomposition space introduced by Martens in [25], see §3 for the necessary background.

Theorem 1.2. The renormalization operator ${\mathcal R}$ acting on the space of dissipative gap mappings has a hyperbolic splitting. More precisely, if f is an infinitely renormalizable ${\mathcal C}^3$ dissipative gap mapping then for any $\delta \in (0,1),$ and for all n sufficiently big, the derivative of the renormalization operator acting on the decomposition space $\underline {\mathcal {D}}$ satisfies the following.

• • $T_{\underline {\mathcal {R}}_{\underline {\mathcal {R}}^n\underline f}} \underline {\mathcal {D}}=E^u\oplus E^s,$ and the subspace $E^u$ is one-dimensional.

• • For any vector $v\in E^u$ , we have that $\|D\underline {\mathcal {R}}_{\underline {\mathcal {R}}^n\underline f}v\|\geq \unicode{x3bb} _1\|v\|$ , where $|\unicode{x3bb} _1|>1/\delta $ .

• • For any $v\in E^s$ , we have that $\|D\underline {\mathcal {R}}_{\underline {\mathcal {R}}^n\underline f}v\|\leq \unicode{x3bb} \|v\|$ , where $|\unicode{x3bb} |<\delta $ .

Gap mappings can be regarded as discontinuous circle mappings, and indeed they have a well-defined rotation number [7], and they are infinitely renormalizable precisely when the rotation number is irrational. Consequently, from a combinatorial point of view they are similar to critical circle mappings. However, unlike critical circle mappings, the geometry of gap mappings is unbounded. For example, for critical circle mappings the quotient of the lengths of successive renormalization intervals is bounded away from zero and infinity [9], but for gap mappings it diverges very fast [17]. As a result, the renormalization operator for gap mappings does not seem to possess a natural extension to the limits of renormalization (cf. [27]).

Renormalization theory was introduced into dynamical systems from statistical physics by Feigenbaum [13], and Tresser and Coullet [45, 46] in the 1970s to explain the universality phenomena they observed in the quadratic family. They conjectured that the period-doubling renormalization operator acting on an appropriate space of analytic unimodal mappings is hyperbolic. The first proof of this conjecture was obtained using computer assistance by Lanford [21]. The conjecture can be extended to all combinatorial types and to multimodal mappings. A conceptual proof was given for analytic unimodal mappings of any combinatorial type in the works of Sullivan [44] (see also [12]), McMullen [31, 32], Lyubich [23, 24], and Avila and Lyubich [4]. This was extended to certain smooth mappings by de Faria, de Melo and Pinto [10], and to analytic mappings with several critical points and bounded combinatorics by Smania [41, 42]. Renormalization is intimately related with rigidity theory, and in many contexts, e.g. interval mappings and critical circle mappings, exponential convergence of renormalization implies that two topologically conjugate infinitely renormalizable mappings are smoothly conjugate on their (measure-theoretic) attractors. However, for gap mappings, it is not the case that exponential convergence of renormalization implies rigidity; indeed, in general, one can not expect topologically conjugate gap mappings to be $\mathcal C^1$ conjugate [17].

The aforementioned results on renormalization of interval mappings all depend on complex analytic tools and, consequently, many of the tools developed in these works can only be applied to mappings with a critical point of integer order. The goal of studying mappings with arbitrary critical order was one of Martens’ motivations for introducing the decomposition space, mentioned above. This purely real approach has led to results on the renormalization in various contexts. Martens [25] used this approach to establish the existence of periodic points of renormalization of any combinatorial type for unimodal mappings $x\mapsto x^\alpha +c$ , where $\alpha>1$ is not necessarily an integer. For Lorenz mappings of certain monotone combinatorial types, Martens and Winckler [29] proved that there exists a global two-dimensional strong unstable manifold at every point in the limit set of renormalization using this approach. Martens and Palmisano [27] studied renormalization acting on the decomposition space for infinitely renormalizable critical circle mappings with a flat interval. They proved that for certain mappings with stationary, Fibonacci, combinatorics that the renormalization operator is hyperbolic, and that the class of mappings with Fibonacci combinatorics is a $\mathcal C^1$ manifold.

Analytic gap mappings were studied by Gouveia and Colli [16, 17] using different methods to those that we use here. In the former paper, they proved hyperbolicity of renormalization in the special case of affine dissipative gap mappings, and in the latter paper, they proved that the topological conjugacy classes of analytic infinitely renormalizable dissipative gap mappings are analytic manifolds. We appropriately generalize these two results to the $\mathcal C^4$ case. Since the renormalization operator does not extend to the limits of renormalization, it seems to be difficult to build on the hyperbolicity result for affine mappings to extend it to smooth mappings (similar to what was done in paper [10]), and so we follow a different approach. Gouveia and Colli [17] also proved that two topologically conjugate dissipative gap mappings are Hölder conjugate. We improve this rigidity result, and give a simple proof that topologically conjugate dissipative gap mappings are quasisymmetrically conjugate, see Proposition 2.8.

This paper is organized as follows: in §2, we will provide the necessary background material on gap mappings, and in §3, we will describe the decomposition space of infinitely renormalizable gap mappings. The estimate of the derivative of renormalization operator is done in §4, and it is the key technical result of our work. In our setting, we are able to obtain fairly complete results without any restrictions on the combinatorics of the mappings. In §5, we use the estimates of §4 and ideas from paper [27] to show that the renormalization operator is hyperbolic and that the conjugacy classes of dissipative gap mappings are $\mathcal C^1$ manifolds.

2 Preliminaries

2.1 The dynamics of gap maps

In this section, we collect the necessary background material on gap mappings, see paper [17] for further results.

A Lorenz map is a function $f:[a_L, a_R] \setminus \{ 0 \} \rightarrow [a_L, a_R]$ satisfying:

1. (i) $a_L< 0 < a_R$ ;

2. (ii) f is continuous and strictly increasing in the intervals $[a_L,0)$ and $(0,a_R]$ ;

3. (iii) the left and right limits at $0$ are $f(0^-)=a_R$ and $f(0^+)=a_L$ .

A gap map is a Lorenz map f that is not surjective, that is, a map satisfying conditions (i), (ii), (iii) with $f(a_L)> f(a_R)$ . In this case the gap is the interval $G_f=(f(a_R), f(a_L))$ . When it will not cause confusion, we omit the subscript and denote the gap by G.

Definition 2.1. A dissipative gap map is a gap map f that is differentiable in $[a_L, a_R] \setminus \{ 0 \}$ and satisfies: $0 < f'(x) \leq \nu $ for every $x \in [a_L, a_R] \setminus \{ 0 \}$ , and for some real number $\nu = \nu _f \in (0,1)$ .

Each dissipative gap mapping is determined by a mapping to the left of the discontinuity, a mapping to the right of the discontinuity and the relative position of the discontinuity in the interval. Hence it is convenient to describe the space of dissipative gap mappings as follows: Consider

(2.1) $$ \begin{align} \mathcal{D}_L^k &= \{ u_L:[-1,0) \rightarrow \mathbb{R}; \; u_L\in\mathrm{Diff}_+^k[-1,0], u_L(0^-)=0, \nonumber\\ &\,\quad \; \text{and} \; \text{there exists}\ \nu \in (0,1) \; \text{such that} \; 0 < u_L'(x) \leq \nu, \; \text{for all}\ \ x \in [-1,0) \}, \end{align} $$

(2.2) $$ \begin{align} \mathcal{D}_R^k &= \{ u_R:(0, +1] \rightarrow \mathbb{R}; \; u_R \in\mathrm{Diff}_+^k[0,1], u_R(0^+)=0, \nonumber\\ &\,\quad \; \text{and} \; \text{there exists}\ \nu \in (0,1) \; \text{such that} \; 0 < u_R'(x) \leq \nu, \; \text{for all}\ \ x \in [0,1) \}, \end{align} $$

and $\mathcal {D}^k = \mathcal {D}_L^k \times \mathcal {D}_R^k \times (0,1)$ , where $\mathrm {Diff}_+^k[x,y]$ denotes the space of orientation preserving $\mathcal C^k$ diffeomorphisms on $(x,y)$ , which are continuous on $[x,y]$ . We will always assume that $k\geq 3,$ and unless otherwise stated, the reader can assume that $k=3.$

For each element $(u_L, u_R, b) \in \mathcal {D}^k$ , we associate a function $f:[-1,1] \setminus \{ 0 \} \rightarrow [-1,1]$ defined by

(2.3) $$ \begin{align} f(x) = \left\{ \begin{array}{@{}ll} u_L(x)+b, & x \in [-1,0), \\[3pt] u_R(x)+b-1, & x \in (0,+1], \end{array}\right. \end{align} $$

and take $\nu = \nu _f \in (0,1)$ that bounds the derivative on each branch from above. It is not difficult to check that the interval $[b-1,b]$ is invariant under f, and f restricted to $[b-1, b] \setminus \{ 0 \}$ is a dissipative gap map. Observe that the parameter b determines the position of the discontinuity in the interval. For the sake of simplicity, we write $f=(u_L, u_R, b)$ , and we use the following notation for the left and right branches of f:

(2.4) $$ \begin{align} \begin{array}{@{}ll} f_L(x) = u_L(x)+b, & x <0,\\ f_R(x) = u_R(x)+b-1, & x>0, \end{array} \end{align} $$

We endow $\mathcal {D}^k = \mathcal {D}_L^k \times \mathcal {D}_R^k \times (0,1)$ with the product topology. It is important to note that a gap map g defined in an interval $[a_L, a_R]$ can be rescaled by a linear conjugacy in such a way as to be defined in $[b-1,b]$ . After rescaling and extending g, we obtain a function f defined in $[-1,1] \setminus \{0\}$ which is a triple $f=(f_L, f_R, b)$ in $\mathcal D^k.$ Since $[b-1,b]$ is a trapping region for f, it will be enough to work with the restriction of f to $[b-1,b] \setminus \{0\}$ and it is not important how f is extended. Thus we set $a_L = b-1$ and $a_R=b.$ For more details, see §1.2 of paper [17].

Definition 2.2. Let $f:[b-1, b] \setminus \{0 \} \rightarrow [b-1, b]$ be a dissipative gap map. We define the sign of f by

(2.5) $$ \begin{align} \sigma_f:= \left\{ \begin{array}{@{}ll} - & \text{ if } b \leq 1/2,\\ + & \text{ if } b> 1/2. \end{array} \right. \end{align} $$

It is an easy consequence of this definition that for a dissipative gap map f, we have $\sigma _f=-$ if $G \subset [b-1,0)$ and $\sigma _f=+$ when $G \subset (0,b]$ .

2.2 Renormalization of dissipative gap mappings

Definition 2.3. A dissipative gap map $f:[b-1, b] \setminus \{0 \} \rightarrow [b-1, b]$ is renormalizable if there exists a positive integer k such that:

1. (a) $0 \notin \bigcup _{i=0}^k \overline {f^i(G)}$ ;

2. (b) either

   • – $\overline {G}, \overline {f(G)}, \ldots , \overline {f^{k-1}(G)} \subset (b-1,0)$ and $\overline {f^k(G)} \subset (0,b)$ or

   • – $\overline {G}, \overline {f(G)}, \ldots , \overline {f^{k-1}(G)} \subset (0,b)$ and $\overline {f^k(G)} \subset (b-1,0).$

Remark 2.4. The positive number k in Definition 2.3 is chosen to be minimal so that (a) and (b) hold.

By [17, Proposition 2.8], and the mean value theorem, the renormalization of a dissipative gap map is again a dissipative gap map.

Definition 2.5. Let $f:[b-1, b] \setminus \{0 \} \rightarrow [b-1, b]$ be a renormalizable dissipative gap map, and consider $I'= [a_L', a_R' ]=I_f'$ the interval containing $0$ whose boundary points are the boundary points of $f^{k-1}(G)$ and $f^k(G)$ which are nearest to $0$ , that is

(2.6) $$ \begin{align} \begin{array}{@{}cl} I'= [f^k(b-1), f^{k+1}(b)] & \text{ for } \sigma_f = -,\\ I'= [f^{k+1}(b-1), f^k(b)] & \text{ for } \sigma_f=+. \end{array} \end{align} $$

The first return map $R=R_f$ to $I'$ is given by

(2.7) $$ \begin{align} R(x)= \left\{ \begin{array}{@{}ll} f^{k+2}(x) & \text{ if } x \in [f^k(b-1),0),\\ f^{k+1}(x) & \text{ if } x \in (0,f^{k+1}(b)], \end{array}\right. \end{align} $$

in the case where $\sigma _f=-$ , and

(2.8) $$ \begin{align} R(x)= \left\{ \begin{array}{@{}ll} f^{k+1}(x) & \text{ if } x \in [f^{k+1}(b-1),0),\\ f^{k+2}(x) & \text{ if } x \in (0,f^{k}(b)], \end{array}\right. \end{align} $$

in the case where $\sigma _f=+$ . The renormalization of f, $\mathcal {R}f$ , is the first return map R rescaled and normalized to the interval $[-1,1]$ and given by

(2.9) $$ \begin{align} \mathcal{R}f(x)= \frac{1}{|I'|}R(|I'|x) \end{align} $$

for every $x \in [-1,1] \setminus \{0 \}$ .

In terms of the branches $f_L$ and $f_R$ defined in (2.4), the first return map R is given by

(2.10) $$ \begin{align} R(x)= \left\{ \begin{array}{@{}ll} f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (x) & \text{ if } x \in [f^k(b-1),0),\\ [4pt] f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (x) & \text{ if } x \in (0,f^{k+1}(b)], \end{array}\right. \end{align} $$

in the case where $\sigma _f=-$ , and

(2.11) $$ \begin{align} R(x)= \left\{ \begin{array}{@{}ll} f_R^k {{\kern0.5pt}\circ{\kern0.5pt}} f_L (x) & \text{ if } x \in [f^{k+1}(b-1),0),\\ [4pt] f_R^k {{\kern0.5pt}\circ{\kern0.5pt}} f_L {{\kern0.5pt}\circ{\kern0.5pt}} f_R (x) & \text{ if } x \in (0,f^{k}(b)], \end{array}\right. \end{align} $$

in the case where $ \sigma _f=+ $ .

From Definition 2.5, we have a natural operator which sends a renormalizable dissipative gap map f to its renormalization $\mathcal {R}f$ , which is also a dissipative gap map.

Definition 2.6. The renormalization operator is defined by

(2.12) $$ \begin{align} \begin{array}{l@{}lll} \mathcal{R} \! :\! &\mathcal{D}_{\mathcal{R}}^k & \rightarrow & \mathcal{D}^k \\ & f & \mapsto & \mathcal{R}f \end{array} \end{align} $$

where $\mathcal {R}f(x)= ({1}/{|I'|})R(|I'|x)$ , and $\mathcal {D}_{\mathcal {R}}^k \subset \mathcal {D}^k$ is the subset of all renormalizable dissipative gap maps in $\mathcal {D}^k$ .

Although a dissipative gap map is not defined at $0$ , we define the lateral orbits of $0$ taking $0_j^+ = f^j(0^+)= \lim _{x \rightarrow 0^+}f^j(x)$ and $0_j^- = f^j(0^-)= \lim _{x \rightarrow 0^-}f^j(x)$ . We first observe that $0_j^+=f^{j-1}(b-1)$ and $0_j^-=f^{j-1}(b)$ . The left and right future orbits of $0$ are the sequences $(0_j^+)_{j \geq 1}$ and $(0_j^-)_{j \geq 1}$ , which are always defined unless there exists $j \geq 1$ such that either $0_j^+ = 0$ or $0_j^-=0$ . Using this notation for the interval $I'$ defined in (2.6), we obtain

(2.13) $$ \begin{align} I'=\left\{ \begin{array}{@{}ll} [0_{k+1}^+,0_{k+2}^-] = [f_L^k (b-1),f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b)] &\text{ for } \sigma_f = -, \\ [4pt] [0_{k+2}^+,0_{k+1}^-] = [f_R^k {{\kern0.5pt}\circ{\kern0.5pt}} f_L(b-1),f_R^k (b)] &\text{ for } \sigma_f=+.\end{array}\right. \end{align} $$

See Figure 1 for an illustration of one example of a case with $\sigma _f=-$ .

Figure 1 $I'$ : the domain of the first return map R in the case where $\sigma =-$ .

One can show inductively that for each gap mapping f there are $n=n(f) \in \{ 0, 1, 2, \ldots \} \cup \{ \infty \} $ and a sequence of nested intervals $(I_i)_{0 \leq i < n+1}$ , each one containing $0$ , such that:

1. (1) the first return map $R_i$ to $I_i$ is a dissipative gap map, for every $0 \leq i <n+1$ ;

2. (2) $I_{i+1} = I_{R_i}'$ , for every $0 \leq i <n$ .

If $n< \infty $ , we say that f is finitely renormalizable and n-times renormalizable, and if $n = \infty $ , we say that f is infinitely renormalizable. Moreover, we call $G_i = G_{R_i}$ , $\sigma _i = \sigma _{R_i}$ , and $k_i = k_{R_i}$ , for every $0 \leq i < n+1$ . In particular, this defines the combinatorics $\Gamma = \Gamma (f)$ for f, given by the (finite or infinite) sequence

(2.14) $$ \begin{align} \Gamma = ((\sigma_i, k_i))_{1 \leq i < n+1}. \end{align} $$

Proposition 2.7. [17]

Two infinitely renormalizable dissipative gap mappings that have the same combinatorics are topologically conjugate.

For more details about this inductive definition and related properties, see paper [17].

2.3 Quasisymmetric rigidity

We know that two dissipative gap mappings with the same irrational rotation number are Hölder conjugate [17, Theorem A]; however, more is true. Let $\kappa \geq 1$ and let I denote an interval in $\mathbb R$ . Recall that a mapping $h:I\to I$ is $\kappa $ -quasisymmetric if for any $x\in I$ and $a>0$ so that $x-a$ and $x+a$ are in I, we have

$$ \begin{align*}\frac{1}{\kappa}\leq\frac{|h(x+a)-h(x)|}{|h(x)-h(x-a)|}\leq \kappa.\end{align*} $$

Proposition 2.8. Suppose that $f,g$ are two dissipative gap maps with the same irrational rotation number, then f and g are quasisymmetrically conjugate.

Proof Let $\phi ,\psi $ denote $f^{-1},g^{-1}$ , respectively. Then $\phi $ and $\psi $ can be extended to expanding, degree-three, covering maps of the circle, which we will continue to denote by $\phi $ and $\psi $ . These extended mappings are topologically conjugate, and so they are quasisymmetrically conjugate. To see this, one may argue exactly as described in II.2, Exercise 2.3 of paper [12]. There exists a quasisymmetric mapping h of the circle so that $h{{\kern0.5pt}\circ{\kern0.5pt}} \phi (z)=\psi {{\kern0.5pt}\circ{\kern0.5pt}} h(z)$ . Thus we have that $h^{-1}{{\kern0.5pt}\circ{\kern0.5pt}} g=f{{\kern0.5pt}\circ{\kern0.5pt}} h^{-1},$ and it is well known that the inverse of a quasisymmetric mapping is quasisymmetric.

2.4 Convergence of renormalization to affine maps

It is convenient for us to introduce the following.

Definition 2.9. The nonlinearity operator $N:\mathrm {Diff}_+^k([0,1]) \rightarrow \mathcal {C}^{k-2}([0,1])$ is defined by

(2.15) $$ \begin{align} N \varphi := D \log D \varphi = \frac{D^2 \varphi}{D \varphi}, \end{align} $$

and $N \varphi $ is called the nonlinearity of $\varphi $ .

Proposition 2.10. Suppose that f is an infinitely renormalizable dissipative gap mapping. Then for any $\varepsilon>0$ , there exists $n_0\in \mathbb N$ so that for all $n\geq n_0,$ there exists an affine gap mapping $g_n$ so that $\|R^n f-g_n\|_{\mathcal C^3}\leq \varepsilon .$

Proof Let us recall the formulas for the nonlinearity, N, and Schwarzian derivative, S, of iterates of f:

(2.16) $$ \begin{align} Nf^k(x)=\sum_{i=0}^{k-1}Nf(f^i(x))|Df^i(x)|, \end{align} $$

and

$$ \begin{align*}Sf^k(x) = \sum_{i=0}^{k-1}Sf^i(x)|Df^i(x)|^2.\end{align*} $$

Since the derivative of f is bounded away from one, these quantities are bounded in terms of $Nf$ and $Sf$ , respectively. But now, since $|Nf|$ is bounded, say by $C_1>0$ , we have that there exists $C_2>0$ so that

$$ \begin{align*}|Nf^k|=\bigg|\frac{D^2f^k}{Df^k}\bigg|<C_2.\end{align*} $$

Since $Df^k\rightarrow 0,$ as k tends to $\infty $ , so does $D^2f^k$ .

Now,

$$ \begin{align*}Sf^k=\frac{D^3f^k}{Df^k}-\frac{3}{2}(Nf^k)^2,\end{align*} $$

and arguing in the same way, we have that $D^3f^k\to 0$ as $k\to \infty .$ Thus by taking k large enough, $f^k$ is arbitrarily close to its affine part in the $\mathcal C^3$ -topology.

3 Renormalization of decomposed mappings

In this section, we recall some background material on the nonlinearity operator and decomposition spaces; for further details see paper [25, 29]. We then define the decomposition space of dissipative gap mappings, and describe the action of renormalization on this space.

3.1 The nonlinearity operator

In Definition 2.9, we introduced the nonlinearity operator. Let us explore some of its properties.

Remark 3.1. For convenience, we use the abbreviated notation

$$ \begin{align*} N \varphi = \eta_{\varphi}. \end{align*} $$

Lemma 3.2. The nonlinearity operator is a bijection.

Proof The operator N has an explicit inverse given by

$$ \begin{align*} N^{-1}f(x) = \frac{\int_0^x e^{\int_0^s f(t)dt}ds}{\int_0^1 e^{\int_0^s f(t)dt}ds}, \end{align*} $$

where $f \in \mathcal {C}^0([0,1])$ .

By Lemma 3.2, we can identify $\text {Diff}_+^{3}([0,1])$ with $\mathcal {C}^{1}([0,1])$ using the nonlinearity operator. It will be convenient to work with the norm induced on $\text {Diff}_+^3([0,1])$ by this identification. For $\varphi \in \mathrm {Diff}_+^3([0,1])$ , we define

$$ \begin{align*} \|\varphi\| = \|N\varphi\|_{\mathcal{C}^1} = \|\eta_{\varphi}\|_{\mathcal{C}^1}. \end{align*} $$

We say that a set T is a time set if it is at most countable and totally ordered. Given a time set T, let X denote the space of decomposed diffeomorphisms labeled by T:

$$ \begin{align*} X = \bigg\{ \underline{\varphi} = ( \varphi_n )_{n \in T}; \; \varphi_n \in \text{Diff}_{+}^{3}([0, 1]) \text{ and } \sum_{n\in T} \|\varphi_n\| < \infty \bigg\}. \end{align*} $$

The norm of an element $\underline {\varphi } \in X $ is defined by

$$ \begin{align*} \|\underline{\varphi}\| = \displaystyle \sum_{n\in T} \|\varphi_n\|. \end{align*} $$

We define the direct sum of time sets and decompositions as follows. Given two time sets $T_1$ and $T_2$ , we define

$$ \begin{align*}T_2\oplus T_1=\{(x,i):x\in T_i,i=1,2\},\end{align*} $$

where $(x,i)<(y,i)$ if and only if $x<y,$ and $(x,2)>(y,1)$ for all $x\in T_2, \ y\in T_1.$ The sum of two decompositions $\underline {\varphi }_1\oplus \underline {\varphi }_2,$ where $\underline {\varphi }_i\in \mathcal D_{T_i}, \ i=1,2$ , is the composition of the diffeomorphisms of $\underline {\varphi }_1,$ in the order of $T_1$ , followed by the diffeomorphisms of $\underline {\varphi }_2,$ in the order of $T_2$ , see paper [29] for further details.

To simplify the following discussion, assume that $T=\{1,2,3,\ldots ,n\}$ or $T=\mathbb N$ . We define the partial composition by

(3.1) $$ \begin{align} \begin{array}{l@{}lll} O_n :\; & X & \rightarrow & \text{Diff}_{+}^{2}([0, 1]) \\ \,& \underline{\varphi} & \mapsto & O_n \underline{\varphi} := \varphi_n {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_{n-1} {{\kern0.5pt}\circ{\kern0.5pt}} \cdots {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_1 \\ \end{array} \end{align} $$

and the complete composition is given by the limit

(3.2) $$ \begin{align} \displaystyle O \underline{\varphi} = \lim_{n \rightarrow \infty} O_n \underline{\varphi} \end{align} $$

which allow us to define the operator

(3.3) $$ \begin{align} \begin{array}{l@{}lll} O :\; & X & \rightarrow & \text{Diff}_{+}^{2}([0, 1]) \\ \,& \underline{\varphi} & \mapsto & O \underline{\varphi}:= \displaystyle \lim_{n \rightarrow \infty } O_n \underline{\varphi}. \\ \end{array} \end{align} $$

Since the space of decompositions is a Banach space [29, Proposition 7.5], to prove that the limit in (3.2) exists, it is enough to prove that $\{ O_n \underline {\varphi } \}_{n}$ is a Cauchy sequence. This follows from the Sandwich Lemma from paper [25], and (2.16).

3.2 The decomposition space for dissipative gap mappings

It will be convenient to introduce a different set of coordinates on the space of gap mappings. Let $I=[a, b] \subset [0, 1]$ and let $1_I:[0,1] \rightarrow [a,b]$ be the affine map

$$ \begin{align*} 1_I(x)=|I|x+a = (b-a)x+a \end{align*} $$

which has the inverse $1_I^{-1}:[a, b] \rightarrow [0,1]$ given by

$$ \begin{align*} 1_I^{-1}(x)= \frac{x-a}{|I|} = \frac{x-a}{b-a}. \end{align*} $$

We denote by $\Sigma $ the unit cube

$$ \begin{align*} \Sigma = (0, 1)^3 = \{ (\alpha, \beta, b ) \in \mathbb{R}^3 \; | \; 0 < \alpha, \beta, b < 1 \}, \end{align*} $$

by $\text {Diff}_+^3([0,1])^2$ the set

$$ \begin{align*} \{ (\varphi_L, \varphi_R) \, | \, \varphi_L, \varphi_R : [0, 1] \!\rightarrow\! [0, 1] \, \text{are orientation preserving } \mathcal{C}^3- \text{diffeomorphisms}\} \end{align*} $$

and by

$$ \begin{align*} \mathcal D' = \Sigma \times \text{Diff}_+^3([0, 1])^2. \end{align*} $$

We define a change of coordinates from $\mathcal D'$ to $\mathcal {D}$ by

(3.4) $$ \begin{align} \begin{array}{l@{}ccl} \Theta :\,\, & \mathcal D' & \rightarrow & \mathcal{D} \\ & (\alpha, \beta, b, \varphi_L, \varphi_R )& \mapsto & \Theta( \alpha, \beta, b, \varphi_L, \varphi_R )=\colon f \\ \end{array} \end{align} $$

where $f:[b-1, b] \setminus \{0\} \rightarrow [b-1,b]$ is defined by

(3.5) $$ \begin{align} f(x) = \left\{ \begin{array}{@{}ll} f_L(x), & x \in [b-1,0), \\ f_R(x), & x \in (0,b], \end{array} \right. \end{align} $$

with

(3.6) $$ \begin{align} \begin{array}{l@{}cll} f_L :\,\, & I_{0,L}=[b-1,0] & \rightarrow & T_{0,L}=[\alpha (b-1)+b, b] \\ \,& x & \mapsto & f_L(x) = 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1}(x) \\ \end{array} \end{align} $$

and

(3.7) $$ \begin{align} \begin{array}{l@{}cll} f_R :\,\, & I_{0,R}=[0, b] & \rightarrow & T_{0,R}=[b-1, \beta b + b-1] \\ \,& x & \mapsto & f_R(x) = 1_{T_{0,R}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}(x). \\ \end{array} \end{align} $$

Note that $f_L$ and $f_R$ are differentiable and strictly increasing functions such that $0 < f_L '(x) \leq \nu < 1$ , for all $x\in [b-1,0]$ , and $0 < f_R '(x) \leq \nu < 1$ , for all $x\in [0, b]$ , where $\nu $ is a positive real number and less than $1$ depending on f, that is, $\nu = \nu _f \in (0,1)$ . The functions $\varphi _L$ and $\varphi _R$ are called the diffeomorphic parts of f. See Figure 2.

Figure 2 Branches $f_L$ and $f_R$ , slopes $\alpha $ and $\beta $ of a gap map f.

Remark 3.3. Depending on the properties of a gap mapping that we wish to emphasize, we can express a gap mapping f in either coordinate system: $f=(f_L, f_R, b)$ or $f=(\alpha ,\beta , b,\varphi _L,\varphi _R),$ and we will move freely between the two coordinate systems.

We define the decomposition space of dissipative gap maps, $\underline {\mathcal {D}}$ , by

$$ \begin{align*} \underline{\mathcal{D}} = (0,1)^3 \times X \times X. \end{align*} $$

The composition operator defined in (3.3) provides a way to project the space $\underline {\mathcal {D}}$ to the space $(0,1)^3 \times \text {Diff}_{+}^{2}([0, 1]) \times \text {Diff}_{+}^{2}([0, 1])$ . More precisely

(3.8) $$ \begin{align} \begin{array}{@{}ccccl} \Xi &\!\!\! : & \underline{\mathcal{D}} & \rightarrow & (0,1)^3 \times \text{Diff}_{+}^{2}([0, 1]) \times \text{Diff}_{+}^{2}([0, 1]) \\ & & (\alpha, \beta, b, \underline{\varphi}_L, \underline{\varphi}_R) & \mapsto & \Xi (\alpha, \beta, b, \underline{\varphi}_L, \underline{\varphi}_R) : = (\alpha, \beta, b, O \underline{\varphi}_L, O \underline{\varphi}_R). \\ \end{array} \end{align} $$

3.3 Renormalization on $\underline {\mathcal {D}}$

It is known that the zoom operator $\varsigma _I : \mathcal {C}^1([0,1]) \rightarrow \mathcal {C}^1([0,1])$ is defined by

(3.9) $$ \begin{align} \varsigma_I \varphi (x) = 1_{\varphi (I)}^{-1} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I}(x). \end{align} $$

Observe that the nonlinearity operator satisfies

$$ \begin{align*} N(\varsigma_I \varphi) = |I| \cdot N \varphi {{\kern0.5pt}\circ{\kern0.5pt}} 1_I. \end{align*} $$

Thus, we define the zoom operator $Z_I: \mathcal {C}^1([0,1]) \rightarrow \mathcal {C}^1([0,1])$ acting on a nonlinearity by

(3.10) $$ \begin{align} Z_I \eta (x) = |I| \cdot \eta {{\kern0.5pt}\circ{\kern0.5pt}} 1_I(x), \end{align} $$

and if $\varphi $ is a $\mathcal C^2$ diffeomorphism, we define $Z_I\varphi $ by

$$ \begin{align*} \begin{array}{l@{}cll} Z_I :\,\, & \text{Diff}_{+}^{r}([0, 1]) & \rightarrow & \mathcal{C}^{r-2}([0,1]) \\ \,\,& \varphi & \mapsto & Z_I \varphi (x) = |I| \cdot \eta_{\varphi} {{\kern0.5pt}\circ{\kern0.5pt}} 1_I(x) \\ \end{array} \end{align*} $$

where $\eta _{\varphi }=N\varphi .$

Let $\mathcal D_0$ denote the set of once renormalizable gap mappings. If $f=(\alpha ,\beta ,b,\varphi _L,\varphi _R)\in \mathcal D_0$ , we let $\tilde f=\mathcal R f=(\tilde \alpha ,\tilde \beta , \tilde b,\tilde \varphi _L,\tilde \varphi _R)$ denote its renormalization. When $\sigma _f=-$ , we have the following expressions for the coordinates of $\tilde f$ :

(3.11) $$ \begin{align} \begin{aligned} \tilde{\alpha} & = \displaystyle \frac{f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+})-0_{k+2}^{-}}{0_{k+1}^{+}} , \\ \tilde{\beta} & = \displaystyle \frac{f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R(0_{k+2}^-)-0_{k+1}^+}{0_{k+2}^-}, \\ \tilde{b} & = \displaystyle \frac{0_{k+2}^-}{|[0_{k+1}^+,0_{k+2}^-]|},\\ \tilde{\varphi}_L & = \displaystyle \varsigma_{[0_{k+1}^+,0]} \tilde{f}_L \quad \text{with } \tilde{f}_L=f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L, \\ \tilde{\varphi}_R & = \displaystyle \varsigma_{[0, 0_{k+2}^-]} \tilde{f}_R \quad \text{with } \tilde{f}_R=f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R. \end{aligned} \end{align} $$

We have similar expressions when $\sigma _f=+,$ which we omit.

To express $\tilde {\underline {f}}\in \underline {\mathcal {D}},$ we write $\tilde {\underline {f}}=(\tilde \alpha ,\tilde \beta , \tilde b,\tilde {\underline {\varphi }}_L,\tilde {\underline {\varphi }}_R)$ , where $\tilde \alpha ,\tilde \beta $ and $\tilde b$ are as in (3.11), and $ \tilde {\underline {\varphi }}_L$ and $\tilde {\underline {\varphi }}_R,$ are defined by

$$ \begin{align*}\displaystyle \tilde{\underline{\varphi}}_L= \varsigma_{U_{k+2}}\underline f_L \oplus \varsigma_{U_{k+1}}\underline f_L \oplus\cdots \varsigma_{U_{2}}\underline f_L\oplus \varsigma_{U_{1}}\underline f_R\oplus\varsigma_{U_{0}}\underline f_L \quad \text{and} \end{align*} $$

$$ \begin{align*}\displaystyle \tilde{\underline{\varphi}}_R= \varsigma_{V_{k+1}}\underline f_L \oplus \varsigma_{V_{k}}\underline f_L \oplus\cdots \varsigma_{V_{1}}\underline f_L\oplus \varsigma_{V_{0}}\underline f_R, \end{align*} $$

where $\underline {f}_L$ and $\underline {f}_R$ are decompositions over a singleton time set (a decomposition associated to a single iterate of a mapping), $U_0=(0^+_{k+1},0),\ U_i=f^i(U_0)$ for $0<i\leq k+2$ , $V_0=(0,0^-_{k+2}),$ and $V_i=f^i(V_0)$ for $0<i\leq k+1.$

Let us comment briefly on this definition. The mappings $\tilde \varphi _L$ and $\tilde \varphi _R$ are the compositions of f corresponding to the left and right branches of the renormalization $\tilde f$ , pre-composed and post-composed with affine mappings, so that they are expressed as mappings from the unit interval onto itself. To define $\tilde {\underline {\varphi }}_L,$ we take the direct sum of terms of the form $\varsigma _{U_i}\underline f_L.$ Each of these terms is the restriction of (a single iterate of) f to $U_i$ , the ith interval in the orbit of either $(0,b)$ or $(b-1,0)$ , depending on whether $\sigma _f = -$ or $+$ , respectively, pre-composed and post-composed by affine mappings, so that it is a mapping from the unit interval onto itself. The direct sum of mappings in the decomposition space corresponds to composition of mappings, so one immediately sees that after composing the decomposed mappings we obtain $\tilde f.$

As we will use the structure of Banach space in $\text {Diff}_{+}^{3}([0, 1])$ given by the nonlinearity operator, we need the expressions for the coordinates functions $\tilde {\varphi }_L$ and $\tilde {\varphi }_R$ in terms of the zoom operator. Note that the coordinates $\tilde {\alpha }$ , $\tilde {\beta }$ , and $\tilde {b}$ remain the same as in (3.11) since they are not affected by the zoom operator. In order to obtain these coordinate functions, we need to apply the zoom operator to each branch of the first return map R on the interval $I'= [0_{k+1}^{+}, 0_{k+2}^{-}]$ , in the case where $\sigma _f=-$ , or on the interval $I'= [0_{k+2}^{+}, 0_{k+1}^{-}]$ , in the case where $\sigma _f=+$ . Then, when $\sigma _f=-$ , we obtain

(3.12) $$ \begin{align} \tilde{\eta}_L & = Z_{[0_{k+1}^{+}, 0]} \eta_{\tilde{f}_L} = |0_{k+1}^{+}| \cdot \eta_{\tilde{f}_L} {{\kern0.5pt}\circ{\kern0.5pt}} 1_{[0_{k+1}^{+}, 0]}^{-1} = |0_{k+1}^{+}| \cdot N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) {{\kern0.5pt}\circ{\kern0.5pt}} 1_{[0_{k+1}^{+}, 0]}^{-1},\nonumber\\ \tilde{\eta}_R & = Z_{[0, 0_{k+2}^{-}]} \eta_{\tilde{f}_R} = |0_{k+2}^{-}| \cdot \eta_{\tilde{f}_R} {{\kern0.5pt}\circ{\kern0.5pt}} 1_{[0, 0_{k+2}^{-}]}^{-1} = |0_{k+2}^{-}| \cdot N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R) {{\kern0.5pt}\circ{\kern0.5pt}} 1_{[0, 0_{k+2}^{-}]}^{-1}. \end{align} $$

The formulas when $\sigma =+$ are similar, and to save space we do not include them.

Remark 3.4. We would like to stress that throughout the remainder of this paper, we will make use of the Banach space structure on $\text {Diff}_{+}^{3}([0, 1])$ given by its identification with $\mathcal C^1([0,1])$ via the nonlinearity operator.

4 The derivative of the renormalization operator

In this section, we will estimate the derivative of the renormalization operator acting on an absorbing set under renormalization in the decomposition space of dissipative gap mappings. A little care is needed since the operator is not differentiable.

Recall that $\mathcal D_0\subset \mathcal C^3$ is the set of once renormalizable dissipative gap mappings. Then $\mathcal R:\mathcal D_0\to \mathcal C^2$ is differentiable, and the derivative $D\mathcal R_f:\mathcal C^3 \to \mathcal C^2$ extends to a bounded operator $D\mathcal R_f:\mathcal C^2\to \mathcal C^2,$ which depends continuously on $f\in \mathcal C^3.$ In paper [27], $\mathcal R$ is called jump-out differentiable.

If $\underline {f}=(\alpha , \beta , b, \underline {\varphi }_L, \underline {\varphi }_R) \in \underline {\mathcal D}_0$ , the derivative of $\underline {\mathcal {R}}_{\underline {f}}$ , $D\underline {\mathcal {R}}_{\underline {f}}$ , is a matrix of the form

(4.1) $$ \begin{align} D \underline{\mathcal{R}}_{\underline{f}} = \left( \begin{array}{@{}cc@{}} A_{\underline{f}} & B_{\underline{f}} \\ C_{\underline{f}} & D_{\underline{f}} \end{array} \right) \end{align} $$

where

• • $A_{\underline {f}}: \mathbb {R}^3 \rightarrow \mathbb {R}^3$ ,

• • $B_{\underline {f}}: X \times X \rightarrow \mathbb {R}^3$ ,

• • $C_{\underline {f}}: \mathbb {R}^3 \rightarrow X \times X$ ,

• • $D_{\underline {f}}: X \times X \rightarrow X \times X.$

We estimate $A_{\underline {f}}$ in Lemma 4.6, $B_{\underline {f}}$ in Lemma 4.8, $C_{\underline {f}}$ in Lemma 4.9, and $D_{\underline {f}}$ in Lemma 4.14.

In order to estimate the entries of matrices $A_{\underline {f}}$ , $B_{\underline {f}}$ , $C_{\underline {f}}$ , and $D_{\underline {f}}$ , we will make use of the partial derivative operator $\partial $ . The main properties of $\partial $ are presented in the next lemma.

Lemma 4.1. [29, Lemma 9.4]

The following equations hold whenever they make sense:

(4.2) $$ \begin{align} \partial (f {{\kern0.5pt}\circ{\kern0.5pt}} g)(x) = \partial f(g(x))+f'(g(x)) \partial g(x) , \end{align} $$

(4.3) $$ \begin{align} \partial (f^{n+1})(x) = \sum_{i=0}^{n}Df^{n-i}(f^{i+1}(x)) \partial f(f^{i}(x)), \end{align} $$

(4.4) $$ \begin{align} \partial (f^{-1})(x) = - \frac{\partial f(f^{-1}(x))}{f'(f^{-1}(x))} , \end{align} $$

(4.5) $$ \begin{align} \partial (f \cdot g)(x) = \partial f(x)g(x) +f(x)\partial g(x) , \end{align} $$

(4.6) $$ \begin{align} \partial (f/g)(x) = \frac{\partial f(x)g(x)-f(x) \partial g(x)}{(g(x))^2}. \end{align} $$

From now on, we will make use of the notation

$$ \begin{align*} g(x) \asymp y \end{align*} $$

to mean that there exists a positive constant $K < \infty $ not depending on g such that $K^{-1}y \leq g(x) \leq K y$ , for all x in the domain of g.

Recall that the inverse of the nonlinearity operator $N: \text {Diff}_+^3([0,1]) \rightarrow \mathcal {C}^1([0,1])$ is given by

(4.7) $$ \begin{align} \varphi (x) = \varphi_{\eta}(x)=N^{-1} \eta (x) = \frac{\int_0^x e^{\int_0^s \eta (t)dt}ds}{\int_0^1 e^{\int_0^s \eta (t)dt}ds}, \end{align} $$

where $\eta \in \mathcal {C}^1([0,1]).$

Lemma 4.2. Let $x \in [0, 1]$ . The evaluation operator $E: \mathrm{Diff}_{+}^2([0,1]) = \mathcal {C}^0([0,1]) \rightarrow \mathbb {R}$

$$ \begin{align*} E: \eta \mapsto \varphi_{\eta}(x) \end{align*} $$

is differentiable with derivative $\displaystyle {\partial \varphi (x)}/{\partial \eta }: \mathcal {C}^0([0,1]) \rightarrow \mathbb {R}$ given by

(4.8) $$ \begin{align} \displaystyle \frac{\partial \varphi (x)}{\partial \eta}(\Delta \eta ) = \bigg( \displaystyle \frac{\int_{0}^{x} \big[ \int_{0}^{s} \Delta \eta \big] e^{\int_{0}^{s} \eta }ds}{ \int_{0}^{x}e^{\int_{0}^{s}\eta} ds} - \displaystyle \frac{\int_{0}^{1} \big[ \int_{0}^{s} \Delta \eta \big] e^{\int_{0}^{s} \eta }ds}{ \int_{0}^{1}e^{\int_{0}^{s}\eta} ds}\bigg) \varphi (x). \end{align} $$

There exists $\varepsilon _0>0$ so that for all $\varepsilon \in (0,\varepsilon _0),$ if $\| D^2\varphi \|_{\mathcal {C}^0} < \varepsilon ,$ we have that

(4.9) $$ \begin{align} \frac{1}{8}\min\{ \varphi (x), 1-\varphi (x)\}\leq \bigg| \displaystyle \frac{\partial \varphi (x)}{\partial \eta} \bigg| \leq 2 \min\{ \varphi (x), 1-\varphi (x)\}. \end{align} $$

Proof In order to prove that the evaluation operator E is (Fréchet) differentiable and obtain (4.8), we just need to use the Gateaux variation to look for a candidate T for its derivative, that is,

(4.10) $$ \begin{align} \begin{array}{@{}r@{}c@{}l} \displaystyle T(\eta) \Delta \eta &\, =\, & \displaystyle \frac{d}{dt}E(\eta + t \Delta \eta ) |_{t=0}. \\ & & \\ \end{array} \end{align} $$

Since this calculation is not difficult, we have left it to the reader. Now we will prove (4.9). Using techniques of integration, we obtain

(4.11) $$ \begin{align} \displaystyle \int_{0}^{x} \bigg[ \int_{0}^{s} \Delta \eta \bigg] e^{\int_{0}^{s} \eta }ds = \displaystyle \bigg( \int_{0}^{x} \Delta \eta \bigg) \cdot \int_{0}^{x} e^{\int_{0}^{t} \eta }ds - \int_{0}^{x} \bigg[ \Delta \eta \cdot \int_{0}^{s} e^{\int_{0}^{t} \eta } \bigg] ds. \end{align} $$

From (4.11), (4.8), and (4.7), and after some manipulations, we obtain

(4.12) $$ \begin{align} \bigg| \displaystyle \frac{\partial \varphi (x)}{\partial \eta}(\Delta \eta ) \bigg| = \varphi (x) \cdot \int_{x}^{1} \Delta \eta\, ds - \varphi (x) \cdot \int_{0}^{1} \Delta \eta \cdot \varphi(s)\, ds + \int_{0}^{x} \Delta \eta \cdot \varphi(s)\, ds. \end{align} $$

From the definition of the norm

$$ \begin{align*} \bigg| \displaystyle \frac{\partial \varphi (x)}{\partial \eta}(\Delta \eta ) \bigg| = \sup_{\|\Delta \eta\| = 1} \bigg| \displaystyle \frac{\partial \varphi (x)}{\partial \eta} \bigg|, \end{align*} $$

we can substitute $\Delta \eta = 1$ into (4.12) and obtain

$$ \begin{align*} \bigg| \displaystyle \frac{\partial \varphi (x)}{\partial \eta}(\Delta \eta ) \bigg| = \varphi (x) \cdot (1-x) - \varphi (x) \cdot \int_{0}^{1} \varphi(s)\, ds + \int_{0}^{x} \varphi(s)\, ds. \end{align*} $$

Using the fact that for deep renormalizations, the map $\varphi $ is close to identity, that is, $\|\varphi (x) - x\|_{\mathcal C^0}$ is small, we get

(4.13) $$ \begin{align} \bigg| \displaystyle \frac{\partial \varphi (x)}{\partial \eta}(\Delta \eta ) \bigg| & \asymp x \cdot (1-x) - x \cdot \int_{0}^{1} s\, ds + \int_{0}^{x} s\, ds \nonumber\\ & = \displaystyle \frac{x}{2} \cdot (1-x). \end{align} $$

Since

$$ \begin{align*} \displaystyle T_{{1}/{4}}(x) \leq \frac{x}{2}(1-x) \leq T_{2}(x) \end{align*} $$

for all $x \in [0, 1]$ , where $T_c(x)$ is the tent map family $T_c:[0,1]\to [0,1]$ , defined by

$$ \begin{align*}T_c(x)=\left\{ \begin{array}{@{}ll} cx & \text{ for } x\in[0,1/2],\\ -cx+c & \text{ for } x\in(1/2,1]. \end{array}\right. \end{align*} $$

The result follows.

Corollary 4.3. [27, Corollary 8.17]

Let $\psi ^+, \psi ^- \in \mathrm{Diff}_{+}^2([0, 1])$ and $x \in [0, 1]$ . The evaluation operator

(4.14) $$ \begin{align} \begin{array}{l@{}lll} E^{\psi_+, \psi^-} :\,\, & \mathrm{Diff}_{+}^2([0, 1]) = \mathcal{C}^0([0,1]) & \rightarrow & \mathbb{R} \\ \,\,& \eta & \mapsto & E^{\psi^+, \psi^-} (\eta) = \psi^+ {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_{\eta} {{\kern0.5pt}\circ{\kern0.5pt}} \psi^- (x) \\ \end{array} \end{align} $$

is differentiable with derivative $\displaystyle {\partial ( \psi ^+ {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _{\eta } {{\kern0.5pt}\circ{\kern0.5pt}} \psi ^- (x) ) }/{\partial \eta } : \mathcal {C}^0([0,1]) \rightarrow \mathbb {R}$ given by

(4.15) $$ \begin{align} \displaystyle \frac{\partial ( \psi^+ {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_{\eta} {{\kern0.5pt}\circ{\kern0.5pt}} \psi^- (x) ) }{\partial \eta } ( \Delta \eta ) = D \psi^+ (\varphi_{\eta} {{\kern0.5pt}\circ{\kern0.5pt}} \psi^- (x)) \cdot \frac{\partial \varphi_{\eta} (\psi^-(x))}{\partial \eta } ( \Delta \eta ). \end{align} $$

The next result follows from a straightforward calculation, and its proof is left to the reader.

Lemma 4.4. The branches $f_L$ and $f_R$ of f defined in (3.5) are differentiable and their partial derivatives are given by

(4.16) $$ \begin{align} \begin{aligned}[b] \displaystyle \hspace{5pt}\frac{\partial f_L}{\partial \alpha }(x) & = (1-b) \cdot \displaystyle \bigg[ \varphi_L \bigg( \frac{x-b+1}{1-b} \bigg) - 1 \bigg], \quad \displaystyle \frac{\partial f_L}{\partial \beta }(x) =0, \\ \displaystyle \frac{\partial f_L}{\partial b }(x) & = 1+ \alpha \cdot \displaystyle \bigg[1- \varphi_L \bigg( \frac{x-b+1}{1-b} \bigg) \bigg] + \frac{\alpha x}{1-b} D \varphi_L \bigg( \frac{x-b+1}{1-b} \bigg), \\ \displaystyle \frac{\partial f_L}{\partial \eta_L }(x) & = \displaystyle |T_{0, L}| \cdot \frac{\partial \varphi_L (1_{I_{0, L}}^{-1}(x))}{\partial \eta_L}, \quad \displaystyle \frac{\partial f_L}{\partial \eta_R }(x) = 0, \end{aligned}\\ \begin{aligned}[b] \displaystyle &\kern-105pt\frac{\partial f_R}{\partial \alpha }(x) = 0, \quad \displaystyle \frac{\partial f_R}{\partial \beta }(x) = b \varphi_R \bigg( \frac{x}{b} \bigg),\\ \displaystyle &\kern-105pt\frac{\partial f_R}{\partial b }(x) = 1+ \beta \cdot \displaystyle \varphi_R \bigg( \frac{x}{b} \bigg) - \frac{\beta x}{b} D \varphi_R \bigg( \frac{x}{b} \bigg),\\ \displaystyle &\kern-105pt\frac{\partial f_R}{\partial \eta_L }(x) = 0, \quad \displaystyle \frac{\partial f_R}{\partial \eta_R }(x) = \displaystyle |T_{0, R}| \cdot \frac{\partial \varphi_R (1_{I_{0, R}}^{-1}(x))}{\partial \eta_R}. \end{aligned} \end{align} $$

Furthermore, all these partial derivatives are bounded.

Let $f=(f_L, f_R, b) \in \mathcal {D}$ be a renormalizable dissipative gap map. The boundaries of the the interval $I'=[0_{k+1}^{+}, 0_{k+2}^{-}]$ for $\sigma _f=-$ , and $I'=[0_{k+2}^{+}, 0_{k+1}^{-}]$ for $\sigma _f=+$ , can be interpreted as evaluation operators, that is,

(4.17) $$ \begin{align} \begin{array}{l@{}lll} E :\,\, & M & \rightarrow & \mathbb{R} \\ \,\,& (\alpha, \beta, b, \varphi_L, \varphi_R)& \mapsto & 0_{j}^{\pm} \end{array} \end{align} $$

where $j \in \{ k+1, k+2 \}$ depending on the sign of f. For convenience, we will call $0_{j}^{\pm }$ as boundary operators. The next result gives us some properties about the boundary operators.

Lemma 4.5. The boundary operators $0_{j}^{\pm }$ are differentiable and the partial derivatives $\displaystyle {\partial 0_{j}^{\pm }}/{\partial *}$ are bounded, where $* \in \{ \alpha , \beta , b, \eta _L, \eta _R \}$ and $j \in \{ k+1, k+2 \}$ , depending on the sign of f.

Proof Consider the boundary operators $0_{k+2}^{-}$ and $0_{k+1}^{+}$ , which are explicitly given by

$$ \begin{align*} 0_{k+1}^{+} = f_L^k(b-1) \quad \text{and} \quad 0_{k+2}^{-} = f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b), \end{align*} $$

when $\sigma _f=-$ , and where $f_L = 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1}$ and $f_R = 1_{T_{0,R}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}$ . Using (4.3) and taking $* \in \{ \alpha , \beta , b, \eta _L, \eta _R \}$ we get

(4.18) $$ \begin{align} \begin{array}{@{}r@{}c@{}l@{}} \displaystyle \frac{\partial }{\partial *} ( 0_{k+1}^{+} ) &\, = \displaystyle \frac{\partial}{\partial *} ( f_L^k (b-1) ) =& \displaystyle \sum_{i=0}^{k-1}Df_L^{k-1-i}(f_L^{i+1} (b-1)) \cdot \frac{\partial f_L}{\partial *} (f_L^i (b-1)), \end{array} \end{align} $$

and

(4.19) $$ \begin{align} \displaystyle \frac{\partial }{\partial *} ( 0_{k+2}^{-} ) = \displaystyle \frac{\partial}{\partial *} ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b) ) &= \displaystyle \sum_{i=0}^{k-1}Df_L^{k-1-i}(f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b)) \cdot \frac{\partial f_L}{\partial *} (f_L^i {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b))\nonumber\\ &\quad \displaystyle +\, Df_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b) \cdot \frac{\partial f_R}{\partial *}(b). \end{align} $$

Using the fact that $0 < f'(x) \leq \nu < 1$ , for all $x \in [b-1,b] \setminus \{ 0 \}$ , and Lemma 4.4, we get that $\displaystyle {\partial }/{\partial *} ( 0_{k+2}^{-} )$ and $\displaystyle {\partial }/{\partial *} ( 0_{k+1}^{+} )$ are bounded. With similar arguments and reasoning, we prove that the other boundary operators have bounded partial derivatives.

4.1 The $A_{\underline {f}}$ matrix

(4.20) $$ \begin{align} A_{\underline{f}} = \left( \begin{array}{@{}ccc@{}} \displaystyle \frac{\partial \tilde{\alpha}}{\partial \alpha } & \displaystyle \frac{\partial \tilde{\alpha}}{\partial \beta } & \displaystyle \frac{\partial \tilde{\alpha}}{\partial b } \\ & & \\ \displaystyle \frac{\partial \tilde{\beta}}{\partial \alpha } & \displaystyle \frac{\partial \tilde{\beta}}{\partial \beta } & \displaystyle \frac{\partial \tilde{\beta}}{\partial b } \\ & & \\ \displaystyle \frac{\partial \tilde{b}}{\partial \alpha } & \displaystyle \frac{\partial \tilde{b}}{\partial \beta } & \displaystyle \frac{\partial \tilde{b}}{\partial b } \\ \end{array} \right). \end{align} $$

All the entries of matrix $A_{\underline {f}}$ can be calculated explicitly by using Lemma 4.1. In order to clarify the calculations, we will compute some of them in the next lemma.

Lemma 4.6. Let $ \underline {f}=(\alpha ,\beta ,b,\underline {\varphi }_R, \underline {\varphi }_L) \in \underline {\mathcal D}_0$ . The map

(4.21) $$ \begin{align} \begin{array}{@{}r@{}c@{}l} (0, 1)^3 \ni (\alpha, \beta, b) &\, \mapsto\, & (\tilde{\alpha}, \tilde{\beta}, \tilde{b}) \in (0, 1)^3\\ \end{array} \end{align} $$

is differentiable. Furthermore, for any $\varepsilon>0, K>0$ if $\underline g\in \underline {\mathcal {D}}_0$ is infinitely renormalizable, there exists $n_0\in \mathbb N,$ so that if $n\geq n_0$ and $\underline f=\underline {\mathcal {R}}^n \underline g,$ then the partial derivatives $\displaystyle |({\partial }/{\partial \alpha }) \tilde {\alpha }|$ , $\displaystyle | ({\partial }/{\partial \beta }) \tilde {\alpha }|$ , $\displaystyle | ({\partial }/{\partial b }) \tilde {\alpha }|$ , $\displaystyle | ({\partial }/{\partial \alpha }) \tilde {\beta }|$ , $\displaystyle |({\partial }/{\partial \beta }) \tilde {\beta }|$ , and $\displaystyle |({\partial }/{\partial b }) \tilde {\beta }|$ are all bounded from above by $\varepsilon $ , and the partial derivatives $\displaystyle |({\partial }/{\partial \alpha }) \tilde {b}|$ , $\displaystyle | ({\partial }/{\partial \beta }) \tilde {b}|$ and $\displaystyle | ({\partial }/{\partial b }) \tilde {b}|$ are bounded from below by K. In particular, $\displaystyle | ({\partial }/{\partial b }) \tilde {b}|\asymp {1}/{|I'|}.$ (See §3.3 for the definition of $I'$ .)

Proof We will prove this lemma in the case where $\sigma _f=-$ . The case $\sigma _f=+$ is similar and we will leave it to the reader. From (3.11) we obtain the partial derivatives

(4.22) $$ \begin{align} \begin{array}[c]{@{}r@{\,}c@{\,}l} \displaystyle \frac{\partial }{\partial *} \tilde{\alpha } &\; =\; & \displaystyle \frac{1}{(0_{k+1}^{+})^2} \cdot \bigg\{ 0_{k+1}^{+} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) ) - 0_{k+1}^{+} \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+2}^{-} ) \\ && -\; \displaystyle [ f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - 0_{k+2}^{-} ] \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+1}^{+} ) \bigg\}, \\ \displaystyle \frac{\partial }{\partial *} \tilde{\beta } &\; =\; & \displaystyle \frac{1}{(0_{k+2}^{-})^2} \cdot \bigg\{ 0_{k+2}^{-} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (0_{k+2}^{-}) ) - 0_{k+2}^{-} \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+1}^{+} ),\\ && -\; \displaystyle [ f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (0_{k+2}^{-}) - 0_{k+1}^{+} ] \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+2}^{-} ) \bigg\} \\ \displaystyle \frac{\partial }{\partial *} \tilde{b} & \;=\; & (1-\tilde{b}) \cdot |I'|^{-1} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b) ) + |I'|^{-1} \cdot \tilde{b} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k (b-1) ),\\ \end{array} \end{align} $$

where $* \in \{ \alpha , \beta , b \}$ . Let us start to deal with the first line of $A_{\underline {f}}$ , that is, with the partial derivatives

$$ \begin{align*} \displaystyle \frac{\partial \tilde{\alpha}}{\partial *} \end{align*} $$

where $* \in \{ \alpha , \beta , b\}$ . Taking $* = \alpha $ , we obtain

(4.23) $$ \begin{align} \begin{array}[b]{@{}r@{}c@{}l} \displaystyle \frac{\partial }{\partial \alpha} \tilde{\alpha } &\,=\, & \displaystyle \frac{1}{(0_{k+1}^{+})^2} \cdot \bigg\{ 0_{k+1}^{+} \cdot \displaystyle \frac{\partial }{\partial \alpha} ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) ) - 0_{k+1}^{+} \cdot \displaystyle \frac{\partial }{\partial \alpha} ( 0_{k+2}^{-} ) \\ && -\; \displaystyle [ f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - 0_{k+2}^{-} ] \cdot \displaystyle \frac{\partial }{\partial \alpha} ( 0_{k+1}^{+} ) \bigg\}. \end{array} \end{align} $$

From (4.2) and using the fact that $f_R$ does not depend on $\alpha $ , we have

(4.24) $$ \begin{align} \displaystyle \frac{\partial }{\partial \alpha} ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) ) &\, =\, \displaystyle \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+})\nonumber \\ &\quad \ +\, D ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R ) {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+}) \cdot \displaystyle \frac{\partial }{\partial \alpha} ( f_L(0_{k+1}^{+}) ) \end{align} $$

Since $0_{k+1}^{+}=f_L^k(b-1)$ , we can apply (4.3) and get

(4.25) $$ \begin{align} \displaystyle \frac{\partial }{\partial \alpha} ( f_L(0_{k+1}^{+}) )\! =\! \displaystyle \frac{\partial }{\partial \alpha} ( f_L^{k+1}(b-1) )\! =\! \displaystyle \sum_{i=0}^{k} Df_L^{k-i}(f_L^{i+1}(b-1)) \cdot \displaystyle \frac{\partial f_L}{\partial \alpha} (f_L^i(b-1)). \end{align} $$

Since $0_{k+2}^{-} = f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b)$ by applying the mean value theorem to the difference $f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - 0_{k+2}^{-} $ , we obtain a point $\xi \in (f_L(0_{k+1}^{+}),b)$ such that

(4.26) $$ \begin{align} \displaystyle f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - 0_{k+2}^{-} &= f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+})-f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b)\nonumber\\ &= D ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R )(\xi) \cdot [ f_L(0_{k+1}^{+})- b ]. \end{align} $$

Since $b=f_L(0^-)$ , by applying the mean value theorem once more, we obtain another point $\zeta \in (0_{k+1}^{+}, 0)$ such that

(4.27) $$ \begin{align} f_L(0_{k+1}^{+})- b = f_L(0_{k+1}^{+})- f_L(0^-) = D f_L (\zeta ) \cdot 0_{k+1}^{+}. \end{align} $$

Substituting (4.27), (4.26), and (4.24) into (4.23), and after some manipulations, we get

(4.28) $$ \begin{align} \displaystyle \frac{\partial }{\partial \alpha} \tilde{\alpha } & = \displaystyle \frac{1}{(0_{k+1}^{+})} \cdot \bigg\{ \displaystyle \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - \displaystyle \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b) \nonumber\\ & \quad + D(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R) {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+}) \cdot \displaystyle \frac{\partial }{\partial \alpha} ( f_L(0_{k+1}^{+}) ) \nonumber\\ & \quad - \displaystyle [ D ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R )(\xi) \cdot D f_L (\zeta ) ] \cdot \displaystyle \frac{\partial }{\partial \alpha} ( 0_{k+1}^{+} ) \bigg\}. \end{align} $$

By (4.3), we obtain

(4.29) $$ \begin{align} &\displaystyle \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b) \nonumber\\ &\quad= \displaystyle \sum_{i=0}^{k-1}Df_L^{k-1-i} ( f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+})) \cdot \displaystyle \frac{\partial f_L}{\partial \alpha}( f_L^i {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+})) \nonumber\\ &\quad\quad- \displaystyle \sum_{i=0}^{k-1}Df_L^{k-1-i} ( f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b)) \cdot \displaystyle \frac{\partial f_L}{\partial \alpha} ( f_L^i {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b)). \end{align} $$

From Lemma 4.4, we know that $\displaystyle ({\partial f_L}/{\partial \alpha })(x)$ is bounded, then putting

$$ \begin{align*} C_1 = \max_{0 \leq i < k } \bigg\{ \displaystyle \bigg| \frac{\partial f_L}{\partial \alpha }(f_L^i {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+})) \bigg|, \bigg| \frac{\partial f_L}{\partial \alpha }(f_L^i {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b)) \bigg| \bigg\}, \end{align*} $$

we obtain

(4.30) $$ \begin{align} &\displaystyle \bigg| \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b) \bigg| \nonumber\\ &\quad\leq C_1 \cdot \displaystyle \sum_{i=0}^{k-1} \bigg| Df_L^{k-1-i} ( f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+})) - Df_L^{k-1-i} ( f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b)) \bigg|. \end{align} $$

Applying the mean value theorem twice, we obtain a point $\xi _i \in (f_{L}^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+}), f_{L}^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b))$ , and a point $\theta _i \in (f_L(0_{k+1}^{+}), b)$ such that

(4.31) $$ \begin{align} &| Df_L^{k-1-i} ( f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+})) - Df_L^{k-1-i} ( f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b)) | \notag \\ &\quad= | D^2 f_L^{k-1-i}(\xi_i)| \cdot | D(f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R) (\theta_i)| \cdot | Df_L(\zeta)| \cdot |0_{k+1}^{+}|. \end{align} $$

From this we obtain

(4.32) $$ \begin{align} &\displaystyle \bigg| \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L (0_{k+1}^{+}) - \frac{\partial }{\partial \alpha} ( f_L^k ) {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b) \bigg|\notag \\ &\quad\leq C_1 \cdot |0_{k+1}^{+}| \cdot \displaystyle \sum_{i=0}^{k-1} | D^2 f_L^{k-1-i}(\xi_i)| \cdot | D(f_L^{i+1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R) (\theta_i)| \cdot | Df_L(\zeta)|\notag \\ &\quad= C_1 \cdot |0_{k+1}^{+}| \cdot | Df_L(\zeta)| \cdot \displaystyle \sum_{i=0}^{k-1} | D^2 f_L^{k-1-i}(\xi_i)| \notag\\ &\quad\quad\cdot\; | Df_L^{i} {{\kern0.5pt}\circ{\kern0.5pt}} f_L {{\kern0.5pt}\circ{\kern0.5pt}} f_R (\theta_i)| \cdot | Df_L {{\kern0.5pt}\circ{\kern0.5pt}} f_R (\theta_i) | \cdot | Df_R(\theta_i) |. \end{align} $$

For the other difference in (4.28), we start by observing that $\displaystyle ({\partial }/{\partial \alpha }) ( f_L(0_{k+1}^{+}))$ and $\displaystyle ({\partial }/{\partial \alpha }) ( 0_{k+1}^{+} )$ are either simultaneously positive or negative. Furthermore, from Lemma 4.5, we have that $\displaystyle ({\partial }/{\partial \alpha }) ( 0_{k+1}^{+} )$ is bounded, and arguing similarly, we have that $\displaystyle {\partial }/{\partial \alpha } ( f_L(0_{k+1}^{+}))$ is also bounded. Thus, there exists a constant $\displaystyle C_2>0$ such that

(4.33) $$ \begin{align} &\bigg| \displaystyle D(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R) {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+}) \cdot \frac{\partial }{\partial \alpha} ( f_L(0_{k+1}^{+})) - \displaystyle [ D ( f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R )(\xi) \cdot D f_L (\zeta ) ] \cdot \displaystyle \frac{\partial }{\partial \alpha} ( 0_{k+1}^{+} ) \bigg| \notag\\ &\quad\leq C_2 \cdot | D(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R) {{\kern0.5pt}\circ{\kern0.5pt}} f_L(0_{k+1}^{+}) - D(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R) (\xi) | \notag\\ &\quad\leq C_2 \cdot | D^2(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R)(w)| \cdot | Df_L (\zeta) | \cdot |0_{k+1}^{+}| \end{align} $$

where $w \in (f_L(0_{k+1}^{+}), \xi )$ is a point given by the mean value theorem.

Substituting (4.32) and (4.33) into (4.28), we obtain

(4.34) $$ \begin{align} \displaystyle \bigg| \frac{\partial }{\partial \alpha} \tilde{\alpha}\bigg| & \leq C_1 \cdot | Df_L(\zeta)| \cdot \displaystyle \sum_{i=0}^{k-1} | D^2 f_L^{k-1-i}(\xi_i)| \cdot | Df_L^{i} {{\kern0.5pt}\circ{\kern0.5pt}} f_L {{\kern0.5pt}\circ{\kern0.5pt}} f_R (\theta_i)| \notag\\ & \quad\cdot\; | Df_L {{\kern0.5pt}\circ{\kern0.5pt}} f_R (\theta_i) | \cdot | Df_R(\theta_i) | + C_2 \cdot | D^2(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R)(w)| \cdot | Df_L (\zeta) |. \end{align} $$

Since the first and second derivatives of f go to zero when the level of renormalization goes to infinity, we conclude that $ \displaystyle | ({\partial }/{\partial \alpha }) \tilde {\alpha } | \longrightarrow 0$ when the level of renormalization goes to infinity. With same arguments and reasoning, we can prove that $\displaystyle | ({\partial }/{\partial \beta }) \tilde {\alpha } |$ , $\displaystyle | ({\partial }/{\partial b}) \tilde {\alpha } |$ , $\displaystyle | ({\partial }/{\partial \alpha }) \tilde {\beta } |$ , $\displaystyle | ({\partial }/{\partial \beta }) \tilde {\beta } |$ , and $\displaystyle | ({\partial }/{\partial b}) \tilde {\beta } |$ all tend to zero as the level of renormalization tends to infinity.

Now we will prove that $\displaystyle | {\partial \tilde {b} }/{\partial b} |$ is big. From (4.22), we have

(4.35) $$ \begin{align} \displaystyle \bigg| \frac{\partial \tilde{b}}{\partial b} \bigg| & = \displaystyle \frac{1}{|I'|^2} \cdot \bigg\{ 0_{k+2}^{-} \cdot \frac{\partial }{\partial b} ( 0_{k+1}^{+} ) - 0_{k+1}^{+} \cdot \frac{\partial }{\partial b} ( 0_{k+2}^{-} ) \bigg\} \notag\\ & \geq \displaystyle \frac{1}{|I'|} \cdot \text{min} \bigg\{ \frac{\partial }{\partial b} ( 0_{k+1}^{+} ), \frac{\partial }{\partial b} ( 0_{k+2}^{-} ) \bigg\} \notag \\ & \geq \displaystyle \frac{1}{|I'|} \cdot \text{min} \bigg\{ \frac{\partial f_L}{\partial b} ( f_L^{k-1}(b-1) ), \frac{\partial f_L}{\partial b} ( f_L^{k-1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b) ) \bigg\} \end{align} $$

which is big since the size of $I'$ goes to 0 when the level of renormalization is deeper, and from Lemma 4.4 we get that $\displaystyle ({\partial f_L}/{\partial b}) ( f_L^{k-1} {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b) )$ and $\displaystyle ({\partial f_L}/{\partial b}) ( f_L^{k-1}(b-1) )$ are both greater than a positive constant $c>1/3$ . With the same arguments, we prove that $\displaystyle | {\partial \tilde {b} }/{\partial \alpha } |$ and $\displaystyle | {\partial \tilde {b} }/{\partial \beta } |$ are big.

Remark 4.7. We note that all the calculations used to get $({\partial \tilde {\alpha }}/{\partial \alpha })(x)$ in the above proof of Lemma 4.6 we can use to get the others partial derivatives $({\partial \tilde {\alpha }}/{\partial \beta })(x)$ , $ ({\partial \tilde {\alpha }}/{\partial b })(x)$ , ${\partial \tilde {\alpha }}/{\partial \eta _L} $ , and $({\partial \tilde {\alpha }}/{\partial \eta _R })(x)$ , just observing that in each case the constants will depend on the specific partial derivative we are calculating, that is, in the calculation of $({\partial \tilde {\alpha }}/{\partial \eta _L })(x)$ the constants $C_1$ and $C_2$ will depend on ${\partial f_L}/{\partial \eta _L }$ .

4.2 The $B_{\underline {f}}$ matrix

(4.36) $$ \begin{align} B_{\underline{f}} = \left( \begin{array}{@{}cc@{}} \displaystyle \frac{\partial \tilde{\alpha}}{\partial \eta_L } & \displaystyle \frac{\partial \tilde{\alpha}}{\partial \eta_R } \\ & \\ \displaystyle \frac{\partial \tilde{\beta}}{\partial \eta_L } & \displaystyle \frac{\partial \tilde{\beta}}{\partial \eta_R } \\ & \\ \displaystyle \frac{\partial \tilde{b}}{\partial \eta_L } & \displaystyle \frac{\partial \tilde{b}}{\partial \eta_R } \\ \end{array} \right). \end{align} $$

Lemma 4.8. Let $\underline {f} \in \underline {\mathcal D}_0$ . The maps

(4.37) $$ \begin{align}\begin{aligned} {\mathcal C}^1([0, 1]) \ni \eta_L & \mapsto (\tilde{\alpha}, \tilde{\beta}, \tilde{b}) \in (0, 1)^3, \\ {\mathcal C}^1([0, 1]) \ni \eta_R & \mapsto (\tilde{\alpha}, \tilde{\beta}, \tilde{b}) \in (0, 1)^3 \end{aligned}\end{align} $$

are differentiable. Moreover, for any $\varepsilon>0$ , if $\underline g\in \underline {\mathcal {D}}$ is infinitely renormalizable, and $\underline f=\underline {\mathcal {R}}^n\underline g$ , then there exists $n_0\in \mathbb N$ so that for $n\geq n_0$ , we have that $\displaystyle | {\partial \tilde {\alpha }}/{\partial \eta _L }|, \displaystyle |{\partial \tilde {\alpha }}/{\partial \eta _R }|, \displaystyle | {\partial \tilde {\beta }}/{\partial \eta _L }|, \displaystyle |{\partial \tilde {\beta }}/{\partial \eta _R }|<\varepsilon $ , $\displaystyle |{\partial \tilde {b}}/{\partial \eta _R }|=0,$ and $\displaystyle | {\partial \tilde {b}}/{\partial \eta _L }|\asymp {b}/{|I'|}$ , where $I'$ is as defined in §3.3.

Proof From (3.11), the expressions of the partial derivatives of $\tilde {\alpha }$ , $\tilde {\beta }$ , and $\tilde {b}$ are given by

(4.38) $$ \begin{align} \begin{aligned} \displaystyle \frac{\partial }{\partial *} \tilde{\alpha } & = \displaystyle \frac{1}{(0_{k+1}^{+})^2} \cdot \bigg\{ 0_{k+1}^{+} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k \circ f_R \circ f_L (0_{k+1}^{+}) ) - 0_{k+1}^{+} \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+2}^{-} ) \\ & \quad \,-\; \displaystyle [ f_L^k \circ f_R \circ f_L (0_{k+1}^{+}) - 0_{k+2}^{-} ] \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+1}^{+} ) \bigg\}, \\ \displaystyle \frac{\partial }{\partial *} \tilde{\beta } & = \displaystyle \frac{1}{(0_{k+2}^{-})^2} \cdot \bigg\{ 0_{k+2}^{-} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k \circ f_R (0_{k+2}^{-}) ) - 0_{k+2}^{-} \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+1}^{+} ) \\ &\quad\, -\;\displaystyle [ f_L^k \circ f_R (0_{k+2}^{-}) - 0_{k+1}^{+} ] \cdot \displaystyle \frac{\partial }{\partial *} ( 0_{k+2}^{-} ) \bigg\},\\ \displaystyle \frac{\partial }{\partial *} \tilde{b} & = (1-\tilde{b}) \cdot |I'|^{-1} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k \circ f_R(b) ) + |I'|^{-1} \cdot \tilde{b} \cdot \displaystyle \frac{\partial }{\partial *} ( f_L^k (b-1) ), \end{aligned} \end{align} $$

where $* \in \{ \eta _L, \eta _R \}$ . With similar arguments used in the proof of Lemma 4.6, we can prove that

$$ \begin{align*} \displaystyle \frac{\partial \tilde{\alpha}}{\partial \eta_L}, \;\; \frac{\partial \tilde{\alpha}}{\partial \eta_R}, \;\; \frac{\partial \tilde{\beta}}{\partial \eta_L}, \;\; \frac{\partial \tilde{\beta}}{\partial \eta_R} \end{align*} $$

are as small as we want.

Now let us estimate

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_L} \tilde{b} \quad \text{and} \quad \frac{\partial }{\partial \eta_R} \tilde{b}. \end{align*} $$

Observe that at deep levels of renormalization, the diffeomorphic parts $\varphi _L$ and $\varphi _R$ are very close to the identity function, so we can assume that

$$ \begin{align*} \varphi_L(x) = x + o(\epsilon), \quad \varphi_R(x) = x + o(\epsilon) \end{align*} $$

where $\epsilon>0$ is arbitrarily small. With some manipulations, we get from (4.38)

(4.39) $$ \begin{align} \displaystyle \frac{\partial }{\partial \eta_L} \tilde{b} & = \displaystyle \frac{1}{|I'|^2} \cdot \bigg\{ 0_{k+2}^{-} \cdot \frac{\partial }{\partial \eta_L} ( 0_{k+1}^{+} ) -0_{k+1}^{+} \cdot \frac{\partial }{\partial \eta_L} ( 0_{k+2}^{-}) \bigg\}. \end{align} $$

Let us analyze each term inside the braces separately. Since

$$ \begin{align*}0_{k+1}^{+} = f_L^k(b-1) = f_L (f_L^{k-1}(b-1))\text{ and }f_L = 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1},\end{align*} $$

we obtain

(4.40) $$ \begin{align} \displaystyle \frac{\partial }{\partial \eta_L} ( 0_{k+1}^{+} ) & = \displaystyle \frac{\partial }{\partial \eta_L} ( f_L (f_L^{k-1}(b-1)) ) \notag \\[5pt] & = D 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} ( \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} {{\kern0.5pt}\circ{\kern0.5pt}} f_L^{k-1}(b-1) ) \cdot \displaystyle \frac{\partial }{\partial \eta_L} ( \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)) ) \notag \\[5pt] & \asymp |T_{0,L}| \cdot \min\{ \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)), 1- \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)) \}\notag \\[5pt] & \asymp |T_{0,L}| \cdot \min\{ 1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)), 1- 1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)) \} \notag\\[5pt] & = |T_{0,L}| \cdot (1-1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)) ). \end{align} $$

By using analogous arguments, we get

(4.41) $$ \begin{align} \displaystyle \frac{\partial }{\partial \eta_L} ( 0_{k+2}^{-} ) & = \displaystyle \frac{\partial }{\partial \eta_L} ( f_L (f_L^{k-1}(f_R(b))) )\notag\\[5pt] & \asymp |T_{0,L}| \cdot (1-1_{I_{0,L}}^{-1} (f_L^{k-1}(f_R(b))) ). \end{align} $$

Substituting (4.41) and (4.40) into (4.39), we get

(4.42) $$ \begin{align} \displaystyle \frac{\partial }{\partial \eta_L} \tilde{b} & \! \asymp \displaystyle \frac{|T_{0,L}|}{|I'|^2}\! \cdot\! \{ 0_{k+2}^{-} \!\cdot\! (1-1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)) )\! - \! 0_{k+1}^{+} \cdot (1-1_{I_{0,L}}^{-1} (f_L^{k-1}(f_R(b))) ) \}\notag\\[5pt] & = \displaystyle \frac{|T_{0,L}|}{|I'|^2} \cdot [ 0_{k+2}^{-} - 0_{k+1}^{+} ] + \displaystyle \frac{|T_{0,L}|}{|I'|^2} \cdot 0_{k+1}^{+} \cdot 1_{I_{0,L}}^{-1} (f_L^{k-1}(f_R(b))) \notag \\[5pt] & \quad- \displaystyle \frac{ |T_{0,L}| }{|I'|^2} \cdot 0_{k+2}^{-} \cdot 1_{I_{0,L}}^{-1} (f_L^{k-1}(b-1)). \end{align} $$

Since the size of the renormalization interval $I'$ goes to zero when the level of renormalization goes to infinity, we can assume that $b-0_{k+2}^{-} \asymp b$ , and then we have

(4.43) $$ \begin{align} |0_{k+1}^{-}|=0-f_L^{k-1}(f_R(b)) = \frac{b-0_{k+2}^{-}}{Df_L(c_1)} \asymp \frac{b}{Df_L(c_1)} \asymp b \cdot \frac{|I_{0,L}|}{|T_{0,L}|}, \end{align} $$

where we use the assumption that

(4.44) $$ \begin{align} \left. \begin{array}{@{}r@{}c@{}l} f_L &\; = \; & 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} \\ \varphi_L & \; \approx \; & \text{identity function} \\ \end{array} \right\} \Rightarrow Df_L = \frac{|T_{0,L}|}{|I_{0,L}|} \cdot D \varphi_L \asymp \frac{|T_{0,L}|}{|I_{0,L}|}. \end{align} $$

By using the approximation (4.44), we have

(4.45) $$ \begin{align} |0_{k}^{+}|=0-f_L^{k-1}(b-1) = \frac{b-0_{k+1}^{+}}{Df_L(c_2)} \asymp (b-0_{k+1}^{+}) \cdot \frac{|I_{0,L}|}{|T_{0,L}|}. \end{align} $$

Using (4.45), (4.44), and the definition of the affine map $1_{I_{0,L}}^{-1}$ by (4.42), we obtain

(4.46) $$ \begin{align} \displaystyle \frac{\partial }{\partial \eta_L} \tilde{b} \asymp \displaystyle \frac{-b}{|I'|} + \frac{0_{k+1}^{+} \cdot 0_{k+2}^{-}}{|I'|^2}. \end{align} $$

Since $I'= [0_{k+1}^{+}, 0_{k+2}^{-}]$ and $|I'| \leq \alpha \cdot \beta \cdot b$ for all $k \geq 1$ , we can conclude that $\displaystyle ({0_{k+1}^{+} \cdot 0_{k+2}^{-}})/{|I'|^2}$ is bounded and thus $\displaystyle | ({\partial }/{\partial \eta _L}) \tilde {b} | \asymp {-b}/{|I'|}.$ For the derivative of $\tilde {b}$ with respect to $\eta _R$ , we start by noting that $0_{k+1}^{+} = f_L^{k}(b-1)$ does not depend on $\eta _R$ . Hence, with similar arguments used to get (4.39), we obtain

(4.47) $$ \begin{align} \displaystyle \frac{\partial }{\partial \eta_R} \tilde{b} & = \displaystyle \frac{1}{|I'|^2} \cdot \bigg\{ 0_{k+2}^{-} \cdot \frac{\partial }{\partial \eta_R} ( 0_{k+1}^{+} ) -0_{k+1}^{+} \cdot \frac{\partial }{\partial \eta_R} ( 0_{k+2}^{-}) \bigg\} \nonumber\\ & = \displaystyle \frac{-0_{k+1}^{+}}{|I'|^2} \cdot Df_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b) \cdot \frac{\partial }{\partial \eta_R} ( f_R(b) ). \end{align} $$

Since $\displaystyle f_R = 1_{T_{o,R}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}$ and the point $1_{I_{0,R}}^{-1}(b)$ is always fixed by any $\varphi _R \in \text {Diff}_{+}^{3}[0,1]$ , we obtain

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_R} ( \varphi_R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}(b) ) =0 \end{align*} $$

and then

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_R} ( f_R(b) ) = D1_{T_{0,R}} {{\kern0.5pt}\circ{\kern0.5pt}} ( \varphi_R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}(b) ) \cdot \frac{\partial }{\partial \eta_R} ( \varphi_R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}(b) ) = 0 \end{align*} $$

which implies in

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_R} \tilde{b}=0 \end{align*} $$

as desired.

4.3 The $C_{\underline {f}}$ matrix

(4.48) $$ \begin{align} C_{\underline{f}} = \left( \begin{array}{@{}ccc@{}} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \alpha } & \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \beta } & \displaystyle \frac{\partial \tilde{\eta}_L}{\partial b } \\[-5pt] & & \\ \displaystyle \frac{\partial \tilde{\eta}_R}{\partial \alpha } & \displaystyle \frac{\partial \tilde{\eta}_R}{\partial \beta } & \displaystyle \frac{\partial \tilde{\eta}_R}{\partial b } \end{array} \right). \end{align} $$

Lemma 4.9. Let $ \underline {f} \in \underline {\mathcal D}_0 $ . The maps

(4.49) $$ \begin{align}\begin{aligned} (0,1)^3 \ni (\alpha, \beta, b) & \mapsto \tilde{\eta}_L \in {\mathcal C}^{1}([0, 1]), \\ {(0, 1)^3} \ni (\alpha, \beta, b) & \mapsto \tilde{\eta}_R \in {\mathcal C}^{1}([0, 1]) \end{aligned} \end{align} $$

are differentiable and the partial derivatives are bounded. Furthermore, for any $\varepsilon>0$ , if $\underline g\in \underline {\mathcal {D}}_0$ is an infinitely renormalizable mapping, there exists $n_0\in \mathbb N$ so that if $n\geq n_0$ and $\underline f=\underline {\mathcal {R}}^n \underline g$ , we have that $ \displaystyle | {\partial \tilde {\eta }_L}/{\partial \beta }|$ and $\displaystyle | {\partial \tilde {\eta }_R}/{\partial \beta }|<\varepsilon $ , when $\sigma _f=-$ , and when $\sigma _f=+$ we have that $ \displaystyle |{\partial \tilde {\eta }_L}/{\partial \alpha }|$ and $\displaystyle |{\partial \tilde {\eta }_R}/{\partial \alpha }|<\varepsilon .$

We will require some preliminary results before proving this lemma. For the next calculations we deal only with the case $\sigma _f=-$ , since case $\sigma _f=+$ is analogous. From (3.12), the partial derivatives of $\tilde {\eta }_L$ with respect to $\alpha $ , $\beta $ , and b are given by

(4.50) $$ \begin{align} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \alpha} & = \displaystyle \frac{\partial }{\partial \alpha} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \nonumber\\[4pt] & = \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \alpha} ( 0_{k+1}^+ ) + \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \alpha} ( \eta_{\tilde{f}_L} ), \end{align} $$

(4.51) $$ \begin{align} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \beta} & = \displaystyle \frac{\partial }{\partial \beta} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \nonumber\\[4pt] & = \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \beta} ( 0_{k+1}^+ ) + \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \beta} ( \eta_{\tilde{f}_L} ), \end{align} $$

(4.52) $$ \begin{align} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial b} & = \displaystyle \frac{\partial }{\partial b} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \nonumber\\[4pt] & = \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial b} ( 0_{k+1}^+ ) + \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial b} ( \eta_{\tilde{f}_L} ). \end{align} $$

We have similar expressions for the partial derivatives of $\tilde {\eta }_R$ with respect to $\alpha , \beta $ , and b; however, we omit them at this point.

In order to prove that all the six entries of the $C_{\underline {f}}$ matrix are bounded, we need to analyze the terms

$$ \begin{align*} \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ), \;\; \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ), \;\; \frac{\partial }{\partial *} ( 0_{k+1}^+ ), \;\; \displaystyle \frac{\partial }{\partial *} ( \eta_{\tilde{f}_L} ) \end{align*} $$

with $* \in \{ \alpha , \beta , b\}$ for $\tilde {\eta }_L$ , and the corresponding ones for $\tilde {\eta }_R$ . This analysis will be done in the following lemmas.

Lemma 4.10. [27, Lemma 8.20]

Let $\varphi \in \mathrm{Diff}_{\kern0.5pt+}^{\kern2pt3}([0,1])$ . The zoom curve $Z:[0,1]^2 \ni (a,b) \mapsto Z_{[a,b]} \varphi \in \mathrm{Diff}_{+}^2([0,1])$ is differentiable with partial derivatives given by

(4.53) $$ \begin{align} \begin{aligned} \displaystyle \frac{\partial Z_{[a,b]} \varphi }{\partial a} & = (b-a)(1-x)D \eta ((b-a)x+a)-\eta ((b-a)x+a), \\ \displaystyle \frac{\partial Z_{[a,b]} \varphi }{\partial b} & = (b-a)xD \eta ((b-a)x+a)+\eta ((b-a)x+a). \end{aligned} \end{align} $$

The norms are bounded by

(4.54) $$ \begin{align} \bigg| \displaystyle \frac{\partial Z_{[a,b]} \varphi }{\partial a} \bigg|_{2}, \bigg| \displaystyle \frac{\partial Z_{[a,b]} \varphi }{\partial b} \bigg|_{2} \leq 2|\varphi|_3. \end{align} $$

Furthermore, by considering a fixed interval $I \subset [0,1]$ , the zoom operator

(4.55) $$ \begin{align} \begin{array}{lllll} Z_I & \!\!\!:\!\!\! &\!\!\!\! \mathcal{C}^1([0,1])& \rightarrow & \mathcal{C}^1([0,1]) \\ & & \varphi & \mapsto & Z_I \varphi, \end{array} \end{align} $$

where $Z_I \varphi (x)$ is defined in (3.10), is differentiable with respect to $\eta $ and its derivative is given by

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \varphi } ( Z_I \varphi ) (\Delta g) = |I| \cdot \Delta g {{\kern0.5pt}\circ{\kern0.5pt}} 1_I, \end{align*} $$

and its norm is given by

$$ \begin{align*} \Bigg\| \displaystyle \frac{\partial }{\partial \varphi } ( Z_I \varphi ) \Bigg\| = |I|. \end{align*} $$

Since the nonlinearity of affine maps is zero, it is not difficult to check that the nonlinearity of the branches $f_L = 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1}$ and $f_R = 1_{T_{0,R}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}$ are

(4.56) $$ \begin{align} \begin{aligned} N f_L & = \displaystyle \frac{1}{|I_{0,L}|} \cdot N \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1}, \\ N f_R & =\displaystyle \frac{1}{|I_{0,R}|} \cdot N \varphi_R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}. \end{aligned} \end{align} $$

Hence, we note that $N f_L$ depends only on b and $\varphi _L$ , while $Nf_R$ depends only on b and $\varphi _R$ . Thus, we can derive $N f_L$ with respect to b and $\varphi _L$ , and we can derive $N f_R$ with respect to b and $\varphi _R$ . This is treated in the next result.

Lemma 4.11. Let $\underline {f} \in \underline {\mathcal D}_0$ and let g be a $\mathcal {C}^1$ function. If the partial derivatives of g with respect to $\alpha $ , $\beta $ , and b are bounded, then whenever the expressions make sense, the compositions $Nf_L {{\kern0.5pt}\circ{\kern0.5pt}} g (x)$ and $Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} g(x)$ are differentiable, and the corresponding partial derivatives are bounded.

Proof From (4.56) and Lemma 4.1, we get

(4.57) $$ \begin{align} \displaystyle \frac{\partial }{\partial b} [ Nf_L {{\kern0.5pt}\circ{\kern0.5pt}} g(x) ] & = \displaystyle \frac{- ({\partial }/{\partial b})|I_{0,L}|}{|I_{0,L}|^2} \cdot N \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} {{\kern0.5pt}\circ{\kern0.5pt}} g(x) \notag\\ &\quad + \displaystyle \frac{1}{|I_{0,L}|} \cdot D N \varphi_L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1} {{\kern0.5pt}\circ{\kern0.5pt}} g(x) \cdot \displaystyle \frac{\partial }{\partial b} ( 1_{I_{0,L}}^{-1} {{\kern0.5pt}\circ{\kern0.5pt}} g(x)). \end{align} $$

For $Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} g(x)$ , we have a similar expression for its derivative with respect to b just changing $I_{0,L}$ by $I_{0,R}$ and $\varphi _L$ by $\varphi _R$ . The other partial derivatives are

(4.58) $$ \begin{align} \begin{aligned} \displaystyle \frac{\partial }{\partial *} [ Nf_L {{\kern0.5pt}\circ{\kern0.5pt}} g(x) ] &= \displaystyle DNf_L {{\kern0.5pt}\circ{\kern0.5pt}} g(x) \cdot \displaystyle \frac{\partial }{\partial *} g(x), \\ \displaystyle \frac{\partial }{\partial *} [ Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} g(x) ] &= \displaystyle DNf_R {{\kern0.5pt}\circ{\kern0.5pt}} g(x) \cdot \displaystyle \frac{\partial }{\partial * } g(x), \end{aligned} \end{align} $$

where $* \in \{ \alpha , \beta \}$ . Since our gap mappings $f=(f_L, f_R, b)$ have Schwarzian derivative $Sf$ and nonlinearity $Nf$ bounded, by the formula of the Schwarzian derivative

$$ \begin{align*} \displaystyle Sf = D(Nf) - \tfrac{1}{2}(Nf)^2, \end{align*} $$

we obtain that the derivative of the nonlinearity $D(Nf)$ is bounded. Using the hypothesis that the function g has bounded partial derivatives, the result follows as desired.

The next result is about a property that the nonlinearity operator satisfies and which we will need. A proof for it can be found in paper [29].

Lemma 4.12. The chain rule for the nonlinearity operator. If $\phi , \psi \in \mathcal {D}^2$ , then

(4.59) $$ \begin{align} N(\psi {{\kern0.5pt}\circ{\kern0.5pt}} \phi) = N \psi {{\kern0.5pt}\circ{\kern0.5pt}} \phi \cdot D \phi + N \phi. \end{align} $$

An immediate consequence of Lemma 4.12 is the following result.

Corollary 4.13. The operators

(4.60) $$ \begin{align} \begin{aligned} (\alpha, \beta, b) & \mapsto \eta_{\tilde{f}_L}:=N(\tilde{f}_L)=N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L),\\ (\alpha, \beta, b) & \mapsto \eta_{\tilde{f}_R}:=N(\tilde{f}_R)=N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R), \end{aligned} \end{align} $$

are differentiable. Furthermore, their partial derivatives are bounded.

Proof From Lemma 4.12, we obtain

(4.61) $$ \begin{align} \begin{aligned} \eta_{\tilde{f}_L} & = N(\tilde{f}_L) = N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \\ & = \displaystyle \sum_{i=1}^{k}Nf_L ( f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L ) \cdot Df_{L}^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \\ &\quad + Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot Df_L + Nf_L, \\ \eta_{\tilde{f}_R} & = N(\tilde{f}_R) = N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R) \\ & = \displaystyle \sum_{i=1}^{k}Nf_L ( f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R ) \cdot Df_{L}^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R \cdot Df_R +Nf_R. \end{aligned} \end{align} $$

Taking $* \in \{ \alpha , \beta , b \}$ , we have

(4.62) $$ \begin{align} \displaystyle \frac{\partial }{\partial *} \eta_{\tilde{f}_L} & = \displaystyle \sum_{i=1}^{k} \frac{\partial }{\partial *} [ Nf_L ( f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L ) \cdot Df_{L}^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) ]\nonumber \\ &\quad + \displaystyle \frac{\partial }{\partial *} [ Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot Df_L ] + \frac{\partial }{\partial *} [ Nf_L ] \end{align} $$

and

(4.63) $$ \begin{align} \displaystyle \frac{\partial }{\partial *} \eta_{\tilde{f}_R} = \displaystyle \sum_{i=1}^{k} \frac{\partial }{\partial *} [ Nf_L ( f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R ) \cdot Df_{L}^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R \cdot Df_R ] + \displaystyle \frac{\partial }{\partial *} [ Nf_R ]. \end{align} $$

Since $f_L = 1_{T_{0,L}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _L {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,L}}^{-1}$ , $f_R = 1_{T_{0,R}} {{\kern0.5pt}\circ{\kern0.5pt}} \varphi _R {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0,R}}^{-1}$ , $T_{0,L}= [\alpha (b-1)+b, b]$ , $T_{0,R}= [b-1, \beta b + b-1]$ , $I_{0,L}= [b-1, 0]$ , and $I_{0,R}= [0, b]$ , we obtain

$$ \begin{align*} Df_L = \displaystyle \frac{|T_{0,L}|}{|I_{0, L}|} \cdot D \varphi_L = \alpha \cdot D \varphi_L \quad \text{and} \quad Df_R = \displaystyle \frac{|T_{0,R}|}{|I_{0, R}|} \cdot D \varphi_R = \beta \cdot D \varphi_R. \end{align*} $$

Hence, we get that

$$ \begin{align*} \displaystyle \frac{\partial }{\partial * } Df_L \quad \text{and} \quad \frac{\partial }{\partial * } Df_R \end{align*} $$

are bounded for $* \in \{ \alpha , \beta , b \}$ . From this and from Lemma 4.11, the result follows.

Proof of Lemma 4.9 Let us assume that $\sigma =-;$ the proof for $\sigma =+$ is similar. By the last four results, we have that the partial derivatives of $\tilde \eta _L$ and $\tilde \eta _R$ , with respect to $\alpha $ and b, are bounded. It remains for us to show that $ \displaystyle | {\partial \tilde {\eta }_L}/{\partial \beta }|$ and $\displaystyle |{\partial \tilde {\eta }_R}/{\partial \beta }|$ are arbitrarily small at sufficiently deep renormalization levels. Notice that we have $0_{k+1}^{+}= f_L^k(b-1)$ and $0_{k+2}^{-}= f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R (b)$ , then $ \displaystyle ({\partial 0_{k+1}^{+}})/{\partial \beta } = 0 $ and

$$ \begin{align*} \displaystyle \frac{\partial 0_{k+2}^{-}}{\partial \beta} = D f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b) \cdot \frac{\partial f_R}{\partial \beta }(b) = b \cdot D f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R(b), \end{align*} $$

which goes to zero when the renormalization level goes to infinity.

4.4 The $D_{\underline {f}}$ matrix

(4.64) $$ \begin{align} D_{\underline{f}} = \left( \begin{array}{@{}cc@{}} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \eta_L } & \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \eta_R } \\[-5pt] & \\ \displaystyle \frac{\partial \tilde{\eta}_R}{\partial \eta_L } & \displaystyle \frac{\partial \tilde{\eta}_R}{\partial \eta_R } \end{array} \right). \end{align} $$

Lemma 4.14. Let $\underline {f} \in \underline {\mathcal D}_0$ . The maps

(4.65) $$ \begin{align} \begin{aligned} {\mathcal C}^1([0, 1])^1 \ni (\eta_L, \eta_R) & \mapsto \tilde{\eta}_L \in {\mathcal C}^1([0, 1]), \\ {\mathcal C}^1([0, 1])^1 \ni (\eta_L, \eta_R) & \mapsto \tilde{\eta}_R \in {\mathcal C}^1([0, 1]) \end{aligned} \end{align} $$

are differentiable. Furthermore, for any $\varepsilon>0$ and infinitely renormalizable $\underline g\in \underline {\mathcal {D}}_0,$ we have that there exists $n_0\in \mathbb N,$ so that if $n\geq n_0$ and $\underline f=\underline {\mathcal {R}}^n \underline g,$ we have that each $\displaystyle |{\partial \tilde \eta _i}/{\partial \eta _j}|<\varepsilon ,$ for $i,j\in \{L,R\}.$

We will prove this lemma after some preparatory results.

Lemma 4.15. Let

(4.66) $$ \begin{align} \begin{array}{@{}lllll} G & \!\!\!:\!\!\! &\!\!\!\! \mathrm{Diff}_+^1([0, 1]) & \rightarrow & \mathcal{C}^1([0, 1]) \\ & & \eta & \mapsto & G(\eta) \\ \end{array} \end{align} $$

be a $\mathcal {C}^1$ operator with bounded derivative. Let $\underline {f} \in \underline {\mathcal D}_0 $ . The operators

(4.67) $$ \begin{align} \begin{array}{@{}lllll} H_1, H_2 & \!\!\!:\!\!\! &\!\!\!\! \mathrm{Diff}_+^3([0, 1]) & \rightarrow & \mathcal{C}^1([0, 1]), \\ & & \eta_{\star} & \mapsto & \left\{ \begin{array}{@{}l} H_1(\eta_{\star})=Nf_{L} {{\kern0.5pt}\circ{\kern0.5pt}} G(\eta_{\star}), \\ H_2(\eta_{\star})=Nf_{R} {{\kern0.5pt}\circ{\kern0.5pt}} G(\eta_{\star}), \end{array} \right. \\ \end{array} \end{align} $$

where $\star \in \{ L, R \}$ , are differentiable.

Proof Using the partial derivative operator $\partial $ , we obtain

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_{\star}} [ H_{1}(\eta_{\star})] = \displaystyle \frac{\partial }{\partial \eta_{\star}} [ Nf_{L}] {{\kern0.5pt}\circ{\kern0.5pt}} G (\eta_{\star}) + D(Nf_{L}) {{\kern0.5pt}\circ{\kern0.5pt}} G(\eta_{\star}) \cdot \frac{\partial }{\partial \eta_{\star}} [ G(\eta_{\star}) ] \end{align*} $$

and

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_{\star}} [ H_{2}(\eta_{\star})] = \displaystyle \frac{\partial }{\partial \eta_{\star}} [ Nf_{R}] {{\kern0.5pt}\circ{\kern0.5pt}} G (\eta_{\star}) + D(Nf_{R}) {{\kern0.5pt}\circ{\kern0.5pt}} G(\eta_{\star}) \cdot \frac{\partial }{\partial \eta_{\star}} [ G(\eta_{\star}) ], \end{align*} $$

with $\star \in \{ L, R \}$ .

Lemma 4.16. The operator $ F : \mathrm{Diff}_{+}^{3}([0, 1])= {\mathcal C}^1([0, 1]) \rightarrow {\mathcal C}^1([0, 1])$

$$ \begin{align*} \begin{array}{@{}lllll} F & \!\!\!:\!\!\! & \eta & \mapsto & F(\eta) = D \varphi_{\eta}(x)\\ \end{array} \end{align*} $$

is differentiable and its derivative is bounded.

Proof Since the nonlinearity is a bijection, given a nonlinearity $\eta \in {\mathcal C}^1([0, 1])$ , its corresponding diffeomorphism is given explicitly by

$$ \begin{align*} \varphi_{\eta}(x) = \displaystyle \frac{\int_{0}^{x}e^{\int_{0}^{s} \eta (t) dt}ds}{\int_{0}^{1}e^{\int_{0}^{s} \eta (t) dt}ds}, \end{align*} $$

and the derivative of $\varphi _{\eta }(x)$ is

$$ \begin{align*} D \varphi_{\eta}(x) = \displaystyle \frac{e^{\int_{0}^{x} \eta (t) dt}}{\int_{0}^{1}e^{\int_{0}^{s} \eta (t) dt}ds}. \end{align*} $$

Thus, the derivative of F can be calculated and is

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta} ( D \varphi_{\eta}(x)) \Delta \eta = \frac{e^{\int_{0}^{x} \eta}}{\big( \int_{0}^{1}e^{\int_{0}^{s} \eta} ds \big)^2} \cdot \bigg[ \int_{0}^{1} e^{\int_{0}^{s} \eta } ds \cdot \int_{0}^{x} \Delta \eta - \int_{0}^{1} \bigg[e^{\int_{0}^{s} \eta } \cdot \int_{0}^{s} \Delta \eta\bigg]\,ds \bigg]. \end{align*} $$

From this expression, it is possible to check and conclude that

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta} ( D \varphi_{\eta}(x)) \Delta \eta \end{align*} $$

is bounded as we desire.

Corollary 4.17. Let

(4.68) $$ \begin{align} \begin{array}{@{}lllll} G & \!\!\!:\!\!\! &\!\!\!\! \mathrm{Diff}_+^1([0, 1]) & \rightarrow & \mathcal{C}^1([0, 1]) \\ & & \eta & \mapsto & G(\eta) \\ \end{array} \end{align} $$

be a $\mathcal {C}^1$ operator with bounded derivative. Let $\underline {f} \in \underline {\mathcal D}_0 $ . The operators

(4.69) $$ \begin{align} \begin{array}{@{}lllll} H_{1}, H_2 & \!\!\!:\!\!\! &\!\!\!\! \mathrm{Diff}_+^3([0, 1]) & \rightarrow & \mathcal{C}^1([0, 1]), \\ & & \eta_{\star} & \mapsto & \left\{ \begin{array}{@{}l} H_{1}(\eta_{\star})=Df_{L} {{\kern0.5pt}\circ{\kern0.5pt}} G(\eta_{\star}), \\ H_{2}(\eta_{\star})=Df_{R} {{\kern0.5pt}\circ{\kern0.5pt}} G(\eta_{\star}), \\ \end{array} \right. \end{array} \end{align} $$

where $\star \in \{ L, R \}$ , are differentiable and their derivatives are bounded.

Now we can make the proof of Lemma 4.14.

Proof of Lemma 4.14 The proof will be done just for the case where $\sigma _f = -$ . The case where $\sigma _f=+$ is analogous and we leave it to the reader. From (3.11), the partial derivatives of $\tilde {\eta }_L$ with respect to $\eta _L$ and $\eta _R$ are given by

(4.70) $$ \begin{align} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \eta_L} & = \displaystyle \frac{\partial }{\partial \eta_L} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} )\notag \\ & = \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \eta_L} ( 0_{k+1}^+ ) + \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \eta_L} ( \eta_{\tilde{f}_L} ) \end{align} $$

and

(4.71) $$ \begin{align} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \eta_R} & = \displaystyle \frac{\partial }{\partial \eta_R} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \notag\\ & = \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \eta_R} ( 0_{k+1}^+ ) + \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \cdot \displaystyle \frac{\partial }{\partial \eta_R} ( \eta_{\tilde{f}_L} ), \end{align} $$

respectively. From Lemma 4.10, we know that

$$ \begin{align*} \displaystyle \frac{\partial }{\partial 0_{k+1}^+} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \end{align*} $$

is bounded and

$$ \begin{align*} \Bigg\| \displaystyle \frac{\partial }{\partial \eta_{\tilde{f}_L}} ( Z_{[0_{k+1}^+,0]} \eta_{\tilde{f}_L} ) \Bigg\| = |0_{k+1}^{+}| \rightarrow 0 \end{align*} $$

when the level of renormalization tends to infinity. Hence, $\| \displaystyle {\partial }/{\partial \eta _{\tilde {f}_L}} ( Z_{[0_{k+1}^+,0]} \eta _{\tilde {f}_L} ) \|$ is as small as we desire. From (4.40) (in the proof of Lemma 4.8), we have

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_L} ( 0_{k+1}^{+} ) \asymp |T_{0,L}| \cdot ( 1- 1_{I_{0,L}}^{-1}(f_L^{k-1}(b-1)) ), \end{align*} $$

which is also as small as we desire. Since $0_{k+1}^{+}=f_L^k(b-1)$ does not depend on $\varphi _R$ , we have

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_R} ( 0_{k+1}^{+} )=0. \end{align*} $$

Hence, in order to prove that

$$ \begin{align*} \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \eta_L} \quad \text{and} \quad \displaystyle \frac{\partial \tilde{\eta}_L}{\partial \eta_R} \end{align*} $$

are tiny, we just need to prove that

$$ \begin{align*} \displaystyle |0_{k+1}^{+}| \cdot \bigg| \frac{\partial }{\partial \eta_L} ( \eta_{\tilde{f}_L} )\bigg| \quad \text{and} \quad \displaystyle |0_{k+1}^{+}| \cdot \bigg| \frac{\partial }{\partial \eta_R} ( \eta_{\tilde{f}_L} ) \bigg| \end{align*} $$

are tiny. Since $\eta _{\tilde {f}_L}=N(\tilde {f}_L)=N(f_L^k {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) $ from (4.62), we obtain

(4.72) $$ \begin{align} \begin{array}{@{}r@{\,}c@{\,}l} \displaystyle \frac{\partial }{\partial \eta_L} ( \eta_{\tilde{f}_L} ) &\; =\; & \displaystyle \sum_{i=1}^{k} \bigg\{ \frac{\partial }{\partial \eta_L} [ N f_L (f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) ] \cdot Df_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \\[-5pt] & & \\ & & \displaystyle +\; N f_L (f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \cdot \frac{\partial }{\partial \eta_L} [ Df_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L ] \cdot D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \\[-5pt] & & \\ & & \displaystyle +\; N f_L (f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \cdot Df_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot \frac{\partial }{\partial \eta_L} [ D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) ] \bigg\} \\ & & \\ & & +\; \displaystyle \frac{\partial }{\partial \eta_L} [ Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L ] \cdot Df_L + Nf_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L \cdot \frac{\partial }{\partial \eta_L} [ Df_L ] + \frac{\partial }{\partial \eta_L} [ Nf_L ]. \\ \end{array} \end{align} $$

Since our gap mappings $f=(f_L, f_R, b)$ have bounded Schwarzian derivative $Sf$ and bounded nonlinearity $Nf$ , by the formula for the Schwarzian derivative of f

$$ \begin{align*} \displaystyle Sf = D(Nf)- \tfrac{1}{2}(Nf)^2, \end{align*} $$

we obtain that $D(Nf_{L})$ and $D(Nf_{R})$ are bounded. As

$$ \begin{align*} Nf_{L} = \displaystyle \frac{1}{|I_{0, {L}}|} \cdot N \varphi_{L} {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0, {L}}} ^{-1} \quad \text{and} \quad Nf_{R} = \displaystyle \frac{1}{|I_{0, {R}}|} \cdot N \varphi_{R} {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0, {R}}} ^{-1} \end{align*} $$

we have

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_{\star}} [ Nf_{L}] = \frac{1}{|I_{0, {L}}|} \cdot \frac{\partial }{\partial \eta_{\star}} [ N \varphi_{L} ] {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0, {L}}} ^{-1} = \frac{1}{|I_{0, {L}}|} \cdot \frac{\partial }{\partial \eta_{\star}} [ \eta_{\varphi_{L} } ] {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0, {L}}} ^{-1}, \end{align*} $$

and

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_{\star}} [ Nf_{R}] = \frac{1}{|I_{0, {R}}|} \cdot \frac{\partial }{\partial \eta_{\star}} [ N \varphi_{R} ] {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0, {R}}} ^{-1} = \frac{1}{|I_{0, {R}}|} \cdot \frac{\partial }{\partial \eta_{\star}} [ \eta_{\varphi_{R} } ] {{\kern0.5pt}\circ{\kern0.5pt}} 1_{I_{0, {R}}} ^{-1}, \end{align*} $$

where $\star \in \{ L, R \}$ and, at this point, we are calling $\eta _{\star } = \eta _{\varphi _{\star }}$ for sake of simplicity. As

$$ \begin{align*} \displaystyle Df_L = \frac{|T_{0,L}|}{|I_{0,L}|} \cdot D \varphi_L \quad \text{and} \quad \displaystyle Df_R = \frac{|T_{0,R}|}{|I_{0,R}|} \cdot D \varphi_L, \end{align*} $$

we obtain that the product

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_L} [ N f_L (f_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) ] \cdot D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) \end{align*} $$

is bounded. From Corollary 4.17, we obtain that all the terms

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_L} [ Df_L^{k-i} {{\kern0.5pt}\circ{\kern0.5pt}} f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L ], \;\; \frac{\partial }{\partial \eta_L} [ D(f_R {{\kern0.5pt}\circ{\kern0.5pt}} f_L) ] \;\; \text{and} \;\;\frac{\partial }{\partial \eta_L} [ Df_L ] \end{align*} $$

are also bounded. From Lemma 4.4, we obtain that

$$ \begin{align*} \displaystyle \frac{\partial }{\partial \eta_L} ( f_L ) \end{align*} $$

is bounded. Furthermore, we know that

$$ \begin{align*} \displaystyle |0_{k+1}^{+}| \cdot \frac{\partial }{\partial \eta_L} [ Nf_L ] \longrightarrow 0 \end{align*} $$

when the level of renormalization tends to infinity. Hence, using Lemma 4.4, Lemma 4.15, Lemma 4.16, and Corollary 4.17, we conclude that

$$ \begin{align*} \displaystyle |0_{k+1}^{+}| \cdot \bigg| \frac{\partial }{\partial \eta_L} ( \eta_{\tilde{f}_L} ) \bigg| \end{align*} $$

is tiny. Analogously, we obtain that

$$ \begin{align*} \displaystyle |0_{k+1}^{+}| \cdot \bigg| \frac{\partial }{\partial \eta_L} ( \eta_{\tilde{f}_R} ) \bigg| \end{align*} $$

is also tiny, which completes the proof of Lemma 4.14, as desired.

5 Manifold structure of the conjugacy classes

5.1 Expanding and contracting directions of $D\underline {\mathcal {R}}_{\underline f}$

Let $\underline f_n$ be the nth renormalization of an infinitely renormalizable dissipative gap mapping in the decomposition space. In this section, we will assume that $\sigma _{f_n}=-$ . The case when $\sigma _{f_n}=+$ is similar. For any $\varepsilon>0$ , there exists $n_0\in \mathbb N$ so that for $n\geq n_0$ , we have that

$$ \begin{align*}D\underline{\mathcal{R}}_{\underline f_n}\asymp\left[\begin{array}{@{}ccccc@{}} \varepsilon & \varepsilon & \varepsilon & \varepsilon &\varepsilon\\ \varepsilon & \varepsilon & \varepsilon & \varepsilon &\varepsilon\\ K_1 & K_2 & \displaystyle\frac{\partial \tilde b}{\partial b} & \displaystyle\frac{\partial \tilde b}{\partial \eta_L} & 0 \\ [4pt] C_1 & \varepsilon & \displaystyle\frac{\partial\tilde \eta_L}{\partial b} &\varepsilon &\varepsilon \\ [4pt] C_2 & C_3 & \displaystyle\frac{\partial\tilde \eta_R}{\partial b} &\varepsilon &\varepsilon \\ \end{array}\right],\end{align*} $$

where $K_i$ are large for $i\in \{1,2\}$ and $C_j$ are bounded for $j\in \{1,2,3\}.$ We highlight the partial derivatives that will be important in the following calculations. Let

$$ \begin{align*} K_3=\partial \tilde b/\partial b, \quad K_4={\partial \tilde b}/{\partial \eta_L} \end{align*} $$

$$ \begin{align*} M_1=\partial\tilde \eta_L/\partial b,\quad \text{ and } \quad M_2=\partial\tilde \eta_R/\partial b. \end{align*} $$

Proposition 5.1. For any $\delta>0$ , there exists $n_0\in \mathbb N,$ so that for all $n\geq n_0$ , we have the following.

• • $T_{\underline {\mathcal {R}}_{\underline {\mathcal {R}}^n\underline f}} \underline {\mathcal {D}}=E^u\oplus E^s,$ and the subspace $E^u$ is one-dimensional.

• • For any vector $v\in E^u$ , we have that $\|D\underline {\mathcal {R}}_{\underline {\mathcal {R}}^n\underline f}v\|\geq \unicode{x3bb} _1\|v\|$ , where $|\unicode{x3bb} _1|>1/\delta $ .

• • For any $v\in E^s$ , we have that $\|D\underline {\mathcal {R}}_{\underline {\mathcal {R}}^n\underline f}v\|\leq \unicode{x3bb} \|v\|$ , where $|\unicode{x3bb} |<\delta $ .

Proof By taking n large, we can assume that $\varepsilon $ is arbitrarily small. To see that for $\varepsilon $ sufficiently small the tangent space admits a hyperbolic splitting, it is enough to check that this holds for the matrix:

$$ \begin{align*}\left[\begin{array}{@{}ccccc@{}} 0 & 0 & 0 & 0& 0\\ 0& 0 & 0& 0& 0\\ K_1 & K_2 & K_3 & K_4 & 0\\ C_1 & 0 & M_1 &0&0\\ C_2 & C_3 & M_2 &0&0 \\ \end{array}\right].\end{align*} $$

Calculating

$$ \begin{align*} \mathrm{det}\left[\begin{array}{@{}ccccc@{}} \unicode{x3bb} & 0 & 0 & 0& 0\\ 0& \unicode{x3bb} & 0& 0& 0\\ -K_1 & -K_2 & \unicode{x3bb}-K_3 & -K_4 & 0\\ C_1 & 0 & -M_1 &\unicode{x3bb}&0\\ C_2 & C_3 & -M_2 &0&\unicode{x3bb} \\ \end{array}\right] &=\unicode{x3bb}^2\mathrm{det} \left[\begin{array}{@{}ccc} \unicode{x3bb}-K_3& -K_4 & 0\\ -M_1 &\unicode{x3bb}&0\\ -M_2 &0&\unicode{x3bb} \\ \end{array}\right]\notag \\ &= \unicode{x3bb}^2((\unicode{x3bb}-K_3)\unicode{x3bb}^2+K_4(-M_1\unicode{x3bb})) \notag\\ &=\unicode{x3bb}^3 ((\unicode{x3bb}-K_3)\unicode{x3bb}+K_4(-M_1) )\end{align*} $$

has zero as a root with multiplicity three, and the remaining roots are the zeros of the quadratic polynomial $\unicode{x3bb} ^2- K_3\unicode{x3bb} -K_4 M_1,$ which are given by

$$ \begin{align*}\frac{K_3\pm\sqrt{K_3^2+4K_4 M_1}}{2}.\end{align*} $$

We immediately see that $({K_3+\sqrt {K_3^2+4K_4 M_1}})/{2}$ is much bigger than one, when $K_3=\partial \tilde b/\partial b$ is large.

Now, we show that

$$ \begin{align*}\bigg|\frac{K_3-\sqrt{K_3^2+4K_4 M_1}}{2}\bigg| =\frac{\sqrt{K_3^2+4K_4 M_1}-K_3}{2} \end{align*} $$

is small.

We have that

$$ \begin{align*}\frac{\sqrt{K_3^2+4K_4 M_1}-K_3}{2}= \frac{K_3}{2}\bigg(\sqrt{1+4\frac{K_4 M_1}{K_3^2}}-1\bigg).\end{align*} $$

By (4.35) and (4.46), we have that

$$ \begin{align*}\bigg|\frac{K_4}{K_3}\bigg|\leq \frac{b/|I'|+C'}{1/3|I|'}\leq C b,\end{align*} $$

where $C,C'$ are bounded. For deep renormalizations, we have that b is arbitrarily close to zero, for otherwise $0$ is contained in the gap $(f_R(b), f_L(b-1)),$ which is close to $(b~-~1,b)$ at deep renormalization levels.

Thus we have that

$$ \begin{align*}\frac{K_3}{2}\bigg(\sqrt{1+4\frac{K_4 M_1}{K_3^2}}-1\bigg) \leq \frac{K_3}{2}\bigg(\sqrt{1+4Cb\frac{M_1 +M_2}{K_3}}-1\bigg).\end{align*} $$

For large $K_3$ , by L’Hopital’s rule, we have that this is approximately

$$ \begin{align*}Cb \frac{M_1 + M_2}{\sqrt{1+4Cb \frac{M_1+M_2}{K_3}}}.\end{align*} $$

Finally by Corollary 4.13, we have that $M_1+M_2$ is bounded. Hence for deep renormalizations,

$$ \begin{align*}\bigg|\frac{K_3-\sqrt{K_3^2+4K_4 M_1}}{2}\bigg|\end{align*} $$

is close to zero.

5.2 Cone field

Recall our expression of

$$ \begin{align*}D\underline{\mathcal{R}}_{\underline f_n}\quad\text{as}\quad \left[\begin{array}{@{}cc@{}} A_{\underline f_n} & B_{\underline f_n}\\ C_{\underline f_n} & D_{\underline f_n} \end{array} \right].\end{align*} $$

We will omit the subscripts when it will not cause confusion.

For $r\in (0,1)$ , we define the cone

$$ \begin{align*}C_r=\{(\Delta\alpha,\Delta\beta,\Delta b)\in(0,1)^3:\Delta\alpha+\Delta\beta\leq r\Delta b \}.\end{align*} $$

Note that we regard cones as being contained in the tangent space of the decomposition space.

Lemma 5.2. For any $\unicode{x3bb} _0>1$ and every $r\in (0,1),$ there exists $n_0$ , so that for all $n\geq n_0$ , the cone $C_r$ is invariant and expansive, that is:

• • $A_{\underline f_n}(C_r)\subset C_{r/3}$ ; and

• • if $v\in C_r$ , then $|A_{\underline f_n}v|>\unicode{x3bb} _0|v|.$

Proof For all n sufficiently large, we have that $A_{\underline f_n}$ is of the order

$$ \begin{align*}\left[\begin{array}{@{}ccc@{}} \varepsilon & \varepsilon & \varepsilon \\ \varepsilon & \varepsilon & \varepsilon \\ K_1 & K_2 & \displaystyle\frac{\partial \tilde b}{\partial b} \end{array}\right] \end{align*} $$

Let $\Delta v=(\Delta \alpha ,\Delta \beta ,\Delta b)\in C_r,$ and $\Delta \tilde v=(\Delta \tilde \alpha ,\Delta \tilde \beta ,\Delta \tilde b)=A_{\underline f_n}\Delta v$ .

To see that the cone is invariant, we estimate

$$ \begin{align*}\frac{|(\Delta \tilde\alpha,\Delta\tilde\beta)|}{|\Delta \tilde b|}\leq \frac{2\varepsilon(|\Delta\alpha +\Delta\beta+\Delta b| )}{K_3|\Delta b|}\leq r/3,\end{align*} $$

provided that

$$ \begin{align*}\frac{1+r}{r}\leq \frac{K_3}{6\varepsilon}.\end{align*} $$

To see that the cone is expansive, we estimate

when $K_3$ is sufficiently large.

Lemma 5.3. For all $0<r<1/2$ and every $\unicode{x3bb}>0$ , there exists $\delta>0$ such that

$$ \begin{align*}C_{r,\delta}=\{\underline f\in\underline{\mathcal{D}}:|\Delta\eta_{L}|,|\Delta\eta_R|\leq \delta\Delta b, \Delta\alpha+\Delta\beta<r\Delta b\}\end{align*} $$

is a cone field in the decomposition space. Moreover, if $\underline f\in \underline {\mathcal {D}}$ is an infinitely renormalizable dissipative gap mapping, then for all n sufficiently big:

• • $D\underline {\mathcal {R}}_{\underline f_n}(C_{r,\delta })\subset C_{r/2,\delta /2}$ ; and

• • if $v\in C_{r,\delta }$ , then $|D\underline {\mathcal {R}}_{\underline f}v|>\unicode{x3bb} |v|.$

Proof Set $\Delta v=(\Delta \alpha , \Delta \beta , \Delta b, \Delta \eta _L,\Delta \eta _R)$ , $\Delta X=(\Delta \alpha , \Delta \beta , \Delta b),$ and $\Delta \Phi =(\Delta \eta _L,\Delta \eta _R).$ As before, we mark the corresponding objects under renormalization with a tilde. Then we have that

$$ \begin{align*}D\underline{\mathcal{R}}_{\underline f_n}(\Delta v)= \left[\begin{array}{@{}cc@{}} A & B\\ C & D \end{array}\right] \left[\begin{array}{@{}c@{}} \Delta X \\ \Delta\Phi\end{array}\right]= \left[\begin{array}{@{}c@{}} A\Delta X + B\Delta\Phi\\ C\Delta X+ D\Delta\Phi\end{array}\right].\end{align*} $$

We let $(\Delta \hat \alpha , \Delta \hat \beta , \Delta \hat b)=A\Delta X.$

First, we show that $|\Delta \tilde b|$ is much bigger than $\Delta b.$ By Lemma 5.2, we have that

where we can take $\unicode{x3bb} _0>0$ arbitrarily large. Thus we have that $(1+r/3)|\Delta \hat b|\geq \unicode{x3bb} _0|\Delta b|,$ and so, since $r\in (0,1),$

To see that $|\Delta \tilde b|$ is much bigger than $\Delta b$ , observe that $|\Delta \tilde b-\Delta \hat b|\leq \varepsilon (\Delta \eta _L+\Delta \eta _R)<2\varepsilon \delta |\Delta b|.$ So

when $\unicode{x3bb} _0$ is large enough.

Now, we prove that the cone is invariant. First of all, we have

for $\unicode{x3bb} _0$ large enough. Second, we have that

$$ \begin{align*} \Delta \tilde \Phi = C\Delta X +D\Delta\Phi, \end{align*} $$

where the entries of C and D are bounded, say by $K>0$ , so that

for $\unicode{x3bb} _0$ sufficiently large.

Now let us show that the cone is expansive.

for $\delta $ small enough. We also have that

$$ \begin{align*} |\Delta v|\leq |\Delta\alpha| +|\Delta \beta|+|\Delta b| +|\Delta \eta_L|+|\Delta\eta_R| \leq (r+1+\delta)|\Delta b|. \end{align*} $$

Hence

which we can take as large as we like.

Lemma 5.4. Let $\underline {f} \in \underline {\mathcal {D}}$ be a renormalizable dissipative gap mapping. If $\Delta \tilde {v} = D \underline {\mathcal {R}}_{\underline {f}} ( \Delta v ) \notin C_{r, \delta }$ , then there exists a constant $K>0$ such that

1. (i) $| \Delta b | \leq K \cdot |I '| \cdot \|\Delta v \|$ ,

2. (ii) $\| \Delta \tilde {v} \| \leq K \|\Delta v\|$ ,

where $I'$ is the domain of the renormalization $\underline {\mathcal {R}}_{\underline {f}}$ before rescaling.

Proof For convenience, in this proof we express $\underline {f}$ in new coordinates, $\underline {f} = (b,x)$ , where $x=(\alpha , \beta , \eta _L, \eta _R )$ . We use the same notation for a vector $\Delta v =(\Delta b, \Delta x)$ , where $\Delta x = (\Delta \alpha , \Delta \beta , \Delta \eta _L, \Delta \eta _R)$ . Since $\Delta \tilde {v} = D \underline {\mathcal {R}}_{\underline {f}} ( \Delta v )$ it is not difficult to check that

$$ \begin{align*} \displaystyle \Delta \tilde{b} = K_1 \cdot \Delta \alpha + K_2 \cdot \Delta \beta + \frac{\partial \tilde{b}}{\partial b} \cdot \Delta b + \frac{\partial \tilde{b}}{\partial \eta_L} \cdot \eta_L +0 \cdot \Delta \eta_R. \end{align*} $$

Using Lemmas 4.6 and 4.8, we get

(5.1) $$ \begin{align} \displaystyle \frac{|\Delta \tilde{b}|}{|\Delta b|} \asymp \frac{1}{|I'|}. \end{align} $$

From the hypothesis $\Delta \tilde {v} = ( \Delta \tilde {b}, \Delta \tilde {x} ) = D \underline {\mathcal {R}}_{\underline {f}} ( \Delta v ) \notin C_{r, \delta }$ we have

(5.2) $$ \begin{align} |\Delta \tilde{b}| \leq C \cdot \|\Delta \tilde{x}\| \end{align} $$

for some constant $C>0$ . This inequality together with (5.1) imply in

$$ \begin{align*} |\Delta b| \asymp C \cdot |I'| \cdot \| \Delta v\|, \end{align*} $$

which proves statement (i). For statement (ii), we just observe that except for two entries on the third line of matrix

$$ \begin{align*} D\underline{\mathcal{R}}_{\underline f_n} = \left[\begin{array}{@{}cc@{}} A_{\underline f_n} & B_{\underline f_n}\\ C_{\underline f_n} & D_{\underline f_n} \end{array} \right], \end{align*} $$

all the others entries are bounded. Then we obtain

(5.3) $$ \begin{align} \| \Delta \tilde{x} \| = O ( \| \Delta v\| ). \end{align} $$

Since

$$ \begin{align*} \| \Delta \tilde{v} \| = |\Delta \tilde{b}| + \| \Delta \tilde{x}, \| \end{align*} $$

from (5.2), we obtain

$$ \begin{align*} \| \Delta \tilde{v} \| \leq C \cdot \| \Delta \tilde{x} \| + \|\Delta \tilde{x} \| \end{align*} $$

and from (5.3), we are done.

5.3 Conjugacy classes are $\mathcal C^1$ manifolds

Let $\underline f\in \underline {\mathcal {D}}$ be an infinitely renormalizable gap mapping, regarded as an element of the decomposition space. Let $\underline {\mathcal {T}}_{\underline f}\subset \underline {\mathcal {D}}$ be the topological conjugacy class of $\underline f$ in $\underline {\mathcal {D}}$ .

Observe that for $M>0$ sufficiently large and $\varepsilon>0$ sufficiently small,

$$ \begin{align*}B_0=\{(\alpha, \beta,\eta_L,\eta_R):|\eta_L|,|\eta_R|<M;\alpha, \beta<\varepsilon\}\end{align*} $$

is an absorbing set for the renormalization operator acting on the decomposition space; that is, for every infinitely renormalizable $\underline f\in \underline {\mathcal {D}},$ there exists $M>0$ with the property that for any $\varepsilon>0,$ there exists $n_0\in \mathbb N,$ so that for any for $n\geq n_0$ , $\underline {\mathcal {R}}^n\underline f\in B_0.$

To conclude the proof of Theorem 1.1, we make use of the graph transform. We refer the reader to §2 of paper [27], for the proofs of some of the results in this section. Let

$$ \begin{align*} X_0=\{w\in C(B,[0,1]): \text{for all } p,q\in \text{graph}(w), q-p\notin C_{r,\delta}\}. \end{align*} $$

A $\mathcal C^1$ curve $\gamma :[0,1]\to \mathcal D$ is called almost horizontal if the tangent vector $ T_{\gamma (\xi )}\gamma (\xi )\in C_{r,\delta }$ , for all $\xi \in (0,1)$ with $\gamma (0)=(\alpha ,\beta ,0,\eta _L,\eta _R)$ , and $\gamma (1)=(\alpha ,\beta ,1,\eta _L,\eta _R)$ . Notice that for any almost horizontal curve $\gamma $ , and $w\in X_0$ , there is a unique point $w^\gamma =\gamma \cap \mathrm {graph}(w)$ . For any $p,q\in \gamma $ , we set $\ell _{\gamma }(p,q)$ to be the length of the shortest curve in $\gamma $ connecting p and q.

For $w_1,w_2\in X_0$ , let

$$ \begin{align*} d_0(w_1,w_2)=\sup_{\gamma}\ell_{\gamma}(w_1^\gamma,w_2^\gamma). \end{align*} $$

It is easy to see that $d_0$ is a complete metric on $X_0$ . Let $w\in X_0$ , $\psi \in B_0$ and let $\gamma _{\psi }$ be the horizontal line at $\psi $ . Then there exists a subcurve of $\gamma _\psi $ corresponding to a renormalization window that is mapped to an almost horizontal curve $\tilde \gamma $ under renormalization.

We define the graph transform by

$$ \begin{align*} Tw(\psi)=\underline{\mathcal{R}}^{-1}((\underline{\mathcal{R}}w)^{\tilde\gamma}). \end{align*} $$

By paper [17], we have that if $\underline f_b=(\alpha , \beta , b,\eta _L,\eta _R)$ and $\underline f_{b'}=(\alpha , \beta , b',\eta _L,\eta _R)$ are two n-times renormalizable dissipative gap mappings with the same combinatorics, then for every $\xi \in [b,b']$ , we have that $(\alpha , \beta , \xi ,\eta _L,\eta _R)$ is n-times renormalizable with the same combinatorics. It follows from the invariance of the cone field that $Tw\in X_0$ and by Lemma 5.3, we have that T is a contraction. From these considerations, we have that T has a fixed point $w^*$ and that the graph of $w^*$ is contained in $\{(\alpha , \beta ,b,\eta _L,\eta _R)\in \mathcal D:(\alpha ,\beta ,\eta _L,\eta _R)\in B_0\}$ .

Proposition 5.5. We have that $\underline {\mathcal {T}}_{\underline f}\cap B_0$ is a $\mathcal C^1$ manifold.

To prove this proposition, we use the graph transform acting to plane fields to show that $\underline {\mathcal {T}}_{\underline f}\cap B_0$ has a continuous field of tangent planes.

A plane is a codimension-one subspace of $\mathbb {R} \times B_0$ which is the graph of a functional $b^* \in \mathrm {Dual}(B_0)$ . By identifying the plane with the corresponding functional $b^*$ , we have that $\mathrm {Dual}(B_0)$ is the space of planes and carries a corresponding complete distance $d^*_{B_0}$ .

Let us fix a constant $\chi>0$ to be chosen later.

Definition 5.6. Let $p=\underline {f} \in \mathrm {graph}(w^*)$ . A plane $V_p$ is admissible for p if it has the following properties:

1. (1) if $(\Delta \alpha , \Delta \beta , \Delta b, \Delta \eta _L, \Delta \eta _R) \in V_p$ , then $|\Delta b| \leq \chi b \|(\Delta \alpha , \Delta \beta , \Delta \eta _L, \Delta \eta _R )\|$ ;

2. (2) $V_p$ depends continuously on p with respect to $d^*_{B_0}$ .

The set of admissible planes for p is denoted by $\mathrm {Dual}_p(B_0)$ .

We let $X_1$ denote the space of all admissible plane fields. For clarity of exposition, we will express $\underline {f}$ in new coordinates: $\underline {f} = (b,x)$ , where $x=(\alpha , \beta , \eta _L, \eta _R )$ . We use the same notation for a vector $\Delta v =(\Delta b, \Delta x)$ , where $\Delta x = (\Delta \alpha , \Delta \beta , \Delta \eta _L, \Delta \eta _R)$ , and although $V_{\underline {f}}^*$ is a subspace of $\mathbb {R} \times B_0$ , for the next result we abuse notation and denote the set $\{p+v | v \in V_{\underline {f}}^* \}$ also by $V_{\underline {f}}^*$ .

Let $p=(b,x)\in w^*$ and define a distance on $\mathrm {Dual}_p(B_0)$ as follows. For any two planes, $V_p,V^{\prime }_p\in \mathrm {Dual}_p(B_0),$ let $\mathcal S$ denote the set of all straight lines $\gamma $ with direction in $C_{r,\delta }.$ Provided that $\varepsilon $ is small enough, $\gamma $ intersects $V_p$ at exactly one point, and likewise for $V^{\prime }_p$ . Let $\Delta q_{\gamma }=(\Delta b_{\gamma },\Delta x_{\gamma })=\gamma \cap V_p$ and $\Delta q^{\prime }_{\gamma }=(\Delta b^{\prime }_{\gamma },\Delta x^{\prime }_{\gamma })=\gamma \cap V_p'.$ We define

$$ \begin{align*}d_{1,p}(V_p,V^{\prime}_p)= \sup_{\gamma\in\mathcal S}\frac{|\Delta b_{\gamma}-\Delta b^{\prime}_{\gamma}|}{\min\{|\Delta q_{\gamma}|,|\Delta q^{\prime}_{\gamma}|\}}.\end{align*} $$

When it will not cause confusion, we will omit $\gamma $ from the notation. It is not hard to see that $d_{1,p}$ is a complete metric. For $V,V'\in X_1,$ we define

$$ \begin{align*}d_1(V,V')=\sup_{p\in w^*}d_{1,p}(V_p,V^{\prime}_p).\end{align*} $$

On an absorbing set for renormalization operator, we have that $d_1$ is metric and $(X_1,d_1)$ is a complete metric space. This follows just as in [27, Lemmas 2.29 and 2.30].

We define the graph transform $Q:X_1\to X_1$ by

$$ \begin{align*}Q V_{\underline f}=D\underline{\mathcal{R}}_{\underline{\mathcal{R}}\underline f}^{-1}(V_{\underline{\mathcal{R}}\underline f}).\end{align*} $$

Lemma 5.7. Admissible plane fields are invariant under Q. Moreover, Q is contraction on the space $(X_1,d_1).$

Proof Let us set $p=\underline f.$ To show invariance, assume that $V_p$ is an admissible plane field, and take $(\Delta b,\Delta x)\in QV_p.$ Set $(\Delta \tilde b,\Delta \tilde x)= D\underline {\mathcal {R}}_p(\Delta b,\Delta x)\in V_{\mathcal R(p)}.$ By Lemma 5.4, we have that $\|\Delta b\|\leq K|I'|\|\Delta v\|,$ but now, since $V_{\underline {\mathcal {R}}(p)}$ is an admissible plane field, we have that $K|I'|\|\Delta v\|\leq K_1|I'|\|\Delta x\|,$ where $K_1=K_1(K,r,\delta ).$ Furthermore, if $QV_p$ is not continuous in p, then there exists a sequence $p_n \rightarrow p$ such that $QV_{p_n}$ does not converge to $QV_p$ . But now, since $QV_{p_n}$ and $QV_p$ are all codimension-one subspaces, there exists $\Delta v \in QV_{p}$ such that $\Delta v$ is transverse to $QV_{p_n}$ for all n sufficiently large. Since $V_{\underline {\mathcal {R}}(p)}$ is admissible, $D \underline {\mathcal R} \Delta v \in V_{\underline {\mathcal {R}}(p)}$ . On the other hand, we can express $\Delta v=\Delta z'\oplus \Delta z$ with $\Delta z\in C_{r,\delta }.$ By the invariance of the cone field, we have that $\Delta \tilde v=\Delta y'\oplus \Delta y$ with $\Delta y\in C_{r,\delta }$ . But now, $\Delta \tilde v$ is transverse to $V_{\underline {\mathcal {R}}(p_n)}$ for all n sufficiently big, which contradicts the admissibility of $V_p.$ Hence we have that $QV_p$ depends continuously on p.

To see that Q is a contraction, take two admissible plane fields $V,V'$ , and line $\gamma \in \mathcal S.$ Define $\Delta q=(\Delta b,\Delta x)\in V$ and $\Delta q'=(\Delta b',\Delta x')\in V$ be as in the definition of $d_{1,p}.$ Let $\Delta \tilde q=(\Delta \tilde b,\Delta \tilde x)=D\underline {\mathcal {R}}_{p}\Delta q$ , and likewise for the objects marked with a prime. Observe that by Lemma 5.4, we have that $\|\Delta q\|\geq ({1}/{C_1})\tilde \|\Delta \tilde q\|,$ and that $|\Delta b|\leq C_2|I'\|\Delta \tilde b|$ . So

$$ \begin{align*}\frac{|\Delta b-\Delta b'|}{\min\{\|\Delta q\|,\|\Delta q'\|\}}\leq C|I'|\frac{|\Delta\tilde b-\Delta\tilde b'|}{\min\{\|\Delta\tilde v\|,\|\Delta\tilde v\|\}}\leq \frac{1}{2}d_{1,\underline{\mathcal{R}}(p)}(V_{\underline{\mathcal{R}}({p)}}, V_{\underline{\mathcal{R}}(p)}').\end{align*} $$

Thus,

$$ \begin{align*}d_{1}(QV,QV')\leq\tfrac{1}{2} d_1(V,V').\\[-32pt]\end{align*} $$

Thus we have that there is an admissible plane field $V^*(\underline f),$ which is an invariant plane field under Q.

Now we conclude the proof of the proposition. We will show that for each $\underline f\in \mathrm {graph}(w^*)$ , $V^*(\underline f)=T_{\underline f}(\mathrm {graph}(w^*)).$

Let $p \in \mathrm {graph}(w^*)$ and take an almost horizontal curve $\gamma $ close enough to p such that $\gamma \cap \mathrm {graph}(w^*) = q =\{ p+ \Delta q = p+ (\Delta \alpha , \Delta \beta , \Delta b, \Delta \eta _L, \Delta \eta _R) \}$ and $\gamma \cap V_{\underline {f}}^* = q'= \{ p+ \Delta q' = p+ (\Delta \alpha ', \Delta \beta ', \Delta b', \Delta \eta _L', \Delta \eta _R') \}$ . We define

$$ \begin{align*} A = \sup_{p} \limsup_{\gamma \rightarrow p} \frac{|\Delta b - \Delta b'|}{|\Delta v|}. \end{align*} $$

A straightforward calculation shows that at deep renormalization levels, we have that $A \leq 1$ , cf. [27, Lemma 2.34].

Figure 3 Notation for the proof of Proposition 5.5.

Proof of Proposition 5.5 We show that at a deep level of renormalization, each point $\underline {f} \in \mathrm {graph(w^*)}$ has a tangent plane $T_{\underline {f}}w^* = V_{\underline {f}}^*$ . To get this result, it is enough to show that $A=0$ . We use the notation from the definition of A and we introduce the following notation:

$$ \begin{align*} \begin{array}{@{}r@{}c@{\,}l} \underline{\mathcal{R}}(\underline{f})& \,=\, & (\tilde{b}, \tilde{x}), \\[4pt] \underline{\mathcal{R}} (\gamma) \cap \mathrm{graph}(w^*) & \,=\, & \tilde{q} = \underline{\mathcal{R}}(q) = \underline{\mathcal{R}}(\underline{f}) + \Delta \tilde{q} = \underline{\mathcal{R}}(\underline{f}) + ( \Delta \tilde{b}, \Delta \tilde{x} ), \\[4pt] \underline{\mathcal{R}}(\gamma) \cap V_{\underline{\mathcal{R}}(\underline{f})}^{*} & \,=\, & z = \underline{\mathcal{R}}(\underline{f})+ \Delta z, \\[4pt] \underline{\mathcal{R}}(q') & \,=\, & \tilde{q}'= \underline{\mathcal{R}}(\underline{f})+ \Delta \tilde{q}' = \underline{\mathcal{R}}(\underline{f})+ ( \Delta \tilde{b}', \Delta \tilde{x}'), \\[4pt] z-\tilde{q} & \,=\, & ( \Delta h_1, \Delta u ), \\[4pt] \tilde{q}' - z & \,=\, & ( \Delta h, \Delta u_1 ), \\[4pt] \Delta \tilde{q}' & \,=\, & D \underline{\mathcal{R}}_{\underline{f}} ( \Delta q') + \Delta \epsilon, \\[4pt] D \underline{\mathcal{R}}_{\underline{f}} ( \Delta q') - \Delta z & \,=\, & ( \Delta h_2, \Delta u_2 ). \end{array} \end{align*} $$

For almost horizontal curves $\gamma $ such that $\gamma \cap \mathrm {graph}(w^*)$ is close enough to p, we get

(5.4) $$ \begin{align} |\Delta \epsilon | = o ( |\Delta q'| ), \end{align} $$

and

(5.5) $$ \begin{align} \| \Delta u_2 - \Delta u_1\| \leq |\Delta \epsilon|. \end{align} $$

Since $\underline {\mathcal {R}}$ has strong expansion on the b direction, and using the differentiability of $\underline {\mathcal {R}}$ , we get

(5.6) $$ \begin{align} |\Delta h_1|+|\Delta h| \geq \displaystyle \frac{1}{|I'|} \cdot |\Delta b - \Delta b'|. \end{align} $$

As $(\Delta h_2, \Delta u_2) \in V_{\underline {f}}^*$ and $V_{\underline {f}}^*$ is an admissible plane, we get

(5.7) $$ \begin{align} |\Delta h_2| \leq 2 \chi \tilde{b} \|\Delta u_2 \|. \end{align} $$

Since $q'-q = (\Delta b'- \Delta b, \Delta x'- \Delta x)$ is a tangent vector to the curve $\gamma $ , it is inside the cone $C_{r, \delta }$ , and then we get

(5.8) $$ \begin{align} \|\Delta x'- \Delta x \| < |\Delta b'- \Delta b|. \end{align} $$

As $(\Delta h, \Delta u_1)$ is a tangent vector to the curve $\underline {\mathcal {R}}_{\underline {f}}(\gamma )$ , by the same reason as before, we get

(5.9) $$ \begin{align} \|\Delta u_1 \| < |\Delta h|. \end{align} $$

By (5.7), (5.5), and (5.9), we have

$$ \begin{align*} |\Delta h | &\leq |\Delta \epsilon| + |\Delta h_2| \leq |\Delta \epsilon| + 2 \chi \tilde{b} \| \Delta u_2\| \\ & \leq |\Delta \epsilon| + 2 \chi \tilde{b} \| \Delta u_2-\Delta u_1\| + 2 \chi \tilde{b} \|\Delta u_1\|\\ & \leq |\Delta \epsilon| + 2 \chi \tilde{b} |\Delta \epsilon|+ 2 \chi \tilde{b} |\Delta h|. \end{align*} $$

Hence, when we are in a deep level of renormalization, we have

(5.10) $$ \begin{align} |\Delta h| \leq 2 |\Delta \epsilon|. \end{align} $$

Since

$$ \begin{align*} |\Delta q'| \leq |\Delta q| + \|q'-q\| = |\Delta q| + \|\Delta x' - \Delta x\| + |\Delta b'- \Delta b|, \end{align*} $$

from (5.4) and (5.8), we obtain

$$ \begin{align*} |\Delta \epsilon| = o ( |\Delta q| + 2 |\Delta b'- \Delta b| ) = o ( |\Delta q| (1+2A ) ). \end{align*} $$

Hence

(5.11) $$ \begin{align} |\Delta \epsilon| = o ( |\Delta q| ). \end{align} $$

From this and using Lemma 5.4, we have

$$ \begin{align*} \displaystyle \frac{|\Delta b - \Delta b'|}{|\Delta v|} & \leq \displaystyle \frac{C_1 \cdot |I'| \cdot ( |\Delta h_1|+|\Delta h|) }{|\Delta v|} = C_1 \cdot |I'| \cdot \frac{|\Delta h_1| }{|\Delta v|} + C_1 \cdot |I'| \cdot \frac{|\Delta h| }{|\Delta v|} \\ & = \displaystyle C_1 \cdot |I'| \cdot \frac{|\Delta \tilde{v}|}{|\Delta v|} \cdot \frac{|\Delta h_1| }{|\Delta \tilde{v}|} + C_1 \cdot |I'| \cdot \frac{|\Delta h| }{|\Delta v|} \\ & \leq C_2 \cdot |I'| \cdot \displaystyle \frac{|\Delta h_1|}{|\Delta \tilde{v}|} + o(1), \end{align*} $$

for a constant $C_2>0$ . Hence, we obtain

$$ \begin{align*} \displaystyle \limsup_{\gamma \rightarrow p} \frac{|\Delta b - \Delta b'|}{|\Delta v|} \leq O(|I'|) A. \end{align*} $$

Since $|I'|$ goes to zero when the level of renormalization goes to infinity, we conclude that $A=0$ , as desired.

Thus we have proved that there is an absorbing set, $B_0,$ for the renormalization operator within which the topological conjugacy class of $\underline f$ is a $\mathcal C^1$ manifold. It remains to prove that it is globally $\mathcal C^1$ .

By [17, Lemma 5.1], each infinitely renormalizable gap mapping $f_0=(f_{R},f_L,b_0)$ can be included in a family $f_t,$ for $t\in (-\varepsilon _0,\varepsilon _0)$ of gap mappings, which is transverse to the topological conjugacy class of $f_0.$ The construction of this family is given by varying the b parameter in a small neighborhood about $b_0$ , and observing that the boundary points of the principal gaps at each renormalization level are strictly increasing functions in b. Thus, we have that the transversality of this family is preserved under renormalization. Let $\Delta f$ denote a vector tangent to the family $f_t$ at $f.$ We have the following.

Lemma 5.8. Let $n_0\in \mathbb N$ be so that $\underline {\mathcal {R}}^{n_0}( \underline f)\in B_0.$ Then $D\underline {\mathcal {R}}^{n_0}(\Delta f)\notin T_{\underline {\mathcal {R}}^{n_0} f} \mathrm {graph}(w^*),$ where $w^*=\mathcal T_{R^{n_0}(\underline f)}\cap B_0.$

Using this lemma, we can argue as in the proof of [10, Theorem 9.1] to conclude the proof Theorem 1.1.

Theorem 5.9. $\mathcal T_f\subset \mathcal D^4$ is a $\mathcal C^1$ manifold.

Note that the application of the implicit function theorem in the proof is why we lose one degree of differentiability.