1. Introduction
GaN has recently been the subject of considerable interest due to its optoelectronic properties. In particular the wide band gap (3.4 eV for wurtzite GaN) makes blue light applications feasible. Defect–induced electronic states in the band gap can significantly alter the optical performance. This fact becomes extremely important in laser devices, where parasitic components in the emission spectrum are highly undesirable. Moreover, point defects could be trapped in the stress field of extended defects giving rise to charge accumulated in the vicinity. The resulting electrostatic field leads to electron scattering which will severely affect the electron mobility (see Look and SizeloveReference Look and Sizelove 2 for a recent model). Therefore, there is considerable interest in understanding the microstructure of extended defects in GaN and their interaction with point defects.
In this paper we present the geometries, energetics and electrical properties of extended defects in GaN using an ab initio local density–functional (LDF) cluster method, AIMPRO, and a self–consistent charge density–functional tight–binding method SCC–DFTB. The latter can be used in large supercells and enables the formation energy of the defects to be found. Details of the methods and their application to GaN and oxygen related defect complexes in GaN have been given previouslyReference Elsner, Jones, Sitch, Porezag, Elstner, Frauenheim, Heggie, Öberg and Briddon 3 –Reference Elsner, Jones, Haugk, Frauenheim, Heggie, Öberg and Briddon 5 and will not be repeated here.
2. Threading Dislocations in GaN
A. Threading Screw Dislocations
We consider first a screw dislocation with a full coreReference Elsner, Jones, Sitch, Porezag, Elstner, Frauenheim, Heggie, Öberg and Briddon 3 . Full core screw dislocations have recently been observed by Xin et al. using the high resolution Z–contrast imaging techniqueReference Xin, Pennycook, Browning, Nelist, Sivananthan, Omnès, Beaumont, Faurie and Gibart 6 . The presence of atoms so close to the dislocation axis leads to severely strained bond lengths distorted by as much as 0.4 Å. Consequently it is not surprising that such dislocations possess deep gap states ranging from E v +0:9 to Ev+1:6 eV and shallow states around Ec − 0:2 eV. An analysis of these gap states revealed that the states above E v are localised on N core atoms, whereas those below E c are localised on both Ga and N core atoms. The strong distortion of the bonds of the core atoms leads to a high line energy within our calculations.
A similar calculation was then carried out with the hexagonal core of the screw dislocation removed giving a core with a narrow opening of ≈7.2 Å. The atoms on the walls adopt three fold coordinations similar to those found on the (10
B. Threading Edge Dislocations
The relaxed core of a threading edge dislocation with Burgers vector a[1

Top view (in [0001]) of the relaxed core of the threading edge dislocation (
Table 1: Bond lengths, min-max (average) in Å and bond angles, min-max (average) in for the most distorted atoms at the core of the threading edge dislocation (
| Atom | bond lengths | bond angles |
| 1 (Ga3×coord.) | 1.85-1.86 (1.85) | 112-118 (116) |
| 2 (N3×coord.) | 1.88-1.89 (1.86) | 106-107 (106) |
| 3/4 (Ga/N4×coord.) | 1.86-1.95 (1.91) | 97-119 |
| 5/6 (Ga/N4×coord.) | 1.92-2.04 (1.97) | 100-129 |
| 7/8 (Ga/N4×coord.) | 1.94-2.21 (2.06) | 94-125 |
| 9/10 (Ga/N4×coord. ) | 1.95-2.21 (2.11) | 100-122 |
In AlN, Wright et al. Reference Wright and Furthmüller 7 have found, using a supercell geometry, that edge dislocations have an empty band of levels ∼ E c – 2 eV, while in n-type material, the core contains VA1 defects. The very much larger band gap of this material might be responsible for the differences of the properties of the edge dislocations from those in GaN.
To summarise, the density functional calculations reveal that the threading screw dislocations in their pure, i.e. impurity free form can exist with full cores and narrow open cores with diameters up to ≈7.2 Å. While full core screws are electrically active, open core screws induce no deep gap states. Threading edge dislocations in wurtzite GaN are stable with full cores and electrically inactive. However, the strained and ‘dangling’ bonds present in their cores could permit impurities and intrinsic defects to be trapped there.
3. Interaction of Oxygen with dislocations
A. Oxygen and Open Core Screw Dislocations
There is experimental evidence that oxygen acts as a donor in bulk GaNReference Wetzel, Suski, Ager, Weber, Haller, Fischer, Meyer, Molnar and Perlin 8 and total energy calculations show that O sits on a N siteReference Neugebauer and Walle 9 . Since the internal surfaces of screw dislocations are very similar to those of the low energy (10
Our calculationsReference Elsner, Jones, Haugk, Gutierrez, Frauenheim, Heggie, Öberg and Briddon 10 showed that VGa–(ON )3 is more stable at the surface than in the bulk by 2.15 eV. Two O neighbours of the surface vacancy lie below the surface and each is bonded to three Ga neighbours, but the surface O is bonded to only two subsurface Ga atoms in a normal oxygen bridge site. The defect is electrically inactive with the O atoms passivating the vacancy in the same way as VH4 in Si.
The question then arises as to the in uence of the defect on the growth of the material. Growth over the defect must proceed by adding a Ga atom to the vacant site but this leaves three electrons in shallow levels near the conduction band resulting in a very high energy. This suggests that the defect can stabilise the surface and thus inhibit growth. From this we can conclude that such defects lead to the formation of nanopipes if we assume that during growth of the epilayers, either nanopipes with very large radii are formed which gradually shrink when their surfaces grow out, or there is a rapid drift of oxygen to a preexisting nanopipe. In either case the concentration of oxygen and VGa–(ON )3 defects increases at the walls of the nanopipe. The maximum concentration of this defect would be reached if 50% (100%) of the first (second) layer N atoms were replaced by O and further growth then would be prevented. It is, however, likely that far less than the maximum concentration is necessary to stabilise the surface and make further shrinkage of the nanopipe impossible. Provided oxygen could di use to the surface fast enough, the diameter and density of the holes would be related to the initial density of oxygen atoms in the bulk. This model requires that the walls of the nanopipe are coated with oxygen although the initial stages of formation of the pipe are obscure.
In conclusion, we have shown that oxygen has a tendency to segregate to the (10
B. Oxygen and Edge Dislocations
The VGa–(ON)3 defect considered above is electrically inactive at a (10
In conclusion, the density functional calculations show that in wurtzite GaN the stress field of threading edge dislocations is likely to trap gallium vacancies and oxygen as well as their complexes resulting in a negatively charged dislocation line in n–type material.
4. Domain boundaries on {11 0} planes
0} planes
In addition to dislocations, two kinds of domain boundaries have also been observedReference Sitar, Paisley, Yan and Davis 14 –Reference Xin, Brown and Humphreys 19 . They lie on {11
In contrast to DB–II type boundaries which originate at the epilayer substrate interface the DB–I type boundaries found in a GaN sample grown by molecular beam epitaxy (MBE) on GaP extend only a short distance along the c–axisReference Xin, Brown and Humphreys 19 . A high resolution Z–contrast image down [0001] reported by Xin et al. Reference Xin, Pennycook, Browning, Nellist, Sivananthan, Faurie, Gibart, Ponce, Den Baars, Meyer, Nakamura and Strite 21 shows clearly that DB–I has a horizontal displacement of
We investigated several modelsReference Elsner, Kaukonen, Heggie, Haugk, Frauenheim and Jones 22 corresponding to this horizontal displacement and found a double position boundary to possess the lowest domain wall energy. This double position boundary has an additional vertical displacement of 1/2〈0001〉 giving a total displacement of 1/2〈10

Top view along [0001] (left) and side view along [10
5. Summary
We have presented density-funcitonal calculations for a variety of extended defects observed in wurtzite GaN. All stable structures consist of four–fold coordinated atoms or possess pairs of three–fold coordinated Ga and N atoms which adopt energetically favorable sp 2 and p 3 positions as at the {10

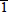 0} type surfaces are electrically inactive. They play an important role in GaN since similar configurations occur at open–core screw dislocations and nanopipes as well as at the core of threading edge dislocations. Domain boundaries are found to consist of four–fold coordinated atoms and are also found to be electrically inactive. Thus, except for full–core screw dislocations which possess heavily strained bonds all investigated extended defects do not induce deep states into the band–gap. However, electrically active impurities in particular gallium vacancies and oxygen related defect complexes are found to be trapped at the stress field of the extended defects.
0} type surfaces are electrically inactive. They play an important role in GaN since similar configurations occur at open–core screw dislocations and nanopipes as well as at the core of threading edge dislocations. Domain boundaries are found to consist of four–fold coordinated atoms and are also found to be electrically inactive. Thus, except for full–core screw dislocations which possess heavily strained bonds all investigated extended defects do not induce deep states into the band–gap. However, electrically active impurities in particular gallium vacancies and oxygen related defect complexes are found to be trapped at the stress field of the extended defects.
