Hostname: page-component-6bf8c574d5-gr6zb Total loading time: 0 Render date: 2025-02-17T01:07:32.573Z Has data issue: false hasContentIssue false
  • English
  • Français

On categories 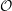${\mathcal{O}}$ for quantized symplectic resolutions

Part of: Homological methods Representation theory of rings and algebras

Published online by Cambridge University Press:  07 September 2017

Ivan Losev
Ivan Losev*
Affiliation:
Department of Mathematics, Northeastern University, 360 Huntington Avenue, Boston, MA 02115, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper we study categories 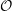${\mathcal{O}}$ over quantizations of symplectic resolutions admitting Hamiltonian tori actions with finitely many fixed points. In this generality, these categories were introduced by Braden et al. We establish a family of standardly stratified structures (in the sense of the author and Webster) on these categories 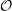${\mathcal{O}}$ . We use these structures to study shuffling functors of Braden et al. (called cross-walling functors in this paper). Most importantly, we prove that all cross-walling functors are derived equivalences that define an action of the Deligne groupoid of a suitable real hyperplane arrangement.

Keywords

category 𝓞symplectic resolutionquantizationhighest-weight structurederived equivalence

MSC classification

Primary: 16E35: Derived categories 16G99: None of the above, but in this section
Type
Research Article
Information
Compositio Mathematica , Volume 153 , Issue 12 , December 2017 , pp. 2445 - 2481
Copyright
© The Author 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baranovsky, V., Ginzburg, V., Kaledin, D. and Pecharich, J., Quantization of line bundles on Lagrangian subvarieties , Selecta Math. 25 (2016), 1–25.CrossRefGoogle Scholar
Bezrukavnikov, R. and Kaledin, D., Fedosov quantization in the algebraic context , Mosc. Math. J. 4 (2004), 559–592.CrossRefGoogle Scholar
Bezrukavnikov, R. and Losev, I., Etingof conjecture for quantized quiver varieties, Preprint (2013), arXiv:1309.1716.Google Scholar
Bialynicki-Birula, A., Some properties of the decompositions of algebraic varieties determined by actions of a torus , Bull. Acad. Polon. Sci. Ser. Sci. Math. Astronom. Phys. 24 (1976), 667–674.Google Scholar
Braden, T., Licata, A., Proudfoot, N. and Webster, B., Quantizations of conical symplectic resolutions II: category O and symplectic duality , Astérisque 384 (2016), 75–179.Google Scholar
Braden, T., Proudfoot, N. and Webster, B., Quantizations of conical symplectic resolutions I: local and global structure , Astérisque 384 (2016), 1–73.Google Scholar
Cline, E., Parshall, B. and Scott, L., Stratifying endomorphism algebras , Mem. Amer. Math. Soc. 124 (1996), no. 591.Google Scholar
Ginzburg, V., Guay, N., Opdam, E. and Rouquier, R., On the category 𝓞 for rational Cherednik algebras , Invent. Math. 154 (2003), 617–651.Google Scholar
Gordon, I., Quiver varieties, category O for rational Cherednik algebras, and Hecke algebras , Int. Math. Res. Pap. IMRP (2008), Art. ID rpn006.Google Scholar
Gordon, I. and Losev, I., On category 𝓞 for cyclotomic rational Cherednik algebras , J. Eur. Math. Soc. 16 (2014), 1017–1079.CrossRefGoogle Scholar
Kaledin, D., On the coordinate ring of a projective Poisson scheme , Math. Res. Lett. 13 (2006), 99–107.Google Scholar
Kaledin, D., Symplectic singularities from the Poisson point of view , J. reine angew. Math. 600 (2006), 135–156.Google Scholar
Losev, I. V., Finite dimensional representations of W-algebras , Duke Math. J. 159 (2011), 99–143.Google Scholar
Losev, I., Isomorphisms of quantizations via quantization of resolutions , Adv. Math. 231 (2012), 1216–1270.CrossRefGoogle Scholar
Losev, I., Holonomic modules and Bernstein inequality , Adv. Math. 308 (2017), 941–963.Google Scholar
Losev, I. and Webster, B., On uniqueness of tensor products of irreducible categorifications , Selecta Math. 21 (2015), 345–377.CrossRefGoogle Scholar
Maulik, D. and Okounkov, A., Quantum groups and quantum cohomology, Preprint (2012), arXiv:1211.1287.Google Scholar
Namikawa, Y., Flops and Poisson deformations of symplectic varieties , Publ. Res. Inst. Math. Sci. 44 (2008), 259–314.CrossRefGoogle Scholar
Namikawa, Y., Poisson deformations and birational geometry , J. Math. Sci. Univ. Tokyo 22 (2015), 339–359.Google Scholar