1. Introduction and statement of the main result
Let ![]() $\mathbb{R}[x, y]$ be the set of all real polynomials in the variables x and y. Consider the planar system
$\mathbb{R}[x, y]$ be the set of all real polynomials in the variables x and y. Consider the planar system
where ![]() $\dot x =dx/dt$,
$\dot x =dx/dt$, ![]() $\dot y =dy/dt$ and
$\dot y =dy/dt$ and ![]() $P,Q \in \mathbb{R}[x,y]$. We define the degree of system (1.1) as
$P,Q \in \mathbb{R}[x,y]$. We define the degree of system (1.1) as ![]() $\max \{\text{deg} P, \text{deg} Q\}$. In case that P and Q have no common factor, we say that (1.1) is non-degenerate. It is known that such a system can be transformed into an associated algebraic first order ordinary differential equation and so throughout the article we also consider the associated differential equation
$\max \{\text{deg} P, \text{deg} Q\}$. In case that P and Q have no common factor, we say that (1.1) is non-degenerate. It is known that such a system can be transformed into an associated algebraic first order ordinary differential equation and so throughout the article we also consider the associated differential equation
and the associated vector field ![]() $\mathcal{X} = P(x,y) \partial /\partial x + Q(x,y) \partial / \partial y$.
$\mathcal{X} = P(x,y) \partial /\partial x + Q(x,y) \partial / \partial y$.
We denote the class of all quadratic differential systems with QS. Planar polynomial differential systems occur very often in various branches of applied mathematics, in modelling natural phenomena, for example, modelling the time evolution of conflicting species, in biology, in chemical reactions, in economics, in astrophysics, in the equations of continuity describing the interactions of ions, electrons, and neutral species in plasma physics (see, for example, [Reference Chandrasekhar3, Reference Lotka12, Reference Roth20, Reference Volterra22]). Polynomial systems appear also in shock waves, in neural networks, etc. Such differential systems have also theoretical importance. Several problems on polynomial differential systems, which were stated more than one hundred years ago, are still open: the second part of Hilbert’s 16th problem stated by Hilbert in 1900, the problem of algebraic integrability stated by Poincaré in 1891 [Reference Poincaré18, Reference Poincaré19], the problem of the centre stated by Poincaré in 1885 [Reference Poincaré17], and problems on integrability resulting from the work of Darboux [Reference Darboux6] published in 1878. With the exception of the problem of the centre for quadratic differential systems, which was solved, all the other problems mentioned above, are still unsolved even in the quadratic case. To advance knowledge on algebraic integrability stated by Poincaré, it is useful to have a large number of examples to analyse. In the literature, scattered isolated examples were analysed but a more systematic approach is still needed. This is ultimately the main motivation for the work in this article. The class QSH (the whole class of non-degenerate planar quadratic systems possessing at least one invariant hyperbola) is a rich family that was studied in [Reference Oliveira, Rezende and Vulpe13–Reference Oliveira, Schlomiuk, Travaglini and Valls16]. The study of integrability of the family QSH was initiated in [Reference Oliveira, Schlomiuk and Travaglini14]. In [Reference Oliveira, Rezende, Schlomiuk and Vulpe15], the authors classified the family QSH, according to their geometric properties encoded in the configurations of invariant hyperbolas and invariant straight lines that these systems possess. The family QSH can be split as: QSH1 of systems which possess either exactly two distinct real singularities at infinity or the line at infinity filled up with singularities and QSH2 of systems which possess three distinct real singularities at infinity in ![]() $\mathbb{P}_2(\mathbb{C})$. In [Reference Oliveira, Rezende, Schlomiuk and Vulpe15], the authors proved that there are exactly 162 distinct configurations and provided necessary and sufficient conditions for a non-degenerate quadratic differential system to have at least one invariant hyperbola and for the realization of each one of the configurations (conditions expressed in terms of the coefficients of the systems).
$\mathbb{P}_2(\mathbb{C})$. In [Reference Oliveira, Rezende, Schlomiuk and Vulpe15], the authors proved that there are exactly 162 distinct configurations and provided necessary and sufficient conditions for a non-degenerate quadratic differential system to have at least one invariant hyperbola and for the realization of each one of the configurations (conditions expressed in terms of the coefficients of the systems).
In [Reference Oliveira, Rezende, Schlomiuk and Vulpe15], the authors provided all the normal forms for the family QSH for the group action of the affine transformations and time rescaling. In particular for the family QSH1, they found 13 different normal forms and in [Reference Oliveira, Schlomiuk, Travaglini and Valls16] the authors proved the integrability for systems QSH1 in 11 of the 13 normal forms of the family and the generic non-integrability for the other 2 normal forms. Although the generic non-integrability (in the sense of Liouville integrability) has been proved, what is left out is a set of measure zero and so the study is not complete and there are families with two parameters for which it is not known if they are Liouvillian integrable or not because the number of invariant curves and exponential factors are not sufficient for finding a Liouvillian first integral or an integrating factor (see §4 for their definitions). More precisely, the families that are left out are the ones in which the parameters of system A and system B satisfy ![]() $(a,g)\in L_1\cup L_2\cup C'$ and
$(a,g)\in L_1\cup L_2\cup C'$ and ![]() $(a,g)\in L_1\cup L_2$, respectively (see the notation of systems A, B, L 1, L 2 in Section 6 and Theorems 6.1 and 6.2 in [Reference Oliveira, Schlomiuk, Travaglini and Valls16]). We just recall here that Liouville integrability is related with the existence of a first integral expressible as quadratures of elementary functions.
$(a,g)\in L_1\cup L_2$, respectively (see the notation of systems A, B, L 1, L 2 in Section 6 and Theorems 6.1 and 6.2 in [Reference Oliveira, Schlomiuk, Travaglini and Valls16]). We just recall here that Liouville integrability is related with the existence of a first integral expressible as quadratures of elementary functions.
The same type of study as done for family QSH1 is done for the family QSH2 in a work in preparation. In that work, the authors prove the integrability for several normal forms in the family QSH2 and the generic non-integrability (in the sense of Liouville integrability). What is left out is a set of measure zero and there are families for which it is not known if they are Liouville integrable or not. One of these families it is the normal form (3.4) in [Reference Oliveira, Rezende, Schlomiuk and Vulpe15] with ![]() $g=1/2$, that is, the two-parameter family
$g=1/2$, that is, the two-parameter family
 \begin{equation}
x' =a(2h-1) +x + \frac 1 2 x^2 + (h-1) x y, \quad y' =-y -\frac 1 2 xy + h y^2
\end{equation}
\begin{equation}
x' =a(2h-1) +x + \frac 1 2 x^2 + (h-1) x y, \quad y' =-y -\frac 1 2 xy + h y^2
\end{equation} where ![]() $a, h \in \mathbb{R}$ with
$a, h \in \mathbb{R}$ with ![]() $a \ne 0$. Note that this family has the invariant hyperbola
$a \ne 0$. Note that this family has the invariant hyperbola ![]() $a +xy=0$. The main objective of the present article is to study the Liouvillian integrability of system (1.3). We have chosen this family because among the families for which it is not known if they are Liouvillian integrable or not it is the one having more free parameters.
$a +xy=0$. The main objective of the present article is to study the Liouvillian integrability of system (1.3). We have chosen this family because among the families for which it is not known if they are Liouvillian integrable or not it is the one having more free parameters.
The following is our main result.
Theorem 1.1 The following holds for system (1.3):
(A) We have Liouvillian integrability in the following cases:
(i) On the cubic curve
 $a (2h+1)^2-4=0$;
$a (2h+1)^2-4=0$;(ii) On the lines
 $h=1/2$ and h = 1;
$h=1/2$ and h = 1;(iii) At the following four points:
 $(a,h)\in \{(-36/25,1/3), (-1/4,-3/2), (-3/25, -3/2), (2/9,2)\}$.
$(a,h)\in \{(-36/25,1/3), (-1/4,-3/2), (-3/25, -3/2), (2/9,2)\}$.
(B) Excluding the cases provided by statement (A), we do not have Liouvillian integrability in the following cases:
(i) In the half plane
 $h \gt 1/2$, with the exception of the line h = 2;
$h \gt 1/2$, with the exception of the line h = 2;(ii) On every line h = C where
 $C \lt 1/2$ and C is not rational;
$C \lt 1/2$ and C is not rational;(iii) On the line
 $h=1/3$ for
$h=1/3$ for  $a \neq -36/25$;
$a \neq -36/25$;(iv) On every line
 $h=n/(2n+4)$ with
$h=n/(2n+4)$ with  $n \in \mathbb{N} \cup \{0\}$.
$n \in \mathbb{N} \cup \{0\}$.
Some of these are shown in figure 1.

Figure 1. Liouvillian Integrability of system (1.3) on the plane ![]() $\overline{ah}$.
$\overline{ah}$.
Corollary 1.2. The points (a, h) in the parameter space of the family (1.3) with a ≠ 0 satisfying ![]() $a (2h+1)^2-4\ne 0$ not covered by theorem 1.1 are:
$a (2h+1)^2-4\ne 0$ not covered by theorem 1.1 are:
(1) (a, h) with
 $h \lt 1/2$,
$h \lt 1/2$,  $h \ne -3/2$, h rational and
$h \ne -3/2$, h rational and  $h \ne \frac{n}{2n+4}$ for any
$h \ne \frac{n}{2n+4}$ for any  $n \in \mathbb{N} \cup \{0\}$;
$n \in \mathbb{N} \cup \{0\}$;(2)
 $(a,2)$ with
$(a,2)$ with  $a \ne 2/9$;
$a \ne 2/9$;(3)
 $(a,-3/2)$ with
$(a,-3/2)$ with  $a \ne -1/4$ and
$a \ne -1/4$ and  $a\ne -3/25$.
$a\ne -3/25$.
It is well-known that a Liouville first integral is constructed from invariant algebraic curves and exponential factors (see §4 for their definition) associated with multiple algebraic curves or to the straight line at infinity, see for instance [Reference Christopher, Llibre and Pereira5]. Both the invariant algebraic curves and the exponential factors have polynomial cofactors and a linear combination of these cofactors is the key to find Liouville first integrals and our approach will be to use Puiseux series to obtain the algebraic invariant curves that together with the exponential factors will allow us to prove theorem 1.1. More precisely, with the definition of invariant algebraic curve (see again §4), it is clear that y = 0 and ![]() $a+xy=0$ are invariant algebraic curves of system (1.1) for any value of the parameters. However, these two invariant algebraic curves are not sufficient to prove Liouvillian integrability for system (1.1) and in order to have it, the existence of more invariant algebraic curves are needed. So, we will investigate under what conditions on the parameters there are more invariant algebraic curves then y and a + xy.
$a+xy=0$ are invariant algebraic curves of system (1.1) for any value of the parameters. However, these two invariant algebraic curves are not sufficient to prove Liouvillian integrability for system (1.1) and in order to have it, the existence of more invariant algebraic curves are needed. So, we will investigate under what conditions on the parameters there are more invariant algebraic curves then y and a + xy.
For this, we will use a novel algebraic method for finding invariant algebraic curves for polynomial vector fields which is based in the representation of such an invariant algebraic curve in the field ![]() $\mathbb{C}_\infty \{y\}$, where
$\mathbb{C}_\infty \{y\}$, where ![]() $\mathbb{C}_\infty \{y\}$ is the set of formal series in fractional powers in the variable y centred at
$\mathbb{C}_\infty \{y\}$ is the set of formal series in fractional powers in the variable y centred at ![]() $\infty$ and possessing coefficients in
$\infty$ and possessing coefficients in ![]() $\mathbb{C}$. These series are called Puiseux series. This representation of the invariant algebraic curves in terms of Puiseux series is given in theorem 4.8 in §4 (see also §4 for the description of the Puiseux series method).
$\mathbb{C}$. These series are called Puiseux series. This representation of the invariant algebraic curves in terms of Puiseux series is given in theorem 4.8 in §4 (see also §4 for the description of the Puiseux series method).
The number and type of Puiseux series are very useful in order to establish a bound for the degree of the invariant algebraic curves of a given system. More precisely, in many situations, depending on the form of the system, and if the parameters of the system satisfy or not certain conditions, such invariant algebraic curve takes the form of a finite product of Puiseux series which allows us to bound the degree of the invariant algebraic curve and so to find all the irreducible invariant algebraic curves in explicit form. Moreover, even if the number of Puiseux series centred at the point ![]() $y=\infty$ is infinite, the computations of the invariant algebraic curves can still be performed obtaining all the irreducible invariant algebraic curves in explicit form for many families of differential equations.
$y=\infty$ is infinite, the computations of the invariant algebraic curves can still be performed obtaining all the irreducible invariant algebraic curves in explicit form for many families of differential equations.
To compute all the Puiseux series in the field ![]() $\mathbb{C}_\infty \{y\}$ satisfying Eq. (1.2), we need to obtain the so-called dominant balances that are combinations of monomials of Eq. (1.2) and can be obtained using the boundary of the associated Newton polygon of Eq. (1.2) (see definition 4.6 in §4). To obtain the Newton polygon, we follow the method described in §4.2.
$\mathbb{C}_\infty \{y\}$ satisfying Eq. (1.2), we need to obtain the so-called dominant balances that are combinations of monomials of Eq. (1.2) and can be obtained using the boundary of the associated Newton polygon of Eq. (1.2) (see definition 4.6 in §4). To obtain the Newton polygon, we follow the method described in §4.2.
From the Newton polygon, we obtain different balances that correspond to the different monomials of Eq. (1.2) that generate the vertices and the edges. Among them, we select the dominant balances and we look for the solutions of the equations associated with these balances that are of the form ![]() $x=cy^r$ with c ≠ 0 and
$x=cy^r$ with c ≠ 0 and ![]() $r\in \mathbb{Q}$. We will call them power solutions (if r is not rational then a power solution does not generate a Puiseux series, see (4.3) of §4). Next, we calculate the Fuchs index which is a complex number attached to the dominant balance at the solution
$r\in \mathbb{Q}$. We will call them power solutions (if r is not rational then a power solution does not generate a Puiseux series, see (4.3) of §4). Next, we calculate the Fuchs index which is a complex number attached to the dominant balance at the solution ![]() $x=cy^r$. Basically, the Fuchs index associated with a power solution
$x=cy^r$. Basically, the Fuchs index associated with a power solution ![]() $x(y)=cy^r$ of the associated equation of a dominant balance is a zero of the Gâteaux derivative of the balance evaluated at the solution. The Fuchs index is important, especially when it is a rational positive number, because then it redefines the integers that appear in the fractional exponents of the Puiseux series (see (4.3) and again §4 for details of the way it is computed). Finally, we recall that to verify the existence of a Puiseux series with the correct exponent after the analysis of the Fuchs index, we need to replace the possible Puiseux series into Eq. (3.1) and get the conditions to vanish all the coefficients, called the compatibility conditions (see again the details in §4 or [Reference Demina and Kudryashov9]).
$x(y)=cy^r$ of the associated equation of a dominant balance is a zero of the Gâteaux derivative of the balance evaluated at the solution. The Fuchs index is important, especially when it is a rational positive number, because then it redefines the integers that appear in the fractional exponents of the Puiseux series (see (4.3) and again §4 for details of the way it is computed). Finally, we recall that to verify the existence of a Puiseux series with the correct exponent after the analysis of the Fuchs index, we need to replace the possible Puiseux series into Eq. (3.1) and get the conditions to vanish all the coefficients, called the compatibility conditions (see again the details in §4 or [Reference Demina and Kudryashov9]).
The article is organized as follows. In §2, we have included auxiliary results that will be used to prove theorem 1.1. The proof of theorem 1.1 is given in §3. The different cases are treated separately. All the algebraic curves used in the proof of statement A of theorem 1.1 are of degree at most two and so we just present them in the article in proposition 2.1 in §2 (they can be obtained directly). However, in order to illustrate how Puiseux series help in these cases, we have included the proof of statement A(i) of theorem 1.1 in §3 (see proposition 3.2). Finally, we have included an Appendix where we provide some general definitions, some properties of the Puiseux series as well as the definition of the Newton polygon, the precise definition of dominant balances and their construction, the precise definition of Fuchs index and the way it is computed and in general and all the necessary information and definitions to obtain Puiseux series.
2. Auxiliary results
The next proposition proves theorem 1.1 (A).
Proposition 2.1. System (1.3) is Liouvillian integrable in the following cases:
(i) On the cubic curve
 $a (2h+1)^2-4=0$;
$a (2h+1)^2-4=0$;(ii) On the lines
 $h=1/2$ and h = 1;
$h=1/2$ and h = 1;(iii) At the following four points:
 $(a,h)\in \{(-36/25,1/3), (-1/4,-3/2), (-3/25, -3/2), (2/9,2)\}$.
$(a,h)\in \{(-36/25,1/3), (-1/4,-3/2), (-3/25, -3/2), (2/9,2)\}$.
Proof. If  $a=\frac{4}{(2h+1)^2}$ system (1.3) has the invariant algebraic curves y, a + xy and
$a=\frac{4}{(2h+1)^2}$ system (1.3) has the invariant algebraic curves y, a + xy and  $x-y+ \frac{4}{1+2h}$. It has the integrating factor of the form
$x-y+ \frac{4}{1+2h}$. It has the integrating factor of the form  $y^{(2h-1)/2} (a+xy)^{h-1} (x-y+ \frac{4}{1+2h})^{-(2h+1)/2}$, and so it is Liouville integrable.
$y^{(2h-1)/2} (a+xy)^{h-1} (x-y+ \frac{4}{1+2h})^{-(2h+1)/2}$, and so it is Liouville integrable.
If ![]() $h=1/2$ system (1.3) is integrable with the polynomial first integral a + xy, and so it is Liouville integrable.
$h=1/2$ system (1.3) is integrable with the polynomial first integral a + xy, and so it is Liouville integrable.
If h = 1 system (1.3) has the invariant algebraic curves y, ![]() $a+ x y$ and
$a+ x y$ and  $a+x+\frac{1}{2}x^2$. It has the integrating factor of the form
$a+x+\frac{1}{2}x^2$. It has the integrating factor of the form  $(y (a+x+\frac{1}{2}x^2)(a+xy))^{-1}$ and so it admits a Liouvillian first integral.
$(y (a+x+\frac{1}{2}x^2)(a+xy))^{-1}$ and so it admits a Liouvillian first integral.
These prove statements A(i), A(ii) of theorem 1.1.
From now on in all the article, we assume that ![]() $h \ne \{1/2,1\}$ and
$h \ne \{1/2,1\}$ and ![]() $a(2h+1)^2 -4 \ne 0$.
$a(2h+1)^2 -4 \ne 0$.
If we assume that ![]() $h=1/3$ and
$h=1/3$ and ![]() $a =-36/25$, system (1.3) has the invariant algebraic curves
$a =-36/25$, system (1.3) has the invariant algebraic curves ![]() $
y, a+xy \text{and }
1+5x/3 -a^{-1} x^2+ a^{-1}x y.$ Then, it has the integrating factor
$
y, a+xy \text{and }
1+5x/3 -a^{-1} x^2+ a^{-1}x y.$ Then, it has the integrating factor
 \begin{equation*}
y^{-2/3} \Big(-\frac{36}{25} + x y\Big)^{1/6} \Big(1+\frac 5 3 x + \frac{25}{36} x^2- \frac{25}{36} x y\Big)^{-5/6}
\end{equation*}
\begin{equation*}
y^{-2/3} \Big(-\frac{36}{25} + x y\Big)^{1/6} \Big(1+\frac 5 3 x + \frac{25}{36} x^2- \frac{25}{36} x y\Big)^{-5/6}
\end{equation*}and so it is Liouvillian integrable.
If ![]() $h=-3/2$ and
$h=-3/2$ and ![]() $a=-1/4$, system (1.3) has the invariant algebraic curves y,
$a=-1/4$, system (1.3) has the invariant algebraic curves y, ![]() $a+ x y$ and
$a+ x y$ and ![]() $2 + 2 x + x^2 + 2 y - 2 x y + y^2$. It has the integrating factor of the form
$2 + 2 x + x^2 + 2 y - 2 x y + y^2$. It has the integrating factor of the form ![]() $y (2 + 2 x + x^2 + 2 y - 2 x y + y^2)^{-1} (a+x y)^{-1/4}$ and so it admits a Liouvillian first integral. Moreover, if
$y (2 + 2 x + x^2 + 2 y - 2 x y + y^2)^{-1} (a+x y)^{-1/4}$ and so it admits a Liouvillian first integral. Moreover, if ![]() $h=-3/2$ and
$h=-3/2$ and ![]() $a =-3/25$ system (1.3) has the invariant algebraic curves y,
$a =-3/25$ system (1.3) has the invariant algebraic curves y, ![]() $a+ x y$ and
$a+ x y$ and ![]() $108 + 180 x + 75 x^2 + 140 y - 150 x y + 75 y^2$. It has the integrating factor of the form
$108 + 180 x + 75 x^2 + 140 y - 150 x y + 75 y^2$. It has the integrating factor of the form ![]() $y^{-2/3} (a+xy)^{-1/2} (108 + 180 x + 75 x^2 + 140 y - 150 x y + 75 y^2)^{-5/6}$ and so it admits a Liouvillian first integral.
$y^{-2/3} (a+xy)^{-1/2} (108 + 180 x + 75 x^2 + 140 y - 150 x y + 75 y^2)^{-5/6}$ and so it admits a Liouvillian first integral.
If h = 2 and ![]() $a=2/9$, system (1.3) has the invariant algebraic curves y,
$a=2/9$, system (1.3) has the invariant algebraic curves y, ![]() $a+ x y$ and
$a+ x y$ and ![]() $4+6x +3 x^2 -4 y$. It has the integrating factor of the form
$4+6x +3 x^2 -4 y$. It has the integrating factor of the form ![]() $(y (4+6x+3 x^2 -4y))^{-1} (a+x y)^{-1/3}$ and so it admits a Liouvillian first integral.
$(y (4+6x+3 x^2 -4y))^{-1} (a+x y)^{-1/3}$ and so it admits a Liouvillian first integral.
This previous analysis concludes the proof of the proposition.
The following two propositions will be used in the proof of theorem 1.1 (B).
Proposition 2.2. The unique monic irreducible invariant algebraic curve of system (1.3) which only depends on y is ![]() $f(y)=y$.
$f(y)=y$.
Proof. Let ![]() $f(y) \in \mathbb{C}[y]$ be an irreducible invariant curve of system (1.3) of degree n. We can write it as
$f(y) \in \mathbb{C}[y]$ be an irreducible invariant curve of system (1.3) of degree n. We can write it as ![]() $f(y)=a + b y$ with
$f(y)=a + b y$ with ![]() $b \ne 0$ (otherwise the curve would have components and would not be irreducible). Since we are assuming that the curve is monic we take b = 1. Note that f satisfies
$b \ne 0$ (otherwise the curve would have components and would not be irreducible). Since we are assuming that the curve is monic we take b = 1. Note that f satisfies
 \begin{align}
& -y(1+ \frac 1 2 x - h y) \frac{d f}{d y} =(K_0 + K_1 x +K_2 y) f, \notag\\
& \quad \text{i.e.} \quad - y (1+ \frac 1 2 x - h y) = (K_0 + K_1 x +K_2 y) (a +y),
\end{align}
\begin{align}
& -y(1+ \frac 1 2 x - h y) \frac{d f}{d y} =(K_0 + K_1 x +K_2 y) f, \notag\\
& \quad \text{i.e.} \quad - y (1+ \frac 1 2 x - h y) = (K_0 + K_1 x +K_2 y) (a +y),
\end{align} where ![]() $K_0, K_1,K_2 \in \mathbb{C}$. If
$K_0, K_1,K_2 \in \mathbb{C}$. If ![]() $a \ne 0$ then evaluating (2.1) on y = 0 we get
$a \ne 0$ then evaluating (2.1) on y = 0 we get ![]() $K_0=K_1=0$ and then, after simplifying by y, Eq. (2.1) becomes
$K_0=K_1=0$ and then, after simplifying by y, Eq. (2.1) becomes
 \begin{equation*}
- (1+ \frac 1 2 x - h y) = K_2 (a +y),
\end{equation*}
\begin{equation*}
- (1+ \frac 1 2 x - h y) = K_2 (a +y),
\end{equation*} which is not possible. So, a = 0 and ![]() $f(y)=y$, as we wanted to prove.
$f(y)=y$, as we wanted to prove.
Proposition 2.3. If y and a + xy, ![]() $a \ne 0$ are the only invariant algebraic curves that are defined by irreducible polynomials of a system (1.3) with
$a \ne 0$ are the only invariant algebraic curves that are defined by irreducible polynomials of a system (1.3) with ![]() $h \ne 1$, then the system is not Liouvillian integrable.
$h \ne 1$, then the system is not Liouvillian integrable.
Proof. We compute the exponential factors. Clearly, we may have the following exponential factors ![]() $\exp(f(x,y))$ where
$\exp(f(x,y))$ where ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$,
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$, ![]() $\exp(f(x,y)/y^n)$ where
$\exp(f(x,y)/y^n)$ where ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with y and some
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with y and some ![]() $n \in \mathbb{N}$,
$n \in \mathbb{N}$, ![]() $\exp(f(x,y)/(a+xy)^n)$ with
$\exp(f(x,y)/(a+xy)^n)$ with ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ being coprime with a + xy and
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ being coprime with a + xy and ![]() $ n\in\mathbb{N} $, or
$ n\in\mathbb{N} $, or ![]() $\exp(f(x,y)/(y^{n_1} (a+xy)^{n_2}))$ with
$\exp(f(x,y)/(y^{n_1} (a+xy)^{n_2}))$ with ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ being coprime with y and a + xy and
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ being coprime with y and a + xy and ![]() $ n_1,n_2 \in\mathbb{N} $.
$ n_1,n_2 \in\mathbb{N} $.
Assume first that we have the exponential factor of the form ![]() $\exp(f(x,y))$ where
$\exp(f(x,y))$ where ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$. Then we have
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$. Then we have
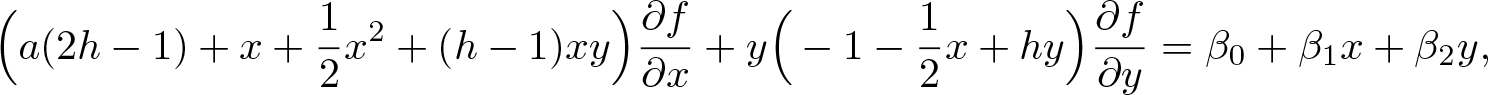 \begin{equation}
\Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y} =\beta_0 + \beta_1 x + \beta_2 y,
\end{equation}
\begin{equation}
\Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y} =\beta_0 + \beta_1 x + \beta_2 y,
\end{equation} with ![]() $\beta_i \in \mathbb{C}$.
$\beta_i \in \mathbb{C}$.
If ![]() $h \ne 0$, evaluating the above equation on
$h \ne 0$, evaluating the above equation on
 \begin{equation*}
(x,y)=(-1\pm \sqrt{1+2a-4ah},0), \quad (x,y)=\Big(-1- \sqrt{1-2ah},\frac{1-\sqrt{1-2ah}}{2h}\Big)
\end{equation*}
\begin{equation*}
(x,y)=(-1\pm \sqrt{1+2a-4ah},0), \quad (x,y)=\Big(-1- \sqrt{1-2ah},\frac{1-\sqrt{1-2ah}}{2h}\Big)
\end{equation*}and
 \begin{equation*}
(x,y)=\left(-1+ \sqrt{1-2ah},\frac{1 -\sqrt{1-2ah}}{2 h}\right)
\end{equation*}
\begin{equation*}
(x,y)=\left(-1+ \sqrt{1-2ah},\frac{1 -\sqrt{1-2ah}}{2 h}\right)
\end{equation*}we get
 \begin{equation*}
\begin{aligned}
0 & =\beta_0 + \beta_1 (-1\pm \sqrt{1+2a-4ah}),\\
0 & = \beta_0 + \beta_1 (-1 \pm \sqrt{1-2ah}) + \beta_2 \frac{1 -\sqrt{1-2ah}}{2 h}
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
0 & =\beta_0 + \beta_1 (-1\pm \sqrt{1+2a-4ah}),\\
0 & = \beta_0 + \beta_1 (-1 \pm \sqrt{1-2ah}) + \beta_2 \frac{1 -\sqrt{1-2ah}}{2 h}
\end{aligned}
\end{equation*} yielding ![]() $ \beta_0=\beta_1=\beta_2=0$ (we recall that
$ \beta_0=\beta_1=\beta_2=0$ (we recall that ![]() $h \ne 1$).
$h \ne 1$).
On the other hand, if h = 0 then evaluating (2) on
 \begin{equation*}
(x,y)=\left(-2,\frac a 2\right), \quad (x,y)=(-1\pm \sqrt{1+2a},0)
\end{equation*}
\begin{equation*}
(x,y)=\left(-2,\frac a 2\right), \quad (x,y)=(-1\pm \sqrt{1+2a},0)
\end{equation*}we get
 \begin{equation*}
0 = \beta_0 -2 \beta_1 + \frac{a \beta_2}2 ,\quad
0 =\beta_0 + \beta_1 (-1\pm \sqrt{1+2a})
\end{equation*}
\begin{equation*}
0 = \beta_0 -2 \beta_1 + \frac{a \beta_2}2 ,\quad
0 =\beta_0 + \beta_1 (-1\pm \sqrt{1+2a})
\end{equation*} which yields again ![]() $ \beta_0=\beta_1=\beta_2=0$. In short
$ \beta_0=\beta_1=\beta_2=0$. In short ![]() $\beta_i=0$ for
$\beta_i=0$ for ![]() $i=0,1,2$ and so
$i=0,1,2$ and so ![]() $\exp(f)$ is an exponential factor which is not possible.
$\exp(f)$ is an exponential factor which is not possible.
Assume now that we have the exponential factor ![]() $\exp(f(x,y)/y^n)$ where
$\exp(f(x,y)/y^n)$ where ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with y and some
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with y and some ![]() $n \in \mathbb{N}$. Then we have
$n \in \mathbb{N}$. Then we have
 \begin{align}
& \Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y} - \notag\\
& \quad -n \Big(-1-\frac 1 2 x + hy\Big)f
=(\beta_0 + \beta_1 x + \beta_2 y)y^n ,
\end{align}
\begin{align}
& \Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y} - \notag\\
& \quad -n \Big(-1-\frac 1 2 x + hy\Big)f
=(\beta_0 + \beta_1 x + \beta_2 y)y^n ,
\end{align} with ![]() $\beta_i \in \mathbb{C}$. Restricting (2.2) on y = 0 and denoting by
$\beta_i \in \mathbb{C}$. Restricting (2.2) on y = 0 and denoting by ![]() $\bar f$ the restriction of f on y = 0 we get that
$\bar f$ the restriction of f on y = 0 we get that ![]() $\bar f =\bar f(x)\ne 0$ (because f and y are coprime) and satisfies
$\bar f =\bar f(x)\ne 0$ (because f and y are coprime) and satisfies
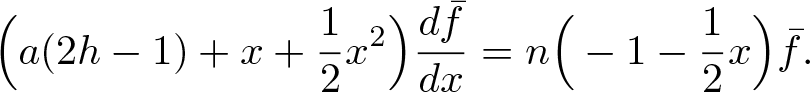 \begin{equation}
\Big( a(2h-1) + x+\frac 1 2 x^2 \Big)\frac{d \bar f}{d x}
=n \Big(-1-\frac 1 2 x \Big)\bar f.
\end{equation}
\begin{equation}
\Big( a(2h-1) + x+\frac 1 2 x^2 \Big)\frac{d \bar f}{d x}
=n \Big(-1-\frac 1 2 x \Big)\bar f.
\end{equation}Solving Eq. (2.3), we get
 \begin{equation*}
\bar f =\kappa (2+x)^{2 a (1-2h)/n} e^{(4-x^2)/(2n)}, \quad \kappa \in \mathbb{R}.
\end{equation*}
\begin{equation*}
\bar f =\kappa (2+x)^{2 a (1-2h)/n} e^{(4-x^2)/(2n)}, \quad \kappa \in \mathbb{R}.
\end{equation*} Note that ![]() $\bar f$ is never a polynomial if
$\bar f$ is never a polynomial if ![]() $\kappa \ne 0$. Therefore,
$\kappa \ne 0$. Therefore, ![]() $\bar f=0$, which is not possible. Hence such an exponential factor cannot exist.
$\bar f=0$, which is not possible. Hence such an exponential factor cannot exist.
Assume that we have the exponential factor ![]() $\exp(f(x,y)/(y^{n_1} (a+xy)^{n_2}))$ where
$\exp(f(x,y)/(y^{n_1} (a+xy)^{n_2}))$ where ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with y and a + xy and some
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with y and a + xy and some ![]() $n_1,n_2 \in \mathbb{N}$. Then we have
$n_1,n_2 \in \mathbb{N}$. Then we have
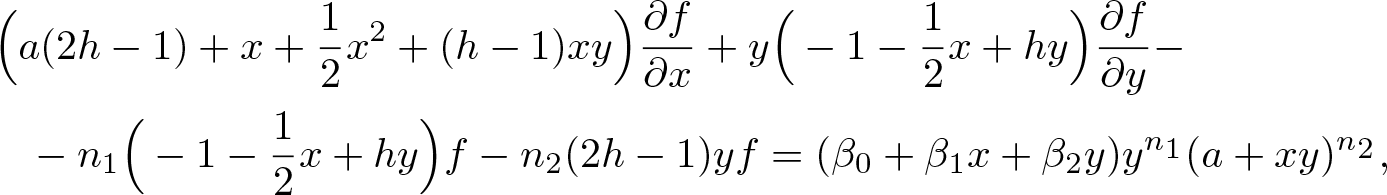 \begin{equation}
\begin{aligned}
& \Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+ y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y}-
\\
& \phantom{\le} -n_1 \Big(-1-\frac 1 2 x + hy\Big)f - n_2 (2h-1)y f
=(\beta_0 + \beta_1 x + \beta_2 y)y^{n_1} (a+xy)^{n_2} ,
\end{aligned}
\end{equation}
\begin{equation}
\begin{aligned}
& \Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+ y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y}-
\\
& \phantom{\le} -n_1 \Big(-1-\frac 1 2 x + hy\Big)f - n_2 (2h-1)y f
=(\beta_0 + \beta_1 x + \beta_2 y)y^{n_1} (a+xy)^{n_2} ,
\end{aligned}
\end{equation} with ![]() $\beta_i \in \mathbb{C}$. Restricting (2.4) on y = 0, taking into account that
$\beta_i \in \mathbb{C}$. Restricting (2.4) on y = 0, taking into account that ![]() $n_1 \ge 1$ and denoting by
$n_1 \ge 1$ and denoting by ![]() $\bar f$ the restriction of f on y = 0 we get that
$\bar f$ the restriction of f on y = 0 we get that ![]() $\bar f =\bar f(x)\ne 0$ (because f and y = 0 are coprime) and satisfies (2.3) with n replaced by n 1. The same arguments used to treat Eq. (2.3) imply that
$\bar f =\bar f(x)\ne 0$ (because f and y = 0 are coprime) and satisfies (2.3) with n replaced by n 1. The same arguments used to treat Eq. (2.3) imply that ![]() $\bar f=0$ which is not possible. Hence such an exponential factor cannot exist.
$\bar f=0$ which is not possible. Hence such an exponential factor cannot exist.
Finally, consider that we have the exponential factor ![]() $\exp(f(x,y)/(a+xy)^n)$ where
$\exp(f(x,y)/(a+xy)^n)$ where ![]() $f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with a + xy and some
$f \in \mathbb{C}[x,y] \setminus \mathbb{C}$ coprime with a + xy and some ![]() $n \in \mathbb{N}$. Then we have
$n \in \mathbb{N}$. Then we have
 \begin{equation}
\begin{aligned}
& \Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y}
\\
& \phantom{\le} - n (2h-1)y f
=(\beta_0 + \beta_1 x + \beta_2 y)(a+x y)^n ,
\end{aligned}
\end{equation}
\begin{equation}
\begin{aligned}
& \Big( a(2h-1) + x+\frac 1 2 x^2+(h-1) xy \Big)\frac{\partial f}{\partial x}
+y\Big(-1-\frac 1 2 x + hy\Big) \frac{\partial f}{\partial y}
\\
& \phantom{\le} - n (2h-1)y f
=(\beta_0 + \beta_1 x + \beta_2 y)(a+x y)^n ,
\end{aligned}
\end{equation} with ![]() $\beta_i \in \mathbb{C}$. Restricting (2.5) on
$\beta_i \in \mathbb{C}$. Restricting (2.5) on ![]() $a+xy=0$ and denoting by
$a+xy=0$ and denoting by ![]() $\bar f$ the restriction of f on
$\bar f$ the restriction of f on ![]() $y=-a/x$ we get that
$y=-a/x$ we get that ![]() $\bar f \ne 0$ (because f and
$\bar f \ne 0$ (because f and ![]() $a+x y$ are coprime and moreover it is of the form a polynomial divided by some power of x). Hence it is a rational function where the denominator is some power of x and satisfies
$a+x y$ are coprime and moreover it is of the form a polynomial divided by some power of x). Hence it is a rational function where the denominator is some power of x and satisfies
 \begin{equation*}
\Big(a h + x+\frac 1 2 x^2\Big)\frac{d \bar f}{d x}
=- n (2h-1)\frac{a}{x} \bar f.
\end{equation*}
\begin{equation*}
\Big(a h + x+\frac 1 2 x^2\Big)\frac{d \bar f}{d x}
=- n (2h-1)\frac{a}{x} \bar f.
\end{equation*}Solving it we get
 \begin{equation*}
\bar f = c_1 \Big(\frac{(\sqrt{2ah-1} + i(1+x))^{1+i/2}
(\sqrt{2ah-1}- i(1+x))^{1-i/2}}{x}\Big)^{\frac{(2h-1)n}{2h}}
\end{equation*}
\begin{equation*}
\bar f = c_1 \Big(\frac{(\sqrt{2ah-1} + i(1+x))^{1+i/2}
(\sqrt{2ah-1}- i(1+x))^{1-i/2}}{x}\Big)^{\frac{(2h-1)n}{2h}}
\end{equation*} when ![]() $h \ne 0$ and
$h \ne 0$ and
 \begin{equation*}
\bar f = c_1 \Big(\frac{2+x}{x}\Big)^{2an} \exp(-\frac{an}x)
\end{equation*}
\begin{equation*}
\bar f = c_1 \Big(\frac{2+x}{x}\Big)^{2an} \exp(-\frac{an}x)
\end{equation*} when h = 0. Clearly, in both cases we get that ![]() $\bar f$ is not of the form a polynomial divided by a power of x. Hence
$\bar f$ is not of the form a polynomial divided by a power of x. Hence ![]() $\bar f=0$, which is not possible. Hence such an exponential factor cannot exist.
$\bar f=0$, which is not possible. Hence such an exponential factor cannot exist.
In short, there are no exponential factors and the unique Darboux functions are of the form
The cofactor of G is
 \begin{equation*}
K(x,y)=\ell_0 \Big(-1-\frac 1 2 x + hy\Big) +\ell_1 (2h-1) y.
\end{equation*}
\begin{equation*}
K(x,y)=\ell_0 \Big(-1-\frac 1 2 x + hy\Big) +\ell_1 (2h-1) y.
\end{equation*} Note that ![]() $K(x,y)=0$ implies that
$K(x,y)=0$ implies that ![]() $\ell_0=\ell_1=0$ and so there are no Darboux first integrals. On the other hand, since the divergence of system (1.3) is
$\ell_0=\ell_1=0$ and so there are no Darboux first integrals. On the other hand, since the divergence of system (1.3) is  $\frac 1 2 x+ (3 h-1) y$, the solution of
$\frac 1 2 x+ (3 h-1) y$, the solution of
 \begin{equation*}
K(x,y)=\ell_0 \Big(-1-\frac 1 2 x + hy\Big) +\ell_1 (2h-1) y=-\frac 1 2 x- (3 h-1) y
\end{equation*}
\begin{equation*}
K(x,y)=\ell_0 \Big(-1-\frac 1 2 x + hy\Big) +\ell_1 (2h-1) y=-\frac 1 2 x- (3 h-1) y
\end{equation*}is empty, so there are no Liouvillian first integrals in this case. This concludes the proof of proposition 2.3.
3. Proof of theorem 1.1
As already pointed out in §1, system (1.1) has always the invariant algebraic curves y = 0 and ![]() $a+xy=0$ (a hyperbola). Now we look for the existence of more invariant algebraic curves through the Puiseux series method.
$a+xy=0$ (a hyperbola). Now we look for the existence of more invariant algebraic curves through the Puiseux series method.
In view of theorem 4.8 in §4 to obtain the representation of a invariant algebraic curve ![]() $F(x,y)$ in terms of the Puiseux series we write Eq. (1.3) in the form of Eq. (1.2), that is
$F(x,y)$ in terms of the Puiseux series we write Eq. (1.3) in the form of Eq. (1.2), that is
 \begin{equation}
2a h-a+h x y-h x_y y^2+\frac{x^2}{2}+\frac{x x_yy}{2}-x y+x+x_y y=0.
\end{equation}
\begin{equation}
2a h-a+h x y-h x_y y^2+\frac{x^2}{2}+\frac{x x_yy}{2}-x y+x+x_y y=0.
\end{equation} Note that in view of proposition 2.2, we have that ![]() $\mu(y)=y$ and we just need to compute all the Puiseux series in the field
$\mu(y)=y$ and we just need to compute all the Puiseux series in the field ![]() $\mathbb{C}_\infty \{y\}$ that satisfy Eq. (3.1). For this, we need to obtain the dominant balances associated to the Newton polygon of equation (3.1). The Newton polygon associated with Eq. (3.1) is shown in figure 2.
$\mathbb{C}_\infty \{y\}$ that satisfy Eq. (3.1). For this, we need to obtain the dominant balances associated to the Newton polygon of equation (3.1). The Newton polygon associated with Eq. (3.1) is shown in figure 2.
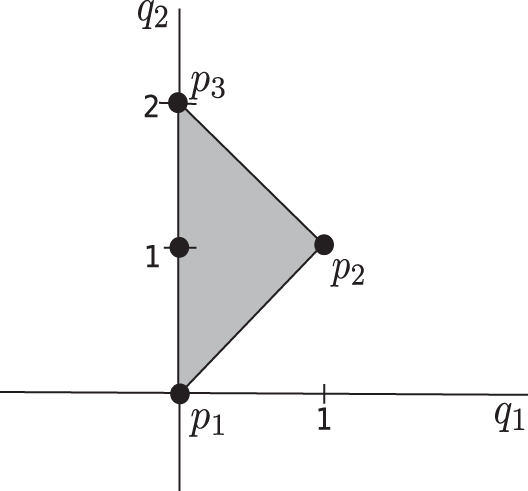
Figure 2. Newton polygon related to Eq. (3.1).
The proof of statement (A) is given in proposition 2.1. From now on in all the article, we assume that ![]() $h \ne \{1/2,1\}$. For Eq. (3.1), there are four dominant balances that can produce Puiseux series in a neighbourhood of
$h \ne \{1/2,1\}$. For Eq. (3.1), there are four dominant balances that can produce Puiseux series in a neighbourhood of ![]() $y=\infty$: two vertices and two edges.
$y=\infty$: two vertices and two edges.
The only vertices that can produce Puiseux series in a neighbourhood of ![]() $y=\infty$ and so that are dominant balances are p 2 and p 3.
$y=\infty$ and so that are dominant balances are p 2 and p 3.
If we consider the vertex p 2, we have that the dominant balance is formed by the monomials ![]() $(h-1)xy$ and
$(h-1)xy$ and ![]() $-hy^2x_y$. Then we look for power solutions of its associated equation, which is of the equation
$-hy^2x_y$. Then we look for power solutions of its associated equation, which is of the equation
 \begin{equation*}
(h-1)xy-hy^2x_y=0.
\end{equation*}
\begin{equation*}
(h-1)xy-hy^2x_y=0.
\end{equation*} This equation has the general solution ![]() $x(y)=cy^{(h-1)/h}$. Note that in order that it generates a Puiseux series we must have
$x(y)=cy^{(h-1)/h}$. Note that in order that it generates a Puiseux series we must have ![]() $h\in \mathbb{Q}$ (for
$h\in \mathbb{Q}$ (for ![]() $h \in \mathbb{R} \setminus \mathbb{Q}$ it does not provide a solution with rational exponent).
$h \in \mathbb{R} \setminus \mathbb{Q}$ it does not provide a solution with rational exponent).
On the other hand, for the vertex p 3, the dominant balance is formed by the monomials  $\frac{1}{2} x^2$ and
$\frac{1}{2} x^2$ and  $\frac{1}{2} xyx_y$ and its associated equation
$\frac{1}{2} xyx_y$ and its associated equation
 \begin{equation*}
\frac{1}{2} x^2+ \frac{1}{2} xyx_y=0
\end{equation*}
\begin{equation*}
\frac{1}{2} x^2+ \frac{1}{2} xyx_y=0
\end{equation*} has the solution ![]() $x(y)=c y^{-1}$.
$x(y)=c y^{-1}$.
Furthermore, the only edges producing Puiseux series in a neighbourhood of infinity (i.e. the unique dominant balances) are the edge ![]() $\overline{p_1p_2}$ and the edge
$\overline{p_1p_2}$ and the edge ![]() $\overline{p_2p_3}$. The balance associated with the edge
$\overline{p_2p_3}$. The balance associated with the edge ![]() $\overline{p_1p_2}$ is
$\overline{p_1p_2}$ is ![]() $a(2h-1)+(h-1)xy-hy^2x_y$ which produces the solution
$a(2h-1)+(h-1)xy-hy^2x_y$ which produces the solution ![]() $x(y)=-ay^{-1}+c y^{(h-1)/h}$ of the equation
$x(y)=-ay^{-1}+c y^{(h-1)/h}$ of the equation
 \begin{equation*}
a(2h-1)+(h-1)xy-hy^2x_y=0.
\end{equation*}
\begin{equation*}
a(2h-1)+(h-1)xy-hy^2x_y=0.
\end{equation*} Then we only have a solution of the required form ![]() $x=b_0y^r$, with
$x=b_0y^r$, with ![]() $b_0\neq 0, $ and
$b_0\neq 0, $ and ![]() $ r\in \mathbb{Q}$ for only c = 0 and in this case the power solution is
$ r\in \mathbb{Q}$ for only c = 0 and in this case the power solution is ![]() $x(y)=-a/y$. Note that
$x(y)=-a/y$. Note that ![]() $(h-1)/h=-1$ implies
$(h-1)/h=-1$ implies ![]() $ h=1/2$, but this case was studied on statement A(ii) of theorem 1.1.
$ h=1/2$, but this case was studied on statement A(ii) of theorem 1.1.
The dominant balance associated with the edge ![]() $\overline{p_2p_3}$ is
$\overline{p_2p_3}$ is  $(-1 + h) y x +\frac{1}{2} x^2 - h y^2 x_y + \frac{1}{2} y x x_y$ which produces the solution
$(-1 + h) y x +\frac{1}{2} x^2 - h y^2 x_y + \frac{1}{2} y x x_y$ which produces the solution ![]() $x(y)=y$ of its associated equation
$x(y)=y$ of its associated equation
 \begin{equation*}
(-1 + h) y x +\frac{1}{2} x^2 - h y^2 x_y + \frac{1}{2} y x x_y=0.
\end{equation*}
\begin{equation*}
(-1 + h) y x +\frac{1}{2} x^2 - h y^2 x_y + \frac{1}{2} y x x_y=0.
\end{equation*}Now we make an analysis of these different cases in order to obtain the corresponding Puiseux series (if it exists). We indicate below for each of the already mentioned vertices and edges of the Newton polygon which form the dominant balances, the associated Fuchs index of each of the power solutions of their associated equations.
In what follows we also indicate whether the compatibility conditions are satisfied and if the Puiseux series are uniquely determined or not.
• The associated equation to the vertex p 3 has the solution
 $x(y)=c y^{-1}$. The Fuchs index is j = 0 and analysing the compatibility conditions we get that there are two possible Puiseux series centred at the point
$x(y)=c y^{-1}$. The Fuchs index is j = 0 and analysing the compatibility conditions we get that there are two possible Puiseux series centred at the point  $y=\infty$.
$y=\infty$.(i) If
 $h=1/n, n\in \mathbb{N}, n\geq 3$, the corresponding Puiseux series is
(3.2)
$h=1/n, n\in \mathbb{N}, n\geq 3$, the corresponding Puiseux series is
(3.2) \begin{align}
x(y) & =\displaystyle\frac{-a}{y}+\displaystyle\frac{A_{n-1}}{y^{n-1}}\left( 1+\displaystyle\frac{n(n-2)A_{n-1}}{y} \right.\notag\\
& \quad \left. + \displaystyle\frac{f_{2n}(a,A_{n-1})}{y^2}+ \displaystyle\frac{f_{3n}(a,A_{n-1})}{y^3}+ \cdots \right),
\end{align}
\begin{align}
x(y) & =\displaystyle\frac{-a}{y}+\displaystyle\frac{A_{n-1}}{y^{n-1}}\left( 1+\displaystyle\frac{n(n-2)A_{n-1}}{y} \right.\notag\\
& \quad \left. + \displaystyle\frac{f_{2n}(a,A_{n-1})}{y^2}+ \displaystyle\frac{f_{3n}(a,A_{n-1})}{y^3}+ \cdots \right),
\end{align}where
 $f_{in}(a,A_{n-1})$,
$f_{in}(a,A_{n-1})$,  $i=2,3,\dots $ is a parameter that depends only on a and
$i=2,3,\dots $ is a parameter that depends only on a and  $A_{n-1}$, being
$A_{n-1}$, being  $A_{n-1} \in \mathbb{R}$. The Puiseux series is not uniquely determined in this case.
$A_{n-1} \in \mathbb{R}$. The Puiseux series is not uniquely determined in this case.(ii) If h is not in the previous form, the corresponding Puiseux series is
(3.3) \begin{equation}
x(y)=\displaystyle\frac{-a}{y}.
\end{equation}
\begin{equation}
x(y)=\displaystyle\frac{-a}{y}.
\end{equation}
• The associated equation to the edge
 $\overline{p_1p_2}$ has the solution
$\overline{p_1p_2}$ has the solution  $x(y)=-a/y$. Here the Fuchs index is
$x(y)=-a/y$. Here the Fuchs index is  $j=(1-2h)/h$. Analysing the compatibility conditions, we get that there are two possible Puiseux series centred at the point
$j=(1-2h)/h$. Analysing the compatibility conditions, we get that there are two possible Puiseux series centred at the point  $y=\infty$.
$y=\infty$.(i) If
 $0 \lt h \lt 1/2$ and
$0 \lt h \lt 1/2$ and  $h=p/q, p\neq 1$ then
$h=p/q, p\neq 1$ then  $j\in \mathbb{Q}^+\setminus \mathbb{N}$ and the Puiseux series is
(3.4)
$j\in \mathbb{Q}^+\setminus \mathbb{N}$ and the Puiseux series is
(3.4) \begin{align}
x(y)& =\displaystyle\frac{-a}{y}+
\displaystyle\frac{A_{p,q}}{y^{(q-p)/p}}\notag\\
& \quad \times \left( 1+\displaystyle\frac{f_{1}(a,A_{p,q})}{y^{1/p}}+ \displaystyle\frac{f_{2}(a,A_{p,q})}{y^{2/p}}+ \displaystyle\frac{f_{3}(a,A_{p,q})}{y^{3/p}}+ \cdots \right),
\end{align}
\begin{align}
x(y)& =\displaystyle\frac{-a}{y}+
\displaystyle\frac{A_{p,q}}{y^{(q-p)/p}}\notag\\
& \quad \times \left( 1+\displaystyle\frac{f_{1}(a,A_{p,q})}{y^{1/p}}+ \displaystyle\frac{f_{2}(a,A_{p,q})}{y^{2/p}}+ \displaystyle\frac{f_{3}(a,A_{p,q})}{y^{3/p}}+ \cdots \right),
\end{align}where
 $f_{i}(a,A_{p,q})$ depends only on a and
$f_{i}(a,A_{p,q})$ depends only on a and  $A_{p,q}$, being
$A_{p,q}$, being  $A_{p,q} \in \mathbb{R}$. The Puiseux series is not uniquely determined in this case.
$A_{p,q} \in \mathbb{R}$. The Puiseux series is not uniquely determined in this case.(ii) If h is not in the previous form, we arrive to (3.3) or to (3.2).
• The associated equation to the edge
 $\overline{p_2p_3}$ has the solution
$\overline{p_2p_3}$ has the solution  $x(y)=y$. The unique Fuchs index is of the form:
$x(y)=y$. The unique Fuchs index is of the form:  $j=2/(1 - 2 h)$. Thus, we consider the Fuchs index only if it is a positive rational number, so a necessary condition is
$j=2/(1 - 2 h)$. Thus, we consider the Fuchs index only if it is a positive rational number, so a necessary condition is  $h \lt 1/2$. There are several Puiseux series in a neighbourhood of infinity.
$h \lt 1/2$. There are several Puiseux series in a neighbourhood of infinity.(i) If
 $h\ne \frac{1}2 -\frac{q}p$ with
$h\ne \frac{1}2 -\frac{q}p$ with  $(p,q)=1$ for any
$(p,q)=1$ for any  $p,q \in \mathbb{Z}$ with pq > 0, the Fuchs index is
$p,q \in \mathbb{Z}$ with pq > 0, the Fuchs index is  $j\neq p/q$. The Puiseux series in (4.3) has the form
(3.5)
$j\neq p/q$. The Puiseux series in (4.3) has the form
(3.5) \begin{equation}
x(y)=y -\frac{4}{2 h+1} -c \left( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \right)
\end{equation}
\begin{equation}
x(y)=y -\frac{4}{2 h+1} -c \left( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \right)
\end{equation}with
(3.6) \begin{equation}
c=\frac{(2h-1)}{2h } \left(a- \frac{4}{(2h+1)^2}\right).
\end{equation}
\begin{equation}
c=\frac{(2h-1)}{2h } \left(a- \frac{4}{(2h+1)^2}\right).
\end{equation}The Puiseux series is uniquely determined.
(ii) If
 $h=\frac{1}2 -\frac{1}p$ with
$h=\frac{1}2 -\frac{1}p$ with  $p \in \mathbb{N}$ (i.e.
$p \in \mathbb{N}$ (i.e.  $h=\frac{p-2}{2p}$, the Fuchs index is
$h=\frac{p-2}{2p}$, the Fuchs index is  $j=p\in \mathbb{N}$). We have different possibilities.
$j=p\in \mathbb{N}$). We have different possibilities.If p = 1 then
 $h=-1/2$, the compatibility conditions are not satisfied and there is no Puiseux series in this case. If p = 2, then h = 0 and it is proved that if
$h=-1/2$, the compatibility conditions are not satisfied and there is no Puiseux series in this case. If p = 2, then h = 0 and it is proved that if  $a \ne 4=4/(2h+1)^2$ (which is the case) the compatibility conditions are not satisfied and there is no Puiseux series.
$a \ne 4=4/(2h+1)^2$ (which is the case) the compatibility conditions are not satisfied and there is no Puiseux series.If p > 2, then we can write
 $h=n/(2n+4)$ with
$h=n/(2n+4)$ with  $n \in \mathbb{N}$. Since
$n \in \mathbb{N}$. Since  $a \ne 4/(2h+1)^2$, there are some particular values of a (that we write
$a \ne 4/(2h+1)^2$, there are some particular values of a (that we write  $\bar a$ in table 1) which depend on n in such a way that if
$\bar a$ in table 1) which depend on n in such a way that if  $a \ne \bar a$ then the compatibility conditions are not satisfied and there is no Puiseux series, and in case that
$a \ne \bar a$ then the compatibility conditions are not satisfied and there is no Puiseux series, and in case that  $a =\bar a$ then there is an infinite number of Puiseux series (depending on some
$a =\bar a$ then there is an infinite number of Puiseux series (depending on some  $b_{n+2} \in \mathbb{R}$) that are of the form
(3.7)
$b_{n+2} \in \mathbb{R}$) that are of the form
(3.7) \begin{equation}
x(y)=y -\frac{4}{2 h+1} -c\left( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+\cdots \right) +
\displaystyle\frac{b_{n+2}}{y^{n+1}}\left(1+\cdots \right)
\end{equation}
\begin{equation}
x(y)=y -\frac{4}{2 h+1} -c\left( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+\cdots \right) +
\displaystyle\frac{b_{n+2}}{y^{n+1}}\left(1+\cdots \right)
\end{equation}with c as in (3.6).
Unfortunately, we do not have a general form for
 $\bar a$ depending on n but in table 1 we summarize the first ten of these values.
$\bar a$ depending on n but in table 1 we summarize the first ten of these values.
Table 1. Some values of
 $\bar{a}$
$\bar{a}$
where
 \begin{equation*}
\begin{array}{cr}
(e_1)& -3507934149 + 2539118016 a + 1890652160 a^2 + 32768000 a^3=0\\
(e_2)& -245120000 - 102999600 a + 356131080 a^2 + 26040609 a^3=0\\
(e_3)& -1963821677983 - 138818972652800 a + 113356300530000 a^2 + \\
& 26395138000000 a^3 + 274400000000 a^4=0\\
(e_4)& 4086726130944 - 13712198664384 a + 4547349639459 a^2 + \\
& 4523567633596 a^3 + 185206073184 a^4=0.\\
\end{array}
\end{equation*}
\begin{equation*}
\begin{array}{cr}
(e_1)& -3507934149 + 2539118016 a + 1890652160 a^2 + 32768000 a^3=0\\
(e_2)& -245120000 - 102999600 a + 356131080 a^2 + 26040609 a^3=0\\
(e_3)& -1963821677983 - 138818972652800 a + 113356300530000 a^2 + \\
& 26395138000000 a^3 + 274400000000 a^4=0\\
(e_4)& 4086726130944 - 13712198664384 a + 4547349639459 a^2 + \\
& 4523567633596 a^3 + 185206073184 a^4=0.\\
\end{array}
\end{equation*}(iii) If
 $h=\frac{1}2 -\frac{q}p$ with
$h=\frac{1}2 -\frac{q}p$ with  $(p,q)=1$,
$(p,q)=1$,  $q \ne 1$,
$q \ne 1$,  $p,q \in \mathbb{Z}$ with pq > 0, Fuchs index
$p,q \in \mathbb{Z}$ with pq > 0, Fuchs index  $j=p/q$, there is an infinite number of Puiseux series whose expressions depend on the values of q and p and they are very complicated (note that if
$j=p/q$, there is an infinite number of Puiseux series whose expressions depend on the values of q and p and they are very complicated (note that if  $q/p \lt 1$ then
$q/p \lt 1$ then  $j=p/q\in \mathbb{Q}^+\setminus \mathbb{N}$ but if
$j=p/q\in \mathbb{Q}^+\setminus \mathbb{N}$ but if  $q/p \gt 1$ then
$q/p \gt 1$ then  $j =p/q$ do not necessarily belong to
$j =p/q$ do not necessarily belong to  $\mathbb{Q}^+\setminus \mathbb{N}$, and so these expressions can be completely different depending on whether
$\mathbb{Q}^+\setminus \mathbb{N}$, and so these expressions can be completely different depending on whether  $q/p \gt 1$ or
$q/p \gt 1$ or  $q/p \lt 1$). Although particular cases with fixed values of p and q could be analysed, we cannot address the general situation because of the complexity and so it is out of the scope of the article.
$q/p \lt 1$). Although particular cases with fixed values of p and q could be analysed, we cannot address the general situation because of the complexity and so it is out of the scope of the article.
• The associated equation to the dominant balance given by the vertex p 2 has the solution
 $x(y)=cy^{(h-1)/h}$. So, if either h = 0 or
$x(y)=cy^{(h-1)/h}$. So, if either h = 0 or  $h \not \in \mathbb{Q}$ the solution x(y) is not of the required form
$h \not \in \mathbb{Q}$ the solution x(y) is not of the required form  $b_0 y^r$ with
$b_0 y^r$ with  $b_0 \ne 0$ and
$b_0 \ne 0$ and  $r \in \mathbb{Q}$ and so there are no Puiseux series associated with this vertex in these cases. For
$r \in \mathbb{Q}$ and so there are no Puiseux series associated with this vertex in these cases. For  $h\in \mathbb{Q}$, the related Fuchs index is j = 0, and the nature of h determines the nature of
$h\in \mathbb{Q}$, the related Fuchs index is j = 0, and the nature of h determines the nature of  $r=(h-1)/h$.
$r=(h-1)/h$.(i) If
 $h=n\in \mathbb{N}$, with n > 1, there is an infinite number of Puiseux series of the form
(3.8)
$h=n\in \mathbb{N}$, with n > 1, there is an infinite number of Puiseux series of the form
(3.8) \begin{equation}
x (y)=A_0 y^{(n-1)/n} -\frac{2n-1}{2n} A_0^2 y^{(n-2)/n}+ \cdots,
\end{equation}
\begin{equation}
x (y)=A_0 y^{(n-1)/n} -\frac{2n-1}{2n} A_0^2 y^{(n-2)/n}+ \cdots,
\end{equation}where
 $A_0 \in \mathbb{R}$. So, the Puiseux series is not uniquely determined.
$A_0 \in \mathbb{R}$. So, the Puiseux series is not uniquely determined.(ii) If
 $h=p/q$ with
$h=p/q$ with  $(p,q)=1$,
$(p,q)=1$,  $p \ne 1$,
$p \ne 1$,  $q \ne 1$ and h > 1 then we have that there is an infinite number of Puiseux series of the form
(3.9)
$q \ne 1$ and h > 1 then we have that there is an infinite number of Puiseux series of the form
(3.9) \begin{equation}
x(y)=A_0y^{(p-q)/p}+c_{1,p,q} A_0^2y^{(p-2q)/p}+\cdots
\end{equation}
\begin{equation}
x(y)=A_0y^{(p-q)/p}+c_{1,p,q} A_0^2y^{(p-2q)/p}+\cdots
\end{equation}where
 $c_{1,p,q} \ne 0$ and
$c_{1,p,q} \ne 0$ and  $A_0 \in \mathbb{R}$. Again the Puiseux series is not uniquely determined. We provide some values of c 1 for different
$A_0 \in \mathbb{R}$. Again the Puiseux series is not uniquely determined. We provide some values of c 1 for different  $h=p/q$.
$h=p/q$.
 \begin{equation*}
c_{1,p,q}=\begin{cases}
-2/3, & p=3, \quad q=2, \\
-4/5, & p=5, \quad q=2, \\
-5/8, & p=4, \quad q=3, \\
-7/10, & p=5, \quad q=3,...
\end{cases}
\end{equation*}
\begin{equation*}
c_{1,p,q}=\begin{cases}
-2/3, & p=3, \quad q=2, \\
-4/5, & p=5, \quad q=2, \\
-5/8, & p=4, \quad q=3, \\
-7/10, & p=5, \quad q=3,...
\end{cases}
\end{equation*}(iii) If
 $h=p/q$ with
$h=p/q$ with  $(p,q)=1$,
$(p,q)=1$,  $p \ne 1$,
$p \ne 1$,  $q \ne 1$ and
$q \ne 1$ and  $1/2 \lt h \lt 1$ then with
$1/2 \lt h \lt 1$ then with  $r=(p-q)/p$ we have an infinite number of Puiseux series of the form
(3.10)
$r=(p-q)/p$ we have an infinite number of Puiseux series of the form
(3.10) \begin{equation}
x(y)=A_0y^{1-q/p}-a y^{-1} -c_{2,p,q} A_0 y^{-q/p} -c_{3,p,q} A_0^2 y^{(p-2q)/p} +\cdots,
\end{equation}
\begin{equation}
x(y)=A_0y^{1-q/p}-a y^{-1} -c_{2,p,q} A_0 y^{-q/p} -c_{3,p,q} A_0^2 y^{(p-2q)/p} +\cdots,
\end{equation}where
 $c_{2,p,q},c_{3,p,q} \gt 0$ and
$c_{2,p,q},c_{3,p,q} \gt 0$ and  $A_0 \in \mathbb{R}$. Again the Puiseux series is not uniquely determined. We provide some values of
$A_0 \in \mathbb{R}$. Again the Puiseux series is not uniquely determined. We provide some values of  $c_{3,p,q}$ for different
$c_{3,p,q}$ for different  $h=p/q$.
$h=p/q$.
 \begin{equation*}
c_{3,p,q}=\begin{cases}
1/6, & p=3, \quad q=5, \\
1/8, & p=4, \quad q=7, \\
1/5, & p=5, \quad q=8, \\
3/8, & p=4, \quad q=5, \\
1/3, & p=3, \quad q=4, \\
1/4, & p=2, \quad q=3.
\end{cases}
\end{equation*}
\begin{equation*}
c_{3,p,q}=\begin{cases}
1/6, & p=3, \quad q=5, \\
1/8, & p=4, \quad q=7, \\
1/5, & p=5, \quad q=8, \\
3/8, & p=4, \quad q=5, \\
1/3, & p=3, \quad q=4, \\
1/4, & p=2, \quad q=3.
\end{cases}
\end{equation*}(iv) If
 $h=p/q$ with
$h=p/q$ with  $0 \lt h \lt 1/2$, the compatibility conditions are not satisfied and there is no Puiseux series in a neighbourhood of the infinity. Note that in particular in this case, we have
$0 \lt h \lt 1/2$, the compatibility conditions are not satisfied and there is no Puiseux series in a neighbourhood of the infinity. Note that in particular in this case, we have  $h=1/n$ with
$h=1/n$ with  $n \ge 3$ and
$n \ge 3$ and  $h=n/(2n+4)$ with
$h=n/(2n+4)$ with  $n \ge 0$.
$n \ge 0$.(a) If h < 0, the compatibility conditions are not satisfied and there is no Puiseux series in a neighbourhood of the infinity possessing the solution
 $x(y)=cy^{(h-1)/h}$ as the highest-order term.
$x(y)=cy^{(h-1)/h}$ as the highest-order term.
We first illustrate the proof of statement (A.1) using Puiseux series.
Proposition 3.1. Assume that a satisfies ![]() $a =4/(1+2h)^2$. In this case, system (1.3) has the invariant algebraic curves y = 0,
$a =4/(1+2h)^2$. In this case, system (1.3) has the invariant algebraic curves y = 0, ![]() $a +xy=0$ and
$a +xy=0$ and ![]() $x-y+4/(1+2h)=0$.
$x-y+4/(1+2h)=0$.
Proof. First note that y = 0, ![]() $a+xy=0$ and
$a+xy=0$ and ![]() $x-y+4/(1+2h)=0$ are invariant algebraic curves for system (1.3) when
$x-y+4/(1+2h)=0$ are invariant algebraic curves for system (1.3) when ![]() $a =4/(1+2h)^2$ with
$a =4/(1+2h)^2$ with ![]() $h \ne -1/2$. We will obtain them from the analysis of the Puiseux series and in particular choosing for some vertices and edges the simplest form of the Puiseux series.
$h \ne -1/2$. We will obtain them from the analysis of the Puiseux series and in particular choosing for some vertices and edges the simplest form of the Puiseux series.
From the vertex p 3, we get that if ![]() $h\neq 1/n$ the Puiseux series is
$h\neq 1/n$ the Puiseux series is ![]() $x(y)=-a/y$ (see (3.3)). On the other hand, if
$x(y)=-a/y$ (see (3.3)). On the other hand, if ![]() $h=1/n$ the Puiseux series is as in (3.2), where
$h=1/n$ the Puiseux series is as in (3.2), where ![]() $A_{n-1} \in \mathbb{R}$ is arbitrary and for the particular case
$A_{n-1} \in \mathbb{R}$ is arbitrary and for the particular case ![]() $A_{n-1}=0$ we get again
$A_{n-1}=0$ we get again ![]() $x(y)=-a/y$. In particular from the vertex p 3, we can always have the Puiseux series
$x(y)=-a/y$. In particular from the vertex p 3, we can always have the Puiseux series ![]() $x(y)=-a/y$.
$x(y)=-a/y$.
From the edge ![]() $p_2p_3$, we have:
$p_2p_3$, we have:
• for
 $h\neq 1/2-q/p$ the Puiseux series is
$h\neq 1/2-q/p$ the Puiseux series is  $x(y)=y-4/(1+2h)$ (see (3.5)).
$x(y)=y-4/(1+2h)$ (see (3.5)).• for
 $h=1/2-q/p$, q ≠ 1, pq > 0,
$h=1/2-q/p$, q ≠ 1, pq > 0,  $p,q\in \mathbb{Z}$ the Puiseux series takes the form
(3.11)
$p,q\in \mathbb{Z}$ the Puiseux series takes the form
(3.11) \begin{equation}
x(y)=y+A_p y^{q_1}+k_1A_py^{q_2}+(-4/(1+2h)+nA_p)+\displaystyle\frac{f_1(A_p)}{y^{1/q}}+\cdots
\end{equation}
\begin{equation}
x(y)=y+A_p y^{q_1}+k_1A_py^{q_2}+(-4/(1+2h)+nA_p)+\displaystyle\frac{f_1(A_p)}{y^{1/q}}+\cdots
\end{equation}where
 $A_p \in \mathbb{R}$ and in the particular case
$A_p \in \mathbb{R}$ and in the particular case  $A_p=0$, we get that
$A_p=0$, we get that  $f_1(0)=0$ and
$f_1(0)=0$ and  $x(y)=y-4/(1+2h)$.
$x(y)=y-4/(1+2h)$.• for
 $h=1/2-1/p$,
$h=1/2-1/p$,  $p\in \mathbb{N}$,
$p\in \mathbb{N}$,  $p\geq 2$ (note that since
$p\geq 2$ (note that since  $a=4/(1+2h)^2$ we have that
$a=4/(1+2h)^2$ we have that  $h \ne -1/2$ and so
$h \ne -1/2$ and so  $p \ne 1$) and in this case the Puiseux series is of the form
$p \ne 1$) and in this case the Puiseux series is of the form
 \begin{equation*}x(y)=y-\displaystyle\frac{4}{1+2h}+\displaystyle\frac{A_p}{y^{p-1}}\left( 1+\displaystyle\frac{n_1 A_p}{y}+\displaystyle\frac{n_2 A_p}{y^2}+\displaystyle\frac{n_3 A_p}{y^3}+\cdots\right),\end{equation*}
\begin{equation*}x(y)=y-\displaystyle\frac{4}{1+2h}+\displaystyle\frac{A_p}{y^{p-1}}\left( 1+\displaystyle\frac{n_1 A_p}{y}+\displaystyle\frac{n_2 A_p}{y^2}+\displaystyle\frac{n_3 A_p}{y^3}+\cdots\right),\end{equation*}where
 $n_i\in \mathbb{N}$,
$n_i\in \mathbb{N}$,  $A_p \in \mathbb{R}$ and in the particular case
$A_p \in \mathbb{R}$ and in the particular case  $A_p=0$ we get
$A_p=0$ we get  $x(y)=y-4/(1+2h)$.
$x(y)=y-4/(1+2h)$.
Note that in particular for the edge ![]() $p_2p_3$, we can always have the Puiseux series
$p_2p_3$, we can always have the Puiseux series ![]() $x(y)=y-4/(1+2h)$.
$x(y)=y-4/(1+2h)$.
It is not necessary to consider the edge ![]() $p_1p_2$ or the vertex p 3 since the Puiseux series that we have obtained are sufficient to form the invariant algebraic curves that will lead to the Liouvillian integrability.
$p_1p_2$ or the vertex p 3 since the Puiseux series that we have obtained are sufficient to form the invariant algebraic curves that will lead to the Liouvillian integrability.
It follows from theorem 4.8 (see §4) and the explanation above that ![]() $F(x,y)$ can have the form
$F(x,y)$ can have the form
 \begin{equation*}F(x,y)=y^{l_1}\left(x+\frac{a}{y}\right)^{l_2}\left(x-y+\frac{4}{1+2h}\right)^{l_3},\end{equation*}
\begin{equation*}F(x,y)=y^{l_1}\left(x+\frac{a}{y}\right)^{l_2}\left(x-y+\frac{4}{1+2h}\right)^{l_3},\end{equation*} with ![]() $l_1,\:l_2,\:l_3\in \{0,1\}$. Since y = 0,
$l_1,\:l_2,\:l_3\in \{0,1\}$. Since y = 0, ![]() $a+xy=0$ and
$a+xy=0$ and ![]() $x-y+4/(1+2h)=0$ are invariant algebraic curves, combining the possibilities for the purpose of getting a first integral for the values of
$x-y+4/(1+2h)=0$ are invariant algebraic curves, combining the possibilities for the purpose of getting a first integral for the values of ![]() $l_1,l_2,l_3$ and taking into account that the invariant algebraic curves are irreducible polynomials, the proposition follows.
$l_1,l_2,l_3$ and taking into account that the invariant algebraic curves are irreducible polynomials, the proposition follows.
Now we make a detailed study of the different cases that we separate into different propositions. From now on, we assume that ![]() $a \ne 4/(1+2h)^2$. This condition will be assumed in the next seven propositions.
$a \ne 4/(1+2h)^2$. This condition will be assumed in the next seven propositions.
Proposition 3.2. Assume that ![]() $h \in \mathbb{R} \setminus \mathbb{Q}$. In this case, the unique invariant algebraic curves of system (1.3) are y and a + xy.
$h \in \mathbb{R} \setminus \mathbb{Q}$. In this case, the unique invariant algebraic curves of system (1.3) are y and a + xy.
Proof. It follows from (3.3), (3.5), theorem 4.8 and the explanation above that ![]() $F(x,y)$ is equal to
$F(x,y)$ is equal to
 \begin{equation*}
\left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right\}_+,
\end{equation*}
\begin{equation*}
\left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right\}_+,
\end{equation*} with ![]() $\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Note that the maximum degree in x is two and the maximum degree of y is two, then F is at most a polynomial of degree three of the form
$\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Note that the maximum degree in x is two and the maximum degree of y is two, then F is at most a polynomial of degree three of the form
with ![]() $\alpha_i \in \mathbb{C}$. Taking into account that the cofactor is of the form
$\alpha_i \in \mathbb{C}$. Taking into account that the cofactor is of the form ![]() $K(x,y)=\beta_0+ \beta_1 x +\beta_2 y$ with
$K(x,y)=\beta_0+ \beta_1 x +\beta_2 y$ with ![]() $\beta_i \in \mathbb{C}$, and imposing that F is an invariant algebraic curve we get that the unique irreducible invariant algebraic curves are y, a + xy and
$\beta_i \in \mathbb{C}$, and imposing that F is an invariant algebraic curve we get that the unique irreducible invariant algebraic curves are y, a + xy and  $\frac{4}{1+2h} + x -y$ whenever
$\frac{4}{1+2h} + x -y$ whenever ![]() $a=4/(1+2h)^2$. Taking into account that we are assuming that
$a=4/(1+2h)^2$. Taking into account that we are assuming that ![]() $a \ne 4/(1+2h)^2$ and using proposition 2.3 we conclude that system (1.3) in this case is not Liouville integrable.
$a \ne 4/(1+2h)^2$ and using proposition 2.3 we conclude that system (1.3) in this case is not Liouville integrable.
Proposition 3.3. Assume that ![]() $h \gt 1/2$ with
$h \gt 1/2$ with ![]() $h \in \mathbb{Q}\setminus\{1,2\}$. In this case, the unique invariant algebraic curves of system (1.3) are y and a + xy.
$h \in \mathbb{Q}\setminus\{1,2\}$. In this case, the unique invariant algebraic curves of system (1.3) are y and a + xy.
Proof. We consider different cases.
Assume first that ![]() $h=n \in \mathbb{N}$ with
$h=n \in \mathbb{N}$ with ![]() $n\geq 3$. Then it follows from (3.3), (3.5), (3.8), theorem 4.8 and the explanation above that
$n\geq 3$. Then it follows from (3.3), (3.5), (3.8), theorem 4.8 and the explanation above that ![]() $F(x,y)$ is equal to
$F(x,y)$ is equal to
 \begin{equation*}
\begin{aligned}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right.\\
& \phantom{\le\le} \left.\prod_{i=1}^{m} \Big\{x-A_{0,i} y^{1-1/n} +\frac{2n-1}{2n} (A_{0,i}^2 y^{1-2/n}+ \cdots \Big\}\right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right.\\
& \phantom{\le\le} \left.\prod_{i=1}^{m} \Big\{x-A_{0,i} y^{1-1/n} +\frac{2n-1}{2n} (A_{0,i}^2 y^{1-2/n}+ \cdots \Big\}\right\}_+,
\end{aligned}
\end{equation*} with ![]() $\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Note that
$\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Note that ![]() $\ell_1 =1$ since otherwise
$\ell_1 =1$ since otherwise ![]() $F(x,y)$ can never be a polynomial.
$F(x,y)$ can never be a polynomial.
Moreover, we note that ![]() $2-2/n \ne 1/m$ for any
$2-2/n \ne 1/m$ for any ![]() $m \in \mathbb{N}$ whenever n > 2. Therefore, for n > 2, computing the terms of the form
$m \in \mathbb{N}$ whenever n > 2. Therefore, for n > 2, computing the terms of the form ![]() $x^{m+\ell_2+\ell_3-1}y^{2-2/n}$ we get that their sum is of the form
$x^{m+\ell_2+\ell_3-1}y^{2-2/n}$ we get that their sum is of the form
 \begin{equation*}
\frac{2n-1}{2n} \sum_{i=1}^m A_{0,i}^2 =0,
\end{equation*}
\begin{equation*}
\frac{2n-1}{2n} \sum_{i=1}^m A_{0,i}^2 =0,
\end{equation*} which yields ![]() $A_{0,i}=0$. Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
$A_{0,i}=0$. Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
Assume now that ![]() $h=p/q$ with
$h=p/q$ with ![]() $(p,q)=1$,
$(p,q)=1$, ![]() $p \ne 1$,
$p \ne 1$, ![]() $q \ne 1$ and h > 1 (so p > q) then it follows from (3.3), (3.5), (3.9), theorem 4.8 and the explanation above that
$q \ne 1$ and h > 1 (so p > q) then it follows from (3.3), (3.5), (3.9), theorem 4.8 and the explanation above that ![]() $F(x,y)$ is equal to
$F(x,y)$ is equal to
 \begin{equation*}
\begin{aligned}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right.\\
& \phantom{\le\le} \left.\prod_{i=1}^{m} \Big\{x-A_0^{(i)}y^{1-q/p}-c_{1,p,q} (A_0^{(i)})^2 y^{1-2q/p}+\cdots \Big\}\right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right.\\
& \phantom{\le\le} \left.\prod_{i=1}^{m} \Big\{x-A_0^{(i)}y^{1-q/p}-c_{1,p,q} (A_0^{(i)})^2 y^{1-2q/p}+\cdots \Big\}\right\}_+,
\end{aligned}
\end{equation*} with ![]() $\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Again,
$\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Again, ![]() $\ell_1 =1$ since otherwise
$\ell_1 =1$ since otherwise ![]() $F(x,y)$ can never be a polynomial. Note that
$F(x,y)$ can never be a polynomial. Note that ![]() $1-2q/p \ne -m$ with
$1-2q/p \ne -m$ with ![]() $m \in \mathbb{N}$ for any
$m \in \mathbb{N}$ for any ![]() $p \ne 1$,
$p \ne 1$, ![]() $q \ne 1$ with p > q. Therefore, computing the terms of the form
$q \ne 1$ with p > q. Therefore, computing the terms of the form ![]() $x^{m+\ell_2+\ell_3-1}\:y^{2-2q/p}$, we get that it is of the form
$x^{m+\ell_2+\ell_3-1}\:y^{2-2q/p}$, we get that it is of the form
 \begin{equation*}
-c_{1,p,q} \sum_{i=1}^m (A_0^{(i)} )^2 =0,
\end{equation*}
\begin{equation*}
-c_{1,p,q} \sum_{i=1}^m (A_0^{(i)} )^2 =0,
\end{equation*} which yields  $A_0^{(i)}=0$ (because
$A_0^{(i)}=0$ (because ![]() $c_{1,p,q} \ne 0$). Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
$c_{1,p,q} \ne 0$). Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
Assume finally that ![]() $h=p/q$ with
$h=p/q$ with ![]() $(p,q)=1$,
$(p,q)=1$, ![]() $p \ne 1$,
$p \ne 1$, ![]() $q \ne 1$ and
$q \ne 1$ and ![]() $1/2 \lt h \lt 1$ with
$1/2 \lt h \lt 1$ with ![]() $h \ne 2/3$. Then it follows from (3.3), (3.5), (3.10), theorem 4.8 and the explanation above that
$h \ne 2/3$. Then it follows from (3.3), (3.5), (3.10), theorem 4.8 and the explanation above that ![]() $F(x,y)$ is equal to
$F(x,y)$ is equal to
 \begin{align*}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right.\\
& \!\!\phantom{\le\le} \left.\prod_{i=1}^{m} \Big\{x-A_{0,i} y^{(p-q)/p}-a y^{-1} -c_{2,p,q} A_{0,i} y^{-q/p} -c_{3,p,q} A_{0,i}^2 y^{(p-2q)/p} +\cdots,\}\right\}_+,
\end{align*}
\begin{align*}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \left(x- y +\frac{4}{2 h+1} +c \Big( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+ \cdots \Big)\right)^{\ell_3}\right.\\
& \!\!\phantom{\le\le} \left.\prod_{i=1}^{m} \Big\{x-A_{0,i} y^{(p-q)/p}-a y^{-1} -c_{2,p,q} A_{0,i} y^{-q/p} -c_{3,p,q} A_{0,i}^2 y^{(p-2q)/p} +\cdots,\}\right\}_+,
\end{align*} with ![]() $\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Again,
$\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Again, ![]() $\ell_1 =1$ since otherwise
$\ell_1 =1$ since otherwise ![]() $F(x,y)$ can never be a polynomial. Note that
$F(x,y)$ can never be a polynomial. Note that ![]() $1+(p-2q)/p \ne -m$ with
$1+(p-2q)/p \ne -m$ with ![]() $m \in \mathbb{N}\setminus \{1\}$ for any
$m \in \mathbb{N}\setminus \{1\}$ for any ![]() $p \ne 1$,
$p \ne 1$, ![]() $q \ne 1$ with
$q \ne 1$ with ![]() $p,q)=1$,
$p,q)=1$, ![]() $p \lt q \lt 2p$. Therefore, for
$p \lt q \lt 2p$. Therefore, for ![]() $h \ne 2/3$, computing the terms of the form
$h \ne 2/3$, computing the terms of the form ![]() $x^{m+\ell_2+\ell_3-1}/y^{1+(p-2q)/p}$ we get that it is of the form
$x^{m+\ell_2+\ell_3-1}/y^{1+(p-2q)/p}$ we get that it is of the form
 \begin{equation*}
-c_{3,p,q} \sum_{i=1}^m A_{0,i}^2 =0,
\end{equation*}
\begin{equation*}
-c_{3,p,q} \sum_{i=1}^m A_{0,i}^2 =0,
\end{equation*} which yields ![]() $A_{0,i}=0$ (because
$A_{0,i}=0$ (because ![]() $c_{2,p,q} \ne 0$ because
$c_{2,p,q} \ne 0$ because ![]() $c_{3,p,q} \ne 0$). Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
$c_{3,p,q} \ne 0$). Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
Finally, we study the case ![]() $h=2/3$. It follows from (3.3), (3.5), (3.10), theorem 4.8 and the explanation above that
$h=2/3$. It follows from (3.3), (3.5), (3.10), theorem 4.8 and the explanation above that ![]() $F(x,y)$ is equal to (we have only written for the vertex p 2 the powers of the form y −n with
$F(x,y)$ is equal to (we have only written for the vertex p 2 the powers of the form y −n with ![]() $n \in \mathbb{N}$. Note that there are also terms with negative fractional powers in y but since they will not be used in the argument of the proof we have not written them.)
$n \in \mathbb{N}$. Note that there are also terms with negative fractional powers in y but since they will not be used in the argument of the proof we have not written them.)
 \begin{equation*}
\begin{aligned}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \Big(x- y +\frac{12}7 -\frac{36-49 a}{196 y} -\frac{36-49 a}{343 y^2}-\frac{3(49a-36)}{1715 y^3} \right.\\
& \quad\!\! + \frac{3 (245a-1524)(49a-36)}{3697540 y^4} \phantom{\le} + \frac{(833a-1908)(49a-36)} {1848770 y^5} +\cdots\Big)^{\ell_3}\\
& \quad\!\! \times \prod_{i=1}^{m} \Big\{x+ \frac{a}{y} -\frac{A_{0,i}^2}{4 y^2} -\frac{3 A_{0,i}^2}{20 y^3} + \frac{3 (35 a- 48)A_{0,i}^2}{1120 y^4} \left. \phantom{\le } + \frac{(49a-36) A_{0,i}^22}{280 y^5} +\cdots\Big\} \right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
& \left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \Big(x- y +\frac{12}7 -\frac{36-49 a}{196 y} -\frac{36-49 a}{343 y^2}-\frac{3(49a-36)}{1715 y^3} \right.\\
& \quad\!\! + \frac{3 (245a-1524)(49a-36)}{3697540 y^4} \phantom{\le} + \frac{(833a-1908)(49a-36)} {1848770 y^5} +\cdots\Big)^{\ell_3}\\
& \quad\!\! \times \prod_{i=1}^{m} \Big\{x+ \frac{a}{y} -\frac{A_{0,i}^2}{4 y^2} -\frac{3 A_{0,i}^2}{20 y^3} + \frac{3 (35 a- 48)A_{0,i}^2}{1120 y^4} \left. \phantom{\le } + \frac{(49a-36) A_{0,i}^22}{280 y^5} +\cdots\Big\} \right\}_+,
\end{aligned}
\end{equation*} with ![]() $\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Note that
$\ell_1,\ell_2, \ell_3 \in \{0,1\}$. Note that ![]() $\ell_1=1$ and
$\ell_1=1$ and ![]() $\ell_3=1$ (otherwise we clearly reach a contradiction).
$\ell_3=1$ (otherwise we clearly reach a contradiction).
Computing the terms of the form ![]() $x^{m+\ell_2}/y$, we get that their sum is of the form
$x^{m+\ell_2}/y$, we get that their sum is of the form
 \begin{equation*}
-\frac 1 4 \sum_{i=1}^m A_{0,i}^2 -\frac{36-49 a}{343} =0,
\end{equation*}
\begin{equation*}
-\frac 1 4 \sum_{i=1}^m A_{0,i}^2 -\frac{36-49 a}{343} =0,
\end{equation*} which yields  $\sum_{i=1}^m A_{0,i}^2 =-\frac{4 (36-49a)}{343}$.
$\sum_{i=1}^m A_{0,i}^2 =-\frac{4 (36-49a)}{343}$.
Computing the terms of the form ![]() $x^{m+\ell_2}/y^{3}$, we get that their sum is of the form
$x^{m+\ell_2}/y^{3}$, we get that their sum is of the form
 \begin{equation*}
\frac{3(35a-48)}{1120}\sum_{i=1}^m A_{0,i}^2 + \frac{3 (245 a -1524)(49 a -36)}{3697540} =0,
\end{equation*}
\begin{equation*}
\frac{3(35a-48)}{1120}\sum_{i=1}^m A_{0,i}^2 + \frac{3 (245 a -1524)(49 a -36)}{3697540} =0,
\end{equation*} and computing the terms of the form ![]() $x^{m+\ell_2}/y^{4}$ we get that their sum is of the form
$x^{m+\ell_2}/y^{4}$ we get that their sum is of the form
 \begin{equation*}
\frac{49a-36}{280}\sum_{i=1}^m A_{0,i}^2 + \frac{(833a-1908)(49a-36)}{1848770} =0,
\end{equation*}
\begin{equation*}
\frac{49a-36}{280}\sum_{i=1}^m A_{0,i}^2 + \frac{(833a-1908)(49a-36)}{1848770} =0,
\end{equation*} which yields  $\sum_{i=1}^m A_{0,i}^2 =0$. Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
$\sum_{i=1}^m A_{0,i}^2 =0$. Now proceeding exactly as in the proof of proposition 3.2, we conclude that system (1.3) in this case is not Liouville integrable.
Proposition 3.4. System (1.3) is not Liouvillian integrable for h = 0 and ![]() $a \ne 4$.
$a \ne 4$.
Proof. It follows from the explanation above that if h = 0 and ![]() $a \ne 4$, the unique Puiseux series is
$a \ne 4$, the unique Puiseux series is ![]() $x-a/y$. Therefore, using theorem 4.8, we obtain
$x-a/y$. Therefore, using theorem 4.8, we obtain
 \begin{equation*}
F(x,y)=y^{\ell_1} \left(x+\frac{a}{y} \right)^{\ell_2},
\end{equation*}
\begin{equation*}
F(x,y)=y^{\ell_1} \left(x+\frac{a}{y} \right)^{\ell_2},
\end{equation*} with ![]() $\ell_1,\ell_2\in \{0,1\}$. Clearly, the unique irreducible invariant algebraic curves are y, a + xy and using proposition 2.3 we conclude that system (1.3) in this case is not Liouville integrable.
$\ell_1,\ell_2\in \{0,1\}$. Clearly, the unique irreducible invariant algebraic curves are y, a + xy and using proposition 2.3 we conclude that system (1.3) in this case is not Liouville integrable.
Proposition 3.5. System (1.3) is not Liouvillian integrable for ![]() $h =n/(2n+4)$ with
$h =n/(2n+4)$ with ![]() $n \ne 1,2,4$.
$n \ne 1,2,4$.
Proof. Note that ![]() $n/(2n+4)=1/m$ if and only if
$n/(2n+4)=1/m$ if and only if ![]() $m=4/n+2$. Moreover,
$m=4/n+2$. Moreover, ![]() $m=4/n+2$ is an integer number if and only if
$m=4/n+2$ is an integer number if and only if ![]() $n=1,2,4$. Since we are assuming
$n=1,2,4$. Since we are assuming ![]() $n \ne 1,2,4$ it follows from (3.3), (3.7), theorem 4.8 and the explanation above that if
$n \ne 1,2,4$ it follows from (3.3), (3.7), theorem 4.8 and the explanation above that if ![]() $a \ne \bar a$ then
$a \ne \bar a$ then
 \begin{equation*}
F(x,y) =y^{\ell_1} \Big(x+
\frac{a}{y} \Big)^{\ell_2},
\end{equation*}
\begin{equation*}
F(x,y) =y^{\ell_1} \Big(x+
\frac{a}{y} \Big)^{\ell_2},
\end{equation*} where ![]() $\ell_1,\ell_2\in \{0,1\}$, and if
$\ell_1,\ell_2\in \{0,1\}$, and if ![]() $a =\bar a$ then
$a =\bar a$ then
 \begin{equation*}
\begin{aligned}
F(x,y) & =\left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \prod_{i=1}^{m} \left\{ x-y +\frac{4}{2 h+1}\right.\right.\\
& \quad \left.\left.+c\left( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+\cdots \right) +
\displaystyle\frac{b_{n+2,i}}{y^{n+1}}\Big(1+\cdots \Big)
\right\}^{\ell_3} \right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
F(x,y) & =\left\{y^{\ell_1} \Big(x+ \frac{a}{y} \Big)^{\ell_2} \prod_{i=1}^{m} \left\{ x-y +\frac{4}{2 h+1}\right.\right.\\
& \quad \left.\left.+c\left( \frac{1}{y}
+\frac{1}{(2h+1)(6h-1) }\frac{1}{y^2}
+\cdots \right) +
\displaystyle\frac{b_{n+2,i}}{y^{n+1}}\Big(1+\cdots \Big)
\right\}^{\ell_3} \right\}_+,
\end{aligned}
\end{equation*} where ![]() $\ell_1,\ell_2, \ell_3\in \{0,1\}$ and c was given in (3.6).
$\ell_1,\ell_2, \ell_3\in \{0,1\}$ and c was given in (3.6).
If ![]() $a \ne \bar a$ then the unique invariant curves are y and a + xy and it follows from proposition 2.3 that system (1.3) in this case is not Liouville integrable.
$a \ne \bar a$ then the unique invariant curves are y and a + xy and it follows from proposition 2.3 that system (1.3) in this case is not Liouville integrable.
Assume now that ![]() $a =\bar a$. If
$a =\bar a$. If ![]() $\ell_3=0$ the result is clear, so we may assume that
$\ell_3=0$ the result is clear, so we may assume that ![]() $\ell_3=1$. Note that if
$\ell_3=1$. Note that if ![]() $\ell_1=1$ and
$\ell_1=1$ and ![]() $\ell_2=1$ then the term of the form
$\ell_2=1$ then the term of the form ![]() $x^m /y$ is of the form
$x^m /y$ is of the form
 \begin{equation}
m c \frac{1 }{(2h+1)(6h-1) }= m \left(a- \frac{4}{(2h+1)^2}\right) \frac{(2h-1)}{2h(2h+1)(6h-1) }
=0.
\end{equation}
\begin{equation}
m c \frac{1 }{(2h+1)(6h-1) }= m \left(a- \frac{4}{(2h+1)^2}\right) \frac{(2h-1)}{2h(2h+1)(6h-1) }
=0.
\end{equation} Since it has to be zero, we must have  $a=\frac{4}{(1+2h)^2}$, which is not possible.
$a=\frac{4}{(1+2h)^2}$, which is not possible.
On the other hand, if ![]() $\ell_1=1$ and
$\ell_1=1$ and ![]() $\ell_2=0$ then the term of the form
$\ell_2=0$ then the term of the form ![]() $x^{m-1}/y$ is of the form also as in (3.12), which is again not possible. On the other hand, if
$x^{m-1}/y$ is of the form also as in (3.12), which is again not possible. On the other hand, if ![]() $\ell_1=0$ and
$\ell_1=0$ and ![]() $\ell_2 =1$ then the term of the form
$\ell_2 =1$ then the term of the form ![]() $x^m /y$ is of the form
$x^m /y$ is of the form
 \begin{equation*}
mc = m \left(a- \frac{4}{(2h+1)^2}\right) \frac{(2h-1)}{2h } =0,
\end{equation*}
\begin{equation*}
mc = m \left(a- \frac{4}{(2h+1)^2}\right) \frac{(2h-1)}{2h } =0,
\end{equation*} which is again not possible, because ![]() $a \ne 4/(2h+1)^2$. This concludes the proof of the because using proposition 2.3 we conclude that system (1.3) in this case is not Liouville integrable.
$a \ne 4/(2h+1)^2$. This concludes the proof of the because using proposition 2.3 we conclude that system (1.3) in this case is not Liouville integrable.
Proposition 3.6. System (1.3) is not Liouvillian integrable for ![]() $h =1/4$ and
$h =1/4$ and ![]() $a \ne 16/9$.
$a \ne 16/9$.
Proof. In this case it follows from (3.2), (3.7), theorem 4.8 and the explanation above that when ![]() $a \ne 16/9$ we get
$a \ne 16/9$ we get
 \begin{equation*}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \prod_{j=1}^{k} \left\{x+\frac{a}{y}-\displaystyle\frac{A_{3,j}}{y^{3}} - \displaystyle\frac{A^2_{3,j}}{y^{7}} -\displaystyle\frac{A^3_{3,j}}{y^{11}}+ \cdots
\right\}^{\ell_2}\right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \prod_{j=1}^{k} \left\{x+\frac{a}{y}-\displaystyle\frac{A_{3,j}}{y^{3}} - \displaystyle\frac{A^2_{3,j}}{y^{7}} -\displaystyle\frac{A^3_{3,j}}{y^{11}}+ \cdots
\right\}^{\ell_2}\right\}_+,
\end{aligned}
\end{equation*} with ![]() $\ell_1,\ell_2 \in \{0,1\}$ (recall that in this case
$\ell_1,\ell_2 \in \{0,1\}$ (recall that in this case ![]() $\bar a = 16/9 = 4/(1+2h)^2$, and so
$\bar a = 16/9 = 4/(1+2h)^2$, and so ![]() $a \ne \bar a$). Computing the terms with
$a \ne \bar a$). Computing the terms with ![]() $x^{k\ell_2 -2}{y^{-10-\ell_1}}$ we get
$x^{k\ell_2 -2}{y^{-10-\ell_1}}$ we get
 \begin{equation*}
\sum_{j=1}^{k\ell_2} A_{3,j}^2 =0,
\end{equation*}
\begin{equation*}
\sum_{j=1}^{k\ell_2} A_{3,j}^2 =0,
\end{equation*} and so ![]() $A_{3,j}=0$ for
$A_{3,j}=0$ for ![]() $j=1,\ldots,k$. In short, we obtain
$j=1,\ldots,k$. In short, we obtain
 \begin{equation}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \left\{x+\frac{a}{y}
\right\}^{\ell_2}\right\}_+,
\end{aligned}
\end{equation}
\begin{equation}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \left\{x+\frac{a}{y}
\right\}^{\ell_2}\right\}_+,
\end{aligned}
\end{equation} with ![]() $\ell_1,\ell_2 \in \{0,1\}$ and proceeding as in the proof of proposition 3.2 we get that system (1.3) in this case is not Liouville integrable.
$\ell_1,\ell_2 \in \{0,1\}$ and proceeding as in the proof of proposition 3.2 we get that system (1.3) in this case is not Liouville integrable.
Proposition 3.7. Assume that ![]() $h =1/3$ and
$h =1/3$ and ![]() $a \neq \pm 36/25$. In this case, the system is not Liouvillian integrable.
$a \neq \pm 36/25$. In this case, the system is not Liouvillian integrable.
Proof. Assume that ![]() $a \ne -36/25$. In this case, it follows from (3.2), (3.7) (and the explanation above (3.7)) together with theorem 4.8 and also the explanation above in the article that
$a \ne -36/25$. In this case, it follows from (3.2), (3.7) (and the explanation above (3.7)) together with theorem 4.8 and also the explanation above in the article that
 \begin{equation*}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \Big(x+\frac{a}{y} \Big)^{\ell_2} \prod_{j=1}^{k} \left\{x+ \frac a y -\displaystyle\frac{A_{2,j}}{y^{2}}\Big(1+\displaystyle\frac{2}{y}+ \cdots \Big)
\right\}^{\ell_3} \right\}_+ \\
&= \left\{y^{\ell_1} \Big(x+\frac{a}{y} \Big)^{\ell_2} \prod_{j=1}^{k} \left\{x+ \frac a y -\displaystyle\frac{A_{2,j}}{y^{2}} -\frac{3 A_{2,j}}{y^3} - \frac{3(a-12)A_{2,j}}{4 y^4} \right. \right. \\
& \quad \left. \left.-
\frac{(108-21a) A_{2,j} + 2 A_{2,j}^2}{4 y^5} + \cdots
\right\}^{\ell_3} \right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \Big(x+\frac{a}{y} \Big)^{\ell_2} \prod_{j=1}^{k} \left\{x+ \frac a y -\displaystyle\frac{A_{2,j}}{y^{2}}\Big(1+\displaystyle\frac{2}{y}+ \cdots \Big)
\right\}^{\ell_3} \right\}_+ \\
&= \left\{y^{\ell_1} \Big(x+\frac{a}{y} \Big)^{\ell_2} \prod_{j=1}^{k} \left\{x+ \frac a y -\displaystyle\frac{A_{2,j}}{y^{2}} -\frac{3 A_{2,j}}{y^3} - \frac{3(a-12)A_{2,j}}{4 y^4} \right. \right. \\
& \quad \left. \left.-
\frac{(108-21a) A_{2,j} + 2 A_{2,j}^2}{4 y^5} + \cdots
\right\}^{\ell_3} \right\}_+,
\end{aligned}
\end{equation*} with ![]() $\ell_1,\ell_2,\ell_3 \in \{0,1\}$. Clearly, if
$\ell_1,\ell_2,\ell_3 \in \{0,1\}$. Clearly, if ![]() $\ell_3 =0$ we are done. So we can assume that
$\ell_3 =0$ we are done. So we can assume that ![]() $\ell_3=1$. Moreover, if
$\ell_3=1$. Moreover, if ![]() $\ell_1=0$ then it is obvious that system (1.3) is not Liouville integrable. So we have
$\ell_1=0$ then it is obvious that system (1.3) is not Liouville integrable. So we have ![]() $\ell_1=\ell_3=1$. Moreover, computing the terms with
$\ell_1=\ell_3=1$. Moreover, computing the terms with ![]() $x^{k+\ell_2-1 }/y$, we obtain
$x^{k+\ell_2-1 }/y$, we obtain
 \begin{equation*}
\sum_{j=1}^k A_{2,j}=0,
\end{equation*}
\begin{equation*}
\sum_{j=1}^k A_{2,j}=0,
\end{equation*} and computing the terms with ![]() $x^{k+\ell_2-1 }/y^4$ we obtain
$x^{k+\ell_2-1 }/y^4$ we obtain
 \begin{equation*}
\sum_{j=1}^k A_{2,j}^2=0,
\end{equation*}
\begin{equation*}
\sum_{j=1}^k A_{2,j}^2=0,
\end{equation*} which yields ![]() $A_{2,j}=0$ for
$A_{2,j}=0$ for ![]() $j=1,\ldots, k$. Now, proceeding as in the proof of proposition 3.6, we get that in this case system (1.3) is not Liouville integrable.
$j=1,\ldots, k$. Now, proceeding as in the proof of proposition 3.6, we get that in this case system (1.3) is not Liouville integrable.
Proposition 3.8. System (1.3) is not Liouvillian integrable for ![]() $h =1/6$ and
$h =1/6$ and ![]() $a \ne 9/4$.
$a \ne 9/4$.
Proof. In this case, it follows from (3.2), (3.7), theorem 4.8 and the explanation above that
 \begin{equation*}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \prod_{j=1}^{k} \left\{x+\frac{a}{y}-\displaystyle\frac{A_{5,j}}{y^{5}} - \displaystyle\frac{A^2_{5,j}}{y^{11}} -\displaystyle\frac{A^3_{5,j}}{y^{17}}+ \cdots
\right\}^{\ell_2}\right\}_+,
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
F(x,y)&=\left\{y^{\ell_1} \prod_{j=1}^{k} \left\{x+\frac{a}{y}-\displaystyle\frac{A_{5,j}}{y^{5}} - \displaystyle\frac{A^2_{5,j}}{y^{11}} -\displaystyle\frac{A^3_{5,j}}{y^{17}}+ \cdots
\right\}^{\ell_2}\right\}_+,
\end{aligned}
\end{equation*} with ![]() $\ell_1,\ell_2\in \{0,1\}$ (note that in this case
$\ell_1,\ell_2\in \{0,1\}$ (note that in this case ![]() $\bar a =9/4=4/(1+2h)^2$ and so
$\bar a =9/4=4/(1+2h)^2$ and so ![]() $a \ne \bar a$). Computing the terms with
$a \ne \bar a$). Computing the terms with ![]() $x^{k\ell_3 -2} y^{-13-\ell_1}$, we get
$x^{k\ell_3 -2} y^{-13-\ell_1}$, we get
 \begin{equation*}
\sum_{j=1}^{k\ell_2} A_{5,j}^2 =0,\end{equation*}
\begin{equation*}
\sum_{j=1}^{k\ell_2} A_{5,j}^2 =0,\end{equation*} and so ![]() $A_{5,j}=0$ for
$A_{5,j}=0$ for ![]() $j=1,\ldots,k$. In short, we get
$j=1,\ldots,k$. In short, we get ![]() $F(x,y)$ as in (3.13) and proceeding as in the proof of proposition 3.2 we get that system (1.3) in this case is not Liouville integrable.
$F(x,y)$ as in (3.13) and proceeding as in the proof of proposition 3.2 we get that system (1.3) in this case is not Liouville integrable.
Proof. Proof of theorem 1.1
The proof of theorem 1.1 statement B follows from propositions 3.2–3.8.
4. Appendix: Irreducible invariant algebraic curves and Puiseux series
We give some definitions and results that appeared in the article.
4.1. Irreducible invariant algebraic curves
An algebraic curve ![]() $C(x, y) = 0$ with
$C(x, y) = 0$ with ![]() $C(x, y) \in \mathbb{C}[x, y]$ is called an invariant algebraic curve of system (1.1) if it satisfies the following identity:
$C(x, y) \in \mathbb{C}[x, y]$ is called an invariant algebraic curve of system (1.1) if it satisfies the following identity:
for some ![]() $K \in \mathbb{C}[x, y]$, called the cofactor of the curve C = 0. For simplicity, we write the curve C instead of the curve C = 0 in
$K \in \mathbb{C}[x, y]$, called the cofactor of the curve C = 0. For simplicity, we write the curve C instead of the curve C = 0 in ![]() $\mathbb{C}^2$. Note that if system (1.1) has degree m then the cofactor of the invariant algebraic curve C of system (1.1) has degree m − 1. When we look for a complex algebraic curve of a real polynomial system, we are thinking of the complexification of the real polynomial system.
$\mathbb{C}^2$. Note that if system (1.1) has degree m then the cofactor of the invariant algebraic curve C of system (1.1) has degree m − 1. When we look for a complex algebraic curve of a real polynomial system, we are thinking of the complexification of the real polynomial system.
Proposition 4.1. [Reference Dumortier, Llibre and Artes10]
For a real polynomial system (1.1), C is a complex invariant algebraic curve with cofactor K if and only if ![]() $\overline C$ is also an invariant algebraic curve with cofactor
$\overline C$ is also an invariant algebraic curve with cofactor ![]() $\overline{K}$.
$\overline{K}$.
Proposition 4.2. [Reference Dumortier, Llibre and Artes10]
If C = 0 is an invariant algebraic curve in ![]() $\mathbb{C}^n$ and
$\mathbb{C}^n$ and ![]() $C = C_1^{n_1} C_2^{n_2} \cdots C_s^{n_s}$ with Ci irreducible over
$C = C_1^{n_1} C_2^{n_2} \cdots C_s^{n_s}$ with Ci irreducible over ![]() $\mathbb{C}$ and
$\mathbb{C}$ and ![]() $n_i \in \mathbb{N}$, then
$n_i \in \mathbb{N}$, then ![]() $C_i=0$ is an irreducible invariant algebraic curve.
$C_i=0$ is an irreducible invariant algebraic curve.
We say that f = 0 is an algebraic solution of (1.1) if it is an invariant algebraic curve and f is an irreducible polynomial over ![]() $\mathbb{C}$.
$\mathbb{C}$.
A function of the form ![]() $f(x,y)=\exp \big(g(x,y)/h(x,y)\big)$ with f and g being bivariate polynomials and
$f(x,y)=\exp \big(g(x,y)/h(x,y)\big)$ with f and g being bivariate polynomials and ![]() $g,h \in \mathbb{C}[x,y]$ coprime is called an exponential factor of system (1.1) if there exists a function
$g,h \in \mathbb{C}[x,y]$ coprime is called an exponential factor of system (1.1) if there exists a function ![]() $L \in \mathbb{C}[x,y]$ (called also the cofactor) of degree at most m − 1 with m the degree of system (1.1) that satisfies the equality
$L \in \mathbb{C}[x,y]$ (called also the cofactor) of degree at most m − 1 with m the degree of system (1.1) that satisfies the equality
for some ![]() $L \in \mathbb{C}[x,y]$ called the cofactor of the exponential factor. We observe that in the definition of exponential factor, the exponential factor is a complex function. Again when we look for a complex exponential factor of a real polynomial system, we are thinking of the complexification of the real polynomial system.
$L \in \mathbb{C}[x,y]$ called the cofactor of the exponential factor. We observe that in the definition of exponential factor, the exponential factor is a complex function. Again when we look for a complex exponential factor of a real polynomial system, we are thinking of the complexification of the real polynomial system.
Proposition 4.3. [Reference Christopher, Llibre and Pereira5]
If ![]() $f=\exp(g/h)$ is an exponential factor of system (1.1) with cofactor L then h = 0 is an invariant algebraic curve of the system with cofactor Kh. The polynomials Kh and g satisfy the equation
$f=\exp(g/h)$ is an exponential factor of system (1.1) with cofactor L then h = 0 is an invariant algebraic curve of the system with cofactor Kh. The polynomials Kh and g satisfy the equation
where ![]() $g,h,L, K_h \in \mathbb{C}[x,y]$.
$g,h,L, K_h \in \mathbb{C}[x,y]$.
Proposition 4.4. [Reference Dumortier, Llibre and Artes10]
For a real system (1.1), the function ![]() $f=\exp(g/h)$ is an exponential factor with cofactor K if and only if the function
$f=\exp(g/h)$ is an exponential factor with cofactor K if and only if the function ![]() $\overline {f}$ is an exponential factor with cofactor
$\overline {f}$ is an exponential factor with cofactor ![]() $\overline{K}$.
$\overline{K}$.
A non-constant function ![]() $H \colon U \subset \mathbb{R}^2 \to \mathbb{R}$ is called a first integral of system (1.1) in the open set U if this function is constant on each solution
$H \colon U \subset \mathbb{R}^2 \to \mathbb{R}$ is called a first integral of system (1.1) in the open set U if this function is constant on each solution ![]() $(x(t),y(t))$ of system (1.1) contained in U. In fact,
$(x(t),y(t))$ of system (1.1) contained in U. In fact, ![]() $H \in C^1(U)$ is a first integral of system (1.1) if and only if
$H \in C^1(U)$ is a first integral of system (1.1) if and only if
Consider the divergence of system (1.1) which is defined as ![]() $\text{div} \, (P,Q)=P_x + Q_y$. Let U be an open subset of
$\text{div} \, (P,Q)=P_x + Q_y$. Let U be an open subset of ![]() $\mathbb{R}^2$ and
$\mathbb{R}^2$ and ![]() $R \colon U \to \mathbb{R}$ be an analytic function which is not identically zero on U. A non-constant function
$R \colon U \to \mathbb{R}$ be an analytic function which is not identically zero on U. A non-constant function ![]() $R \colon U \subset \mathbb{R}^2 \to \mathbb{R}$ is called an integrating factor of system (1.1) on U if
$R \colon U \subset \mathbb{R}^2 \to \mathbb{R}$ is called an integrating factor of system (1.1) on U if
The integrating factor R is associated with a first integral H when ![]() $HP =-H_y$ and
$HP =-H_y$ and ![]() $HQ=H_x$. Moreover,
$HQ=H_x$. Moreover, ![]() $V=1/R$ is called an inverse integrating factor in
$V=1/R$ is called an inverse integrating factor in ![]() $U \setminus \{R=0\}$.
$U \setminus \{R=0\}$.
If system (1.1) has a first integral of the form
 \begin{equation}
H(x,y)=C_1^{\lambda_1} \cdots C_p^{\lambda_p} f_1^{\mu_1} \cdots f_q^{\mu_q}
\end{equation}
\begin{equation}
H(x,y)=C_1^{\lambda_1} \cdots C_p^{\lambda_p} f_1^{\mu_1} \cdots f_q^{\mu_q}
\end{equation} where Ci and Fj are invariant algebraic curves and exponential factors of system (1.1), respectively, and ![]() $\lambda_i, \mu_j \in \mathbb{C}$, then we say that system (1.1) is generalized Darboux integrable and we call the function H a generalized Darboux function.
$\lambda_i, \mu_j \in \mathbb{C}$, then we say that system (1.1) is generalized Darboux integrable and we call the function H a generalized Darboux function.
Liouvillian functions are functions that are built up from rational functions using exponentiation, integration, and algebraic functions, or equivalently, a class of functions which are obtainable ‘by quadratures’. A first integral which is a Liouvillian function is said to be a Liouvillian first integral. We refer the reader to [Reference Christopher and Llibre4] where it is given the definition of Liouvillian functions through differential algebra.
Theorem 4.5 [Reference Singer21]
A system (1.1) it has a Liouvillian first integral if and only if has a generalized Darboux integrating factor.
Therefore, the method of Darboux finds all Liouvillian first integrals. It is important to mention that a polynomial differential system (1.1) that is Liouvillian integrable does not always have an affine invariant algebraic curve. In [Reference Gine and Llibre11], there is an example of such a polynomial differential system.
4.2. Puiseux series
Given the differential system (1.1), we will use the Puiseux series in order to study its invariant algebraic curves. In this sense, we follow the method developed in [Reference Demina7] (see also [Reference Demina8], [Reference Demina and Kudryashov9]) related with Puiseux series near infinite points, to analyse the maximum degree of all irreducible invariant algebraic curves and to obtain them. Although this method is not ours, we summarize it here for the sake of clarity.
Let ![]() $\mathbb{C}_{\infty}\{y\}$ be the set of formal series in fractional powers in the variable y centred at the point
$\mathbb{C}_{\infty}\{y\}$ be the set of formal series in fractional powers in the variable y centred at the point ![]() $\infty$ and possessing coefficients in
$\infty$ and possessing coefficients in ![]() $\mathbb{C}$. These series are called Puiseux series.
$\mathbb{C}$. These series are called Puiseux series.
More precisely, a Puiseux series in a neighbourhood of the point ![]() $y=\infty$ is given by
$y=\infty$ is given by
 \begin{equation}
x(y)=\sum_{l=0}^{+\infty}c_ly^{\frac{l_0-l}{n_0}},
\end{equation}
\begin{equation}
x(y)=\sum_{l=0}^{+\infty}c_ly^{\frac{l_0-l}{n_0}},
\end{equation} where ![]() $l_0\in\mathbb{Z}$ and
$l_0\in\mathbb{Z}$ and ![]() $n_0\in\mathbb{N}$.
$n_0\in\mathbb{N}$.
We privilege the variable x with respect to the variable y and consider x as a function of the variable y. The implicit function theorem can be used to establish that a Puiseux series of the form (4.3) satisfying the equation ![]() $F(x,y)=0$, where
$F(x,y)=0$, where ![]() $F (x,y)$ is an element of the ring
$F (x,y)$ is an element of the ring ![]() $\mathbb{C}[x,y]$, is convergent in a neighbourhood of the point
$\mathbb{C}[x,y]$, is convergent in a neighbourhood of the point ![]() $y=\infty$ (the point
$y=\infty$ (the point ![]() $y=\infty$ is excluded from domain of convergence whenever
$y=\infty$ is excluded from domain of convergence whenever ![]() $l_0 \gt 0$). If
$l_0 \gt 0$). If ![]() $n_0 \gt 1$ then the convergence of the corresponding series is understood in the sense that a certain branch of the n 0th root is chosen and a cut forbidding going around the branch point is introduced.
$n_0 \gt 1$ then the convergence of the corresponding series is understood in the sense that a certain branch of the n 0th root is chosen and a cut forbidding going around the branch point is introduced.
The construction of Puiseux series solving Eq. (1.2) can be performed with the following algorithm considering the Newton polygon related to Eq. (1.2). For this, we consider Eq. (1.2) that we write it as ![]() $E(x,y,x_y)=0$, and we shall consider
$E(x,y,x_y)=0$, and we shall consider ![]() $E(x,y,x_y)$ as the sum of differential monomials given by
$E(x,y,x_y)$ as the sum of differential monomials given by
 \begin{equation}
M[x(y),y]=m_0x^{j_0}y^l\left\{\frac{d x}{dy}\right\}^{j_1},\quad m_0\in\mathbb{C}\setminus\{0\},\quad l,j_0,j_1\in\mathbb{N}_0.
\end{equation}
\begin{equation}
M[x(y),y]=m_0x^{j_0}y^l\left\{\frac{d x}{dy}\right\}^{j_1},\quad m_0\in\mathbb{C}\setminus\{0\},\quad l,j_0,j_1\in\mathbb{N}_0.
\end{equation} The set of all the differential monomials of the form (4.4) will be referred to as ![]() $\mathbb{M}$.
$\mathbb{M}$.
Define the map ![]() $q:$
$q:$ ![]() $\mathbb{M} \rightarrow \mathbb{R}^2$ by the following rules
$\mathbb{M} \rightarrow \mathbb{R}^2$ by the following rules
 \begin{equation*}
m_0x^{q_1}y^{q_2} \mapsto q=(q_2,q_1), \qquad
\frac{d^ky}{dx^k} \mapsto q=(-k,1),\quad q(M_1M_2)=q(M_1)+q(M_2),
\end{equation*}
\begin{equation*}
m_0x^{q_1}y^{q_2} \mapsto q=(q_2,q_1), \qquad
\frac{d^ky}{dx^k} \mapsto q=(-k,1),\quad q(M_1M_2)=q(M_1)+q(M_2),
\end{equation*} where ![]() $m_0\in \mathbb{C}\setminus\{0\}$ is a constant, M 1 and M 2 are differential monomials. We denote the set of all points
$m_0\in \mathbb{C}\setminus\{0\}$ is a constant, M 1 and M 2 are differential monomials. We denote the set of all points ![]() $p\in \mathbb{R}^2$ corresponding to the monomials of Eq. (1.2) as S(E). The convex hull of S(E) is called the Newton polygon equation (1.2). The name of Newton polygon is due to its similarity in construction and purpose as the Newton polygon constructed for obtaining the leading order terms of the solutions of
$p\in \mathbb{R}^2$ corresponding to the monomials of Eq. (1.2) as S(E). The convex hull of S(E) is called the Newton polygon equation (1.2). The name of Newton polygon is due to its similarity in construction and purpose as the Newton polygon constructed for obtaining the leading order terms of the solutions of ![]() $f(x,y)=0$ (a polynomial over the complex field) but in our case for
$f(x,y)=0$ (a polynomial over the complex field) but in our case for ![]() $E(x,y,x_y)=0$. The boundary of the Newton polygon consists of vertices and edges. Selecting all the differential monomials of the original equation that generate the vertices and the edges of the Newton polygon, we obtain a number of balances. The balance for a vertex is defined as the sum of those differential monomials in
$E(x,y,x_y)=0$. The boundary of the Newton polygon consists of vertices and edges. Selecting all the differential monomials of the original equation that generate the vertices and the edges of the Newton polygon, we obtain a number of balances. The balance for a vertex is defined as the sum of those differential monomials in ![]() $E(x,y,x_y)$ that are mapped into the vertex by q. The balance for an edge is defined as the sum of differential monomials in
$E(x,y,x_y)$ that are mapped into the vertex by q. The balance for an edge is defined as the sum of differential monomials in ![]() $E(x,y,x_y)$ whose images belong to the edge. We also need to introduce the following definition of dominant balance which is very important in our study.
$E(x,y,x_y)$ whose images belong to the edge. We also need to introduce the following definition of dominant balance which is very important in our study.
Definition 4.6. We say that an algebraic ordinary differential equation ![]() $E(x,y,x_y)=0$ has a dominant balance
$E(x,y,x_y)=0$ has a dominant balance ![]() $E_0[x(y),y]$ (where again this expression denotes a polynomial in
$E_0[x(y),y]$ (where again this expression denotes a polynomial in ![]() $x(y),y$ and the derivatives of x(y)), related to the point
$x(y),y$ and the derivatives of x(y)), related to the point ![]() $y=\infty$ if the following conditions are satisfied:
$y=\infty$ if the following conditions are satisfied:
• each differential monomial
 $M[x(y),y]$ appearing in
$M[x(y),y]$ appearing in  $E_0[x(y),y]$ is also involved in the original equation
$E_0[x(y),y]$ is also involved in the original equation  $E(x,y,x_y)=0$;
$E(x,y,x_y)=0$;• there exists a function
 $x(y)=b_0 y^r$ with
$x(y)=b_0 y^r$ with  $b_0\neq 0, r\in \mathbb{C}$ such that all the monomials
$b_0\neq 0, r\in \mathbb{C}$ such that all the monomials  $M[x(y),y]$ of
$M[x(y),y]$ of  $E_0[x(y),y]$ have the same exponent
$E_0[x(y),y]$ have the same exponent  $s\in \mathbb{C}$ in the relation
$s\in \mathbb{C}$ in the relation  $M[b_0y^r,y]=C_my^s$;
$M[b_0y^r,y]=C_my^s$;• for all the monomials
 $M[x(y),y]$ of equation
$M[x(y),y]$ of equation  $E(x,y,x_y)=0$ that are not involved in
$E(x,y,x_y)=0$ that are not involved in  $E_0[x(y),y]$ we obtain
$E_0[x(y),y]$ we obtain  $M[b_0y^r,y]=C_M y^{P_M}$, for some
$M[b_0y^r,y]=C_M y^{P_M}$, for some  $C_M \in \mathbb{C}$, where
$C_M \in \mathbb{C}$, where  $Re \: P_M \lt Re \: s$.
$Re \: P_M \lt Re \: s$.
As pointed out before with the Newton polygon, we can identify the balances selecting all the different monomials that generate its vertices and edges. Among them, we obtain the dominant balances ![]() $E_0[x(y),y]$ and obtain power solutions
$E_0[x(y),y]$ and obtain power solutions ![]() $x(y)=b_0y^r$, where
$x(y)=b_0y^r$, where ![]() $b_0\neq 0$,
$b_0\neq 0$, ![]() $r\in \mathbb{Q}$ of the equation
$r\in \mathbb{Q}$ of the equation ![]() $E_0[x(y),y]=0$ that we will call the associated equation to the dominant balance
$E_0[x(y),y]=0$ that we will call the associated equation to the dominant balance ![]() $E_0[x(y),y]$.
$E_0[x(y),y]$.
Since we are actually in ![]() $\mathbb{R}^2$, we consider the origin
$\mathbb{R}^2$, we consider the origin ![]() $(q_1,q_2) = (0,0)$ and for an edge of the Newton polygon we denote ψ the angle between its external normal and the unit vector
$(q_1,q_2) = (0,0)$ and for an edge of the Newton polygon we denote ψ the angle between its external normal and the unit vector  $\overrightarrow{e_{q_1}}$ directed along the axis q 1. On the other hand, for a vertex of the Newton polygon, we consider the angle ψ between the vector
$\overrightarrow{e_{q_1}}$ directed along the axis q 1. On the other hand, for a vertex of the Newton polygon, we consider the angle ψ between the vector ![]() $\delta (\mbox{Re } r,1)$ and
$\delta (\mbox{Re } r,1)$ and  $\overrightarrow{e_{q_1}}$, where r is the exponent in
$\overrightarrow{e_{q_1}}$, where r is the exponent in ![]() $x=c \: y^r$, and
$x=c \: y^r$, and ![]() $\delta=\pm 1$ is such that
$\delta=\pm 1$ is such that ![]() $\delta(\mbox{Re } r,1)$ lies in the region bounded by the rays associated with the external normals of the edges attached to the vertex. The dominant balance is related to the point
$\delta(\mbox{Re } r,1)$ lies in the region bounded by the rays associated with the external normals of the edges attached to the vertex. The dominant balance is related to the point ![]() $y=\infty$ if
$y=\infty$ if ![]() $0\leq \psi \leq \pi/2$. Whenever the Newton polygon degenerates to an edge or a vertex it is necessary to consider both normals (for the edge) and both vectors
$0\leq \psi \leq \pi/2$. Whenever the Newton polygon degenerates to an edge or a vertex it is necessary to consider both normals (for the edge) and both vectors ![]() $ \pm(\mbox{Re } r,1) $ (for the vertex) (see [Reference Bruno1, Reference Bruno2, Reference Demina and Kudryashov9] for more details).
$ \pm(\mbox{Re } r,1) $ (for the vertex) (see [Reference Bruno1, Reference Bruno2, Reference Demina and Kudryashov9] for more details).
To find the Puiseux series, we have to calculate the Gâteaux derivative of the dominant balance ![]() $E_0[x(y),y]$ at the solution
$E_0[x(y),y]$ at the solution ![]() $x(y)=b_0y^r$ of its associated equation in order to compute the Fuchs index of the dominant balance
$x(y)=b_0y^r$ of its associated equation in order to compute the Fuchs index of the dominant balance ![]() $E_0[x(y),y]$. The Gâteaux derivative of the dominant balance
$E_0[x(y),y]$. The Gâteaux derivative of the dominant balance ![]() $E_0[x(y),y]$ at the solution
$E_0[x(y),y]$ at the solution ![]() $x(y)=b_0y^r$ is
$x(y)=b_0y^r$ is
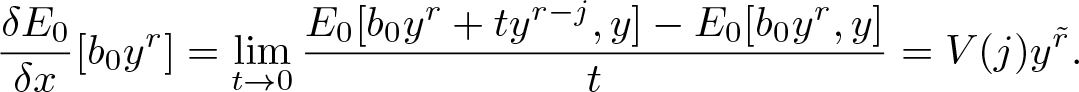 \begin{equation}
\frac{\delta E_0}{\delta x}[b_0y^r]=\displaystyle\lim_{t\to 0} \displaystyle\frac{E_0[b_0y^r+ty^{r-j},y]-E_0[b_0y^r,y]}{t}=V(j)y^{\tilde{r}}.
\end{equation}
\begin{equation}
\frac{\delta E_0}{\delta x}[b_0y^r]=\displaystyle\lim_{t\to 0} \displaystyle\frac{E_0[b_0y^r+ty^{r-j},y]-E_0[b_0y^r,y]}{t}=V(j)y^{\tilde{r}}.
\end{equation} Here V(j) is a first-degree polynomial in the variable j. Recall that we consider first-order ordinary differential equations. The coefficients of the polynomial V(j) depend on b 0 and the parameters (if any) of the original equation involved in the dominant balance ![]() $E_0[y(x),x]$. The zeros j 0 of V(j) are called the Fuchs indices of the dominant balance
$E_0[y(x),x]$. The zeros j 0 of V(j) are called the Fuchs indices of the dominant balance ![]() $E_0[y(x),x]$ and of the power solution
$E_0[y(x),x]$ and of the power solution ![]() $x(y)=b_0y^r$ of its associated equation. The Fuchs indices in
$x(y)=b_0y^r$ of its associated equation. The Fuchs indices in ![]() $\mathbb{Q}^+$ take relevance on the construction of the Puiseux series. Let
$\mathbb{Q}^+$ take relevance on the construction of the Puiseux series. Let ![]() $\text{lcm} (n,m)$ be the lowest common multiple of two integer numbers n and m. If the Fuchs index j 0 is not a positive rational number, then the number n 0 in the expression of the Puiseux series (4.3) is given by
$\text{lcm} (n,m)$ be the lowest common multiple of two integer numbers n and m. If the Fuchs index j 0 is not a positive rational number, then the number n 0 in the expression of the Puiseux series (4.3) is given by ![]() $n_0=r_2$ where r 2 is defined as
$n_0=r_2$ where r 2 is defined as ![]() $r=r_1/r_2$ with r 1 and r 2 being coprime numbers,
$r=r_1/r_2$ with r 1 and r 2 being coprime numbers, ![]() $r_1 \in \mathbb{Z}$ and
$r_1 \in \mathbb{Z}$ and ![]() $r_2 \in \mathbb{N}$. Otherwise, if the Fuchs index is a positive rational number
$r_2 \in \mathbb{N}$. Otherwise, if the Fuchs index is a positive rational number ![]() $j_0=j_1/j_2 $, we obtain
$j_0=j_1/j_2 $, we obtain ![]() $n_0 =\text{lcm}(j_2,r_2)$ where r 2 was defined previously and
$n_0 =\text{lcm}(j_2,r_2)$ where r 2 was defined previously and ![]() $j_0=j_1/j_2$ with coprime natural numbers j 1 and j 2.
$j_0=j_1/j_2$ with coprime natural numbers j 1 and j 2.
Finally, it is important to verify the existence of the Puiseux series of the form (4.3) with ![]() $l_0=rn_0$. If the dominant balance
$l_0=rn_0$. If the dominant balance ![]() $E_0[x(y),y]$ corresponds to a vertex of the Newton polygon, then the Puiseux series always exists and possesses an arbitrary coefficient c 0. In this case, the Fuchs index is equal to zero. Now let us suppose that the dominant balance
$E_0[x(y),y]$ corresponds to a vertex of the Newton polygon, then the Puiseux series always exists and possesses an arbitrary coefficient c 0. In this case, the Fuchs index is equal to zero. Now let us suppose that the dominant balance ![]() $E_0[x(y),y]$ corresponds to an edge of the Newton polygon. Substituting series (4.3) into the equation
$E_0[x(y),y]$ corresponds to an edge of the Newton polygon. Substituting series (4.3) into the equation ![]() $E(x,y,x_y)=0$, one can find the recurrence relation for its coefficients. This relation takes the form
$E(x,y,x_y)=0$, one can find the recurrence relation for its coefficients. This relation takes the form
 \begin{equation*}
H\left(\frac{k}{n_0}\right)c_k=R_k(c_0,\ldots,c_{k-1}),\quad k\in\mathbb{N},
\end{equation*}
\begin{equation*}
H\left(\frac{k}{n_0}\right)c_k=R_k(c_0,\ldots,c_{k-1}),\quad k\in\mathbb{N},
\end{equation*} where Rk is a polynomial of its arguments. Note that Rk can also depend on the parameters (if any) of the original equation. The equation ![]() $R_{n_0j_0}=0$ is called the compatibility condition. If the compatibility condition is not satisfied, then the Puiseux series under consideration does not exist. Otherwise, the corresponding Puiseux series exists and possesses an arbitrary coefficient
$R_{n_0j_0}=0$ is called the compatibility condition. If the compatibility condition is not satisfied, then the Puiseux series under consideration does not exist. Otherwise, the corresponding Puiseux series exists and possesses an arbitrary coefficient ![]() $c_{n_0j_{0}}$ and so we have infinitely many Puiseux series. Consequently, we conclude that the Puiseux series in question has uniquely determined coefficients provided that there are no non-negative rational Fuchs indices.
$c_{n_0j_{0}}$ and so we have infinitely many Puiseux series. Consequently, we conclude that the Puiseux series in question has uniquely determined coefficients provided that there are no non-negative rational Fuchs indices.
In [Reference Demina8], it was established the relation between Puiseux series and a irreducible invariant algebraic curve. The results are the following:
Theorem 4.7 Let ![]() $F(x,y)\in \mathbb{C}[x,y] \setminus \mathbb{C}$,
$F(x,y)\in \mathbb{C}[x,y] \setminus \mathbb{C}$, ![]() $F_x\not \equiv 0$ be an irreducible invariant algebraic curve of the polynomial vector field
$F_x\not \equiv 0$ be an irreducible invariant algebraic curve of the polynomial vector field ![]() $\mathcal{X}$ and its related differential system (1.1). Then
$\mathcal{X}$ and its related differential system (1.1). Then ![]() $F(x,y)$ takes the form
$F(x,y)$ takes the form
 \begin{equation}
F(x,y)=\left\{\mu(y) \displaystyle\prod_{j=1}^{N}\{x-x_j(y)\} \right\}_+, \qquad N\in \mathbb{N},
\end{equation}
\begin{equation}
F(x,y)=\left\{\mu(y) \displaystyle\prod_{j=1}^{N}\{x-x_j(y)\} \right\}_+, \qquad N\in \mathbb{N},
\end{equation} where ![]() $\mu(y)\in \mathbb{C}[y]$ and
$\mu(y)\in \mathbb{C}[y]$ and ![]() $x_1(y), x_2(y), \dots, x_N(y)$ are pairwise distinct Puiseux series in a neighbourhood of the point
$x_1(y), x_2(y), \dots, x_N(y)$ are pairwise distinct Puiseux series in a neighbourhood of the point ![]() $y=\infty$ that satisfy
$y=\infty$ that satisfy ![]() $P(x,y) -Q(x,y)x_y=0$. The symbol
$P(x,y) -Q(x,y)x_y=0$. The symbol ![]() $\left\{W(x,y)\right\}_+$ means that we take the polynomial part of the expression
$\left\{W(x,y)\right\}_+$ means that we take the polynomial part of the expression ![]() $W(x,y)$. Moreover, the degree of
$W(x,y)$. Moreover, the degree of ![]() $F(x,y)$ with respect to x does not exceed the number of distinct Puiseux series of the form (4.3) satisfying equation
$F(x,y)$ with respect to x does not exceed the number of distinct Puiseux series of the form (4.3) satisfying equation ![]() $P(x,y) -Q(x,y)x_y=0$ whenever the latter is finite. Furthermore,
$P(x,y) -Q(x,y)x_y=0$ whenever the latter is finite. Furthermore, ![]() $\mu(y)$ is either constant or an invariant algebraic curve of
$\mu(y)$ is either constant or an invariant algebraic curve of ![]() $\mathcal{X}$.
$\mathcal{X}$.
Theorem 4.8 Suppose that ![]() $x_1(y), x_2(y), \dots, x_N(y)$ are pairwise distinct Puiseux series in a neighbourhood of the point
$x_1(y), x_2(y), \dots, x_N(y)$ are pairwise distinct Puiseux series in a neighbourhood of the point ![]() $y=\infty$ that satisfy equation
$y=\infty$ that satisfy equation ![]() $P(x,y) -Q(x,y)x_y=0$. Let the polynomial
$P(x,y) -Q(x,y)x_y=0$. Let the polynomial ![]() $\mu(y)\in \mathbb{C}[y]$ be such that the following expression
$\mu(y)\in \mathbb{C}[y]$ be such that the following expression
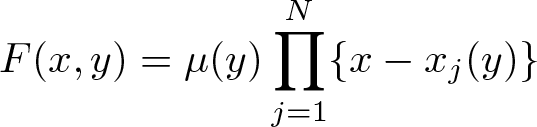 \begin{equation}
F(x,y)= \mu(y) \displaystyle\prod_{j=1}^{N}\{x-x_j(y)\}
\end{equation}
\begin{equation}
F(x,y)= \mu(y) \displaystyle\prod_{j=1}^{N}\{x-x_j(y)\}
\end{equation} is irreducible in ![]() $ \mathbb{C}[x,y]$, i.e. the non-polynomial part in (4.7) vanished producing the polynomial
$ \mathbb{C}[x,y]$, i.e. the non-polynomial part in (4.7) vanished producing the polynomial ![]() $F(x,y)$, then
$F(x,y)$, then ![]() $F(x,y)$ is an invariant algebraic curve of the polynomial vector field
$F(x,y)$ is an invariant algebraic curve of the polynomial vector field ![]() $\mathcal{X}$ and its related differential system (1.1).
$\mathcal{X}$ and its related differential system (1.1).
Acknowledgements
The authors want to thank the anonymous reviewer for her/his very helpful and constructive comments in previous versions of the article.
The first author is supported by the H2020 European Research Council grant MSCA-RISE-2017-777911, Universidad del Bío-Bío through 2120114 IF/I, ANID Fondecyt Iniciación F11230544, and VRIP-UBB-GI2310532. The second author is supported by FCT/ Portugal through UID/MAT/04459/2019.



























