No CrossRef data available.
 $\mathbf {MMTL}\boldsymbol {\forall }$
$\mathbf {MMTL}\boldsymbol {\forall }$Published online by Cambridge University Press: 18 October 2023
Monoidal t-norm based logic  $\mathbf {MTL}$ is the weakest t-norm based residuated fuzzy logic, which is a
$\mathbf {MTL}$ is the weakest t-norm based residuated fuzzy logic, which is a  $[0,1]$-valued propositional logical system having a t-norm and its residuum as truth function for conjunction and implication. Monadic fuzzy predicate logic
$[0,1]$-valued propositional logical system having a t-norm and its residuum as truth function for conjunction and implication. Monadic fuzzy predicate logic  $\mathbf {mMTL\forall }$ that consists of the formulas with unary predicates and just one object variable, is the monadic fragment of fuzzy predicate logic
$\mathbf {mMTL\forall }$ that consists of the formulas with unary predicates and just one object variable, is the monadic fragment of fuzzy predicate logic  $\mathbf {MTL\forall }$, which is indeed the predicate version of monoidal t-norm based logic
$\mathbf {MTL\forall }$, which is indeed the predicate version of monoidal t-norm based logic  $\mathbf {MTL}$. The main aim of this paper is to give an algebraic proof of the completeness theorem for monadic fuzzy predicate logic
$\mathbf {MTL}$. The main aim of this paper is to give an algebraic proof of the completeness theorem for monadic fuzzy predicate logic  $\mathbf {mMTL\forall }$ and some of its axiomatic extensions. Firstly, we survey the axiomatic system of monadic algebras for t-norm based residuated fuzzy logic and amend some of them, thus showing that the relationships for these monadic algebras completely inherit those for corresponding algebras. Subsequently, using the equivalence between monadic fuzzy predicate logic
$\mathbf {mMTL\forall }$ and some of its axiomatic extensions. Firstly, we survey the axiomatic system of monadic algebras for t-norm based residuated fuzzy logic and amend some of them, thus showing that the relationships for these monadic algebras completely inherit those for corresponding algebras. Subsequently, using the equivalence between monadic fuzzy predicate logic  $\mathbf {mMTL\forall }$ and S5-like fuzzy modal logic
$\mathbf {mMTL\forall }$ and S5-like fuzzy modal logic  $\mathbf {S5(MTL)}$, we prove that the variety of monadic MTL-algebras is actually the equivalent algebraic semantics of the logic
$\mathbf {S5(MTL)}$, we prove that the variety of monadic MTL-algebras is actually the equivalent algebraic semantics of the logic  $\mathbf {mMTL\forall }$, giving an algebraic proof of the completeness theorem for this logic via functional monadic MTL-algebras. Finally, we further obtain the completeness theorem of some axiomatic extensions for the logic
$\mathbf {mMTL\forall }$, giving an algebraic proof of the completeness theorem for this logic via functional monadic MTL-algebras. Finally, we further obtain the completeness theorem of some axiomatic extensions for the logic  $\mathbf {mMTL\forall }$, and thus give a major application, namely, proving the strong completeness theorem for monadic fuzzy predicate logic based on involutive monoidal t-norm logic
$\mathbf {mMTL\forall }$, and thus give a major application, namely, proving the strong completeness theorem for monadic fuzzy predicate logic based on involutive monoidal t-norm logic 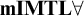 $\mathbf {mIMTL\forall }$ via functional representation of finitely subdirectly irreducible monadic IMTL-algebras.
$\mathbf {mIMTL\forall }$ via functional representation of finitely subdirectly irreducible monadic IMTL-algebras.
Please note a has been issued for this article.