The Coase theorem: coherent, logical, and not disproved

Abstract:

There exists a long line of challengers to the ‘Coase Theorem’. All of these rest on fundamental misconceptions of property rights, transaction costs, and their interaction. Here I examine two attacks that have gone unchallenged: one by Halpin, the other by Usher. I argue that both, in failing to either use or understand an adequate definition of transaction costs, fail to deliver a fatal blow to Coase's famous idea.

The central argument is not only a tautology, it is false. (Stigler, 1977: 442).

Technically speaking, there is no ‘Coase Theorem’ . . . there are many of them.¹ I have claimed that the ‘Coase Theorem’ can be simply stated: if transaction costs are zero, then the allocation of resources is independent of the distribution of property rights. ² Yet, despite its simplicity, the ‘Coase Theorem’ has been attacked and mocked from all sides. Although I would claim that all of these challenges result from a misunderstanding of the terms ‘transaction costs’ and ‘property right’, my purpose here is to merely demonstrate that the two most recent attacks have this feature, and therefore, lack merit.³

Andrew Halpin (2007) argued that the fundamental reasoning of Coase is flawed, and therefore the ‘theorem is disproved’. The problem is, in his analysis of Coase's reasoning, Halpin misreasons himself and slips some positive transaction costs in through a back door. Hidden or not, once positive transaction costs are introduced, it is no surprise that the Coasean logic does not go through. Halpin's error – a classic one – results from a failure to define and understand the implications of zero transaction costs.

Dan Usher (1998), on the other hand, has argued that the ‘Coase Theorem’ is inherently ambiguous, and that clarifying the ambiguity leads to two possible versions of the theorem. Remarkably, his dramatic conclusion is that one version is false and the other a useless tautology. Unlike Halpin who has an implicit notion of transaction costs, Usher's has an explicit, and inadequate, definition of transaction costs. When this narrow definition is combined with an equally inappropriate and narrow definition of property rights, the mysterious ‘tautological/error’ conclusion is understandable. Usher's definitional inadequacies stem from a failure to understand the relationship between economic property rights, legal property rights, and transaction costs – again, a rather common blunder. Ironically, this failure leads to many statements that are either wrong or incoherent – the very criticism he directs at the ‘Coase Theorem’.

Both examples highlight a lesson I articulated over 20 years ago: there can be no understanding of the ‘Coase Theorem’ or the meaning of Coase's two great papers unless there is a proper understanding of the terms and conditions of the concepts that form their foundation.⁴

1. Transaction costs . . . yet again

Any definition of transaction costs that excludes efforts to create property rights or includes costs that arise only out of scarcity cannot be consistent with the Coase theorem. (Allen, 1991: 13).

Transaction costs are often misunderstood. One source of misunderstanding ironically comes from efforts to find definitional meaning in the words of Coase.

Looking for a transaction cost definition in Coase's writings is futile on several grounds. First, and unfortunately, Coase did not fully understand the concept himself, and is often led astray in many of his arguments defending his work.⁵ Second, Coase simply never defined the term, and even years later only resorted to the use of examples.⁶ Third, he interchangeably used the term ‘costly transacting’, but this term seems clearly limited to an exchange of some type. Finally, even in his 1960 paper he contrasts ‘costly market transacting’ with ‘administrative costs’ and ‘governmental costs’, which he referred to as organizing costs within a firm or government, which implies that transaction costs are just one type of a more general cost of coordination.

However, the most common source of misunderstanding stems from the presumption that transaction costs are simple, obvious, ordinary costs that do not depend on, or result from, an information problem. This, usually unstated, neoclassical notion of transaction costs actually pre-dates Coase with Hicks’ (1935) work on frictions in money demand. Throughout the 1950s this treatment became commonplace within discussions of the ‘transaction demand for money’, and it became fully entrenched in the broader profession with Demsetz's (1968) classic work.⁷ Unfortunately, defining a transaction cost as a simple friction that happens within an exchange is inadequate for the types of issues that Coase was concerned about, and simply leads to an analysis not unlike a simple treatment of taxation. When applied to the matters of liability or general matters of organization, it is an easy exercise to show that when these types of costs are zero, the ‘Coase Theorem’ does not always hold.⁸

Rather than seeking a definition at the literature source or through an analogy with taxation, the key to defining transaction costs is to ask the following question: what type of cost would violate the ‘Coase Theorem’? Such a cost, if zero, would lead to the ‘Coase Theorem’ holding. Such a cost, if positive, would cause the theorem not to hold. That is what ultimately matters in terms of a proper definition.⁹

The definition of transaction costs that works is fundamentally related to ‘economic property rights’. Namely, transaction costs are the costs of establishing and maintaining economic property rights. ¹⁰ Following others, economic property rights are defined as the ability to freely exercise a choice. Property rights can be complete, meaning all attributes of the thing are owned and not in the public domain; and they can be perfect, meaning that the actual choice is fully manifested. Transaction costs are defined with respect to perfection.

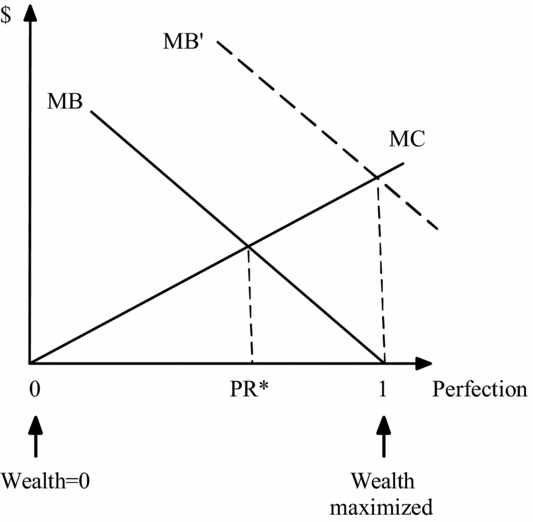
Consider Figure 1, which has perfection on the horizontal axis. The domain for perfection is (0,1), and one might consider this value as the degree of property right perfection. Hence, when the degree of property rights is zero, then no choice can be made, and under such an unimaginable circumstance no wealth is possible. On the other hand, when property rights are perfect – meaning all choices made are carried out freely – then, as everyone from Edgeworth to Coase have pointed out, wealth is maximized.¹¹Figure 1 is drawn under two stylized assumptions. First, there is some rising marginal cost of perfection, and therefore, given the definition above, the transaction costs in this case would be the area under the MC curve up to PR*. Second, the initial MB function is assumed to be zero when the degree of property right is one. Under such a circumstance, the net wealth, that is, the level of wealth net of the transaction costs, would be maximized at PR*.

Figure 1. The Marginal Costs and Benefits of Property Right Perfection.

In other words, when transaction costs are positive, the optimal degree of rights is not perfect, and as has been shown many times, the level of wealth depends on the distribution of these property rights. What is also clear from this graph is that had the MC function been zero, that is, had the transaction costs been zero, then property rights would be perfect, wealth would have been maximized, and the distribution of rights irrelevant. Finally, what the graph also shows is that the value of property rights might be so great (e.g., MB′) that perfect rights might attain even in a world where transaction costs are positive.¹² In other words, the ‘Coase Theorem’ only works one way: if transaction costs are zero, then property rights are perfect; but if property rights are perfect, transaction costs may be positive.¹³

2. Must wealth be constant for the Coase theorem?

Most objections to the Coase Theorem seem to underestimate what costless transacting could accomplish. (Coase, 1988: 163).

A common qualifier, and a source of many of the misdirected critiques of the ‘Coase Theorem’, is the claim that in order for the invariance result to hold, the wealth of the participants must remain constant.¹⁴ Since any change in property rights leads to a change in wealth, the ‘Coase Theorem’ never holds. This claim results from a misunderstanding of transaction costs and their relationship with property rights, because if transaction costs are zero, then property rights are defined perfectly and wealth must be constant when there is a transfer of rights.

Consider the famous rancher and farmer example, and suppose the rancher is not liable for the damage caused by his trespassing cattle. Now suppose the liability rule is reversed. How did this reversal come about? Ubiquitously, economists just assume that the switch happened without compensation. Indeed, this is the source of the claim that wealth must be held constant.

But does this make any sense? If a rancher has a legal liability right, this influences his economic property right over his cattle choices. Since transaction costs are assumed to be zero, the economic property right is perfect. But this means that the legal right must also be perfect. If the state (or anyone else) exogenously takes the legal right away and gives it to the farmer without compensation, then a theft has been committed. The legal right clearly was not perfect, the economic property right was not perfect, and the assumption of zero transaction costs was violated. An uncompensated transfer of rights is prima facie evidence that transaction costs are positive! They have sneaked through a back door unnoticed.¹⁵

There is an alternative way for the switch in liability rule to occur: the farmer could agree to accept liability because the rancher fully compensates him for it. Hence, the distribution of wealth obviously remains constant.¹⁶

We cannot have it both ways: either the rancher perfectly owns the right and must be compensated when it is removed, or he does not completely own it, and loses when it is taken away. Wealth only changes in this latter case where transaction costs are positive and the ‘Coase Theorem’ does not apply.

3. Disproving Halpin

Halpin (2007) considers the case where parties A and B have a conflict over the use of a piece of land, as in the standard rancher/farmer example. Denoting the value of the land use to A as v_a in activity a, the value of the land use to B as v_b in activity b, he then articulates Coase's ‘counterintuitive insight’ as:

1. (1) If v_a > v_b, A will continue with a

   EITHER (i) with entitlement and retain v_a

   OR (ii) where v_a − v_b = s

   by paying (v_b + (s − n)) to B and retaining n;

2. (2) If v_b > v_a, B will continue with b

   EITHER (i) with entitlement and retain v_b

   OR (ii) where v_b − v_a = s

   by paying (v_a + (s − n)) to A and retaining n.¹⁷

In other words, it doesn't matter if scenario (1) or (2) holds, the outcome is invariant. However, Halpin goes on to note the obvious: wealth is lower when either A or B has to purchase the right compared to when they have the entitlement. In his words,

In the case where A has the more valuable activity but lacks legal entitlement, he sees the value of a diminish by a sum of (v_a − n) due to the imposition of legal liability. (2007: 328).

Because the wealth of continuing in activity a is lower without the entitlement, Halpin notes that A may consider

. . . whether instead of buying out B he would be better off changing to a non-conflicting activity on the land, or moving his activity to other land where the conflict would not arise. (2007: 328).

If p represents a third alternative activity for A, with v_p the value of this activity, then ‘. . .the challenge to the counterintuitive insight’ is:

1. (3) If v_a > v_b, A will continue with a

   EITHER (i) with entitlement and retain v_a

   OR (ii) where v_a − v_b = s

   by paying (v_b + (s − n)) to B and retaining n > v_p

   OR (iii) will not continue with a where v_p > n.

There is a similar condition if v_b > v_a, with the alternative being activity q. Clearly, whether activity a, b, p, or q take place depend on the rule of liability. Hence, according to Halpin, the ‘Coase Theorem’ is wrong.

Although dressed up in notation, it is an old objection. Different legal entitlements lead to different levels of wealth. Since there are other lower valued activities for the land, a case of no entitlement may lead the landowner to switch the occupation of the land. Hence, the allocation of resources depends on the legal rule.

Given what was said above, however, regarding the relationship of zero transaction costs and the constancy of wealth, the mistake Halpin makes is rather obvious. If the wealth level in case 1(i) is different from 1(ii), then that means transaction costs are not zero, and of course the allocation will depend on the endowment of liability.¹⁸ Had the rights of A been perfect, then the lost entitlement would have been fully compensated for and the ‘challenge’ would not have arisen. Halpin is disproved. His challenge, like so many before his, is the result of a failure to appreciate what a zero transaction cost world would look like. In such a world – like in a perfectly competitive neoclassical model – all transfers of rights happen voluntarily through an exchange.

4. The logic of Usher

Usher's (1998) attack on the ‘Coase Theorem’ stands out because it is so brazen. Unlike Halpin who misunderstands the implication of zero transaction costs, Usher's error results from an inappropriate understanding of the meaning of ‘property rights’. Namely, although he uses a proper type of transaction costs in his examples, he only considers ‘property rights’ as ‘legal property rights’. Not surprisingly, with an inappropriately narrow definition, he finds the ‘Coase Theorem’ incoherent.

The distinction in definitions is critical because the ‘Coase Theorem’ is a statement about the property rights definition of transaction costs, and economic property rights. Many get derailed on the concept of transaction costs because they ignore the concept of property rights.¹⁹ However, the two concepts – economic property rights and transaction costs – are fundamentally inter-linked through the ‘Coase Theorem’, and are really two sides of the same coin. Using a narrow legal notion of property is inappropriate for considerations of how economic property rights are distributed, and this explains why Usher draws the conclusion that the ‘Coase Theorem’ is nonsense. He's trying to fit a square peg into a round hole.

Usher claims that the ‘Coase Theorem’ as normally stated is ambiguous, and therefore, in an attempt to nail it down he claims it can mean one of two things:

1. B1. If transaction costs are zero any assignment of property right gives rise to an efficient allocation of resources. Efficiency requires some assignment of property rights.

2. B2. If transaction costs are zero, resources will be allocated efficiently regardless of whether or not there is an assignment of property rights.

Although Usher correctly points out that Posner and others (like Alchian, Barzel, Becker, Cheung, Coase, Stigler, etc.) in the law and economics literature use the B1 definition, he claims that B1 is false. This leaves B2 as the only alternative, but he then argues that B2 is tautological and misleading; hence the title of his paper: the ‘Coase Theorem’ is either tautological, incoherent, or wrong. Unfortunately for Usher, when the proper definitions are used, it is B1 that is logical and coherent and B2 that is incoherent and irrelevant. Usher got it completely backwards.

Usher's argument for why B1 is false rests on his conclusion that ‘the premise of costless bargaining’ means that ‘an efficient output can be attained not just for any initial allocation of property rights, but without property rights at all’!^”20 The claim is that B1 is false because it is too strong. According to Usher, property rights are simply not necessary when bargaining (transaction costs are zero).

Usher takes two shots at making this case. First he provides the following no property rights scenario:

. . . imagine what would happen if the cowboy and farmer find themselves side by side with no property rights assigned. Do they fight? Perhaps. Regardless, something must happen . . . (1998: 7).

Usher implies that since something happens, and since ‘bargaining costs are zero’, we end up at the efficient outcome. But such a conclusion implicitly assumes that an economic property right exists, since clearly if ‘something must happen’ then efforts are being made to establish property rights. For example, if ‘the two might fight’ then they must be endowed with strength and means to increase what they already have. That is, Usher starts from a position of positive and imperfect economic property rights not zero property rights. The only charitable way to make sense of what Usher is saying is to read between the lines. When he claims that property rights are zero, he must simply mean that the legal rights are undefined. But the ‘Coase Theorem’ rests on economic property rights: the ability to freely exercise choices, not just under the law, but in reality. As such, Usher's argument has nothing to do with the ‘Coase Theorem’ because imperfect economic property rights means that transaction costs are positive.²¹

Usher goes on to make his case more formal by arguing that there is some (perhaps low) certainty equivalent to (now) ‘insecure’ property rights. As a result, ‘no property rights’ simply means a different starting point for bargaining. Given any starting point, the rancher and the cowboy still end up at the optimal allocation because, by assumption, it costs nothing to bargain. If Usher literally means there are no economic property rights, then his claim is a non-sequitur. Zero economic property rights leads to zero trade. How can there be an exchange of economic property rights when there are no economic property rights? If Usher simply means there are no legal property rights, but the economic property rights are perfect, then calling this a case of ‘insecure’ rights is a misnomer, and the whole exercise a mere sleight of hand.

Usher's argument becomes incoherent because he misses the point that zero transaction costs necessarily means that economic property rights are perfectly defined over all goods, including the gains from trade. For example, Usher states that the gains from trade are ‘somehow’ divided between the farmer and the rancher. Presumably, if any effort is devoted to splitting the pie (and why wouldn't there be if the surplus is in the public domain?), then once again we have a world of positive transaction costs and the ‘Coase Theorem’ does not apply. He also states that ‘The initial allocation of rights sets bounds on the final allocation of income, but does not determine it uniquely’. But why not? Either all property rights are defined perfectly or they are not – Usher cannot have his cake and eat it too. The gains from trade must also be allocated exogenously. Clearly, Usher's analysis contains some hidden constraints if some property rights are perfectly defined, while others are prohibitively costly to define.²²

Having shown that B1 is false, and noting that it is mutually exclusive with B2, Usher concludes that B2 is true. But he also claims it is practically a tautology and misleading. The reason being that for him, to say there is zero transaction costs (no costs of bargaining) is to say the parties reach an efficient outcome. The caveat of property rights is irrelevant. Again, this claim only makes sense if by property rights he means ‘legal property rights’. Otherwise, if transaction costs are zero, then economic property rights must be perfect. If property rights are perfect, they certainly exist and Usher's definition of the theorem is contradictory. Hence, Usher's finding that his second version of the theorem is ‘grossly, almost ludicrously, misleading’ is not too surprising, and results from his confusion over economic and legal property rights.²³

5. Conclusion

The irony over so many of the discussions of the first five sections of ‘The Problem of Social Cost’ – those that articulate the ideas behind the ‘Coase Theorem’ – is that the matters discussed are ones that Coase never bothered about. Coase was the first critic of the ‘Coase Theorem’ and he was merely pointing out how problems of social cost disappeared when transaction costs are zero. On the contrary, many responses to the ‘Coase Theorem’ are reactions to the reality of invariance and the fact that the outcome is ‘efficient’. Indeed, in the case of Usher he notes that the theorem may be useful ‘as a lesson about how property rights promote efficiency in the economy’.²⁴ But a focus on efficiency is completely off target. Efficiency comes from assuming maximization not zero transaction costs. If transaction costs are zero the outcome is first-best efficient, if they are positive it is second-best efficient.²⁵ Those who utilize Coase's key idea to understand the real world recognize that the entire point of the ‘Coase Theorem’ is to draw attention to the fact that if one is interested in the allocation of property rights – the rules, laws, customs, and methods of exchange and production – then one must consider a model in which transaction costs are positive.

Of course, this begs the question: what are these transaction costs? The only concept that works is the property rights definition: transaction costs are the costs of defining and maintaining economic property rights. Any attempt to understand the ‘Coase Theorem’ that (1) uses an improper definition of either transaction costs or property rights, (2) fails to understand the relationship between these two ideas, or (3) fails to understand the subtle implications of zero transaction costs, can only lead to a logical dead end. All critiques of Coase stem from one of these three issues. Here I have examined two of the most recent attacks, and shown where they have gone off the rails. Sooner or later, the profession is bound to figure it out.