Slow-wave coplanar waveguides based on inductive and capacitive loading and application to compact and harmonic suppressed power splitters

Abstract

In this paper, a slow-wave transmission line implemented in coplanar waveguide technology, based on simultaneous inductive and capacitive loading, is presented for the first time. The shunt capacitors are achieved by periodically etching transverse strips in the back substrate side, connected to the central strip through metallic vias. The series inductors are implemented by etching rectangular slots in the ground plane. The effect of these reactive elements is an enhancement of the effective shunt capacitance and series inductance of the line, leading to a significant reduction of the phase velocity (slow-wave effect). Consequently, the guided wavelength is also reduced, and these lines can be applied to the miniaturization of microwave components. Moreover, due to periodicity, these artificial lines exhibit stop bands (Bragg effect) useful for spurious or harmonic suppression. A compact harmonic suppressed power splitter, based on a slow wave 35.35 Ω impedance inverter, has been designed and fabricated in order to demonstrate the potential of the proposed approach. The length of the inverter is 48% the length of the conventional counterpart, and measured power splitting at the first (3f₀) and second (5f₀) harmonic frequencies is rejected more than 49 and 23 dB, respectively.

I. INTRODUCTION

Slow-wave transmission lines are artificial lines exhibiting small phase velocity as compared with ordinary lines [1, 2]. This slow-wave effect is typically achieved by externally loading a host line with reactive elements, including distributed [3–10] or lumped/semi-lumped (i.e. electrically small) components [11–34]. Most realizations of slow-wave transmission lines based on lumped or semi-lumped components use periodic capacitive loading [11–24]. By periodically loading the host line with shunt capacitors, the effective capacitance of the line is enhanced, and therefore the phase velocity is reduced. Alternatively, series connected inductances have been used as a means to achieve the slow-wave effect (in this case resulting as consequence of the enhancement of the effective line inductance) [26–29]. Obviously, by replacing the ordinary lines with slow-wave artificial lines exhibiting the required characteristic impedance and electrical length at the design frequency, it is possible to substantially reduce the size of the microwave components. Additionally, if the slow-wave transmission lines are periodic, it is possible to suppress undesired spurious or harmonic bands by virtue of the Bragg effect, related to periodicity and providing stop bands in the transmission response of the artificial lines [2, 35, 36].

The implementation of slow-wave transmission lines with simultaneous inductive and capacitive loading is less common [32, 37, 38]. The presence of both reactive elements is useful in order to achieve further levels of miniaturization. Moreover, further design flexibility can be achieved due to the presence of both reactive elements. In this paper, we report a slow-wave transmission line implemented in coplanar waveguide (CPW) technology by loading the line with capacitive and inductive elements. The shunt capacitors are implemented by means of transverse strips, etched in the back substrate side of the CPW, similar to the work presented in [39]. The series inductive elements are implemented by etching rectangular slots in the ground plane, following the approach first presented in [28] by some of the authors. Then these lines are applied to the miniaturization and harmonic suppression of a power splitter based on a slow-wave impedance inverter.

The work is organized as follows. In Section II, the circuit schematic and the topology of these lines are reported, and the expressions providing the electrical length of the unit cell and the characteristic impedance are derived from Floquet analysis of periodic structures. The number of cells necessary to efficiently suppress the harmonic responses in quarter-wavelength impedance inverters (later used for the implementation of a power splitter) is also obtained in this section. Section III is focused on the synthesis of a 35.35 Ω slow-wave impedance inverter. The designed and fabricated power splitter is reported in Section IV. Finally, the main conclusions are highlighted in Section V.

II. SLOW-WAVE TRANSMISSION LINE: TOPOLOGY, CIRCUIT SCHEMATIC, AND ANALYSIS

The topology (unit cell) and circuit schematic of the proposed slow-wave CPW transmission lines are depicted in Fig. 1. The host line is described by the characteristic impedance Z ₀, and by the electrical length (kl), where k is the phase constant and l is the total (physical) length of the unit cell. The loading reactive elements are the series inductance, L _ls, and the shunt capacitance, C _ls. Losses are excluded in this model.

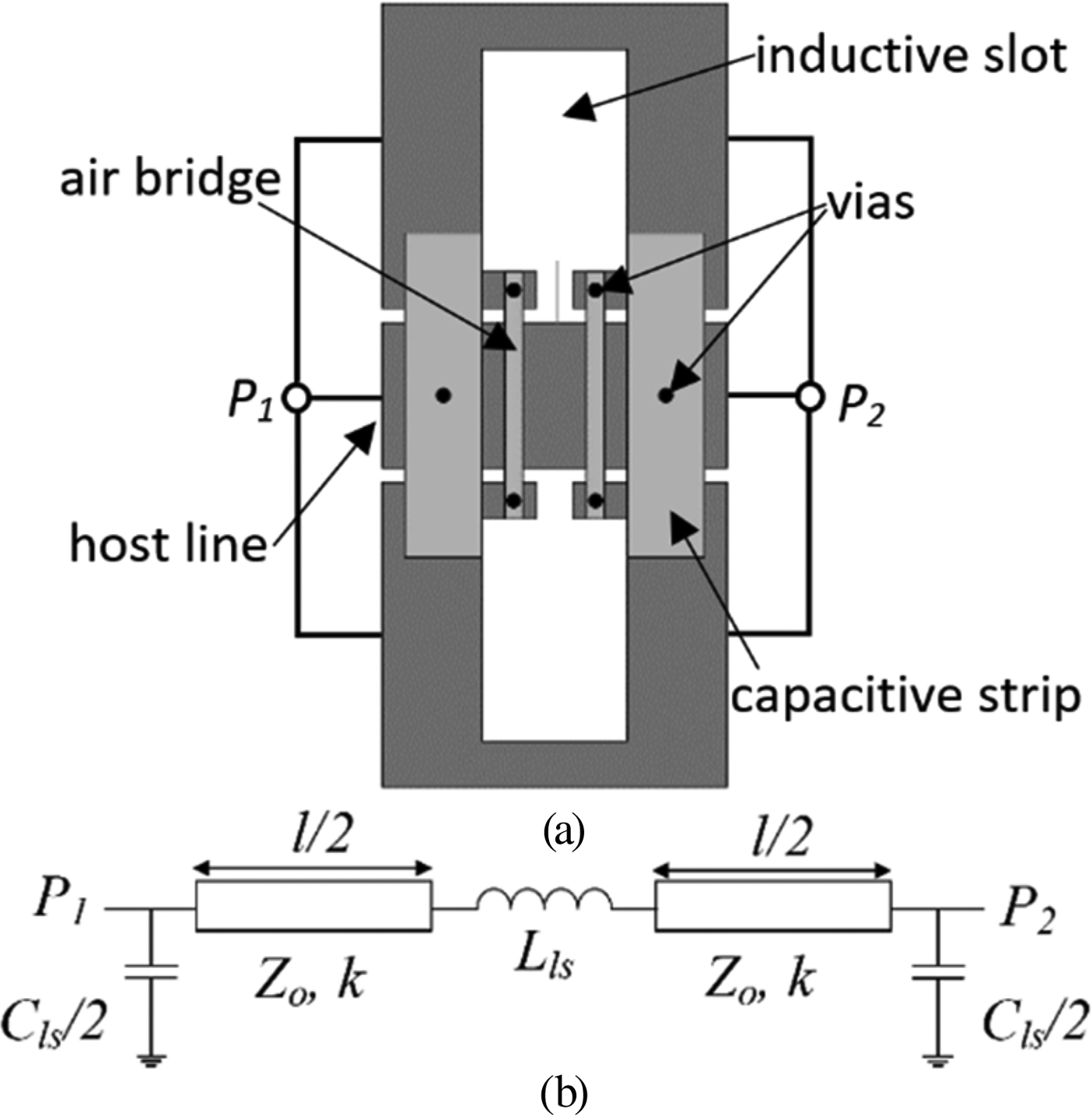
Fig. 1. Topology (unit cell) (a) and circuit schematic (b) of the slow-wave CPW transmission line under consideration.

The unit-cell electrical length, βl, and characteristic impedance, Z _B, of the loaded line are given by [2, 40]:

(1)$$\cos (\beta l) = A$$

and

(2)$$Z_B = \displaystyle{B \over {\sqrt {A^2 - 1}}}, $$

where A and B are the first row elements of the transmission ABCD matrix of the two-port unit cell (note that expressions (1) and (2) are valid as long as the structure is symmetric with regard to the midplane between the input and the output ports).

The circuit schematic of Fig. 1(b) is composed by the cascade of five simple two-ports, consisting of shunt capacitors (external two-ports), series inductor (central two-port), and transmission line sections (intermediate two-ports). The ABCD matrix of the whole structure is given by the product of the individual matrices of each constitutive two-port, given by:

(3a)$$[{\bf A}]_L = \left( {\matrix{ 1 & {L\omega j} \cr 0 & 1 \cr}} \right)$$

(3b)$$[{\bf A}]_C = \left( {\matrix{ 1 & 0 \cr {C\omega j} & 1 \cr}} \right)$$

(3c)$$[{\bf A}]_{TL} = \left( {\matrix{ {\cos (kl)} & {jZ_0\sin (kl)} \cr {\displaystyle{j \over {Z_0}}\sin (kl)} & {\cos (kl)} \cr}} \right),$$

where the subscripts L, C, and TL are used to differentiate the ABCD matrices of a series inductance, L, shunt capacitance, C, and transmission line section with electrical length kl and characteristic impedance Z ₀, respectively. From expressions (3), the ABCD matrix of the two-port of Fig. 1(b) can be easily inferred, and expressions (1) and (2) are found to be:

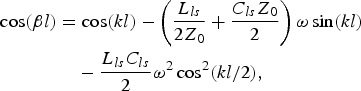(4)$$\eqalign{\cos (\beta l) & = \cos (kl) - \left( {\displaystyle{{L_{ls}} \over {2Z_0}} + \displaystyle{{C_{ls}Z_0} \over 2}} \right)\omega \sin (kl) \cr & \quad - \displaystyle{{L_{ls}C_{ls}} \over 2}\omega ^2\cos ^2(kl/2),} $$

(5)$$Z_B = \displaystyle{{ - jB} \over {\sin (\beta l)}} \equiv \displaystyle{{Z_0\sin (kl) + \omega L_{ls}\;{\cos} ^2(kl/2)} \over {\sin (\beta l)}},$$

where ω is the angular frequency. The unit-cell electrical length, βl, and characteristic impedance, Z _B, of the loaded lines are design parameters, given by the specific application. An additional bound for the four unknowns (Z ₀, kl, L _ls, and C _ls) is given by the so-called slow-wave ratio, swr, defined by

(6)$$swr = \displaystyle{{v_{pL}} \over {v_{po}}} = \displaystyle{{\omega /\beta} \over {\omega /k}} = \displaystyle{{kl} \over {\beta l}},$$

where v _pl and v _po are the phase velocities of the loaded and unloaded lines, respectively. The swr is a fundamental parameter determining the miniaturization level. Theoretically, the physical length of the slow-wave transmission line, as compared with one of the ordinary lines, is dictated by the swr. However, the reactive elements loading the line have finite size and, hence, the length reduction given by the swr is not achievable in practice.

Note that once βl and swr are set to a certain value (the former given by design specifications and the latter dictated by the compactness level required), kl is given by (6). However, the three remaining parameters characterizing the unit cell of Fig. 1(b), i.e. Z ₀, L _ls, and C _ls, are not univocally determined by equations (4) and (5). As Z ₀ increases, C _ls increases and L _ls decreases (see Fig. 2). Hence, a tradeoff is necessary in order to avoid extreme values of L _ls and C _ls.

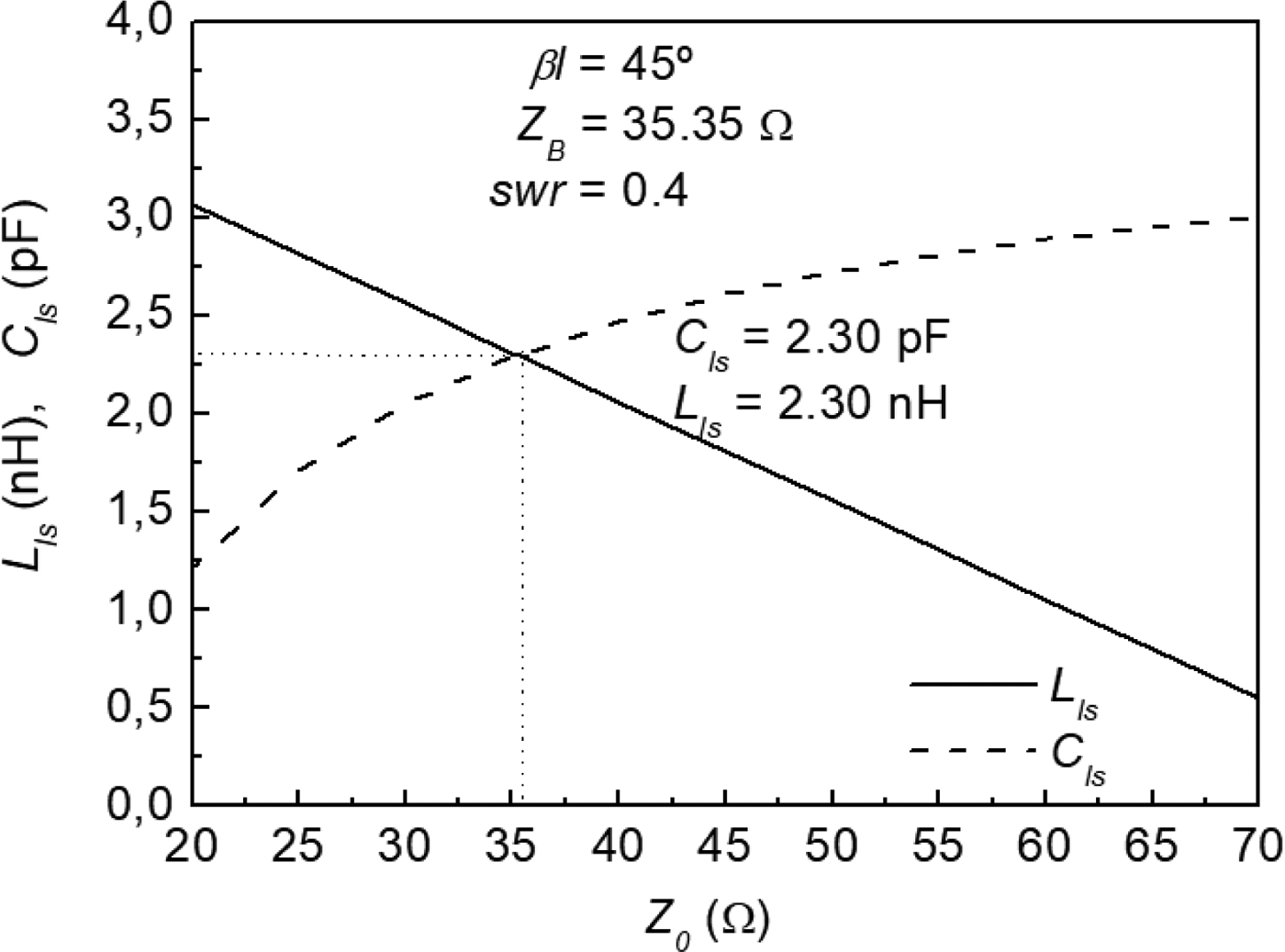
Fig. 2. Variation of L _ls and C _ls with Z ₀ that result from the solution of (4)–(6) with βl = 45°, Z _B = 35.35 Ω, and swr = 0.4.

Another important parameter is the number of cells of each transmission line section under consideration, N. This parameter determines the bandwidth and position of the stop band of the periodic structure, and is therefore relevant in applications where spurious or harmonic suppression is desired. Of particular interest is the cutoff frequency of the slow-wave transmission line, which corresponds to the onset of the stop band. This frequency can be determined from (4). Let us consider that the required electrical length of the slow-wave transmission line is θ, and that such line is implemented with N unit cells (i.e. βl = θ/N). In the limit of the first transmission band, βl = π (just above this frequency β is purely imaginary). Therefore, the cutoff frequency, f _C, can be inferred from the first frequency satisfying:

(7)$$\eqalign{- 1 & = \cos \left( {swr\displaystyle{\theta \over N}\displaystyle{\omega \over {\omega _0}}} \right) - \left( {\displaystyle{{L_{ls}} \over {2Z_0}} + \displaystyle{{C_{ls}Z_0} \over 2}} \right)\omega \sin \left( {swr\displaystyle{\theta \over N}\displaystyle{\omega \over {\omega _0}}} \right) \cr & \quad - \displaystyle{{L_{ls}C_{ls}} \over 2}\omega ^2\cos ^2\left( {swr\displaystyle{\theta \over {2N}}\displaystyle{\omega \over {\omega _0}}} \right)}$$

and note that this frequency depends on N, as anticipated. Inspection of (7) reveals that this equation has multiple solutions. In particular, the frequencies satisfying

(8)$$swr\displaystyle{\theta \over N}\displaystyle{\omega \over {\omega _0}} = (2n + 1)\pi {\rm, with}\; n = 0,1,2,...$$

correspond to the upper limits of the multiple stop bands that these periodic structures support. The solution that results by considering n = 0 provides the upper limit of the first stop band, the one of interest, i.e.

(9)$$\omega _s = 2\pi f_s = \displaystyle{{N\omega _0\pi} \over {swr \cdot \theta}}. $$

For which concern the lower limit of that band, f _C, it is not possible to obtain it analytically from (7). Despite the fact that such frequency can be solved numerically, it can be estimated from the approximate lumped element model of the structure, given in Fig. 3, namely [2]

(10)$$f_C = \displaystyle{1 \over {\pi \sqrt {(L + L_{ls})(C + C_{ls})}}}. $$

Fig. 3. Lumped element equivalent circuit of the unit cell of Fig. 1.

Note that the validity of this approximation improves as the loading reactive elements increase as compared with the element values describing the host line (L and C), as discussed in [2]. Since large element values are necessary to achieve small slow-wave ratios, swr, it follows that the estimation of f _C from the lumped element model is reasonable in applications where high compactness levels are pursued.

According to the lumped element circuit model, the electrical length of the unit cell can be expressed as

(11)$$\beta l = \displaystyle{\theta \over N} = 2\pi f_0\sqrt {(L + L_{ls})(C + C_{ls})}. $$

Consequently, the cutoff frequency and the design frequency are related by

(12)$$\displaystyle{{f_C} \over {f_0}} = \displaystyle{{2N} \over \theta}. $$

Expression (12) is fundamental in order to determine the number of cells, N, necessary to efficiently suppress spurious or harmonic bands. For quarter-wavelength impedance inverters, with θ = π/2, and devices based on it, the first harmonic band appears at 3f ₀. This means that (12) must be smaller than 3 if the first harmonic band must be suppressed, and, as a result, N = 1 or 2. In principle, the preferred solution should be N = 2 for two main reasons: (i) f _C is more separated from f ₀, avoiding the alteration of the response in the region of interest, and (ii) the stop band bandwidth is larger since, according to (9), f _s is also larger with N = 2. Nevertheless, the frequency given by (9) with N = 2 is typically very large, and the predictions of the model of Fig. 1 in the vicinity of that frequency do not match with the responses of any planar implementation of the structure, since the lumped elements L _ls and C _ls do not provide a good description of the semi-lumped (electrically small and planar) components that are typically used (transverse strips for the capacitors and slots in the ground planes for the inductors, in the present work, based on slow-wave CPWs). Thus, in any practical scenario, the upper frequency of the stop band inferred from (9) is overestimated. Despite of that fact, the harmonic suppression efficiency improves by choosing N = 2. Since the device proposed in this paper, a compact and harmonic suppressed power splitter, is based on an impedance inverter, the number of cells of the constitutive slow-wave transmission line (acting as impedance inverter) is N = 2.

III. SYNTHESIS OF THE SLOW-WAVE IMPEDANCE INVERTER

The slow-wave impedance inverter under consideration, to be later applied to the design of a compact power splitter, has an impedance of Z _B = 35.35 Ω, and, obviously, an electrical length of θ = 90° (the considered operating frequency has been set to f ₀ = 1 GHz). According to the previous section, the number of cells of the inverter is set to N = 2; consequently, βl = 45°. We have set the slow-wave ratio to swr = 0.4, and, therefore, kl = 18°. Note that the remaining unknowns, Z ₀, C _ls, and L _ls, are not unequivocally determined from (4) and (5). We have set the characteristic impedance of the host line to Z ₀ = 35.35 Ω, providing the following reactive values: C _ls = 2.30 pF and L _ls = 2.30 nH, which are easily implementable in CPW technology. Nevertheless, the variation of C _ls and L _ls with Z ₀ is depicted in Fig. 2, from which it follows that by choosing Z ₀ = 35.35 Ω, extreme reactive values are avoided.

Once the element values of the circuit of Fig. 1(b) are determined, the next step is the generation of the layout. As mentioned in the introduction, our aim in the paper has been the implementation of the inverter (and the subsequent power splitter) in CPW technology by loading the line with inductive slots in the ground plane and capacitive transverse strips in the back substrate side (the latter connected to the central strip of the CPW by means of vias). To this end, we have independently determined the slot dimensions providing the required inductance values, as well as the dimensions of the transverse strips necessary to achieve the necessary shunt capacitance. Nevertheless, some post-optimization has been necessary to adjust the characteristic impedance and electrical length to the design values at the operating frequency. The layout of the unit cell, as well as the characteristic impedance and electrical length are depicted in Fig. 4. It can be appreciated that the required characteristic impedance (Z _B = 35.35 Ω) at f ₀ is achieved and the electrical length of the unit cell is roughly the nominal value of 45° (thus providing an electrical length of 90° for the two-cell, i.e. N = 2, impedance inverter). The considered substrate is Rogers RO3010 with thickness h = 1.27 mm, dielectric constant ε _r = 10.2, and loss tangent tanδ = 0.0023.

Fig. 4. Layout (a), characteristic impedance (b), and electrical length (c) of the inverter unit cell. Dimensions are: L _w = 3.20 mm, W _w = 4.9 mm, L _C = 1.7 mm, W _C = 7.14 mm, w = 3.2 mm, s = 0.32 mm.

IV. DESIGNED AND FABRICATED SLOW WAVE SPLITTER

The layout of the designed slow-wave power splitter is depicted in Fig. 5, where it is compared to the layout of the conventional CPW implementation. The simulated frequency response of both structures, inferred from Keysight Momentum, can be seen in Fig. 6. It can be appreciated that the response of the slow-wave power splitter is roughly the same than one of the ordinary splitters in the region of interest (vicinity of f ₀). However, the first (at 3f ₀) and second (at 5f ₀) harmonic bands of the conventional splitter are significantly suppressed in the slow-wave implementation.

Fig. 5. Layouts of the slow-wave (a) and ordinary (b) CPW power splitters. These layouts are drawn to scale for easy comparison. Relevant dimensions (i.e. inverter lengths) are: L′ = 13.20 mm and L = 27.27 mm.

Fig. 6. Simulated frequency response of the splitters of Fig. 5.

The designed slow-wave power splitter has been fabricated by means of a LPKF-H100 drilling machine. The photograph is depicted in Fig. 7, whereas the measured response, inferred by means of the Keysight PNA 5221A vector network analyzer, is shown in Fig. 8, where it is compared to the simulated response. The agreement between both responses is very good. The measured matching at f ₀ is S ₁₁ = −35 dB, whereas the measured power splitting is S ₂₁ = −3.3 dB and S ₃₁ = −3.2 dB. These values are good, with power splitting very close to the ideal value of −3 dB. Concerning the filtering capability of the designed slow-wave splitter, the measured rejection levels at the harmonic frequencies are better than 49 dB (at 3f ₀) and 23 dB (at 5f ₀).

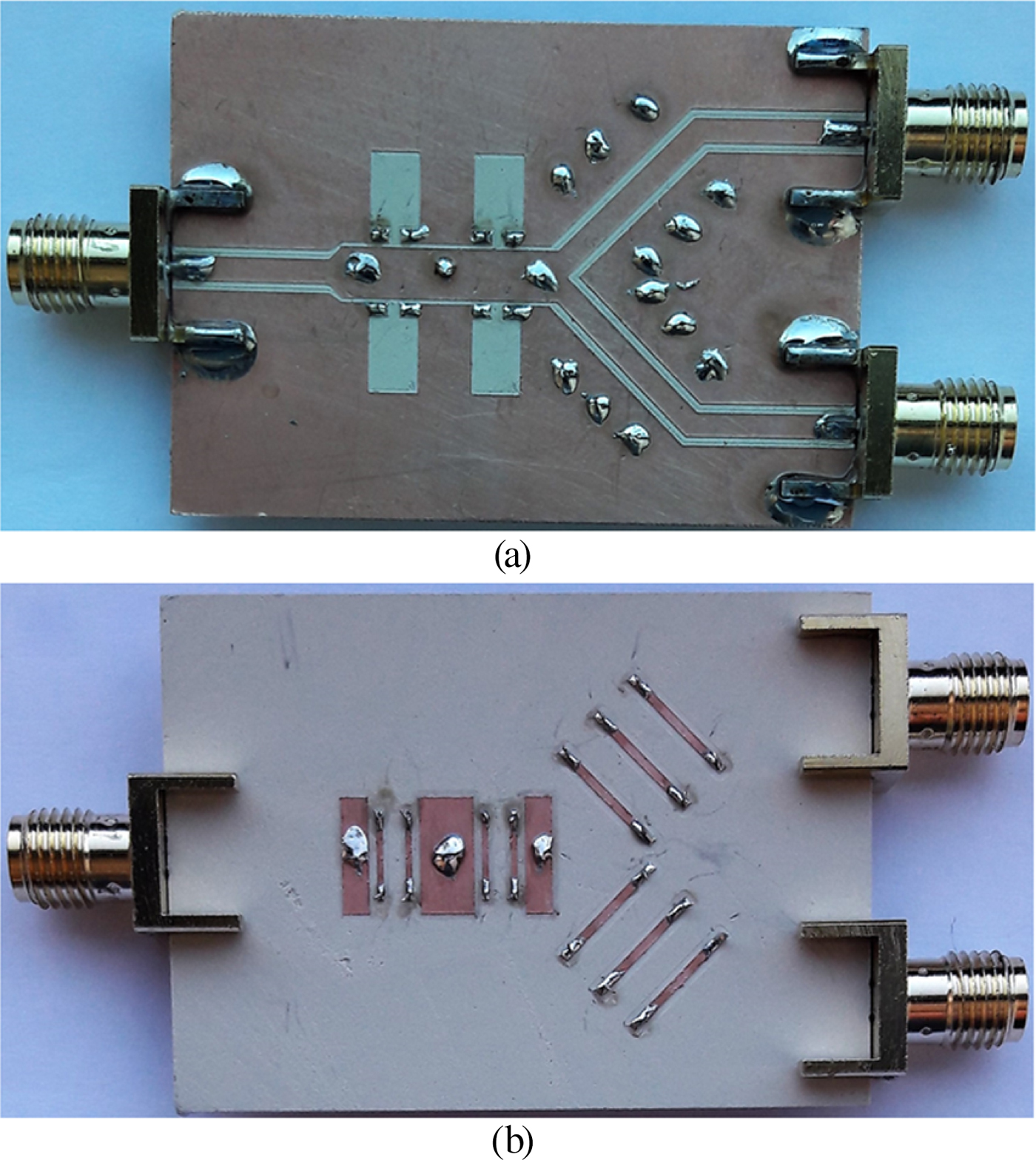
Fig. 7. Photograph of the fabricated slow-wave power splitter. (a) Top; (b) bottom.

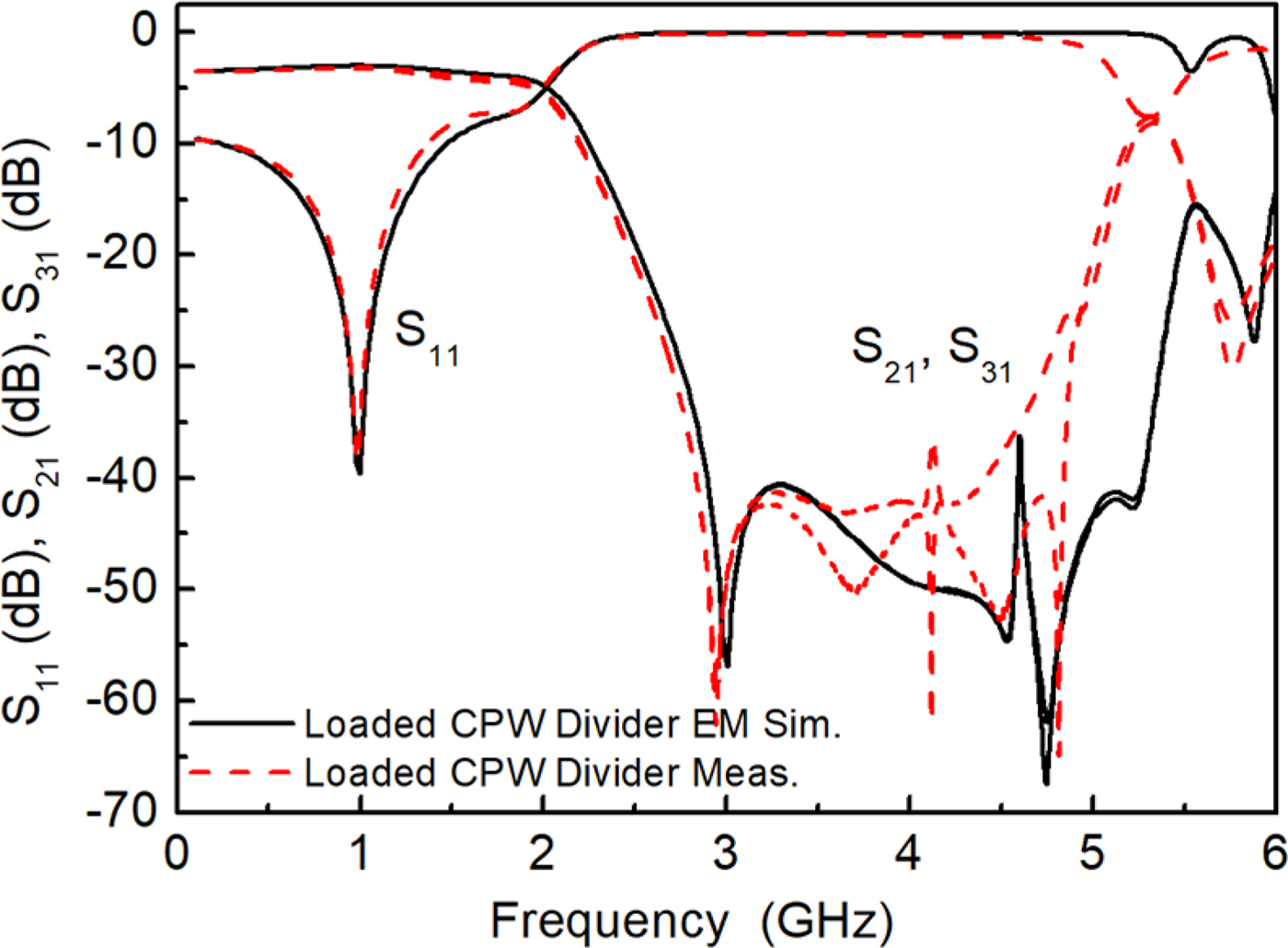
Fig. 8. Measured and simulated frequency response of the designed and fabricated slow-wave splitter.

Concerning dimensions, it is remarkable that the length of the slow-wave inverter used to implement the splitter is 48% the length of the ordinary counterpart. Obviously the form factor in the proposed slow-wave inverter is worst as compared with one of the conventional inverters, i.e. the new inverter is wider, due to the inductive slots etched in the ground plane. Nevertheless, the key parameter for the splitter in terms of size is the inverter length, since the transverse dimensions of the whole splitter are dictated by the output access lines.

Compact power splitters implemented by means of left-handed or composite right- and left-handed lines with similar size reduction to the one achieved in this paper have been reported [41, 42]. In [41], the bandwidth of the reported splitter is smaller than one of the conventional counterparts, contrary to the splitter reported in this paper, where bandwidth is very similar to one of the conventional splitters, as Fig. 8 indicates. Moreover, the splitter in [41] does not have harmonic suppression capability. With regard to the splitter of [42], size reduction of the constitutive lines is comparable. However, the device in [42] is focused on dual-band functionality, and for this reason, comparing device performance is not significant in this case.

V. CONCLUSION

In conclusion, a CPW slow-wave power splitter with reduced size and harmonic suppression capability has been presented in this paper. Size reduction and harmonic suppression has been achieved by implementing the 35.35 Ω quarter-wavelength impedance inverter, necessary to achieve the power division by the considered topology, by means of a capacitively and inductively loaded slow-wave CPW transmission line. The series inductors have been implemented by means of rectangular slots, etched in the ground plane, whereas the shunt capacitive effect has been achieved by etching transverse strips in the back substrate side, connected to the central strip of the CPW line through metallic vias. The device has been fabricated, and the measured response has shown that the device functionality at the design frequency has been achieved, with very similar response to one of the ordinary CPW implementation in the region of interest. However, because the Bragg effect is associated with periodicity, a band gap in the inverter response opens, and this has been used to reject the first harmonic bands of the designed power splitter. The proposed structure is a multifunctional device with power splitting and filtering capability simultaneously, of interest in applications where size is a critical aspect.