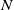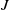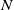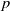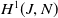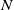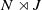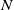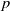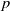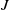Actions of nilpotent groups on nilpotent groups

Abstract

For finite nilpotent groups $J$ and $N$, suppose $J$ acts on $N$ via automorphisms. We exhibit a decomposition of the first cohomology set in terms of the first cohomologies of the Sylow $p$-subgroups of $J$ that mirrors the primary decomposition of $H^1(J,N)$ for abelian $N$. We then show that if $N \rtimes J$ acts on some non-empty set $\Omega$, where the action of $N$ is transitive and for each prime $p$ a Sylow $p$-subgroup of $J$ fixes an element of $\Omega$, then $J$ fixes an element of $\Omega$.

1. Introduction

Given a finite nilpotent group $J$ acting on a finite nilpotent group $N$ via automorphisms, crossed homomorphisms are maps $\varphi \,:\, J \to N$ satisfying $\varphi (\,jj^{\prime}) = \varphi (\,j) \varphi (\,j^{\prime})^{j^{-1}}$ for all $j,\,j^{\prime} \in J$ . Two such maps $\varphi$ and $\varphi ^{\prime}$ are cohomologous if there exists $n \in N$ such that $\varphi ^{\prime}(\,j) = n^{-1} \varphi (\,j) n^{j^{-1}}$ for all $j\in J$ ; in this case, we write $\varphi \sim \varphi ^{\prime}$ . We define the first cohomology $H^1(J,N)$ to be the pointed set $Z^1(J,N)$ of crossed homomorphisms modulo this equivalence relation where the distinguished point corresponds to the class containing the map taking each element of $J$ to the identity of $N$ .

We first show that the cohomology set $H^1(J,N)$ decomposes in terms of the first cohomologies of the Sylow $p$ -subgroups $J_p$ of $J$ as follows:

Lemma 1. For finite nilpotent groups $J$ and $N$ , suppose $J$ acts on $N$ via automorphisms. Then the map $\varphi \mapsto \times _{p\in \mathcal {D}} \varphi |_{J_p}$ for $\varphi \in H^1(J,N)$ induces an isomorphism $H^1(J,N) \cong \times _{p\in \mathcal {D}} H^1(J_p, N)^{J_p^{\prime}}$ of pointed sets, where $\mathcal D$ denotes the shared prime divisors of $\lvert J\rvert$ and $\lvert N\rvert$ , and for each $p$ , $J_p$ is the Sylow $p$ -subgroup of $J$ and $J_p^{\prime}$ is the Hall $p^{\prime}$ -subgroup of $J$ .

This parallels the well-known primary decomposition of $H^1(J,N)$ for abelian $N$ (see Section 3 for details). As the bijective correspondence between $H^1(J,N)$ and the $N$ -conjugacy classes of complements to $N$ in $N\rtimes J$ continues to hold for nonabelian $N$ [6, Exer. 1 in §I.5.1], Lemma1 provides an alternate proof of a result of Losey and Stonehewer [5]:

Proposition 2 (Losey and Stonehewer). Two nilpotent complements of a normal nilpotent subgroup in a finite group are conjugate if and only if they are locally conjugate.

Here, two subgroups $H, H^{\prime}\leq G$ are locally conjugate if a Sylow $p$ -subgroup of $H$ is conjugate to a Sylow $p$ -subgroup of $H^{\prime}$ for each prime $p$ . It also readily follows that:

Proposition 3. Let $G$ be a finite split extension over a nilpotent subgroup $N$ such that $G/N$ is nilpotent. If for each prime $p$ , there is a Sylow $p$ -subgroup $S$ of $G$ such that any two complements of $S\cap N$ in $S$ are conjugate in $G$ , then any two complements of $N$ in $G$ are conjugate.

We then establish a fixed point result for nilpotent-by-nilpotent actions in the style of Glauberman:

Theorem 4. For finite nilpotent groups $J$ and $N$ , suppose $J$ acts on $N$ via automorphisms and that the induced semidirect product $N\rtimes J$ acts on some non-empty set $\Omega$ where the action of $N$ is transitive. If for each prime $p$ , a Sylow $p$ -subgroup of $J$ fixes an element of $\Omega$ , then $J$ fixes an element of $\Omega$ .

Glauberman showed that this result holds whenever the orders of $N$ and $J$ are coprime, without any further restrictions on $N$ or $J$ [4, Thm. 4]. Thus, this result is only interesting when $\lvert N\rvert$ and $\lvert J\rvert$ share one or more prime divisors (i.e. when the action is non-coprime). Analogous results hold if $N$ is abelian or if $N$ is nilpotent and $N\rtimes J$ is supersoluble [2].

1.1. Outline

In the remainder of this section, we introduce some notation. We then prove the results in Section 2 and conclude in Section 3.

1.2. Notation

All groups in this note are finite. For a nilpotent group $J$ , we let $J_p\in \textrm {Syl}_p(J)$ denote its unique Sylow $p$ -subgroup and $J_p^{\prime}$ denote its Hall $p^{\prime}$ -subgroup so that $J \cong J_p \times J_p^{\prime}$ . We let $g^\gamma =\gamma ^{-1}g\gamma$ for $g,\gamma \in G$ . We otherwise use standard notation from group theory that can be found in Doerk and Hawkes [3].

For a subgroup $K\leq J$ , we let $\varphi |_{K}$ denote the restriction of $\varphi \in Z^1(J,N)$ to $K$ and let $\textrm {res}^J_K \,:\, H^1(J,N) \to H^1(K,N)$ be the map induced in cohomology. For $\varphi \in Z^1(K,N)$ and $j\in J$ , define $\varphi ^j(x) = \varphi (x^{j^{-1}})^{j}$ . We say $\varphi$ is $J$ -invariant if $\textrm {res}^K_{K\cap K^j} \varphi \sim \textrm {res}^{K^j}_{K\cap K^j} \varphi ^j$ for all $j\in J$ and let $\textrm {inv}_J H^1(K,N)$ be the set of $J$ -invariant elements in $H^1(K,N)$ . For any $\varphi \in Z^1(J,N)$ , we have $\varphi ^j(x) = n^{-1} \varphi (x) n^{x^{-1}}$ where $n= \varphi (\,j^{-1})$ so that $\varphi ^j \sim \varphi$ . Consequently, $\textrm {res}^J_K H^1(J,N) \subseteq \textrm {inv}_J H^1(K, N)$ .

For nilpotent $J$ and $\varphi \in Z^1(J_p,N)$ , any $j\in J$ may be written $j=j_p\times j_p^{\prime}$ for $j_p \in J_p$ and $j_p^{\prime} \in J_p^{\prime}$ so that $\varphi ^j(x) = \varphi (x^{j_p^{-1}})^{j_pj_p^{\prime}} = \varphi ^{\prime}(x)^{j_p^{\prime}}$ for some $\varphi ^{\prime}\sim \varphi$ . It follows that $\textrm {inv}_J H^1(J_p, N) = H^1(J_p, N)^{J_p^{\prime}}$ , that is, the $J$ -invariant elements of $H^1(J_p, N)$ are those fixed under conjugation by $J_p^{\prime}$ .

To each complement $K$ of $N$ in $NJ$ , we associate $\varphi _K\in Z^1(J,N)$ as follows. For $j\in J$ , we have $j=n_j^{-1}k_j$ for unique $n_j\in N$ and $k_j\in K$ ; we then let $\varphi _K(\,j) = n_j = k_j j^{-1}$ . Conversely, for any $\varphi \in Z^1(J,N)$ , the subgroup $F(\varphi ) = \{\varphi (\,j)j\}_{j\in J}$ complements $N$ in $NJ$ . In particular, $F(\varphi _K) = K$ . Furthermore, $F(\varphi )$ and $F(\varphi ^{\prime})$ are $N$ -conjugate in $NJ$ if and only if $\varphi \sim \varphi ^{\prime}$ so that $F$ induces a correspondence between $H^1(J,N)$ and the $N$ -conjugacy classes of complements to $N$ in $NJ$ . See Serre [6, Ch. I §5] for further details on nonabelian group cohomology.

2. Proofs of results

We begin by establishing Lemma1.

Proof of Lemma 1. As $N$ is nilpotent, the natural projection maps $N \to N_p$ induce an isomorphism:

(1) \begin{equation} H^1(J,N) \cong \times _{p\in \mathcal D} H^1(J, N_p), \end{equation}

where terms $p\notin \mathcal {D}$ drop by the Schur–Zassenhaus theorem [3, Thm. A.11.3]. Thus, we may focus our attention on $H^1(J, N_q)$ for some prime $q$ . If $J$ is also a $q$ -group, we are done. Otherwise, we may consider the inflation-restriction exact sequence [6, Sec. I.5.8]:

\begin{equation*} 1 \to H^1(J_q^{\prime}, N_q^{\,J_q}) \to H^1(J,N_q) \xrightarrow {\textrm {res}^J_{J_q}} H^1(J_q,N_q)^{J_q^{\prime}}. \end{equation*}

As $H^1(J_q^{\prime}, N_q^{\,J_q})$ is trivial, $\textrm {res}^J_{J_q}$ is injective. For any $\varphi \in H^1(J_q,N_q)^{J_q^{\prime}}$ , we may define $\tilde \varphi$ in terms of a representative crossed homomorphism as $\tilde \varphi (\,j^{\prime}j) = \varphi (\,j)$ for $j\in J_q$ and $j^{\prime}\in J_q^{\prime}$ . It is straightforward to verify that $\tilde \varphi \in Z^1(J, N_q)$ and $\tilde \varphi |_{J_q} \sim \varphi$ . Thus, $\textrm {res}^J_{J_q}$ is also surjective and thus an isomorphism. Let $v\,:\, H^1(J_q, N_q) \to H^1(J_q, N)$ denote the map induced by inclusion. From the decomposition (1), $H^1(J_q, N_q) \cong H^1(J_q, N)$ , and as

\begin{equation*} H^1(J_q, N_q) \xrightarrow {\nu } H^1(J_q, N) \to H^1(J_q, N_q^{\prime}) \end{equation*}

is exact [6, Prop. I.38] where $H^1(J_q, N_q^{\prime})$ is trivial, it follows that $v$ is surjective and hence an isomorphism. As $\varphi \in H^1(J_p, N_p)^{J_p^{\prime}}$ if and only if $v(\varphi )\in H^1(J_p, N)^{J_p^{\prime}}$ , it follows that $\Phi \,:\, H^1(J,N) \to \times _{p\in \mathcal {D}} H^1(J_p, N)^{J_p^{\prime}}$ given by the composition $\varphi \mapsto \times _{p\in \mathcal {D}} \varphi |_{J_p}$ induces the desired isomorphism:

(2) \begin{equation} H^1(J,N) \cong \textstyle \times _{p\in \mathcal {D}} H^1(J_p, N_p)^{J_p^{\prime}} \cong \textstyle \times _{p\in \mathcal {D}} H^1(J_p, N)^{J_p^{\prime}}. \end{equation}

We now show how Propositions2 and 3 follow from Lemma1.

Proof of Proposition 2. Suppose $J$ and $J^{\prime}$ each complement $N\vartriangleleft G$ as described in the hypotheses of the proposition. Let $\varphi$ be a crossed homomorphism representing $J^{\prime}$ in $H^1(J,N)$ . By hypothesis, $\varphi |_{J_p}\sim 1|_{J_p}$ for every prime $p$ , where $1 \in Z^1(J,N)$ represents the distinguished point. Lemma1 implies $\varphi \sim 1$ so that $J$ and $J^{\prime}$ are conjugate.

Proof of Proposition 3. Suppose $G \cong N \rtimes J$ satisfies the hypotheses of the proposition. Fix a prime $p$ . Without loss, we may suppose any two complements of $N_p$ in $S=J_pN_p$ are conjugate in $G$ . If $J_p^{\prime}$ is such a complement, then $J_p^{\prime} = (J_p)^g$ for some $g\in G$ so that $J_p^{\prime} = (J_p)^{jn} = (J_p)^n$ for some $j\in J$ and $n\in N$ . In particular, $J_p^{\prime}$ is conjugate to $J_p$ in $J_pN$ . Thus, $H^1(J_p,N)$ is trivial. As the choice of prime $p$ was arbitrary, Lemma1 implies that $H^1(J,N)$ is also trivial, allowing us to conclude.

To prove Theorem4, we also require:

Proposition 5. Let $H$ be a subgroup of $G\cong N \rtimes J$ where $N$ and $J$ are nilpotent. If for each prime $p$ , $H$ contains a conjugate of some $J_p \in \textrm {Syl}_p(J)$ , then $H$ contains a conjugate of $J$ .

Proof of Proposition 5. It follows from the hypotheses that $H$ supplements $N$ in $G$ . We induct on the order of $G$ . If $H$ is a $p$ -group or all of $G$ , the result is immediate. If multiple primes divide $\lvert N\rvert$ , we have the nontrivial decomposition $N\cong N_p \times N_p^{\prime}$ for some prime $p$ . Induction in $G/N_p$ implies $J^{n_0} \leq H N_p$ for some $n_0\in N_p^{\prime}$ . Induction in $G/N_p^{\prime}$ implies $J^{n_1} \leq H N_p^{\prime}$ for some $n_1\in N_p$ . Thus, $J^{n_0n_1} \leq H N_p \cap HN_p^{\prime} = H$ , where the last equality proceeds from the following argument of Losey and Stonehewer [5]. Suppose $g\in H N_p \cap HN_p^{\prime}$ so that $g=h_0n_0 = h_1n_1$ for some $h_0, h_1\in H$ , $n_0\in N_p$ and $n_1\in N_p^{\prime}$ . Then $(h_1)^{-1} h_0 = n_1(n_0)^{-1} \in H$ . As $n_0$ and $n_1$ commute and have coprime orders, it follows that $n_0, n_1 \in H$ so $g\in H$ .

We now proceed under the assumption that $N=N_q$ for some prime $q$ . Upon switching to a conjugate of $H$ if necessary, we may suppose that $J_q \leq H$ . Let $Z$ denote the center of $N$ . If $Z \cap H$ were nontrivial, then induction in $G/(Z\cap H)$ would allow us to conclude. Otherwise, in $G/Z$ , induction implies that $J^gZ/Z \leq ZH/Z$ for some $g\in G$ . Let $\psi \,:\, H \to HZ/Z$ denote the isomorphism between $H$ and $HZ/Z$ . Then $K = \psi ^{-1}(J^gZ/Z)$ complements $N\cap H$ in $H$ and $N$ in $G$ . Let $\varphi \in Z^1(K,N)$ correspond to $J$ . Then $\varphi |_{K_q}$ corresponds to $J_q$ where $[\varphi |_{K_q}]\in H^1(K_q, H \cap N)^{K_q^{\prime}}\cong H^1(K, H \cap N)$ . In particular, there exists a complement, say $L$ , to $H\cap N$ in $H$ that contains $J_q$ . $L$ will also complement $N$ in $G$ , and as $\textrm {Syl}_p(L) \subseteq \textrm {Syl}_p(G)$ for all primes $p\ne q$ , we may apply Proposition2 to conclude that $J^{g^{\prime}} = L \leq H$ for some $g^{\prime} \in G$ .

With this, we are prepared to prove Theorem4.

Proof of Theorem 4. Given $J$ , $N$ , and $\Omega$ as described in the hypotheses of the theorem, let $G= N \rtimes J$ denote the induced semidirect product and consider the stabilizer subgroup $G_\alpha$ for some $\alpha \in \Omega$ . As $N$ acts transitively, $G=NG_\alpha$ . For each prime $p$ , the hypotheses of the theorem imply $(J_p)^{n_p} \leq G_\alpha$ for some $J_p \in \textrm {Syl}_p(J)$ and $n_p\in N$ so that Proposition5 allows us to conclude $J^g \leq G_\alpha$ for some $g\in G$ . It follows that $J$ fixes $g\cdot \alpha$ .

3. Conclusion

We conclude with a brief discussion of analogous results in the abelian case. For arbitrary $J$ acting on abelian $N$ , the restriction map $\textrm {res}^J_{J_p}\,:\, H^1(J,N)_{(p)} \xrightarrow {\cong } \textrm {inv}_J H^1(J_p, N)$ induces an isomorphism for each prime $p$ , where $H^1(J,N)_{(p)}$ is the $p$ -primary component of $H^1(J,N)$ and $J_p\in \textrm {Syl}_p(J)$ . Consequently, it follows from the primary decomposition of $H^1(J,N)$ that [1, Thm. III.10.3]:

(3) \begin{equation} H^1(J,N) \cong \oplus _{p\in \mathcal {D}} \textrm {inv}_J H^1(J_p, N). \end{equation}

Furthermore, for abelian $N$ , suppose $G = N \rtimes J$ acts on some non-empty set $\Omega$ , where the action of $N$ is transitive, and for each prime $p$ a Sylow $p$ -subgroup of $J$ fixes an element of $\Omega$ . Then, for arbitrary $\alpha \in \Omega$ , the stabilizer $G_\alpha$ splits over $G_\alpha \cap N$ by Gaschütz’s theorem [3, Thm. A.11.2] and is locally conjugate and thus conjugate to $J$ by an argument analogous to the proof of Proposition5. In particular, $J$ fixes an element of $\Omega$ . In this note, we find that the decomposition (3) and fixed point result continue to hold for nilpotent $N$ if $J$ is also nilpotent.