Article contents
Dynamical behavior of alternate base expansions
Published online by Cambridge University Press: 22 December 2021
Abstract
We generalize the greedy and lazy
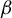 $\beta $
-transformations for a real base
$\beta $
-transformations for a real base
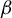 $\beta $
to the setting of alternate bases
$\beta $
to the setting of alternate bases
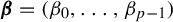 ${\boldsymbol {\beta }}=(\beta _0,\ldots ,\beta _{p-1})$
, which were recently introduced by the first and second authors as a particular case of Cantor bases. As in the real base case, these new transformations, denoted
${\boldsymbol {\beta }}=(\beta _0,\ldots ,\beta _{p-1})$
, which were recently introduced by the first and second authors as a particular case of Cantor bases. As in the real base case, these new transformations, denoted
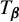 $T_{{\boldsymbol {\beta }}}$
and
$T_{{\boldsymbol {\beta }}}$
and
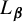 $L_{{\boldsymbol {\beta }}}$
respectively, can be iterated in order to generate the digits of the greedy and lazy
$L_{{\boldsymbol {\beta }}}$
respectively, can be iterated in order to generate the digits of the greedy and lazy
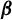 ${\boldsymbol {\beta }}$
-expansions of real numbers. The aim of this paper is to describe the measure-theoretical dynamical behaviors of
${\boldsymbol {\beta }}$
-expansions of real numbers. The aim of this paper is to describe the measure-theoretical dynamical behaviors of
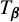 $T_{{\boldsymbol {\beta }}}$
and
$T_{{\boldsymbol {\beta }}}$
and
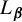 $L_{{\boldsymbol {\beta }}}$
. We first prove the existence of a unique absolutely continuous (with respect to an extended Lebesgue measure, called the p-Lebesgue measure)
$L_{{\boldsymbol {\beta }}}$
. We first prove the existence of a unique absolutely continuous (with respect to an extended Lebesgue measure, called the p-Lebesgue measure)
 $T_{{\boldsymbol {\beta }}}$
-invariant measure. We then show that this unique measure is in fact equivalent to the p-Lebesgue measure and that the corresponding dynamical system is ergodic and has entropy
$T_{{\boldsymbol {\beta }}}$
-invariant measure. We then show that this unique measure is in fact equivalent to the p-Lebesgue measure and that the corresponding dynamical system is ergodic and has entropy
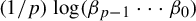 $({1}/{p})\log (\beta _{p-1}\cdots \beta _0)$
. We give an explicit expression of the density function of this invariant measure and compute the frequencies of letters in the greedy
$({1}/{p})\log (\beta _{p-1}\cdots \beta _0)$
. We give an explicit expression of the density function of this invariant measure and compute the frequencies of letters in the greedy
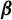 ${\boldsymbol {\beta }}$
-expansions. The dynamical properties of
${\boldsymbol {\beta }}$
-expansions. The dynamical properties of
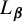 $L_{{\boldsymbol {\beta }}}$
are obtained by showing that the lazy dynamical system is isomorphic to the greedy one. We also provide an isomorphism with a suitable extension of the
$L_{{\boldsymbol {\beta }}}$
are obtained by showing that the lazy dynamical system is isomorphic to the greedy one. We also provide an isomorphism with a suitable extension of the
 $\beta $
-shift. Finally, we show that the
$\beta $
-shift. Finally, we show that the
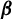 ${\boldsymbol {\beta }}$
-expansions can be seen as
${\boldsymbol {\beta }}$
-expansions can be seen as
 $(\beta _{p-1}\cdots \beta _0)$
-representations over general digit sets and we compare both frameworks.
$(\beta _{p-1}\cdots \beta _0)$
-representations over general digit sets and we compare both frameworks.
MSC classification
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 5
- Cited by



