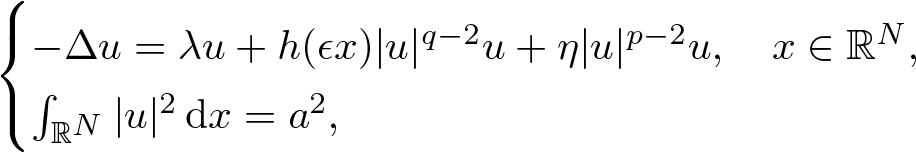1. Introduction and main results
In this paper, we study the multiplicity and stability of normalized solutions to the non-autonomous Schrödinger equation with mixed nonlinearities:
 \begin{equation}
\begin{cases}
-\Delta u=\lambda u+h(\epsilon x)|u|^{q-2}u+\eta |u|^{p-2}u,\quad
x\in \mathbb{R}^N, \\
\int_{\mathbb{R}^N}|u|^2\,\textrm{d}x=a^2,
\end{cases}
\end{equation}
\begin{equation}
\begin{cases}
-\Delta u=\lambda u+h(\epsilon x)|u|^{q-2}u+\eta |u|^{p-2}u,\quad
x\in \mathbb{R}^N, \\
\int_{\mathbb{R}^N}|u|^2\,\textrm{d}x=a^2,
\end{cases}
\end{equation} where ![]() $N\geq 1, a, \epsilon, \eta \gt 0$,
$N\geq 1, a, \epsilon, \eta \gt 0$,  $2 \lt q \lt 2 + {4 \over N} \lt p\left\{ {\matrix{ { \lt + \infty ,} \hfill & {N = 1,2,} \hfill \cr { \le {2^*}: = {{2N} \over {N - 2}},} \hfill & {N \ge 3,} \hfill \cr } } \right.$ and
$2 \lt q \lt 2 + {4 \over N} \lt p\left\{ {\matrix{ { \lt + \infty ,} \hfill & {N = 1,2,} \hfill \cr { \le {2^*}: = {{2N} \over {N - 2}},} \hfill & {N \ge 3,} \hfill \cr } } \right.$ and ![]() $\lambda\in \mathbb{R}$ is an unknown parameter that appears as a Lagrange multiplier. The function h satisfies the following conditions:
$\lambda\in \mathbb{R}$ is an unknown parameter that appears as a Lagrange multiplier. The function h satisfies the following conditions:
(h 1) ![]() $h\in C(\mathbb{R}^N,\mathbb{R})$ and
$h\in C(\mathbb{R}^N,\mathbb{R})$ and ![]() $0 \lt h_0=\inf_{x\in
\mathbb{R}^N}h(x)\leq \max_{x\in \mathbb{R}^N}h(x)=h_{\text{max}}$;
$0 \lt h_0=\inf_{x\in
\mathbb{R}^N}h(x)\leq \max_{x\in \mathbb{R}^N}h(x)=h_{\text{max}}$;
(h 2) ![]() $h_{\infty}=\lim_{|x|\to +\infty}h(x) \lt h_{\text{max}}$;
$h_{\infty}=\lim_{|x|\to +\infty}h(x) \lt h_{\text{max}}$;
(h 3) ![]() $h^{-1}(h_{\text{max}})=\{a_1,a_2,\ldots,a_l\}$ with
$h^{-1}(h_{\text{max}})=\{a_1,a_2,\ldots,a_l\}$ with ![]() $a_1=0$ and
$a_1=0$ and ![]() $a_j\neq a_i$, if i ≠ j.
$a_j\neq a_i$, if i ≠ j.
A solution u to the problem (1.1) corresponds to a critical point of the functional:
 \begin{equation}
E_{\epsilon}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x)|u|^{q}\,{\rm d}x-\frac{\eta}{p}\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x,
\end{equation}
\begin{equation}
E_{\epsilon}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x)|u|^{q}\,{\rm d}x-\frac{\eta}{p}\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x,
\end{equation}restricted to the sphere:
 \begin{equation*}
S(a):=\{u\in H^1(\mathbb{R}^N):\int_{\mathbb{R}^N}|u|^2\,\textrm{d}x=a^2\}.
\end{equation*}
\begin{equation*}
S(a):=\{u\in H^1(\mathbb{R}^N):\int_{\mathbb{R}^N}|u|^2\,\textrm{d}x=a^2\}.
\end{equation*} It is well known that ![]() $E_{\epsilon}\in
C^1(H^1(\mathbb{R}^N),\mathbb{R})$ and
$E_{\epsilon}\in
C^1(H^1(\mathbb{R}^N),\mathbb{R})$ and
 \begin{equation*}
E_{\epsilon}^\prime(u)\varphi=\int_{\mathbb{R}^N}\nabla u\nabla \varphi
\,{\rm d}x-\int_{\mathbb{R}^N}h(\epsilon x)|u|^{q-2}u\varphi
\,{\rm d}x-\eta\int_{\mathbb{R}^N}|u|^{p-2}u\varphi \,{\rm d}x,
\end{equation*}
\begin{equation*}
E_{\epsilon}^\prime(u)\varphi=\int_{\mathbb{R}^N}\nabla u\nabla \varphi
\,{\rm d}x-\int_{\mathbb{R}^N}h(\epsilon x)|u|^{q-2}u\varphi
\,{\rm d}x-\eta\int_{\mathbb{R}^N}|u|^{p-2}u\varphi \,{\rm d}x,
\end{equation*} for any ![]() $\varphi\in H^1(\mathbb{R}^N)$.
$\varphi\in H^1(\mathbb{R}^N)$.
One motivation driving the search for normalized solutions to Equation (1.1) is the nonlinear Schrödinger equation:
 \begin{equation}
i\frac{\partial \psi}{\partial t}+\Delta \psi+g(|\psi|^2)\psi=0,\quad
(t,x)\in \mathbb{R}\times\mathbb{R}^N.
\end{equation}
\begin{equation}
i\frac{\partial \psi}{\partial t}+\Delta \psi+g(|\psi|^2)\psi=0,\quad
(t,x)\in \mathbb{R}\times\mathbb{R}^N.
\end{equation} Searching for standing wave solution ![]() $\psi(t,x)=e^{-i\lambda t}u(x)$ of Equation (1.3) leads to Equation (1.1) for u if
$\psi(t,x)=e^{-i\lambda t}u(x)$ of Equation (1.3) leads to Equation (1.1) for u if ![]() $g(|s|^2)s = h(\epsilon x)|s|^{q-2}s+\eta
|s|^{p-2}s$. Since the mass
$g(|s|^2)s = h(\epsilon x)|s|^{q-2}s+\eta
|s|^{p-2}s$. Since the mass  $\int_{\mathbb{R}^N}|\psi|^2\,\textrm{d}x$ is preserved along the flow associated with (1.3), it is natural to consider the L 2-norm of u as prescribed. Moreover, the variational characterization of normalized solutions is often a strong help to analyse their orbital stability, see [Reference Bellazzini, Jeanjean and Luo7, Reference Cazenave and Lions10, Reference Soave33, Reference Soave34] and the references therein.
$\int_{\mathbb{R}^N}|\psi|^2\,\textrm{d}x$ is preserved along the flow associated with (1.3), it is natural to consider the L 2-norm of u as prescribed. Moreover, the variational characterization of normalized solutions is often a strong help to analyse their orbital stability, see [Reference Bellazzini, Jeanjean and Luo7, Reference Cazenave and Lions10, Reference Soave33, Reference Soave34] and the references therein.
In the study of normalized solutions to the Schrödinger equation:
the number ![]() $\bar{p}:= 2 + 4/ N$, labelled L 2-critical exponent, is a very important number, because in the study of Equation (1.4) using variational methods, the functional
$\bar{p}:= 2 + 4/ N$, labelled L 2-critical exponent, is a very important number, because in the study of Equation (1.4) using variational methods, the functional
 \begin{equation*}
J(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{p}\int_{\mathbb{R}^N}|u|^p\,{\rm d}x,\quad u\in
H^1(\mathbb{R}^N),
\end{equation*}
\begin{equation*}
J(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{p}\int_{\mathbb{R}^N}|u|^p\,{\rm d}x,\quad u\in
H^1(\mathbb{R}^N),
\end{equation*} is bounded from below on S(a) for the L 2-subcritical problem, i.e., ![]() $2 \lt p \lt 2 + 4/ N$. Thus, a solution of Equation (1.4) can be found as a global minimizer of
$2 \lt p \lt 2 + 4/ N$. Thus, a solution of Equation (1.4) can be found as a global minimizer of ![]() $J|_{S(a)}$, see [Reference Shibata32, Reference Stuart36]. While for the L 2-supercritical problem, i.e.,
$J|_{S(a)}$, see [Reference Shibata32, Reference Stuart36]. While for the L 2-supercritical problem, i.e., ![]() $2+ 4/N \lt p \lt 2^*$,
$2+ 4/N \lt p \lt 2^*$, ![]() $J|_{S(a)}$ is unbounded from below (and from above). Related to this case, a seminal paper due to Jeanjean [Reference Jeanjean17] exploited the mountain pass geometry to get a normalized solution, see [Reference Bartsch and de Valeriola3, Reference Bartsch and Soave4, Reference Bieganowski and Mederski8, Reference Hirata and Tanaka14, Reference Ikoma and Tanaka16, Reference Jeanjean and Lu20, Reference Jeanjean and Lu21] for more results about problems with unbounded functional. In the purely L 2-critical case (i.e.,
$J|_{S(a)}$ is unbounded from below (and from above). Related to this case, a seminal paper due to Jeanjean [Reference Jeanjean17] exploited the mountain pass geometry to get a normalized solution, see [Reference Bartsch and de Valeriola3, Reference Bartsch and Soave4, Reference Bieganowski and Mederski8, Reference Hirata and Tanaka14, Reference Ikoma and Tanaka16, Reference Jeanjean and Lu20, Reference Jeanjean and Lu21] for more results about problems with unbounded functional. In the purely L 2-critical case (i.e., ![]() $p =
2+ 4/N$), the result is delicate. Recently, Soave [Reference Soave33, Reference Soave34] considered the Schrödinger equation with double power form nonlinearity:
$p =
2+ 4/N$), the result is delicate. Recently, Soave [Reference Soave33, Reference Soave34] considered the Schrödinger equation with double power form nonlinearity:
Under different ranges of p and q, they investigated the geometric characteristics of the functional corresponding to Equation (1.5) and studied the existence, multiplicity, orbital stability and instability of the normalized solutions, see [Reference Alves, Ji and Miyagaki2, Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Jeanjean and Le19, Reference Li23, Reference Stefanov35, Reference Wei and Wu39] for more results. The multiplicity of normalized solutions to the autonomous Schrödinger equation or systems has also been considered extensively at the last years, see [Reference Alves, Ji and Miyagaki2, Reference Bartsch and de Valeriola3, Reference Bartsch and Soave5, Reference Gou and Jeanjean12, Reference Hirata and Tanaka14, Reference Ikoma and Tanaka16, Reference Jeanjean and Le19–Reference Jeanjean and Lu21, Reference Luo, Wei, Yang and Zhen27, Reference Luo, Yang and Zou28].
As to the non-autonomous Schrödinger equation:
under different assumptions on V and f, the existence of normalized solutions to (1.6) has been studied by many researchers. Ikoma and Miyamoto [Reference Ikoma and Miyamoto15] and Zhong and Zou [Reference Zhong and Zou43] considered Equation (1.6) with general L 2-subcritical nonlinearities by applying the standard concentration compactness arguments, see also [Reference Li and Zhao24, Reference Pellacci, Pistoia, Vaira and Verzini30, Reference Tang, Zhang, Zhang and Zhou37] for results to Equation (1.6) with special L 2-subcritical nonlinearities ![]() $f(u)=|u|^{p-2}u$. See [Reference Luo, Peng and Yan25, Reference Pellacci, Pistoia, Vaira and Verzini30] for results to Equation (1.6) with special L 2-critical nonlinearities, [Reference Bellazzini, Boussaïd, Jeanjean and Visciglia6, Reference Molle, Riey and Verzini29, Reference Pellacci, Pistoia, Vaira and Verzini30, Reference Tang, Zhang, Zhang and Zhou37] for results to Equation (1.6) with special L 2-supercritical nonlinearities, and [Reference Ding and Zhong11] for results to Equation (1.6) with general L 2-supercritical nonlinearities. In addition, the orbital stability of the set consisting of the normalized ground state solutions to Equation (1.6) was studied [Reference Bellazzini, Boussaïd, Jeanjean and Visciglia6, Reference Ikoma and Miyamoto15]. There are relatively few studies about the mixed problem:
$f(u)=|u|^{p-2}u$. See [Reference Luo, Peng and Yan25, Reference Pellacci, Pistoia, Vaira and Verzini30] for results to Equation (1.6) with special L 2-critical nonlinearities, [Reference Bellazzini, Boussaïd, Jeanjean and Visciglia6, Reference Molle, Riey and Verzini29, Reference Pellacci, Pistoia, Vaira and Verzini30, Reference Tang, Zhang, Zhang and Zhou37] for results to Equation (1.6) with special L 2-supercritical nonlinearities, and [Reference Ding and Zhong11] for results to Equation (1.6) with general L 2-supercritical nonlinearities. In addition, the orbital stability of the set consisting of the normalized ground state solutions to Equation (1.6) was studied [Reference Bellazzini, Boussaïd, Jeanjean and Visciglia6, Reference Ikoma and Miyamoto15]. There are relatively few studies about the mixed problem:
Li and Zhao [Reference Li and Zhao24] studied the existence and orbital stability of normalized ground state solutions to Equation (1.7) for q is L 2-subcritical and p is L 2-critical. When  $p=\frac{2N}{N-2}$, Kang and Tang [Reference Kang and Tang22] studied the existence of normalized ground state solutions to Equation (1.7) in each of these cases
$p=\frac{2N}{N-2}$, Kang and Tang [Reference Kang and Tang22] studied the existence of normalized ground state solutions to Equation (1.7) in each of these cases  $2 \lt p \lt 2+\frac{4}{N}$,
$2 \lt p \lt 2+\frac{4}{N}$,  $p=2+\frac{4}{N}$ and
$p=2+\frac{4}{N}$ and  $2+\frac{4}{N} \lt p \lt \frac{2N}{N-2}$. Moreover, the strong instability of such solutions was also studied for the case
$2+\frac{4}{N} \lt p \lt \frac{2N}{N-2}$. Moreover, the strong instability of such solutions was also studied for the case  $2+\frac{4}{N}\leq p \lt \frac{2N}{N-2}$. As to the studies about the multiplicity of normalized solutions to the non-autonomous Schrödinger equation, Yang et al. [Reference Yang, Qi and Zou42] studied Equation (1.6) with f being L 2-subcritical and satisfying Berestycki–Lions type conditions. Alves [Reference Alves1] considered the multiplicity of normalized solutions to
$2+\frac{4}{N}\leq p \lt \frac{2N}{N-2}$. As to the studies about the multiplicity of normalized solutions to the non-autonomous Schrödinger equation, Yang et al. [Reference Yang, Qi and Zou42] studied Equation (1.6) with f being L 2-subcritical and satisfying Berestycki–Lions type conditions. Alves [Reference Alves1] considered the multiplicity of normalized solutions to
with f being L 2-subcritical. As far as we know, there are no studies about multiplicity of normalized solutions to non-autonomous Schrödinger equation with mixed nonlinearities. In this paper, motivated by [Reference Alves1], we study the multiplicity of normalized solutions to Equation (1.1).
To state the main results, let us first introduce some necessary notations. For ![]() $r\geq 1$, the Lr-norm of
$r\geq 1$, the Lr-norm of ![]() $u\in
L^r(\mathbb{R}^N)$ is denoted by
$u\in
L^r(\mathbb{R}^N)$ is denoted by ![]() $\|u\|_r$. For every
$\|u\|_r$. For every ![]() $N\geq 3$, there exists an optimal constant S > 0 depending only on N such that
$N\geq 3$, there exists an optimal constant S > 0 depending only on N such that
where ![]() $D^{1,2}(\mathbb{R}^N)$ denotes the completion of
$D^{1,2}(\mathbb{R}^N)$ denotes the completion of ![]() $C_c^{\infty}(\mathbb{R}^N)$ with respect to the norm
$C_c^{\infty}(\mathbb{R}^N)$ with respect to the norm ![]() $\|u\|_{D^{1,2}}:=\|\nabla u\|_2$. Let
$\|u\|_{D^{1,2}}:=\|\nabla u\|_2$. Let
 \begin{equation*}\
2 \lt t \lt \left\{
\begin{array}{ll}
\infty, & N=1,2,\\
2^*, &N\geq 3
\end{array}
\right. \quad \text{and}\quad \gamma_t:=\frac{N}{2}-\frac{N}{t}.\end{equation*}
\begin{equation*}\
2 \lt t \lt \left\{
\begin{array}{ll}
\infty, & N=1,2,\\
2^*, &N\geq 3
\end{array}
\right. \quad \text{and}\quad \gamma_t:=\frac{N}{2}-\frac{N}{t}.\end{equation*} The Gagliardo–Nirenberg inequality (see [Reference Weinstein40]) says that there exists an optimal constant ![]() $C_{N,t} \gt 0$ depending on N and t such that
$C_{N,t} \gt 0$ depending on N and t such that
 \begin{equation}
\|u\|_{t}\leq C_{N,t}\|u\|_2^{1-\gamma_t}\|\nabla u\|_2^{\gamma_t},\quad \forall u\in H^{1}(\mathbb{R}^N).
\end{equation}
\begin{equation}
\|u\|_{t}\leq C_{N,t}\|u\|_2^{1-\gamma_t}\|\nabla u\|_2^{\gamma_t},\quad \forall u\in H^{1}(\mathbb{R}^N).
\end{equation} Note that if we let  $ C_{N,2^*}:=S^{-\frac{1}{2}}$, then (1.9) and (1.10) can be written in a unified form:
$ C_{N,2^*}:=S^{-\frac{1}{2}}$, then (1.9) and (1.10) can be written in a unified form:
 \begin{equation}
\|u\|_{t}\leq C_{N,t}\|u\|_2^{1-\gamma_t}\|\nabla u\|_2^{\gamma_t},\quad 2 \lt t\left\{
\begin{array}{ll} \lt \infty, & N=1,2,\\
\leq 2^*, &N\geq 3.
\end{array}
\right.
\end{equation}
\begin{equation}
\|u\|_{t}\leq C_{N,t}\|u\|_2^{1-\gamma_t}\|\nabla u\|_2^{\gamma_t},\quad 2 \lt t\left\{
\begin{array}{ll} \lt \infty, & N=1,2,\\
\leq 2^*, &N\geq 3.
\end{array}
\right.
\end{equation}Let p and q be as in Equation (1.1) and set
 \begin{equation}
B:=\frac{p\gamma_p-q\gamma_q}{2-q\gamma_q}\left(\frac{2-q\gamma_q}{p\gamma_p-2}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\frac{C_{N,q}^q}{q}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\frac{C_{N,p}^p}{p}\right)^{\frac{2-q\gamma_q}{p\gamma_p-q\gamma_q}}.
\end{equation}
\begin{equation}
B:=\frac{p\gamma_p-q\gamma_q}{2-q\gamma_q}\left(\frac{2-q\gamma_q}{p\gamma_p-2}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\frac{C_{N,q}^q}{q}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\frac{C_{N,p}^p}{p}\right)^{\frac{2-q\gamma_q}{p\gamma_p-q\gamma_q}}.
\end{equation}We make the following assumptions on hmax, η and a:
 \begin{equation}
\left(h_{\text{max}}a^{q(1-\gamma_q)}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\eta
a^{p(1-\gamma_p)}\right)^{\frac{2-q\gamma_q}{p\gamma_p-q\gamma_q}} \lt \frac{1}{2B},
\end{equation}
\begin{equation}
\left(h_{\text{max}}a^{q(1-\gamma_q)}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\eta
a^{p(1-\gamma_p)}\right)^{\frac{2-q\gamma_q}{p\gamma_p-q\gamma_q}} \lt \frac{1}{2B},
\end{equation}and
 \begin{equation}
\begin{split}
\left(h_{\text{max}}a^{q(1-\gamma_q)}\right)^{\frac{1}{p\gamma_p-q\gamma_q}}
&\eta^{\frac{N-2}{4}-\frac{1}{p\gamma_p-q\gamma_q}}\\
&\leq \left(\frac{(2-q\gamma_q)C_{N,q}^q2^*S^{\frac{2^*}{2}}}
{q(p-2)}\right)^{\frac{-1}{p\gamma_p-q\gamma_q}}S^{\frac{N}{4}}.
\end{split}
\end{equation}
\begin{equation}
\begin{split}
\left(h_{\text{max}}a^{q(1-\gamma_q)}\right)^{\frac{1}{p\gamma_p-q\gamma_q}}
&\eta^{\frac{N-2}{4}-\frac{1}{p\gamma_p-q\gamma_q}}\\
&\leq \left(\frac{(2-q\gamma_q)C_{N,q}^q2^*S^{\frac{2^*}{2}}}
{q(p-2)}\right)^{\frac{-1}{p\gamma_p-q\gamma_q}}S^{\frac{N}{4}}.
\end{split}
\end{equation}The multiplicity result of this paper is as follows.
Theorem 1.1. Let ![]() $N,\epsilon,a,\eta,p,q,h$ be as in Equation (1.1),
$N,\epsilon,a,\eta,p,q,h$ be as in Equation (1.1), ![]() $h_{\text{max}}, a$ and η satisfy condition (1.13). If
$h_{\text{max}}, a$ and η satisfy condition (1.13). If ![]() $p=2^*$, we further assume that Equation (1.14) holds. Then, there exists
$p=2^*$, we further assume that Equation (1.14) holds. Then, there exists ![]() $\epsilon_0 \gt 0$ such that Equation (1.1) admits at least l couples
$\epsilon_0 \gt 0$ such that Equation (1.1) admits at least l couples ![]() $(u_j,\lambda_j)\in H^1(\mathbb{R}^N)\times \mathbb{R}$ of weak solutions for
$(u_j,\lambda_j)\in H^1(\mathbb{R}^N)\times \mathbb{R}$ of weak solutions for ![]() $\epsilon\in (0,\epsilon_0)$ with
$\epsilon\in (0,\epsilon_0)$ with  $\int_{\mathbb{R}^N}|u_j|^2\,\textrm{d}x=a^2$,
$\int_{\mathbb{R}^N}|u_j|^2\,\textrm{d}x=a^2$, ![]() $\lambda_j \lt 0$ and
$\lambda_j \lt 0$ and ![]() $E_{\epsilon}(u_j) \lt 0$ for
$E_{\epsilon}(u_j) \lt 0$ for ![]() $j=1,2,\ldots,l$.
$j=1,2,\ldots,l$.
Remark 1.2. We make some notes on conditions (1.13) and (1.14):
(1) In studying the normalized solutions to the autonomous Schrödinger equation (1.5) with mixed nonlinearities  $q \lt 2+\frac{4}{N} \lt p$, the first step is to study the lower bound function of the functional to the problem (1.5) and then assume the maximum of the lower bound function is positive, see [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33, Reference Soave34] for more details. In our non-autonomous problem (1.1), we also obtain a lower bound function
$q \lt 2+\frac{4}{N} \lt p$, the first step is to study the lower bound function of the functional to the problem (1.5) and then assume the maximum of the lower bound function is positive, see [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33, Reference Soave34] for more details. In our non-autonomous problem (1.1), we also obtain a lower bound function ![]() $g_a(r)$ for the functional
$g_a(r)$ for the functional ![]() $E_{\epsilon}(u)$ and the condition (1.13) is added just to guarantee the maximum of
$E_{\epsilon}(u)$ and the condition (1.13) is added just to guarantee the maximum of ![]() $g_a(r)$ is positive (see
$g_a(r)$ is positive (see ![]() $\S$ 2) so that we can truncate the functional
$\S$ 2) so that we can truncate the functional ![]() $E_{\epsilon}(u)$ and obtain the local minimizer of the functional with negative energy. So in some sense, the condition (1.13) is very weak.
$E_{\epsilon}(u)$ and obtain the local minimizer of the functional with negative energy. So in some sense, the condition (1.13) is very weak.
(2) When ![]() $p=2^*$, the condition (1.14) is added for technical reasons in order to obtain the PS condition for the truncated functional
$p=2^*$, the condition (1.14) is added for technical reasons in order to obtain the PS condition for the truncated functional ![]() $E_{\epsilon,T}(u)$ (see Lemmas 4.4) and it can be weakened if we can give the explicit expression of R 0 (see Lemma 4.4).
$E_{\epsilon,T}(u)$ (see Lemmas 4.4) and it can be weakened if we can give the explicit expression of R 0 (see Lemma 4.4).
To prove Theorem 1.1, we follow the arguments of [Reference Alves1] (see also [Reference Cao and Noussair9]). In [Reference Alves1], multiple solutions to the problem (1.8) are perturbed from the global minimizer of the functional  $\tilde{J}|_{S(a)}$ corresponding to the limit problem:
$\tilde{J}|_{S(a)}$ corresponding to the limit problem:
There the arguments depend on the existence of a global minimizer and the relative compactness of any minimizing sequence of the functional  $\tilde{J}|_{S(a)}$. While in our problem (1.1), the appearance of the L 2-supercritical term
$\tilde{J}|_{S(a)}$. While in our problem (1.1), the appearance of the L 2-supercritical term ![]() $\eta|u|^{p-2}u$ implies that the functional to the limit problem:
$\eta|u|^{p-2}u$ implies that the functional to the limit problem:
with ![]() $q \lt 2+4/N \lt p$ is unbounded from below (and from above). In view of the studies of [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33], we know that the functional in this case has a local minimizer. So we try to perturb multiple solutions to Equation (1.1) from the local minimizer of the limit problem (1.16). If we restrict the limit functional to a bounded region to obtain the local minimizer as did in articles [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33], we cannot obtain the connection of the functional to Equation (1.1) and the limit functional to Equation (1.16) for the appearance of the potential. To avoid this difficulty, we employ the truncated skill used in [Reference Alves, Ji and Miyagaki2, Reference Peral Alonso31], and then we can isolate the local minimizer and finally obtain the multiplicity of normalized solutions to the problem (1.1). The application of truncated functions and the appearance of the Sobolev critical exponent
$q \lt 2+4/N \lt p$ is unbounded from below (and from above). In view of the studies of [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33], we know that the functional in this case has a local minimizer. So we try to perturb multiple solutions to Equation (1.1) from the local minimizer of the limit problem (1.16). If we restrict the limit functional to a bounded region to obtain the local minimizer as did in articles [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33], we cannot obtain the connection of the functional to Equation (1.1) and the limit functional to Equation (1.16) for the appearance of the potential. To avoid this difficulty, we employ the truncated skill used in [Reference Alves, Ji and Miyagaki2, Reference Peral Alonso31], and then we can isolate the local minimizer and finally obtain the multiplicity of normalized solutions to the problem (1.1). The application of truncated functions and the appearance of the Sobolev critical exponent ![]() $p=2^*$ make the analysis challenging. In [Reference Alves, Ji and Miyagaki2], the authors studied the multiplicity of normalized solutions to the autonomous Schrödinger equation (1.16) with
$p=2^*$ make the analysis challenging. In [Reference Alves, Ji and Miyagaki2], the authors studied the multiplicity of normalized solutions to the autonomous Schrödinger equation (1.16) with ![]() $q \lt 2+4/N \lt p=2^*$ in the radially symmetric space
$q \lt 2+4/N \lt p=2^*$ in the radially symmetric space ![]() $H_{rad}^1(\mathbb{R}^N)$ by using truncated skill and genus theory. Note that our problem (1.1) is non-autonomous and not radially symmetric, so their method does not work in our problem.
$H_{rad}^1(\mathbb{R}^N)$ by using truncated skill and genus theory. Note that our problem (1.1) is non-autonomous and not radially symmetric, so their method does not work in our problem.
We also consider the stability of the solutions obtained in Theorem 1.1. For this aim, we give the definition of stability.
Definition 1.3. A set ![]() $\Omega\subset H^1(\mathbb{R}^N)$ is stable under the flow associated with the problem:
$\Omega\subset H^1(\mathbb{R}^N)$ is stable under the flow associated with the problem:
 \begin{equation}
\begin{cases}
i\frac{\partial \psi}{\partial t}+\Delta \psi+h(\epsilon
x)|\psi|^{q-2}\psi+\eta |\psi|^{p-2}\psi=0,\quad t \gt 0,x\in\mathbb{R}^N,\\
\psi(0,x)=u_0(x)
\end{cases}
\end{equation}
\begin{equation}
\begin{cases}
i\frac{\partial \psi}{\partial t}+\Delta \psi+h(\epsilon
x)|\psi|^{q-2}\psi+\eta |\psi|^{p-2}\psi=0,\quad t \gt 0,x\in\mathbb{R}^N,\\
\psi(0,x)=u_0(x)
\end{cases}
\end{equation} if for any θ > 0 there exists γ > 0 such that for any ![]() $u_0\in H^1(\mathbb{R}^N)$ satisfying
$u_0\in H^1(\mathbb{R}^N)$ satisfying
 \begin{equation*}
\text{dist}_{H^1(\mathbb{R}^N)}(u_0,\Omega) \lt \gamma,
\end{equation*}
\begin{equation*}
\text{dist}_{H^1(\mathbb{R}^N)}(u_0,\Omega) \lt \gamma,
\end{equation*} the solution ![]() $\psi(t, \cdot)$ of problem (1.17) with
$\psi(t, \cdot)$ of problem (1.17) with ![]() $\psi(0,
x) =u_0$ satisfies
$\psi(0,
x) =u_0$ satisfies
 \begin{equation*}
\sup_{t\in
\mathbb{R}^+}\text{dist}_{H^1(\mathbb{R}^N)}(\psi(t,\cdot),\Omega) \lt \theta.
\end{equation*}
\begin{equation*}
\sup_{t\in
\mathbb{R}^+}\text{dist}_{H^1(\mathbb{R}^N)}(\psi(t,\cdot),\Omega) \lt \theta.
\end{equation*}Theorem 1.4. Let ![]() $N,a,\eta,q,h$ and ϵ 0 be as in Theorem 1.1,
$N,a,\eta,q,h$ and ϵ 0 be as in Theorem 1.1, ![]() $p \lt 2^*$,
$p \lt 2^*$, ![]() $\epsilon\in (0,\epsilon_0)$, (1.13) hold. Then,
$\epsilon\in (0,\epsilon_0)$, (1.13) hold. Then, ![]() $\Omega_i \, (i=1,\ldots,l)$ is stable under the flow associated with the problem (1.17), where
$\Omega_i \, (i=1,\ldots,l)$ is stable under the flow associated with the problem (1.17), where ![]() $\Omega_i$ is defined in Equation (5.1). Since the definition of
$\Omega_i$ is defined in Equation (5.1). Since the definition of ![]() $\Omega_i$ needs many notations used in the proof of Theorem 1.1 in
$\Omega_i$ needs many notations used in the proof of Theorem 1.1 in ![]() $\S$ 2–4, so we do not give it here.
$\S$ 2–4, so we do not give it here.
Theorem 1.5. Let ![]() $N,a,\eta,q,h$ and ϵ 0 be as in Theorem 1.1,
$N,a,\eta,q,h$ and ϵ 0 be as in Theorem 1.1, ![]() $p=2^*$,
$p=2^*$, ![]() $\epsilon\in(0,\epsilon_0)$, (1.13) and (1.14) hold. Further assume that
$\epsilon\in(0,\epsilon_0)$, (1.13) and (1.14) hold. Further assume that ![]() $h(x)\in C^1(\mathbb{R}^N)$ and
$h(x)\in C^1(\mathbb{R}^N)$ and ![]() $h^\prime(x)\in
L^{\infty}(\mathbb{R}^N)$. Then,
$h^\prime(x)\in
L^{\infty}(\mathbb{R}^N)$. Then, ![]() $\Omega_i \, (i=1,\ldots,l)$ is stable under the flow associated with the problem (1.17).
$\Omega_i \, (i=1,\ldots,l)$ is stable under the flow associated with the problem (1.17).
Remark 1.6. (1) The definition of the sets ![]() $\Omega_i \, (i=1,\ldots,l)$ seems so natural. They act like potential wells to attract solutions that start around them and guarantee the stability, see the proof of Theorem 1.4 in
$\Omega_i \, (i=1,\ldots,l)$ seems so natural. They act like potential wells to attract solutions that start around them and guarantee the stability, see the proof of Theorem 1.4 in ![]() $\S$ 5 which is very interesting.
$\S$ 5 which is very interesting.
(2) In this paper, the solutions obtained in Theorem 1.1 are local minimizers and they are probably not ground state solutions, since the potential h does not have symmetry and thus these l different solutions probably have different energies. So in some sense, we obtain the stability of local minimizer set ![]() $\Omega_i$, which is different from most of the existing results concerning the stability of ground state solutions set (see [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Luo26, Reference Soave33]). Moreover, by the definition of
$\Omega_i$, which is different from most of the existing results concerning the stability of ground state solutions set (see [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Luo26, Reference Soave33]). Moreover, by the definition of ![]() $\Omega_i$, we know
$\Omega_i$, we know ![]() $\Omega_i\cap \Omega_j=\emptyset$ for i ≠ j. So we obtain the stability of l different sets
$\Omega_i\cap \Omega_j=\emptyset$ for i ≠ j. So we obtain the stability of l different sets ![]() $\Omega_i \, (i=1,\ldots,l)$, which is very different from the existing results about the stability of the only one set of ground state solutions.
$\Omega_i \, (i=1,\ldots,l)$, which is very different from the existing results about the stability of the only one set of ground state solutions.
(3) For the limit problem (1.16) with ![]() $q \lt 2+4/N \lt p$, authors [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33] obtained a normalized local minimizer to (1.16) with negative energy which is also a ground state solution and the ground state solutions set is stable. In view of the results of this paper, it seems that the appearance of the potential h increases the numbers of the local minimizers and the numbers of the stable sets.
$q \lt 2+4/N \lt p$, authors [Reference Jeanjean, Jendrej, Le and Visciglia18, Reference Soave33] obtained a normalized local minimizer to (1.16) with negative energy which is also a ground state solution and the ground state solutions set is stable. In view of the results of this paper, it seems that the appearance of the potential h increases the numbers of the local minimizers and the numbers of the stable sets.
This paper is organized as follows. In ![]() $\S$ 2, we define the truncated functional used in the study. In
$\S$ 2, we define the truncated functional used in the study. In ![]() $\S$ 3, we study the properties of the truncated autonomous functional. In
$\S$ 3, we study the properties of the truncated autonomous functional. In ![]() $\S$ 4, we study the truncated non-autonomous problem and give the proof of Theorem 1.1. In
$\S$ 4, we study the truncated non-autonomous problem and give the proof of Theorem 1.1. In ![]() $\S$ 5, we prove the stability results Theorem 1.4.
$\S$ 5, we prove the stability results Theorem 1.4.
Notation: The usual norm of ![]() $u\in
H^1(\mathbb{R}^N)$ is denoted by
$u\in
H^1(\mathbb{R}^N)$ is denoted by ![]() $\|u\|$.
$\|u\|$. ![]() $C,C_1,C_2,\ldots$ denotes any positive constant, whose value is not relevant and maybe change from line to line.
$C,C_1,C_2,\ldots$ denotes any positive constant, whose value is not relevant and maybe change from line to line. ![]() $o_n(1)$ denotes a real sequence with
$o_n(1)$ denotes a real sequence with ![]() $o_n(1) \to
0$ as
$o_n(1) \to
0$ as ![]() $n\to +\infty$. ‘
$n\to +\infty$. ‘![]() $\rightarrow$’ denotes strong convergence and ‘
$\rightarrow$’ denotes strong convergence and ‘![]() $\rightharpoonup$’ denotes weak convergence.
$\rightharpoonup$’ denotes weak convergence. ![]() $B_{r}(x_0):=\{x\in\mathbb{R}^N:|x-x_0| \lt r\}$.
$B_{r}(x_0):=\{x\in\mathbb{R}^N:|x-x_0| \lt r\}$.
2. Truncated functionals
In the proof of Theorem 1.1, we will adapt for our case a truncated function found in Peral Alonso ([Reference Peral Alonso31], Chapter 2, Theorem 2.4.6).
In what follows, we will consider the functional Eϵ given by Equation (1.2) restricted to S(a). By the Sobolev inequality and the Gagliardo–Nirenberg inequality (1.11), we have
 \begin{equation}
\begin{split}
E_{\epsilon}(u)&\geq \frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}h_{{\rm max}}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x-\frac{\eta}{p}\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x\\
&\geq \frac{1}{2}\|\nabla
u\|_2^2-\frac{1}{q}h_{{\rm max}}C_{N,q}^qa^{q(1-\gamma_q)}\|\nabla
u\|_2^{q\gamma_q}-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}\|\nabla
u\|_2^{p\gamma_p}\\
&=g_a(\|\nabla u\|_2),
\end{split}
\end{equation}
\begin{equation}
\begin{split}
E_{\epsilon}(u)&\geq \frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}h_{{\rm max}}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x-\frac{\eta}{p}\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x\\
&\geq \frac{1}{2}\|\nabla
u\|_2^2-\frac{1}{q}h_{{\rm max}}C_{N,q}^qa^{q(1-\gamma_q)}\|\nabla
u\|_2^{q\gamma_q}-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}\|\nabla
u\|_2^{p\gamma_p}\\
&=g_a(\|\nabla u\|_2),
\end{split}
\end{equation} for any ![]() $u\in S(a)$, where
$u\in S(a)$, where
 \begin{equation*}
g_a(r):=\frac{1}{2}r^2-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}r^{q\gamma_q}
-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}r^{p\gamma_p},\quad r \gt 0.
\end{equation*}
\begin{equation*}
g_a(r):=\frac{1}{2}r^2-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}r^{q\gamma_q}
-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}r^{p\gamma_p},\quad r \gt 0.
\end{equation*} Set ![]() $g_a(r)=r^2w_a(r)$ with
$g_a(r)=r^2w_a(r)$ with
 \begin{equation*}
w_a(r):=\frac{1}{2}-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}r^{q\gamma_q-2}
-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}r^{p\gamma_p-2},\quad r \gt 0.
\end{equation*}
\begin{equation*}
w_a(r):=\frac{1}{2}-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}r^{q\gamma_q-2}
-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}r^{p\gamma_p-2},\quad r \gt 0.
\end{equation*} Now we study the properties of ![]() $w_a(r)$. Note that
$w_a(r)$. Note that
 \begin{equation*}
t\gamma_t\left\{
\begin{array}{ll} \lt 2, & 2 \lt t \lt 2+4/N,\\
=2, & t=2+4/N,\\ \gt 2, & 2+4/N \lt t\leq 2^*
\end{array}
\right.\qquad\text{and}\qquad \gamma_{2^*}=1.
\end{equation*}
\begin{equation*}
t\gamma_t\left\{
\begin{array}{ll} \lt 2, & 2 \lt t \lt 2+4/N,\\
=2, & t=2+4/N,\\ \gt 2, & 2+4/N \lt t\leq 2^*
\end{array}
\right.\qquad\text{and}\qquad \gamma_{2^*}=1.
\end{equation*} It is obvious that ![]() $\lim_{r\to 0^+}w_a(r)=-\infty$ and
$\lim_{r\to 0^+}w_a(r)=-\infty$ and ![]() $\lim_{r\to
+\infty}w_a(r)=-\infty$. By direct calculations, we obtain that
$\lim_{r\to
+\infty}w_a(r)=-\infty$. By direct calculations, we obtain that
 \begin{equation*}
w_a^\prime(r)=-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}(q\gamma_q-2)r^{q\gamma_q-3}
-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}(p\gamma_p-2)r^{p\gamma_p-3}.
\end{equation*}
\begin{equation*}
w_a^\prime(r)=-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}(q\gamma_q-2)r^{q\gamma_q-3}
-\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}(p\gamma_p-2)r^{p\gamma_p-3}.
\end{equation*} Then, the equation ![]() $w_a^\prime(r)=0$ has a unique solution:
$w_a^\prime(r)=0$ has a unique solution:
 \begin{equation}
r_0=\left(\frac{(2-q\gamma_q)\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}}
{(p\gamma_p-2)\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}}\right)^{\frac{1}{p\gamma_p-q\gamma_q}},
\end{equation}
\begin{equation}
r_0=\left(\frac{(2-q\gamma_q)\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}}
{(p\gamma_p-2)\frac{\eta}{p}C_{N,p}^pa^{p(1-\gamma_p)}}\right)^{\frac{1}{p\gamma_p-q\gamma_q}},
\end{equation} and the maximum of ![]() $w_a(r)$ is
$w_a(r)$ is
 \begin{equation*}
w_a(r_0)=\frac{1}{2}-B\left(h_{\text{max}}a^{q(1-\gamma_q)}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\eta
a^{p(1-\gamma_p)}\right)^{\frac{2-q\gamma_q}{p\gamma_p-q\gamma_q}},
\end{equation*}
\begin{equation*}
w_a(r_0)=\frac{1}{2}-B\left(h_{\text{max}}a^{q(1-\gamma_q)}\right)^{\frac{p\gamma_p-2}{p\gamma_p-q\gamma_q}}
\left(\eta
a^{p(1-\gamma_p)}\right)^{\frac{2-q\gamma_q}{p\gamma_p-q\gamma_q}},
\end{equation*} where B is defined in Equation (1.12). Thus under the assumption (1.13), the maximum of ![]() $w_a(r)$ is positive and
$w_a(r)$ is positive and ![]() $w_a(r)$ has exactly two zeros
$w_a(r)$ has exactly two zeros ![]() $0 \lt R_0 \lt R_1 \lt \infty$, which are also the zeros of
$0 \lt R_0 \lt R_1 \lt \infty$, which are also the zeros of ![]() $g_a(r)$. It is obvious that
$g_a(r)$. It is obvious that ![]() $g_a(r)$ has the following properties:
$g_a(r)$ has the following properties:
 \begin{equation}
\begin{cases}
&g_a(0)=g_a(R_0)=g_a(R_1)=0;\quad g_a(r) \lt 0\ \text{for} \ r \gt 0\
\text{small};\\
&\lim_{r\to+\infty}g_a(r)=-\infty;\quad g_a(r)\ \text{has}\ \text{exactly}\
\text{two}\
\text{critical}\ \text{points;} \ \\
&r_1\in (0,R_0)\ \text{and}\ r_2\in (R_0,R_1)\quad \text{with}\
g_a(r_1) \lt 0 \ \text{and}\ g_a(r_2) \gt 0.
\end{cases}
\end{equation}
\begin{equation}
\begin{cases}
&g_a(0)=g_a(R_0)=g_a(R_1)=0;\quad g_a(r) \lt 0\ \text{for} \ r \gt 0\
\text{small};\\
&\lim_{r\to+\infty}g_a(r)=-\infty;\quad g_a(r)\ \text{has}\ \text{exactly}\
\text{two}\
\text{critical}\ \text{points;} \ \\
&r_1\in (0,R_0)\ \text{and}\ r_2\in (R_0,R_1)\quad \text{with}\
g_a(r_1) \lt 0 \ \text{and}\ g_a(r_2) \gt 0.
\end{cases}
\end{equation} Now fix ![]() $\tau: (0,+\infty)\to [0,1]$ as being a non-increasing and
$\tau: (0,+\infty)\to [0,1]$ as being a non-increasing and ![]() $C^\infty$ function that satisfies:
$C^\infty$ function that satisfies:
 \begin{equation}
\tau(x)=\left\{
\begin{array}{ll}
1, &\text{if}\ x\leq R_0\\
0, &\text{if}\ x\geq R_1
\end{array},
\right.
\end{equation}
\begin{equation}
\tau(x)=\left\{
\begin{array}{ll}
1, &\text{if}\ x\leq R_0\\
0, &\text{if}\ x\geq R_1
\end{array},
\right.
\end{equation}and consider the truncated functional:
 \begin{equation}
E_{\epsilon,T}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x)|u|^{q}\,{\rm d}x-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x.
\end{equation}
\begin{equation}
E_{\epsilon,T}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x)|u|^{q}\,{\rm d}x-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x.
\end{equation}Thus,
 \begin{equation}
\begin{split}
E_{\epsilon,T}(u)&\geq \frac{1}{2}\|\nabla
u\|_2^2-\frac{1}{q}h_{{\rm max}}C_{N,q}^qa^{q(1-\gamma_q)}\|\nabla
u\|_2^{q\gamma_q}\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
u\|_2)C_{N,p}^pa^{p(1-\gamma_p)}\|\nabla
u\|_2^{p\gamma_p}\\
&=\bar{g}_a(\|\nabla u\|_2),
\end{split}
\end{equation}
\begin{equation}
\begin{split}
E_{\epsilon,T}(u)&\geq \frac{1}{2}\|\nabla
u\|_2^2-\frac{1}{q}h_{{\rm max}}C_{N,q}^qa^{q(1-\gamma_q)}\|\nabla
u\|_2^{q\gamma_q}\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
u\|_2)C_{N,p}^pa^{p(1-\gamma_p)}\|\nabla
u\|_2^{p\gamma_p}\\
&=\bar{g}_a(\|\nabla u\|_2),
\end{split}
\end{equation} for any ![]() $u\in S(a)$, where
$u\in S(a)$, where
 \begin{equation*}
\bar{g}_a(r):=\frac{1}{2}r^2-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}r^{q\gamma_q}
-\frac{\eta}{p}\tau(r)C_{N,p}^pa^{p(1-\gamma_p)}r^{p\gamma_p}.
\end{equation*}
\begin{equation*}
\bar{g}_a(r):=\frac{1}{2}r^2-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}r^{q\gamma_q}
-\frac{\eta}{p}\tau(r)C_{N,p}^pa^{p(1-\gamma_p)}r^{p\gamma_p}.
\end{equation*} It is easy to see that ![]() $\bar{g}_a(r)$ has the following properties:
$\bar{g}_a(r)$ has the following properties:
 \begin{equation}
\begin{cases}
&\bar{g}_a(r)\equiv {g}_a(r)\ \text{for}\ \text{all}\ r\in [0,R_0];\\
&\bar{g}_a(r)\ \text{is}\ \text{positive}\ \text{and}\ \text{strictly}\ \text{increasing}\ \text{in}\
(R_0,+\infty).
\end{cases}
\end{equation}
\begin{equation}
\begin{cases}
&\bar{g}_a(r)\equiv {g}_a(r)\ \text{for}\ \text{all}\ r\in [0,R_0];\\
&\bar{g}_a(r)\ \text{is}\ \text{positive}\ \text{and}\ \text{strictly}\ \text{increasing}\ \text{in}\
(R_0,+\infty).
\end{cases}
\end{equation} Correspondingly, for any ![]() $\mu\in (0,h_{\text{max}}]$, we denote by
$\mu\in (0,h_{\text{max}}]$, we denote by ![]() $J_{\mu},\ J_{\mu,T}: H^1(\mathbb{R}^N)\to\mathbb{R}$ the following functionals:
$J_{\mu},\ J_{\mu,T}: H^1(\mathbb{R}^N)\to\mathbb{R}$ the following functionals:
 \begin{equation}
J_{\mu}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x-\frac{\eta}{p}\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x
\end{equation}
\begin{equation}
J_{\mu}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x-\frac{\eta}{p}\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x
\end{equation}and
 \begin{equation}
J_{\mu,T}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x.
\end{equation}
\begin{equation}
J_{\mu,T}(u):=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x.
\end{equation} The properties of ![]() $J_{\mu,T}$ and
$J_{\mu,T}$ and ![]() $E_{\epsilon,T}$ will be studied in
$E_{\epsilon,T}$ will be studied in ![]() $\S$ 3 and 4, respectively.
$\S$ 3 and 4, respectively.
3. The truncated autonomous functional
In this section, we study the properties of the functional ![]() $J_{\mu,T}$ defined in Equation (2.9) restricted to
$J_{\mu,T}$ defined in Equation (2.9) restricted to ![]() $S(a_1)$, where
$S(a_1)$, where ![]() $\mu\in(0,h_{\text{max}}]$ and
$\mu\in(0,h_{\text{max}}]$ and ![]() $a_1\in (0,a]$.
$a_1\in (0,a]$.
Lemma 3.1. Let ![]() $N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold,
$N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold, ![]() $\mu\in (0,h_{\text{max}}]$,
$\mu\in (0,h_{\text{max}}]$, ![]() $0 \lt a_1\leq a$. Then the functional
$0 \lt a_1\leq a$. Then the functional ![]() $J_{\mu,T}$ is bounded from below on
$J_{\mu,T}$ is bounded from below on ![]() $S(a_1)$.
$S(a_1)$.
Proof. By Equations (2.6) and (2.7), for any ![]() $u\in S(a_1)$,
$u\in S(a_1)$,
 \begin{equation*}
\begin{split}
J_{\mu,T}(u)&\geq J_{{\rm max},T}(u) \\
& \geq \frac{1}{2}\|\nabla
u\|_2^2-\frac{1}{q}h_{{\rm max}}C_{N,q}^qa_1^{q(1-\gamma_q)}\|\nabla
u\|_2^{q\gamma_q}\\
&\qquad\qquad-\frac{\eta}{p}\tau(\|\nabla
u\|_2)C_{N,p}^pa_1^{p(1-\gamma_p)}\|\nabla u\|_2^{p\gamma_p}\\
&\geq \bar{g}_a(\|\nabla u\|_2)\geq \inf_{r\geq
0}\bar{g}_a(r) \gt -\infty.
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
J_{\mu,T}(u)&\geq J_{{\rm max},T}(u) \\
& \geq \frac{1}{2}\|\nabla
u\|_2^2-\frac{1}{q}h_{{\rm max}}C_{N,q}^qa_1^{q(1-\gamma_q)}\|\nabla
u\|_2^{q\gamma_q}\\
&\qquad\qquad-\frac{\eta}{p}\tau(\|\nabla
u\|_2)C_{N,p}^pa_1^{p(1-\gamma_p)}\|\nabla u\|_2^{p\gamma_p}\\
&\geq \bar{g}_a(\|\nabla u\|_2)\geq \inf_{r\geq
0}\bar{g}_a(r) \gt -\infty.
\end{split}
\end{equation*}The proof is complete.
Lemma 3.2. Let ![]() $N, a,\eta,p,q$ be as in Equations (1.1) and (1.13) hold,
$N, a,\eta,p,q$ be as in Equations (1.1) and (1.13) hold, ![]() $\mu\in (0,h_{\text{max}}]$,
$\mu\in (0,h_{\text{max}}]$, ![]() $0 \lt a_1\leq a$.
$0 \lt a_1\leq a$. ![]() $\Upsilon_{\mu,T,a_1}:=\inf_{u\in S(a_1)}J_{\mu,T}(u) \lt 0$.
$\Upsilon_{\mu,T,a_1}:=\inf_{u\in S(a_1)}J_{\mu,T}(u) \lt 0$.
Proof. Fix ![]() $u\in S(a_1)$. For t > 0, we define
$u\in S(a_1)$. For t > 0, we define  $u_t(x)=t^{\frac{N}{2}}u(tx)$. Then,
$u_t(x)=t^{\frac{N}{2}}u(tx)$. Then, ![]() $u_t\in S(a_1)$ for all t > 0. By
$u_t\in S(a_1)$ for all t > 0. By ![]() $\tau\geq 0$ and
$\tau\geq 0$ and ![]() $q\gamma_q \lt 2$, we obtain that
$q\gamma_q \lt 2$, we obtain that
 \begin{equation*}
\begin{split}
J_{\mu,T}(u_t)&\leq \frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u_t|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|u_t|^{q}\,{\rm d}x\\
&=\frac{1}{2}t^2\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{\mu}{q}t^{q\gamma_q}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x,\\
& \lt 0
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
J_{\mu,T}(u_t)&\leq \frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u_t|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|u_t|^{q}\,{\rm d}x\\
&=\frac{1}{2}t^2\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{\mu}{q}t^{q\gamma_q}\int_{\mathbb{R}^N}|u|^{q}\,{\rm d}x,\\
& \lt 0
\end{split}
\end{equation*} for t > 0 small enough. Thus, ![]() $\Upsilon_{\mu,T,a_1} \lt 0$. The proof is complete.
$\Upsilon_{\mu,T,a_1} \lt 0$. The proof is complete.
Lemma 3.3. Let ![]() $N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold,
$N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold, ![]() $\mu\in (0,h_{\text{max}}]$. Then
$\mu\in (0,h_{\text{max}}]$. Then
(1) ![]() $J_{\mu,T}\in C^1(H^1(\mathbb{R}^N),\mathbb{R})$;
$J_{\mu,T}\in C^1(H^1(\mathbb{R}^N),\mathbb{R})$;
(2) Let ![]() $a_1\in (0,a]$. If
$a_1\in (0,a]$. If ![]() $u\in S(a_1)$ such that
$u\in S(a_1)$ such that ![]() $J_{\mu,T}(u) \lt 0$, then
$J_{\mu,T}(u) \lt 0$, then ![]() $\|\nabla u\|_2 \lt R_0$ and
$\|\nabla u\|_2 \lt R_0$ and ![]() $J_{\mu,T}(v) = J_{\mu} (v)$ for all v satisfying
$J_{\mu,T}(v) = J_{\mu} (v)$ for all v satisfying ![]() $\|v\|_2\leq a$ and being in a small neighborhood of u in
$\|v\|_2\leq a$ and being in a small neighborhood of u in ![]() $H^1(\mathbb{R}^N)$.
$H^1(\mathbb{R}^N)$.
Proof. (1) is trivial. Now we prove (2). It follows from ![]() $J_{\mu,T}(u) \lt 0$ and
$J_{\mu,T}(u) \lt 0$ and
that ![]() $\bar{g}_a(\|\nabla u\|_2) \lt 0$, which implies that
$\bar{g}_a(\|\nabla u\|_2) \lt 0$, which implies that ![]() $\|\nabla u\|_2 \lt R_0$ by (2.7). By (1) and
$\|\nabla u\|_2 \lt R_0$ by (2.7). By (1) and ![]() $J_{\mu,T}(u) \lt 0$, we obtain that
$J_{\mu,T}(u) \lt 0$, we obtain that ![]() $J_{\mu,T}(v) \lt 0$ for all v in a small neighborhood of u in
$J_{\mu,T}(v) \lt 0$ for all v in a small neighborhood of u in ![]() $H^1 (\mathbb{R}^N)$, which combined with
$H^1 (\mathbb{R}^N)$, which combined with ![]() $\|v\|_2\leq a$ gives that
$\|v\|_2\leq a$ gives that ![]() $\|\nabla v\|_2 \lt R_0$ and thus
$\|\nabla v\|_2 \lt R_0$ and thus ![]() $J_{\mu,T}(v) = J_{\mu} (v)$. The proof is complete.
$J_{\mu,T}(v) = J_{\mu} (v)$. The proof is complete.
For any ![]() $a_1\in (0,a]$, we define
$a_1\in (0,a]$, we define
 \begin{equation*}
m_{\mu}(a_1):=\inf_{u\in V(a_1)}J_\mu(u),\ V(a_1):=\{u\in
S(a_1):\|\nabla u\|_2 \lt R_0\}.
\end{equation*}
\begin{equation*}
m_{\mu}(a_1):=\inf_{u\in V(a_1)}J_\mu(u),\ V(a_1):=\{u\in
S(a_1):\|\nabla u\|_2 \lt R_0\}.
\end{equation*} Since ![]() $J_{\mu,T}(u)\geq \bar{g}_{a_1}(\|\nabla u\|_2)\geq
\bar{g}_{a}(\|\nabla u\|_2)$ for any
$J_{\mu,T}(u)\geq \bar{g}_{a_1}(\|\nabla u\|_2)\geq
\bar{g}_{a}(\|\nabla u\|_2)$ for any ![]() $u\in S(a_1)$, by Lemma 3.1, we obtain that
$u\in S(a_1)$, by Lemma 3.1, we obtain that
 \begin{equation}
\Upsilon_{\mu,T,a_1}=\inf_{u\in S(a_1)}J_{\mu,T}(u)=m_{\mu}(a_1).
\end{equation}
\begin{equation}
\Upsilon_{\mu,T,a_1}=\inf_{u\in S(a_1)}J_{\mu,T}(u)=m_{\mu}(a_1).
\end{equation}In ([Reference Jeanjean, Jendrej, Le and Visciglia18], Lemma 2.6 and Theorem 1.2), the authors obtained that
Lemma 3.4. Let ![]() $N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold,
$N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold, ![]() $\mu\in (0,h_{\text{max}}]$. Then
$\mu\in (0,h_{\text{max}}]$. Then
(1) ![]() $a_1\in (0,a]\mapsto m_{\mu}(a_1)$ is continuous;
$a_1\in (0,a]\mapsto m_{\mu}(a_1)$ is continuous;
(2) Let ![]() $0 \lt a_1 \lt a_2\leq a$, then
$0 \lt a_1 \lt a_2\leq a$, then  $\frac{a_1^2}{a_2^2}m_{\mu}(a_2) \lt m_{\mu}(a_1) \lt 0$.
$\frac{a_1^2}{a_2^2}m_{\mu}(a_2) \lt m_{\mu}(a_1) \lt 0$.
Consequently, by Equation (3.1) and Lemma 3.4, we obtain that
Lemma 3.5. Let ![]() $N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold,
$N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold, ![]() $\mu\in (0,h_{\text{max}}]$. Then
$\mu\in (0,h_{\text{max}}]$. Then
(1) ![]() $a_1\in (0,a]\mapsto \Upsilon_{\mu,T,a_1}$ is continuous;
$a_1\in (0,a]\mapsto \Upsilon_{\mu,T,a_1}$ is continuous;
(2) Let ![]() $0 \lt a_1 \lt a_2\leq a$, then
$0 \lt a_1 \lt a_2\leq a$, then  $\frac{a_1^2}{a_2^2}\Upsilon_{\mu,T,a_2} \lt \Upsilon_{\mu,T,a_1} \lt 0$.
$\frac{a_1^2}{a_2^2}\Upsilon_{\mu,T,a_2} \lt \Upsilon_{\mu,T,a_1} \lt 0$.
The next compactness lemma is useful in the study of the autonomous problem as well as in the non-autonomous problem.
Lemma 3.6. Let ![]() $N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold,
$N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold, ![]() $\mu\in (0,h_{\text{max}}]$,
$\mu\in (0,h_{\text{max}}]$, ![]() $a_1\in(0,a]$.
$a_1\in(0,a]$. ![]() $\{u_n\}\subset S(a_1)$ be a minimizing sequence with respect to
$\{u_n\}\subset S(a_1)$ be a minimizing sequence with respect to ![]() $\Upsilon_{\mu,T,a_1}$. Then, for some subsequence, either
$\Upsilon_{\mu,T,a_1}$. Then, for some subsequence, either
(i) ![]() $\{u_n\}$ is strongly convergent,
$\{u_n\}$ is strongly convergent,
or
(ii) There exists ![]() $\{y_n\}\subset \mathbb{R}^N$ with
$\{y_n\}\subset \mathbb{R}^N$ with ![]() $|y_n|\to\infty$ such that the sequence
$|y_n|\to\infty$ such that the sequence ![]() $v_n(x) = u_n(x + y_n)$ is strongly convergent to a function
$v_n(x) = u_n(x + y_n)$ is strongly convergent to a function ![]() $v\in S(a_1)$ with
$v\in S(a_1)$ with ![]() $J_{\mu,T}(v)=\Upsilon_{\mu,T,a_1}$.
$J_{\mu,T}(v)=\Upsilon_{\mu,T,a_1}$.
Proof. Noting that ![]() $\|\nabla u_n\|_2 \lt R_0$ for n large enough, there exists
$\|\nabla u_n\|_2 \lt R_0$ for n large enough, there exists ![]() $u\in H^1(\mathbb{R}^N)$ such that
$u\in H^1(\mathbb{R}^N)$ such that ![]() $u_n\rightharpoonup u$ in
$u_n\rightharpoonup u$ in ![]() $H^1(\mathbb{R}^N)$ up to a subsequence. Now we consider the following three possibilities.
$H^1(\mathbb{R}^N)$ up to a subsequence. Now we consider the following three possibilities.
(1) If ![]() $u\not\equiv0$ and
$u\not\equiv0$ and ![]() $\|u\|_2=b\neq a_1$, we must have
$\|u\|_2=b\neq a_1$, we must have ![]() $b\in
(0,a_1)$. Setting
$b\in
(0,a_1)$. Setting ![]() $v_n=u_n-u$,
$v_n=u_n-u$, ![]() $d_n=\|v_n\|_2$, and by using
$d_n=\|v_n\|_2$, and by using
we obtain that ![]() $\|v_n\|_2\to d$, where
$\|v_n\|_2\to d$, where ![]() $a_1^2=d^2+b^2$. Noting that
$a_1^2=d^2+b^2$. Noting that ![]() $d_n\in (0,a_1)$ for n large enough, and using the Brézis–Lieb Lemma (see [Reference Willem41]), Lemma 3.5,
$d_n\in (0,a_1)$ for n large enough, and using the Brézis–Lieb Lemma (see [Reference Willem41]), Lemma 3.5, ![]() $\|\nabla u_n\|_2^2=\|\nabla u\|_2^2+\|\nabla v_n\|_2^2+o_n(1)$,
$\|\nabla u_n\|_2^2=\|\nabla u\|_2^2+\|\nabla v_n\|_2^2+o_n(1)$, ![]() $\|\nabla u\|_2^2\leq \liminf_{n\to+\infty}\|\nabla u_n\|_2^2$, τ is continuous and non-increasing, we obtain that
$\|\nabla u\|_2^2\leq \liminf_{n\to+\infty}\|\nabla u_n\|_2^2$, τ is continuous and non-increasing, we obtain that
 \begin{equation*}
\begin{split}
\Upsilon_{\mu,T,a_1}+o_n(1)=J_{\mu,T}(u_n)&=\frac{1}{2}\|\nabla
v_n\|_2^2-\frac{\mu}{q}\|v_n\|_q^q-\frac{\eta}{p}\tau(\|\nabla
u_n\|_2)\|v_n\|_p^p\\
&\quad + \frac{1}{2}\|\nabla
u\|_2^2-\frac{\mu}{q}\|u\|_q^q-\frac{\eta}{p}\tau(\|\nabla
u_n\|_2)\|u\|_p^p+o_n(1)\\
&\geq
J_{\mu,T}(v_n)+J_{\mu,T}(u)+o_n(1)\\
&\geq \Upsilon_{\mu,T,d_n}+\Upsilon_{\mu,T,b}+o_n(1)\\
&\geq
\frac{d_n^2}{a_1^2}\Upsilon_{\mu,T,a_1}+\Upsilon_{\mu,T,b}+o_n(1).
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
\Upsilon_{\mu,T,a_1}+o_n(1)=J_{\mu,T}(u_n)&=\frac{1}{2}\|\nabla
v_n\|_2^2-\frac{\mu}{q}\|v_n\|_q^q-\frac{\eta}{p}\tau(\|\nabla
u_n\|_2)\|v_n\|_p^p\\
&\quad + \frac{1}{2}\|\nabla
u\|_2^2-\frac{\mu}{q}\|u\|_q^q-\frac{\eta}{p}\tau(\|\nabla
u_n\|_2)\|u\|_p^p+o_n(1)\\
&\geq
J_{\mu,T}(v_n)+J_{\mu,T}(u)+o_n(1)\\
&\geq \Upsilon_{\mu,T,d_n}+\Upsilon_{\mu,T,b}+o_n(1)\\
&\geq
\frac{d_n^2}{a_1^2}\Upsilon_{\mu,T,a_1}+\Upsilon_{\mu,T,b}+o_n(1).
\end{split}
\end{equation*} Letting ![]() $n\to+\infty$, we find that
$n\to+\infty$, we find that
 \begin{equation*}
\begin{split}
\Upsilon_{\mu,T,a_1}&\geq
\frac{d^2}{a_1^2}\Upsilon_{\mu,T,a_1}+\Upsilon_{\mu,T,b}\\
& \gt \frac{d^2}{a_1^2}\Upsilon_{\mu,T,a_1}+\frac{b^2}{a_1^2}\Upsilon_{\mu,T,a_1}
=\Upsilon_{\mu,T,a_1},
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
\Upsilon_{\mu,T,a_1}&\geq
\frac{d^2}{a_1^2}\Upsilon_{\mu,T,a_1}+\Upsilon_{\mu,T,b}\\
& \gt \frac{d^2}{a_1^2}\Upsilon_{\mu,T,a_1}+\frac{b^2}{a_1^2}\Upsilon_{\mu,T,a_1}
=\Upsilon_{\mu,T,a_1},
\end{split}
\end{equation*}which is a contradiction. So this possibility can not exist.
(2) If ![]() $\|u\|_2=a_1$, then
$\|u\|_2=a_1$, then ![]() $u_n\to u$ in
$u_n\to u$ in ![]() $L^2(\mathbb{R}^N)$ and thus
$L^2(\mathbb{R}^N)$ and thus ![]() $u_n\to u$ in
$u_n\to u$ in ![]() $L^t(\mathbb{R}^N)$ for all
$L^t(\mathbb{R}^N)$ for all ![]() $t\in (2,2^*)$.
$t\in (2,2^*)$.
Case ![]() $p \lt 2^*$, then
$p \lt 2^*$, then
 \begin{equation*}
\begin{split}
\Upsilon_{\mu,T,a_1}&=\lim_{n\to+\infty}J_{\mu,T}(u_n)\\
&= \lim_{n\to+\infty}\left(\frac{1}{2}\|\nabla
u_n\|_2^2-\frac{\mu}{q}\|u_n\|_q^q-\frac{\eta}{p}\tau(\|\nabla
u_n\|_2)\|u_n\|_p^p\right)\\
&\geq J_{\mu,T}(u).
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
\Upsilon_{\mu,T,a_1}&=\lim_{n\to+\infty}J_{\mu,T}(u_n)\\
&= \lim_{n\to+\infty}\left(\frac{1}{2}\|\nabla
u_n\|_2^2-\frac{\mu}{q}\|u_n\|_q^q-\frac{\eta}{p}\tau(\|\nabla
u_n\|_2)\|u_n\|_p^p\right)\\
&\geq J_{\mu,T}(u).
\end{split}
\end{equation*} As ![]() $u\in S(a_1)$, we infer that
$u\in S(a_1)$, we infer that ![]() $J_{\mu,T}(u)=\Upsilon_{\mu,T,a_1}$, then
$J_{\mu,T}(u)=\Upsilon_{\mu,T,a_1}$, then ![]() $\|\nabla u_n\|_2\to \|\nabla u\|_2$ and thus
$\|\nabla u_n\|_2\to \|\nabla u\|_2$ and thus ![]() $u_n\to u$ in
$u_n\to u$ in ![]() $H^1(\mathbb{R}^N)$, which implies that (i) occurs.
$H^1(\mathbb{R}^N)$, which implies that (i) occurs.
Case ![]() $p=2^*$, noting that
$p=2^*$, noting that ![]() $\|\nabla v_n\|_2\leq \|\nabla
u_n\|_2 \lt R_0$ for n large enough, and using the Sobolev inequality, we have
$\|\nabla v_n\|_2\leq \|\nabla
u_n\|_2 \lt R_0$ for n large enough, and using the Sobolev inequality, we have
 \begin{equation}
\begin{split}
J_{\mu,T}(v_n)=J_{\mu}(v_n)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x
-\frac{\eta}{p}\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\\
&\geq \frac{1}{2}\|\nabla
v_n\|_2^2-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}\|\nabla
v_n\|_2^{2^*}+o_n(1)\\
&=\|\nabla
v_n\|_2^2\left(\frac{1}{2}-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}\|\nabla
v_n\|_2^{2^*-2}\right)+o_n(1)\\
&\geq \|\nabla
v_n\|_2^2\left(\frac{1}{2}-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}R_0^{2^*-2}\right)
+o_n(1)\\
&= \|\nabla
v_n\|_2^2\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}R_0^{q\gamma_q-2}
+o_n(1),
\end{split}
\end{equation}
\begin{equation}
\begin{split}
J_{\mu,T}(v_n)=J_{\mu}(v_n)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\frac{\mu}{q}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x
-\frac{\eta}{p}\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\\
&\geq \frac{1}{2}\|\nabla
v_n\|_2^2-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}\|\nabla
v_n\|_2^{2^*}+o_n(1)\\
&=\|\nabla
v_n\|_2^2\left(\frac{1}{2}-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}\|\nabla
v_n\|_2^{2^*-2}\right)+o_n(1)\\
&\geq \|\nabla
v_n\|_2^2\left(\frac{1}{2}-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}R_0^{2^*-2}\right)
+o_n(1)\\
&= \|\nabla
v_n\|_2^2\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}R_0^{q\gamma_q-2}
+o_n(1),
\end{split}
\end{equation} because  $w_a(R_0)=\frac{1}{2}-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}R_0^{2^*-2}
-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}R_0^{q\gamma_q-2}=0$. Now we remember that
$w_a(R_0)=\frac{1}{2}-\frac{\eta}{2^*}\frac{1}{S^{\frac{2^*}{2}}}R_0^{2^*-2}
-\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}R_0^{q\gamma_q-2}=0$. Now we remember that
Since ![]() $u\in S(a_1)$, we have
$u\in S(a_1)$, we have ![]() $J_{\mu,T}(u)\geq
\Upsilon_{\mu,T,a_1}$, which combined with Equations (3.2) and (3.3) gives that
$J_{\mu,T}(u)\geq
\Upsilon_{\mu,T,a_1}$, which combined with Equations (3.2) and (3.3) gives that ![]() $\|\nabla v_n\|_2^2\to 0$ and then
$\|\nabla v_n\|_2^2\to 0$ and then ![]() $u_n\to
u$ in
$u_n\to
u$ in ![]() $H^1(\mathbb{R}^N)$. This implies that (i) occurs.
$H^1(\mathbb{R}^N)$. This implies that (i) occurs.
(3) If ![]() $u\equiv 0$, that is,
$u\equiv 0$, that is, ![]() $u_n\rightharpoonup 0$ in
$u_n\rightharpoonup 0$ in ![]() $H^1(\mathbb{R}^N)$. We claim that there exist
$H^1(\mathbb{R}^N)$. We claim that there exist ![]() $R, \beta \gt 0$ and
$R, \beta \gt 0$ and ![]() $\{y_n\}\subset \mathbb{R}^N$ such that
$\{y_n\}\subset \mathbb{R}^N$ such that
 \begin{equation}
\int_{B_R(y_n)}|u_n|^2\,\textrm{d}x\geq \beta,\quad \text{for}\ \text{all} \ n.
\end{equation}
\begin{equation}
\int_{B_R(y_n)}|u_n|^2\,\textrm{d}x\geq \beta,\quad \text{for}\ \text{all} \ n.
\end{equation} Indeed, otherwise we must have ![]() $u_n\to 0$ in
$u_n\to 0$ in ![]() $L^t(\mathbb{R}^N)$ for all
$L^t(\mathbb{R}^N)$ for all ![]() $t\in(2, 2^*)$. Thus, for
$t\in(2, 2^*)$. Thus, for ![]() $p \lt 2^*$,
$p \lt 2^*$,  $J_{\mu,T}(u_n)\geq
\frac{1}{2}\|\nabla u_n\|_2^2+o_n(1)$, which contradicts
$J_{\mu,T}(u_n)\geq
\frac{1}{2}\|\nabla u_n\|_2^2+o_n(1)$, which contradicts ![]() $J_{\mu,T}(u_n)\to \Upsilon_{\mu,T,a_1} \lt 0$. For
$J_{\mu,T}(u_n)\to \Upsilon_{\mu,T,a_1} \lt 0$. For ![]() $p=2^*$, similarly to (3.2), we obtain that
$p=2^*$, similarly to (3.2), we obtain that
 \begin{equation*}
J_{\mu,T}(u_n)\geq
\|\nabla
u_n\|_2^2\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}R_0^{q\gamma_q-2}
+o_n(1).
\end{equation*}
\begin{equation*}
J_{\mu,T}(u_n)\geq
\|\nabla
u_n\|_2^2\frac{1}{q}h_{\text{max}}C_{N,q}^qa^{q(1-\gamma_q)}R_0^{q\gamma_q-2}
+o_n(1).
\end{equation*} We also get a contradiction in this case. Hence, in all cases, Equation (3.4) holds and ![]() $|y_n|\to +\infty$ obviously. From this, considering
$|y_n|\to +\infty$ obviously. From this, considering ![]() $\bar{u}_n(x) = u_n(x+y_n)$, clearly
$\bar{u}_n(x) = u_n(x+y_n)$, clearly ![]() $\{\bar{u}_n\}\subset S(a_1)$ and it is also a minimizing sequence with respect to
$\{\bar{u}_n\}\subset S(a_1)$ and it is also a minimizing sequence with respect to ![]() $\Upsilon_{\mu,T,a_1}$. Moreover, there exists
$\Upsilon_{\mu,T,a_1}$. Moreover, there exists ![]() $\bar{u}\in H^1(\mathbb{R}^N)\backslash\{0\}$ such that
$\bar{u}\in H^1(\mathbb{R}^N)\backslash\{0\}$ such that ![]() $\bar{u}_n\rightharpoonup \bar{u}$ in
$\bar{u}_n\rightharpoonup \bar{u}$ in ![]() $H^1(\mathbb{R}^N)$. Following as in the first two possibilities of the proof, we derive that
$H^1(\mathbb{R}^N)$. Following as in the first two possibilities of the proof, we derive that ![]() $\bar{u}_n\to\bar{u}$ in
$\bar{u}_n\to\bar{u}$ in ![]() $H^1(\mathbb{R}^N)$, which implies that (ii) occurs.
$H^1(\mathbb{R}^N)$, which implies that (ii) occurs.
Lemma 3.7. Let ![]() $N, a,\eta,p,q$ be as in Equation (1.1),
$N, a,\eta,p,q$ be as in Equation (1.1), ![]() $\mu\in
(0,h_{\text{max}}]$,
$\mu\in
(0,h_{\text{max}}]$, ![]() $a_1\in(0,a]$, (1.13) hold. Then,
$a_1\in(0,a]$, (1.13) hold. Then, ![]() $\Upsilon_{\mu,T,a_1}$ is attained.
$\Upsilon_{\mu,T,a_1}$ is attained.
Proof. By Lemma 3.1, there exists a bounded minimizing sequence ![]() $\{u_n\} \subset S(a_1)$ satisfying
$\{u_n\} \subset S(a_1)$ satisfying ![]() $J_{\mu,T}(u_n)\to \Upsilon_{\mu,T,a_1}$ as
$J_{\mu,T}(u_n)\to \Upsilon_{\mu,T,a_1}$ as ![]() $n\to+\infty$. Now, applying Lemma 3.6, there exists
$n\to+\infty$. Now, applying Lemma 3.6, there exists ![]() $u\in S(a_1)$ such that
$u\in S(a_1)$ such that ![]() $J_{\mu,T}(u)= \Upsilon_{\mu,T,a_1}$. The proof is complete.
$J_{\mu,T}(u)= \Upsilon_{\mu,T,a_1}$. The proof is complete.
An immediate consequence of Lemma 3.7 is the following corollary.
Corollary 3.8. Let ![]() $N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold. Fix
$N, a,\eta,p$ and q be as in Equations (1.1) and (1.13) hold. Fix ![]() $a_1\in (0,a]$ and let
$a_1\in (0,a]$ and let ![]() $0 \lt \mu_1 \lt \mu_2\leq h_{\text{max}}$. Then,
$0 \lt \mu_1 \lt \mu_2\leq h_{\text{max}}$. Then, ![]() $\Upsilon_{\mu_2,T,a_1} \lt \Upsilon_{\mu_1,T,a_1}$.
$\Upsilon_{\mu_2,T,a_1} \lt \Upsilon_{\mu_1,T,a_1}$.
Proof. Let ![]() $u\in S(a_1)$ satisfy
$u\in S(a_1)$ satisfy ![]() $J_{\mu_1,T}(u)=\Upsilon_{\mu_1,T,a_1}$. Then,
$J_{\mu_1,T}(u)=\Upsilon_{\mu_1,T,a_1}$. Then, ![]() $\Upsilon_{\mu_2,T,a_1}\leq J_{\mu_2,T}(u) \lt J_{\mu_1,T}(u) =
\Upsilon_{\mu_1,T,a_1}$.
$\Upsilon_{\mu_2,T,a_1}\leq J_{\mu_2,T}(u) \lt J_{\mu_1,T}(u) =
\Upsilon_{\mu_1,T,a_1}$.
4. Proof of Theorem 1.1
In this section, we first prove some properties of the functional ![]() $E_{\epsilon,T}$ defined in Equation (2.5) restricted to the sphere S(a), and then give the proof of Theorem 1.1.
$E_{\epsilon,T}$ defined in Equation (2.5) restricted to the sphere S(a), and then give the proof of Theorem 1.1.
Denote
and
It is obvious that ![]() $J_{\infty,T}(u)\geq J_{\text{max},T}(u)$ and
$J_{\infty,T}(u)\geq J_{\text{max},T}(u)$ and ![]() $E_{\epsilon,T}(u)\geq J_{\text{max},T}(u)$ for any
$E_{\epsilon,T}(u)\geq J_{\text{max},T}(u)$ for any ![]() $u\in S(a)$. By Lemma 3.1, the definition:
$u\in S(a)$. By Lemma 3.1, the definition:
 \begin{equation*}
\Gamma_{\epsilon,T,a}:=\inf_{u\in S(a)}E_{\epsilon,T}(u),
\end{equation*}
\begin{equation*}
\Gamma_{\epsilon,T,a}:=\inf_{u\in S(a)}E_{\epsilon,T}(u),
\end{equation*} is well defined and ![]() $\Gamma_{\epsilon,T,a}\geq
\Upsilon_{\text{max},T,a}$.
$\Gamma_{\epsilon,T,a}\geq
\Upsilon_{\text{max},T,a}$.
The next lemma establishes some crucial relations involving the levels ![]() $\Gamma_{\epsilon,T,a}$,
$\Gamma_{\epsilon,T,a}$, ![]() $\Upsilon_{\infty,T,a}$ and
$\Upsilon_{\infty,T,a}$ and ![]() $\Upsilon_{\text{max},T,a}$.
$\Upsilon_{\text{max},T,a}$.
Lemma 4.1. Let ![]() $N, a,\eta,p,q, h$ and ϵ be as in Equations (1.1) and (1.13) hold. Then
$N, a,\eta,p,q, h$ and ϵ be as in Equations (1.1) and (1.13) hold. Then
 \begin{equation*}\limsup_{\epsilon\to 0^+}\Gamma_{\epsilon,T,a}\leq
\Upsilon_{\text{max},T,a} \lt \Upsilon_{\infty,T,a} \lt 0.\end{equation*}
\begin{equation*}\limsup_{\epsilon\to 0^+}\Gamma_{\epsilon,T,a}\leq
\Upsilon_{\text{max},T,a} \lt \Upsilon_{\infty,T,a} \lt 0.\end{equation*}Proof. By Lemma 3.7, choose ![]() $u \in S(a)$ such that
$u \in S(a)$ such that ![]() $J_{\text{max},T}(u)=\Upsilon_{\text{max},T,a}$. Then,
$J_{\text{max},T}(u)=\Upsilon_{\text{max},T,a}$. Then,
 \begin{equation*}
\begin{split}
\Gamma_{\epsilon,T,a}\leq E_{\epsilon,
T}(u)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x)|u|^{q}\,{\rm d}x\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x.
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
\Gamma_{\epsilon,T,a}\leq E_{\epsilon,
T}(u)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x)|u|^{q}\,{\rm d}x\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x.
\end{split}
\end{equation*} Letting ![]() $\epsilon\to 0^+$, by the Lebesgue dominated convergence theorem, we deduce that
$\epsilon\to 0^+$, by the Lebesgue dominated convergence theorem, we deduce that
 \begin{equation*}
\limsup_{\epsilon\to 0^+}\Gamma_{\epsilon,T,a}\leq
\limsup_{\epsilon\to 0^+} E_{\epsilon, T}(u)=J_{h(0),T}(u)=
J_{\text{max},T}(u)=\Upsilon_{\text{max},T,a},
\end{equation*}
\begin{equation*}
\limsup_{\epsilon\to 0^+}\Gamma_{\epsilon,T,a}\leq
\limsup_{\epsilon\to 0^+} E_{\epsilon, T}(u)=J_{h(0),T}(u)=
J_{\text{max},T}(u)=\Upsilon_{\text{max},T,a},
\end{equation*}which combined with Lemma 3.2 and Corollary 3.8 completes the proof.
By Lemma 4.1, there exists ![]() $\epsilon_1 \gt 0$ such that
$\epsilon_1 \gt 0$ such that ![]() $\Gamma_{\epsilon,T,a} \lt \Upsilon_{\infty,T,a}$ for all
$\Gamma_{\epsilon,T,a} \lt \Upsilon_{\infty,T,a}$ for all ![]() $\epsilon\in
(0,\epsilon_1)$. In the following, we always assume that
$\epsilon\in
(0,\epsilon_1)$. In the following, we always assume that ![]() $\epsilon\in (0,\epsilon_1)$. Similarly to the proof of Lemma 3.3, we have the following result, whose proof is omitted.
$\epsilon\in (0,\epsilon_1)$. Similarly to the proof of Lemma 3.3, we have the following result, whose proof is omitted.
Lemma 4.2. Let ![]() $N, a,\eta,p,q, h, \epsilon$ be as in (1.1),
$N, a,\eta,p,q, h, \epsilon$ be as in (1.1), ![]() $\epsilon\in(0,\epsilon_1)$, (1.13) hold. Then
$\epsilon\in(0,\epsilon_1)$, (1.13) hold. Then
(1) ![]() $E_{\epsilon,T}\in C^1(H^1(\mathbb{R}^N),\mathbb{R})$;
$E_{\epsilon,T}\in C^1(H^1(\mathbb{R}^N),\mathbb{R})$;
(2) If ![]() $u\in S(a)$ such that
$u\in S(a)$ such that ![]() $E_{\epsilon,T}(u) \lt 0$, then
$E_{\epsilon,T}(u) \lt 0$, then ![]() $\|\nabla
u\|_2 \lt R_0$ and
$\|\nabla
u\|_2 \lt R_0$ and ![]() $E_{\epsilon,T}(v) = E_{\epsilon} (v)$ for all v satisfying
$E_{\epsilon,T}(v) = E_{\epsilon} (v)$ for all v satisfying ![]() $\|v\|_2\leq a$ and being in a small neighborhood of u in
$\|v\|_2\leq a$ and being in a small neighborhood of u in ![]() $H^1(\mathbb{R}^N)$.
$H^1(\mathbb{R}^N)$.
The next two lemmas will be used to prove the PS condition for ![]() $E_{\epsilon,T}$ restricted to S(a) at some levels.
$E_{\epsilon,T}$ restricted to S(a) at some levels.
Lemma 4.3. Let ![]() $N, a,\eta,p,q, h$ be as in (1.1),
$N, a,\eta,p,q, h$ be as in (1.1), ![]() $\epsilon\in
(0,\epsilon_1)$, (1.13) hold. Assume
$\epsilon\in
(0,\epsilon_1)$, (1.13) hold. Assume ![]() $\{u_n\}\subset S(a)$ such that
$\{u_n\}\subset S(a)$ such that ![]() $E_{\epsilon,T}(u_n)\to c$ as
$E_{\epsilon,T}(u_n)\to c$ as ![]() $n\to+\infty$ with
$n\to+\infty$ with ![]() $c \lt \Upsilon_{\infty,T,a}$. If
$c \lt \Upsilon_{\infty,T,a}$. If ![]() $u_n\rightharpoonup u$ in
$u_n\rightharpoonup u$ in ![]() $H^1(\mathbb{R}^N)$, then
$H^1(\mathbb{R}^N)$, then ![]() $u\not\equiv 0$.
$u\not\equiv 0$.
Proof. Assume by contradiction that ![]() $u\equiv0$. Then,
$u\equiv0$. Then,
 \begin{equation*}
c+o_n(1)=E_{\epsilon,T}(u_n)=J_{\infty,T}(u_n)+\frac{1}{q}\int_{\mathbb{R}^N}(h_{\infty}-h(\epsilon
x))|u_n|^q\,\textrm{d}x.
\end{equation*}
\begin{equation*}
c+o_n(1)=E_{\epsilon,T}(u_n)=J_{\infty,T}(u_n)+\frac{1}{q}\int_{\mathbb{R}^N}(h_{\infty}-h(\epsilon
x))|u_n|^q\,\textrm{d}x.
\end{equation*} By (h 2), for any given δ > 0, there exists R > 0 such that ![]() $h_{\infty}\geq h(x)-\delta$ for all
$h_{\infty}\geq h(x)-\delta$ for all ![]() $|x|\geq R$. Hence,
$|x|\geq R$. Hence,
 \begin{equation*}
\begin{split}
c+o_n(1)=E_{\epsilon,T}(u_n)&\geq
J_{\infty,T}(u_n)+\frac{1}{q}\int_{B_{R/\epsilon}(0)}(h_{\infty}
-h(\epsilon
x))|u_n|^q\,{\rm d}x\\
&\qquad-\frac{\delta}{q}\int_{B^c_{R/\epsilon}(0)}|u_n|^q\,{\rm d}x.
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
c+o_n(1)=E_{\epsilon,T}(u_n)&\geq
J_{\infty,T}(u_n)+\frac{1}{q}\int_{B_{R/\epsilon}(0)}(h_{\infty}
-h(\epsilon
x))|u_n|^q\,{\rm d}x\\
&\qquad-\frac{\delta}{q}\int_{B^c_{R/\epsilon}(0)}|u_n|^q\,{\rm d}x.
\end{split}
\end{equation*} Recalling that ![]() $\{u_n\}$ is bounded in
$\{u_n\}$ is bounded in ![]() $H^1(\mathbb{R}^N)$ and
$H^1(\mathbb{R}^N)$ and ![]() $u_n\to 0$ in
$u_n\to 0$ in  $L^t(B_{R/\epsilon}(0))$ for all
$L^t(B_{R/\epsilon}(0))$ for all ![]() $t\in[1, 2^*)$, it follows that
$t\in[1, 2^*)$, it follows that
for some C > 0. Since δ > 0 is arbitrary, we deduce that ![]() $c\geq \Upsilon_{\infty,T,a}$, which is a contradiction. Thus,
$c\geq \Upsilon_{\infty,T,a}$, which is a contradiction. Thus, ![]() $u\not\equiv 0$.
$u\not\equiv 0$.
Lemma 4.4. Let ![]() $N, a,\eta,p,q$ and h be as in Equation (1.1),
$N, a,\eta,p,q$ and h be as in Equation (1.1), ![]() $\epsilon\in
(0,\epsilon_1)$, Equation (1.13) hold. If
$\epsilon\in
(0,\epsilon_1)$, Equation (1.13) hold. If ![]() $p=2^*$, we further assume that Equation (1.14) holds. Let
$p=2^*$, we further assume that Equation (1.14) holds. Let ![]() $\{u_{n}\}$ be a
$\{u_{n}\}$ be a ![]() $(PS)_c$ sequence of
$(PS)_c$ sequence of ![]() $E_{\epsilon,T}$ restricted to S(a) with
$E_{\epsilon,T}$ restricted to S(a) with ![]() $c \lt \Upsilon_{\infty,T,a}$ and let
$c \lt \Upsilon_{\infty,T,a}$ and let ![]() $u_n\rightharpoonup u_\epsilon$ in
$u_n\rightharpoonup u_\epsilon$ in ![]() $H^1(\mathbb{R}^N)$. If un does not converge to uϵ strongly in
$H^1(\mathbb{R}^N)$. If un does not converge to uϵ strongly in ![]() $H^1(\mathbb{R}^N)$, there exists β > 0 independent of
$H^1(\mathbb{R}^N)$, there exists β > 0 independent of ![]() $\epsilon\in (0,\epsilon_1)$ such that
$\epsilon\in (0,\epsilon_1)$ such that
 \begin{equation*}
\limsup_{n\to +\infty}\|u_n-u_{\epsilon}\|_2\geq \beta.
\end{equation*}
\begin{equation*}
\limsup_{n\to +\infty}\|u_n-u_{\epsilon}\|_2\geq \beta.
\end{equation*}Proof. By Lemma 4.2, we must have ![]() $\|\nabla u_n\|_2 \lt R_0$ for n large enough, and so,
$\|\nabla u_n\|_2 \lt R_0$ for n large enough, and so, ![]() $\{u_n\}$ is also a
$\{u_n\}$ is also a ![]() $(PS)_c$ sequence of Eϵ restricted to S(a). Hence,
$(PS)_c$ sequence of Eϵ restricted to S(a). Hence,
 \begin{equation*}
E_{\epsilon}(u_n)\to c\qquad {\rm and}\
\|E_{\epsilon}|^\prime_{S(a)}(u_n)\|\to 0\quad {\rm as}\ n\to+\infty.
\end{equation*}
\begin{equation*}
E_{\epsilon}(u_n)\to c\qquad {\rm and}\
\|E_{\epsilon}|^\prime_{S(a)}(u_n)\|\to 0\quad {\rm as}\ n\to+\infty.
\end{equation*} Setting the functional ![]() $\Psi: H^1(\mathbb{R}^N)\to \mathbb{R}$ given by:
$\Psi: H^1(\mathbb{R}^N)\to \mathbb{R}$ given by:
 \begin{equation*}
\Psi(u)=\frac{1}{2}\int_{\mathbb{R}^N}|u|^2\,\textrm{d}x,
\end{equation*}
\begin{equation*}
\Psi(u)=\frac{1}{2}\int_{\mathbb{R}^N}|u|^2\,\textrm{d}x,
\end{equation*} it follows that ![]() $S(a) =\Psi^{-1}(a^2/2)$. Then, by Willem ([Reference Willem41], Proposition 5.12), there exists
$S(a) =\Psi^{-1}(a^2/2)$. Then, by Willem ([Reference Willem41], Proposition 5.12), there exists ![]() $\{\lambda_n\}\subset \mathbb{R}$ such that
$\{\lambda_n\}\subset \mathbb{R}$ such that
 \begin{equation}
\|E^\prime_{\epsilon}(u_n)-\lambda_n\Psi^\prime(u_n)\|_{H^{-1}(\mathbb{R}^N)}\to
0,\quad \text{as}\ n\to+\infty.
\end{equation}
\begin{equation}
\|E^\prime_{\epsilon}(u_n)-\lambda_n\Psi^\prime(u_n)\|_{H^{-1}(\mathbb{R}^N)}\to
0,\quad \text{as}\ n\to+\infty.
\end{equation} By the boundedness of ![]() $\{u_n\}$ in
$\{u_n\}$ in ![]() $H^1(\mathbb{R}^N)$, we know
$H^1(\mathbb{R}^N)$, we know ![]() $\{\lambda_n\}$ is bounded and thus, up to a subsequence, there exists λϵ such that
$\{\lambda_n\}$ is bounded and thus, up to a subsequence, there exists λϵ such that ![]() $\lambda_n\to
\lambda_{\epsilon}$ as
$\lambda_n\to
\lambda_{\epsilon}$ as ![]() $n\to+\infty$. This together with Equation (4.1) leads to
$n\to+\infty$. This together with Equation (4.1) leads to
and then
 \begin{equation}
\|E^\prime_{\epsilon}(v_n)-\lambda_\epsilon\Psi^\prime(v_n)\|_{H^{-1}(\mathbb{R}^N)}\to
0,\quad \text{as}\ n\to+\infty,
\end{equation}
\begin{equation}
\|E^\prime_{\epsilon}(v_n)-\lambda_\epsilon\Psi^\prime(v_n)\|_{H^{-1}(\mathbb{R}^N)}\to
0,\quad \text{as}\ n\to+\infty,
\end{equation} where ![]() $v_n:=u_n-u_\epsilon$. By direct calculations, we get that
$v_n:=u_n-u_\epsilon$. By direct calculations, we get that
 \begin{equation*}
\begin{split}
\Upsilon_{\infty,T,a}& \gt \lim_{n\to+\infty}E_{\epsilon}(u_n)\\
&=\lim_{n\to+\infty}\left(E_{\epsilon}(u_n)-\frac{1}{2}E_{\epsilon}^\prime(u_n)u_n+\frac{1}{2}\lambda_n\|u_n\|_2^2+o_n(1)\right)\\
&=\lim_{n\to+\infty}\left[\left(\frac{1}{2}-\frac{1}{q}\right)\int_{\mathbb{R}^N}h(\epsilon
x)|u_n|^q\,{\rm d}x\right.\\
&\qquad\qquad\qquad\left.+\left(\frac{1}{2}-\frac{1}{p}\right)\eta\int_{\mathbb{R}^N}|u_n|^p\,{\rm d}x+\frac{1}{2}\lambda_na^2+o_n(1)\right]\\
&\geq \frac{1}{2}\lambda_{\epsilon}a^2,
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
\Upsilon_{\infty,T,a}& \gt \lim_{n\to+\infty}E_{\epsilon}(u_n)\\
&=\lim_{n\to+\infty}\left(E_{\epsilon}(u_n)-\frac{1}{2}E_{\epsilon}^\prime(u_n)u_n+\frac{1}{2}\lambda_n\|u_n\|_2^2+o_n(1)\right)\\
&=\lim_{n\to+\infty}\left[\left(\frac{1}{2}-\frac{1}{q}\right)\int_{\mathbb{R}^N}h(\epsilon
x)|u_n|^q\,{\rm d}x\right.\\
&\qquad\qquad\qquad\left.+\left(\frac{1}{2}-\frac{1}{p}\right)\eta\int_{\mathbb{R}^N}|u_n|^p\,{\rm d}x+\frac{1}{2}\lambda_na^2+o_n(1)\right]\\
&\geq \frac{1}{2}\lambda_{\epsilon}a^2,
\end{split}
\end{equation*}which implies that
 \begin{equation}
\lambda_{\epsilon}\leq \frac{2\Upsilon_{\infty,T,a}}{a^2} \lt 0,\quad
\text{for}\ \text{all} \ \epsilon\in (0,\epsilon_1).
\end{equation}
\begin{equation}
\lambda_{\epsilon}\leq \frac{2\Upsilon_{\infty,T,a}}{a^2} \lt 0,\quad
\text{for}\ \text{all} \ \epsilon\in (0,\epsilon_1).
\end{equation}By Equation (4.3), we know
 \begin{equation}
\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\lambda_{\epsilon}\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x-\int_{\mathbb{R}^N}h(\epsilon
x)|v_n|^{q}\,{\rm d}x-\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x=o_n(1),
\end{equation}
\begin{equation}
\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\lambda_{\epsilon}\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x-\int_{\mathbb{R}^N}h(\epsilon
x)|v_n|^{q}\,{\rm d}x-\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x=o_n(1),
\end{equation}which combined with Equation (4.4) gives that
 \begin{equation}
\begin{split}
\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x&-\frac{2\Upsilon_{\infty,T,a}}{a^2}\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x\\
&\leq
h_{{\rm max}}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x+\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x+o_n(1).
\end{split}
\end{equation}
\begin{equation}
\begin{split}
\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x&-\frac{2\Upsilon_{\infty,T,a}}{a^2}\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x\\
&\leq
h_{{\rm max}}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x+\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x+o_n(1).
\end{split}
\end{equation} If un does not converge to uϵ strongly in ![]() $H^1(\mathbb{R}^N)$, that is, vn does not converge to 0 strongly in
$H^1(\mathbb{R}^N)$, that is, vn does not converge to 0 strongly in ![]() $H^1(\mathbb{R}^N)$, by (4.6) and the Sobolev inequality, we deduce that
$H^1(\mathbb{R}^N)$, by (4.6) and the Sobolev inequality, we deduce that
 \begin{equation*}
\begin{split}
\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x&-\frac{2\Upsilon_{\infty,T,a}}{a^2}\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x\\
&\leq h_{{\rm max}}C_{N,q}^q\|v_n\|^q+\eta
C_{N,p}^p\|v_n\|^p+o_n(1).
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x&-\frac{2\Upsilon_{\infty,T,a}}{a^2}\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x\\
&\leq h_{{\rm max}}C_{N,q}^q\|v_n\|^q+\eta
C_{N,p}^p\|v_n\|^p+o_n(1).
\end{split}
\end{equation*} So there exists C > 0 independent of ϵ such that ![]() $\|v_n\|\geq C$ and then by Equation (4.6):
$\|v_n\|\geq C$ and then by Equation (4.6):
 \begin{equation}
\limsup_{n\to
+\infty}\left(h_{{\rm max}}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x+\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\right)\geq
C.
\end{equation}
\begin{equation}
\limsup_{n\to
+\infty}\left(h_{{\rm max}}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x+\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\right)\geq
C.
\end{equation} Case ![]() $p \lt 2^*$, by (4.7) and the Gagliardo–Nirenberg inequality (1.11), there exists β > 0 independent of
$p \lt 2^*$, by (4.7) and the Gagliardo–Nirenberg inequality (1.11), there exists β > 0 independent of ![]() $\epsilon\in (0,\epsilon_1)$ such that
$\epsilon\in (0,\epsilon_1)$ such that
 \begin{equation}
\limsup_{n\to +\infty}\|v_n\|_2\geq \beta.
\end{equation}
\begin{equation}
\limsup_{n\to +\infty}\|v_n\|_2\geq \beta.
\end{equation} Case ![]() $p=2^*$, if
$p=2^*$, if
 \begin{equation*}
\limsup_{n\to +\infty}\int_{\mathbb{R}^N}|v_n|^{q}\,\textrm{d}x\geq C,
\end{equation*}
\begin{equation*}
\limsup_{n\to +\infty}\int_{\mathbb{R}^N}|v_n|^{q}\,\textrm{d}x\geq C,
\end{equation*}for some C > 0 independent of ϵ, we obtain (4.8) as well. If
 \begin{equation*} \liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x=0\qquad {\rm and}\qquad
\liminf_{\epsilon\to 0^+}\limsup_{n\to +\infty}\|v_n\|_2=0,
\end{equation*}
\begin{equation*} \liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\int_{\mathbb{R}^N}|v_n|^{q}\,{\rm d}x=0\qquad {\rm and}\qquad
\liminf_{\epsilon\to 0^+}\limsup_{n\to +\infty}\|v_n\|_2=0,
\end{equation*}by Equation (4.7) we have
 \begin{equation*}
\liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\int_{\mathbb{R}^N}|v_n|^{p}\,\textrm{d}x\geq C,
\end{equation*}
\begin{equation*}
\liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\int_{\mathbb{R}^N}|v_n|^{p}\,\textrm{d}x\geq C,
\end{equation*}and by Equation (4.5) we have
 \begin{equation*}
\liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\int_{\mathbb{R}^N}|\nabla v_n|^2\,{\rm d}x=\liminf_{\epsilon\to
0^+}\limsup_{n\to +\infty}\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x.
\end{equation*}
\begin{equation*}
\liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\int_{\mathbb{R}^N}|\nabla v_n|^2\,{\rm d}x=\liminf_{\epsilon\to
0^+}\limsup_{n\to +\infty}\eta\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x.
\end{equation*}Applying the Sobolev inequality to the above equality, we obtain that
 \begin{equation*}
\liminf_{\epsilon\to 0^+}\limsup_{n\to +\infty}\|\nabla
v_n\|_2^2=\liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\eta\|v_n\|_{2^*}^{2^*}\leq\liminf_{\epsilon\to
0^+}\limsup_{n\to +\infty}\eta S^{-2^*/2}\|\nabla v_n\|_2^{2^*},
\end{equation*}
\begin{equation*}
\liminf_{\epsilon\to 0^+}\limsup_{n\to +\infty}\|\nabla
v_n\|_2^2=\liminf_{\epsilon\to 0^+}\limsup_{n\to
+\infty}\eta\|v_n\|_{2^*}^{2^*}\leq\liminf_{\epsilon\to
0^+}\limsup_{n\to +\infty}\eta S^{-2^*/2}\|\nabla v_n\|_2^{2^*},
\end{equation*}which implies that
 \begin{equation}
R_0\geq \liminf_{\epsilon\to 0^+}\limsup_{n\to +\infty}\|\nabla
v_n\|_2\geq \eta^{-\frac{N-2}{4}}S^{N/4}.
\end{equation}
\begin{equation}
R_0\geq \liminf_{\epsilon\to 0^+}\limsup_{n\to +\infty}\|\nabla
v_n\|_2\geq \eta^{-\frac{N-2}{4}}S^{N/4}.
\end{equation}On the other hand, by the assumption (1.14), we have
 \begin{equation}
R_0 \lt \eta^{-\frac{N-2}{4}}S^{N/4}.
\end{equation}
\begin{equation}
R_0 \lt \eta^{-\frac{N-2}{4}}S^{N/4}.
\end{equation}Indeed, by the expression of r 0 in Equation (2.2), Equation (1.14) is equivalent to
 \begin{equation}
r_0 \lt \eta^{-\frac{N-2}{4}}S^{\frac{N}{4}}.
\end{equation}
\begin{equation}
r_0 \lt \eta^{-\frac{N-2}{4}}S^{\frac{N}{4}}.
\end{equation} Since ![]() $R_0 \lt r_0 \lt R_1$ (see
$R_0 \lt r_0 \lt R_1$ (see ![]() $\S$ 2), we obtain Equation (4.10), which contradicts Equation (4.9). So we must have Equation (4.8) for the case
$\S$ 2), we obtain Equation (4.10), which contradicts Equation (4.9). So we must have Equation (4.8) for the case ![]() $p=2^*$. The proof is complete.
$p=2^*$. The proof is complete.
Now, we give the compactness lemma.
Lemma 4.5. Let ![]() $N, a,\eta,p,q$ and h be as in Equation (1.1),
$N, a,\eta,p,q$ and h be as in Equation (1.1), ![]() $\epsilon\in
(0,\epsilon_1)$, β be as in Lemma 4.4,
$\epsilon\in
(0,\epsilon_1)$, β be as in Lemma 4.4,
 \begin{equation*}0 \lt \rho_0\leq
\min\left\{\Upsilon_{\infty,T,a}
-\Upsilon_{\text{max},T,a},\frac{\beta^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a})\right\},\end{equation*}
\begin{equation*}0 \lt \rho_0\leq
\min\left\{\Upsilon_{\infty,T,a}
-\Upsilon_{\text{max},T,a},\frac{\beta^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a})\right\},\end{equation*} and Equation (1.13) hold. If ![]() $p=2^*$, we further assume that Equation (1.14) holds. Then,
$p=2^*$, we further assume that Equation (1.14) holds. Then, ![]() $E_{\epsilon,T}$ satisfies the
$E_{\epsilon,T}$ satisfies the ![]() $(PS)_c$ condition restricted to S(a) if
$(PS)_c$ condition restricted to S(a) if ![]() $c \lt \Upsilon_{\text{max},T,a}+\rho_0$.
$c \lt \Upsilon_{\text{max},T,a}+\rho_0$.
Proof. Let ![]() $\{u_{n}\}\subset S(a)$ be a
$\{u_{n}\}\subset S(a)$ be a ![]() $(PS)_c$ sequence of
$(PS)_c$ sequence of ![]() $E_{\epsilon,T}$ restricted to S(a). Noting that
$E_{\epsilon,T}$ restricted to S(a). Noting that ![]() $c \lt \Upsilon_{\infty,T,a} \lt 0$, by Lemma 4.2,
$c \lt \Upsilon_{\infty,T,a} \lt 0$, by Lemma 4.2, ![]() $\{u_n\}$ is bounded in
$\{u_n\}$ is bounded in ![]() $H^1(\mathbb{R}^N)$. Let
$H^1(\mathbb{R}^N)$. Let ![]() $u_n\rightharpoonup u_\epsilon$ in
$u_n\rightharpoonup u_\epsilon$ in ![]() $H^1(\mathbb{R}^N)$. By Lemma 4.3,
$H^1(\mathbb{R}^N)$. By Lemma 4.3, ![]() $u_\epsilon\not\equiv
0$. Set
$u_\epsilon\not\equiv
0$. Set ![]() $v_n:=u_n-u_{\epsilon}$. If
$v_n:=u_n-u_{\epsilon}$. If ![]() $u_n\to u_\epsilon$ in
$u_n\to u_\epsilon$ in ![]() $H^1(\mathbb{R}^N)$, the proof is complete. If un does not converge to uϵ strongly in
$H^1(\mathbb{R}^N)$, the proof is complete. If un does not converge to uϵ strongly in ![]() $H^1(\mathbb{R}^N)$ for some
$H^1(\mathbb{R}^N)$ for some ![]() $\epsilon\in
(0,\epsilon_1)$, by Lemma 4.4,
$\epsilon\in
(0,\epsilon_1)$, by Lemma 4.4,
 \begin{equation*}
\limsup_{n\to +\infty}\|v_n\|_2\geq \beta.
\end{equation*}
\begin{equation*}
\limsup_{n\to +\infty}\|v_n\|_2\geq \beta.
\end{equation*} Set ![]() $b=\|u_{\epsilon}\|_2,\ d_n = \|v_n\|_2$ and suppose that
$b=\|u_{\epsilon}\|_2,\ d_n = \|v_n\|_2$ and suppose that ![]() $\|v_n\|_2\to d$, then we get
$\|v_n\|_2\to d$, then we get ![]() $d\geq \beta \gt 0$ and
$d\geq \beta \gt 0$ and ![]() $a^2 = b^2 +
d^2$. From
$a^2 = b^2 +
d^2$. From ![]() $d_n\in(0, a)$ for n large enough, we have
$d_n\in(0, a)$ for n large enough, we have
Since ![]() $v_n\rightharpoonup 0$ in
$v_n\rightharpoonup 0$ in ![]() $H^1(\mathbb{R}^N)$, similarly to the proof of Lemma 4.3, we deduce that
$H^1(\mathbb{R}^N)$, similarly to the proof of Lemma 4.3, we deduce that
for any δ > 0, where C > 0 is a constant independent of ![]() $\delta,\ \epsilon$ and n. By (4.12) and (4.13), we obtain that
$\delta,\ \epsilon$ and n. By (4.12) and (4.13), we obtain that
 \begin{equation*}
\begin{split}
c+o_n(1)=E_{\epsilon,T}(u_n)&\geq
J_{\infty,T}(v_n)+E_{\epsilon,T}(u_{\epsilon})-\delta C+o_n(1)\\
&\geq \Upsilon_{\infty,T,d_n}+\Upsilon_{\text{max},T,b}-\delta
C+o_n(1).
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
c+o_n(1)=E_{\epsilon,T}(u_n)&\geq
J_{\infty,T}(v_n)+E_{\epsilon,T}(u_{\epsilon})-\delta C+o_n(1)\\
&\geq \Upsilon_{\infty,T,d_n}+\Upsilon_{\text{max},T,b}-\delta
C+o_n(1).
\end{split}
\end{equation*} Letting ![]() $n\to +\infty$, by Lemma 3.5 and the arbitrariness of δ > 0, we obtain that
$n\to +\infty$, by Lemma 3.5 and the arbitrariness of δ > 0, we obtain that
 \begin{equation*}
\begin{split}
c\geq \Upsilon_{\infty,T,d}+\Upsilon_{\text{max},T,b}&\geq
\frac{d^2}{a^2}\Upsilon_{\infty,T,a}+\frac{b^2}{a^2}\Upsilon_{\text{max},T,a}\\
&=\Upsilon_{\text{max},T,a}+\frac{d^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a})\\
&\geq
\Upsilon_{\text{max},T,a}+\frac{\beta^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a}),
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
c\geq \Upsilon_{\infty,T,d}+\Upsilon_{\text{max},T,b}&\geq
\frac{d^2}{a^2}\Upsilon_{\infty,T,a}+\frac{b^2}{a^2}\Upsilon_{\text{max},T,a}\\
&=\Upsilon_{\text{max},T,a}+\frac{d^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a})\\
&\geq
\Upsilon_{\text{max},T,a}+\frac{\beta^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a}),
\end{split}
\end{equation*} which contradicts  $c \lt \Upsilon_{\text{max},T,a}+\frac{\beta^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a})$. Thus, we must have
$c \lt \Upsilon_{\text{max},T,a}+\frac{\beta^2}{a^2}(\Upsilon_{\infty,T,a}-\Upsilon_{\text{max},T,a})$. Thus, we must have ![]() $u_n \to u_{\epsilon}$ in
$u_n \to u_{\epsilon}$ in ![]() $H^1(\mathbb{R}^N)$.
$H^1(\mathbb{R}^N)$.
In what follows, let us fix ![]() $\tilde{\rho}, \tilde{r} \gt 0$ satisfying:
$\tilde{\rho}, \tilde{r} \gt 0$ satisfying:
•
 $\overline{B_{\tilde{\rho}}(a_i)}\cap \overline{B_{\tilde{\rho}}(a_j)}=\emptyset$ for i ≠ j and
$\overline{B_{\tilde{\rho}}(a_i)}\cap \overline{B_{\tilde{\rho}}(a_j)}=\emptyset$ for i ≠ j and  $i,j\in\{1,\ldots,l\}$;
$i,j\in\{1,\ldots,l\}$;•
 $\cup_{i=1}^{l}B_{\tilde{\rho}}(a_i) \subset B_{\tilde{r}}(0)$;
$\cup_{i=1}^{l}B_{\tilde{\rho}}(a_i) \subset B_{\tilde{r}}(0)$;•
 $K_{\frac{\tilde{\rho}}{2}}=\cup_{i=1}^{l}\overline{B_{\frac{\tilde{\rho}}{2}}(a_i)}$.
$K_{\frac{\tilde{\rho}}{2}}=\cup_{i=1}^{l}\overline{B_{\frac{\tilde{\rho}}{2}}(a_i)}$.
We also set the function ![]() $Q_\epsilon:
H^1(\mathbb{R}^N)\backslash\{0\}\to \mathbb{R}^N$ by
$Q_\epsilon:
H^1(\mathbb{R}^N)\backslash\{0\}\to \mathbb{R}^N$ by
 \begin{equation*}
Q_\epsilon(u):=\frac{\int_{\mathbb{R}^N}\chi(\epsilon
x)|u|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u|^2\,{\rm d}x},
\end{equation*}
\begin{equation*}
Q_\epsilon(u):=\frac{\int_{\mathbb{R}^N}\chi(\epsilon
x)|u|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u|^2\,{\rm d}x},
\end{equation*} where ![]() $\chi:\mathbb{R}^N\to \mathbb{R}^N$ is given by:
$\chi:\mathbb{R}^N\to \mathbb{R}^N$ is given by:
 \begin{equation*}
\chi(x):=\left\{
\begin{array}{ll}
x, &\ \text{if}\ |x|\leq \tilde{r},\\
\tilde{r}\frac{x}{|x|}, &\ \text{if}\ |x| \gt \tilde{r}.
\end{array}
\right.
\end{equation*}
\begin{equation*}
\chi(x):=\left\{
\begin{array}{ll}
x, &\ \text{if}\ |x|\leq \tilde{r},\\
\tilde{r}\frac{x}{|x|}, &\ \text{if}\ |x| \gt \tilde{r}.
\end{array}
\right.
\end{equation*} The next two lemmas will be useful to get important PS sequences for ![]() $E_{\epsilon,T}$ restricted to S(a).
$E_{\epsilon,T}$ restricted to S(a).
Lemma 4.6. Let ![]() $N, a,\eta,p,q$ and h be as in Equations (1.1) and (1.13) hold. Then, there exist
$N, a,\eta,p,q$ and h be as in Equations (1.1) and (1.13) hold. Then, there exist ![]() $\epsilon_2\in(0,\epsilon_1], \rho_1\in(0,\rho_0]$ such that if
$\epsilon_2\in(0,\epsilon_1], \rho_1\in(0,\rho_0]$ such that if ![]() $\epsilon\in(0, \epsilon_2)$,
$\epsilon\in(0, \epsilon_2)$, ![]() $u\in S(a)$ and
$u\in S(a)$ and ![]() $E_{\epsilon,T}(u)\leq \Upsilon_{\text{max},T,a}+\rho_1$, then
$E_{\epsilon,T}(u)\leq \Upsilon_{\text{max},T,a}+\rho_1$, then
 \begin{equation*}
Q_\epsilon(u)\in K_{\frac{\tilde{\rho}}{2}}.
\end{equation*}
\begin{equation*}
Q_\epsilon(u)\in K_{\frac{\tilde{\rho}}{2}}.
\end{equation*}Proof. If the lemma does not occur, there must be ![]() $\rho_n\to 0,
\epsilon_n\to 0$ and
$\rho_n\to 0,
\epsilon_n\to 0$ and ![]() $\{u_n\}\subset S(a)$ such that
$\{u_n\}\subset S(a)$ such that
 \begin{equation}
E_{\epsilon_n,T}(u_n)\leq \Upsilon_{\text{max},T,a}+\rho_n\
\qquad \text{and}\qquad Q_{\epsilon_n}(u_n)\not\in K_{\frac{\tilde{\rho}}{2}}.
\end{equation}
\begin{equation}
E_{\epsilon_n,T}(u_n)\leq \Upsilon_{\text{max},T,a}+\rho_n\
\qquad \text{and}\qquad Q_{\epsilon_n}(u_n)\not\in K_{\frac{\tilde{\rho}}{2}}.
\end{equation}Consequently,
then
According to Lemma 3.6, we have two cases:
(i) ![]() $u_n\to u$ in
$u_n\to u$ in ![]() $H^1(\mathbb{R}^N)$ for some
$H^1(\mathbb{R}^N)$ for some ![]() $u\in S(a)$,
$u\in S(a)$,
or
(ii) There exists ![]() $\{y_n\}\subset \mathbb{R}^N$ with
$\{y_n\}\subset \mathbb{R}^N$ with ![]() $|y_n|\to
+\infty$ such that
$|y_n|\to
+\infty$ such that ![]() $v_n(x) = u_n(x+ y_n)$ converges in
$v_n(x) = u_n(x+ y_n)$ converges in ![]() $H^1(\mathbb{R}^N)$ to some
$H^1(\mathbb{R}^N)$ to some ![]() $v\in S(a)$.
$v\in S(a)$.
Analysis of (i): By the Lebesgue dominated convergence theorem,
 \begin{equation*}
Q_{\epsilon_n}(u_n)=\frac{\int_{\mathbb{R}^N}\chi(\epsilon_n
x)|u_n|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u_n|^2\,{\rm d}x}\to
\frac{\int_{\mathbb{R}^N}\chi(0)|u|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u|^2\,{\rm d}x}=0\in
K_{\frac{\tilde{\rho}}{2}}.
\end{equation*}
\begin{equation*}
Q_{\epsilon_n}(u_n)=\frac{\int_{\mathbb{R}^N}\chi(\epsilon_n
x)|u_n|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u_n|^2\,{\rm d}x}\to
\frac{\int_{\mathbb{R}^N}\chi(0)|u|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u|^2\,{\rm d}x}=0\in
K_{\frac{\tilde{\rho}}{2}}.
\end{equation*} From this,  $Q_{\epsilon_n}(u_n)\in K_{\frac{\tilde{\rho}}{2}}$ for n large enough, which contradicts
$Q_{\epsilon_n}(u_n)\in K_{\frac{\tilde{\rho}}{2}}$ for n large enough, which contradicts  $Q_{\epsilon_n}(u_n)\not\in
K_{\frac{\tilde{\rho}}{2}}$ in Equation (4.14).
$Q_{\epsilon_n}(u_n)\not\in
K_{\frac{\tilde{\rho}}{2}}$ in Equation (4.14).
Analysis of (ii): Now, we will study two cases: (I) ![]() $|\epsilon_ny_n|\to +\infty$ and (II)
$|\epsilon_ny_n|\to +\infty$ and (II) ![]() $\epsilon_ny_n\to y$ for some
$\epsilon_ny_n\to y$ for some ![]() $y\in \mathbb{R}^N$.
$y\in \mathbb{R}^N$.
If (I) holds, the limit ![]() $v_n \to v$ in
$v_n \to v$ in ![]() $H^1(\mathbb{R}^N)$ provides
$H^1(\mathbb{R}^N)$ provides
 \begin{equation*}
\begin{split}
E_{\epsilon_n,T}(u_n)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon_n
x+\epsilon_ny_n)|v_n|^{q}\,{\rm d}x\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
v_n\|_2)\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\\
&\to J_{\infty,T}(v).
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
E_{\epsilon_n,T}(u_n)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon_n
x+\epsilon_ny_n)|v_n|^{q}\,{\rm d}x\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
v_n\|_2)\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\\
&\to J_{\infty,T}(v).
\end{split}
\end{equation*} Since ![]() $E_{\epsilon_n,T}(u_n)\leq \Upsilon_{\text{max},T,a}+\rho_n$, we deduce that
$E_{\epsilon_n,T}(u_n)\leq \Upsilon_{\text{max},T,a}+\rho_n$, we deduce that
which contradicts ![]() $\Upsilon_{\infty,T,a} \gt \Upsilon_{\text{max},T,a}$ in Lemma 4.1.
$\Upsilon_{\infty,T,a} \gt \Upsilon_{\text{max},T,a}$ in Lemma 4.1.
Now if (II) holds, then
 \begin{equation*}
\begin{split}
E_{\epsilon_n,T}(u_n)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon_n
x+\epsilon_ny_n)|v_n|^{q}\,{\rm d}x\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
v_n\|_2)\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\\
&\to J_{h(y),T}(v),
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
E_{\epsilon_n,T}(u_n)&=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
v_n|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon_n
x+\epsilon_ny_n)|v_n|^{q}\,{\rm d}x\\
&\qquad-\frac{\eta}{p}\tau(\|\nabla
v_n\|_2)\int_{\mathbb{R}^N}|v_n|^{p}\,{\rm d}x\\
&\to J_{h(y),T}(v),
\end{split}
\end{equation*} which combined with ![]() $E_{\epsilon_n,T}(u_n)\leq
\Upsilon_{\text{max},T,a}+\rho_n$ gives that
$E_{\epsilon_n,T}(u_n)\leq
\Upsilon_{\text{max},T,a}+\rho_n$ gives that
By Corollary 3.8, we must have ![]() $h(y)=h_{\text{max}}$ and
$h(y)=h_{\text{max}}$ and ![]() $y=a_i$ for some
$y=a_i$ for some ![]() $i=1,2,\ldots, l$. Hence,
$i=1,2,\ldots, l$. Hence,
 \begin{equation*}
\begin{split}
Q_{\epsilon_n}(u_n)=\frac{\int_{\mathbb{R}^N}\chi(\epsilon_n
x)|u_n|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u_n|^2\,{\rm d}x}
&=\frac{\int_{\mathbb{R}^N}\chi(\epsilon_n
x+\epsilon_ny_n)|v_n|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x}\\
&\to
\frac{\int_{\mathbb{R}^N}\chi(y)|v|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|v|^2\,{\rm d}x}=a_i\in
K_{\frac{\tilde{\rho}}{2}},
\end{split}
\end{equation*}
\begin{equation*}
\begin{split}
Q_{\epsilon_n}(u_n)=\frac{\int_{\mathbb{R}^N}\chi(\epsilon_n
x)|u_n|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u_n|^2\,{\rm d}x}
&=\frac{\int_{\mathbb{R}^N}\chi(\epsilon_n
x+\epsilon_ny_n)|v_n|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|v_n|^2\,{\rm d}x}\\
&\to
\frac{\int_{\mathbb{R}^N}\chi(y)|v|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|v|^2\,{\rm d}x}=a_i\in
K_{\frac{\tilde{\rho}}{2}},
\end{split}
\end{equation*} which implies that  $Q_{\epsilon_n}(u_n)\in
K_{\frac{\tilde{\rho}}{2}}$ for n large enough. That contradicts Equation (4.14). The proof is complete.
$Q_{\epsilon_n}(u_n)\in
K_{\frac{\tilde{\rho}}{2}}$ for n large enough. That contradicts Equation (4.14). The proof is complete.
From now on, we will use the following notations:
•
 $\theta_{\epsilon}^i:=\{u\in S(a):|Q_\epsilon(u)-a_i|\leq \tilde{\rho}\}$;
$\theta_{\epsilon}^i:=\{u\in S(a):|Q_\epsilon(u)-a_i|\leq \tilde{\rho}\}$;•
 $\partial\theta_{\epsilon}^i:=\{u\in S(a):|Q_\epsilon(u)-a_i|=\tilde{\rho}\}$;
$\partial\theta_{\epsilon}^i:=\{u\in S(a):|Q_\epsilon(u)-a_i|=\tilde{\rho}\}$;•
 $\beta_{\epsilon}^{i}:=\inf_{u\in\theta_{\epsilon}^i}E_{\epsilon,T}(u)$;
$\beta_{\epsilon}^{i}:=\inf_{u\in\theta_{\epsilon}^i}E_{\epsilon,T}(u)$;•
 $\tilde{\beta}_{\epsilon}^{i}:=\inf_{u\in\partial\theta_{\epsilon}^i}E_{\epsilon,T}(u)$.
$\tilde{\beta}_{\epsilon}^{i}:=\inf_{u\in\partial\theta_{\epsilon}^i}E_{\epsilon,T}(u)$.
Lemma 4.7. Let ![]() $N, a,\eta,p,q$ and h be as in Equations (1.1) and (1.13) hold, ϵ 2 and ρ 1 be obtained in Lemma 4.6. Then, there exists
$N, a,\eta,p,q$ and h be as in Equations (1.1) and (1.13) hold, ϵ 2 and ρ 1 be obtained in Lemma 4.6. Then, there exists ![]() $\epsilon_3\in (0,\epsilon_2]$ such that
$\epsilon_3\in (0,\epsilon_2]$ such that
 \begin{equation*}
\beta_{\epsilon}^i \lt \Upsilon_{\text{max},T,a}+\frac{\rho_1}{2}\qquad
\text{and}\qquad \beta_{\epsilon}^{i} \lt \tilde{\beta}_{\epsilon}^{i},\quad
\text{for}\ \text{any}\ \epsilon\in(0,\epsilon_3).
\end{equation*}
\begin{equation*}
\beta_{\epsilon}^i \lt \Upsilon_{\text{max},T,a}+\frac{\rho_1}{2}\qquad
\text{and}\qquad \beta_{\epsilon}^{i} \lt \tilde{\beta}_{\epsilon}^{i},\quad
\text{for}\ \text{any}\ \epsilon\in(0,\epsilon_3).
\end{equation*}Proof. Let ![]() $u\in S(a)$ be such that
$u\in S(a)$ be such that
For ![]() $1\leq i\leq l$, we define
$1\leq i\leq l$, we define
 \begin{equation*}
\hat{u}_{\epsilon}^{i}(x):=u\left(x-\frac{a_i}{\epsilon}\right),\quad
x\in \mathbb{R}^N.
\end{equation*}
\begin{equation*}
\hat{u}_{\epsilon}^{i}(x):=u\left(x-\frac{a_i}{\epsilon}\right),\quad
x\in \mathbb{R}^N.
\end{equation*} Then, ![]() $\hat{u}_{\epsilon}^{i}\in S(a)$ for all ϵ > 0 and
$\hat{u}_{\epsilon}^{i}\in S(a)$ for all ϵ > 0 and ![]() $1\leq i\leq l$. Direct calculations give that
$1\leq i\leq l$. Direct calculations give that
 \begin{equation*}
E_{\epsilon,T}(\hat{u}_{\epsilon}^{i})=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x+a_i)|u|^{q}\,{\rm d}x-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x,
\end{equation*}
\begin{equation*}
E_{\epsilon,T}(\hat{u}_{\epsilon}^{i})=\frac{1}{2}\int_{\mathbb{R}^N}|\nabla
u|^2\,{\rm d}x-\frac{1}{q}\int_{\mathbb{R}^N}h(\epsilon
x+a_i)|u|^{q}\,{\rm d}x-\frac{\eta}{p}\tau(\|\nabla
u\|_2)\int_{\mathbb{R}^N}|u|^{p}\,{\rm d}x,
\end{equation*}and then
 \begin{equation}
\lim_{\epsilon\to
0^+}E_{\epsilon,T}(\hat{u}_{\epsilon}^{i})=J_{h(a_i),T}(u)=J_{\text{max},T}(u)
=\Upsilon_{\text{max},T,a}.
\end{equation}
\begin{equation}
\lim_{\epsilon\to
0^+}E_{\epsilon,T}(\hat{u}_{\epsilon}^{i})=J_{h(a_i),T}(u)=J_{\text{max},T}(u)
=\Upsilon_{\text{max},T,a}.
\end{equation}Note that
 \begin{equation*}
Q_{\epsilon}(\hat{u}_{\epsilon}^i)=\frac{\int_{\mathbb{R}^N}\chi(\epsilon
x+a_i)|u|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u|^2\,{\rm d}x}\to a_i\text{ as}\
\epsilon\to 0^+.
\end{equation*}
\begin{equation*}
Q_{\epsilon}(\hat{u}_{\epsilon}^i)=\frac{\int_{\mathbb{R}^N}\chi(\epsilon
x+a_i)|u|^2\,{\rm d}x}{\int_{\mathbb{R}^N}|u|^2\,{\rm d}x}\to a_i\text{ as}\
\epsilon\to 0^+.
\end{equation*} So ![]() $\hat{u}_{\epsilon}^i\in \theta_{\epsilon}^i$ for ϵ small enough, which combined with Equation (4.15) implies that there exists
$\hat{u}_{\epsilon}^i\in \theta_{\epsilon}^i$ for ϵ small enough, which combined with Equation (4.15) implies that there exists ![]() $\epsilon_3\in(0,\epsilon_2]$ such that
$\epsilon_3\in(0,\epsilon_2]$ such that
 \begin{equation*}
\beta_{\epsilon}^i \lt \Upsilon_{\text{max},T,a}+\frac{\rho_1}{2},\quad\text{
for} \ \text{any}\ \epsilon\in (0,\epsilon_3).
\end{equation*}
\begin{equation*}
\beta_{\epsilon}^i \lt \Upsilon_{\text{max},T,a}+\frac{\rho_1}{2},\quad\text{
for} \ \text{any}\ \epsilon\in (0,\epsilon_3).
\end{equation*} For any ![]() $v\in \partial\theta_{\epsilon}^i$, that is,
$v\in \partial\theta_{\epsilon}^i$, that is, ![]() $v\in S(a)$ and
$v\in S(a)$ and ![]() $|Q_\epsilon(v)-a_i|=\tilde{\rho}$, we obtain that
$|Q_\epsilon(v)-a_i|=\tilde{\rho}$, we obtain that  $|Q_\epsilon(v)\not\in K_{\frac{\tilde{\rho}}{2}}$. Thus, by Lemma 4.6,
$|Q_\epsilon(v)\not\in K_{\frac{\tilde{\rho}}{2}}$. Thus, by Lemma 4.6,
which implies that
 \begin{equation*}
\tilde{\beta}_{\epsilon}^{i}=\inf_{v\in\partial\theta_{\epsilon}^i}
E_{\epsilon,T}(v)\geq \Upsilon_{\text{max},T,a}+\rho_1,\quad \text{for}\
\text{all} \ \epsilon\in(0,\epsilon_3).
\end{equation*}
\begin{equation*}
\tilde{\beta}_{\epsilon}^{i}=\inf_{v\in\partial\theta_{\epsilon}^i}
E_{\epsilon,T}(v)\geq \Upsilon_{\text{max},T,a}+\rho_1,\quad \text{for}\
\text{all} \ \epsilon\in(0,\epsilon_3).
\end{equation*}Thus,
 \begin{equation*}
\beta_{\epsilon}^{i} \lt \tilde{\beta}_{\epsilon}^{i},\quad \text{for}\ \text{all} \
\epsilon\in (0,\epsilon_3).
\end{equation*}
\begin{equation*}
\beta_{\epsilon}^{i} \lt \tilde{\beta}_{\epsilon}^{i},\quad \text{for}\ \text{all} \
\epsilon\in (0,\epsilon_3).
\end{equation*}Now we are ready to prove Theorem 1.1.
Proof of Theorem 1.1
Set ![]() $\epsilon_0:=\epsilon_3$, where ϵ 3 is obtained in Lemma 4.7. Let
$\epsilon_0:=\epsilon_3$, where ϵ 3 is obtained in Lemma 4.7. Let ![]() $\epsilon\in (0,\epsilon_0)$. By Lemma 4.7, for each
$\epsilon\in (0,\epsilon_0)$. By Lemma 4.7, for each ![]() $i\in \{1,2,\ldots, l\}$, we can use the Ekeland’s variational principle to find a sequence
$i\in \{1,2,\ldots, l\}$, we can use the Ekeland’s variational principle to find a sequence ![]() $\{u_n^{i})\subset \theta_{\epsilon}^i$ satisfying:
$\{u_n^{i})\subset \theta_{\epsilon}^i$ satisfying:
 \begin{equation*}
E_{\epsilon,T}(u_n^i)\to \beta_{\epsilon}^i\qquad {\rm and}\qquad
\|E_{\epsilon,T}|_{S(a)}^\prime(u_n^i)\|\to 0,\quad {\rm as}\ n\to +\infty,
\end{equation*}
\begin{equation*}
E_{\epsilon,T}(u_n^i)\to \beta_{\epsilon}^i\qquad {\rm and}\qquad
\|E_{\epsilon,T}|_{S(a)}^\prime(u_n^i)\|\to 0,\quad {\rm as}\ n\to +\infty,
\end{equation*} that is, ![]() $\{u_n^i\}_n$ is a
$\{u_n^i\}_n$ is a  $(PS)_{\beta_{\epsilon}^i}$ sequence for
$(PS)_{\beta_{\epsilon}^i}$ sequence for ![]() $E_{\epsilon,T}$ restricted to S(a). Since
$E_{\epsilon,T}$ restricted to S(a). Since ![]() $\beta_{\epsilon}^i \lt \Upsilon_{\text{max},T,a}+\rho_0$, it follows from Lemma 4.5 that there exists ui such that
$\beta_{\epsilon}^i \lt \Upsilon_{\text{max},T,a}+\rho_0$, it follows from Lemma 4.5 that there exists ui such that ![]() $u_n^i\to u^i$ in
$u_n^i\to u^i$ in ![]() $H^1(\mathbb{R}^N)$. Thus
$H^1(\mathbb{R}^N)$. Thus
 \begin{equation*}
u^i\in \theta_{\epsilon}^i,\ E_{\epsilon,T}(u^i)=\beta_{\epsilon}^i\qquad
\text{and}\qquad E_{\epsilon,T}|_{S(a)}^\prime(u^i)=0.
\end{equation*}
\begin{equation*}
u^i\in \theta_{\epsilon}^i,\ E_{\epsilon,T}(u^i)=\beta_{\epsilon}^i\qquad
\text{and}\qquad E_{\epsilon,T}|_{S(a)}^\prime(u^i)=0.
\end{equation*}As
 \begin{equation*}
Q_{\epsilon}(u^i)\in \overline{B_{\tilde{\rho}}(a_i)},\qquad
Q_{\epsilon}(u^j)\in \overline{B_{\tilde{\rho}}(a_j)},
\end{equation*}
\begin{equation*}
Q_{\epsilon}(u^i)\in \overline{B_{\tilde{\rho}}(a_i)},\qquad
Q_{\epsilon}(u^j)\in \overline{B_{\tilde{\rho}}(a_j)},
\end{equation*}and
 \begin{equation*}
\overline{B_{\tilde{\rho}}(a_i)}\cap
\overline{B_{\tilde{\rho}}(a_j)}=\emptyset\ ,\quad \text{for}\ i\neq j,
\end{equation*}
\begin{equation*}
\overline{B_{\tilde{\rho}}(a_i)}\cap
\overline{B_{\tilde{\rho}}(a_j)}=\emptyset\ ,\quad \text{for}\ i\neq j,
\end{equation*} we conclude that ![]() $u^i\not\equiv u^j$ for i ≠ j while
$u^i\not\equiv u^j$ for i ≠ j while ![]() $1\leq i, j
\leq l$. Therefore,
$1\leq i, j
\leq l$. Therefore, ![]() $E_{\epsilon, T}$ has at least l non-trivial critical points for all
$E_{\epsilon, T}$ has at least l non-trivial critical points for all ![]() $\epsilon\in (0, \epsilon_0)$.
$\epsilon\in (0, \epsilon_0)$.
As ![]() $E_{\epsilon, T}(u^i) \lt 0$ for any
$E_{\epsilon, T}(u^i) \lt 0$ for any ![]() $i=1,2,\ldots,l$, by Lemma 4.2,
$i=1,2,\ldots,l$, by Lemma 4.2, ![]() $u^i\, (i=1,2,\ldots,l)$ is in fact the critical point of Eϵ on S(a) with
$u^i\, (i=1,2,\ldots,l)$ is in fact the critical point of Eϵ on S(a) with ![]() $E_{\epsilon}(u^i) \lt 0$ and then there exists
$E_{\epsilon}(u^i) \lt 0$ and then there exists ![]() $\lambda_i\in \mathbb{R}$ such that
$\lambda_i\in \mathbb{R}$ such that
By using ![]() $E_{\epsilon}(u^i)=\beta_{\epsilon}^i \lt 0$ and
$E_{\epsilon}(u^i)=\beta_{\epsilon}^i \lt 0$ and ![]() $E_{\epsilon}^\prime(u^i)u^i=\lambda_ia^2$, we obtain that
$E_{\epsilon}^\prime(u^i)u^i=\lambda_ia^2$, we obtain that
 \begin{equation*}
\frac{1}{2}\lambda_ia^2=E_{\epsilon}(u^i)+\left(\frac{1}{q}-\frac{1}{2}\right)
\int_{\mathbb{R}^N}h(\epsilon
x)|u^i|^q\,{\rm d}x+\left(\frac{1}{p}-\frac{1}{2}\right)
\int_{\mathbb{R}^N}|u^i|^p\,{\rm d}x,
\end{equation*}
\begin{equation*}
\frac{1}{2}\lambda_ia^2=E_{\epsilon}(u^i)+\left(\frac{1}{q}-\frac{1}{2}\right)
\int_{\mathbb{R}^N}h(\epsilon
x)|u^i|^q\,{\rm d}x+\left(\frac{1}{p}-\frac{1}{2}\right)
\int_{\mathbb{R}^N}|u^i|^p\,{\rm d}x,
\end{equation*} which implies that ![]() $\lambda_i \lt 0$ for
$\lambda_i \lt 0$ for ![]() $i=1,2,\ldots,l$. The proof is complete.
$i=1,2,\ldots,l$. The proof is complete.
5. Stability
In this section, we investigate the stability of the solutions obtained in Theorem 1.1. For any ![]() $i=1,2,\ldots,l$, we define
$i=1,2,\ldots,l$, we define
 \begin{equation}
\begin{split}
\Omega_i:&=\{v\in \theta_{\epsilon}^i:\
E_{\epsilon,T}|_{S(a)}^\prime(v)=0,\quad
E_{\epsilon,T}(v)=\beta_{\epsilon}^i\}\\
&=\{v\in \theta_{\epsilon}^i:\ E_{\epsilon}|_{S(a)}^\prime(v)=0,\quad
E_{\epsilon}(v)=\beta_{\epsilon}^i, \ \|\nabla v\|_2\leq R_0\}.
\end{split}
\end{equation}
\begin{equation}
\begin{split}
\Omega_i:&=\{v\in \theta_{\epsilon}^i:\
E_{\epsilon,T}|_{S(a)}^\prime(v)=0,\quad
E_{\epsilon,T}(v)=\beta_{\epsilon}^i\}\\
&=\{v\in \theta_{\epsilon}^i:\ E_{\epsilon}|_{S(a)}^\prime(v)=0,\quad
E_{\epsilon}(v)=\beta_{\epsilon}^i, \ \|\nabla v\|_2\leq R_0\}.
\end{split}
\end{equation} Next we show the stability of the sets ![]() $\Omega_i\, (i=1,\ldots,l)$ in two cases
$\Omega_i\, (i=1,\ldots,l)$ in two cases ![]() $p \lt 2^*$ or
$p \lt 2^*$ or ![]() $p=2^*$.
$p=2^*$.
Proof of Theorem 1.4
Letting r 1 be such that ![]() $\bar{g}_a(r_1)=\beta_{\epsilon}^i$, and considering Equation (2.6), the definition of
$\bar{g}_a(r_1)=\beta_{\epsilon}^i$, and considering Equation (2.6), the definition of ![]() $\Omega_i$ and
$\Omega_i$ and ![]() $\beta_{\epsilon}^i \lt 0$, we know that
$\beta_{\epsilon}^i \lt 0$, we know that
Let ![]() $a_1 \gt a$ be such that
$a_1 \gt a$ be such that  $\bar{g}_{a_1}(R_0)=\frac{\beta_{\epsilon}^i}{2}$. There exists δ > 0 such that if
$\bar{g}_{a_1}(R_0)=\frac{\beta_{\epsilon}^i}{2}$. There exists δ > 0 such that if
 \begin{equation*}
u_0\in H^1(\mathbb{R}^N)\qquad \text{and}\qquad
\text{dist}_{H^1(\mathbb{R}^N)}(u_0,\Omega_i) \lt \delta,
\end{equation*}
\begin{equation*}
u_0\in H^1(\mathbb{R}^N)\qquad \text{and}\qquad
\text{dist}_{H^1(\mathbb{R}^N)}(u_0,\Omega_i) \lt \delta,
\end{equation*}then
 \begin{equation*}
\|u_0\|_2\leq a_1,\quad \|\nabla u_0\|_2\leq r_1+\frac{R_0-r_1}{2},\quad
E_{\epsilon,T}(u_0)\leq \frac{2}{3}\beta_{\epsilon}^i.
\end{equation*}
\begin{equation*}
\|u_0\|_2\leq a_1,\quad \|\nabla u_0\|_2\leq r_1+\frac{R_0-r_1}{2},\quad
E_{\epsilon,T}(u_0)\leq \frac{2}{3}\beta_{\epsilon}^i.
\end{equation*} Denoting by ![]() $\psi(t,\cdot)$ the solution to Equation (1.17) with initial value u 0 and denoting by
$\psi(t,\cdot)$ the solution to Equation (1.17) with initial value u 0 and denoting by ![]() $[0, T_{\text{max}})$ the maximal existence interval for
$[0, T_{\text{max}})$ the maximal existence interval for ![]() $\psi(t,\cdot)$, we have classically that either
$\psi(t,\cdot)$, we have classically that either ![]() $\psi(t,\cdot)$ is globally defined for positive times, or
$\psi(t,\cdot)$ is globally defined for positive times, or ![]() $\|\nabla \psi(t,\cdot)\|_2=+\infty$ as
$\|\nabla \psi(t,\cdot)\|_2=+\infty$ as ![]() $t\to T_{\text{max}}^-$, see ([Reference Tao, Visan and Zhang38], Section 3). Set
$t\to T_{\text{max}}^-$, see ([Reference Tao, Visan and Zhang38], Section 3). Set ![]() $\tilde{a}=\|u_0\|_2$. Note that
$\tilde{a}=\|u_0\|_2$. Note that ![]() $\|\psi(t,\cdot)\|_2=\|u_0\|_2$ for all
$\|\psi(t,\cdot)\|_2=\|u_0\|_2$ for all ![]() $t\in
(0,T_{\text{max}})$ by the conservation of the mass. If there exists
$t\in
(0,T_{\text{max}})$ by the conservation of the mass. If there exists ![]() $\tilde{t}\in (0,T_{\text{max}})$ such that
$\tilde{t}\in (0,T_{\text{max}})$ such that ![]() $\|\nabla
\psi(\tilde{t},\cdot)\|_2=R_0$, then
$\|\nabla
\psi(\tilde{t},\cdot)\|_2=R_0$, then
 \begin{equation*}
E_{\epsilon}(\psi(\tilde{t},\cdot))=E_{\epsilon,T}(\psi(\tilde{t},\cdot))
\geq \bar{g}_{\tilde{a}}(R_0)\geq
\bar{g}_{a_1}(R_0)=\frac{\beta_{\epsilon}^i}{2},
\end{equation*}
\begin{equation*}
E_{\epsilon}(\psi(\tilde{t},\cdot))=E_{\epsilon,T}(\psi(\tilde{t},\cdot))
\geq \bar{g}_{\tilde{a}}(R_0)\geq
\bar{g}_{a_1}(R_0)=\frac{\beta_{\epsilon}^i}{2},
\end{equation*}which contradicts the conservation of the energy:
 \begin{equation*}
E_{\epsilon}(\psi(t,\cdot))=E_{\epsilon}(u_0)\leq
\frac{2}{3}\beta_{\epsilon}^i,\quad \text{for}\ \text{all} \ t\in
(0,T_{\text{max}}).
\end{equation*}
\begin{equation*}
E_{\epsilon}(\psi(t,\cdot))=E_{\epsilon}(u_0)\leq
\frac{2}{3}\beta_{\epsilon}^i,\quad \text{for}\ \text{all} \ t\in
(0,T_{\text{max}}).
\end{equation*}Thus,
which implies that ![]() $\psi(t,\cdot)$ is globally defined for positive times.
$\psi(t,\cdot)$ is globally defined for positive times.
Next we prove that ![]() $\Omega_i$ is stable. The validity of Lemma 4.5 for complex valued function can be proved exactly as in Theorem 3.1 in [Reference Hajaiej and Stuart13]. Thus, the stability of
$\Omega_i$ is stable. The validity of Lemma 4.5 for complex valued function can be proved exactly as in Theorem 3.1 in [Reference Hajaiej and Stuart13]. Thus, the stability of ![]() $\Omega_i$ can be proved by modifying the classical Cazenave–Lions argument [Reference Cazenave and Lions10] (see also [Reference Li and Zhao24]). For the completeness, we give the proof here. Suppose by contradiction that there exist sequences
$\Omega_i$ can be proved by modifying the classical Cazenave–Lions argument [Reference Cazenave and Lions10] (see also [Reference Li and Zhao24]). For the completeness, we give the proof here. Suppose by contradiction that there exist sequences ![]() $\{u_{0,n}\}\subset H^1(\mathbb{R}^N)$ and
$\{u_{0,n}\}\subset H^1(\mathbb{R}^N)$ and ![]() $\{t_{n}\}\subset
\mathbb{R}^+$ and a constant
$\{t_{n}\}\subset
\mathbb{R}^+$ and a constant ![]() $\theta_0 \gt 0$ such that for all
$\theta_0 \gt 0$ such that for all ![]() $n\geq
1$,
$n\geq
1$,
 \begin{equation}
\inf_{v\in \Omega_i}\|u_{0,n}-v\| \lt \frac{1}{n}
\end{equation}
\begin{equation}
\inf_{v\in \Omega_i}\|u_{0,n}-v\| \lt \frac{1}{n}
\end{equation}and
 \begin{equation}
\inf_{v\in \Omega_i}\|\psi_n(t_n,\cdot)-v\|\geq \theta_0,
\end{equation}
\begin{equation}
\inf_{v\in \Omega_i}\|\psi_n(t_n,\cdot)-v\|\geq \theta_0,
\end{equation} where ![]() $\psi_n(t,\cdot)$ is the solution to (1.17) with initial value
$\psi_n(t,\cdot)$ is the solution to (1.17) with initial value ![]() $u_{0,n}$. By Equation (5.2), there exists n 0 such that for
$u_{0,n}$. By Equation (5.2), there exists n 0 such that for ![]() $n \gt n_0$ it holds that
$n \gt n_0$ it holds that ![]() $\|\nabla \psi_n(t,\cdot)\|_2 \lt R_0$ for all
$\|\nabla \psi_n(t,\cdot)\|_2 \lt R_0$ for all ![]() $t\geq 0$.
$t\geq 0$.
By Equation (5.3), there exists ![]() $\{v_n\}\subset \Omega_i$ such that
$\{v_n\}\subset \Omega_i$ such that
 \begin{equation}
\|u_{0,n}-v_n\| \lt \frac{2}{n}.
\end{equation}
\begin{equation}
\|u_{0,n}-v_n\| \lt \frac{2}{n}.
\end{equation} That ![]() $\{v_n\} \subset \Omega_i$ implies that
$\{v_n\} \subset \Omega_i$ implies that ![]() $\{v_n\}\subset
\theta_{\epsilon}^i$ is a
$\{v_n\}\subset
\theta_{\epsilon}^i$ is a  $(PS)_{\beta_{\epsilon}^i}$ sequence of
$(PS)_{\beta_{\epsilon}^i}$ sequence of ![]() $E_{\epsilon,T}$ restricted to S(a). From the proof of Theorem 1.1, there exists
$E_{\epsilon,T}$ restricted to S(a). From the proof of Theorem 1.1, there exists ![]() $v\in \Omega_i$ such that
$v\in \Omega_i$ such that
 \begin{equation*}
\lim_{n\to +\infty}\|v_n-v\|=0,
\end{equation*}
\begin{equation*}
\lim_{n\to +\infty}\|v_n-v\|=0,
\end{equation*}which combined with (5.5) gives that
 \begin{equation}
\lim_{n\to +\infty}\|u_{0,n}-v\|=0.
\end{equation}
\begin{equation}
\lim_{n\to +\infty}\|u_{0,n}-v\|=0.
\end{equation}Hence,
 \begin{equation*}
\lim_{n\to +\infty}\|u_{0,n}\|_2=\|v\|_2=a,\qquad \ \lim_{n\to
+\infty}E_{\epsilon}(u_{0,n})=E_{\epsilon}(v)={\beta}_{\epsilon}^i \lt \tilde{\beta}_{\epsilon}^i.
\end{equation*}
\begin{equation*}
\lim_{n\to +\infty}\|u_{0,n}\|_2=\|v\|_2=a,\qquad \ \lim_{n\to
+\infty}E_{\epsilon}(u_{0,n})=E_{\epsilon}(v)={\beta}_{\epsilon}^i \lt \tilde{\beta}_{\epsilon}^i.
\end{equation*}Then by the conservation of mass and energy, we obtain that
 \begin{equation}
\lim_{n\to +\infty}\|\psi_n(t,\cdot)\|_2=a,\qquad \ \lim_{n\to
+\infty}E_{\epsilon}(\psi_n(t,\cdot))={\beta}_{\epsilon}^i,\quad
\text{for}\ \text{any} \ t\geq 0.
\end{equation}
\begin{equation}
\lim_{n\to +\infty}\|\psi_n(t,\cdot)\|_2=a,\qquad \ \lim_{n\to
+\infty}E_{\epsilon}(\psi_n(t,\cdot))={\beta}_{\epsilon}^i,\quad
\text{for}\ \text{any} \ t\geq 0.
\end{equation}Define
 \begin{equation*}
\varphi_n(t,\cdot)=\frac{a\psi_n(t,\cdot)}{\|\psi_n(t,\cdot)\|_2},\quad
t\geq 0.
\end{equation*}
\begin{equation*}
\varphi_n(t,\cdot)=\frac{a\psi_n(t,\cdot)}{\|\psi_n(t,\cdot)\|_2},\quad
t\geq 0.
\end{equation*} Then ![]() $\varphi_n(t,\cdot)\in S(a)$ and
$\varphi_n(t,\cdot)\in S(a)$ and
which combined with Equation (5.6) gives that
 \begin{equation*}
\lim_{n\to+\infty}\|\varphi_n(0,\cdot)-v\|=\lim_{n\to+\infty}
\left\|\frac{au_{0,n}}{\|u_{0,n}\|_2}-v\right\|=0.
\end{equation*}
\begin{equation*}
\lim_{n\to+\infty}\|\varphi_n(0,\cdot)-v\|=\lim_{n\to+\infty}
\left\|\frac{au_{0,n}}{\|u_{0,n}\|_2}-v\right\|=0.
\end{equation*} Hence, ![]() $\varphi_n(0,\cdot)\in \theta_{\epsilon}^{i}\backslash
\partial\theta_{\epsilon}^{i}$ for n large enough because
$\varphi_n(0,\cdot)\in \theta_{\epsilon}^{i}\backslash
\partial\theta_{\epsilon}^{i}$ for n large enough because ![]() $v\in \theta_{\epsilon}^{i}\backslash
\partial\theta_{\epsilon}^{i}$. Using the method of continuity,
$v\in \theta_{\epsilon}^{i}\backslash
\partial\theta_{\epsilon}^{i}$. Using the method of continuity, ![]() $\lim_{n\to
+\infty}E_{\epsilon}(\varphi_n(t,\cdot))={\beta}_{\epsilon}^i$ for all
$\lim_{n\to
+\infty}E_{\epsilon}(\varphi_n(t,\cdot))={\beta}_{\epsilon}^i$ for all ![]() $t\geq 0$, and
$t\geq 0$, and ![]() ${\beta}_{\epsilon}^i \lt \tilde{\beta}_{\epsilon}^i$, we obtain that:
${\beta}_{\epsilon}^i \lt \tilde{\beta}_{\epsilon}^i$, we obtain that:
From (5.7)–(5.9), ![]() $\{\varphi_n(t_n,\cdot)\}\subset
\theta_{\epsilon}^i$ is a minimizing sequence of
$\{\varphi_n(t_n,\cdot)\}\subset
\theta_{\epsilon}^i$ is a minimizing sequence of ![]() $E_{\epsilon,T}$ at level
$E_{\epsilon,T}$ at level ![]() $\beta_{\epsilon}^i$, and from the proof of Theorem 1.1, there exists
$\beta_{\epsilon}^i$, and from the proof of Theorem 1.1, there exists ![]() $\tilde{v}\in \theta_{\epsilon}^i$ such that
$\tilde{v}\in \theta_{\epsilon}^i$ such that
 \begin{equation}
\lim_{n\to +\infty}\|\varphi_n(t_n,\cdot)-\tilde{v}\|=0,
\end{equation}
\begin{equation}
\lim_{n\to +\infty}\|\varphi_n(t_n,\cdot)-\tilde{v}\|=0,
\end{equation}which combined with (5.8) gives that
 \begin{equation*}
\lim_{n\to +\infty}\|\psi_n(t_n,\cdot)-\tilde{v}\|=0.
\end{equation*}
\begin{equation*}
\lim_{n\to +\infty}\|\psi_n(t_n,\cdot)-\tilde{v}\|=0.
\end{equation*} That contradicts (5.4). Hence ![]() $\Omega_i$ is stable for any
$\Omega_i$ is stable for any ![]() $i=1,2,\ldots,l$.
$i=1,2,\ldots,l$.
Proof of Theorem 1.5
The proof can be done by modifying the arguments of ![]() $\S$ 3 and 4 in [Reference Jeanjean, Jendrej, Le and Visciglia18] and using the arguments of the proof of Theorem 1.4, so we omit it.
$\S$ 3 and 4 in [Reference Jeanjean, Jendrej, Le and Visciglia18] and using the arguments of the proof of Theorem 1.4, so we omit it.
Funding Statement
This work is supported by the National Natural Science Foundation of China (No. 12001403).