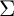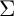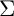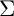Introduction
Let
![]() $\Sigma \subseteq \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
be a subset of the set of prime numbers
$\Sigma \subseteq \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
be a subset of the set of prime numbers
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
which is either equal to
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
which is either equal to
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
or of cardinality one. In the present paper, we continue our study of the pro-
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
or of cardinality one. In the present paper, we continue our study of the pro-
![]() $\Sigma $
fundamental groups of hyperbolic curves and their associated configuration spaces over algebraically closed fields in which the primes of
$\Sigma $
fundamental groups of hyperbolic curves and their associated configuration spaces over algebraically closed fields in which the primes of
![]() $\Sigma $
are invertible (cf. [Reference Hoshi and Mochizuki8]–[Reference Hoshi and Mochizuki11], [Reference Mochizuki18], [Reference Mochizuki20], [Reference Mochizuki and Tamagawa23]). The present paper focuses on the topic of understanding the relationship between the theory developed in earlier papers concerning pro-
$\Sigma $
are invertible (cf. [Reference Hoshi and Mochizuki8]–[Reference Hoshi and Mochizuki11], [Reference Mochizuki18], [Reference Mochizuki20], [Reference Mochizuki and Tamagawa23]). The present paper focuses on the topic of understanding the relationship between the theory developed in earlier papers concerning pro-
![]() $\Sigma $
fundamental groups and various discrete versions of this theory. This topic of comparison of pro-
$\Sigma $
fundamental groups and various discrete versions of this theory. This topic of comparison of pro-
![]() $\Sigma $
and discrete versions of the theory turns out to be closely related, in many situations, to the theory of sections of various natural surjections of profinite groups. Indeed, this relationship with the theory of sections is, in some sense, not surprising, inasmuch as sections typically amount to some sort of fixed point within a profinite continuum. That is to say, such fixed points are often closely related to the identification of a rigid discrete structure within the profinite continuum.
$\Sigma $
and discrete versions of the theory turns out to be closely related, in many situations, to the theory of sections of various natural surjections of profinite groups. Indeed, this relationship with the theory of sections is, in some sense, not surprising, inasmuch as sections typically amount to some sort of fixed point within a profinite continuum. That is to say, such fixed points are often closely related to the identification of a rigid discrete structure within the profinite continuum.
In §§1 and 2, we study two different aspects of this topic of comparison of pro-
![]() $\Sigma $
and discrete structures. Both §§1 and 2 follow the same pattern: we begin by proving an abstract and somewhat technical combinatorial result and then proceed to discuss various applications of this combinatorial result.
$\Sigma $
and discrete structures. Both §§1 and 2 follow the same pattern: we begin by proving an abstract and somewhat technical combinatorial result and then proceed to discuss various applications of this combinatorial result.
In §1, the main technical combinatorial result is summarized in Theorem A below (where
![]() $\Sigma $
is allowed to be an arbitrary nonempty set of prime numbers). This result consists of versions of the section conjecture and Grothendieck conjecture—that is, the central issues of concern in anabelian geometry—for outer representations of ENN-type (cf. Definition 1.7(i)). Here, we remark that outer representations of ENN-type are generalizations of the outer representations of NN-type studied in [Reference Hoshi and Mochizuki8]. Just as an outer representation of NN-type may be described, roughly speaking, as a purely combinatorial object modeled on the outer Galois representation arising from a hyperbolic curve over a complete discretely valued field whose residue field is separably closed, an outer representation of ENN-type may be described, again roughly speaking, as an analogous sort of purely combinatorial object that arises in the case where the residue field is not necessarily separably closed. The pro-
$\Sigma $
is allowed to be an arbitrary nonempty set of prime numbers). This result consists of versions of the section conjecture and Grothendieck conjecture—that is, the central issues of concern in anabelian geometry—for outer representations of ENN-type (cf. Definition 1.7(i)). Here, we remark that outer representations of ENN-type are generalizations of the outer representations of NN-type studied in [Reference Hoshi and Mochizuki8]. Just as an outer representation of NN-type may be described, roughly speaking, as a purely combinatorial object modeled on the outer Galois representation arising from a hyperbolic curve over a complete discretely valued field whose residue field is separably closed, an outer representation of ENN-type may be described, again roughly speaking, as an analogous sort of purely combinatorial object that arises in the case where the residue field is not necessarily separably closed. The pro-
![]() $\Sigma $
section conjecture portion of Theorem A (i.e., Theorem 1.13(i)) is then obtained by combining
$\Sigma $
section conjecture portion of Theorem A (i.e., Theorem 1.13(i)) is then obtained by combining
-
• the essential uniqueness of fixed points of certain group actions on profinite graphs given in [Reference Hoshi and Mochizuki8, Prop. 3.9(i)–(iii)] with
-
• an essentially classical result concerning the existence of fixed points (cf. Lemma 1.6 and Remarks 1.6.1 and 1.6.2), which amounts, in essence, to a geometric reformulation of the well-known fact that free pro-
 $\Sigma $
groups are torsion-free (cf. Remarks 1.13.1 and 1.15.2(i)).
$\Sigma $
groups are torsion-free (cf. Remarks 1.13.1 and 1.15.2(i)).
The argument applied to prove this pro-
![]() $\Sigma $
section conjecture portion of Theorem A is essentially similar to the argument applied in the tempered case discussed in [Reference Mochizuki17, Ths. 3.7 and 5.4], which is reviewed (in slightly greater generality) in the tempered section conjecture portion of Theorem A (cf. Theorem 1.13(ii)). These section conjecture portions of Theorem A imply, under suitable conditions, that there is a natural bijection between conjugacy classes of pro-
$\Sigma $
section conjecture portion of Theorem A is essentially similar to the argument applied in the tempered case discussed in [Reference Mochizuki17, Ths. 3.7 and 5.4], which is reviewed (in slightly greater generality) in the tempered section conjecture portion of Theorem A (cf. Theorem 1.13(ii)). These section conjecture portions of Theorem A imply, under suitable conditions, that there is a natural bijection between conjugacy classes of pro-
![]() $\Sigma $
and tempered sections (cf. Theorem 1.13(iii)). This implication may be regarded as an important example of the phenomenon discussed above, that is, that considerations concerning sections are closely related to the topic of comparison of pro-
$\Sigma $
and tempered sections (cf. Theorem 1.13(iii)). This implication may be regarded as an important example of the phenomenon discussed above, that is, that considerations concerning sections are closely related to the topic of comparison of pro-
![]() $\Sigma $
and discrete structures. Finally, by combining the pro-
$\Sigma $
and discrete structures. Finally, by combining the pro-
![]() $\Sigma $
section conjecture portion of Theorem A with the combinatorial version of the Grothendieck conjecture obtained in [Reference Hoshi and Mochizuki10, Th. 1.9(i)], one obtains the Grothendieck conjecture portion of Theorem A (cf. Corollary 1.14).
$\Sigma $
section conjecture portion of Theorem A with the combinatorial version of the Grothendieck conjecture obtained in [Reference Hoshi and Mochizuki10, Th. 1.9(i)], one obtains the Grothendieck conjecture portion of Theorem A (cf. Corollary 1.14).
Theorem A (Combinatorial versions of the section conjecture and Grothen dieck conjecture).
Let
![]() $\Sigma $
be a nonempty set of prime numbers, let
$\Sigma $
be a nonempty set of prime numbers, let
![]() $\mathcal {G}$
be a semi-graph of anabelioids of pro-
$\mathcal {G}$
be a semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type, let G be a profinite group, and let
$\Sigma $
PSC-type, let G be a profinite group, and let
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism that is of ENN-type for a conducting subgroup
$\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism that is of ENN-type for a conducting subgroup
![]() $I_G\subseteq G$
(cf. Definition 1.7(i)). Write
$I_G\subseteq G$
(cf. Definition 1.7(i)). Write
![]() $\Pi _{\mathcal {G}}$
for the (pro-
$\Pi _{\mathcal {G}}$
for the (pro-
![]() $\Sigma $
) fundamental group of
$\Sigma $
) fundamental group of
![]() $\mathcal {G}$
and
$\mathcal {G}$
and
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
for the tempered fundamental group of
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
for the tempered fundamental group of
![]() $\mathcal {G}$
(cf. [Reference Mochizuki17, Exam. 2.10] and the discussion preceding [Reference Mochizuki17, Prop. 3.6]). (Thus, we have a natural outer injection
$\mathcal {G}$
(cf. [Reference Mochizuki17, Exam. 2.10] and the discussion preceding [Reference Mochizuki17, Prop. 3.6]). (Thus, we have a natural outer injection
![]() $\Pi _{\mathcal {G}} ^{\mathrm {tp}} \hookrightarrow \Pi _{\mathcal {G}}$
—cf. [Reference Hoshi and Mochizuki11, Lem. 3.2(i)] and the proof of [Reference Hoshi and Mochizuki11, Prop. 3.3(i) and (ii)].) Write
$\Pi _{\mathcal {G}} ^{\mathrm {tp}} \hookrightarrow \Pi _{\mathcal {G}}$
—cf. [Reference Hoshi and Mochizuki11, Lem. 3.2(i)] and the proof of [Reference Hoshi and Mochizuki11, Prop. 3.3(i) and (ii)].) Write
![]() $\Pi _{G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } G$
(cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]);
$\Pi _{G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } G$
(cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]);
![]() $\Pi _{G}^{\mathrm {tp}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^{\mathrm {tp}}\stackrel {\mathrm {out}}{\rtimes } G$
;
$\Pi _{G}^{\mathrm {tp}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^{\mathrm {tp}}\stackrel {\mathrm {out}}{\rtimes } G$
;
![]() $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
,
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
,
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow {\mathcal {G}}$
for the universal pro-
$\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow {\mathcal {G}}$
for the universal pro-
![]() $\Sigma $
and pro-tempered coverings of
$\Sigma $
and pro-tempered coverings of
![]() $\mathcal {G}$
corresponding to
$\mathcal {G}$
corresponding to
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
;
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
;
![]() $\mathrm {VCN}(-)$
for the set of vertices, cusps, and nodes of the underlying (pro-)semi-graph of a (pro-)semi-graph of anabelioids (cf. Definition 1.1(i)). Thus, we have a natural commutative diagram
$\mathrm {VCN}(-)$
for the set of vertices, cusps, and nodes of the underlying (pro-)semi-graph of a (pro-)semi-graph of anabelioids (cf. Definition 1.1(i)). Thus, we have a natural commutative diagram

—where the horizontal sequences are exact, and the vertical arrows are outer injections;
![]() $\Pi _{G}^{\mathrm {tp}}$
acts naturally on
$\Pi _{G}^{\mathrm {tp}}$
acts naturally on
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}}$
;
$\widetilde {\mathcal {G}}^{\mathrm {tp}}$
;
![]() $\Pi _{G}$
acts naturally on
$\Pi _{G}$
acts naturally on
![]() $\widetilde {\mathcal {G}}$
. Then the following hold:
$\widetilde {\mathcal {G}}$
. Then the following hold:
-
(i) Suppose that
 $\rho $
is l-cyclotomically full (cf. Definition 1.7(ii)) for some
$\rho $
is l-cyclotomically full (cf. Definition 1.7(ii)) for some
 $l\in \Sigma $
. Let
$l\in \Sigma $
. Let
 $s \colon G \rightarrow \Pi _{G}$
be a continuous section of the natural surjection
$s \colon G \rightarrow \Pi _{G}$
be a continuous section of the natural surjection
 $\Pi _{G} \twoheadrightarrow G$
. Then, relative to the action of
$\Pi _{G} \twoheadrightarrow G$
. Then, relative to the action of
 $\Pi _{G}$
on
$\Pi _{G}$
on
 $\mathrm {VCN}(\widetilde {\mathcal {G}})$
via conjugation of VCN-subgroups, the image of s stabilizes some element of
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
via conjugation of VCN-subgroups, the image of s stabilizes some element of
 $\mathrm {VCN}(\widetilde {\mathcal {G}})$
.
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
. -
(ii) Let
 $s \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be a continuous section of the natural surjection
$s \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be a continuous section of the natural surjection
 $\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then, relative to the action of
$\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then, relative to the action of
 $\Pi _{G}^{\mathrm {tp}}$
on
$\Pi _{G}^{\mathrm {tp}}$
on
 $\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
via conjugation of VCN-subgroups (cf. Definition 1.9), the image of s stabilizes some element of
$\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
via conjugation of VCN-subgroups (cf. Definition 1.9), the image of s stabilizes some element of
 $\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
.
$\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
. -
(iii) Write
 $\mathrm {Sect}(\Pi _{G} / G)$
for the set of
$\mathrm {Sect}(\Pi _{G} / G)$
for the set of
 $\Pi _{\mathcal {G}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
$\Pi _{\mathcal {G}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
 $\Pi _{G} \twoheadrightarrow G$
and
$\Pi _{G} \twoheadrightarrow G$
and
 $\mathrm {Sect}(\Pi _{G}^{\mathrm {tp}} / G)$
for the set of
$\mathrm {Sect}(\Pi _{G}^{\mathrm {tp}} / G)$
for the set of
 $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
 $\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then the natural map is injective. If, moreover,
$\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then the natural map is injective. If, moreover, $$\begin{align*}\mathrm{Sect}(\Pi_{G}^{\mathrm{tp}} / G) \longrightarrow \mathrm{Sect}(\Pi_{G} / G) \end{align*}$$
$$\begin{align*}\mathrm{Sect}(\Pi_{G}^{\mathrm{tp}} / G) \longrightarrow \mathrm{Sect}(\Pi_{G} / G) \end{align*}$$
 $\rho $
is l-cyclotomically full for some
$\rho $
is l-cyclotomically full for some
 $l\in \Sigma $
, then this map is bijective.
$l\in \Sigma $
, then this map is bijective.
-
(iv) Let
 $\mathcal {H}$
be a semi-graph of anabelioids of pro-
$\mathcal {H}$
be a semi-graph of anabelioids of pro-
 $\Sigma $
PSC-type, let H be a profinite group, and let
$\Sigma $
PSC-type, let H be a profinite group, and let
 $\rho _{\mathcal {H}}\colon H\rightarrow \mathrm {Aut}(\mathcal {H})$
be a continuous homomorphism that is of ENN-type for a conducting subgroup
$\rho _{\mathcal {H}}\colon H\rightarrow \mathrm {Aut}(\mathcal {H})$
be a continuous homomorphism that is of ENN-type for a conducting subgroup
 $I_{H} \subseteq H$
. Write
$I_{H} \subseteq H$
. Write
 $\Pi _{\mathcal {H}}$
for the (pro-
$\Pi _{\mathcal {H}}$
for the (pro-
 $\Sigma $
) fundamental group of
$\Sigma $
) fundamental group of
 $\mathcal {H}$
. Suppose further that
$\mathcal {H}$
. Suppose further that
 $\rho $
is verticially quasi-split (cf. Definition 1.7(i)). Let
$\rho $
is verticially quasi-split (cf. Definition 1.7(i)). Let
 $\beta \colon G \stackrel {\sim }{\rightarrow } H$
be a continuous isomorphism such that
$\beta \colon G \stackrel {\sim }{\rightarrow } H$
be a continuous isomorphism such that
 $\beta (I_{G})=I_{H}$
; let
$\beta (I_{G})=I_{H}$
; let
 $l\in \Sigma $
be a prime number such that
$l\in \Sigma $
be a prime number such that
 $\rho _{\mathcal {G}} \stackrel {\mathrm {def}}{=} \rho $
and
$\rho _{\mathcal {G}} \stackrel {\mathrm {def}}{=} \rho $
and
 $\rho _{\mathcal {H}}$
are l-cyclotomically full; let
$\rho _{\mathcal {H}}$
are l-cyclotomically full; let
 $\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
be a continuous isomorphism such that the diagram —where the right-hand vertical arrow is the isomorphism obtained by conjugating by
$\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
be a continuous isomorphism such that the diagram —where the right-hand vertical arrow is the isomorphism obtained by conjugating by
 $\alpha $
—commutes. Then
$\alpha $
—commutes. Then
 $\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
$\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
The purely combinatorial theory of §1—that is, the theory surrounding and including Theorem A—has important applications to scheme theory—that is, to the theory of hyperbolic curves over quite general complete discretely valued fields—as follows:
-
(A-1) We observe that a quite general result in the style of the main results of [Reference Pop and Stix26] concerning valuations fixed by sections of the arithmetic fundamental group follows formally, in the case of hyperbolic curves over quite general complete discretely valued fields, from Theorem A (cf. Corollary 1.15(iii) and Remark 1.15.2(i) and (ii)). The quite substantial generality of this result is a reflection of the purely combinatorial nature of Theorem A. This approach contrasts substantially with the approach of [Reference Pop and Stix26] via essentially scheme-theoretic techniques such as the local–global principle for the Brauer group (cf. Remark 1.15.2(i)). The approach of the present paper also differs substantially from [Reference Pop and Stix26] in that the transition from fixed points of graphs to fixed valuations is treated as a formal consequence of well-known elementary properties of Berkovich spaces, that is, in essence the compactness of the unit interval
 $[0, 1] \subseteq \mathbb {R}$
(cf. Remark 1.15.2(ii)).
$[0, 1] \subseteq \mathbb {R}$
(cf. Remark 1.15.2(ii)). -
(A-2) We observe that the natural bijection between conjugacy classes of pro-
 $\Sigma $
and tempered sections discussed in the purely combinatorial setting of Theorem A implies a similar bijection in the case of hyperbolic curves over quite general complete discretely valued fields (cf. Corollary 1.15(vi)). This portion of the theory was partially motivated by discussions between the second author and Y. André.
$\Sigma $
and tempered sections discussed in the purely combinatorial setting of Theorem A implies a similar bijection in the case of hyperbolic curves over quite general complete discretely valued fields (cf. Corollary 1.15(vi)). This portion of the theory was partially motivated by discussions between the second author and Y. André.
In the context of (A-1), we remark that, in the Appendix to the present paper, we give an elementary exposition from the point of view of two-dimensional log regular log schemes of the phenomenon of convergence of valuations, without applying the language or notions, such as Stone–Čech compactifications, typically applied in expositions of the theory of Berkovich spaces.
In §2, we turn to the task of formulating discrete analogues of a substantial portion of the theory developed in earlier papers. This formulation centers around the notion of a semi-graph of temperoids of HSD-type (i.e., “hyperbolic surface decomposition type”—cf. Definition 2.3(iii)), which may be thought of as a natural discrete analogue of the notion of a semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). As the name suggests, this notion may be thought of as referring to the sort of collection of discrete combinatorial data that one may associate with a decomposition of a hyperbolic surface into hyperbolic subsurfaces. Alternatively, it may be thought of as referring to the sort of collection of combinatorial data that arise from systems of topological coverings of the system of topological spaces naturally associated with a stable log curve over a log point whose underlying scheme is the spectrum of the field of complex numbers (cf. Example 2.4(i)). After discussing various basic properties and terms related to semi-graphs of temperoids of HSD-type (cf. Proposition 2.5 and Definitions 2.6 and 2.7), we observe that the fundamental operations of restriction, partial compactification, resolution, and generization discussed in [Reference Hoshi and Mochizuki9, §2], admit natural compatible analogues for semi-graphs of temperoids of HSD-type (cf. Definitions 2.8 and 2.9 and Proposition 2.10).
$\Sigma $
PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). As the name suggests, this notion may be thought of as referring to the sort of collection of discrete combinatorial data that one may associate with a decomposition of a hyperbolic surface into hyperbolic subsurfaces. Alternatively, it may be thought of as referring to the sort of collection of combinatorial data that arise from systems of topological coverings of the system of topological spaces naturally associated with a stable log curve over a log point whose underlying scheme is the spectrum of the field of complex numbers (cf. Example 2.4(i)). After discussing various basic properties and terms related to semi-graphs of temperoids of HSD-type (cf. Proposition 2.5 and Definitions 2.6 and 2.7), we observe that the fundamental operations of restriction, partial compactification, resolution, and generization discussed in [Reference Hoshi and Mochizuki9, §2], admit natural compatible analogues for semi-graphs of temperoids of HSD-type (cf. Definitions 2.8 and 2.9 and Proposition 2.10).
The main technical combinatorial result of §2 is summarized in Theorem B below. This result asserts, in effect, that discrete subgroups of the discrete fundamental group of a semi-graph of temperoids of HSD-type satisfy various properties of interest if and only if the profinite completions of these discrete subgroups satisfy analogous properties (cf. Theorem 2.15 and Corollary 2.19(i)). The main technical tool that is applied in order to derive this result is the fact that any inclusion of a finitely generated group into a (finitely generated) free discrete group is, after possibly passing to a suitable finite index subgroup, necessarily split (cf. [Reference Mochizuki17, Cor. 1.6(ii)], which is applied in the proof of Lemma 2.14(i) of the present paper). Here, we recall that in [Reference Mochizuki17], this fact (i.e., [Reference Mochizuki17, Cor. 1.6(ii)]) is obtained as an immediate consequence of “Zariski’s main theorem for semi-graphs” (cf. [Reference Mochizuki17, Th. 1.2]).
Theorem B (Profinite versus discrete subgroups).
Let
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
be semi-graphs of temperoids of HSD-type (cf. Definition 2.3(iii)). Write
$\mathcal {H}$
be semi-graphs of temperoids of HSD-type (cf. Definition 2.3(iii)). Write
![]() $\widehat {\mathcal {G}}$
,
$\widehat {\mathcal {G}}$
,
![]() $\widehat {\mathcal {H}}$
for the semi-graphs of anabelioids of pro-
$\widehat {\mathcal {H}}$
for the semi-graphs of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type determined by
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type determined by
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
(cf. Proposition 2.5(iii) in the case where
$\mathcal {H}$
(cf. Proposition 2.5(iii) in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
), respectively;
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
), respectively;
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {H}}$
for the respective fundamental groups of
$\Pi _{\mathcal {H}}$
for the respective fundamental groups of
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
(cf. Proposition 2.5(i));
$\mathcal {H}$
(cf. Proposition 2.5(i));
![]() $\Pi _{\widehat {\mathcal {G}}}$
,
$\Pi _{\widehat {\mathcal {G}}}$
,
![]() $\Pi _{\widehat {\mathcal {H}}}$
for the respective (profinite) fundamental groups of
$\Pi _{\widehat {\mathcal {H}}}$
for the respective (profinite) fundamental groups of
![]() $\widehat {\mathcal {G}}$
,
$\widehat {\mathcal {G}}$
,
![]() $\widehat {\mathcal {H}}$
. Then the following hold:
$\widehat {\mathcal {H}}$
. Then the following hold:
-
(i) Let H,
 $J \subseteq \Pi _{\mathcal {G}}$
be subgroups. Since
$J \subseteq \Pi _{\mathcal {G}}$
be subgroups. Since
 $\Pi _{\mathcal {G}}$
injects into its pro-l completion for any
$\Pi _{\mathcal {G}}$
injects into its pro-l completion for any
 $l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Remark 2.5.1), let us regard subgroups of
$l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Remark 2.5.1), let us regard subgroups of
 $\Pi _{\mathcal {G}}$
as subgroups of the profinite completion
$\Pi _{\mathcal {G}}$
as subgroups of the profinite completion
 $\widehat {\Pi }_{\mathcal {G}}$
of
$\widehat {\Pi }_{\mathcal {G}}$
of
 $\Pi _{\mathcal {G}}$
. Write
$\Pi _{\mathcal {G}}$
. Write
 $\overline {H}$
,
$\overline {H}$
,
 $\overline {J} \subseteq \widehat {\Pi }_{\mathcal {G}}$
for the closures of H, J in
$\overline {J} \subseteq \widehat {\Pi }_{\mathcal {G}}$
for the closures of H, J in
 $\widehat {\Pi }_{\mathcal {G}}$
, respectively. Suppose that the following conditions are satisfied:
$\widehat {\Pi }_{\mathcal {G}}$
, respectively. Suppose that the following conditions are satisfied:-
(a) The subgroups H and J are finitely generated.
-
(b) If J is of infinite index in
 $\Pi _{\mathcal {G}}$
, then
$\Pi _{\mathcal {G}}$
, then
 $\overline J$
is of infinite index in
$\overline J$
is of infinite index in
 $\widehat {\Pi }_{\mathcal {G}}$
.
$\widehat {\Pi }_{\mathcal {G}}$
.
(Here, we note that condition (b) is automatically satisfied whenever
 $\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
—cf. [Reference Mochizuki17, Cor. 1.6(ii)].) Then the following hold:
$\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
—cf. [Reference Mochizuki17, Cor. 1.6(ii)].) Then the following hold:-
(1) It holds that
 $J = \overline {J} \cap \Pi _{\mathcal {G}}$
.
$J = \overline {J} \cap \Pi _{\mathcal {G}}$
. -
(2) Suppose that there exists an element
 $\widehat {\gamma } \in \widehat {\Pi }_{\mathcal {G}}$
such that Then there exists an element
$\widehat {\gamma } \in \widehat {\Pi }_{\mathcal {G}}$
such that Then there exists an element $$\begin{align*}H \subseteq \widehat{\gamma} \cdot \overline{J} \cdot \widehat{\gamma}^{-1}. \end{align*}$$
$$\begin{align*}H \subseteq \widehat{\gamma} \cdot \overline{J} \cdot \widehat{\gamma}^{-1}. \end{align*}$$
 $\delta \in \Pi _{\mathcal {G}}$
such that
$\delta \in \Pi _{\mathcal {G}}$
such that  $$\begin{align*}H \subseteq \delta \cdot J \cdot \delta^{-1}. \end{align*}$$
$$\begin{align*}H \subseteq \delta \cdot J \cdot \delta^{-1}. \end{align*}$$
-
-
(ii) Let
be an outer isomorphism. Write $$ \begin{align*}\alpha\colon \Pi_{\mathcal{G}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{H}}\end{align*} $$
$$ \begin{align*}\alpha\colon \Pi_{\mathcal{G}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{H}}\end{align*} $$
 $\widehat {\alpha }\colon \Pi _{\widehat {\mathcal {G}}}\stackrel {\sim }{\rightarrow }\Pi _{\widehat {\mathcal {H}}}$
for the outer isomorphism determined by
$\widehat {\alpha }\colon \Pi _{\widehat {\mathcal {G}}}\stackrel {\sim }{\rightarrow }\Pi _{\widehat {\mathcal {H}}}$
for the outer isomorphism determined by
 $\alpha $
and the natural outer isomorphisms
$\alpha $
and the natural outer isomorphisms
 $\widehat {\Pi }_{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {G}}}$
,
$\widehat {\Pi }_{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {G}}}$
,
 $\widehat {\Pi }_{\mathcal {H}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {H}}}$
of Proposition 2.5(iii). Then the outer isomorphism
$\widehat {\Pi }_{\mathcal {H}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {H}}}$
of Proposition 2.5(iii). Then the outer isomorphism
 $\alpha $
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal; graphic) (cf. Definition 2.7(i) and (ii)) if and only if the outer isomorphism
$\alpha $
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal; graphic) (cf. Definition 2.7(i) and (ii)) if and only if the outer isomorphism
 $\widehat {\alpha }$
is group-theoretically verticial (cf. [Reference Mochizuki18, Def. 1.4(iv)]) (resp. group-theoretically cuspidal [cf. [Reference Mochizuki18, Def. 1.4(iv)]]; group-theoretically nodal [cf. [Reference Hoshi and Mochizuki8, Def. 1.12]]; graphic [cf. [Reference Mochizuki18, Def. 1.4(i)]]).
$\widehat {\alpha }$
is group-theoretically verticial (cf. [Reference Mochizuki18, Def. 1.4(iv)]) (resp. group-theoretically cuspidal [cf. [Reference Mochizuki18, Def. 1.4(iv)]]; group-theoretically nodal [cf. [Reference Hoshi and Mochizuki8, Def. 1.12]]; graphic [cf. [Reference Mochizuki18, Def. 1.4(i)]]).
The significance of Theorem B lies in the fact that it renders possible a fairly straightforward translation of a substantial portion of the profinite results obtained in earlier papers by the authors into discrete versions, as follows:
-
(B-1) the partial combinatorial cuspidalization obtained in [Reference Hoshi and Mochizuki9, Th. A] and [Reference Hoshi and Mochizuki10, Th. A] (cf. Corollary 2.20 of the present paper);
-
(B-2) the theory of Dehn multi-twists summarized in [Reference Hoshi and Mochizuki9, Th. B] (cf. Corollary 2.21 of the present paper);
-
(B-3) the theory of the tripod homomorphism and metric-admissibility summarized in [Reference Hoshi and Mochizuki10, Th. C] and [Reference Hoshi and Mochizuki11, Ths. A, C, and D] (cf. Theorem 2.24 of the present paper);
-
(B-4) the archimedean analogue (cf. Corollary 2.25 of the present paper) of the characterization, given in [Reference Hoshi and Mochizuki11, Th. B], of nonarchimedean local Galois groups in the global Galois image associated with a hyperbolic curve.
Finally, in §3, we examine the theory of canonical liftings of cycles discussed in [Reference Boggi5] from the point of view of the profinite theory developed so far by the authors. This approach contrasts substantially with the intuitive topological approach of [Reference Boggi5] in the discrete case. From a naive topological point of view, the canonical liftings of cycles in question amount to once-punctured tubular neighborhoods of the given cycles (cf. Figure 1), that is, to the construction of a tripod (i.e., a copy of the projective line minus three points) canonically and functorially associated with the cycle. This tripod satisfies a remarkable rigidity property, that is, it admits a canonical isomorphism, subject to almost no indeterminacies, with a given fixed tripod that is independent of the choice of the cycle. Moreover, this canonical isomorphism is functorial with respect to “geometric” outer automorphisms of the profinite fundamental group of the stable log curve under consideration that lift to automorphisms of the profinite fundamental group of a configuration space (associated with the stable log curve) of sufficiently high dimension. Here, by “geometric,” we mean that the outer automorphism under consideration lies in the kernel of the tripod homomorphism studied in [Reference Hoshi and Mochizuki10, §3]. Indeed, this remarkable rigidity property is obtained as an immediate consequence of the theory of tripod synchronization developed in [Reference Hoshi and Mochizuki10, §3].

Figure 1 A cycle and lifting cycles.
The profinite version of the theory of canonical liftings of cycles developed in §3 is summarized in Theorem C below (cf. Theorem 3.10). By applying the translation apparatus developed in §2 to this profinite version of the theory, we also obtain a corresponding discrete version of the theory of canonical liftings of cycles (cf. Theorem 3.14).
Theorem C (Canonical liftings of cycles).
Let
![]() $(g,r)$
be a pair of nonnegative integers such that
$(g,r)$
be a pair of nonnegative integers such that
![]() $2g-2+r> 0$
; let
$2g-2+r> 0$
; let
![]() $\Sigma $
be a set of prime numbers which is either equal to the entire set of prime numbers or of cardinality one; let k be an algebraically closed field of characteristic
$\Sigma $
be a set of prime numbers which is either equal to the entire set of prime numbers or of cardinality one; let k be an algebraically closed field of characteristic
![]() $\not \in \Sigma $
; let
$\not \in \Sigma $
; let
![]() $S^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)^{\mathrm {log}}$
be the log scheme obtained by equipping
$S^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)^{\mathrm {log}}$
be the log scheme obtained by equipping
![]() $S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)$
with the log structure determined by the fs chart
$S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)$
with the log structure determined by the fs chart
![]() $\mathbb {N} \rightarrow k$
that maps
$\mathbb {N} \rightarrow k$
that maps
![]() $1 \mapsto 0$
; let
$1 \mapsto 0$
; let
![]() $X^{\mathrm {log}} = X_1^{\mathrm {log}}$
be a stable log curve of type
$X^{\mathrm {log}} = X_1^{\mathrm {log}}$
be a stable log curve of type
![]() $(g,r)$
over
$(g,r)$
over
![]() $S^{\mathrm {log}}$
. For positive integers
$S^{\mathrm {log}}$
. For positive integers
![]() $m\leq n$
, write
$m\leq n$
, write
for the nth log configuration space of the stable log curve
![]() $X^{\mathrm {log}}$
(cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]);
$X^{\mathrm {log}}$
(cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]);
for the maximal pro-
![]() $\Sigma $
quotient of the kernel of the natural surjection
$\Sigma $
quotient of the kernel of the natural surjection
![]() $\pi _1(X_n^{\mathrm {log}})\twoheadrightarrow \pi _1(S^{\mathrm {log}})$
;
$\pi _1(X_n^{\mathrm {log}})\twoheadrightarrow \pi _1(S^{\mathrm {log}})$
;
for the objects defined in the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3] and [Reference Hoshi and Mochizuki10, Def. 3.1]. Let
![]() $I \subseteq \Pi _{2/1} \subseteq \Pi _{2}$
be a cuspidal inertia group associated with the diagonal cusp of a fiber of
$I \subseteq \Pi _{2/1} \subseteq \Pi _{2}$
be a cuspidal inertia group associated with the diagonal cusp of a fiber of
![]() $p_{2/1}^{\mathrm {log}}$
; let
$p_{2/1}^{\mathrm {log}}$
; let
![]() $\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}$
be a
$\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}$
be a
![]() $3$
-central
$3$
-central
![]() $\{1,2,3\}$
-tripod of
$\{1,2,3\}$
-tripod of
![]() $\Pi _{3}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]); let
$\Pi _{3}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]); let
![]() $I_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
be a cuspidal subgroup of
$I_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
be a cuspidal subgroup of
![]() $\Pi _{\mathrm {tpd}}$
that does not arise from a cusp of a fiber of
$\Pi _{\mathrm {tpd}}$
that does not arise from a cusp of a fiber of
![]() $p_{3/2}^{\mathrm {log}}$
; let
$p_{3/2}^{\mathrm {log}}$
; let
![]() $J^{*}_{\mathrm {tpd}}$
,
$J^{*}_{\mathrm {tpd}}$
,
![]() $J^{**}_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
be cuspidal subgroups of
$J^{**}_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
be cuspidal subgroups of
![]() $\Pi _{\mathrm {tpd}}$
such that
$\Pi _{\mathrm {tpd}}$
such that
![]() $I_{\mathrm {tpd}}$
,
$I_{\mathrm {tpd}}$
,
![]() $J^{*}_{\mathrm {tpd}}$
, and
$J^{*}_{\mathrm {tpd}}$
, and
![]() $J^{**}_{\mathrm {tpd}}$
determine three distinct
$J^{**}_{\mathrm {tpd}}$
determine three distinct
![]() $\Pi _{\mathrm {tpd}}$
-conjugacy classes of closed subgroups of
$\Pi _{\mathrm {tpd}}$
-conjugacy classes of closed subgroups of
![]() $\Pi _{\mathrm {tpd}}$
. (Note that one verifies immediately from the various definitions involved that such cuspidal subgroups
$\Pi _{\mathrm {tpd}}$
. (Note that one verifies immediately from the various definitions involved that such cuspidal subgroups
![]() $I_{\mathrm {tpd}}$
,
$I_{\mathrm {tpd}}$
,
![]() $J^{*}_{\mathrm {tpd}}$
, and
$J^{*}_{\mathrm {tpd}}$
, and
![]() $J^{**}_{\mathrm {tpd}}$
always exist.) For positive integers
$J^{**}_{\mathrm {tpd}}$
always exist.) For positive integers
![]() $n\geq 2$
,
$n\geq 2$
,
![]() $m \leq n$
, and
$m \leq n$
, and
![]() $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
(cf. [Reference Mochizuki20, Def. 1.1(ii)]), write
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
(cf. [Reference Mochizuki20, Def. 1.1(ii)]), write
for the automorphism of
![]() $\Pi _{m}$
determined by
$\Pi _{m}$
determined by
![]() $\alpha $
;
$\alpha $
;
for the subgroup consisting of
![]() $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that
![]() $\beta _{2}(I) = I$
;
$\beta _{2}(I) = I$
;
for the subgroup consisting of
![]() $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that the image of
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that the image of
![]() $\beta $
via the composite
$\beta $
via the composite
![]() $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}) \twoheadrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{n}) \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1}) \rightarrow \mathrm {Out}(\Pi _{\mathcal {G}})$
—where the second arrow is the natural injection of [Reference Hoshi and Mochizuki8, Th. B] and the third arrow is the homomorphism induced by the natural outer isomorphism
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}) \twoheadrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{n}) \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1}) \rightarrow \mathrm {Out}(\Pi _{\mathcal {G}})$
—where the second arrow is the natural injection of [Reference Hoshi and Mochizuki8, Th. B] and the third arrow is the homomorphism induced by the natural outer isomorphism
![]() $\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
—is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]);
$\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
—is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]);
for the set of n-cuspidalizable cycle-subgroups of
![]() $\Pi _{1}$
(cf. Definition 3.5(i) and (ii));
$\Pi _{1}$
(cf. Definition 3.5(i) and (ii));
for the set of closed subgroups
![]() $T \subseteq \Pi _{2/1}$
such that T is a tripodal subgroup associated with some
$T \subseteq \Pi _{2/1}$
such that T is a tripodal subgroup associated with some
![]() $2$
-cuspidalizable cycle-subgroup of
$2$
-cuspidalizable cycle-subgroup of
![]() $\Pi _{1}$
(cf. Definition 3.6(i)), and, moreover, I is a distinguished cuspidal subgroup (cf. Definition 3.6(ii)) of T. Then the following hold:
$\Pi _{1}$
(cf. Definition 3.6(i)), and, moreover, I is a distinguished cuspidal subgroup (cf. Definition 3.6(ii)) of T. Then the following hold:
-
(i) Let
 $n\geq 3$
be a positive integer. Then
$n\geq 3$
be a positive integer. Then
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
acts naturally on
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
acts naturally on
 $\mathrm {Cycle}^{n}(\Pi _{1})$
,
$\mathrm {Cycle}^{n}(\Pi _{1})$
,
 $\mathrm {Tpd}_I(\Pi _{2/1})$
; there exists a unique
$\mathrm {Tpd}_I(\Pi _{2/1})$
; there exists a unique
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariant map such that, for every
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariant map such that, for every $$ \begin{align*}\mathfrak{C}_I \colon \mathrm{Cycle}^{n}(\Pi_{1}) \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1}) \end{align*} $$
$$ \begin{align*}\mathfrak{C}_I \colon \mathrm{Cycle}^{n}(\Pi_{1}) \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1}) \end{align*} $$
 $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
,
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
,
 $\mathfrak {C}_I(J)$
is a tripodal subgroup associated with J (cf. Definition 3.6(i)). Moreover, there exists an assignment —where
$\mathfrak {C}_I(J)$
is a tripodal subgroup associated with J (cf. Definition 3.6(i)). Moreover, there exists an assignment —where $$\begin{align*}\mathrm{Cycle}^{n}(\Pi_{1}) \ni J \, \, \mapsto \, \, \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
$$\begin{align*}\mathrm{Cycle}^{n}(\Pi_{1}) \ni J \, \, \mapsto \, \, \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
denotes an I-conjugacy class of isomorphisms
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
denotes an I-conjugacy class of isomorphisms
 $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
— such that:
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
— such that:
-
(a)
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps
 $I_{\mathrm {tpd}}$
bijectively onto I,
$I_{\mathrm {tpd}}$
bijectively onto I, -
(b)
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps the subgroups
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps the subgroups
 $J^{*}_{\mathrm {tpd}}$
,
$J^{*}_{\mathrm {tpd}}$
,
 $J^{**}_{\mathrm {tpd}}$
bijectively onto lifting cycle-subgroups of
$J^{**}_{\mathrm {tpd}}$
bijectively onto lifting cycle-subgroups of
 $\mathfrak {C}_I(J)$
(cf. Definition 3.6(ii)), and
$\mathfrak {C}_I(J)$
(cf. Definition 3.6(ii)), and -
(c) for
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, the diagram (of
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, the diagram (of
 $I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) —where the upper horizontal arrow is the (uniquely determined—cf. the commensurable terminality of
$I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) —where the upper horizontal arrow is the (uniquely determined—cf. the commensurable terminality of
 $I_{\mathrm {tpd}}$
in
$I_{\mathrm {tpd}}$
in
 $\Pi _{\mathrm {tpd}}$
discussed in [Reference Mochizuki18, Prop. 1.2(ii)])
$\Pi _{\mathrm {tpd}}$
discussed in [Reference Mochizuki18, Prop. 1.2(ii)])
 $I_{\mathrm {tpd}}$
-conjugacy class of automorphisms of
$I_{\mathrm {tpd}}$
-conjugacy class of automorphisms of
 $\Pi _{\mathrm {tpd}}$
that lifts
$\Pi _{\mathrm {tpd}}$
that lifts
 $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]) and preserves
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]) and preserves
 $I_{\mathrm {tpd}}$
; the lower horizontal arrow is the I-conjugacy class of isomorphisms induced by
$I_{\mathrm {tpd}}$
; the lower horizontal arrow is the I-conjugacy class of isomorphisms induced by
 $\alpha _2$
(cf. the “
$\alpha _2$
(cf. the “
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariance” mentioned above)—commutes up to possible composition with the cycle symmetry of
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariance” mentioned above)—commutes up to possible composition with the cycle symmetry of
 $\mathfrak {C}_I(\alpha _1(J))$
associated with I (cf. Definition 3.8).
$\mathfrak {C}_I(\alpha _1(J))$
associated with I (cf. Definition 3.8).
Finally, the assignment
is uniquely determined, up to possible composition with cycle symmetries, by these conditions (a)–(c). $$\begin{align*}J\mapsto \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
$$\begin{align*}J\mapsto \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
-
-
(ii) Let
 $n\geq 4$
be a positive integer,
$n\geq 4$
be a positive integer,
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, and
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, and
 $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
. Then there exists an automorphism
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
. Then there exists an automorphism
 $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
such that the FC-admissible outer automorphism of
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
such that the FC-admissible outer automorphism of
 $\Pi _{3}$
determined by
$\Pi _{3}$
determined by
 $\beta _3$
lies in the kernel of the tripod homomorphism
$\beta _3$
lies in the kernel of the tripod homomorphism
 $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}$
of [Reference Hoshi and Mochizuki10, Def. 3.19], and, moreover,
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}$
of [Reference Hoshi and Mochizuki10, Def. 3.19], and, moreover,
 $\alpha _1(J) = \beta _1(J)$
. Finally, the diagram (of
$\alpha _1(J) = \beta _1(J)$
. Finally, the diagram (of
 $I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) —where the lower horizontal arrow is the isomorphism induced by
$I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) —where the lower horizontal arrow is the isomorphism induced by
 $\beta _2$
(cf. the “
$\beta _2$
(cf. the “
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariance” mentioned in (i))—commutes up to possible composition with the cycle symmetry of
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariance” mentioned in (i))—commutes up to possible composition with the cycle symmetry of
 $\mathfrak {C}_I(\alpha _1(J))= \mathfrak {C}_I(\beta _1(J))$
associated with I.
$\mathfrak {C}_I(\alpha _1(J))= \mathfrak {C}_I(\beta _1(J))$
associated with I.
0 Notations and conventions
Sets: Let S be a finite set. Then we shall write
![]() $S^\sharp $
for the cardinality of S.
$S^\sharp $
for the cardinality of S.
Let S be a set equipped with an action by a group G. Then we shall write
![]() $S^G \subseteq S$
for the subset consisting of elements of S fixed by the action of G on S.
$S^G \subseteq S$
for the subset consisting of elements of S fixed by the action of G on S.
Numbers: Write
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
for the set of all prime numbers. Let
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
for the set of all prime numbers. Let
![]() $\Sigma $
be a set of prime numbers. Then we shall refer to a nonzero integer n as a
$\Sigma $
be a set of prime numbers. Then we shall refer to a nonzero integer n as a
![]() $\Sigma $
-integer if every prime divisor of n is contained in
$\Sigma $
-integer if every prime divisor of n is contained in
![]() $\Sigma $
. The notation
$\Sigma $
. The notation
![]() $\mathbb {R}$
will be used to denote the set, additive group, or field of real numbers, each of which we regard as being equipped with its usual topology. The notation
$\mathbb {R}$
will be used to denote the set, additive group, or field of real numbers, each of which we regard as being equipped with its usual topology. The notation
![]() $\mathbb {C}$
will be used to denote the set, additive group, or field of complex numbers, each of which we regard as being equipped with its usual topology.
$\mathbb {C}$
will be used to denote the set, additive group, or field of complex numbers, each of which we regard as being equipped with its usual topology.
Groups: Let
![]() $\Sigma $
be a set of prime numbers, and let
$\Sigma $
be a set of prime numbers, and let
![]() $f\colon G\rightarrow H$
be a homomorphism (resp. outer homomorphism) of groups. Then we shall say that f is
$f\colon G\rightarrow H$
be a homomorphism (resp. outer homomorphism) of groups. Then we shall say that f is
![]() $\Sigma $
-compatible if the homomorphism (resp. outer homomorphism)
$\Sigma $
-compatible if the homomorphism (resp. outer homomorphism)
![]() $f^{\Sigma }\colon G^{\Sigma } \rightarrow H^{\Sigma }$
between pro-
$f^{\Sigma }\colon G^{\Sigma } \rightarrow H^{\Sigma }$
between pro-
![]() $\Sigma $
completions induced by f is injective. Note that one verifies easily that if G is a group, and
$\Sigma $
completions induced by f is injective. Note that one verifies easily that if G is a group, and
![]() $H\subseteq G$
is a subgroup of G of finite index, then the natural inclusion
$H\subseteq G$
is a subgroup of G of finite index, then the natural inclusion
![]() $H\hookrightarrow G$
is
$H\hookrightarrow G$
is
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible. If G is a topological group, then we shall write
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible. If G is a topological group, then we shall write
for the abelianization of G, that is, the quotient of G by the closed normal subgroup of G generated by the commutators of G. If G is a profinite group, then we shall write
for the maximal pro-
![]() $\Sigma $
abelian torsion-free quotient of G. We shall use the terms normally terminal and commensurably terminal as they are defined in the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]. If I,
$\Sigma $
abelian torsion-free quotient of G. We shall use the terms normally terminal and commensurably terminal as they are defined in the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]. If I,
![]() $J \subseteq G$
are closed subgroups of a topological group G, then we shall write
$J \subseteq G$
are closed subgroups of a topological group G, then we shall write
if some open subgroup of I is contained in J.
1 The combinatorial section conjecture
In the present section, we study outer representations of ENN-type (cf. Definition 1.7(i) below) on the fundamental group of a semi-graph of anabelioids of PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). Roughly speaking, such outer representations may be thought of as an abstract combinatorial version of the natural outer representation of the maximal tamely ramified quotient of the absolute Galois group of a complete local field on the logarithmic fundamental group of the geometric special fiber of a stable model of a pointed stable curve over the complete local field. By comparison to the outer representation of NN-type studied in [Reference Hoshi and Mochizuki8], outer representations of ENN-type correspond to the situation in which the residue field of the complete local field under consideration is not necessarily separably closed. Such outer representations of ENN-type give rise to a surjection of profinite groups, which corresponds, in the case of pointed stable curves over complete local fields, to the surjection from the arithmetic fundamental group to (some quotient of) the absolute Galois group of the base field. Our first main result (cf. Theorem 1.13(i) below) asserts that, under the additional assumption that the associated cyclotomic character has open image, any section of this surjection necessarily admits a fixed point (i.e., a fixed vertex or edge). This “combinatorial section conjecture” is obtained as an immediate consequence of an essentially classical result concerning fixed points of group actions on graphs (cf. Lemma 1.6 below). By applying this existence of fixed points, we show that there is a natural bijection between conjugacy classes of profinite sections and conjugacy classes of tempered sections (cf. Theorem 1.13(iii) below) and derive a rather strong version of the combinatorial Grothendieck conjecture (cf. [Reference Hoshi and Mochizuki8, Th. A] and [Reference Hoshi and Mochizuki10, Th. 1.9]) for cyclotomically full outer representations of ENN-type (cf. Corollary 1.14 below). We also observe in passing that a generalization of the main result of [Reference Pop and Stix26] may be obtained as a consequence of the theory discussed in the present section (cf. Corollary 1.15 below).
In the present section, let
![]() $\Sigma $
be a nonempty set of prime numbers and let
$\Sigma $
be a nonempty set of prime numbers and let
![]() $\mathcal {G}$
be a semi-graph of anabelioids of pro-
$\mathcal {G}$
be a semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). Write
$\Sigma $
PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). Write
![]() $\mathbb {G}$
for the underlying semi-graph of
$\mathbb {G}$
for the underlying semi-graph of
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\Pi _{\mathcal {G}}$
for the (pro-
$\Pi _{\mathcal {G}}$
for the (pro-
![]() $\Sigma $
) fundamental group of
$\Sigma $
) fundamental group of
![]() $\mathcal {G}$
, and
$\mathcal {G}$
, and
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
for the tempered fundamental group of
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
for the tempered fundamental group of
![]() $\mathcal {G}$
(cf. [Reference Mochizuki17, Exam. 2.10] and the discussion preceding [Reference Mochizuki17, Prop. 3.6]). Thus, we have a natural outer injection
$\mathcal {G}$
(cf. [Reference Mochizuki17, Exam. 2.10] and the discussion preceding [Reference Mochizuki17, Prop. 3.6]). Thus, we have a natural outer injection
(cf. [Reference Hoshi and Mochizuki11, Lem. 3.2(i)] and the proof of [Reference Hoshi and Mochizuki11, Prop. 3.3(i) and (ii)]). Let us write
for the universal pro-
![]() $\Sigma $
and pro-tempered coverings of
$\Sigma $
and pro-tempered coverings of
![]() $\mathcal {G}$
corresponding to
$\mathcal {G}$
corresponding to
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
, and
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
, and
where
![]() $\mathcal {H}$
(resp.
$\mathcal {H}$
(resp.
![]() $\mathcal {H}^{\mathrm {tp}}$
) ranges over the subcoverings of
$\mathcal {H}^{\mathrm {tp}}$
) ranges over the subcoverings of
![]() $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
(resp.
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
(resp.
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow \mathcal {G}$
) corresponding to open subgroups of
$\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow \mathcal {G}$
) corresponding to open subgroups of
![]() $\Pi _{\mathcal {G}}$
(resp.
$\Pi _{\mathcal {G}}$
(resp.
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
), and
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
), and
![]() $\mathrm {VCN}(-)$
denotes the “
$\mathrm {VCN}(-)$
denotes the “
![]() $\mathrm {VCN}(-)$
” of the underlying semi-graph of the semi-graph of anabelioids in parentheses (cf. Definition 1.1(i) below and [Reference Hoshi and Mochizuki8, Def. 1.1(iii)]).
$\mathrm {VCN}(-)$
” of the underlying semi-graph of the semi-graph of anabelioids in parentheses (cf. Definition 1.1(i) below and [Reference Hoshi and Mochizuki8, Def. 1.1(iii)]).
We begin by reviewing certain well-known facts concerning semi-graphs and group actions on semi-graphs.
Definition 1.1. Let
![]() $\Gamma $
be a semi-graph (cf. the discussion at the beginning of [Reference Mochizuki17, §1]).
$\Gamma $
be a semi-graph (cf. the discussion at the beginning of [Reference Mochizuki17, §1]).
-
(i) We shall write
 $\mathrm {Vert}(\Gamma )$
(resp.
$\mathrm {Vert}(\Gamma )$
(resp.
 $\mathrm {Cusp}(\Gamma )$
;
$\mathrm {Cusp}(\Gamma )$
;
 $\mathrm {Node}(\Gamma )$
) for the set of vertices (resp. open edges, i.e., “cusps”; closed edges, i.e., “nodes”) of
$\mathrm {Node}(\Gamma )$
) for the set of vertices (resp. open edges, i.e., “cusps”; closed edges, i.e., “nodes”) of
 $\Gamma $
. We shall write
$\Gamma $
. We shall write
 $\mathrm {Edge}(\Gamma ) \stackrel {\mathrm {def}}{=} \mathrm {Cusp}(\Gamma ) \sqcup \mathrm {Node}(\Gamma )$
;
$\mathrm {Edge}(\Gamma ) \stackrel {\mathrm {def}}{=} \mathrm {Cusp}(\Gamma ) \sqcup \mathrm {Node}(\Gamma )$
;
 $\mathrm {VCN}(\Gamma ) \stackrel {\mathrm {def}}{=} \mathrm {Vert}(\Gamma ) \sqcup \mathrm {Edge}(\Gamma )$
.
$\mathrm {VCN}(\Gamma ) \stackrel {\mathrm {def}}{=} \mathrm {Vert}(\Gamma ) \sqcup \mathrm {Edge}(\Gamma )$
. -
(ii) We shall write
 $$\begin{align*}\mathcal{V}_\Gamma \colon \mathrm{Edge}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Vert}(\Gamma)} \end{align*}$$
$$\begin{align*}\mathcal{V}_\Gamma \colon \mathrm{Edge}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Vert}(\Gamma)} \end{align*}$$
 $$\begin{align*}( \mathrm{respectively,} \, \, \mathcal{C}_\Gamma \colon \mathrm{Vert}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Cusp}(\Gamma)}; \end{align*}$$
$$\begin{align*}( \mathrm{respectively,} \, \, \mathcal{C}_\Gamma \colon \mathrm{Vert}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Cusp}(\Gamma)}; \end{align*}$$
 $$\begin{align*}\mathcal{N}_\Gamma \colon \mathrm{Vert}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Node}(\Gamma)}; \end{align*}$$
(cf. (i); the discussion entitled “Sets” in [Reference Hoshi and Mochizuki9, §0]) for the map obtained by sending
$$\begin{align*}\mathcal{N}_\Gamma \colon \mathrm{Vert}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Node}(\Gamma)}; \end{align*}$$
(cf. (i); the discussion entitled “Sets” in [Reference Hoshi and Mochizuki9, §0]) for the map obtained by sending $$\begin{align*}\mathcal{E}_\Gamma \colon \mathrm{Vert}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Edge}(\Gamma)}) \end{align*}$$
$$\begin{align*}\mathcal{E}_\Gamma \colon \mathrm{Vert}(\Gamma)\ \longrightarrow\ 2^{\mathrm{Edge}(\Gamma)}) \end{align*}$$
 $e\in \mathrm {Edge}(\Gamma )$
(resp.
$e\in \mathrm {Edge}(\Gamma )$
(resp.
 $v\in \mathrm {Vert}(\Gamma )$
;
$v\in \mathrm {Vert}(\Gamma )$
;
 $v\in \mathrm {Vert}(\Gamma )$
;
$v\in \mathrm {Vert}(\Gamma )$
;
 $v\in \mathrm {Vert}(\Gamma )$
) to the set of vertices (resp. open edges; closed edges; edges) of
$v\in \mathrm {Vert}(\Gamma )$
) to the set of vertices (resp. open edges; closed edges; edges) of
 $\Gamma $
to which e abuts (resp. which abut to v; which abut to v; which abut to v). For simplicity, we shall write
$\Gamma $
to which e abuts (resp. which abut to v; which abut to v; which abut to v). For simplicity, we shall write
 $\mathcal {V}$
(resp
$\mathcal {V}$
(resp
 $\mathcal {C}$
;
$\mathcal {C}$
;
 $\mathcal {N}$
;
$\mathcal {N}$
;
 $\mathcal {E}$
) instead of
$\mathcal {E}$
) instead of
 $\mathcal {V}_\Gamma $
(resp
$\mathcal {V}_\Gamma $
(resp
 $\mathcal {C}_\Gamma $
;
$\mathcal {C}_\Gamma $
;
 $\mathcal {N}_\Gamma $
;
$\mathcal {N}_\Gamma $
;
 $\mathcal {E}_\Gamma $
) when there is no danger of confusion.
$\mathcal {E}_\Gamma $
) when there is no danger of confusion.
-
(iii) Let n be a nonnegative integer; v,
 $w \in \mathrm {Vert}(\Gamma )$
(cf. (i)). Then we shall write
$w \in \mathrm {Vert}(\Gamma )$
(cf. (i)). Then we shall write
 $\delta (v, w) \leq n$
if the following conditions are satisfied:
$\delta (v, w) \leq n$
if the following conditions are satisfied:-
• If
 $n = 0$
, then
$n = 0$
, then
 $v = w$
.
$v = w$
. -
• If
 $n \geq 1$
, then either
$n \geq 1$
, then either
 $\delta (v, w) \leq n-1$
or there exist n closed edges
$\delta (v, w) \leq n-1$
or there exist n closed edges
 $e_1 , \dots , e_{n} \in \mathrm {Node}(\Gamma )$
of
$e_1 , \dots , e_{n} \in \mathrm {Node}(\Gamma )$
of
 $\Gamma $
(cf. (i)) and
$\Gamma $
(cf. (i)) and
 $n+1$
vertices
$n+1$
vertices
 $v_0 , \dots , v_n \in \mathrm {Vert}(\Gamma )$
of
$v_0 , \dots , v_n \in \mathrm {Vert}(\Gamma )$
of
 $\Gamma $
such that
$\Gamma $
such that
 $v_0=v$
,
$v_0=v$
,
 $v_n=w$
, and, for
$v_n=w$
, and, for
 $1 \leq i \leq n$
, it holds that
$1 \leq i \leq n$
, it holds that
 $\mathcal {V}(e_i) = \{v_{i-1}, v_{i}\}$
(cf. (ii)).
$\mathcal {V}(e_i) = \{v_{i-1}, v_{i}\}$
(cf. (ii)).
Moreover, we shall write
 $\delta (v,w)=n$
if
$\delta (v,w)=n$
if
 $\delta (v,w)\leq n$
, but
$\delta (v,w)\leq n$
, but
 $\delta (v,w)\not \leq n-1$
. If
$\delta (v,w)\not \leq n-1$
. If
 $\delta (v,w)=n$
, then we shall say that the distance between v and w is equal to n.
$\delta (v,w)=n$
, then we shall say that the distance between v and w is equal to n. -
Definition 1.2. Let
![]() $\Gamma $
be a semi-graph.
$\Gamma $
be a semi-graph.
-
(i) Let G be a group that acts on
 $\Gamma $
. Then (by a slight abuse of notation, relative to the notation defined in the discussion entitled “Sets” in §0) we shall write for the semi-graph (i.e., the “G-invariant portion of
$\Gamma $
. Then (by a slight abuse of notation, relative to the notation defined in the discussion entitled “Sets” in §0) we shall write for the semi-graph (i.e., the “G-invariant portion of $$ \begin{align*}\Gamma^G\end{align*} $$
$$ \begin{align*}\Gamma^G\end{align*} $$
 $\Gamma $
”) defined as follows:
$\Gamma $
”) defined as follows:
-
• We take
 $\mathrm {Vert}(\Gamma ^G)$
to be
$\mathrm {Vert}(\Gamma ^G)$
to be
 $\mathrm {Vert}(\Gamma )^G$
(cf. Definition 1.1(i); the discussion entitled “Sets” in §0).
$\mathrm {Vert}(\Gamma )^G$
(cf. Definition 1.1(i); the discussion entitled “Sets” in §0). -
• We take
 $\mathrm {Edge}(\Gamma ^G)$
to be
$\mathrm {Edge}(\Gamma ^G)$
to be
 $\mathrm {Edge}(\Gamma )^G$
(cf. Definition 1.1(i); the discussion entitled “Sets” in §0).
$\mathrm {Edge}(\Gamma )^G$
(cf. Definition 1.1(i); the discussion entitled “Sets” in §0). -
• Let
 $e \in \mathrm {Edge}(\Gamma ^G) = \mathrm {Edge}(\Gamma )^G$
. Then the coincidence map of
$e \in \mathrm {Edge}(\Gamma ^G) = \mathrm {Edge}(\Gamma )^G$
. Then the coincidence map of $$ \begin{align*}\zeta_e\colon e\ \longrightarrow\ \mathrm{Vert}(\Gamma^G)\ \cup\ \{\mathrm{Vert}(\Gamma^G)\}\end{align*} $$
$$ \begin{align*}\zeta_e\colon e\ \longrightarrow\ \mathrm{Vert}(\Gamma^G)\ \cup\ \{\mathrm{Vert}(\Gamma^G)\}\end{align*} $$
 $\Gamma ^G$
(cf. item (3) of the discussion at the beginning of [Reference Mochizuki17, §1]) is defined as follows: write
$\Gamma ^G$
(cf. item (3) of the discussion at the beginning of [Reference Mochizuki17, §1]) is defined as follows: write
 $\zeta ^\Gamma _e\colon e \rightarrow \mathrm {Vert}(\Gamma ) \cup \{\mathrm {Vert}(\Gamma )\}$
for the coincidence map associated with
$\zeta ^\Gamma _e\colon e \rightarrow \mathrm {Vert}(\Gamma ) \cup \{\mathrm {Vert}(\Gamma )\}$
for the coincidence map associated with
 $\Gamma $
. Then, for
$\Gamma $
. Then, for
 $b \in e$
, if
$b \in e$
, if
 $b \in e^G$
and
$b \in e^G$
and
 $\zeta _e^\Gamma (b) \in \mathrm {Vert}(\Gamma )^G$
(resp. if either
$\zeta _e^\Gamma (b) \in \mathrm {Vert}(\Gamma )^G$
(resp. if either
 $b \not \in e^G$
or
$b \not \in e^G$
or
 $\zeta _e^\Gamma (b) \not \in \mathrm {Vert}(\Gamma )^G$
), then we set
$\zeta _e^\Gamma (b) \not \in \mathrm {Vert}(\Gamma )^G$
), then we set
 $\zeta _e(b) \stackrel {\mathrm {def}}{=} \zeta _e^\Gamma (b)$
(resp.
$\zeta _e(b) \stackrel {\mathrm {def}}{=} \zeta _e^\Gamma (b)$
(resp.
 $\stackrel {\mathrm {def}}{=} \mathrm {Vert}(\Gamma ^G)$
). In particular, it holds that
$\stackrel {\mathrm {def}}{=} \mathrm {Vert}(\Gamma ^G)$
). In particular, it holds that
 $\mathcal {V}_{\Gamma ^G}(e) = \mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma )^G$
(cf. Definition 1.1(ii)) whenever it holds either that
$\mathcal {V}_{\Gamma ^G}(e) = \mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma )^G$
(cf. Definition 1.1(ii)) whenever it holds either that
 $\Gamma $
is untangled (i.e., every node abuts to two distinct vertices—cf. the discussion entitled “Semi-graphs” in [Reference Hoshi and Mochizuki8, §0]) or that G acts on
$\Gamma $
is untangled (i.e., every node abuts to two distinct vertices—cf. the discussion entitled “Semi-graphs” in [Reference Hoshi and Mochizuki8, §0]) or that G acts on
 $\Gamma $
without inversion (i.e., that if
$\Gamma $
without inversion (i.e., that if
 $e \in \mathrm {Edge}(\Gamma )^G$
, then
$e \in \mathrm {Edge}(\Gamma )^G$
, then
 $e = e^G$
).
$e = e^G$
).
-
-
(ii) We shall write
for the semi-graph (i.e., the result of “subdividing” $$ \begin{align*}\Gamma^{\div}\end{align*} $$
$$ \begin{align*}\Gamma^{\div}\end{align*} $$
 $\Gamma $
) defined as follows:
$\Gamma $
) defined as follows:
-
• We take
 $\mathrm {Vert}(\Gamma ^{\div })$
to be
$\mathrm {Vert}(\Gamma ^{\div })$
to be
 $\mathrm {Vert}(\Gamma ) \sqcup \mathrm {Edge}(\Gamma )$
.
$\mathrm {Vert}(\Gamma ) \sqcup \mathrm {Edge}(\Gamma )$
. -
• We take
 $\mathrm {Edge}(\Gamma ^{\div })$
to be the set of branches of
$\mathrm {Edge}(\Gamma ^{\div })$
to be the set of branches of
 $\Gamma $
.
$\Gamma $
. -
• Let b be a branch of an edge e of
 $\Gamma $
. Write
$\Gamma $
. Write
 $e(b) \in \mathrm {Edge}(\Gamma ^{\div })$
,
$e(b) \in \mathrm {Edge}(\Gamma ^{\div })$
,
 $v(e) \in \mathrm {Vert}(\Gamma ^{\div })$
for the edge and vertex of
$v(e) \in \mathrm {Vert}(\Gamma ^{\div })$
for the edge and vertex of
 $\Gamma ^{\div }$
corresponding to b, e, respectively. If b abuts, relative to
$\Gamma ^{\div }$
corresponding to b, e, respectively. If b abuts, relative to
 $\Gamma $
, to a vertex
$\Gamma $
, to a vertex
 $v \in \mathrm {Vert}(\Gamma )$
, then we take the edge
$v \in \mathrm {Vert}(\Gamma )$
, then we take the edge
 $e(b)$
to be a node that abuts to
$e(b)$
to be a node that abuts to
 $v(e)$
and the vertex of
$v(e)$
and the vertex of
 $\Gamma ^{\div }$
corresponding to
$\Gamma ^{\div }$
corresponding to
 $v \in \mathrm {Vert}(\Gamma )$
. If b does not abut, relative to
$v \in \mathrm {Vert}(\Gamma )$
. If b does not abut, relative to
 $\Gamma $
, to a vertex of
$\Gamma $
, to a vertex of
 $\Gamma $
, then we take the edge
$\Gamma $
, then we take the edge
 $e(b)$
to be a cusp that abuts to
$e(b)$
to be a cusp that abuts to
 $v(e)$
.
$v(e)$
.
-
Definition 1.3. Let
![]() $\Gamma $
be a semi-graph, and let
$\Gamma $
be a semi-graph, and let
![]() $\Gamma _{0} \subseteq \Gamma $
be a sub-semi-graph (cf. [Reference Mochizuki17, the discussion following the figure entitled “A Typical Semi-graph”]) of
$\Gamma _{0} \subseteq \Gamma $
be a sub-semi-graph (cf. [Reference Mochizuki17, the discussion following the figure entitled “A Typical Semi-graph”]) of
![]() $\Gamma $
.
$\Gamma $
.
-
(i) We shall write
for the sub-semi-graph of $$ \begin{align*}\Gamma_{0}^{\multimap} \subseteq \Gamma\end{align*} $$
$$ \begin{align*}\Gamma_{0}^{\multimap} \subseteq \Gamma\end{align*} $$
 $\Gamma $
(i.e., whenever a suitable condition is satisfied [cf. Lemma 1.4(v) below], a sort of “open neighborhood” of
$\Gamma $
(i.e., whenever a suitable condition is satisfied [cf. Lemma 1.4(v) below], a sort of “open neighborhood” of
 $\Gamma _{0}$
) whose sets of vertices and edges are defined as follows. (Here, we recall that it follows immediately from the definition of a sub-semi-graph that a sub-semi-graph is completely determined by its sets of vertices and edges.)
$\Gamma _{0}$
) whose sets of vertices and edges are defined as follows. (Here, we recall that it follows immediately from the definition of a sub-semi-graph that a sub-semi-graph is completely determined by its sets of vertices and edges.)
-
• We take
 $\mathrm {Vert}(\Gamma _{0}^{\multimap })$
to be
$\mathrm {Vert}(\Gamma _{0}^{\multimap })$
to be
 $\mathrm {Vert}(\Gamma _{0})$
.
$\mathrm {Vert}(\Gamma _{0})$
. -
• We take
 $\mathrm {Edge}(\Gamma _{0}^{\multimap })$
to be the set of edges e of
$\mathrm {Edge}(\Gamma _{0}^{\multimap })$
to be the set of edges e of
 $\Gamma $
such that
$\Gamma $
such that
 $\mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma _{0}) \neq \emptyset $
.
$\mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma _{0}) \neq \emptyset $
.
-
-
(ii) We shall write
for the sub-semi-graph of $$ \begin{align*}\Gamma_{0}^{\not\in} \subseteq \Gamma\end{align*} $$
$$ \begin{align*}\Gamma_{0}^{\not\in} \subseteq \Gamma\end{align*} $$
 $\Gamma $
whose sets of vertices and edges are taken to be
$\Gamma $
whose sets of vertices and edges are taken to be
 $\mathrm {Vert}(\Gamma ) \setminus \mathrm {Vert}(\Gamma _{0})$
,
$\mathrm {Vert}(\Gamma ) \setminus \mathrm {Vert}(\Gamma _{0})$
,
 $\mathrm {Edge}(\Gamma ) \setminus \mathrm {Edge}(\Gamma _{0})$
, respectively.
$\mathrm {Edge}(\Gamma ) \setminus \mathrm {Edge}(\Gamma _{0})$
, respectively.
-
(iii) We shall write
 $\Gamma _{0}^{\not \in \multimap } \stackrel {\mathrm {def}}{=} (\Gamma _{0}^{\not \in })^{\multimap }$
(cf. (i) and (ii)).
$\Gamma _{0}^{\not \in \multimap } \stackrel {\mathrm {def}}{=} (\Gamma _{0}^{\not \in })^{\multimap }$
(cf. (i) and (ii)). -
(iv) We shall say that an edge e of
 $\Gamma $
is a
$\Gamma $
is a
 $\Gamma _{0}$
-bridge if
$\Gamma _{0}$
-bridge if
 $\mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma _{0})$
,
$\mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma _{0})$
,
 $\mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma _{0}^{\not \in }) \neq \emptyset $
. (Thus, one verifies easily that every
$\mathcal {V}_\Gamma (e) \cap \mathrm {Vert}(\Gamma _{0}^{\not \in }) \neq \emptyset $
. (Thus, one verifies easily that every
 $\Gamma _{0}$
-bridge is a node.) We shall write
$\Gamma _{0}$
-bridge is a node.) We shall write
 $\mathrm {Brdg}(\Gamma _{0}\subseteq \Gamma ) \subseteq \mathrm {Node}(\Gamma )$
for the set of
$\mathrm {Brdg}(\Gamma _{0}\subseteq \Gamma ) \subseteq \mathrm {Node}(\Gamma )$
for the set of
 $\Gamma _{0}$
-bridges of
$\Gamma _{0}$
-bridges of
 $\Gamma $
. By abuse of notation, we shall write
$\Gamma $
. By abuse of notation, we shall write
 $\mathrm {Brdg}(\Gamma _{0}\subseteq \Gamma ) \subseteq \Gamma $
for the sub-semi-graph of
$\mathrm {Brdg}(\Gamma _{0}\subseteq \Gamma ) \subseteq \Gamma $
for the sub-semi-graph of
 $\Gamma $
whose sets of vertices and edges are taken to be
$\Gamma $
whose sets of vertices and edges are taken to be
 $\emptyset $
(i.e., the empty set),
$\emptyset $
(i.e., the empty set),
 $\mathrm {Brdg}(\Gamma _{0}\subseteq \Gamma ) \subseteq \mathrm {Node}(\Gamma )$
, respectively.
$\mathrm {Brdg}(\Gamma _{0}\subseteq \Gamma ) \subseteq \mathrm {Node}(\Gamma )$
, respectively.
Lemma 1.4 (Basic properties of sub-semi-graphs).
Let
![]() $\Gamma $
be a semi-graph,
$\Gamma $
be a semi-graph,
![]() $\Gamma _{0} \subseteq \Gamma $
a sub-semi-graph (cf. [Reference Mochizuki17, the discussion following the figure entitled “A Typical Semi-graph”]) of
$\Gamma _{0} \subseteq \Gamma $
a sub-semi-graph (cf. [Reference Mochizuki17, the discussion following the figure entitled “A Typical Semi-graph”]) of
![]() $\Gamma $
, G a group, and
$\Gamma $
, G a group, and
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\Gamma )$
an action of G on
$\rho \colon G\rightarrow \mathrm {Aut}(\Gamma )$
an action of G on
![]() $\Gamma $
. Then the following hold:
$\Gamma $
. Then the following hold:
-
(i) Suppose either that
 $\Gamma $
is untangled or that G acts on
$\Gamma $
is untangled or that G acts on
 $\Gamma $
without inversion. Then the semi-graph
$\Gamma $
without inversion. Then the semi-graph
 $\Gamma ^G$
(cf. Definition 1.2(i)) may be regarded, in a natural way, as a sub-semi-graph of
$\Gamma ^G$
(cf. Definition 1.2(i)) may be regarded, in a natural way, as a sub-semi-graph of
 $\Gamma $
.
$\Gamma $
. -
(ii) Suppose that G acts on
 $\Gamma $
without inversion, and that every edge of
$\Gamma $
without inversion, and that every edge of
 $\Gamma $
abuts to at least one vertex of
$\Gamma $
abuts to at least one vertex of
 $\Gamma $
. Then every edge of
$\Gamma $
. Then every edge of
 $\Gamma ^G$
abuts to at least one vertex of
$\Gamma ^G$
abuts to at least one vertex of
 $\Gamma ^G$
.
$\Gamma ^G$
. -
(iii) The semi-graph
 $\Gamma ^{\div }$
(cf. Definition 1.2(ii)) is untangled.
$\Gamma ^{\div }$
(cf. Definition 1.2(ii)) is untangled. -
(iv) There exists a natural injection
 $ \mathrm {Aut}(\Gamma ) \hookrightarrow \mathrm {Aut}(\Gamma ^{\div })$
. Moreover, the resulting action
$ \mathrm {Aut}(\Gamma ) \hookrightarrow \mathrm {Aut}(\Gamma ^{\div })$
. Moreover, the resulting action
 $\rho ^{\div }$
of G on
$\rho ^{\div }$
of G on
 $\Gamma ^{\div }$
(i.e., the composite
$\Gamma ^{\div }$
(i.e., the composite
 $G\stackrel {\rho }{\rightarrow }\mathrm {Aut}(\Gamma ) \hookrightarrow \mathrm {Aut}(\Gamma ^{\div })$
) is an action without inversion. Finally, it holds that
$G\stackrel {\rho }{\rightarrow }\mathrm {Aut}(\Gamma ) \hookrightarrow \mathrm {Aut}(\Gamma ^{\div })$
) is an action without inversion. Finally, it holds that
 $\Gamma ^G =\emptyset $
if and only if
$\Gamma ^G =\emptyset $
if and only if
 $(\Gamma ^{\div })^G = \emptyset $
.
$(\Gamma ^{\div })^G = \emptyset $
. -
(v) Suppose that every edge of
 $\Gamma _{0}$
abuts to at least one vertex of
$\Gamma _{0}$
abuts to at least one vertex of
 $\Gamma _{0}$
. Then
$\Gamma _{0}$
. Then
 $\Gamma _{0}$
may be regarded, in a natural way, as a sub-semi-graph of
$\Gamma _{0}$
may be regarded, in a natural way, as a sub-semi-graph of
 $\Gamma _{0}^{\multimap }$
(cf. Definition 1.3(i)).
$\Gamma _{0}^{\multimap }$
(cf. Definition 1.3(i)). -
(vi) We have an equality of subsets of
 $\mathrm {Edge}(\Gamma )$
:
$\mathrm {Edge}(\Gamma )$
:  $$ \begin{align*}\mathrm{Edge}(\Gamma_{0}^{\multimap})\ \cap\ \mathrm{Edge}(\Gamma_{0}^{\not\in\multimap})\ =\ \mathrm{Brdg}(\Gamma_{0}\subseteq \Gamma) .\end{align*} $$
$$ \begin{align*}\mathrm{Edge}(\Gamma_{0}^{\multimap})\ \cap\ \mathrm{Edge}(\Gamma_{0}^{\not\in\multimap})\ =\ \mathrm{Brdg}(\Gamma_{0}\subseteq \Gamma) .\end{align*} $$
Proof. The assertions of Lemma 1.4 follow immediately from the various definitions involved.
Lemma 1.5 (Sub-semi-graphs of invariants).
In the situation of Lemma 1.4, suppose either that
![]() $\Gamma $
is untangled or that G acts on
$\Gamma $
is untangled or that G acts on
![]() $\Gamma $
without inversion. Suppose, moreover, that the sub-semi-graph
$\Gamma $
without inversion. Suppose, moreover, that the sub-semi-graph
![]() $\Gamma _{0} \subseteq \Gamma $
is a connected component of the sub-semi-graph
$\Gamma _{0} \subseteq \Gamma $
is a connected component of the sub-semi-graph
![]() $\Gamma ^G \subseteq \Gamma $
(cf. Lemma 1.4(i)). Then the following hold:
$\Gamma ^G \subseteq \Gamma $
(cf. Lemma 1.4(i)). Then the following hold:
-
(i) The action
 $\rho $
naturally determines actions of G on
$\rho $
naturally determines actions of G on
 $\Gamma _{0}$
,
$\Gamma _{0}$
,
 $\Gamma _{0}^{\multimap }$
,
$\Gamma _{0}^{\multimap }$
,
 $\Gamma _{0}^{\not \in \multimap }$
, respectively.
$\Gamma _{0}^{\not \in \multimap }$
, respectively. -
(ii) The intersection of
 $\Gamma _{0}^{\multimap } \subseteq \Gamma $
with any connected component of
$\Gamma _{0}^{\multimap } \subseteq \Gamma $
with any connected component of
 $\Gamma ^G \subseteq \Gamma $
that is
$\Gamma ^G \subseteq \Gamma $
that is
 $\neq \Gamma _{0}$
is empty.
$\neq \Gamma _{0}$
is empty. -
(iii) We have an equality of subsets of
 $\mathrm {Edge}(\Gamma )$
:
$\mathrm {Edge}(\Gamma )$
:  $$ \begin{align*}\mathrm{Edge}(\Gamma^G)\ \cap\ \mathrm{Brdg}(\Gamma_{0}^{\multimap} \subseteq \Gamma)\ =\ \emptyset .\end{align*} $$
$$ \begin{align*}\mathrm{Edge}(\Gamma^G)\ \cap\ \mathrm{Brdg}(\Gamma_{0}^{\multimap} \subseteq \Gamma)\ =\ \emptyset .\end{align*} $$
Proof. The assertions of Lemma 1.5 follow immediately from the various definitions involved.
Lemma 1.6 (Existence of fixed points).
Let
![]() $\Gamma $
be a finite connected (hence nonempty) semi-graph, let G be a finite solvable group whose order is a
$\Gamma $
be a finite connected (hence nonempty) semi-graph, let G be a finite solvable group whose order is a
![]() $\Sigma $
-integer (cf. the discussion entitled “Numbers” in §0), and let
$\Sigma $
-integer (cf. the discussion entitled “Numbers” in §0), and let
be an action of G on
![]() $\Gamma $
. Write
$\Gamma $
. Write
![]() $\Pi _{\Gamma }^{\mathrm {disc}}$
for the (discrete) topological fundamental group of
$\Pi _{\Gamma }^{\mathrm {disc}}$
for the (discrete) topological fundamental group of
![]() $\Gamma $
;
$\Gamma $
;
![]() $\Pi _{\Gamma }^{\Sigma }$
for the pro-
$\Pi _{\Gamma }^{\Sigma }$
for the pro-
![]() $\Sigma $
completion of
$\Sigma $
completion of
![]() $\Pi _{\Gamma }^{\mathrm {disc}}$
;
$\Pi _{\Gamma }^{\mathrm {disc}}$
;
![]() $\widetilde {\Gamma }^{\mathrm {disc}} \rightarrow \Gamma $
,
$\widetilde {\Gamma }^{\mathrm {disc}} \rightarrow \Gamma $
,
![]() $\widetilde {\Gamma }^{\Sigma } \rightarrow \Gamma $
for the discrete, pro-
$\widetilde {\Gamma }^{\Sigma } \rightarrow \Gamma $
for the discrete, pro-
![]() $\Sigma $
universal coverings of
$\Sigma $
universal coverings of
![]() $\Gamma $
corresponding to
$\Gamma $
corresponding to
![]() $\Pi _{\Gamma }^{\mathrm {disc}}$
,
$\Pi _{\Gamma }^{\mathrm {disc}}$
,
![]() $\Pi _{\Gamma }^{\Sigma }$
, respectively. Let
$\Pi _{\Gamma }^{\Sigma }$
, respectively. Let
![]() $\Box \in \{\mathrm {disc}, \Sigma \}$
. Write
$\Box \in \{\mathrm {disc}, \Sigma \}$
. Write
![]() $\mathrm {Aut}(\widetilde {\Gamma }^{\Box } \rightarrow \Gamma )\subseteq \mathrm {Aut}(\widetilde {\Gamma }^{\Box })$
for the group of automorphisms
$\mathrm {Aut}(\widetilde {\Gamma }^{\Box } \rightarrow \Gamma )\subseteq \mathrm {Aut}(\widetilde {\Gamma }^{\Box })$
for the group of automorphisms
![]() $\widetilde {\alpha }$
of
$\widetilde {\alpha }$
of
![]() $\widetilde {\Gamma }^{\Box }$
such that
$\widetilde {\Gamma }^{\Box }$
such that
![]() $\widetilde {\alpha }$
lies over a(n) (necessarily unique) automorphism
$\widetilde {\alpha }$
lies over a(n) (necessarily unique) automorphism
![]() $\alpha $
of
$\alpha $
of
![]() $\Gamma $
;
$\Gamma $
;
 $$\begin{align*}\begin{array}{cccc} & \mathrm{Aut}(\widetilde{\Gamma}^{\Box} \rightarrow \Gamma) & \longrightarrow & \mathrm{Aut}(\Gamma) \\ & \widetilde{\alpha} & \mapsto & \alpha \end{array}\end{align*}$$
$$\begin{align*}\begin{array}{cccc} & \mathrm{Aut}(\widetilde{\Gamma}^{\Box} \rightarrow \Gamma) & \longrightarrow & \mathrm{Aut}(\Gamma) \\ & \widetilde{\alpha} & \mapsto & \alpha \end{array}\end{align*}$$
for the resulting natural homomorphism;
for the fiber product of the natural homomorphism
![]() $\mathrm {Aut}(\widetilde {\Gamma }^{\Box } \rightarrow \Gamma )\rightarrow \mathrm {Aut}(\Gamma )$
and the action
$\mathrm {Aut}(\widetilde {\Gamma }^{\Box } \rightarrow \Gamma )\rightarrow \mathrm {Aut}(\Gamma )$
and the action
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\Gamma )$
. Thus, one verifies easily that
$\rho \colon G\rightarrow \mathrm {Aut}(\Gamma )$
. Thus, one verifies easily that
![]() $\Pi _{\Gamma // G}^{\Box }$
fits into an exact sequence
$\Pi _{\Gamma // G}^{\Box }$
fits into an exact sequence
Let
![]() $s\colon G \rightarrow \Pi _{\Gamma // G}^{\Box }$
be a section of the above exact sequence. Write
$s\colon G \rightarrow \Pi _{\Gamma // G}^{\Box }$
be a section of the above exact sequence. Write
![]() $\widetilde {\rho }_s^{\Box }\colon G \rightarrow \mathrm {Aut}(\widetilde {\Gamma }^{\Box })$
for the action obtained by forming the composite
$\widetilde {\rho }_s^{\Box }\colon G \rightarrow \mathrm {Aut}(\widetilde {\Gamma }^{\Box })$
for the action obtained by forming the composite
![]() $G \stackrel {s}{\rightarrow }\Pi _{\Gamma // G}^{\Box } \stackrel {\mathrm {pr}_{1}}{\rightarrow } \mathrm {Aut}(\widetilde {\Gamma }^{\Box } \rightarrow \Gamma ) \hookrightarrow \mathrm {Aut}(\widetilde {\Gamma }^{\Box })$
. We shall say that a connected finite subcovering
$G \stackrel {s}{\rightarrow }\Pi _{\Gamma // G}^{\Box } \stackrel {\mathrm {pr}_{1}}{\rightarrow } \mathrm {Aut}(\widetilde {\Gamma }^{\Box } \rightarrow \Gamma ) \hookrightarrow \mathrm {Aut}(\widetilde {\Gamma }^{\Box })$
. We shall say that a connected finite subcovering
![]() $\Gamma _* \rightarrow \Gamma $
of
$\Gamma _* \rightarrow \Gamma $
of
![]() $\widetilde {\Gamma }^{\Sigma }\rightarrow \Gamma $
is
$\widetilde {\Gamma }^{\Sigma }\rightarrow \Gamma $
is
![]() $G$
-compatible if
$G$
-compatible if
![]() $\Gamma _* \rightarrow \Gamma $
is Galois, and, moreover, the corresponding normal open subgroup of
$\Gamma _* \rightarrow \Gamma $
is Galois, and, moreover, the corresponding normal open subgroup of
![]() $\Pi _{\Gamma }^{\Sigma }$
is preserved by the outer action of G, via
$\Pi _{\Gamma }^{\Sigma }$
is preserved by the outer action of G, via
![]() $\rho $
, on
$\rho $
, on
![]() $\Pi _{\Gamma }^{\Sigma }$
. If
$\Pi _{\Gamma }^{\Sigma }$
. If
![]() $\Gamma _* \rightarrow \Gamma $
is a G-compatible connected finite subcovering of
$\Gamma _* \rightarrow \Gamma $
is a G-compatible connected finite subcovering of
![]() $\widetilde {\Gamma }^{\Sigma }\rightarrow \Gamma $
, then let us write
$\widetilde {\Gamma }^{\Sigma }\rightarrow \Gamma $
, then let us write
![]() $\rho _{s,*}\colon G \rightarrow \mathrm {Aut}(\Gamma _*)$
for the action of G on
$\rho _{s,*}\colon G \rightarrow \mathrm {Aut}(\Gamma _*)$
for the action of G on
![]() $\Gamma _*$
determined by
$\Gamma _*$
determined by
![]() $\widetilde \rho _s^{\Box }$
;
$\widetilde \rho _s^{\Box }$
;
![]() $\Gamma _*^G$
for the semi-graph defined in Definition 1.2(i), with respect to the action
$\Gamma _*^G$
for the semi-graph defined in Definition 1.2(i), with respect to the action
![]() $\rho _{s,*}$
. (Thus, if
$\rho _{s,*}$
. (Thus, if
![]() $\Gamma $
, hence also
$\Gamma $
, hence also
![]() $\Gamma _*$
, is untangled, then
$\Gamma _*$
, is untangled, then
![]() $\Gamma _*^G$
is a sub-semi-graph of
$\Gamma _*^G$
is a sub-semi-graph of
![]() $\Gamma _*$
—cf. Lemma 1.4(i).) Then the following hold:
$\Gamma _*$
—cf. Lemma 1.4(i).) Then the following hold:
-
(i) Suppose that
 $\Gamma $
is untangled. Then, for each G-compatible connected finite subcovering
$\Gamma $
is untangled. Then, for each G-compatible connected finite subcovering
 $\Gamma _* \rightarrow \Gamma $
of
$\Gamma _* \rightarrow \Gamma $
of
 $\widetilde \Gamma ^{\Sigma }\rightarrow \Gamma $
, the sub-semi-graph
$\widetilde \Gamma ^{\Sigma }\rightarrow \Gamma $
, the sub-semi-graph
 $\Gamma _*^G \subseteq \Gamma _*$
coincides with the disjoint union of some (possibly empty) collection of connected components of
$\Gamma _*^G \subseteq \Gamma _*$
coincides with the disjoint union of some (possibly empty) collection of connected components of
 $\Gamma _*|_{\Gamma ^G}\stackrel {\mathrm {def}}{=} \Gamma _*\times _{\Gamma }\Gamma ^G \subseteq \Gamma _*$
.
$\Gamma _*|_{\Gamma ^G}\stackrel {\mathrm {def}}{=} \Gamma _*\times _{\Gamma }\Gamma ^G \subseteq \Gamma _*$
. -
(ii) Suppose that
 $\Gamma $
is untangled, and that G is isomorphic to
$\Gamma $
is untangled, and that G is isomorphic to
 $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
 $l\in \Sigma $
. Then, for every G-compatible connected finite subcovering
$l\in \Sigma $
. Then, for every G-compatible connected finite subcovering
 $\Gamma _* \rightarrow \Gamma $
of
$\Gamma _* \rightarrow \Gamma $
of
 $\widetilde \Gamma ^{\Sigma }\rightarrow \Gamma $
, the sub-semi-graph
$\widetilde \Gamma ^{\Sigma }\rightarrow \Gamma $
, the sub-semi-graph
 $\Gamma _*^G \subseteq \Gamma _*$
is nonempty.
$\Gamma _*^G \subseteq \Gamma _*$
is nonempty. -
(iii) Suppose that
 $\Box = \mathrm {disc}$
. Write
$\Box = \mathrm {disc}$
. Write
 $(\widetilde \Gamma ^{\mathrm {disc}})^G$
for the sub-semi-graph (cf. Lemma 1.4(i)) of (the necessarily untangled semi-graph!)
$(\widetilde \Gamma ^{\mathrm {disc}})^G$
for the sub-semi-graph (cf. Lemma 1.4(i)) of (the necessarily untangled semi-graph!)
 $\widetilde \Gamma ^{\mathrm {disc}}$
defined in Definition 1.2(i), with respect to the action
$\widetilde \Gamma ^{\mathrm {disc}}$
defined in Definition 1.2(i), with respect to the action
 $\widetilde \rho _s^{\mathrm {disc}}$
. Then
$\widetilde \rho _s^{\mathrm {disc}}$
. Then
 $(\widetilde \Gamma ^{\mathrm {disc}})^G$
is nonempty and connected. If, moreover, we write
$(\widetilde \Gamma ^{\mathrm {disc}})^G$
is nonempty and connected. If, moreover, we write
 $(\Gamma ^G)_{0} \subseteq \Gamma ^G$
for the image of the composite
$(\Gamma ^G)_{0} \subseteq \Gamma ^G$
for the image of the composite
 $(\widetilde \Gamma ^{\mathrm {disc}})^G \hookrightarrow \widetilde \Gamma ^{\mathrm {disc}} \rightarrow \Gamma $
, then the resulting morphism
$(\widetilde \Gamma ^{\mathrm {disc}})^G \hookrightarrow \widetilde \Gamma ^{\mathrm {disc}} \rightarrow \Gamma $
, then the resulting morphism
 $(\widetilde \Gamma ^{\mathrm {disc}})^G\rightarrow (\Gamma ^G)_{0}$
is a (discrete) universal covering of
$(\widetilde \Gamma ^{\mathrm {disc}})^G\rightarrow (\Gamma ^G)_{0}$
is a (discrete) universal covering of
 $(\Gamma ^G)_{0}$
.
$(\Gamma ^G)_{0}$
. -
(iv) Suppose that
 $\Box =\mathrm {disc}$
(resp.
$\Box =\mathrm {disc}$
(resp.
 $\Box =\Sigma $
). Then the set where, in the resp’d case, the projective limit is taken over the G-compatible connected finite subcoverings
$\Box =\Sigma $
). Then the set where, in the resp’d case, the projective limit is taken over the G-compatible connected finite subcoverings $$\begin{align*}\mathrm{VCN}(\widetilde\Gamma^{\mathrm{disc}})^G \quad (\mathrm{resp}. \, \, \mathrm{VCN}(\widetilde\Gamma^{\Sigma})^G \, \, \stackrel{\mathrm{def}}{=} \, \, \varprojlim \, \, \mathrm{VCN}(\Gamma_*)^G), \end{align*}$$
$$\begin{align*}\mathrm{VCN}(\widetilde\Gamma^{\mathrm{disc}})^G \quad (\mathrm{resp}. \, \, \mathrm{VCN}(\widetilde\Gamma^{\Sigma})^G \, \, \stackrel{\mathrm{def}}{=} \, \, \varprojlim \, \, \mathrm{VCN}(\Gamma_*)^G), \end{align*}$$
 $\Gamma _*\rightarrow \Gamma $
of
$\Gamma _*\rightarrow \Gamma $
of
 $\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
, is nonempty.
$\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
, is nonempty.
-
(v) Suppose that
 $\Box =\Sigma $
, that
$\Box =\Sigma $
, that
 $\Gamma $
is untangled, and that G is isomorphic to
$\Gamma $
is untangled, and that G is isomorphic to
 $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
 $l\in \Sigma $
. Let
$l\in \Sigma $
. Let
 $(\Gamma ^G)_0\subseteq \Gamma ^G$
be a (nonempty) connected component of
$(\Gamma ^G)_0\subseteq \Gamma ^G$
be a (nonempty) connected component of
 $\Gamma ^G$
such that
$\Gamma ^G$
such that  $$\begin{align*}\mathrm{VCN}((\Gamma^G)_0)\ \cap\ \mathrm{Im}\bigl(\mathrm{VCN}(\widetilde\Gamma^{\Sigma})^G \rightarrow \mathrm{VCN}(\Gamma)\bigr)\ \neq\ \emptyset \end{align*}$$
$$\begin{align*}\mathrm{VCN}((\Gamma^G)_0)\ \cap\ \mathrm{Im}\bigl(\mathrm{VCN}(\widetilde\Gamma^{\Sigma})^G \rightarrow \mathrm{VCN}(\Gamma)\bigr)\ \neq\ \emptyset \end{align*}$$
(cf. (iv)). Then there exists a G-compatible connected finite subcovering
 $\Gamma _* \rightarrow \Gamma $
of
$\Gamma _* \rightarrow \Gamma $
of
 $\widetilde \Gamma ^{\Sigma }\rightarrow \Gamma $
such that the image of
$\widetilde \Gamma ^{\Sigma }\rightarrow \Gamma $
such that the image of
 $\Gamma _*^G\subseteq \Gamma _*$
in
$\Gamma _*^G\subseteq \Gamma _*$
in
 $\Gamma $
coincides with
$\Gamma $
coincides with
 $(\Gamma ^G)_0\subseteq \Gamma ^G$
.
$(\Gamma ^G)_0\subseteq \Gamma ^G$
. -
(vi) Suppose that
 $\Box =\Sigma $
, and that
$\Box =\Sigma $
, and that
 $\Gamma $
is untangled. Then the sub-pro-semi-graph
$\Gamma $
is untangled. Then the sub-pro-semi-graph
 $(\widetilde \Gamma ^{\Sigma })^G$
of
$(\widetilde \Gamma ^{\Sigma })^G$
of
 $\widetilde \Gamma ^{\Sigma }$
determined by the projective system of sub-semi-graphs
$\widetilde \Gamma ^{\Sigma }$
determined by the projective system of sub-semi-graphs
 $\Gamma _*^G$
—where
$\Gamma _*^G$
—where
 $\Gamma _*\rightarrow \Gamma $
ranges over the G-compatible connected finite subcoverings of
$\Gamma _*\rightarrow \Gamma $
ranges over the G-compatible connected finite subcoverings of
 $\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
—is nonempty and connected. If, moreover, we write
$\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
—is nonempty and connected. If, moreover, we write
 $(\Gamma ^G)_{0} \subseteq \Gamma ^G$
for the image of the composite
$(\Gamma ^G)_{0} \subseteq \Gamma ^G$
for the image of the composite
 $(\widetilde \Gamma ^{\Sigma })^G \hookrightarrow \widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
, then the resulting morphism
$(\widetilde \Gamma ^{\Sigma })^G \hookrightarrow \widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
, then the resulting morphism
 $(\widetilde \Gamma ^{\Sigma })^G\rightarrow (\Gamma ^G)_{0}$
is a pro-
$(\widetilde \Gamma ^{\Sigma })^G\rightarrow (\Gamma ^G)_{0}$
is a pro-
 $\Sigma $
universal covering of
$\Sigma $
universal covering of
 $(\Gamma ^G)_{0}$
.
$(\Gamma ^G)_{0}$
.
Proof. First, we verify assertion (i). Let us first observe that one verifies immediately that there is an inclusion of sub-semi-graphs
![]() $\Gamma _*^G \subseteq \Gamma _*|_{\Gamma ^G}$
(cf. Lemma 1.4(i)). Next, let us observe that it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
$\Gamma _*^G \subseteq \Gamma _*|_{\Gamma ^G}$
(cf. Lemma 1.4(i)). Next, let us observe that it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
![]() $\Gamma $
by
$\Gamma $
by
![]() $\Gamma ^{\div }$
, we may assume without loss of generality that G acts without inversion on
$\Gamma ^{\div }$
, we may assume without loss of generality that G acts without inversion on
![]() $\Gamma $
(which implies that G acts trivially on
$\Gamma $
(which implies that G acts trivially on
![]() $\Gamma ^G$
—cf. Definition 1.2(i)). Thus, to complete the verification of assertion (i), it suffices to verify that the following assertion holds:
$\Gamma ^G$
—cf. Definition 1.2(i)). Thus, to complete the verification of assertion (i), it suffices to verify that the following assertion holds:
Claim 1.6.A: Let
![]() $(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*|_{\Gamma ^G}$
be a connected component of
$(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*|_{\Gamma ^G}$
be a connected component of
![]() $\Gamma _*|_{\Gamma ^G}$
such that
$\Gamma _*|_{\Gamma ^G}$
such that
![]() $(\Gamma _*|_{\Gamma ^G})_0 \cap \Gamma _*^G \neq \emptyset $
. Then
$(\Gamma _*|_{\Gamma ^G})_0 \cap \Gamma _*^G \neq \emptyset $
. Then
![]() $(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*^G$
.
$(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*^G$
.
To verify Claim 1.6.A, let us observe that since
![]() $(\Gamma _*|_{\Gamma ^G})_0 \cap \Gamma _*^G \neq \emptyset $
, the action
$(\Gamma _*|_{\Gamma ^G})_0 \cap \Gamma _*^G \neq \emptyset $
, the action
![]() $\rho _{s,*}$
of G on
$\rho _{s,*}$
of G on
![]() $\Gamma _*$
stabilizes
$\Gamma _*$
stabilizes
![]() $(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*$
. In particular, we obtain an action of G on
$(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*$
. In particular, we obtain an action of G on
![]() $(\Gamma _*|_{\Gamma ^G})_0$
over
$(\Gamma _*|_{\Gamma ^G})_0$
over
![]() $\Gamma ^G$
. Thus, since the action of G on
$\Gamma ^G$
. Thus, since the action of G on
![]() $\Gamma ^G$
is trivial, and the composite
$\Gamma ^G$
is trivial, and the composite
![]() $(\Gamma _*|_{\Gamma ^G})_0 \hookrightarrow \Gamma _*|_{\Gamma ^G} \rightarrow \Gamma ^G$
is a connected finite covering of some connected component of
$(\Gamma _*|_{\Gamma ^G})_0 \hookrightarrow \Gamma _*|_{\Gamma ^G} \rightarrow \Gamma ^G$
is a connected finite covering of some connected component of
![]() $\Gamma ^G$
, again by our assumption that
$\Gamma ^G$
, again by our assumption that
![]() $(\Gamma _*|_{\Gamma ^G})_0 \cap \Gamma _*^G \neq \emptyset $
, we conclude that the action of G on
$(\Gamma _*|_{\Gamma ^G})_0 \cap \Gamma _*^G \neq \emptyset $
, we conclude that the action of G on
![]() $(\Gamma _*|_{\Gamma ^G})_0$
is trivial, that is, that there is an inclusion of sub-semi-graphs
$(\Gamma _*|_{\Gamma ^G})_0$
is trivial, that is, that there is an inclusion of sub-semi-graphs
![]() $(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*^G$
. This completes the proof of Claim 1.6.A, hence also of assertion (i).
$(\Gamma _*|_{\Gamma ^G})_0 \subseteq \Gamma _*^G$
. This completes the proof of Claim 1.6.A, hence also of assertion (i).
Next, we verify assertion (ii). One verifies immediately that we may assume without loss of generality that
![]() $\Gamma _* = \Gamma $
. Now suppose that
$\Gamma _* = \Gamma $
. Now suppose that
![]() $\Gamma ^G = \emptyset $
. Then since
$\Gamma ^G = \emptyset $
. Then since
![]() $G \cong \mathbb {Z}/l\mathbb {Z}$
, it follows that the action of G on
$G \cong \mathbb {Z}/l\mathbb {Z}$
, it follows that the action of G on
![]() $\Gamma $
is free, which thus implies that the quotient map
$\Gamma $
is free, which thus implies that the quotient map
![]() $\Gamma \twoheadrightarrow \Gamma / G$
is a covering of
$\Gamma \twoheadrightarrow \Gamma / G$
is a covering of
![]() $\Gamma /G$
. In particular,
$\Gamma /G$
. In particular,
![]() $\Pi _{\Gamma // G}^{\Sigma }$
is isomorphic to the pro-
$\Pi _{\Gamma // G}^{\Sigma }$
is isomorphic to the pro-
![]() $\Sigma $
completion of the topological fundamental group of the semi-graph
$\Sigma $
completion of the topological fundamental group of the semi-graph
![]() $\Gamma /G$
. Thus, the pro-
$\Gamma /G$
. Thus, the pro-
![]() $\Sigma $
group
$\Sigma $
group
![]() $\Pi _{\Gamma // G}^{\Sigma }$
is free, hence, in particular, torsion-free. But this contradicts the existence of the section of the surjection
$\Pi _{\Gamma // G}^{\Sigma }$
is free, hence, in particular, torsion-free. But this contradicts the existence of the section of the surjection
![]() $\Pi _{\Gamma // G}^{\Sigma } \twoheadrightarrow G$
determined by s. This completes the proof of assertion (ii).
$\Pi _{\Gamma // G}^{\Sigma } \twoheadrightarrow G$
determined by s. This completes the proof of assertion (ii).
Next, we verify the resp’d portion of assertion (iv) (i.e., the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
) in the case where G is isomorphic to
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
) in the case where G is isomorphic to
![]() $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
![]() $l \in \Sigma $
. Let us first observe that it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
$l \in \Sigma $
. Let us first observe that it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
![]() $\Gamma $
by
$\Gamma $
by
![]() $\Gamma ^{\div }$
, we may assume without loss of generality that
$\Gamma ^{\div }$
, we may assume without loss of generality that
![]() $\Gamma $
is untangled. Thus, the assertion that
$\Gamma $
is untangled. Thus, the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
follows immediately from assertion (ii), together with the well-known elementary fact that a projective limit of nonempty finite sets is nonempty. This completes the proof of the assertion that
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
follows immediately from assertion (ii), together with the well-known elementary fact that a projective limit of nonempty finite sets is nonempty. This completes the proof of the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
in the case where G is isomorphic to
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
in the case where G is isomorphic to
![]() $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
![]() $l \in \Sigma $
.
$l \in \Sigma $
.
Next, we verify assertion (iii). Let us first observe that since
![]() $\widetilde \Gamma ^{\mathrm {disc}}$
is a tree, hence untangled, it follows from Lemma 1.4(i) that
$\widetilde \Gamma ^{\mathrm {disc}}$
is a tree, hence untangled, it follows from Lemma 1.4(i) that
![]() $(\widetilde \Gamma ^{\mathrm {disc}})^G$
is a sub-semi-graph of
$(\widetilde \Gamma ^{\mathrm {disc}})^G$
is a sub-semi-graph of
![]() $\widetilde \Gamma ^{\mathrm {disc}}$
. Next, let us observe that it follows immediately from Lemma 1.4(iv) that, by replacing
$\widetilde \Gamma ^{\mathrm {disc}}$
. Next, let us observe that it follows immediately from Lemma 1.4(iv) that, by replacing
![]() $\Gamma $
by
$\Gamma $
by
![]() $\Gamma ^{\div }$
, we may assume without loss of generality that G acts without inversion on
$\Gamma ^{\div }$
, we may assume without loss of generality that G acts without inversion on
![]() $\Gamma $
. Thus, the assertion that
$\Gamma $
. Thus, the assertion that
![]() $(\widetilde \Gamma ^{\mathrm {disc}})^G$
is nonempty and connected follows immediately from [Reference Mochizuki17, Lem. 1.8(ii)]. The remainder of assertion (iii) follows from a similar argument to the argument applied in the proof of assertion (i). This completes the proof of assertion (iii). In particular, the unresp’d portion of assertion (iv) (i.e., the assertion that
$(\widetilde \Gamma ^{\mathrm {disc}})^G$
is nonempty and connected follows immediately from [Reference Mochizuki17, Lem. 1.8(ii)]. The remainder of assertion (iii) follows from a similar argument to the argument applied in the proof of assertion (i). This completes the proof of assertion (iii). In particular, the unresp’d portion of assertion (iv) (i.e., the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\mathrm {disc}})^G \neq \emptyset $
) holds.
$\mathrm {VCN}(\widetilde \Gamma ^{\mathrm {disc}})^G \neq \emptyset $
) holds.
Next, we verify assertion (v). Let us first observe that, to verify assertion (v), it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
![]() $\Gamma $
by
$\Gamma $
by
![]() $\Gamma ^{\div }$
, we may assume without loss of generality that the action
$\Gamma ^{\div }$
, we may assume without loss of generality that the action
![]() $\rho $
is an action without inversion, and that every edge of
$\rho $
is an action without inversion, and that every edge of
![]() $\Gamma $
abuts to at least one vertex of
$\Gamma $
abuts to at least one vertex of
![]() $\Gamma $
. In particular, since (we have assumed that)
$\Gamma $
. In particular, since (we have assumed that)
![]() $(\Gamma ^G)_0 \neq \emptyset $
, it follows from Lemma 1.4(ii) and (v) that
$(\Gamma ^G)_0 \neq \emptyset $
, it follows from Lemma 1.4(ii) and (v) that
![]() $(\Gamma ^G)_0^{\multimap } \neq \emptyset $
(cf. Definition 1.3(i)). Now if
$(\Gamma ^G)_0^{\multimap } \neq \emptyset $
(cf. Definition 1.3(i)). Now if
![]() $\Gamma ^G$
is connected, then one verifies immediately that the trivial covering
$\Gamma ^G$
is connected, then one verifies immediately that the trivial covering
![]() $\Gamma \stackrel {\mathrm {id}}{\rightarrow } \Gamma $
satisfies the condition imposed on “
$\Gamma \stackrel {\mathrm {id}}{\rightarrow } \Gamma $
satisfies the condition imposed on “
![]() $\Gamma _* \rightarrow \Gamma $
” in the statement of assertion (v). Thus, to complete the verification of assertion (v), we may assume without loss of generality that
$\Gamma _* \rightarrow \Gamma $
” in the statement of assertion (v). Thus, to complete the verification of assertion (v), we may assume without loss of generality that
![]() $\Gamma ^G$
is not connected, hence (cf. Lemma 1.4(ii)) contains at least one vertex
$\Gamma ^G$
is not connected, hence (cf. Lemma 1.4(ii)) contains at least one vertex
![]() $\not \in \mathrm {Vert}( (\Gamma ^G)_0)$
. In particular,
$\not \in \mathrm {Vert}( (\Gamma ^G)_0)$
. In particular,
![]() $(\Gamma ^G)_0^{\not \in \multimap } \neq \emptyset $
(cf. Definition 1.3(iii)).
$(\Gamma ^G)_0^{\not \in \multimap } \neq \emptyset $
(cf. Definition 1.3(iii)).
Write
![]() $((\Gamma ^G)_0^{\multimap })^{\coprod } \rightarrow (\Gamma ^G)_0^{\multimap }$
for the trivial
$((\Gamma ^G)_0^{\multimap })^{\coprod } \rightarrow (\Gamma ^G)_0^{\multimap }$
for the trivial
![]() $\mathbb {Z}/l\mathbb {Z}$
-covering obtained by taking a disjoint union of copies of
$\mathbb {Z}/l\mathbb {Z}$
-covering obtained by taking a disjoint union of copies of
![]() $(\Gamma ^G)_0^{\multimap }$
indexed by the elements of
$(\Gamma ^G)_0^{\multimap }$
indexed by the elements of
![]() $\mathbb {Z}/l\mathbb {Z}$
;
$\mathbb {Z}/l\mathbb {Z}$
;
![]() $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } \rightarrow (\Gamma ^G)_0^{\not \in \multimap }$
for the trivial
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } \rightarrow (\Gamma ^G)_0^{\not \in \multimap }$
for the trivial
![]() $\mathbb {Z}/l\mathbb {Z}$
-covering obtained by taking a disjoint union of copies of
$\mathbb {Z}/l\mathbb {Z}$
-covering obtained by taking a disjoint union of copies of
![]() $(\Gamma ^G)_0^{\not \in \multimap }$
indexed by the elements of
$(\Gamma ^G)_0^{\not \in \multimap }$
indexed by the elements of
![]() $\mathbb {Z}/l\mathbb {Z}$
. Then the natural actions of G on
$\mathbb {Z}/l\mathbb {Z}$
. Then the natural actions of G on
![]() $((\Gamma ^G)_0^{\multimap })^{\coprod }$
,
$((\Gamma ^G)_0^{\multimap })^{\coprod }$
,
![]() $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
(cf. Lemma 1.5(i)) determine natural actions of
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
(cf. Lemma 1.5(i)) determine natural actions of
![]() $G\times \mathbb {Z}/l\mathbb {Z}$
on
$G\times \mathbb {Z}/l\mathbb {Z}$
on
![]() $((\Gamma ^G)_0^{\multimap })^{\coprod }$
,
$((\Gamma ^G)_0^{\multimap })^{\coprod }$
,
![]() $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
, that is, we have homomorphisms
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
, that is, we have homomorphisms
Let
![]() $\phi \colon G\stackrel {\sim }{\rightarrow } \mathbb {Z}/l\mathbb {Z}$
be an isomorphism. Write
$\phi \colon G\stackrel {\sim }{\rightarrow } \mathbb {Z}/l\mathbb {Z}$
be an isomorphism. Write
 $$\begin{align*}\begin{array}{cccccc} \rho^{\not\in\multimap}_\phi \colon & G\times\mathbb{Z}/l\mathbb{Z} & \longrightarrow & G\times\mathbb{Z}/l\mathbb{Z} & \stackrel{\rho^{\not\in\multimap}}{\longrightarrow} & \mathrm{Aut}\bigl( ((\Gamma^G)_0^{\not\in\multimap})^{\coprod} \bigr) \\ & (a,b) & \mapsto & (a, \phi(a) + b) \end{array} \end{align*}$$
$$\begin{align*}\begin{array}{cccccc} \rho^{\not\in\multimap}_\phi \colon & G\times\mathbb{Z}/l\mathbb{Z} & \longrightarrow & G\times\mathbb{Z}/l\mathbb{Z} & \stackrel{\rho^{\not\in\multimap}}{\longrightarrow} & \mathrm{Aut}\bigl( ((\Gamma^G)_0^{\not\in\multimap})^{\coprod} \bigr) \\ & (a,b) & \mapsto & (a, \phi(a) + b) \end{array} \end{align*}$$
for the composite of
![]() $\rho ^{\not \in \multimap }$
with the homomorphism described in the second line of the display.
$\rho ^{\not \in \multimap }$
with the homomorphism described in the second line of the display.
Next, for
![]() $e \in \mathrm {Brdg} \stackrel {\mathrm {def}}{=} \mathrm {Brdg}((\Gamma ^G)_0 \subseteq \Gamma )$
(cf. Definition 1.3(iv)), write
$e \in \mathrm {Brdg} \stackrel {\mathrm {def}}{=} \mathrm {Brdg}((\Gamma ^G)_0 \subseteq \Gamma )$
(cf. Definition 1.3(iv)), write
![]() $G\cdot e \subseteq \mathrm {Edge}((\Gamma ^G)_0^{\multimap })$
for the G-orbit of e. Then it is immediate that
$G\cdot e \subseteq \mathrm {Edge}((\Gamma ^G)_0^{\multimap })$
for the G-orbit of e. Then it is immediate that
![]() $G\cdot e \subseteq \mathrm {Brdg}$
; moreover, since
$G\cdot e \subseteq \mathrm {Brdg}$
; moreover, since
![]() $G \cong \mathbb {Z}/l\mathbb {Z}$
, it follows immediately from Lemma 1.5(iii) that
$G \cong \mathbb {Z}/l\mathbb {Z}$
, it follows immediately from Lemma 1.5(iii) that
![]() $G\cdot e$
is a G-torsor. Next, let us write
$G\cdot e$
is a G-torsor. Next, let us write
(cf. Lemma 1.4(vi)). Then one verifies easily from the various definitions involved that the following hold:
-
(a) The actions
 $\rho ^{\multimap }$
,
$\rho ^{\multimap }$
,
 $\rho ^{\not \in \multimap }_\phi $
of
$\rho ^{\not \in \multimap }_\phi $
of
 $G\times \mathbb {Z}/l\mathbb {Z}$
on
$G\times \mathbb {Z}/l\mathbb {Z}$
on
 $((\Gamma ^G)_0^{\multimap })^{\coprod }$
,
$((\Gamma ^G)_0^{\multimap })^{\coprod }$
,
 $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
determine actions on these fibers
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
determine actions on these fibers
 $((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e}$
,
$((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e}$
,
 $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
.
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
. -
(b) These fibers
 $((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e}$
,
$((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e}$
,
 $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
are
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
are
 $(G\times \mathbb {Z}/l\mathbb {Z})$
-torsors with respect to the actions of (a).
$(G\times \mathbb {Z}/l\mathbb {Z})$
-torsors with respect to the actions of (a). -
(c) There is a natural isomorphism of semi-graphs
 $((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e}\stackrel {\sim }{\rightarrow } ((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
(cf. Lemma 1.4(vi)), which we shall use to identify these two semi-graphs.
$((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e}\stackrel {\sim }{\rightarrow } ((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
(cf. Lemma 1.4(vi)), which we shall use to identify these two semi-graphs. -
(d) Let
 $e_{\mathrm {base}} \in ((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e} = ((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
(cf. (c)) be a lifting of
$e_{\mathrm {base}} \in ((\Gamma ^G)_0^{\multimap })^{\coprod } |_{G\cdot e} = ((\Gamma ^G)_0^{\not \in \multimap })^{\coprod } |_{G\cdot e}$
(cf. (c)) be a lifting of
 $e \in \mathrm {Brdg}$
. Then there is a uniquely determined (cf. (b)) isomorphism of
$e \in \mathrm {Brdg}$
. Then there is a uniquely determined (cf. (b)) isomorphism of $$ \begin{align*}\iota_{e_{\mathrm{base}}}\colon ((\Gamma^G)_0^{\multimap})^{\coprod} |_{G\cdot e} \stackrel{\sim}{\longrightarrow} ((\Gamma^G)_0^{\not\in\multimap})^{\coprod} |_{G\cdot e}\end{align*} $$
$$ \begin{align*}\iota_{e_{\mathrm{base}}}\colon ((\Gamma^G)_0^{\multimap})^{\coprod} |_{G\cdot e} \stackrel{\sim}{\longrightarrow} ((\Gamma^G)_0^{\not\in\multimap})^{\coprod} |_{G\cdot e}\end{align*} $$
 $(G\times \mathbb {Z}/l\mathbb {Z})$
-torsors (cf. (b)) that maps
$(G\times \mathbb {Z}/l\mathbb {Z})$
-torsors (cf. (b)) that maps
 $e_{\mathrm {base}}$
to
$e_{\mathrm {base}}$
to
 $e_{\mathrm {base}}$
.
$e_{\mathrm {base}}$
.
Let
![]() $\mathbb {B}$
be a collection of elements “
$\mathbb {B}$
be a collection of elements “
![]() $e_{\mathrm {base}}$
” as in (d) such that the map
$e_{\mathrm {base}}$
” as in (d) such that the map
![]() $e_{\mathrm {base}} \mapsto e$
determines a bijection between
$e_{\mathrm {base}} \mapsto e$
determines a bijection between
![]() $\mathbb {B}$
and the set of G-orbits of
$\mathbb {B}$
and the set of G-orbits of
![]() $\mathrm {Brdg}$
. Thus, by gluing
$\mathrm {Brdg}$
. Thus, by gluing
![]() $((\Gamma ^G)_0^{\multimap })^{\coprod }$
to
$((\Gamma ^G)_0^{\multimap })^{\coprod }$
to
![]() $((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
by means of the collection of isomorphisms
$((\Gamma ^G)_0^{\not \in \multimap })^{\coprod }$
by means of the collection of isomorphisms
![]() $\{ \iota _{e_{\mathrm {base}}} \}_{e_{\mathrm {base}} \in \mathbb {B}}$
of (d) (cf. Lemma 1.4(vi)), we obtain a finite covering
$\{ \iota _{e_{\mathrm {base}}} \}_{e_{\mathrm {base}} \in \mathbb {B}}$
of (d) (cf. Lemma 1.4(vi)), we obtain a finite covering
![]() $\Gamma _* \rightarrow \Gamma $
, together with an action of
$\Gamma _* \rightarrow \Gamma $
, together with an action of
![]() $G\times \mathbb {Z}/l\mathbb {Z}$
on
$G\times \mathbb {Z}/l\mathbb {Z}$
on
![]() $\Gamma _*$
(i.e., obtained by gluing the actions
$\Gamma _*$
(i.e., obtained by gluing the actions
![]() $\rho ^{\multimap }$
,
$\rho ^{\multimap }$
,
![]() $\rho ^{\not \in \multimap }_\phi $
), such that the morphism
$\rho ^{\not \in \multimap }_\phi $
), such that the morphism
![]() $\Gamma _* \rightarrow \Gamma $
is equivariant with respect to this action of
$\Gamma _* \rightarrow \Gamma $
is equivariant with respect to this action of
![]() $G\times \mathbb {Z}/l\mathbb {Z}$
on
$G\times \mathbb {Z}/l\mathbb {Z}$
on
![]() $\Gamma _*$
and the action of
$\Gamma _*$
and the action of
![]() $G\times \mathbb {Z}/l\mathbb {Z}$
on
$G\times \mathbb {Z}/l\mathbb {Z}$
on
![]() $\Gamma $
obtained by composing the projection
$\Gamma $
obtained by composing the projection
![]() $G\times \mathbb {Z}/l\mathbb {Z}\rightarrow G$
with the given action of G on
$G\times \mathbb {Z}/l\mathbb {Z}\rightarrow G$
with the given action of G on
![]() $\Gamma $
. Next, let us observe that since
$\Gamma $
. Next, let us observe that since
![]() $\phi $
is an isomorphism, and both
$\phi $
is an isomorphism, and both
![]() $(\Gamma ^G)_0$
and
$(\Gamma ^G)_0$
and
![]() $(\Gamma ^G)_0^{\not \in \multimap }$
contain vertices fixed by G (cf. the discussion at the beginning of the present proof of assertion (v)), one verifies immediately—for example, by considering the orbit by the action of
$(\Gamma ^G)_0^{\not \in \multimap }$
contain vertices fixed by G (cf. the discussion at the beginning of the present proof of assertion (v)), one verifies immediately—for example, by considering the orbit by the action of
![]() $G\times \{1\} (\subseteq G\times \mathbb {Z}/l\mathbb {Z})$
of some lifting to
$G\times \{1\} (\subseteq G\times \mathbb {Z}/l\mathbb {Z})$
of some lifting to
![]() $\Gamma _*$
(which may be chosen to pass through an element of
$\Gamma _*$
(which may be chosen to pass through an element of
![]() $\mathbb {B}$
) of a path of minimal length between such vertices fixed by G—that
$\mathbb {B}$
) of a path of minimal length between such vertices fixed by G—that
![]() $\Gamma _*$
is connected. Moreover, it follows from the definition of
$\Gamma _*$
is connected. Moreover, it follows from the definition of
![]() $\Gamma _*$
that the covering
$\Gamma _*$
that the covering
![]() $\Gamma _* \rightarrow \Gamma $
is Galois, G-compatible, and equipped with a natural isomorphism
$\Gamma _* \rightarrow \Gamma $
is Galois, G-compatible, and equipped with a natural isomorphism
![]() $\mathrm {Gal}(\Gamma _* / \Gamma ) \stackrel {\sim }{\rightarrow } \mathbb {Z}/l\mathbb {Z}$
; in particular,
$\mathrm {Gal}(\Gamma _* / \Gamma ) \stackrel {\sim }{\rightarrow } \mathbb {Z}/l\mathbb {Z}$
; in particular,
![]() $\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
factors as a composite
$\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
factors as a composite
![]() $\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma _* \rightarrow \Gamma $
.
$\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma _* \rightarrow \Gamma $
.
Next, let us observe that, for each
![]() $g \in G$
, the automorphism
$g \in G$
, the automorphism
![]() $\alpha _g$
of
$\alpha _g$
of
![]() $\Gamma _*$
obtained by considering the difference between
$\Gamma _*$
obtained by considering the difference between
![]() $\rho _{s,*}(g)$
and the action of g (i.e.,
$\rho _{s,*}(g)$
and the action of g (i.e.,
![]() $(g,0)\in G\times \mathbb {Z}/l\mathbb {Z}$
) on
$(g,0)\in G\times \mathbb {Z}/l\mathbb {Z}$
) on
![]() $\Gamma _*$
defined above is an automorphism over
$\Gamma _*$
defined above is an automorphism over
![]() $\Gamma $
. Moreover, it follows immediately from our assumption that
$\Gamma $
. Moreover, it follows immediately from our assumption that
that
![]() $\alpha _g$
fixes an element of
$\alpha _g$
fixes an element of
![]() $\mathrm {VCN}(\Gamma _*)$
that maps to
$\mathrm {VCN}(\Gamma _*)$
that maps to
![]() $\mathrm {VCN}((\Gamma ^G)_0) \subseteq \mathrm {VCN}(\Gamma )$
. But this implies that
$\mathrm {VCN}((\Gamma ^G)_0) \subseteq \mathrm {VCN}(\Gamma )$
. But this implies that
![]() $\alpha _g$
is trivial, that is, that the action
$\alpha _g$
is trivial, that is, that the action
![]() $\rho _{s,*}$
of G coincides with the action of
$\rho _{s,*}$
of G coincides with the action of
![]() $G ( = G\times \{0\} \subseteq G \times \mathbb {Z}/l\mathbb {Z})$
on
$G ( = G\times \{0\} \subseteq G \times \mathbb {Z}/l\mathbb {Z})$
on
![]() $\Gamma _*$
defined above.
$\Gamma _*$
defined above.
On the other hand, since
![]() $\phi $
is an isomorphism, it follows that
$\phi $
is an isomorphism, it follows that
![]() $(\Gamma _*)^G \subseteq \Gamma _*$
is contained in the sub-semi-graph of
$(\Gamma _*)^G \subseteq \Gamma _*$
is contained in the sub-semi-graph of
![]() $\Gamma _*$
determined by
$\Gamma _*$
determined by
![]() $((\Gamma ^G)_0^{\multimap })^{\coprod }$
. In particular, it follows immediately from Lemma 1.5(ii) that the image of
$((\Gamma ^G)_0^{\multimap })^{\coprod }$
. In particular, it follows immediately from Lemma 1.5(ii) that the image of
![]() $\Gamma _*^G\subseteq \Gamma _*$
in
$\Gamma _*^G\subseteq \Gamma _*$
in
![]() $\Gamma $
is contained in
$\Gamma $
is contained in
![]() $(\Gamma ^G)_0\subseteq \Gamma ^G$
. Thus, it follows immediately from assertion (i) that the image of
$(\Gamma ^G)_0\subseteq \Gamma ^G$
. Thus, it follows immediately from assertion (i) that the image of
![]() $\Gamma _*^G\subseteq \Gamma _*$
in
$\Gamma _*^G\subseteq \Gamma _*$
in
![]() $\Gamma $
coincides with
$\Gamma $
coincides with
![]() $(\Gamma ^G)_0\subseteq \Gamma ^G$
. This completes the proof of assertion (v).
$(\Gamma ^G)_0\subseteq \Gamma ^G$
. This completes the proof of assertion (v).
Next, we verify assertion (vi). First, we claim that the following assertion holds:
Claim 1.6.B: If G is isomorphic to
![]() $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
![]() $l\in \Sigma $
, then assertion (vi) holds.
$l\in \Sigma $
, then assertion (vi) holds.
Indeed, it follows from the resp’d portion of assertion (iv) (i.e., the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
) in the case where G is isomorphic to
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
) in the case where G is isomorphic to
![]() $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
![]() $l \in \Sigma $
(i.e., the case that has already been verified!) that
$l \in \Sigma $
(i.e., the case that has already been verified!) that
![]() $(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
. On the other hand, it follows immediately from assertion (v) (i.e., by allowing “
$(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
. On the other hand, it follows immediately from assertion (v) (i.e., by allowing “
![]() $\Gamma $
” to vary among the G-compatible connected finite subcoverings of
$\Gamma $
” to vary among the G-compatible connected finite subcoverings of
![]() $\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
) that
$\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
) that
![]() $(\widetilde \Gamma ^{\Sigma })^G$
is connected. Thus, the final portion of assertion (vi) (in the case where G is isomorphic to
$(\widetilde \Gamma ^{\Sigma })^G$
is connected. Thus, the final portion of assertion (vi) (in the case where G is isomorphic to
![]() $\mathbb {Z}/l\mathbb {Z}$
for some prime number
$\mathbb {Z}/l\mathbb {Z}$
for some prime number
![]() $l\in \Sigma $
) follows immediately from assertion (i) (and the evident pro-
$l\in \Sigma $
) follows immediately from assertion (i) (and the evident pro-
![]() $\Sigma $
version of [Reference Mochizuki17, Prop. 2.5(i)]). This completes the proof of Claim 1.6.B.
$\Sigma $
version of [Reference Mochizuki17, Prop. 2.5(i)]). This completes the proof of Claim 1.6.B.
Next, we verify assertion (vi) for arbitrary finite solvable G by induction on
![]() $G^\sharp $
. Let us first observe that it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
$G^\sharp $
. Let us first observe that it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
![]() $\Gamma $
by
$\Gamma $
by
![]() $\Gamma ^{\div }$
, we may assume without loss of generality that the action
$\Gamma ^{\div }$
, we may assume without loss of generality that the action
![]() $\rho $
is an action without inversion. Next, observe that since G is finite and solvable, there exists a normal subgroup
$\rho $
is an action without inversion. Next, observe that since G is finite and solvable, there exists a normal subgroup
![]() $N\subseteq G$
of G such that
$N\subseteq G$
of G such that
![]() $G/N$
is a nontrivial finite group of prime order. Then it follows from the induction hypothesis that if we write
$G/N$
is a nontrivial finite group of prime order. Then it follows from the induction hypothesis that if we write
![]() $(\Gamma ^N)_{0} \subseteq \Gamma ^N$
for the (nonempty, connected!) image of the composite
$(\Gamma ^N)_{0} \subseteq \Gamma ^N$
for the (nonempty, connected!) image of the composite
![]() $(\widetilde \Gamma ^{\Sigma })^N \hookrightarrow \widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
, then the resulting morphism
$(\widetilde \Gamma ^{\Sigma })^N \hookrightarrow \widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
, then the resulting morphism
![]() $(\widetilde \Gamma ^{\Sigma })^N\rightarrow (\Gamma ^N)_{0}$
is a pro-
$(\widetilde \Gamma ^{\Sigma })^N\rightarrow (\Gamma ^N)_{0}$
is a pro-
![]() $\Sigma $
universal covering of
$\Sigma $
universal covering of
![]() $(\Gamma ^N)_{0}$
, and, moreover, (since the action
$(\Gamma ^N)_{0}$
, and, moreover, (since the action
![]() $\rho $
is an action without inversion) N acts trivially on
$\rho $
is an action without inversion) N acts trivially on
![]() $(\widetilde \Gamma ^{\Sigma })^N$
. Next, let us observe that since N is normal in G, (one verifies immediately that) the action
$(\widetilde \Gamma ^{\Sigma })^N$
. Next, let us observe that since N is normal in G, (one verifies immediately that) the action
![]() $\widetilde \rho _s^{\Sigma }$
of G on
$\widetilde \rho _s^{\Sigma }$
of G on
![]() $\widetilde \Gamma ^{\Sigma }$
preserves
$\widetilde \Gamma ^{\Sigma }$
preserves
![]() $(\widetilde \Gamma ^{\Sigma })^N \subseteq \widetilde \Gamma ^{\Sigma }$
. Thus, by replacing
$(\widetilde \Gamma ^{\Sigma })^N \subseteq \widetilde \Gamma ^{\Sigma }$
. Thus, by replacing
![]() $(\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma , G)$
by
$(\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma , G)$
by
![]() $((\widetilde \Gamma ^{\Sigma })^N \rightarrow (\Gamma ^N)_{0}, G/N)$
and applying Claim 1.6.B, we conclude that assertion (vi) holds for the given G. This completes the proof of assertion (vi).
$((\widetilde \Gamma ^{\Sigma })^N \rightarrow (\Gamma ^N)_{0}, G/N)$
and applying Claim 1.6.B, we conclude that assertion (vi) holds for the given G. This completes the proof of assertion (vi).
Finally, we verify the resp’d portion of assertion (iv) (i.e., the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
). Let us first observe that, to verify the assertion that
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
). Let us first observe that, to verify the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
, it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
, it follows immediately from Lemma 1.4(iii) and (iv) that, by replacing
![]() $\Gamma $
by
$\Gamma $
by
![]() $\Gamma ^{\div }$
, we may assume without loss of generality that
$\Gamma ^{\div }$
, we may assume without loss of generality that
![]() $\Gamma $
is untangled. Thus, the assertion that
$\Gamma $
is untangled. Thus, the assertion that
![]() $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
follows immediately from assertion (vi). This completes the proof of Lemma 1.6.
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
follows immediately from assertion (vi). This completes the proof of Lemma 1.6.
Remark 1.6.1. The conclusion of Lemma 1.6(vi) follows for an arbitrary (i.e., not necessarily solvable!) finite group G from [Reference Zalesskii and Mel’nikov30, Ths. 2.8 and 2.10]. That is to say, the proof given above of Lemma 1.6(vi) may be regarded as an alternative proof of these results of [Reference Zalesskii and Mel’nikov30] in the case where G is solvable. In this context, it is also perhaps of interest to observe that, by considering Lemma 1.6(vi) in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
and “
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
and “
![]() $\Gamma $
” is taken to be some finite connected sub-semi-graph of
$\Gamma $
” is taken to be some finite connected sub-semi-graph of
![]() $\widetilde {\Gamma } ^{\mathrm {disc}}$
that is stabilized by the action of G (where we note that one verifies easily that
$\widetilde {\Gamma } ^{\mathrm {disc}}$
that is stabilized by the action of G (where we note that one verifies easily that
![]() $\widetilde {\Gamma } ^{\mathrm {disc}}$
is a union of such sub-semi-graphs), one obtains an alternative proof of the classical result concerning actions of finite groups on trees quoted in the proofs of Lemma 1.6(iii) and [Reference Mochizuki17, Lem. 1.8(ii)]—hence also alternative proofs of Lemma 1.6(iii) and [Reference Mochizuki17, Lem. 1.8(ii)]—in the case where the finite group under consideration is solvable.
$\widetilde {\Gamma } ^{\mathrm {disc}}$
is a union of such sub-semi-graphs), one obtains an alternative proof of the classical result concerning actions of finite groups on trees quoted in the proofs of Lemma 1.6(iii) and [Reference Mochizuki17, Lem. 1.8(ii)]—hence also alternative proofs of Lemma 1.6(iii) and [Reference Mochizuki17, Lem. 1.8(ii)]—in the case where the finite group under consideration is solvable.
Remark 1.6.2.
-
(i) In the situation of Lemma 1.6, if G is isomorphic to
 $\mathbb {Z}/l^n\mathbb {Z}$
for some prime number
$\mathbb {Z}/l^n\mathbb {Z}$
for some prime number
 $l \in \Sigma $
and a positive integer n, then the conclusion of the resp’d portion of Lemma 1.6(iv) may be verified by the following easier argument: since (as is well known) a projective limit of nonempty finite sets is nonempty, to verify the assertion that
$l \in \Sigma $
and a positive integer n, then the conclusion of the resp’d portion of Lemma 1.6(iv) may be verified by the following easier argument: since (as is well known) a projective limit of nonempty finite sets is nonempty, to verify the assertion that
 $\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
, it suffices to verify that
$\mathrm {VCN}(\widetilde \Gamma ^{\Sigma })^G \neq \emptyset $
, it suffices to verify that
 $\mathrm {VCN}(\Gamma _*)^G\neq \emptyset $
for every G-compatible connected finite subcovering
$\mathrm {VCN}(\Gamma _*)^G\neq \emptyset $
for every G-compatible connected finite subcovering
 $\Gamma _*\rightarrow \Gamma $
of
$\Gamma _*\rightarrow \Gamma $
of
 $\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
. Moreover, one verifies immediately that we may assume without loss of generality that
$\widetilde \Gamma ^{\Sigma } \rightarrow \Gamma $
. Moreover, one verifies immediately that we may assume without loss of generality that
 $\Gamma _*=\Gamma $
. Next, let us observe that it follows immediately from Lemma 1.4(iv) that, by replacing
$\Gamma _*=\Gamma $
. Next, let us observe that it follows immediately from Lemma 1.4(iv) that, by replacing
 $\Gamma $
by
$\Gamma $
by
 $\Gamma ^{\div }$
, we may assume without loss of generality that G acts on
$\Gamma ^{\div }$
, we may assume without loss of generality that G acts on
 $\Gamma $
without inversion. Write
$\Gamma $
without inversion. Write
 $H\subseteq G$
for the unique subgroup such that
$H\subseteq G$
for the unique subgroup such that
 $Q \stackrel {\mathrm {def}}{=} G/H$
is of order l;
$Q \stackrel {\mathrm {def}}{=} G/H$
is of order l;
 $\Gamma _Q \stackrel {\mathrm {def}}{=} \Gamma /H$
for the “quotient semi-graph,” that is, the semi-graph whose vertices, edges, and branches are, respectively, the H-orbits of the vertices, edges, and branches of
$\Gamma _Q \stackrel {\mathrm {def}}{=} \Gamma /H$
for the “quotient semi-graph,” that is, the semi-graph whose vertices, edges, and branches are, respectively, the H-orbits of the vertices, edges, and branches of
 $\Gamma $
(cf. the fact that G acts on
$\Gamma $
(cf. the fact that G acts on
 $\Gamma $
without inversion). Then one verifies immediately that the natural morphism of semi-graphs
$\Gamma $
without inversion). Then one verifies immediately that the natural morphism of semi-graphs
 $\Gamma \twoheadrightarrow \Gamma _Q$
determines an outer homomorphism (cf. the notation of the statement of Lemma 1.6). Now since
$\Gamma \twoheadrightarrow \Gamma _Q$
determines an outer homomorphism (cf. the notation of the statement of Lemma 1.6). Now since $$ \begin{align*}\Pi_{\Gamma // G}^{\Sigma} \longrightarrow \Pi_{\Gamma_Q // Q}^{\Sigma}\end{align*} $$
$$ \begin{align*}\Pi_{\Gamma // G}^{\Sigma} \longrightarrow \Pi_{\Gamma_Q // Q}^{\Sigma}\end{align*} $$
 $\Pi _{\Gamma _Q}^{\Sigma }$
is a free pro-
$\Pi _{\Gamma _Q}^{\Sigma }$
is a free pro-
 $\Sigma $
group, hence torsion-free, it follows that the restriction
$\Sigma $
group, hence torsion-free, it follows that the restriction
 $s(H) \rightarrow \Pi _{\Gamma _Q // Q}^{\Sigma }$
(which clearly factors through
$s(H) \rightarrow \Pi _{\Gamma _Q // Q}^{\Sigma }$
(which clearly factors through
 $\Pi _{\Gamma _Q}^{\Sigma } \subseteq \Pi _{\Gamma _Q // Q}^{\Sigma }$
) of the outer homomorphism
$\Pi _{\Gamma _Q}^{\Sigma } \subseteq \Pi _{\Gamma _Q // Q}^{\Sigma }$
) of the outer homomorphism
 $\Pi _{\Gamma // G}^{\Sigma } \rightarrow \Pi _{\Gamma _Q // Q}^{\Sigma }$
to
$\Pi _{\Gamma // G}^{\Sigma } \rightarrow \Pi _{\Gamma _Q // Q}^{\Sigma }$
to
 $s(H) \subseteq \Pi _{\Gamma // G}^{\Sigma }$
is trivial, hence that s determines a section
$s(H) \subseteq \Pi _{\Gamma // G}^{\Sigma }$
is trivial, hence that s determines a section
 $s_Q\colon Q \rightarrow \Pi _{\Gamma _Q // Q}^{\Sigma }$
of the natural surjection
$s_Q\colon Q \rightarrow \Pi _{\Gamma _Q // Q}^{\Sigma }$
of the natural surjection
 $\Pi _{\Gamma _Q // Q}^{\Sigma } \twoheadrightarrow Q$
. In particular, by applying Lemma 1.6(ii), we thus conclude that
$\Pi _{\Gamma _Q // Q}^{\Sigma } \twoheadrightarrow Q$
. In particular, by applying Lemma 1.6(ii), we thus conclude that
 $\mathrm {VCN}(\Gamma _Q)^Q \neq \emptyset $
. Let
$\mathrm {VCN}(\Gamma _Q)^Q \neq \emptyset $
. Let
 $z_Q \in \mathrm {VCN}(\Gamma _Q)^Q$
, let
$z_Q \in \mathrm {VCN}(\Gamma _Q)^Q$
, let
 $z \in \mathrm {VCN}(\Gamma )$
be a lifting of
$z \in \mathrm {VCN}(\Gamma )$
be a lifting of
 $z_Q$
, and let
$z_Q$
, and let
 $g \in G$
be a generator of G. Then since Q fixes
$g \in G$
be a generator of G. Then since Q fixes
 $z_Q$
, it follows that
$z_Q$
, it follows that
 $z^g = z^h$
, for some
$z^g = z^h$
, for some
 $h \in H$
, hence that z is fixed by
$h \in H$
, hence that z is fixed by
 $g\cdot h^{-1}\in G$
. On the other hand, since
$g\cdot h^{-1}\in G$
. On the other hand, since
 $g\cdot h^{-1}$
generates G, we thus conclude that z is fixed by G, that is, that
$g\cdot h^{-1}$
generates G, we thus conclude that z is fixed by G, that is, that
 $\mathrm {VCN}(\Gamma _*)^G\neq \emptyset $
, as desired.
$\mathrm {VCN}(\Gamma _*)^G\neq \emptyset $
, as desired.
-
(ii) The proof of Lemma 1.6(ii), as well as the argument of (i) above, is essentially the same as the argument applied in [Reference Mochizuki19] to prove [Reference Mochizuki19, Lem. 2.1(iii)].
Remark 1.6.3. In the respective situations of Lemma 1.6(iii) and (vi), the sub-semi-graph
![]() $(\widetilde \Gamma ^{\mathrm {disc}})^G$
and the sub-pro-semi-graph
$(\widetilde \Gamma ^{\mathrm {disc}})^G$
and the sub-pro-semi-graph
![]() $(\widetilde \Gamma ^{\Sigma })^G$
are necessarily connected (cf. Lemma 1.6(iii) and (vi)). On the other hand,
$(\widetilde \Gamma ^{\Sigma })^G$
are necessarily connected (cf. Lemma 1.6(iii) and (vi)). On the other hand,
![]() $\Gamma ^G$
is not, in general, connected. This phenomenon may be seen in the following example: suppose that
$\Gamma ^G$
is not, in general, connected. This phenomenon may be seen in the following example: suppose that
![]() $2\in \Sigma $
, and that
$2\in \Sigma $
, and that
![]() $\widetilde \Gamma ^{\mathrm {disc}}$
is the graph given by the integral points of the real line
$\widetilde \Gamma ^{\mathrm {disc}}$
is the graph given by the integral points of the real line
![]() $\mathbb {R}$
, that is, the vertices are given by the elements of
$\mathbb {R}$
, that is, the vertices are given by the elements of
![]() $\mathbb {Z}\subseteq \mathbb {R}$
, and the edges are given by the closed line segments joining adjacent elements of
$\mathbb {Z}\subseteq \mathbb {R}$
, and the edges are given by the closed line segments joining adjacent elements of
![]() $\mathbb {Z}$
. For
$\mathbb {Z}$
. For
![]() $N=2M$
a positive even integer, write
$N=2M$
a positive even integer, write
![]() $\Gamma _N$
for the quotient of
$\Gamma _N$
for the quotient of
![]() $\widetilde \Gamma ^{\mathrm {disc}}$
by the evident action of
$\widetilde \Gamma ^{\mathrm {disc}}$
by the evident action of
![]() $N \in \mathbb {Z}$
on
$N \in \mathbb {Z}$
on
![]() $\widetilde \Gamma ^{\mathrm {disc}}$
via translations. Thus, we have a diagram of natural covering maps
$\widetilde \Gamma ^{\mathrm {disc}}$
via translations. Thus, we have a diagram of natural covering maps
and the group
![]() $G=\mathbb {Z}/2\mathbb {Z}$
acts equivariantly on this diagram via multiplication by
$G=\mathbb {Z}/2\mathbb {Z}$
acts equivariantly on this diagram via multiplication by
![]() $\pm 1$
. Here, we observe that since N is even, one verifies immediately that G acts on
$\pm 1$
. Here, we observe that since N is even, one verifies immediately that G acts on
![]() $\Gamma _N$
without inversion. Then one computes easily that
$\Gamma _N$
without inversion. Then one computes easily that
In particular, the pro-semi-graph
![]() $(\widetilde \Gamma ^{\Sigma })^G$
corresponds to the inverse limit
$(\widetilde \Gamma ^{\Sigma })^G$
corresponds to the inverse limit
hence consists of a single pro-vertex and no pro-edge and, in particular, is nonempty and connected. On the other hand, each
![]() $\Gamma _N^G$
consists of precisely two vertices and no edges, hence is not connected.
$\Gamma _N^G$
consists of precisely two vertices and no edges, hence is not connected.
Definition 1.7. Let G be a profinite group, and let
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism.
$\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism.
-
(i) We shall say that
 $\rho $
is of ENN-type (where the “ENN” stands for “extended NN”) (resp. of EPIPSC-type (where the “EPIPSC” stands for “extended PIPSC”]) if there exists a normal closed subgroup
$\rho $
is of ENN-type (where the “ENN” stands for “extended NN”) (resp. of EPIPSC-type (where the “EPIPSC” stands for “extended PIPSC”]) if there exists a normal closed subgroup
 $I_G \subseteq G$
of G such that, for every open subgroup
$I_G \subseteq G$
of G such that, for every open subgroup
 $J\subseteq I_G$
of
$J\subseteq I_G$
of
 $I_G$
, the composite
$I_G$
, the composite
 $J\hookrightarrow G \stackrel {\rho }{\rightarrow }\mathrm {Aut}(\mathcal {G})$
factors as a composite
$J\hookrightarrow G \stackrel {\rho }{\rightarrow }\mathrm {Aut}(\mathcal {G})$
factors as a composite
 $J\twoheadrightarrow J^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow \mathrm {Aut}(\mathcal {G})$
(cf. the discussion entitled “Groups” in §0), where the second arrow is of NN-type (cf. [Reference Hoshi and Mochizuki8, Def. 2.4(iii)]) (resp. of PIPSC-type (cf. [Reference Hoshi and Mochizuki11, Def. 1.3]). In this situation, we shall refer to
$J\twoheadrightarrow J^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow \mathrm {Aut}(\mathcal {G})$
(cf. the discussion entitled “Groups” in §0), where the second arrow is of NN-type (cf. [Reference Hoshi and Mochizuki8, Def. 2.4(iii)]) (resp. of PIPSC-type (cf. [Reference Hoshi and Mochizuki11, Def. 1.3]). In this situation, we shall refer to
 $I_G$
as a conducting subgroup. Suppose that
$I_G$
as a conducting subgroup. Suppose that
 $\rho $
is of ENN-type for some conducting subgroup
$\rho $
is of ENN-type for some conducting subgroup
 $I_G \subseteq G$
. Then we shall say that
$I_G \subseteq G$
. Then we shall say that
 $\rho $
is verticially quasi-split if there exists an open subgroup
$\rho $
is verticially quasi-split if there exists an open subgroup
 $H\subseteq G$
that acts as the identity (i.e., relative to the action induced by
$H\subseteq G$
that acts as the identity (i.e., relative to the action induced by
 $\rho $
) on the underlying semi-graph
$\rho $
) on the underlying semi-graph
 $\mathbb {G}$
of
$\mathbb {G}$
of
 $\mathcal {G}$
and, moreover, for every
$\mathcal {G}$
and, moreover, for every
 $v\in \mathrm {Vert}(\mathcal {G})$
, satisfies the following condition: the extension of profinite groups (cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9], §0) where
$v\in \mathrm {Vert}(\mathcal {G})$
, satisfies the following condition: the extension of profinite groups (cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9], §0) where $$\begin{align*}1 \longrightarrow \Pi_{v} \stackrel{}{\longrightarrow} \Pi_{v}\stackrel{\mathrm{out}}{\rtimes} H \stackrel{}{\longrightarrow} H \longrightarrow 1, \end{align*}$$
$$\begin{align*}1 \longrightarrow \Pi_{v} \stackrel{}{\longrightarrow} \Pi_{v}\stackrel{\mathrm{out}}{\rtimes} H \stackrel{}{\longrightarrow} H \longrightarrow 1, \end{align*}$$
 $\Pi _{v}\subseteq \Pi _{\mathcal {G}}$
is a verticial subgroup associated with
$\Pi _{v}\subseteq \Pi _{\mathcal {G}}$
is a verticial subgroup associated with
 $v \in \mathrm {Vert}(\mathcal {G})$
, associated with the outer action of H on
$v \in \mathrm {Vert}(\mathcal {G})$
, associated with the outer action of H on
 $\Pi _{v}$
determined by
$\Pi _{v}$
determined by
 $\rho $
(cf. [Reference Mochizuki18, Prop. 1.2(ii)]; [Reference Hoshi and Mochizuki9, Lem. 2.12]) admits a splitting
$\rho $
(cf. [Reference Mochizuki18, Prop. 1.2(ii)]; [Reference Hoshi and Mochizuki9, Lem. 2.12]) admits a splitting
 $s_v:H \rightarrow \Pi _{v} \stackrel {\mathrm {out}}{\rtimes } H$
such that the image of the restriction of
$s_v:H \rightarrow \Pi _{v} \stackrel {\mathrm {out}}{\rtimes } H$
such that the image of the restriction of
 $s_v$
to
$s_v$
to
 $I_G \cap H$
commutes with
$I_G \cap H$
commutes with
 $\Pi _{v}$
.
$\Pi _{v}$
.
-
(ii) Let
 $l \in \Sigma $
. Then we shall say that
$l \in \Sigma $
. Then we shall say that
 $\rho $
is l-cyclotomically full if the image of the composite
$\rho $
is l-cyclotomically full if the image of the composite
 $G \stackrel {\rho }{\rightarrow }\mathrm {Aut}(\mathcal {G}) \stackrel {\chi _{\mathcal {G}}}{\rightarrow } (\widehat {\mathbb {Z}}^{\Sigma })^\times \twoheadrightarrow \mathbb {Z}_l^\times $
(cf. [Reference Hoshi and Mochizuki9, Def. 3.8(ii)]) is open.
$G \stackrel {\rho }{\rightarrow }\mathrm {Aut}(\mathcal {G}) \stackrel {\chi _{\mathcal {G}}}{\rightarrow } (\widehat {\mathbb {Z}}^{\Sigma })^\times \twoheadrightarrow \mathbb {Z}_l^\times $
(cf. [Reference Hoshi and Mochizuki9, Def. 3.8(ii)]) is open.
Remark 1.7.1. It follows immediately from [Reference Hoshi and Mochizuki11, Rem. 1.6.2] that the following implication holds:
Lemma 1.8 (Outer representations induced on pro-l completions).
Let G be a profinite group, and let
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism of ENN-type (resp. of EPIPSC-type) for a conducting subgroup
$\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism of ENN-type (resp. of EPIPSC-type) for a conducting subgroup
![]() $I_G\subseteq G$
(cf. Definition 1.7(i)). For
$I_G\subseteq G$
(cf. Definition 1.7(i)). For
![]() $l\in \Sigma $
, write
$l\in \Sigma $
, write
![]() $\mathcal {G}^{\{l\}}$
for the semi-graph of anabelioids of pro-
$\mathcal {G}^{\{l\}}$
for the semi-graph of anabelioids of pro-
![]() $\{l\}$
PSC-type obtained by forming the pro-l completion of
$\{l\}$
PSC-type obtained by forming the pro-l completion of
![]() $\mathcal {G}$
(cf. [Reference Mochizuki17, Def. 2.9(ii)]). Then the composite
$\mathcal {G}$
(cf. [Reference Mochizuki17, Def. 2.9(ii)]). Then the composite
![]() $G\stackrel {\rho }{\rightarrow }\mathrm {Aut}(\mathcal {G})\rightarrow \mathrm {Aut}(\mathcal {G}^{\{l\}})$
is of ENN-type (resp. of EPIPSC-type) for some conducting subgroup
$G\stackrel {\rho }{\rightarrow }\mathrm {Aut}(\mathcal {G})\rightarrow \mathrm {Aut}(\mathcal {G}^{\{l\}})$
is of ENN-type (resp. of EPIPSC-type) for some conducting subgroup
![]() $\subseteq G$
, which may be taken to be a normal open subgroup of
$\subseteq G$
, which may be taken to be a normal open subgroup of
![]() $I_G$
.
$I_G$
.
Proof. This follows immediately from the various definitions involved (cf. also [Reference Hoshi and Mochizuki9, Th. 4.8(iv)]; [Reference Hoshi and Mochizuki9, Cor. 5.9(ii) and (iii)]).
Definition 1.9. Let
![]() $z \in \mathrm {VCN}(\mathcal {G})$
. If
$z \in \mathrm {VCN}(\mathcal {G})$
. If
![]() $z \in \mathrm {Vert}(\mathcal {G})$
(resp.
$z \in \mathrm {Vert}(\mathcal {G})$
(resp.
![]() $z \in \mathrm {Edge}(\mathcal {G})$
), then we shall refer to a verticial (resp. an edge-like) subgroup of
$z \in \mathrm {Edge}(\mathcal {G})$
), then we shall refer to a verticial (resp. an edge-like) subgroup of
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with z (cf. [Reference Mochizuki17, Th. 3.7(i) and (iii)]) as a VCN-subgroup of
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with z (cf. [Reference Mochizuki17, Th. 3.7(i) and (iii)]) as a VCN-subgroup of
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with z. For
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with z. For
![]() $\widetilde {z} \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
, we shall also speak of VCN-subgroups of
$\widetilde {z} \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
, we shall also speak of VCN-subgroups of
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with
![]() $\widetilde z$
.
$\widetilde z$
.
Definition 1.10.
-
(i) Let
 $\Gamma $
be a semi-graph and
$\Gamma $
be a semi-graph and
 $v \in \mathrm {Vert}(\Gamma )$
. Then we shall write
$v \in \mathrm {Vert}(\Gamma )$
. Then we shall write
 $\mathcal {V}^{\delta \leq 1}(v) \subseteq \mathrm {Vert}(\Gamma )$
for the subset consisting of
$\mathcal {V}^{\delta \leq 1}(v) \subseteq \mathrm {Vert}(\Gamma )$
for the subset consisting of
 $w \in \mathrm {Vert}(\Gamma )$
such that either
$w \in \mathrm {Vert}(\Gamma )$
such that either
 $w = v$
or
$w = v$
or
 $\mathcal {N}(v) \cap \mathcal {N}(w) \neq \emptyset $
. Also, we shall write
$\mathcal {N}(v) \cap \mathcal {N}(w) \neq \emptyset $
. Also, we shall write
 $\mathrm {Star}(v) \stackrel {\mathrm {def}}{=} \mathcal {V}^{\delta \leq 1}(v) \sqcup \mathcal {E}(v) \subseteq \mathrm {VCN}(\Gamma )$
.
$\mathrm {Star}(v) \stackrel {\mathrm {def}}{=} \mathcal {V}^{\delta \leq 1}(v) \sqcup \mathcal {E}(v) \subseteq \mathrm {VCN}(\Gamma )$
. -
(ii) Let
 $v \in \mathrm {Vert}(\mathcal {G})$
. Then we shall write
$v \in \mathrm {Vert}(\mathcal {G})$
. Then we shall write
 $\mathcal {V}^{\delta \leq 1}(v) \subseteq \mathrm {Vert}(\mathcal {G})$
,
$\mathcal {V}^{\delta \leq 1}(v) \subseteq \mathrm {Vert}(\mathcal {G})$
,
 $\mathrm {Star}(v) \subseteq \mathrm {VCN}(\mathcal {G})$
for the respective subsets of (i) applied to the underlying semi-graph of
$\mathrm {Star}(v) \subseteq \mathrm {VCN}(\mathcal {G})$
for the respective subsets of (i) applied to the underlying semi-graph of
 $\mathcal {G}$
.
$\mathcal {G}$
. -
(iii) Let
 $\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
. Then we shall write
$\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
. Then we shall write
 $\mathcal {V}^{\delta \leq 1}(\widetilde v) \subseteq \mathrm {Vert}(\widetilde {\mathcal {G}})$
,
$\mathcal {V}^{\delta \leq 1}(\widetilde v) \subseteq \mathrm {Vert}(\widetilde {\mathcal {G}})$
,
 $\mathrm {Star}(\widetilde v) \subseteq \mathrm {VCN}(\widetilde {\mathcal {G}})$
for the respective projective limits of the subsets of (ii), that is, where the constructions of these subsets are applied to the images of
$\mathrm {Star}(\widetilde v) \subseteq \mathrm {VCN}(\widetilde {\mathcal {G}})$
for the respective projective limits of the subsets of (ii), that is, where the constructions of these subsets are applied to the images of
 $\widetilde v$
in the connected finite etale subcoverings of
$\widetilde v$
in the connected finite etale subcoverings of
 $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
.
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
.
Lemma 1.11 (VCN-subgroups and sections).
Let G be a profinite group, let
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism, let
$\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism, let
![]() $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, let
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, let
![]() $\widetilde z^{\mathrm {tp}} \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
, let
$\widetilde z^{\mathrm {tp}} \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
, let
![]() $\Pi _{\widetilde z} \subseteq \Pi _{\mathcal {G}}$
be a VCN-subgroup of
$\Pi _{\widetilde z} \subseteq \Pi _{\mathcal {G}}$
be a VCN-subgroup of
![]() $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
![]() $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, and let
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, and let
![]() $\Pi _{\widetilde z^{\mathrm {tp}}} \subseteq \Pi _{\mathcal {G}}^{\mathrm {tp}}$
be a VCN-subgroup of
$\Pi _{\widetilde z^{\mathrm {tp}}} \subseteq \Pi _{\mathcal {G}}^{\mathrm {tp}}$
be a VCN-subgroup of
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
associated with
![]() $\widetilde z^{\mathrm {tp}}$
(cf. Definition 1.9). Write
$\widetilde z^{\mathrm {tp}}$
(cf. Definition 1.9). Write
![]() $\Pi _{G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } G$
,
$\Pi _{G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } G$
,
![]() $\Pi _{G}^{\mathrm {tp}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^{\mathrm {tp}}\stackrel {\mathrm {out}}{\rtimes } G$
(cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]). Thus, we have a natural commutative diagram
$\Pi _{G}^{\mathrm {tp}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^{\mathrm {tp}}\stackrel {\mathrm {out}}{\rtimes } G$
(cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]). Thus, we have a natural commutative diagram

—where the horizontal sequences are exact, the vertical arrows are outer injections,
![]() $\Pi _{G}^{\mathrm {tp}}$
acts naturally on
$\Pi _{G}^{\mathrm {tp}}$
acts naturally on
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}}$
, and
$\widetilde {\mathcal {G}}^{\mathrm {tp}}$
, and
![]() $\Pi _{G}$
acts naturally on
$\Pi _{G}$
acts naturally on
![]() $\widetilde {\mathcal {G}}$
. Then the following hold:
$\widetilde {\mathcal {G}}$
. Then the following hold:
-
(i) It holds that
 $$ \begin{align*}\Pi_{\widetilde z} = N_{\Pi_{G}}(\Pi_{\widetilde z}) \cap \Pi_{\mathcal{G}} = C_{\Pi_{G}}(\Pi_{\widetilde z}) \cap \Pi_{\mathcal{G}}, \end{align*} $$
$$ \begin{align*}\Pi_{\widetilde z} = N_{\Pi_{G}}(\Pi_{\widetilde z}) \cap \Pi_{\mathcal{G}} = C_{\Pi_{G}}(\Pi_{\widetilde z}) \cap \Pi_{\mathcal{G}}, \end{align*} $$
 $$ \begin{align*}D_{\widetilde z} \stackrel{\mathrm{def}}{=} N_{\Pi_{G}}(\Pi_{\widetilde z}) = C_{\Pi_{G}}(\Pi_{\widetilde z}) = N_{\Pi_{G}}(D_{\widetilde z}) = C_{\Pi_{G}}(D_{\widetilde z}), \end{align*} $$
$$ \begin{align*}D_{\widetilde z} \stackrel{\mathrm{def}}{=} N_{\Pi_{G}}(\Pi_{\widetilde z}) = C_{\Pi_{G}}(\Pi_{\widetilde z}) = N_{\Pi_{G}}(D_{\widetilde z}) = C_{\Pi_{G}}(D_{\widetilde z}), \end{align*} $$
 $$ \begin{align*}\Pi_{\widetilde z^{\mathrm{tp}}} = N_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) \cap \Pi_{\mathcal{G}}^{\mathrm{tp}} = C_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) \cap \Pi_{\mathcal{G}}^{\mathrm{tp}}, \end{align*} $$
$$ \begin{align*}\Pi_{\widetilde z^{\mathrm{tp}}} = N_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) \cap \Pi_{\mathcal{G}}^{\mathrm{tp}} = C_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) \cap \Pi_{\mathcal{G}}^{\mathrm{tp}}, \end{align*} $$
 $$ \begin{align*}D_{\widetilde z^{\mathrm{tp}}} \stackrel{\mathrm{def}}{=} N_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) = C_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) = N_{\Pi_{G}^{\mathrm{tp}}}(D_{\widetilde z^{\mathrm{tp}}}) = C_{\Pi_{G}^{\mathrm{tp}}}(D_{\widetilde z^{\mathrm{tp}}}). \end{align*} $$
$$ \begin{align*}D_{\widetilde z^{\mathrm{tp}}} \stackrel{\mathrm{def}}{=} N_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) = C_{\Pi_{G}^{\mathrm{tp}}}(\Pi_{\widetilde z^{\mathrm{tp}}}) = N_{\Pi_{G}^{\mathrm{tp}}}(D_{\widetilde z^{\mathrm{tp}}}) = C_{\Pi_{G}^{\mathrm{tp}}}(D_{\widetilde z^{\mathrm{tp}}}). \end{align*} $$
-
(ii) Suppose that
 $\rho $
is of ENN-type for a conducting subgroup
$\rho $
is of ENN-type for a conducting subgroup
 $I_G\subseteq G$
(cf. Definition 1.7(i)). Let S be a nonempty subset of
$I_G\subseteq G$
(cf. Definition 1.7(i)). Let S be a nonempty subset of
 $\mathrm {VCN}(\widetilde {\mathcal {G}})$
, and let
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
, and let
 $s \colon G\rightarrow \Pi _{G}$
be a section of the surjection
$s \colon G\rightarrow \Pi _{G}$
be a section of the surjection
 $\Pi _{G} \twoheadrightarrow G$
such that, for each
$\Pi _{G} \twoheadrightarrow G$
such that, for each
 $\widetilde y \in S$
, it holds that
$\widetilde y \in S$
, it holds that
 $s(I_G) \prec D_{\widetilde y}$
(cf. the discussion entitled “Groups” in §0). Then there exists an element
$s(I_G) \prec D_{\widetilde y}$
(cf. the discussion entitled “Groups” in §0). Then there exists an element
 $\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
such that
$\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
such that
 $S \subseteq \mathrm {Star}(\widetilde v)$
(cf. Definition 1.10(iii)).
$S \subseteq \mathrm {Star}(\widetilde v)$
(cf. Definition 1.10(iii)). -
(iii) Suppose that
 $\rho $
is of ENN-type for a conducting subgroup
$\rho $
is of ENN-type for a conducting subgroup
 $I_G\subseteq G$
. Let
$I_G\subseteq G$
. Let
 $s \colon G\rightarrow \Pi _{G}$
be a section of the surjection
$s \colon G\rightarrow \Pi _{G}$
be a section of the surjection
 $\Pi _{G} \twoheadrightarrow G$
such that
$\Pi _{G} \twoheadrightarrow G$
such that
 $s(I_G) \prec D_{\widetilde z}$
(cf. the discussion entitled “Groups” in §0). Write
$s(I_G) \prec D_{\widetilde z}$
(cf. the discussion entitled “Groups” in §0). Write
 $G_s \stackrel {\mathrm {def}}{=} C_{\Pi _G}(s(I_G))$
. Then there exists an element
$G_s \stackrel {\mathrm {def}}{=} C_{\Pi _G}(s(I_G))$
. Then there exists an element
 $\widetilde z' \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
such that
$\widetilde z' \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
such that
 $s(G) \subseteq G_s \subseteq D_{\widetilde z'}$
.
$s(G) \subseteq G_s \subseteq D_{\widetilde z'}$
. -
(iv) Suppose that
 $\rho $
is of ENN-type for a conducting subgroup
$\rho $
is of ENN-type for a conducting subgroup
 $I_G\subseteq G$
. Let
$I_G\subseteq G$
. Let
 $s \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be a section of the surjection
$s \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be a section of the surjection
 $\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
such that
$\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
such that
 $s(I_G) \prec D_{\widetilde z^{\mathrm {tp}}} $
(cf. the discussion entitled “Groups” in §0). Write
$s(I_G) \prec D_{\widetilde z^{\mathrm {tp}}} $
(cf. the discussion entitled “Groups” in §0). Write
 $G_s \stackrel {\mathrm {def}}{=} C_{\Pi ^{\mathrm {tp}}_G}(s(I_G))$
. Then there exists an element
$G_s \stackrel {\mathrm {def}}{=} C_{\Pi ^{\mathrm {tp}}_G}(s(I_G))$
. Then there exists an element
 $(\widetilde z')^{\mathrm {tp}} \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
such that
$(\widetilde z')^{\mathrm {tp}} \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
such that
 $s(G) \subseteq G_s \subseteq D_{(\widetilde z')^{\mathrm {tp}}}$
. In particular,
$s(G) \subseteq G_s \subseteq D_{(\widetilde z')^{\mathrm {tp}}}$
. In particular,
 $G_s$
is contained in a profinite subgroup of
$G_s$
is contained in a profinite subgroup of
 $\Pi ^{\mathrm {tp}}_G$
(cf. (i)).
$\Pi ^{\mathrm {tp}}_G$
(cf. (i)).
Proof. First, we verify assertion (i). The two equalities of the first (resp. third) line of the display and the first “
![]() $=$
” of the second (resp. fourth) line of the display follow immediately from [Reference Mochizuki18, Prop. 1.2(i) and (ii)] (resp. [Reference Mochizuki18, Prop. 1.2(i) and (ii)], together with the injection reviewed at the beginning of the present §1). Thus, the second and third “
$=$
” of the second (resp. fourth) line of the display follow immediately from [Reference Mochizuki18, Prop. 1.2(i) and (ii)] (resp. [Reference Mochizuki18, Prop. 1.2(i) and (ii)], together with the injection reviewed at the beginning of the present §1). Thus, the second and third “
![]() $=$
” of the second (resp. fourth) line of the display follow immediately from the chain of inclusions
$=$
” of the second (resp. fourth) line of the display follow immediately from the chain of inclusions
(resp.
where the third “
![]() $\subseteq $
” follows immediately from [Reference Hoshi and Mochizuki10, Lem. 3.9(i)] (resp. the [easily verified] tempered version of [Reference Hoshi and Mochizuki10, Lem. 3.9(i)]). This completes the proof of assertion (i).
$\subseteq $
” follows immediately from [Reference Hoshi and Mochizuki10, Lem. 3.9(i)] (resp. the [easily verified] tempered version of [Reference Hoshi and Mochizuki10, Lem. 3.9(i)]). This completes the proof of assertion (i).
Next, we verify assertion (ii). Let us first observe that it follows from the definition of the term “ENN-type” that the restriction of
![]() $\rho $
to
$\rho $
to
![]() $I_G\subseteq G$
factors through the quotient
$I_G\subseteq G$
factors through the quotient
![]() $I_G \twoheadrightarrow I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}$
(cf. the discussion entitled “Groups” in §0). Write
$I_G \twoheadrightarrow I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}$
(cf. the discussion entitled “Groups” in §0). Write
![]() $\Pi _{I_G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } I_G$
and
$\Pi _{I_G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } I_G$
and
![]() $\Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}$
. Thus, we have a commutative diagram
$\Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}$
. Thus, we have a commutative diagram

—where the horizontal sequences are exact, the upper vertical arrows are injective, the lower vertical arrows are surjective, and the two right-hand squares are Cartesian. Next, let us observe that we may assume without loss of generality that S is equal to the set of all
![]() $\widetilde y \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
such that
$\widetilde y \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
such that
![]() $s(I_G) \prec D_{\widetilde y}$
. Now since
$s(I_G) \prec D_{\widetilde y}$
. Now since
![]() $s(I_G) \prec D_{\widetilde y} = C_{\Pi _{G}}(\Pi _{\widetilde y})$
(cf. assertion (i)) for every
$s(I_G) \prec D_{\widetilde y} = C_{\Pi _{G}}(\Pi _{\widetilde y})$
(cf. assertion (i)) for every
![]() $\widetilde y \in S$
, it holds that, for each
$\widetilde y \in S$
, it holds that, for each
![]() $\widetilde y \in S$
, some open subgroup of the image
$\widetilde y \in S$
, some open subgroup of the image
![]() $J\subseteq \Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}}$
of
$J\subseteq \Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}}$
of
![]() $I_G \stackrel {s}{\rightarrow }\Pi _{I_G} \twoheadrightarrow \Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}}$
is contained in
$I_G \stackrel {s}{\rightarrow }\Pi _{I_G} \twoheadrightarrow \Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}}$
is contained in
![]() $C_{\Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}}} (\Pi _{\widetilde y})$
. In particular, it follows from [Reference Hoshi and Mochizuki8, Props. 3.8(i) and 3.9(i)–(iii)] that:
$C_{\Pi _{I_G^{\Sigma \text {-}\mathrm {ab}\text {-}\mathrm {free}}}} (\Pi _{\widetilde y})$
. In particular, it follows from [Reference Hoshi and Mochizuki8, Props. 3.8(i) and 3.9(i)–(iii)] that:
-
• every pair of edges
 $\in S$
abut to a common vertex
$\in S$
abut to a common vertex
 $\in S$
;
$\in S$
; -
• the distance between any two vertices
 $\in S$
is
$\in S$
is
 $\leq 2$
(cf. Definition 1.1(iii)), and the edges “
$\leq 2$
(cf. Definition 1.1(iii)), and the edges “
 $e_1, \dots , e_n$
” and vertices “
$e_1, \dots , e_n$
” and vertices “
 $v_0, \dots , v_n$
” of Definition 1.1(iii) may be taken to be
$v_0, \dots , v_n$
” of Definition 1.1(iii) may be taken to be
 $\in S$
;
$\in S$
; -
• if
 $\widetilde {e} \in S$
is an edge, then
$\widetilde {e} \in S$
is an edge, then
 $\mathcal {V}(\widetilde {e}) \subseteq S$
.
$\mathcal {V}(\widetilde {e}) \subseteq S$
.
It is now a matter of elementary combinatorial graph theory (cf. also [Reference Hoshi and Mochizuki8, Lem. 1.8]) to conclude that
![]() $S \subseteq \mathrm {Star}(\widetilde v)$
for some
$S \subseteq \mathrm {Star}(\widetilde v)$
for some
![]() $\widetilde v\in \mathrm {Vert}(\widetilde {\mathcal {G}})$
, as desired. This completes the proof of assertion (ii).
$\widetilde v\in \mathrm {Vert}(\widetilde {\mathcal {G}})$
, as desired. This completes the proof of assertion (ii).
Next, we verify assertion (iii). Since
![]() $s(I_G) \prec D_{\widetilde z}$
, the action of some open subgroup of
$s(I_G) \prec D_{\widetilde z}$
, the action of some open subgroup of
![]() $I_G$
on
$I_G$
on
![]() $\widetilde {\mathcal {G}}$
determined by
$\widetilde {\mathcal {G}}$
determined by
![]() $s|_{I_G}$
fixes
$s|_{I_G}$
fixes
![]() $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
. Thus, it follows from the definition of
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
. Thus, it follows from the definition of
![]() $G_s$
that, if, for
$G_s$
that, if, for
![]() $\gamma \in G_s$
, we write
$\gamma \in G_s$
, we write
![]() $\widetilde z^\gamma \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
for the image of
$\widetilde z^\gamma \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
for the image of
![]() $\widetilde z$
by the action of
$\widetilde z$
by the action of
![]() $\gamma \in G_s$
, then the action of some open subgroup of
$\gamma \in G_s$
, then the action of some open subgroup of
![]() $I_G$
on
$I_G$
on
![]() $\widetilde {\mathcal {G}}$
fixes
$\widetilde {\mathcal {G}}$
fixes
![]() $\widetilde z^\gamma \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, that is,
$\widetilde z^\gamma \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, that is,
![]() $s(I_G) \prec D_{\widetilde z^\gamma }$
for every
$s(I_G) \prec D_{\widetilde z^\gamma }$
for every
![]() $\gamma \in G_s$
.
$\gamma \in G_s$
.
Now suppose that
![]() $\widetilde z \in \mathrm {Edge}(\widetilde {\mathcal {G}})$
. Then it follows from assertion (ii) that there exists a vertex
$\widetilde z \in \mathrm {Edge}(\widetilde {\mathcal {G}})$
. Then it follows from assertion (ii) that there exists a vertex
![]() $\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
such that
$\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
such that
![]() $\{\,\widetilde z^\gamma \,| \, \gamma \in G_s\,\} \subseteq \mathcal {E}(\widetilde v)$
. Now if
$\{\,\widetilde z^\gamma \,| \, \gamma \in G_s\,\} \subseteq \mathcal {E}(\widetilde v)$
. Now if
![]() $\{\,\widetilde z^\gamma \,| \, \gamma \in G_s\,\}^\sharp = 1$
, then it is immediate that
$\{\,\widetilde z^\gamma \,| \, \gamma \in G_s\,\}^\sharp = 1$
, then it is immediate that
![]() $G_s \subseteq D_{\widetilde z}$
. On the other hand, if
$G_s \subseteq D_{\widetilde z}$
. On the other hand, if
![]() $\{\,\widetilde z^\gamma \,| \, \gamma \in G_s\,\}^\sharp \geq 2$
, then one verifies immediately from the various definitions involved (cf. also [Reference Hoshi and Mochizuki8, Lem. 1.8]) that the action of
$\{\,\widetilde z^\gamma \,| \, \gamma \in G_s\,\}^\sharp \geq 2$
, then one verifies immediately from the various definitions involved (cf. also [Reference Hoshi and Mochizuki8, Lem. 1.8]) that the action of
![]() $G_s$
fixes
$G_s$
fixes
![]() $\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
, which thus implies that
$\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
, which thus implies that
![]() $G_s \subseteq D_{\widetilde v}$
. This completes the proof of assertion (iii) in the case where
$G_s \subseteq D_{\widetilde v}$
. This completes the proof of assertion (iii) in the case where
![]() $\widetilde z \in \mathrm {Edge}(\widetilde {\mathcal {G}})$
.
$\widetilde z \in \mathrm {Edge}(\widetilde {\mathcal {G}})$
.
Next, suppose that
![]() $\widetilde z \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
. Then it follows from assertion (ii) that the set
$\widetilde z \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
. Then it follows from assertion (ii) that the set
![]() $S_\delta $
of vertices
$S_\delta $
of vertices
![]() $\widetilde v\in \mathrm {Vert}(\widetilde {\mathcal {G}})$
such that
$\widetilde v\in \mathrm {Vert}(\widetilde {\mathcal {G}})$
such that
-
•
 $S_{\widetilde z} \stackrel {\mathrm {def}}{=} \{\, \widetilde z^\gamma \, | \, \gamma \in G_s \, \} \subseteq \mathcal {V}^{\delta \leq 1}(\widetilde v)$
;
$S_{\widetilde z} \stackrel {\mathrm {def}}{=} \{\, \widetilde z^\gamma \, | \, \gamma \in G_s \, \} \subseteq \mathcal {V}^{\delta \leq 1}(\widetilde v)$
; -
• any edge
 $\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
that abuts to two distinct elements of
$\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
that abuts to two distinct elements of
 $S_{\widetilde z}$
(hence is fixed by the action, determined by
$S_{\widetilde z}$
(hence is fixed by the action, determined by
 $s|_{I_G}$
, of some open subgroup of
$s|_{I_G}$
, of some open subgroup of
 $I_G$
—cf. [Reference Hoshi and Mochizuki8, Prop. 3.9(ii)]) necessarily abuts to
$I_G$
—cf. [Reference Hoshi and Mochizuki8, Prop. 3.9(ii)]) necessarily abuts to
 $\widetilde v$
$\widetilde v$
is nonempty. If the action of
![]() $G_s$
fixes some
$G_s$
fixes some
![]() $\widetilde y \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, then
$\widetilde y \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, then
![]() $G_s \subseteq D_{\widetilde y}$
. Thus, we may assume without loss of generality that the action of
$G_s \subseteq D_{\widetilde y}$
. Thus, we may assume without loss of generality that the action of
![]() $G_s$
does not fix any element of
$G_s$
does not fix any element of
![]() $\mathrm {VCN}(\widetilde {\mathcal {G}})$
. In particular, it follows that the (nonempty!) sets
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
. In particular, it follows that the (nonempty!) sets
![]() $S_{\widetilde z}$
and
$S_{\widetilde z}$
and
![]() $S_\delta $
—both of which are clearly preserved by the action of
$S_\delta $
—both of which are clearly preserved by the action of
![]() $G_s$
—are of cardinality
$G_s$
—are of cardinality
![]() $\geq 2$
. In a similar vein,
$\geq 2$
. In a similar vein,
![]() $S_\delta \setminus (S_\delta \cap S_{\widetilde z})$
is either empty or of cardinality
$S_\delta \setminus (S_\delta \cap S_{\widetilde z})$
is either empty or of cardinality
![]() $\geq 2$
. Moreover, the latter case contradicts [Reference Hoshi and Mochizuki8, Lem. 1.8. Thus, we conclude that
$\geq 2$
. Moreover, the latter case contradicts [Reference Hoshi and Mochizuki8, Lem. 1.8. Thus, we conclude that
![]() $S_\delta \subseteq S_{\widetilde z}$
, which, by the definition of
$S_\delta \subseteq S_{\widetilde z}$
, which, by the definition of
![]() $S_{\widetilde z}$
and
$S_{\widetilde z}$
and
![]() $S_\delta $
, implies that
$S_\delta $
, implies that
![]() $S_\delta =S_{\widetilde z}$
, that is, that, for any two distinct
$S_\delta =S_{\widetilde z}$
, that is, that, for any two distinct
![]() $\widetilde z_1$
,
$\widetilde z_1$
,
![]() $\widetilde z_2 \in S_{\widetilde z}$
, there exists a (unique, by [Reference Hoshi and Mochizuki8, Lem. 1.8])
$\widetilde z_2 \in S_{\widetilde z}$
, there exists a (unique, by [Reference Hoshi and Mochizuki8, Lem. 1.8])
![]() $\widetilde e \in \mathrm {Edge}(\widetilde {\mathcal {G}})$
such that
$\widetilde e \in \mathrm {Edge}(\widetilde {\mathcal {G}})$
such that
![]() $\mathcal {V}(\widetilde e)=\{\widetilde z_1,\widetilde z_2\}$
. But, in light of the definition of
$\mathcal {V}(\widetilde e)=\{\widetilde z_1,\widetilde z_2\}$
. But, in light of the definition of
![]() $S_\delta $
, this implies that
$S_\delta $
, this implies that
![]() $S_{\widetilde z}^\sharp =2$
, and hence that
$S_{\widetilde z}^\sharp =2$
, and hence that
![]() $\mathrm {Edge}(\widetilde {\mathcal {G}})$
contains an element fixed by the action of
$\mathrm {Edge}(\widetilde {\mathcal {G}})$
contains an element fixed by the action of
![]() $G_s$
—a contradiction! This completes the proof of assertion (iii) in the case where
$G_s$
—a contradiction! This completes the proof of assertion (iii) in the case where
![]() $\widetilde z \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
, hence also of assertion (iii). Assertion (iv) follows immediately from a similar argument to the argument applied in the proof of assertion (iii). This completes the proof of Lemma 1.11.
$\widetilde z \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
, hence also of assertion (iii). Assertion (iv) follows immediately from a similar argument to the argument applied in the proof of assertion (iii). This completes the proof of Lemma 1.11.
Lemma 1.12 (Triviality via passage to abelianizations).
Let G and J be profinite groups, and let
![]() $\phi \colon J\rightarrow G$
be a continuous homomorphism. Then the following hold:
$\phi \colon J\rightarrow G$
be a continuous homomorphism. Then the following hold:
-
(i) Let
 $\gamma \in G$
be such that, for every open subgroup
$\gamma \in G$
be such that, for every open subgroup
 $H\subseteq G$
of G that contains
$H\subseteq G$
of G that contains
 $\gamma $
, the image of
$\gamma $
, the image of
 $\gamma $
in
$\gamma $
in
 $H^{\mathrm {ab}}$
is trivial. Then
$H^{\mathrm {ab}}$
is trivial. Then
 $\gamma $
is trivial.
$\gamma $
is trivial. -
(ii) Suppose that, for every open subgroup
 $H\subseteq G$
of G, the composite
$H\subseteq G$
of G, the composite
 $\phi ^{-1}(H) \stackrel {\phi }{\rightarrow } H \twoheadrightarrow H^{\mathrm {ab}}$
is trivial. Then
$\phi ^{-1}(H) \stackrel {\phi }{\rightarrow } H \twoheadrightarrow H^{\mathrm {ab}}$
is trivial. Then
 $\phi $
is trivial.
$\phi $
is trivial.
Proof. First, we verify assertion (i). Assume that
![]() $\gamma $
is nontrivial. Then it is immediate that there exists a normal open subgroup
$\gamma $
is nontrivial. Then it is immediate that there exists a normal open subgroup
![]() $N\subseteq G$
of G such that
$N\subseteq G$
of G such that
![]() $\gamma \not \in N$
. Write
$\gamma \not \in N$
. Write
![]() $H \subseteq G$
for the closed subgroup of G topologically generated by N and
$H \subseteq G$
for the closed subgroup of G topologically generated by N and
![]() $\gamma $
. Then the image of
$\gamma $
. Then the image of
![]() $\gamma $
in the abelian quotient
$\gamma $
in the abelian quotient
![]() $H\twoheadrightarrow H/N$
is nontrivial. This completes the proof of assertion (i). Assertion (ii) follows immediately from assertion (i). This completes the proof of Lemma 1.12.
$H\twoheadrightarrow H/N$
is nontrivial. This completes the proof of assertion (i). Assertion (ii) follows immediately from assertion (i). This completes the proof of Lemma 1.12.
Theorem 1.13 (The combinatorial section conjecture for outer representations of ENN-type).
Let
![]() $\Sigma $
be a nonempty set of prime numbers, let
$\Sigma $
be a nonempty set of prime numbers, let
![]() $\mathcal {G}$
be a semi-graph of anabelioids of pro-
$\mathcal {G}$
be a semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type, let G be a profinite group, and let
$\Sigma $
PSC-type, let G be a profinite group, and let
![]() $\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism that is of ENN-type for a conducting subgroup
$\rho \colon G\rightarrow \mathrm {Aut}(\mathcal {G})$
be a continuous homomorphism that is of ENN-type for a conducting subgroup
![]() $I_G\subseteq G$
(cf. Definition 1.7(i)). Write
$I_G\subseteq G$
(cf. Definition 1.7(i)). Write
![]() $\Pi _{\mathcal {G}}$
for the (pro-
$\Pi _{\mathcal {G}}$
for the (pro-
![]() $\Sigma $
) fundamental group of
$\Sigma $
) fundamental group of
![]() $\mathcal {G}$
and
$\mathcal {G}$
and
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
for the tempered fundamental group of
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
for the tempered fundamental group of
![]() $\mathcal {G}$
(cf. [Reference Mochizuki17, Exam. 2.10]; the discussion preceding [Reference Mochizuki17, Prop. 3.6]). (Thus, we have a natural outer injection
$\mathcal {G}$
(cf. [Reference Mochizuki17, Exam. 2.10]; the discussion preceding [Reference Mochizuki17, Prop. 3.6]). (Thus, we have a natural outer injection
![]() $\Pi _{\mathcal {G}} ^{\mathrm {tp}} \hookrightarrow \Pi _{\mathcal {G}}$
—cf. [Reference Hoshi and Mochizuki11, Lem. 3.2(i)]; the proof of [Reference Hoshi and Mochizuki11, Prop. 3.3(i) and (ii)].) Write
$\Pi _{\mathcal {G}} ^{\mathrm {tp}} \hookrightarrow \Pi _{\mathcal {G}}$
—cf. [Reference Hoshi and Mochizuki11, Lem. 3.2(i)]; the proof of [Reference Hoshi and Mochizuki11, Prop. 3.3(i) and (ii)].) Write
![]() $\Pi _{G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } G$
(cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]);
$\Pi _{G} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}} \stackrel {\mathrm {out}}{\rtimes } G$
(cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]);
![]() $\Pi _{G}^{\mathrm {tp}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^{\mathrm {tp}}\stackrel {\mathrm {out}}{\rtimes } G$
;
$\Pi _{G}^{\mathrm {tp}} \stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^{\mathrm {tp}}\stackrel {\mathrm {out}}{\rtimes } G$
;
![]() $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
,
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
,
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow \mathcal {G}$
for the universal pro-
$\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow \mathcal {G}$
for the universal pro-
![]() $\Sigma $
and pro-tempered coverings of
$\Sigma $
and pro-tempered coverings of
![]() $\mathcal {G}$
corresponding to
$\mathcal {G}$
corresponding to
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
;
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
;
![]() $\mathrm {VCN}(-)$
for the set of vertices, cusps, and nodes of the underlying (pro-)semi-graph of a (pro-)semi-graph of anabelioids (cf. Definition 1.1(i)). Thus, we have a natural commutative diagram
$\mathrm {VCN}(-)$
for the set of vertices, cusps, and nodes of the underlying (pro-)semi-graph of a (pro-)semi-graph of anabelioids (cf. Definition 1.1(i)). Thus, we have a natural commutative diagram

—where the horizontal sequences are exact, the vertical arrows are outer injections,
![]() $\Pi _{G}^{\mathrm {tp}}$
acts naturally on
$\Pi _{G}^{\mathrm {tp}}$
acts naturally on
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}}$
, and
$\widetilde {\mathcal {G}}^{\mathrm {tp}}$
, and
![]() $\Pi _{G}$
acts naturally on
$\Pi _{G}$
acts naturally on
![]() $\widetilde {\mathcal {G}}$
. Then the following hold:
$\widetilde {\mathcal {G}}$
. Then the following hold:
-
(i) Suppose that
 $\rho $
is l-cyclotomically full (cf. Definition 1.7(ii)) for some
$\rho $
is l-cyclotomically full (cf. Definition 1.7(ii)) for some
 $l\in \Sigma $
. Let
$l\in \Sigma $
. Let
 $s \colon G \rightarrow \Pi _{G}$
be a continuous section of the natural surjection
$s \colon G \rightarrow \Pi _{G}$
be a continuous section of the natural surjection
 $\Pi _{G} \twoheadrightarrow G$
. Then, relative to the action of
$\Pi _{G} \twoheadrightarrow G$
. Then, relative to the action of
 $\Pi _{G}$
on
$\Pi _{G}$
on
 $\mathrm {VCN}(\widetilde {\mathcal {G}})$
via conjugation of VCN-subgroups, the image of s stabilizes some element of
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
via conjugation of VCN-subgroups, the image of s stabilizes some element of
 $\mathrm {VCN}(\widetilde {\mathcal {G}})$
.
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
. -
(ii) Let
 $s \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be a continuous section of the natural surjection
$s \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be a continuous section of the natural surjection
 $\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then, relative to the action of
$\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then, relative to the action of
 $\Pi _{G}^{\mathrm {tp}}$
on
$\Pi _{G}^{\mathrm {tp}}$
on
 $\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
via conjugation of VCN-subgroups (cf. Definition 1.9), the image of s stabilizes some element of
$\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
via conjugation of VCN-subgroups (cf. Definition 1.9), the image of s stabilizes some element of
 $\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
.
$\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
. -
(iii) Write
 $\mathrm {Sect}(\Pi _{G} / G)$
for the set of
$\mathrm {Sect}(\Pi _{G} / G)$
for the set of
 $\Pi _{\mathcal {G}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
$\Pi _{\mathcal {G}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
 $\Pi _{G} \twoheadrightarrow G$
and
$\Pi _{G} \twoheadrightarrow G$
and
 $\mathrm {Sect}(\Pi _{G}^{\mathrm {tp}} / G)$
for the set of
$\mathrm {Sect}(\Pi _{G}^{\mathrm {tp}} / G)$
for the set of
 $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
-conjugacy classes of continuous sections of the natural surjective homomorphism
 $\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then the natural map is injective. If, moreover,
$\Pi _{G}^{\mathrm {tp}} \twoheadrightarrow G$
. Then the natural map is injective. If, moreover, $$\begin{align*}\mathrm{Sect}(\Pi_{G}^{\mathrm{tp}} / G) \longrightarrow \mathrm{Sect}(\Pi_{G} / G) \end{align*}$$
$$\begin{align*}\mathrm{Sect}(\Pi_{G}^{\mathrm{tp}} / G) \longrightarrow \mathrm{Sect}(\Pi_{G} / G) \end{align*}$$
 $\rho $
is l-cyclotomically full for some
$\rho $
is l-cyclotomically full for some
 $l\in \Sigma $
, then this map is bijective.
$l\in \Sigma $
, then this map is bijective.
Proof. First, we verify assertion (i). Let us first observe that by replacing
![]() $I_G$
by a suitable open subgroup of
$I_G$
by a suitable open subgroup of
![]() $I_G$
and
$I_G$
and
![]() $\mathcal {G}$
by the pro-l completion of the finite étale covering of
$\mathcal {G}$
by the pro-l completion of the finite étale covering of
![]() $\mathcal {G}$
determined by a varying normal open subgroup
$\mathcal {G}$
determined by a varying normal open subgroup
![]() $H \subseteq \Pi _{G}$
such that
$H \subseteq \Pi _{G}$
such that
![]() $s(G) \subseteq H$
(cf. Lemma 1.8; [Reference Hoshi and Mochizuki11, Lem. 1.5]), it follows immediately from the well-known fact that a projective limit of nonempty finite sets is nonempty that we may assume without loss of generality that
$s(G) \subseteq H$
(cf. Lemma 1.8; [Reference Hoshi and Mochizuki11, Lem. 1.5]), it follows immediately from the well-known fact that a projective limit of nonempty finite sets is nonempty that we may assume without loss of generality that
![]() $\Sigma =\{l\}$
.
$\Sigma =\{l\}$
.
Next, let us observe that we may assume without loss of generality that
![]() $\mathcal {G}$
has at least one node. In particular, it follows immediately from Lemma 1.11(iii) that, to verify assertion (i), by replacing
$\mathcal {G}$
has at least one node. In particular, it follows immediately from Lemma 1.11(iii) that, to verify assertion (i), by replacing
![]() $\Pi _{G}$
by a suitable open subgroup of
$\Pi _{G}$
by a suitable open subgroup of
![]() $\Pi _{G}$
, we may assume without loss of generality—that is, by arguing as in the discussion entitled “Curves” in [Reference Mochizuki21, §0]—that the pro-l completion
$\Pi _{G}$
, we may assume without loss of generality—that is, by arguing as in the discussion entitled “Curves” in [Reference Mochizuki21, §0]—that the pro-l completion
![]() $\Pi _{\mathbb {G}}$
of the topological fundamental group of the underlying semi-graph
$\Pi _{\mathbb {G}}$
of the topological fundamental group of the underlying semi-graph
![]() $\mathbb {G}$
of
$\mathbb {G}$
of
![]() $\mathcal {G}$
is a free pro-l group of rank
$\mathcal {G}$
is a free pro-l group of rank
![]() $\geq 2$
, hence, in particular, center-free.
$\geq 2$
, hence, in particular, center-free.
Then we claim that the following assertion holds:
Claim 1.13.A: For every connected finite étale Galois subcovering
![]() $\mathcal {H} \rightarrow \mathcal {G}$
of
$\mathcal {H} \rightarrow \mathcal {G}$
of
![]() $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
that determines a normal open subgroup of
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
that determines a normal open subgroup of
![]() $\Pi _{G}$
, the action of
$\Pi _{G}$
, the action of
![]() $I_G$
on
$I_G$
on
![]() $\mathcal {H}$
, via s, fixes an element of
$\mathcal {H}$
, via s, fixes an element of
![]() $\mathrm {VCN}(\mathcal {H})$
.
$\mathrm {VCN}(\mathcal {H})$
.
To verify Claim 1.13.A, let us observe that, by replacing
![]() $\mathcal {H}$
by
$\mathcal {H}$
by
![]() $\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki11, Lem. 1.5]), we may assume without loss of generality that
$\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki11, Lem. 1.5]), we may assume without loss of generality that
![]() $\mathcal {H} = \mathcal {G}$
. Next, let us observe that since the underlying semi-graph
$\mathcal {H} = \mathcal {G}$
. Next, let us observe that since the underlying semi-graph
![]() $\mathbb {G}$
of
$\mathbb {G}$
of
![]() $\mathcal {G}$
is finite, the continuous action of G on
$\mathcal {G}$
is finite, the continuous action of G on
![]() $\mathbb {G}$
factors through a finite quotient
$\mathbb {G}$
factors through a finite quotient
![]() $G \twoheadrightarrow Q$
, that is, by a normal open subgroup of G. Write
$G \twoheadrightarrow Q$
, that is, by a normal open subgroup of G. Write
![]() $\Pi _{\mathbb {G} // Q} \stackrel {\mathrm {def}}{=} \Pi _{\mathbb {G}} \stackrel {\mathrm {out}}{\rtimes } Q$
(i.e., notation which is well-defined since
$\Pi _{\mathbb {G} // Q} \stackrel {\mathrm {def}}{=} \Pi _{\mathbb {G}} \stackrel {\mathrm {out}}{\rtimes } Q$
(i.e., notation which is well-defined since
![]() $\Pi _{\mathbb {G}}$
is center-free—cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]; the notational conventions of Lemma 1.6, in the case where “
$\Pi _{\mathbb {G}}$
is center-free—cf. the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]; the notational conventions of Lemma 1.6, in the case where “
![]() $\Sigma $
” is taken to be
$\Sigma $
” is taken to be
![]() $\{l\}$
). Thus, we obtain a commutative diagram
$\{l\}$
). Thus, we obtain a commutative diagram

—where the horizontal sequences are exact, and the vertical arrows are surjective. Write
![]() ${I_G \twoheadrightarrow I_Q}$
for the (finite) quotient of
${I_G \twoheadrightarrow I_Q}$
for the (finite) quotient of
![]() $I_G$
determined by the quotient
$I_G$
determined by the quotient
![]() $G\twoheadrightarrow Q$
,
$G\twoheadrightarrow Q$
,
![]() $N_G \stackrel {\mathrm {def}}{=} \mathrm {Ker}(G\twoheadrightarrow Q)$
, and
$N_G \stackrel {\mathrm {def}}{=} \mathrm {Ker}(G\twoheadrightarrow Q)$
, and
![]() $N_I \stackrel {\mathrm {def}}{=} \mathrm {Ker}(I_G\twoheadrightarrow I_Q)$
. Now let us observe that
$N_I \stackrel {\mathrm {def}}{=} \mathrm {Ker}(I_G\twoheadrightarrow I_Q)$
. Now let us observe that
-
(a) since Q is finite, it is immediate that
 $N_G$
,
$N_G$
,
 $N_I$
are open in G,
$N_I$
are open in G,
 $I_G$
, respectively, and, moreover,
$I_G$
, respectively, and, moreover, -
(b) it follows from the definition of the term “ENN-type” that, by replacing
 $G\twoheadrightarrow Q$
by a suitable quotient of Q if necessary, we may assume without loss of generality that the quotient
$G\twoheadrightarrow Q$
by a suitable quotient of Q if necessary, we may assume without loss of generality that the quotient
 $I_G \twoheadrightarrow I_Q$
factors through the quotient
$I_G \twoheadrightarrow I_Q$
factors through the quotient
 $I_G\twoheadrightarrow I_G^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}}$
(cf. the discussion entitled “Groups” in §0), hence is cyclic of order a power of l.
$I_G\twoheadrightarrow I_G^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}}$
(cf. the discussion entitled “Groups” in §0), hence is cyclic of order a power of l.
Next, let us observe that the composite
![]() $N_G \hookrightarrow G\stackrel {s}{\rightarrow } \Pi _{G} \twoheadrightarrow \Pi _{\mathbb {G}// Q}$
determines a commutative diagram
$N_G \hookrightarrow G\stackrel {s}{\rightarrow } \Pi _{G} \twoheadrightarrow \Pi _{\mathbb {G}// Q}$
determines a commutative diagram

—where the upper horizontal arrow is the natural inclusion. Now we claim that the following assertion holds:
Claim 1.13.B: The left-hand vertical arrow
![]() $N_I \rightarrow \Pi _{\mathbb {G}}$
of the above diagram is the trivial homomorphism.
$N_I \rightarrow \Pi _{\mathbb {G}}$
of the above diagram is the trivial homomorphism.
Indeed, let
![]() $H \subseteq \Pi _{\mathbb {G}}$
be an open subgroup and write
$H \subseteq \Pi _{\mathbb {G}}$
be an open subgroup and write
![]() $N_{I,H} \subseteq N_I$
and
$N_{I,H} \subseteq N_I$
and
![]() $N_{G,H} \subseteq N_G$
for the open subgroups obtained by forming the inverse image of
$N_{G,H} \subseteq N_G$
for the open subgroups obtained by forming the inverse image of
![]() $H \subseteq \Pi _{\mathbb {G}}$
via the vertical arrows of the above commutative diagram. Thus,
$H \subseteq \Pi _{\mathbb {G}}$
via the vertical arrows of the above commutative diagram. Thus,
![]() $N_{G,H}$
normalizes
$N_{G,H}$
normalizes
![]() $N_{I,H}$
; the action of
$N_{I,H}$
; the action of
![]() $N_{G,H}$
on H by conjugation induces the trivial action of
$N_{G,H}$
on H by conjugation induces the trivial action of
![]() $N_{G,H}$
on
$N_{G,H}$
on
![]() $H^{\mathrm {ab}}$
. Next, let us observe that since
$H^{\mathrm {ab}}$
. Next, let us observe that since
![]() $H^{\mathrm {ab}}$
is a free
$H^{\mathrm {ab}}$
is a free
![]() $\mathbb {Z}_l$
-module, the left-hand vertical arrow under consideration determines a homomorphism
$\mathbb {Z}_l$
-module, the left-hand vertical arrow under consideration determines a homomorphism
![]() $N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow H^{\mathrm {ab}}$
of free
$N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow H^{\mathrm {ab}}$
of free
![]() $\mathbb {Z}_l$
-modules of finite rank (cf. Definition 1.7(i)), which is
$\mathbb {Z}_l$
-modules of finite rank (cf. Definition 1.7(i)), which is
![]() $N_{G,H}$
-equivariant (with respect to the actions of
$N_{G,H}$
-equivariant (with respect to the actions of
![]() $N_{G,H}$
by conjugation). On the other hand, since the action of
$N_{G,H}$
by conjugation). On the other hand, since the action of
![]() $N_{G,H}$
on
$N_{G,H}$
on
![]() $H^{\mathrm {ab}}$
is trivial, the
$H^{\mathrm {ab}}$
is trivial, the
![]() $N_{G,H}$
-equivariant homomorphism
$N_{G,H}$
-equivariant homomorphism
![]() $N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow H^{\mathrm {ab}}$
factors through a quotient of
$N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow H^{\mathrm {ab}}$
factors through a quotient of
![]() $N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}}$
on which
$N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}}$
on which
![]() $N_{G,H}$
acts trivially. Thus, since
$N_{G,H}$
acts trivially. Thus, since
![]() $\rho $
is l-cyclotomically full, and
$\rho $
is l-cyclotomically full, and
![]() $N_{G,H}$
acts on
$N_{G,H}$
acts on
![]() $N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}}$
via the cyclotomic character (cf. Definition 1.7(i); [Reference Hoshi and Mochizuki9, Lem. 5.2(ii)]), we conclude that the
$N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}}$
via the cyclotomic character (cf. Definition 1.7(i); [Reference Hoshi and Mochizuki9, Lem. 5.2(ii)]), we conclude that the
![]() $N_{G,H}$
-equivariant homomorphism
$N_{G,H}$
-equivariant homomorphism
![]() $N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow H^{\mathrm {ab}}$
is trivial. In particular, Claim 1.13.B follows from Lemma 1.12(ii). This completes the proof of Claim 1.13.B.
$N_{I,H}^{\{l\}\text {-}\mathrm {ab}\text {-}\mathrm {free}} \rightarrow H^{\mathrm {ab}}$
is trivial. In particular, Claim 1.13.B follows from Lemma 1.12(ii). This completes the proof of Claim 1.13.B.
Next, let us observe that it follows immediately from Claim 1.13.B that the section s determines a section of the natural surjection
Thus, it follows immediately from the resp’d portion of Lemma 1.6(iv) together with the observation (b) discussed above (cf. also Remark 1.13.1 below), that Claim 1.13.A holds. This completes the proof of Claim 1.13.A.
Now, by allowing the subcovering
![]() $\mathcal {H}$
in Claim 1.13.A to vary, we conclude immediately (from the well-known fact that a projective limit of nonempty finite sets is nonempty) that
$\mathcal {H}$
in Claim 1.13.A to vary, we conclude immediately (from the well-known fact that a projective limit of nonempty finite sets is nonempty) that
![]() $s(I_G)$
stabilizes some element of
$s(I_G)$
stabilizes some element of
![]() $\mathrm {VCN}(\widetilde {\mathcal {G}})$
. Thus, it follows from Lemma 1.11(iii) that the image
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
. Thus, it follows from Lemma 1.11(iii) that the image
![]() $s(G)$
stabilizes some element of
$s(G)$
stabilizes some element of
![]() $\mathrm {VCN}(\widetilde {\mathcal {G}})$
. This completes the proof of assertion (i).
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
. This completes the proof of assertion (i).
Assertion (ii) follows, by applying [Reference Hoshi and Mochizuki8, Prop. 3.9(i)], from a similar argument to the argument applied to prove [Reference Mochizuki17, Ths. 3.7 and 5.4]. That is to say, instead of considering “subjoints” (i.e., paths of length
![]() $2$
) as in the proof of [Reference Mochizuki17, Th. 3.7], the content of [Reference Hoshi and Mochizuki8, Prop. 3.9(i)] requires us to consider paths of length 3. This completes the proof of assertion (ii).
$2$
) as in the proof of [Reference Mochizuki17, Th. 3.7], the content of [Reference Hoshi and Mochizuki8, Prop. 3.9(i)] requires us to consider paths of length 3. This completes the proof of assertion (ii).
Finally, we verify assertion (iii). Let s,
![]() $t \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be sections of the surjection
$t \colon G\rightarrow \Pi _{G}^{\mathrm {tp}}$
be sections of the surjection
![]() $\Pi _{G}^{\mathrm {tp}}\twoheadrightarrow G$
such that there exists an element
$\Pi _{G}^{\mathrm {tp}}\twoheadrightarrow G$
such that there exists an element
![]() $\gamma \in \Pi _{\mathcal {G}}$
such that the composite
$\gamma \in \Pi _{\mathcal {G}}$
such that the composite
![]() $\widehat s \colon G\stackrel {s}{\rightarrow }\Pi _{G}^{\mathrm {tp}} \hookrightarrow \Pi _{G}$
is the conjugate by
$\widehat s \colon G\stackrel {s}{\rightarrow }\Pi _{G}^{\mathrm {tp}} \hookrightarrow \Pi _{G}$
is the conjugate by
![]() $\gamma \in \Pi _{\mathcal {G}}$
of the composite
$\gamma \in \Pi _{\mathcal {G}}$
of the composite
![]() $\widehat t \colon G\stackrel {t}{\rightarrow }\Pi _{G}^{\mathrm {tp}} \hookrightarrow \Pi _{G}$
. Thus, it follows from assertion (ii) (applied to both s and t) that there exist elements
$\widehat t \colon G\stackrel {t}{\rightarrow }\Pi _{G}^{\mathrm {tp}} \hookrightarrow \Pi _{G}$
. Thus, it follows from assertion (ii) (applied to both s and t) that there exist elements
![]() $\widetilde y$
,
$\widetilde y$
,
![]() $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
such that if we write
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
such that if we write
![]() $\widetilde z^\gamma \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
for the image of
$\widetilde z^\gamma \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
for the image of
![]() $\widetilde z$
by the action of
$\widetilde z$
by the action of
![]() $\gamma $
, then
$\gamma $
, then
![]() $\widehat s$
stabilizes both
$\widehat s$
stabilizes both
![]() $\widetilde y$
and
$\widetilde y$
and
![]() $\widetilde z^\gamma $
. In particular, we conclude from Lemma 1.11(ii) that the distance between
$\widetilde z^\gamma $
. In particular, we conclude from Lemma 1.11(ii) that the distance between
![]() $\widetilde y$
and
$\widetilde y$
and
![]() $\widetilde z^\gamma $
is finite, hence that, for each subcovering
$\widetilde z^\gamma $
is finite, hence that, for each subcovering
![]() $\mathcal {H} \rightarrow \mathcal {G}$
of
$\mathcal {H} \rightarrow \mathcal {G}$
of
![]() $\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow \mathcal {G}$
that arises from an open subgroup of
$\widetilde {\mathcal {G}}^{\mathrm {tp}} \rightarrow \mathcal {G}$
that arises from an open subgroup of
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
, the distance between the images of
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
, the distance between the images of
![]() $\widetilde z$
and
$\widetilde z$
and
![]() $\widetilde z^\gamma $
in
$\widetilde z^\gamma $
in
![]() $\mathcal {H}$
is finite, which implies that
$\mathcal {H}$
is finite, which implies that
![]() $\gamma \in \Pi _{\mathcal {G}}^{\mathrm {tp}}$
. This completes the proof of the injectivity portion of assertion (iii). Since (one verifies immediately that) every element of
$\gamma \in \Pi _{\mathcal {G}}^{\mathrm {tp}}$
. This completes the proof of the injectivity portion of assertion (iii). Since (one verifies immediately that) every element of
![]() $\mathrm {VCN}(\widetilde {\mathcal {G}})$
lies in the
$\mathrm {VCN}(\widetilde {\mathcal {G}})$
lies in the
![]() $\Pi _{\mathcal {G}}$
-orbit of an element of
$\Pi _{\mathcal {G}}$
-orbit of an element of
![]() $\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
, the final portion of assertion (iii) follows immediately from assertion (i). This completes the proof of Theorem 1.13.
$\mathrm {VCN}(\widetilde {\mathcal {G}}^{\mathrm {tp}})$
, the final portion of assertion (iii) follows immediately from assertion (i). This completes the proof of Theorem 1.13.
Remark 1.13.1. We observe in passing, with regard to the application of Lemma 1.6(iv) in the proof of Theorem 1.13(i) that, in fact, Lemma 1.6(iv) is only applied in the case where the group “G” of Lemma 1.6 is cyclic and of order a power of l. That is to say, we only apply Lemma 1.6(iv) in the case that, as discussed in Remark 1.6.2(i) admits a relatively simple proof.
Corollary 1.14 (A combinatorial version of the Grothendieck conjecture for outer representations of ENN-type).
Let
![]() $\Sigma $
be a nonempty set of prime numbers;
$\Sigma $
be a nonempty set of prime numbers;
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
semi-graphs of anabelioids of pro-
$\mathcal {H}$
semi-graphs of anabelioids of pro-
![]() $\Sigma $
PSC-type;
$\Sigma $
PSC-type;
![]() $G_{\mathcal {G}}$
,
$G_{\mathcal {G}}$
,
![]() $G_{\mathcal {H}}$
profinite groups;
$G_{\mathcal {H}}$
profinite groups;
![]() $\beta \colon G_{\mathcal {G}} \stackrel {\sim }{\rightarrow } G_{\mathcal {H}}$
a continuous isomorphism;
$\beta \colon G_{\mathcal {G}} \stackrel {\sim }{\rightarrow } G_{\mathcal {H}}$
a continuous isomorphism;
![]() $\rho _{\mathcal {G}}\colon G_{\mathcal {G}}\rightarrow \mathrm {Aut}(\mathcal {G})$
,
$\rho _{\mathcal {G}}\colon G_{\mathcal {G}}\rightarrow \mathrm {Aut}(\mathcal {G})$
,
![]() $\rho _{\mathcal {H}}\colon G_{\mathcal {H}}\rightarrow \mathrm {Aut}(\mathcal {H})$
continuous homomorphisms that are of ENN-type for conducting subgroups
$\rho _{\mathcal {H}}\colon G_{\mathcal {H}}\rightarrow \mathrm {Aut}(\mathcal {H})$
continuous homomorphisms that are of ENN-type for conducting subgroups
![]() $I_{G_{\mathcal {G}}} \subseteq G_{\mathcal {G}}$
,
$I_{G_{\mathcal {G}}} \subseteq G_{\mathcal {G}}$
,
![]() $I_{G_{\mathcal {H}}} \subseteq G_{\mathcal {H}}$
(cf. Definition 1.7(i)) such that
$I_{G_{\mathcal {H}}} \subseteq G_{\mathcal {H}}$
(cf. Definition 1.7(i)) such that
![]() $\beta (I_{G_{\mathcal {G}}})=I_{G_{\mathcal {H}}}$
;
$\beta (I_{G_{\mathcal {G}}})=I_{G_{\mathcal {H}}}$
;
![]() $l\in \Sigma $
a prime number such that
$l\in \Sigma $
a prime number such that
![]() $\rho _{\mathcal {G}}$
and
$\rho _{\mathcal {G}}$
and
![]() $\rho _{\mathcal {H}}$
are l-cyclotomically full (cf. Definition 1.7(ii)). Suppose further that
$\rho _{\mathcal {H}}$
are l-cyclotomically full (cf. Definition 1.7(ii)). Suppose further that
![]() $\rho _{\mathcal {G}}$
is verticially quasi-split (cf. Definition 1.7(i)). Write
$\rho _{\mathcal {G}}$
is verticially quasi-split (cf. Definition 1.7(i)). Write
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {H}}$
for the (pro-
$\Pi _{\mathcal {H}}$
for the (pro-
![]() $\Sigma $
) fundamental groups of
$\Sigma $
) fundamental groups of
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
, respectively. Let
$\mathcal {H}$
, respectively. Let
![]() $\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
be a continuous isomorphism such that the diagram
$\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
be a continuous isomorphism such that the diagram

—where the right-hand vertical arrow is the isomorphism obtained by conjugating by
![]() $\alpha $
—commutes. Then
$\alpha $
—commutes. Then
![]() $\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
$\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
Proof. First, let us observe that by [Reference Mochizuki18, Cor. 2.7(i)], it follows from our assumption that
![]() $\rho _{\mathcal {G}}$
and
$\rho _{\mathcal {G}}$
and
![]() $\rho _{\mathcal {H}}$
are l-cyclotomically full that
$\rho _{\mathcal {H}}$
are l-cyclotomically full that
![]() $\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is group-theoretically cuspidal. Thus, by applying [Reference Mochizuki18, Prop. 1.5(ii)] and [Reference Hoshi and Mochizuki8, Lem. 1.14], we conclude that it suffices to verify that
$\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is group-theoretically cuspidal. Thus, by applying [Reference Mochizuki18, Prop. 1.5(ii)] and [Reference Hoshi and Mochizuki8, Lem. 1.14], we conclude that it suffices to verify that
![]() $\alpha $
is group-theoretically verticial under the additional assumption that
$\alpha $
is group-theoretically verticial under the additional assumption that
![]() $\mathcal {G}$
and
$\mathcal {G}$
and
![]() $\mathcal {H}$
are noncuspidal. Write
$\mathcal {H}$
are noncuspidal. Write
![]() $\Pi _{G_{\mathcal {G}}}$
,
$\Pi _{G_{\mathcal {G}}}$
,
![]() $\Pi _{G_{\mathcal {H}}}$
for the profinite groups “
$\Pi _{G_{\mathcal {H}}}$
for the profinite groups “
![]() $\Pi _G$
” (cf. Theorem 1.13) associated with
$\Pi _G$
” (cf. Theorem 1.13) associated with
![]() $\rho _{\mathcal {G}}$
,
$\rho _{\mathcal {G}}$
,
![]() $\rho _{\mathcal {H}}$
. Then it follows immediately from our assumption that
$\rho _{\mathcal {H}}$
. Then it follows immediately from our assumption that
![]() $\rho _{\mathcal {G}}$
is verticially quasi-split that we may assume, after possibly replacing
$\rho _{\mathcal {G}}$
is verticially quasi-split that we may assume, after possibly replacing
![]() $G_{\mathcal {G}}$
and
$G_{\mathcal {G}}$
and
![]() $G_{\mathcal {H}}$
by corresponding open subgroups, that there exists a section
$G_{\mathcal {H}}$
by corresponding open subgroups, that there exists a section
![]() $s_{\mathcal {G}}\colon G_{\mathcal {G}} \rightarrow \Pi _{G_{\mathcal {G}}}$
such that the image of the restriction of
$s_{\mathcal {G}}\colon G_{\mathcal {G}} \rightarrow \Pi _{G_{\mathcal {G}}}$
such that the image of the restriction of
![]() $s_{\mathcal {G}}$
to
$s_{\mathcal {G}}$
to
![]() $I_{G_{\mathcal {G}}}$
commutes with some verticial subgroup of
$I_{G_{\mathcal {G}}}$
commutes with some verticial subgroup of
![]() $\Pi _{\mathcal {G}}$
. In particular,
$\Pi _{\mathcal {G}}$
. In particular,
![]() $s_{\mathcal {G}}$
satisfies the conditions imposed on the section “
$s_{\mathcal {G}}$
satisfies the conditions imposed on the section “
![]() $s\colon G \rightarrow \Pi _G$
” in Lemma 1.11(ii), for some nonempty subset “S.” Moreover, it follows from Theorem 1.13(i) that the isomorphism
$s\colon G \rightarrow \Pi _G$
” in Lemma 1.11(ii), for some nonempty subset “S.” Moreover, it follows from Theorem 1.13(i) that the isomorphism
![]() $\Pi _{G_{\mathcal {G}}} \stackrel {\sim }{\rightarrow } \Pi _{G_{\mathcal {H}}}$
determined by
$\Pi _{G_{\mathcal {G}}} \stackrel {\sim }{\rightarrow } \Pi _{G_{\mathcal {H}}}$
determined by
![]() $\alpha $
and
$\alpha $
and
![]() $\beta $
maps
$\beta $
maps
![]() $s_{\mathcal {G}}$
to a section
$s_{\mathcal {G}}$
to a section
![]() $s_{\mathcal {H}}\colon G_{\mathcal {H}} \rightarrow \Pi _{G_{\mathcal {H}}}$
that is contained in the normalizer in
$s_{\mathcal {H}}\colon G_{\mathcal {H}} \rightarrow \Pi _{G_{\mathcal {H}}}$
that is contained in the normalizer in
![]() $\Pi _{G_{\mathcal {H}}}$
of a VCN-subgroup of
$\Pi _{G_{\mathcal {H}}}$
of a VCN-subgroup of
![]() $\Pi _{\mathcal {H}}$
. In particular, after possibly replacing
$\Pi _{\mathcal {H}}$
. In particular, after possibly replacing
![]() $G_{\mathcal {G}}$
and
$G_{\mathcal {G}}$
and
![]() $G_{\mathcal {H}}$
by corresponding open subgroups, we may assume (cf. [Reference Mochizuki18, Prop 1.2(ii)] and [Reference Hoshi and Mochizuki8, Rem. 2.7.1]) that the image of the restriction of
$G_{\mathcal {H}}$
by corresponding open subgroups, we may assume (cf. [Reference Mochizuki18, Prop 1.2(ii)] and [Reference Hoshi and Mochizuki8, Rem. 2.7.1]) that the image of the restriction of
![]() $s_{\mathcal {H}}$
to
$s_{\mathcal {H}}$
to
![]() $I_{G_{\mathcal {H}}}$
commutes with some nontrivial verticial element of
$I_{G_{\mathcal {H}}}$
commutes with some nontrivial verticial element of
![]() $\Pi _{\mathcal {H}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 1.1]). Thus, by restricting these sections
$\Pi _{\mathcal {H}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 1.1]). Thus, by restricting these sections
![]() $s_{\mathcal {G}}$
,
$s_{\mathcal {G}}$
,
![]() $s_{\mathcal {H}}$
to the respective conducting subgroups and forming appropriate centralizers [cf. [Reference Hoshi and Mochizuki8, Lem. 3.6(i)], applied to the restriction of
$s_{\mathcal {H}}$
to the respective conducting subgroups and forming appropriate centralizers [cf. [Reference Hoshi and Mochizuki8, Lem. 3.6(i)], applied to the restriction of
![]() $s_{\mathcal {G}}$
to
$s_{\mathcal {G}}$
to
![]() $I_{G_{\mathcal {G}}}$
], we conclude from the assumption that
$I_{G_{\mathcal {G}}}$
], we conclude from the assumption that
![]() $\beta $
is compatible with the respective conducting subgroups that
$\beta $
is compatible with the respective conducting subgroups that
![]() $\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
maps some nontrivial verticial element of
$\alpha \colon \Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
maps some nontrivial verticial element of
![]() $\Pi _{\mathcal {G}}$
to a nontrivial verticial element of
$\Pi _{\mathcal {G}}$
to a nontrivial verticial element of
![]() $\Pi _{\mathcal {H}}$
. In particular, it follows from the implication (3)
$\Pi _{\mathcal {H}}$
. In particular, it follows from the implication (3)
![]() $\Rightarrow $
(1) of [Reference Hoshi and Mochizuki10, Th. 1.9(i)] that
$\Rightarrow $
(1) of [Reference Hoshi and Mochizuki10, Th. 1.9(i)] that
![]() $\alpha $
is group-theoretically verticial, as desired.
$\alpha $
is group-theoretically verticial, as desired.
Remark 1.14.1. It is not difficult to verify that the assumption in the statement of Corollary 1.14 that
![]() $\beta (I_{G_{\mathcal {G}}})=I_{G_{\mathcal {H}}}$
cannot be omitted. Indeed, if one omits this assumption, then a counterexample to the graphicity asserted in Corollary 1.14 may be obtained as follows: let
$\beta (I_{G_{\mathcal {G}}})=I_{G_{\mathcal {H}}}$
cannot be omitted. Indeed, if one omits this assumption, then a counterexample to the graphicity asserted in Corollary 1.14 may be obtained as follows: let
![]() $\mathcal {J}$
be a semi-graph of anabelioids of pro-
$\mathcal {J}$
be a semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type and
$\Sigma $
PSC-type and
![]() $e_{\mathcal {G}}$
,
$e_{\mathcal {G}}$
,
![]() $e_{\mathcal {H}}$
distinct nodes of
$e_{\mathcal {H}}$
distinct nodes of
![]() $\mathcal {J}$
. Write
$\mathcal {J}$
. Write
![]() $\mathcal {G}$
(resp.
$\mathcal {G}$
(resp.
![]() $\mathcal {H}$
) for the semi-graph of anabelioids of pro-
$\mathcal {H}$
) for the semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type
$\Sigma $
PSC-type
![]() $\mathcal {J}_{\leadsto {{\mathrm {Node}}(\mathcal {J})\setminus \{e_{\mathcal {G}}\}}}$
(resp.
$\mathcal {J}_{\leadsto {{\mathrm {Node}}(\mathcal {J})\setminus \{e_{\mathcal {G}}\}}}$
(resp.
![]() $\mathcal {J}_{\leadsto {{\mathrm {Node}}(\mathcal {J})\setminus \{e_{\mathcal {H}}\}}}$
) obtained by deforming the nodes of
$\mathcal {J}_{\leadsto {{\mathrm {Node}}(\mathcal {J})\setminus \{e_{\mathcal {H}}\}}}$
) obtained by deforming the nodes of
![]() $\mathcal {J}$
that are
$\mathcal {J}$
that are
![]() $\neq e_{\mathcal {G}}$
(resp.
$\neq e_{\mathcal {G}}$
(resp.
![]() $\neq e_{\mathcal {H}}$
) (cf. [Reference Hoshi and Mochizuki9, Def. 2.8]);
$\neq e_{\mathcal {H}}$
) (cf. [Reference Hoshi and Mochizuki9, Def. 2.8]);
![]() $I_{G_{\mathcal {G}}}$
(resp.
$I_{G_{\mathcal {G}}}$
(resp.
![]() $I_{G_{\mathcal {H}}}$
) for the (necessarily normal—cf. [Reference Hoshi and Mochizuki9, Th. 4.8(i) and (v)]) closed subgroup of
$I_{G_{\mathcal {H}}}$
) for the (necessarily normal—cf. [Reference Hoshi and Mochizuki9, Th. 4.8(i) and (v)]) closed subgroup of
![]() $\mathrm {Aut}^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|}(\mathcal {J})$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.6(i)]) generated by the profinite Dehn twists that arise from the direct summand of the direct sum decomposition in the display of [Reference Hoshi and Mochizuki9, Th. 4.8(iv)], labeled by
$\mathrm {Aut}^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|}(\mathcal {J})$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.6(i)]) generated by the profinite Dehn twists that arise from the direct summand of the direct sum decomposition in the display of [Reference Hoshi and Mochizuki9, Th. 4.8(iv)], labeled by
![]() $e_{\mathcal {G}}$
(resp.
$e_{\mathcal {G}}$
(resp.
![]() $e_{\mathcal {H}}$
). Next, let
$e_{\mathcal {H}}$
). Next, let
![]() $G_{\mathcal {G}} = G_{\mathcal {H}}$
be a closed subgroup of
$G_{\mathcal {G}} = G_{\mathcal {H}}$
be a closed subgroup of
![]() $\mathrm {Aut}^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|}(\mathcal {J})$
such that:
$\mathrm {Aut}^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|}(\mathcal {J})$
such that:
-
•
 $G_{\mathcal {G}} = G_{\mathcal {H}}$
contains both
$G_{\mathcal {G}} = G_{\mathcal {H}}$
contains both
 $I_{G_{\mathcal {G}}}$
and
$I_{G_{\mathcal {G}}}$
and
 $I_{G_{\mathcal {H}}}$
,
$I_{G_{\mathcal {H}}}$
, -
• the natural inclusion
 $G_{\mathcal {G}} = G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} (\mathcal {J}) $
is l-cyclotomically full for some
$G_{\mathcal {G}} = G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} (\mathcal {J}) $
is l-cyclotomically full for some
 $l \in \Sigma $
, and, moreover,
$l \in \Sigma $
, and, moreover, -
• if we write
 $\rho _{\mathcal {G}}$
(resp.
$\rho _{\mathcal {G}}$
(resp.
 $\rho _{\mathcal {H}}$
) for the continuous injection
$\rho _{\mathcal {H}}$
) for the continuous injection
 $G_{\mathcal {G}} \hookrightarrow \mathrm {Aut}(\mathcal {G})$
(resp.
$G_{\mathcal {G}} \hookrightarrow \mathrm {Aut}(\mathcal {G})$
(resp.
 $G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} (\mathcal {H}) $
) obtained by forming the composite of the natural inclusion
$G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} (\mathcal {H}) $
) obtained by forming the composite of the natural inclusion
 $ G_{\mathcal {G}} = G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} ^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|} (\mathcal {J}) $
and the injection
$ G_{\mathcal {G}} = G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} ^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|} (\mathcal {J}) $
and the injection
 $\mathrm {Aut} ^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|} (\mathcal {J}) \hookrightarrow \mathrm {Aut}( \mathcal {G} ) $
(resp.
$\mathrm {Aut} ^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|} (\mathcal {J}) \hookrightarrow \mathrm {Aut}( \mathcal {G} ) $
(resp.
 $\mathrm {Aut} ^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|} (\mathcal {J}) \hookrightarrow \mathrm {Aut}( \mathcal {H} ) $
) (cf. [Reference Hoshi and Mochizuki9, Prop. 2.9(ii)]), then
$\mathrm {Aut} ^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|} (\mathcal {J}) \hookrightarrow \mathrm {Aut}( \mathcal {H} ) $
) (cf. [Reference Hoshi and Mochizuki9, Prop. 2.9(ii)]), then
 $\rho _{\mathcal {G}}$
is verticially quasi-split.
$\rho _{\mathcal {G}}$
is verticially quasi-split.
(Note that one verifies easily the existence of such a closed subgroup of
![]() $\mathrm {Aut}^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|}(\mathcal {J})$
by considering, for instance, a homomorphism
$\mathrm {Aut}^{|\{e_{\mathcal {G}},e_{\mathcal {H}}\}|}(\mathcal {J})$
by considering, for instance, a homomorphism
![]() $G_{\mathcal {G}} = G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} (\mathcal {J})$
of EPIPSC-type that arises from a suitable stable log curve—cf. also Remark 1.7.1, [Reference Hoshi and Mochizuki9, Lem. 5.4(ii)], and [Reference Hoshi and Mochizuki9, Prop. 5.6(ii)].) Then if one takes the “
$G_{\mathcal {G}} = G_{\mathcal {H}} \hookrightarrow \mathrm {Aut} (\mathcal {J})$
of EPIPSC-type that arises from a suitable stable log curve—cf. also Remark 1.7.1, [Reference Hoshi and Mochizuki9, Lem. 5.4(ii)], and [Reference Hoshi and Mochizuki9, Prop. 5.6(ii)].) Then if one takes the “
![]() $\alpha $
” of Corollary 1.14 to be the outer isomorphism determined by the specialization outer isomorphisms
$\alpha $
” of Corollary 1.14 to be the outer isomorphism determined by the specialization outer isomorphisms
![]() $\Phi _{\mathcal {J}_{\leadsto {\mathrm {Node}}}(\mathcal {J})\setminus \{e_{\mathcal {G}}\}}$
,
$\Phi _{\mathcal {J}_{\leadsto {\mathrm {Node}}}(\mathcal {J})\setminus \{e_{\mathcal {G}}\}}$
,
![]() $\Phi _{\mathcal {J}_{\leadsto {\mathrm {Node}}}(\mathcal {J})\setminus \{e_{\mathcal {H}}\}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]) and the “
$\Phi _{\mathcal {J}_{\leadsto {\mathrm {Node}}}(\mathcal {J})\setminus \{e_{\mathcal {H}}\}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]) and the “
![]() $\beta $
” of Corollary 1.14 to be the identity isomorphism, then one verifies immediately from [Reference Hoshi and Mochizuki9, Cor. 3.9(i)] and [Reference Hoshi and Mochizuki9, Cor. 5.9(iii)] that one obtains a counterexample as desired.
$\beta $
” of Corollary 1.14 to be the identity isomorphism, then one verifies immediately from [Reference Hoshi and Mochizuki9, Cor. 3.9(i)] and [Reference Hoshi and Mochizuki9, Cor. 5.9(iii)] that one obtains a counterexample as desired.
Let R be a complete discrete valuation ring whose residue characteristic we denote by p (so p may be zero);
![]() $\overline {K}$
a separable closure of the field of fractions K of R;
$\overline {K}$
a separable closure of the field of fractions K of R;
a stable log curve (cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]) over the log regular log scheme
![]() $\mathrm {Spec}(R)^{\mathrm {log}}$
obtained by equipping
$\mathrm {Spec}(R)^{\mathrm {log}}$
obtained by equipping
![]() $\mathrm {Spec}(R)$
with the log structure determined by the maximal ideal
$\mathrm {Spec}(R)$
with the log structure determined by the maximal ideal
![]() $\mathfrak {m}_R \subseteq R$
of R. Suppose, for simplicity, that
$\mathfrak {m}_R \subseteq R$
of R. Suppose, for simplicity, that
![]() $\mathcal {X}^{\mathrm {log}}$
is split, that is, that the natural action of
$\mathcal {X}^{\mathrm {log}}$
is split, that is, that the natural action of
![]() $\mathrm {Gal}(\overline K/K)$
on the dual semi-graph
$\mathrm {Gal}(\overline K/K)$
on the dual semi-graph
![]() $\Gamma _{X^{\mathrm {log}}}$
associated with the geometric special fiber of
$\Gamma _{X^{\mathrm {log}}}$
associated with the geometric special fiber of
![]() $\mathcal {X}^{\mathrm {log}}$
is trivial. Write
$\mathcal {X}^{\mathrm {log}}$
is trivial. Write
![]() $X^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathcal {X}^{\mathrm {log}} \times _R K$
;
$X^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathcal {X}^{\mathrm {log}} \times _R K$
;
![]() $\mathrm {Vert}(X^{\mathrm {log}})$
(resp.
$\mathrm {Vert}(X^{\mathrm {log}})$
(resp.
![]() $\mathrm {Cusp}(X^{\mathrm {log}})$
;
$\mathrm {Cusp}(X^{\mathrm {log}})$
;
![]() $\mathrm {Node}(X^{\mathrm {log}})$
) for the set of vertices (resp. open edges; closed edges) of
$\mathrm {Node}(X^{\mathrm {log}})$
) for the set of vertices (resp. open edges; closed edges) of
![]() $\Gamma _{X^{\mathrm {log}}}$
, that is, the set of connected components of the complement of the cusps and nodes (resp. the set of cusps; the set of nodes) of the special fiber of
$\Gamma _{X^{\mathrm {log}}}$
, that is, the set of connected components of the complement of the cusps and nodes (resp. the set of cusps; the set of nodes) of the special fiber of
![]() $\mathcal {X}^{\mathrm {log}}$
;
$\mathcal {X}^{\mathrm {log}}$
;
Before proceeding, we recall that
to each element
![]() $z\in \mathrm {VCN}(X^{\mathrm {log}})$
, one may associate, in a way that is functorial with respect to arbitrary automorphisms of the log scheme
$z\in \mathrm {VCN}(X^{\mathrm {log}})$
, one may associate, in a way that is functorial with respect to arbitrary automorphisms of the log scheme
![]() $X^{\mathrm {log}}$
, a discrete valuation that dominates R on the residue field of some point of X, which is closed if and only if z is a cusp.
$X^{\mathrm {log}}$
, a discrete valuation that dominates R on the residue field of some point of X, which is closed if and only if z is a cusp.
Indeed, this is immediate if z is a vertex, since a vertex corresponds to a prime of height
![]() $1$
of
$1$
of
![]() $\mathcal {X}$
. This is also immediate if z is a cusp, since the residue field of the closed point of X that corresponds to z is finite over (the complete discrete valuation field) K, which implies that the discrete valuation of K extends uniquely to a discrete valuation on the residue field of a cusp. Now suppose that z is a node that is locally defined by an equation of the form
$\mathcal {X}$
. This is also immediate if z is a cusp, since the residue field of the closed point of X that corresponds to z is finite over (the complete discrete valuation field) K, which implies that the discrete valuation of K extends uniquely to a discrete valuation on the residue field of a cusp. Now suppose that z is a node that is locally defined by an equation of the form
![]() $s_1 s_2 - a$
, for some
$s_1 s_2 - a$
, for some
![]() $a\in \mathfrak {m}_R$
(cf., e.g., the discussion of [Reference Hoshi and Mochizuki9, Def. 5.3(ii)]). By descent, we may assume without loss of generality that a admits a square root b in R. Then one associates with z the discrete valuation determined by the exceptional divisor of the blowup of
$a\in \mathfrak {m}_R$
(cf., e.g., the discussion of [Reference Hoshi and Mochizuki9, Def. 5.3(ii)]). By descent, we may assume without loss of generality that a admits a square root b in R. Then one associates with z the discrete valuation determined by the exceptional divisor of the blowup of
![]() $\mathcal {X}$
at the locus
$\mathcal {X}$
at the locus
![]() $(s_1,s_2,b)$
. (One verifies immediately that this construction is compatible with arbitrary automorphisms of
$(s_1,s_2,b)$
. (One verifies immediately that this construction is compatible with arbitrary automorphisms of
![]() $X^{\mathrm {log}}$
.)
$X^{\mathrm {log}}$
.)
Corollary 1.15 (Fixed points associated with Galois sections).
Let
![]() $\Sigma $
be a set of prime numbers;
$\Sigma $
be a set of prime numbers;
![]() $\Sigma ^\dagger \subseteq \Sigma $
a subset;
$\Sigma ^\dagger \subseteq \Sigma $
a subset;
![]() $l\in \Sigma ^\dagger $
; R a complete discrete valuation ring of residue characteristic
$l\in \Sigma ^\dagger $
; R a complete discrete valuation ring of residue characteristic
![]() $p\not \in \Sigma ^\dagger $
(so p may be zero);
$p\not \in \Sigma ^\dagger $
(so p may be zero);
![]() $\overline {K}$
a separable closure of the field of fractions K of R;
$\overline {K}$
a separable closure of the field of fractions K of R;
a stable log curve (cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]) over the log regular log scheme
![]() $\mathrm {Spec}(R)^{\mathrm {log}}$
obtained by equipping
$\mathrm {Spec}(R)^{\mathrm {log}}$
obtained by equipping
![]() $\mathrm {Spec}(R)$
with the log structure determined by the maximal ideal of R. Write
$\mathrm {Spec}(R)$
with the log structure determined by the maximal ideal of R. Write
![]() $G_K\stackrel {\mathrm {def}}{=} \mathrm {Gal}(\overline {K} / K)$
for the absolute Galois group of K;
$G_K\stackrel {\mathrm {def}}{=} \mathrm {Gal}(\overline {K} / K)$
for the absolute Galois group of K;
![]() $I_K \subseteq G_K$
for the inertia subgroup of
$I_K \subseteq G_K$
for the inertia subgroup of
![]() $G_K$
;
$G_K$
;
![]() $X^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathcal {X}^{\mathrm {log}} \times _R K$
;
$X^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathcal {X}^{\mathrm {log}} \times _R K$
;
![]() $X_{\overline {K}}^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathcal {X}^{\mathrm {log}} \times _R \overline {K}$
;
$X_{\overline {K}}^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathcal {X}^{\mathrm {log}} \times _R \overline {K}$
;
for the pro-
![]() $\Sigma $
log fundamental group of
$\Sigma $
log fundamental group of
![]() $X_{\overline {K}}^{\mathrm {log}}$
(i.e., the maximal pro-
$X_{\overline {K}}^{\mathrm {log}}$
(i.e., the maximal pro-
![]() $\Sigma $
quotient of the log fundamental group of
$\Sigma $
quotient of the log fundamental group of
![]() $X^{\mathrm {log}}_{\overline {K}}$
);
$X^{\mathrm {log}}_{\overline {K}}$
);
for the geometrically pro-
![]() $\Sigma $
log fundamental group of
$\Sigma $
log fundamental group of
![]() $X^{\mathrm {log}}$
(i.e., the quotient of the log fundamental group of
$X^{\mathrm {log}}$
(i.e., the quotient of the log fundamental group of
![]() $X^{\mathrm {log}}$
by the kernel of the natural surjection from the log fundamental group of
$X^{\mathrm {log}}$
by the kernel of the natural surjection from the log fundamental group of
![]() $X^{\mathrm {log}}_{\overline {K}}$
onto
$X^{\mathrm {log}}_{\overline {K}}$
onto
![]() $\Delta _{X^{\mathrm {log}}}$
). Thus, we have a natural exact sequence of profinite groups
$\Delta _{X^{\mathrm {log}}}$
). Thus, we have a natural exact sequence of profinite groups
Write
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
for the profinite log étale covering of
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
for the profinite log étale covering of
![]() $X^{\mathrm {log}}$
corresponding to
$X^{\mathrm {log}}$
corresponding to
![]() $\Pi _{X^{\mathrm {log}}}$
. If
$\Pi _{X^{\mathrm {log}}}$
. If
![]() $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a finite connected subcovering of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a finite connected subcovering of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
![]() $\mathcal {Y}^{\mathrm {log}}$
over the normalization
$\mathcal {Y}^{\mathrm {log}}$
over the normalization
![]() $R_Y$
of R in Y, then let us write
$R_Y$
of R in Y, then let us write
![]() $\Gamma _{Y^{\mathrm {log}}}$
for the dual semi-graph determined by the geometric special fiber of
$\Gamma _{Y^{\mathrm {log}}}$
for the dual semi-graph determined by the geometric special fiber of
![]() $\mathcal {Y}^{\mathrm {log}}$
over
$\mathcal {Y}^{\mathrm {log}}$
over
![]() $R_Y$
;
$R_Y$
;
![]() $\mathrm {Vert}(Y^{\mathrm {log}})$
(resp.
$\mathrm {Vert}(Y^{\mathrm {log}})$
(resp.
![]() $\mathrm {Cusp}(Y^{\mathrm {log}})$
;
$\mathrm {Cusp}(Y^{\mathrm {log}})$
;
![]() $\mathrm {Node}(Y^{\mathrm {log}})$
) for the set of vertices (resp. open edges; closed edges) of
$\mathrm {Node}(Y^{\mathrm {log}})$
) for the set of vertices (resp. open edges; closed edges) of
![]() $\Gamma _{Y^{\mathrm {log}}}$
, that is, the set of connected components of the complement of the cusps and nodes (resp. the set of cusps; the set of nodes) of the geometric special fiber of
$\Gamma _{Y^{\mathrm {log}}}$
, that is, the set of connected components of the complement of the cusps and nodes (resp. the set of cusps; the set of nodes) of the geometric special fiber of
![]() $\mathcal {Y}^{\mathrm {log}}$
over
$\mathcal {Y}^{\mathrm {log}}$
over
![]() $R_Y$
;
$R_Y$
;
where the projective limit is over all finite connected subcoverings
![]() $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
as above, and, moreover, for each finite connected subcovering
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
as above, and, moreover, for each finite connected subcovering
![]() $Y_1^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
$Y_1^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
![]() $\mathcal {Y}_1^{\mathrm {log}}$
over the normalization of R in
$\mathcal {Y}_1^{\mathrm {log}}$
over the normalization of R in
![]() $Y_1$
, the transition map for a finite connected subcovering
$Y_1$
, the transition map for a finite connected subcovering
![]() $Y_2^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
of
$Y_2^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
of
![]() $\widetilde X^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
that admits a stable model
$\widetilde X^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
that admits a stable model
![]() $\mathcal {Y}_2^{\mathrm {log}}$
over the normalization of R in
$\mathcal {Y}_2^{\mathrm {log}}$
over the normalization of R in
![]() $Y_2$
is defined, for
$Y_2$
is defined, for
![]() $z \in \mathrm {VCN}(Y_2^{\mathrm {log}})$
, as follows:
$z \in \mathrm {VCN}(Y_2^{\mathrm {log}})$
, as follows:
-
• If the connected component/cusp/node corresponding to z maps, via the extension
 $\mathcal {Y}_2^{\mathrm {log}} \rightarrow \mathcal {Y}_1^{\mathrm {log}}$
of
$\mathcal {Y}_2^{\mathrm {log}} \rightarrow \mathcal {Y}_1^{\mathrm {log}}$
of
 $Y_2^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
(cf., e.g., [Reference Mochizuki14, Th. C]), to a cusp or node of the geometric special fiber of
$Y_2^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
(cf., e.g., [Reference Mochizuki14, Th. C]), to a cusp or node of the geometric special fiber of
 $\mathcal {Y}_1$
, then the image of
$\mathcal {Y}_1$
, then the image of
 $z \in \mathrm {VCN}(Y_2^{\mathrm {log}})$
in
$z \in \mathrm {VCN}(Y_2^{\mathrm {log}})$
in
 $\mathrm {VCN}(Y_1^{\mathrm {log}})$
is defined to be the element of
$\mathrm {VCN}(Y_1^{\mathrm {log}})$
is defined to be the element of
 $\mathrm {Edge}(Y_1^{\mathrm {log}})$
corresponding to the cusp or node.
$\mathrm {Edge}(Y_1^{\mathrm {log}})$
corresponding to the cusp or node. -
• If the generic point of the connected component/cusp/node corresponding to z maps, via the extension
 ${\mathcal {Y}_2^{\mathrm {log}} \rightarrow \mathcal {Y}_1^{\mathrm {log}}}$
of
${\mathcal {Y}_2^{\mathrm {log}} \rightarrow \mathcal {Y}_1^{\mathrm {log}}}$
of
 $Y_2^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
, to a point of the geometric special fiber of
$Y_2^{\mathrm {log}} \rightarrow Y_1^{\mathrm {log}}$
, to a point of the geometric special fiber of
 $\mathcal {Y}_1$
that is neither a cusp nor node, then the image of
$\mathcal {Y}_1$
that is neither a cusp nor node, then the image of
 $z \in \mathrm {VCN}(Y_2^{\mathrm {log}})$
in
$z \in \mathrm {VCN}(Y_2^{\mathrm {log}})$
in
 $\mathrm {VCN}(Y_1^{\mathrm {log}})$
is defined to be the element of
$\mathrm {VCN}(Y_1^{\mathrm {log}})$
is defined to be the element of
 $\mathrm {Vert}(Y_1^{\mathrm {log}})$
corresponding to the connected component on which the point lies.
$\mathrm {Vert}(Y_1^{\mathrm {log}})$
corresponding to the connected component on which the point lies.
If
![]() $\widetilde z \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
, and
$\widetilde z \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
, and
![]() $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a finite connected subcovering of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a finite connected subcovering of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
![]() $\mathcal {Y}^{\mathrm {log}}$
over the normalization of R in Y, then let us write
$\mathcal {Y}^{\mathrm {log}}$
over the normalization of R in Y, then let us write
![]() $\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
for the element of
$\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
for the element of
![]() $\mathrm {VCN}(Y^{\mathrm {log}})$
determined by
$\mathrm {VCN}(Y^{\mathrm {log}})$
determined by
![]() $\widetilde z$
. Let
$\widetilde z$
. Let
![]() $H \subseteq G_K$
be a closed subgroup such that the image of
$H \subseteq G_K$
be a closed subgroup such that the image of
via the natural surjection
![]() $I_K \twoheadrightarrow I_K^{\Sigma ^\dagger }$
to the pro-
$I_K \twoheadrightarrow I_K^{\Sigma ^\dagger }$
to the pro-
![]() $\Sigma ^\dagger $
completion
$\Sigma ^\dagger $
completion
![]() $I_K^{\Sigma ^\dagger }$
of
$I_K^{\Sigma ^\dagger }$
of
![]() $I_K$
is an open subgroup of
$I_K$
is an open subgroup of
![]() $I_K^{\Sigma ^\dagger }$
and
$I_K^{\Sigma ^\dagger }$
and
a section of the restriction to
![]() $H\subseteq G_K$
of the above exact sequence
$H\subseteq G_K$
of the above exact sequence
![]() $1 \rightarrow \Delta _{X^{\mathrm {log}}} \rightarrow \Pi _{X^{\mathrm {log}}} \rightarrow G_K\rightarrow 1$
. Then the following hold:
$1 \rightarrow \Delta _{X^{\mathrm {log}}} \rightarrow \Pi _{X^{\mathrm {log}}} \rightarrow G_K\rightarrow 1$
. Then the following hold:
-
(i) If we write
 $\Delta _{X^{\mathrm {log}}}^\dagger $
for the maximal pro-
$\Delta _{X^{\mathrm {log}}}^\dagger $
for the maximal pro-
 $\Sigma ^\dagger $
quotient of
$\Sigma ^\dagger $
quotient of
 $\Delta _{X^{\mathrm {log}}}$
and regard, via the specialization outer isomorphism with respect to
$\Delta _{X^{\mathrm {log}}}$
and regard, via the specialization outer isomorphism with respect to
 $\mathcal {X}^{\mathrm {log}}$
, the pro-
$\mathcal {X}^{\mathrm {log}}$
, the pro-
 $\Sigma ^\dagger $
group
$\Sigma ^\dagger $
group
 $\Delta _{X^{\mathrm {log}}}^\dagger $
as the (pro-
$\Delta _{X^{\mathrm {log}}}^\dagger $
as the (pro-
 $\Sigma ^\dagger $
) fundamental group of the semi-graph of anabelioids of pro-
$\Sigma ^\dagger $
) fundamental group of the semi-graph of anabelioids of pro-
 $\Sigma ^\dagger $
PSC-type determined by the geometric special fiber of the stable model
$\Sigma ^\dagger $
PSC-type determined by the geometric special fiber of the stable model
 $\mathcal {X}^{\mathrm {log}}$
(cf. [Reference Mochizuki18, Exam. 2.5]), then the natural outer Galois action determined by the above exact sequence is of EPIPSC-type for the conducting subgroup
$\mathcal {X}^{\mathrm {log}}$
(cf. [Reference Mochizuki18, Exam. 2.5]), then the natural outer Galois action determined by the above exact sequence is of EPIPSC-type for the conducting subgroup $$\begin{align*}H \longrightarrow \mathrm{Out}(\Delta_{X^{\mathrm{log}}}^\dagger)\end{align*}$$
$$\begin{align*}H \longrightarrow \mathrm{Out}(\Delta_{X^{\mathrm{log}}}^\dagger)\end{align*}$$
 $I_H \subseteq H$
(cf. Definition 1.7(i)). If, moreover, H is l-cyclotomically full, that is, the image of
$I_H \subseteq H$
(cf. Definition 1.7(i)). If, moreover, H is l-cyclotomically full, that is, the image of
 $H\subseteq G_K$
via the l-adic cyclotomic character on
$H\subseteq G_K$
via the l-adic cyclotomic character on
 $G_K$
is open, then the above outer Galois action is l-cyclotomically full (cf. Definition 1.7(ii)).
$G_K$
is open, then the above outer Galois action is l-cyclotomically full (cf. Definition 1.7(ii)).
-
(ii) Suppose that the residue field of R is countable. Let
 $\widetilde z \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
and
$\widetilde z \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
and
 $\mathcal {S} = \{Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}\}$
a cofinal system consisting of finite Galois subcoverings
$\mathcal {S} = \{Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}\}$
a cofinal system consisting of finite Galois subcoverings
 $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
 $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
such that
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
such that
 $Y^{\mathrm {log}}$
admits a split stable model over the normalization
$Y^{\mathrm {log}}$
admits a split stable model over the normalization
 $R_Y$
of R in Y. Then there exist a valuation
$R_Y$
of R in Y. Then there exist a valuation
 $v_{\widetilde z}$
on the residue field of some point of the underlying scheme
$v_{\widetilde z}$
on the residue field of some point of the underlying scheme
 $\widetilde X$
of
$\widetilde X$
of
 $\widetilde X^{\mathrm {log}}$
(i.e., a bounded multiplicative seminorm—cf., e.g., [Reference Berkovich2, §§1.1 and 1.2]) and a countably indexed cofinal subsystem
$\widetilde X^{\mathrm {log}}$
(i.e., a bounded multiplicative seminorm—cf., e.g., [Reference Berkovich2, §§1.1 and 1.2]) and a countably indexed cofinal subsystem
 $\mathcal {S}'$
of
$\mathcal {S}'$
of
 $\mathcal {S}$
such that if
$\mathcal {S}$
such that if
 $Z^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a member of
$Z^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a member of
 $\mathcal {S}'$
, then, as
$\mathcal {S}'$
, then, as
 $Y^{\mathrm {log}}\rightarrow X^{\mathrm {log}}$
ranges over the members of
$Y^{\mathrm {log}}\rightarrow X^{\mathrm {log}}$
ranges over the members of
 $\mathcal {S}'$
that lie over
$\mathcal {S}'$
that lie over
 $Z^{\mathrm {log}}$
, the discrete valuations on residue fields of points of the underlying scheme Z of
$Z^{\mathrm {log}}$
, the discrete valuations on residue fields of points of the underlying scheme Z of
 $Z^{\mathrm {log}}$
determined by the elements
$Z^{\mathrm {log}}$
determined by the elements
 $\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
(cf. the discussion preceding the present Corollary 1.15) converge in the “Berkovich space topology”—that is, as bounded multiplicative seminorms—to the valuation on the residue field of some point of Z determined by
$\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
(cf. the discussion preceding the present Corollary 1.15) converge in the “Berkovich space topology”—that is, as bounded multiplicative seminorms—to the valuation on the residue field of some point of Z determined by
 $v_{\widetilde z}$
.
$v_{\widetilde z}$
. -
(iii) Write
 $\mathrm {Stab}(s) \subseteq \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
for the subset consisting of elements
$\mathrm {Stab}(s) \subseteq \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
for the subset consisting of elements
 $\widetilde z \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
such that the image of s stabilizes
$\widetilde z \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
such that the image of s stabilizes
 $\widetilde z$
. Suppose that H is l-cyclotomically full (cf. (i)). Then it holds that
$\widetilde z$
. Suppose that H is l-cyclotomically full (cf. (i)). Then it holds that  $$ \begin{align*}\mathrm{Stab}(s)\neq\emptyset .\end{align*} $$
$$ \begin{align*}\mathrm{Stab}(s)\neq\emptyset .\end{align*} $$
In particular, if
 $\widetilde z\in \mathrm {Stab}(s)$
, and the residue field of R is countable, then the image of s lies in the decomposition group of any valuation
$\widetilde z\in \mathrm {Stab}(s)$
, and the residue field of R is countable, then the image of s lies in the decomposition group of any valuation
 $v_{\widetilde z}$
as in (ii).
$v_{\widetilde z}$
as in (ii). -
(iv) Let
 $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
be a finite connected subcovering of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
be a finite connected subcovering of
 $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model over the normalization
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model over the normalization
 $ R_Y$
of R in Y;
$ R_Y$
of R in Y;
 $\widetilde z_1$
,
$\widetilde z_1$
,
 $\widetilde z_2 \in \mathrm {Stab}(s)$
(cf. (iii)). Then one of the following four (mutually exclusive) conditions is satisfied:
$\widetilde z_2 \in \mathrm {Stab}(s)$
(cf. (iii)). Then one of the following four (mutually exclusive) conditions is satisfied:-
(a)
 $\widetilde z_1(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and
 $\delta ( \widetilde z_1(Y^{\mathrm {log}}), \widetilde z_2(Y^{\mathrm {log}}) ) \leq 2$
(cf. Definition 1.1(iii)).
$\delta ( \widetilde z_1(Y^{\mathrm {log}}), \widetilde z_2(Y^{\mathrm {log}}) ) \leq 2$
(cf. Definition 1.1(iii)). -
(b)
 $\widetilde z_1(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
 $ \mathcal {V}( \widetilde z_1(Y^{\mathrm {log}}) ) $
$ \mathcal {V}( \widetilde z_1(Y^{\mathrm {log}}) ) $
 $\cap \mathcal {V}( \widetilde z_2(Y^{\mathrm {log}}) ) \neq \emptyset $
.
$\cap \mathcal {V}( \widetilde z_2(Y^{\mathrm {log}}) ) \neq \emptyset $
. -
(c)
 $\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
 $\mathcal {V}^{\delta \leq 1}(\widetilde z_1(Y^{\mathrm {log}})) \cap \mathcal {V}(\widetilde z_2(Y^{\mathrm {log}}))\neq \emptyset $
(cf. Definition 1.10(i)).
$\mathcal {V}^{\delta \leq 1}(\widetilde z_1(Y^{\mathrm {log}})) \cap \mathcal {V}(\widetilde z_2(Y^{\mathrm {log}}))\neq \emptyset $
(cf. Definition 1.10(i)). -
(d)
 $\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and, moreover,
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and, moreover,
 $ \mathcal {V}(\widetilde z_1(Y^{\mathrm {log}})) \cap \mathcal {V}^{\delta \leq 1}(\widetilde z_2(Y^{\mathrm {log}})) \neq \emptyset $
.
$ \mathcal {V}(\widetilde z_1(Y^{\mathrm {log}})) \cap \mathcal {V}^{\delta \leq 1}(\widetilde z_2(Y^{\mathrm {log}})) \neq \emptyset $
.
-
-
(v) In the situation of (iv), suppose, moreover, that the following assertion—that is, concerning “resolution of nonsingularities” (cf. Remark 1.15.1 below)—holds:
Then every finite connected subcovering $(\dagger ^{\mathrm {RNS}})$
: Let
$(\dagger ^{\mathrm {RNS}})$
: Let
 $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
be a finite connected subcovering of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
be a finite connected subcovering of
 $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model
 $\mathcal {Y}^{\mathrm {log}}$
over
$\mathcal {Y}^{\mathrm {log}}$
over
 $R_Y$
and
$R_Y$
and
 $y \in \mathcal {Y}$
a node of
$y \in \mathcal {Y}$
a node of
 $\mathcal {Y}$
. Then there exists a finite connected subcovering
$\mathcal {Y}$
. Then there exists a finite connected subcovering
 $Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
of
$Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
of
 $\widetilde X^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
that admits a stable model
$\widetilde X^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
that admits a stable model
 $\mathcal {Z}^{\mathrm {log}}$
over
$\mathcal {Z}^{\mathrm {log}}$
over
 $R_Z$
such that the fiber over y of the morphism
$R_Z$
such that the fiber over y of the morphism
 $\mathcal {Z} \rightarrow \mathcal {Y}$
determined by
$\mathcal {Z} \rightarrow \mathcal {Y}$
determined by
 $Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
is not finite.
$Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
is not finite. $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
 $\widetilde X^{\mathrm {log}}\rightarrow X^{\mathrm {log}}$
that admits a stable model over
$\widetilde X^{\mathrm {log}}\rightarrow X^{\mathrm {log}}$
that admits a stable model over
 $R_Y$
satisfies one of the following four (mutually exclusive) conditions:
$R_Y$
satisfies one of the following four (mutually exclusive) conditions:
-
(a′)
 $\widetilde z_1(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and
 $\widetilde z_1(Y^{\mathrm {log}})=\widetilde z_2(Y^{\mathrm {log}})$
.
$\widetilde z_1(Y^{\mathrm {log}})=\widetilde z_2(Y^{\mathrm {log}})$
. -
(b′)
 $\widetilde z_1(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
 $ \mathcal {V}( \widetilde z_1(Y^{\mathrm {log}}) ) $
$ \mathcal {V}( \widetilde z_1(Y^{\mathrm {log}}) ) $
 $\cap \mathcal {V}( \widetilde z_2(Y^{\mathrm {log}}) ) \neq \emptyset $
.
$\cap \mathcal {V}( \widetilde z_2(Y^{\mathrm {log}}) ) \neq \emptyset $
. -
(c′)
 $\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
, and, moreover,
 $\widetilde z_1(Y^{\mathrm {log}}) \in \mathcal {V}(\widetilde z_2(Y^{\mathrm {log}}))$
.
$\widetilde z_1(Y^{\mathrm {log}}) \in \mathcal {V}(\widetilde z_2(Y^{\mathrm {log}}))$
. -
(d′)
 $\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
,
$\widetilde z_1(Y^{\mathrm {log}}) \in \mathrm {Edge}(Y^{\mathrm {log}})$
,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and, moreover,
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathrm {Vert}(Y^{\mathrm {log}})$
, and, moreover,
 $\widetilde z_2(Y^{\mathrm {log}}) \in \mathcal {V}(\widetilde z_1(Y^{\mathrm {log}}))$
.
$\widetilde z_2(Y^{\mathrm {log}}) \in \mathcal {V}(\widetilde z_1(Y^{\mathrm {log}}))$
.
-
-
(vi) Write
 $\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
for the
$\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
for the
 $\Sigma $
-tempered fundamental group of
$\Sigma $
-tempered fundamental group of
 $X^{\mathrm {log}}_{\overline K}$
(cf. [Reference Hoshi and Mochizuki11, Def. 3.1(ii)]);
$X^{\mathrm {log}}_{\overline K}$
(cf. [Reference Hoshi and Mochizuki11, Def. 3.1(ii)]);
 $\Pi _{X^{\mathrm {log}}}^{\mathrm {tp}}$
for the geometrically
$\Pi _{X^{\mathrm {log}}}^{\mathrm {tp}}$
for the geometrically
 $\Sigma $
-tempered fundamental group of
$\Sigma $
-tempered fundamental group of
 $X^{\mathrm {log}}$
(i.e., the quotient of the tempered fundamental group of
$X^{\mathrm {log}}$
(i.e., the quotient of the tempered fundamental group of
 $X^{\mathrm {log}}$
by the kernel of the natural surjection from the tempered fundamental group of
$X^{\mathrm {log}}$
by the kernel of the natural surjection from the tempered fundamental group of
 $X^{\mathrm {log}}_{\overline K}$
onto
$X^{\mathrm {log}}_{\overline K}$
onto
 $\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
). Thus, we have a natural exact sequence of topological groups Write
$\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
). Thus, we have a natural exact sequence of topological groups Write $$\begin{align*}1 \longrightarrow \Delta_{X^{\mathrm{log}}}^{\mathrm{tp}} \longrightarrow \Pi_{X^{\mathrm{log}}}^{\mathrm{tp}} \longrightarrow G_K \longrightarrow 1. \end{align*}$$
$$\begin{align*}1 \longrightarrow \Delta_{X^{\mathrm{log}}}^{\mathrm{tp}} \longrightarrow \Pi_{X^{\mathrm{log}}}^{\mathrm{tp}} \longrightarrow G_K \longrightarrow 1. \end{align*}$$
 $\mathrm {Sect}(\Pi _{X^{\mathrm {log}}} /H)$
for the set of
$\mathrm {Sect}(\Pi _{X^{\mathrm {log}}} /H)$
for the set of
 $\Delta _{X^{\mathrm {log}}}$
-conjugacy classes of continuous sections of the restriction to
$\Delta _{X^{\mathrm {log}}}$
-conjugacy classes of continuous sections of the restriction to
 $H\subseteq G_K$
of the natural surjection
$H\subseteq G_K$
of the natural surjection
 $\Pi _{X^{\mathrm {log}}}\twoheadrightarrow G_K$
and
$\Pi _{X^{\mathrm {log}}}\twoheadrightarrow G_K$
and
 $\mathrm {Sect}(\Pi _{X^{\mathrm {log}}}^{\mathrm {tp}}/H)$
for the set of
$\mathrm {Sect}(\Pi _{X^{\mathrm {log}}}^{\mathrm {tp}}/H)$
for the set of
 $\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
-conjugacy classes of continuous sections of the restriction to
$\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
-conjugacy classes of continuous sections of the restriction to
 $H\subseteq G_K$
of the natural surjection
$H\subseteq G_K$
of the natural surjection
 $\Pi _{X^{\mathrm {log}}}^{\mathrm {tp}}\twoheadrightarrow G_K$
. Then the natural map is injective. If, moreover, H is l-cyclotomically full (cf. (i)), then this map is bijective.
$\Pi _{X^{\mathrm {log}}}^{\mathrm {tp}}\twoheadrightarrow G_K$
. Then the natural map is injective. If, moreover, H is l-cyclotomically full (cf. (i)), then this map is bijective. $$\begin{align*}\mathrm{Sect}(\Pi_{X^{\mathrm{log}}}^{\mathrm{tp}}/H) \longrightarrow \mathrm{Sect}(\Pi_{X^{\mathrm{log}}}/H) \end{align*}$$
$$\begin{align*}\mathrm{Sect}(\Pi_{X^{\mathrm{log}}}^{\mathrm{tp}}/H) \longrightarrow \mathrm{Sect}(\Pi_{X^{\mathrm{log}}}/H) \end{align*}$$
Proof. Assertion (i) follows immediately from the definition of the term “IPSC-type” (cf. [Reference Hoshi and Mochizuki8, Def. 2.4(i)]), together with the well-known structure of the maximal pro-
![]() $\Sigma ^\dagger $
quotient of
$\Sigma ^\dagger $
quotient of
![]() $I_K$
. Next, we verify assertion (ii). Let us first observe that it follows immediately from our countability assumption on the residue field of R that the following three assertions hold:
$I_K$
. Next, we verify assertion (ii). Let us first observe that it follows immediately from our countability assumption on the residue field of R that the following three assertions hold:
-
• If
 $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a member of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a member of
 $\mathcal {S}$
, and
$\mathcal {S}$
, and
 $\widetilde z(Y^{\mathrm {log}}) \not \in \mathrm {Cusp}(Y^{\mathrm {log}})$
, then the function field of Y admits a subset which is countable and dense, that is, with respect to the topology determined by the discrete valuation determined by the element
$\widetilde z(Y^{\mathrm {log}}) \not \in \mathrm {Cusp}(Y^{\mathrm {log}})$
, then the function field of Y admits a subset which is countable and dense, that is, with respect to the topology determined by the discrete valuation determined by the element
 $\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
.
$\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
. -
• If
 $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a member of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
is a member of
 $\mathcal {S}$
, and
$\mathcal {S}$
, and
 $\widetilde z(Y^{\mathrm {log}}) \in \mathrm {Cusp}(Y^{\mathrm {log}})$
, then the normalization
$\widetilde z(Y^{\mathrm {log}}) \in \mathrm {Cusp}(Y^{\mathrm {log}})$
, then the normalization
 $R_Y$
of R in Y admits a subset which is countable and dense, that is, with respect to the topology determined by the discrete valuation determined by the element
$R_Y$
of R in Y admits a subset which is countable and dense, that is, with respect to the topology determined by the discrete valuation determined by the element
 $\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
.
$\widetilde z(Y^{\mathrm {log}}) \in \mathrm {VCN}(Y^{\mathrm {log}})$
. -
• There exists a countably indexed cofinal subsystem of
 $\mathcal {S}$
(cf., e.g., [Reference Mochizuki21, Lem. 2.1]).
$\mathcal {S}$
(cf., e.g., [Reference Mochizuki21, Lem. 2.1]).
Thus, assertion (ii) follows immediately, by applying a standard argument involving Cantor diagonalization, from the well-known (local) compactness of Berkovich spaces (cf., e.g., [Reference Berkovich2, Th. 1.2.1]). Here, we recall in passing that this compactness is, in essence, a consequence of the compactness of a product of copies of the closed interval
![]() $[0,1]\subseteq \mathbb {R}$
. This completes the proof of assertion (ii). We refer to Theorem A.7 in the Appendix for another approach to proving assertion (ii).
$[0,1]\subseteq \mathbb {R}$
. This completes the proof of assertion (ii). We refer to Theorem A.7 in the Appendix for another approach to proving assertion (ii).
Assertion (iii) follows immediately from the observation that, by applying Theorem 1.13(i) (cf. also Remark 1.7.1; assertion (i) of the present Corollary 1.15; [Reference Mochizuki18, Prop. 1.2(i)]), together with the well-known fact that a projective limit of nonempty finite sets is nonempty, to the various finite connected subcoverings of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, one may conclude that the action of
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, one may conclude that the action of
![]() $G_K$
, via s, on
$G_K$
, via s, on
![]() $\widetilde X^{\mathrm {log}}$
fixes some element
$\widetilde X^{\mathrm {log}}$
fixes some element
![]() $\widetilde z_s \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
of
$\widetilde z_s \in \mathrm {VCN}(\widetilde X^{\mathrm {log}})$
of
![]() $\mathrm {VCN}(\widetilde X^{\mathrm {log}})$
. (Here, we note that when one applies Theorem 1.13(i) to the various finite connected subcoverings of
$\mathrm {VCN}(\widetilde X^{\mathrm {log}})$
. (Here, we note that when one applies Theorem 1.13(i) to the various finite connected subcoverings of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, the conducting subgroup “
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, the conducting subgroup “
![]() $I_G$
” of Theorem 1.13(i) must be allowed to vary among suitable open subgroups of the original conducting subgroup
$I_G$
” of Theorem 1.13(i) must be allowed to vary among suitable open subgroups of the original conducting subgroup
![]() $I_G$
.) Assertion (iv) follows immediately (cf. also Remark 1.7.1; assertion (i) of the present Corollary 1.15) from Lemma 1.11(ii).
$I_G$
.) Assertion (iv) follows immediately (cf. also Remark 1.7.1; assertion (i) of the present Corollary 1.15) from Lemma 1.11(ii).
Next, we verify assertion (v). Let us first observe that it follows immediately from assertion (iv) that if
![]() $Y^{\mathrm {log}}\rightarrow X^{\mathrm {log}}$
is a finite connected subcovering of
$Y^{\mathrm {log}}\rightarrow X^{\mathrm {log}}$
is a finite connected subcovering of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model over
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
that admits a stable model over
![]() $R_Y$
, then
$R_Y$
, then
![]() $\widetilde z_1(Y^{\mathrm {log}})$
and
$\widetilde z_1(Y^{\mathrm {log}})$
and
![]() $\widetilde z_2(Y^{\mathrm {log}})$
lie in a connected sub-semi-graph
$\widetilde z_2(Y^{\mathrm {log}})$
lie in a connected sub-semi-graph
![]() $\Gamma ^*$
of
$\Gamma ^*$
of
![]() $\Gamma _{Y^{\mathrm {log}}}$
such that
$\Gamma _{Y^{\mathrm {log}}}$
such that
Now one verifies immediately that this uniform bound “
![]() $5$
” implies that there exists a cofinal system
$5$
” implies that there exists a cofinal system
![]() $\mathcal {S} = \{Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}\}$
consisting of finite Galois subcoverings
$\mathcal {S} = \{Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}\}$
consisting of finite Galois subcoverings
![]() $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
such that
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
such that
![]() $Y^{\mathrm {log}}$
admits a stable model over
$Y^{\mathrm {log}}$
admits a stable model over
![]() $R_Y$
, and, moreover,
$R_Y$
, and, moreover,
![]() $\Gamma _{Y^{\mathrm {log}}}$
admits a connected sub-semi-graph
$\Gamma _{Y^{\mathrm {log}}}$
admits a connected sub-semi-graph
![]() $\Gamma ^*_{Y^{\mathrm {log}}}$
such that:
$\Gamma ^*_{Y^{\mathrm {log}}}$
such that:
-
•
 $\widetilde z_1(Y^{\mathrm {log}})$
and
$\widetilde z_1(Y^{\mathrm {log}})$
and
 $\widetilde z_2(Y^{\mathrm {log}})$
lie in
$\widetilde z_2(Y^{\mathrm {log}})$
lie in
 $\Gamma ^*_{Y^{\mathrm {log}}}$
;
$\Gamma ^*_{Y^{\mathrm {log}}}$
; -
•
 $\mathrm {VCN}(\Gamma ^*_{Y^{\mathrm {log}}})^\sharp \leq 5$
;
$\mathrm {VCN}(\Gamma ^*_{Y^{\mathrm {log}}})^\sharp \leq 5$
; -
• the semi-graphs
 $\Gamma ^*_{Y^{\mathrm {log}}}$
map isomorphically to one another as one varies
$\Gamma ^*_{Y^{\mathrm {log}}}$
map isomorphically to one another as one varies
 $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
.
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
.
Write
![]() $\mathcal {V}^*(Y^{\mathrm {log}}) \stackrel {\mathrm {def}}{=} \mathrm {Vert}(\Gamma ^*_{Y^{\mathrm {log}}})$
. Then it follows immediately from assertion (iv) that, to complete the verification of assertion (v), it suffices to verify that the following assertion holds:
$\mathcal {V}^*(Y^{\mathrm {log}}) \stackrel {\mathrm {def}}{=} \mathrm {Vert}(\Gamma ^*_{Y^{\mathrm {log}}})$
. Then it follows immediately from assertion (iv) that, to complete the verification of assertion (v), it suffices to verify that the following assertion holds:
Claim 1.15.A:
![]() $\mathcal {V}^*(Y^{\mathrm {log}})^\sharp \leq 1$
.
$\mathcal {V}^*(Y^{\mathrm {log}})^\sharp \leq 1$
.
Indeed, suppose that
![]() $\mathcal {V}^*(Y^{\mathrm {log}})^\sharp \geq 2$
. Then it follows immediately that there exists a compatible system of nodes
$\mathcal {V}^*(Y^{\mathrm {log}})^\sharp \geq 2$
. Then it follows immediately that there exists a compatible system of nodes
![]() $e(Y^{\mathrm {log}})$
of
$e(Y^{\mathrm {log}})$
of
![]() $\Gamma ^*_{Y^{\mathrm {log}}}$
(i.e., compatible as one varies
$\Gamma ^*_{Y^{\mathrm {log}}}$
(i.e., compatible as one varies
![]() $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
in
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
in
![]() $\mathcal {S}$
), each of which abuts to distinct vertices
$\mathcal {S}$
), each of which abuts to distinct vertices
![]() $v_\alpha (Y^{\mathrm {log}})$
,
$v_\alpha (Y^{\mathrm {log}})$
,
![]() $v_\beta (Y^{\mathrm {log}})$
of
$v_\beta (Y^{\mathrm {log}})$
of
![]() $\Gamma ^*_{Y^{\mathrm {log}}}$
. (Thus, one may assume that the vertices
$\Gamma ^*_{Y^{\mathrm {log}}}$
. (Thus, one may assume that the vertices
![]() $v_\alpha (-)$
[resp.
$v_\alpha (-)$
[resp.
![]() $v_\beta (-)$
] form a compatible system of vertices.) But this implies that for every
$v_\beta (-)$
] form a compatible system of vertices.) But this implies that for every
![]() $Z^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
in
$Z^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
in
![]() $\mathcal {S}$
that lies over
$\mathcal {S}$
that lies over
![]() $Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
in
$Y^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
in
![]() $\mathcal {S}$
, if we write
$\mathcal {S}$
, if we write
![]() $\mathcal {Y}^{\mathrm {log}}$
,
$\mathcal {Y}^{\mathrm {log}}$
,
![]() $\mathcal {Z}^{\mathrm {log}}$
for the respective stable models of
$\mathcal {Z}^{\mathrm {log}}$
for the respective stable models of
![]() $Y^{\mathrm {log}}$
,
$Y^{\mathrm {log}}$
,
![]() $Z^{\mathrm {log}}$
(so the morphism
$Z^{\mathrm {log}}$
(so the morphism
![]() $Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
extends to a morphism
$Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
extends to a morphism
![]() $\mathcal {Z}^{\mathrm {log}} \rightarrow \mathcal {Y}^{\mathrm {log}}$
—cf., e.g., [Reference Mochizuki14, Th. C]), then the inverse image in
$\mathcal {Z}^{\mathrm {log}} \rightarrow \mathcal {Y}^{\mathrm {log}}$
—cf., e.g., [Reference Mochizuki14, Th. C]), then the inverse image in
![]() $\mathcal {Z}^{\mathrm {log}}$
of the node
$\mathcal {Z}^{\mathrm {log}}$
of the node
![]() $e(Y^{\mathrm {log}})$
admits at least one isolated point (i.e.,
$e(Y^{\mathrm {log}})$
admits at least one isolated point (i.e.,
![]() $e(Z^{\mathrm {log}})$
), and hence (since the covering
$e(Z^{\mathrm {log}})$
), and hence (since the covering
![]() $Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
is Galois) the entire inverse image in
$Z^{\mathrm {log}} \rightarrow Y^{\mathrm {log}}$
is Galois) the entire inverse image in
![]() $\mathcal {Z}^{\mathrm {log}}$
of
$\mathcal {Z}^{\mathrm {log}}$
of
![]() $e(Y^{\mathrm {log}})$
is of dimension zero. On the other hand, this contradicts the assertion
$e(Y^{\mathrm {log}})$
is of dimension zero. On the other hand, this contradicts the assertion
![]() $(\dagger ^{\mathrm {RNS}})$
in the statement of assertion (v). This completes the proof of assertion (v).
$(\dagger ^{\mathrm {RNS}})$
in the statement of assertion (v). This completes the proof of assertion (v).
Finally, we verify assertion (vi). The injectivity portion of assertion (v) follows immediately from the injectivity portion of Theorem 1.13(iii) (cf. also Remark 1.7.1; assertion (i) of the present Corollary 1.15), applied to the various finite connected subcoverings of
![]() $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, where we take the “
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, where we take the “
![]() $\Sigma $
” of Theorem 1.13 to be
$\Sigma $
” of Theorem 1.13 to be
![]() $\Sigma ^\dagger $
(cf. also the fact that, in the notation of Theorem 1.13, “
$\Sigma ^\dagger $
(cf. also the fact that, in the notation of Theorem 1.13, “
![]() $\Pi _{\mathcal {G}}^{\mathrm {tp}}$
” is dense in “
$\Pi _{\mathcal {G}}^{\mathrm {tp}}$
” is dense in “
![]() $\Pi _{\mathcal {G}}$
” in the profinite topology). Here, we note that
$\Pi _{\mathcal {G}}$
” in the profinite topology). Here, we note that
-
• when one applies Theorem 1.13(iii) to the various finite connected subcoverings of
 $\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, the conducting subgroup “
$\widetilde X^{\mathrm {log}} \rightarrow X^{\mathrm {log}}$
, the conducting subgroup “
 $I_G$
” of Theorem 1.13(iii) must be allowed to vary among suitable open subgroups of the original conducting subgroup
$I_G$
” of Theorem 1.13(iii) must be allowed to vary among suitable open subgroups of the original conducting subgroup
 $I_G$
, and that
$I_G$
, and that -
• it follows immediately from the final portion of Lemma 1.11(iv) that the resulting conjugacy indeterminacies that occur at various subcoverings are uniquely determined up to profinite centralizers of the sections that appear, hence converge in
 $\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
(i.e., if one passes to an appropriate subsequence of the system of subcoverings under consideration).
$\Delta _{X^{\mathrm {log}}}^{\mathrm {tp}}$
(i.e., if one passes to an appropriate subsequence of the system of subcoverings under consideration).
If H is l-cyclotomically full, then the surjectivity of the map
follows formally (cf. the proof of the final portion of Theorem 1.13(iii)) from the nonemptiness verified in assertion (iii). This completes the proof of assertion (vi).
Remark 1.15.1. It follows from [Reference Tamagawa29, Th. 0.2(v)] that if K is of characteristic zero, the residue field of R is algebraic over
![]() $\mathbb {F}_p$
, and
$\mathbb {F}_p$
, and
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
, then the assertion
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
, then the assertion
![]() $(\dagger ^{\mathrm {RNS}})$
in the statement of Corollary 1.15(v) holds.
$(\dagger ^{\mathrm {RNS}})$
in the statement of Corollary 1.15(v) holds.
Remark 1.15.2.
-
(i) Corollary 1.15(iii) and (v) (cf. also [Reference Mochizuki17, Lem. 5.5]) may be regarded as a generalization of the Main Result of [Reference Pop and Stix26]. These results are obtained in the present paper (cf. the proof of Theorem 1.13(i)) by, in essence, combining, via a similar argument to the argument applied in the tempered case treated in [Reference Mochizuki17, Ths. 3.7 and 5.4] (cf. also the proof of Theorem 1.13(ii) of the present paper), the uniqueness result given in [Reference Hoshi and Mochizuki8, Props. 3.8(i) and 3.9(i)–(iii)] (cf. the proof of Lemma 1.11(ii)), with the existence of fixed points of actions of finite groups on graphs that follows as a consequence of the classical fact that (discrete or pro-
 $\Sigma $
) free groups are torsion-free (cf. Remarks 1.6.2 and 1.13.1; the proof of Lemma 1.6(ii)). One slight difference between the profinite and tempered cases is that, whereas, in the tempered case, it follows from the discreteness of the fundamental groups of graphs that appear that the actions of profinite groups on universal coverings of such graphs necessarily factor through finite quotients, the corresponding fact in the profinite case is obtained as a consequence of the fact that, under a suitable assumption on the cyclotomic characters that appear, any homomorphism from a “positive slope” module to a torsion-free “slope zero” module necessarily vanishes (cf. the proof of Claim 1.13.B in Theorem 1.13(i)). That is to say, in a word, these results are obtained in the present paper as a consequence ofThis a priori irrelevance of scheme theory to such abstract considerations is reflected both in the variety of the results obtained in the present §1 as consequences of Theorem 1.13, as well as in the generality of Corollary 1.15. This approach contrasts quite substantially with the approach of [Reference Pop and Stix26], that is, where the main results are derived as a consequence of highly scheme-theoretic considerations concerning stable curves over complete discrete valuation rings, in which the theory of the Brauer group of the function field of such a curve plays a central role (cf. [Reference Pop and Stix26, §4]).
$\Sigma $
) free groups are torsion-free (cf. Remarks 1.6.2 and 1.13.1; the proof of Lemma 1.6(ii)). One slight difference between the profinite and tempered cases is that, whereas, in the tempered case, it follows from the discreteness of the fundamental groups of graphs that appear that the actions of profinite groups on universal coverings of such graphs necessarily factor through finite quotients, the corresponding fact in the profinite case is obtained as a consequence of the fact that, under a suitable assumption on the cyclotomic characters that appear, any homomorphism from a “positive slope” module to a torsion-free “slope zero” module necessarily vanishes (cf. the proof of Claim 1.13.B in Theorem 1.13(i)). That is to say, in a word, these results are obtained in the present paper as a consequence ofThis a priori irrelevance of scheme theory to such abstract considerations is reflected both in the variety of the results obtained in the present §1 as consequences of Theorem 1.13, as well as in the generality of Corollary 1.15. This approach contrasts quite substantially with the approach of [Reference Pop and Stix26], that is, where the main results are derived as a consequence of highly scheme-theoretic considerations concerning stable curves over complete discrete valuation rings, in which the theory of the Brauer group of the function field of such a curve plays a central role (cf. [Reference Pop and Stix26, §4]).abstract considerations concerning abstract profinite groups acting on abstract semi-graphs that may, for instance, arise as dual semi-graphs of geometric special fibers of stable models of curves that appear in scheme theory, but, a priori, have nothing to do with scheme theory.
-
(ii) The essential equivalence between the issue of considering valuations fixed by Galois actions and the issue of considering vertices or edges of associated dual semi-graphs fixed by Galois actions may be seen in the well-known functorial homotopy equivalence between the Berkovich space associated with a stable curve over a complete discrete valuation ring and the associated dual graph (cf. [Reference Berkovich3, Ths. 8.1 and 8.2]). Moreover, the issue of convergence of (sub)sequences of valuations fixed by Galois actions is an easy consequence of the well-known (local) compactness of Berkovich spaces (cf. the proof of Corollary 1.15(ii); [Reference Berkovich2, Th. 1.2.1]), that is, in essence, a consequence of the well-known compactness of a product of copies of the closed interval
 $[0,1] \subseteq \mathbb {R}$
. That is to say, there is no need to consider the quite complicated (and, at the time of writing, not well understood!) structure of inductive limits of local rings, as discussed in [Reference Pop and Stix26, §1.6].
$[0,1] \subseteq \mathbb {R}$
. That is to say, there is no need to consider the quite complicated (and, at the time of writing, not well understood!) structure of inductive limits of local rings, as discussed in [Reference Pop and Stix26, §1.6].
Remark 1.15.3. Recall that in Corollary 1.15(ii) and the final portion of Corollary 1.15(iii), we assume that the residue field of R is countable. In fact, however, it is not difficult to see that, in the situation of Corollary 1.15, there exists a complete discrete valuation ring
![]() $R^\dagger $
that is dominated by R, and whose residue field is countable such that
$R^\dagger $
that is dominated by R, and whose residue field is countable such that
-
• the smooth log curve
 $X^{\mathrm {log}}$
,
$X^{\mathrm {log}}$
, -
• the closed subgroup
 $H \subseteq G_K$
, and
$H \subseteq G_K$
, and -
• the section
 $s\colon H \rightarrow \Pi _{X^{\mathrm {log}}}$
$s\colon H \rightarrow \Pi _{X^{\mathrm {log}}}$
descend to the field of fractions of
![]() $R^\dagger $
. Indeed, let us first observe that since the moduli stack of pointed stable curves of a given type over
$R^\dagger $
. Indeed, let us first observe that since the moduli stack of pointed stable curves of a given type over
![]() $\mathbb {Z}$
is of finite type over
$\mathbb {Z}$
is of finite type over
![]() $\mathbb {Z}$
, there exists a complete discrete valuation ring
$\mathbb {Z}$
, there exists a complete discrete valuation ring
![]() $R^\ddagger $
that is dominated by R, and whose residue field is countable such that the smooth log curve
$R^\ddagger $
that is dominated by R, and whose residue field is countable such that the smooth log curve
![]() $X^{\mathrm {log}}$
descends to the field of fractions of
$X^{\mathrm {log}}$
descends to the field of fractions of
![]() $R^\ddagger $
. Next, let us observe that since (cf., e.g., [Reference Mochizuki15, Prop. 2.3(ii)]) the geometric fundamental group “
$R^\ddagger $
. Next, let us observe that since (cf., e.g., [Reference Mochizuki15, Prop. 2.3(ii)]) the geometric fundamental group “
![]() $\Delta _{X^{\mathrm {log}}}$
” associated with the smooth log curve
$\Delta _{X^{\mathrm {log}}}$
” associated with the smooth log curve
![]() $X^{\mathrm {log}}$
(i.e., over the field of fractions of R) is naturally isomorphic to the geometric fundamental group “
$X^{\mathrm {log}}$
(i.e., over the field of fractions of R) is naturally isomorphic to the geometric fundamental group “
![]() $\Delta _{X^{\mathrm {log}}}$
” associated with the descended smooth log curve (i.e., over the field of fractions of
$\Delta _{X^{\mathrm {log}}}$
” associated with the descended smooth log curve (i.e., over the field of fractions of
![]() $R^\ddagger $
), it follows that both of these geometric fundamental groups are topologically finitely generated (cf., e.g., [Reference Mochizuki and Tamagawa23, Prop. 2.2(ii)]), and hence that there exists a countably indexed open basis
$R^\ddagger $
), it follows that both of these geometric fundamental groups are topologically finitely generated (cf., e.g., [Reference Mochizuki and Tamagawa23, Prop. 2.2(ii)]), and hence that there exists a countably indexed open basis
of characteristic open subgroups of
![]() $\Delta _{X^{\mathrm {log}}}$
. In particular, there exists a complete discrete valuation ring
$\Delta _{X^{\mathrm {log}}}$
. In particular, there exists a complete discrete valuation ring
![]() $R^\dagger $
that is dominated by R, and whose residue field is countable such that, for each positive integer n, the finite collection of finite étale coverings (which are defined by means of finitely many polynomials, with finitely many coefficients) corresponding to
$R^\dagger $
that is dominated by R, and whose residue field is countable such that, for each positive integer n, the finite collection of finite étale coverings (which are defined by means of finitely many polynomials, with finitely many coefficients) corresponding to
-
• the finite quotient
 $\Pi _{X^{\mathrm {log}}} \twoheadrightarrow Q_n$
determined by the image of the composite of the conjugation action
$\Pi _{X^{\mathrm {log}}} \twoheadrightarrow Q_n$
determined by the image of the composite of the conjugation action
 $\Pi _{X^{\mathrm {log}}} \rightarrow \mathrm {Aut}(\Delta _{X^{\mathrm {log}}})$
and the natural homomorphism
$\Pi _{X^{\mathrm {log}}} \rightarrow \mathrm {Aut}(\Delta _{X^{\mathrm {log}}})$
and the natural homomorphism
 $\mathrm {Aut}(\Delta _{X^{\mathrm {log}}}) \rightarrow \mathrm {Aut}(\Delta _{X^{\mathrm {log}}} / U_n)$
and
$\mathrm {Aut}(\Delta _{X^{\mathrm {log}}}) \rightarrow \mathrm {Aut}(\Delta _{X^{\mathrm {log}}} / U_n)$
and -
• the subgroup
 $H_n \subseteq Q_n$
obtained by forming the image of the composite of the section
$H_n \subseteq Q_n$
obtained by forming the image of the composite of the section
 $s\colon H \rightarrow \Pi _{X^{\mathrm {log}}}$
and the natural surjective homomorphism
$s\colon H \rightarrow \Pi _{X^{\mathrm {log}}}$
and the natural surjective homomorphism
 $\Pi _{X^{\mathrm {log}}} \twoheadrightarrow Q_n$
$\Pi _{X^{\mathrm {log}}} \twoheadrightarrow Q_n$
descends to the field of fractions of
![]() $R^\dagger $
.
$R^\dagger $
.
2 Discrete combinatorial anabelian geometry
In the present section, we introduce the notion of a semi-graph of temperoids of HSD-type (i.e., “hyperbolic surface decomposition type”—cf. Definition 2.3(iii)) and discuss discrete versions of the profinite results obtained in [Reference Hoshi and Mochizuki8], [Reference Hoshi and Mochizuki9], [Reference Hoshi and Mochizuki10], [Reference Hoshi and Mochizuki11]. A semi-graph of temperoids of HSD-type arises naturally from a decomposition (satisfying certain properties) of a hyperbolic topological surface and may be regarded as a discrete analogue of the notion of a semi-graph of anabelioids of PSC-type. The main technical result of the present section is Theorem 2.15, one immediate consequence of which is the following (cf. Corollary 2.19):
An isomorphism of groups between the discrete fundamental groups of a pair of semi-graphs of temperoids of HSD-type arises from an isomorphism between the semi-graphs of temperoids of HSD-type if and only if the induced isomorphism between profinite completions of fundamental groups arises from an isomorphism between the associated semi-graphs of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type.
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type.
In the present §2, let
![]() $\Sigma $
be a nonempty set of prime numbers.
$\Sigma $
be a nonempty set of prime numbers.
Definition 2.1.
-
(i) We shall refer to as a semi-graph of temperoids
 $\mathcal {G}$
a collection of data as follows:
$\mathcal {G}$
a collection of data as follows:-
• a semi-graph
 $\mathbb {G}$
(cf. the discussion at the beginning of [Reference Mochizuki17, §1]),
$\mathbb {G}$
(cf. the discussion at the beginning of [Reference Mochizuki17, §1]), -
• for each vertex v of
 $\mathbb {G}$
, a connected temperoid
$\mathbb {G}$
, a connected temperoid
 $\mathcal {G}_v$
(cf. [Reference Mochizuki17, Def. 3.1(ii)]),
$\mathcal {G}_v$
(cf. [Reference Mochizuki17, Def. 3.1(ii)]), -
• for each edge e of
 $\mathbb {G}$
, a connected temperoid
$\mathbb {G}$
, a connected temperoid
 $\mathcal {G}_e$
, together with, for each branch
$\mathcal {G}_e$
, together with, for each branch
 $b \in e$
abutting to a vertex v, a morphism of temperoids
$b \in e$
abutting to a vertex v, a morphism of temperoids
 $b_*\colon \mathcal {G}_e \rightarrow \mathcal {G}_v$
(cf. [Reference Mochizuki17, Def. 3.1(iii)]).
$b_*\colon \mathcal {G}_e \rightarrow \mathcal {G}_v$
(cf. [Reference Mochizuki17, Def. 3.1(iii)]).
We shall refer to a semi-graph of temperoids whose underlying semi-graph is connected as a connected semi-graph of temperoids. Given two semi-graphs of temperoids, there is an evident notion of (1-)morphism (cf. [Reference Mochizuki17, Def. 2.1]; [Reference Mochizuki17, Rem. 2.4.2]) between semi-graphs of temperoids.
-
-
(ii) Let
 $\mathcal {T}$
be a connected temperoid. We shall say that a connected object H of
$\mathcal {T}$
be a connected temperoid. We shall say that a connected object H of
 $\mathcal {T}$
is
$\mathcal {T}$
is
 $\Sigma $
-finite if there exists a morphism
$\Sigma $
-finite if there exists a morphism
 $J\rightarrow H$
in
$J\rightarrow H$
in
 $\mathcal {T}$
such that J is Galois (hence connected—cf. [Reference Mochizuki17, Def. 3.1(iv)]), and, moreover,
$\mathcal {T}$
such that J is Galois (hence connected—cf. [Reference Mochizuki17, Def. 3.1(iv)]), and, moreover,
 $\mathrm {Aut}_{\mathcal {T}}(J)$
is a finite group whose order is a
$\mathrm {Aut}_{\mathcal {T}}(J)$
is a finite group whose order is a
 $\Sigma $
-integer (cf. the discussion entitled “Numbers” in §0). We shall say that an object H of
$\Sigma $
-integer (cf. the discussion entitled “Numbers” in §0). We shall say that an object H of
 $\mathcal {T}$
is
$\mathcal {T}$
is
 $\Sigma $
-finite if H is isomorphic to a disjoint union of finitely many connected
$\Sigma $
-finite if H is isomorphic to a disjoint union of finitely many connected
 $\Sigma $
-finite objects. We shall say that an object H of
$\Sigma $
-finite objects. We shall say that an object H of
 $\mathcal {T}$
is a finite object if H is
$\mathcal {T}$
is a finite object if H is
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-finite. We shall write for the connected anabelioid (cf. [Reference Mochizuki16, Def. 1.1.1]) obtained by forming the full subcategory of
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-finite. We shall write for the connected anabelioid (cf. [Reference Mochizuki16, Def. 1.1.1]) obtained by forming the full subcategory of $$\begin{align*}\mathcal{T}^{\Sigma} \end{align*}$$
$$\begin{align*}\mathcal{T}^{\Sigma} \end{align*}$$
 $\mathcal {T}$
whose objects are the
$\mathcal {T}$
whose objects are the
 $\Sigma $
-finite objects of
$\Sigma $
-finite objects of
 $\mathcal {T}$
. Thus, we have a natural morphism of temperoids (cf. Remark 2.1.1 below) We shall write
$\mathcal {T}$
. Thus, we have a natural morphism of temperoids (cf. Remark 2.1.1 below) We shall write $$\begin{align*}\mathcal{T} \longrightarrow \mathcal{T}^{\Sigma}. \end{align*}$$
(cf. the discussion entitled “Numbers” in §0). Finally, we observe that if
$$\begin{align*}\mathcal{T} \longrightarrow \mathcal{T}^{\Sigma}. \end{align*}$$
(cf. the discussion entitled “Numbers” in §0). Finally, we observe that if $$ \begin{align*}\widehat{\mathcal{T}} \, \, \stackrel{\mathrm{def}}{=} \, \, \mathcal{T}^{\mathfrak{P}\mathfrak{r}\mathfrak{i}\mathfrak{m}\mathfrak{e}\mathfrak{s}} \end{align*} $$
$$ \begin{align*}\widehat{\mathcal{T}} \, \, \stackrel{\mathrm{def}}{=} \, \, \mathcal{T}^{\mathfrak{P}\mathfrak{r}\mathfrak{i}\mathfrak{m}\mathfrak{e}\mathfrak{s}} \end{align*} $$
 $\mathcal {T} = \mathcal {B}^{\mathrm {tp}}( \Pi )$
, where
$\mathcal {T} = \mathcal {B}^{\mathrm {tp}}( \Pi )$
, where
 $\Pi $
is a tempered group (cf. [Reference Mochizuki17, Def. 3.1(i)]), and “
$\Pi $
is a tempered group (cf. [Reference Mochizuki17, Def. 3.1(i)]), and “
 $\mathcal {B}^{\mathrm {tp}}(-)$
” denotes the category “
$\mathcal {B}^{\mathrm {tp}}(-)$
” denotes the category “
 $\mathcal {B}^{\mathrm {temp}}(-)$
” of the discussion at the beginning of [Reference Mochizuki17, §3], then
$\mathcal {B}^{\mathrm {temp}}(-)$
” of the discussion at the beginning of [Reference Mochizuki17, §3], then
 $\mathcal {T}^\Sigma $
may be naturally identified with
$\mathcal {T}^\Sigma $
may be naturally identified with
 $\mathcal {B}( \Pi ^\Sigma )$
, that is, the connected anabelioid (cf. [Reference Mochizuki16, Def. 1.1.1]; the discussion at the beginning of [Reference Mochizuki16, §1]) determined by the pro-
$\mathcal {B}( \Pi ^\Sigma )$
, that is, the connected anabelioid (cf. [Reference Mochizuki16, Def. 1.1.1]; the discussion at the beginning of [Reference Mochizuki16, §1]) determined by the pro-
 $\Sigma $
completion
$\Sigma $
completion
 $\Pi ^\Sigma $
of
$\Pi ^\Sigma $
of
 $\Pi $
.
$\Pi $
.
-
(iii) Let
 $\mathcal {G}$
be a semi-graph of temperoids (cf. (i)). Then, by replacing the connected temperoids “
$\mathcal {G}$
be a semi-graph of temperoids (cf. (i)). Then, by replacing the connected temperoids “
 $\mathcal {G}_{(-)}$
” corresponding to the vertices and edges “
$\mathcal {G}_{(-)}$
” corresponding to the vertices and edges “
 $(-)$
” by the connected anabelioids “
$(-)$
” by the connected anabelioids “
 $\mathcal {G}^{\Sigma }_{(-)}$
” (cf. (ii)), we obtain a semi-graph of anabelioids, which we denote by (cf. [Reference Mochizuki17, Def. 2.1]). Thus, it follows immediately from the various definitions involved that the various morphisms “
$\mathcal {G}^{\Sigma }_{(-)}$
” (cf. (ii)), we obtain a semi-graph of anabelioids, which we denote by (cf. [Reference Mochizuki17, Def. 2.1]). Thus, it follows immediately from the various definitions involved that the various morphisms “ $$ \begin{align*}\mathcal{G}^{\Sigma}\end{align*} $$
$$ \begin{align*}\mathcal{G}^{\Sigma}\end{align*} $$
 $\mathcal {G}_{(-)} \rightarrow \mathcal {G}^{\Sigma }_{(-)}$
” of (ii) determine a natural morphism of semi-graphs of temperoids (cf. Remark 2.1.1 below) We shall write
$\mathcal {G}_{(-)} \rightarrow \mathcal {G}^{\Sigma }_{(-)}$
” of (ii) determine a natural morphism of semi-graphs of temperoids (cf. Remark 2.1.1 below) We shall write $$\begin{align*}\mathcal{G} \longrightarrow \mathcal{G}^{\Sigma}. \end{align*}$$
$$\begin{align*}\mathcal{G} \longrightarrow \mathcal{G}^{\Sigma}. \end{align*}$$
 $\widehat {\mathcal {G}} \stackrel {\mathrm {def}}{=} \mathcal {G}^{\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}}$
. One verifies easily that if
$\widehat {\mathcal {G}} \stackrel {\mathrm {def}}{=} \mathcal {G}^{\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}}$
. One verifies easily that if
 $\mathcal {G}$
is a connected semi-graph of temperoids (cf. (i)), then
$\mathcal {G}$
is a connected semi-graph of temperoids (cf. (i)), then
 $\mathcal {G}^{\Sigma }$
is a connected semi-graph of anabelioids.
$\mathcal {G}^{\Sigma }$
is a connected semi-graph of anabelioids.
-
(iv) Let
 $\mathcal {G}$
be a connected semi-graph of temperoids (cf. (i)). Suppose that (the underlying semi-graph of)
$\mathcal {G}$
be a connected semi-graph of temperoids (cf. (i)). Suppose that (the underlying semi-graph of)
 $\mathcal {G}$
has at least one vertex. Then we shall write (cf. (iii); the discussion following [Reference Mochizuki17, Def. 2.1]) for the connected anabelioid determined by the connected semi-graph of anabelioids
$\mathcal {G}$
has at least one vertex. Then we shall write (cf. (iii); the discussion following [Reference Mochizuki17, Def. 2.1]) for the connected anabelioid determined by the connected semi-graph of anabelioids $$\begin{align*}\mathcal{B}(\mathcal{G}) \, \, \stackrel{\mathrm{def}}{=} \, \, \mathcal{B}(\widehat{\mathcal{G}}) \end{align*}$$
$$\begin{align*}\mathcal{B}(\mathcal{G}) \, \, \stackrel{\mathrm{def}}{=} \, \, \mathcal{B}(\widehat{\mathcal{G}}) \end{align*}$$
 $\widehat {\mathcal {G}}$
.
$\widehat {\mathcal {G}}$
.
-
(v) Let
 $\mathcal {G}$
be a semi-graph of temperoids. Then we shall write
$\mathcal {G}$
be a semi-graph of temperoids. Then we shall write
 $\mathrm {Vert}(\mathcal {G})$
,
$\mathrm {Vert}(\mathcal {G})$
,
 $\mathrm {Cusp}(\mathcal {G})$
,
$\mathrm {Cusp}(\mathcal {G})$
,
 $\mathrm {Node}(\mathcal {G})$
,
$\mathrm {Node}(\mathcal {G})$
,
 $\mathrm {Edge}(\mathcal {G})$
,
$\mathrm {Edge}(\mathcal {G})$
,
 $\mathrm {VCN}(\mathcal {G})$
,
$\mathrm {VCN}(\mathcal {G})$
,
 $\mathcal {V}$
,
$\mathcal {V}$
,
 $\mathcal {C}$
,
$\mathcal {C}$
,
 $\mathcal {N}$
,
$\mathcal {N}$
,
 $\mathcal {E}$
, and
$\mathcal {E}$
, and
 $\delta $
for the
$\delta $
for the
 $\mathrm {Vert}$
,
$\mathrm {Vert}$
,
 $\mathrm {Cusp}$
,
$\mathrm {Cusp}$
,
 $\mathrm {Node}$
,
$\mathrm {Node}$
,
 $\mathrm {Edge}$
,
$\mathrm {Edge}$
,
 $\mathrm {VCN}$
,
$\mathrm {VCN}$
,
 $\mathcal {V}$
,
$\mathcal {V}$
,
 $\mathcal {C}$
,
$\mathcal {C}$
,
 $\mathcal {N}$
,
$\mathcal {N}$
,
 $\mathcal {E}$
, and
$\mathcal {E}$
, and
 $\delta $
of Definition 1.1(i)–(iii), applied to the underlying semi-graph of
$\delta $
of Definition 1.1(i)–(iii), applied to the underlying semi-graph of
 $\mathcal {G}$
.
$\mathcal {G}$
. -
(vi) Let
 $\mathcal {G}$
be a connected semi-graph of temperoids (cf. (i)). Suppose that (the underlying semi-graph of)
$\mathcal {G}$
be a connected semi-graph of temperoids (cf. (i)). Suppose that (the underlying semi-graph of)
 $\mathcal {G}$
has at least one vertex. Then we shall write for the category whose objects are given by collections of data
$\mathcal {G}$
has at least one vertex. Then we shall write for the category whose objects are given by collections of data $$ \begin{align*}\mathcal{B}^{\mathrm{tp}}(\mathcal{G})\end{align*} $$
where v (resp. e) ranges over the elements of
$$ \begin{align*}\mathcal{B}^{\mathrm{tp}}(\mathcal{G})\end{align*} $$
where v (resp. e) ranges over the elements of $$ \begin{align*}\{S_v, \phi_e\}\end{align*} $$
$$ \begin{align*}\{S_v, \phi_e\}\end{align*} $$
 $\mathrm {Vert}(\mathcal {G})$
(resp.
$\mathrm {Vert}(\mathcal {G})$
(resp.
 $\mathrm {Edge}(\mathcal {G})$
) (cf. (v)); for each
$\mathrm {Edge}(\mathcal {G})$
) (cf. (v)); for each
 $v\in \mathrm {Vert}(\mathcal {G})$
,
$v\in \mathrm {Vert}(\mathcal {G})$
,
 $S_v$
is an object of the temperoid
$S_v$
is an object of the temperoid
 $\mathcal {G}_v$
corresponding to v; for each
$\mathcal {G}_v$
corresponding to v; for each
 $e\in \mathrm {Edge}(\mathcal {G})$
, with branches
$e\in \mathrm {Edge}(\mathcal {G})$
, with branches
 $b_1$
,
$b_1$
,
 $b_2$
abutting to vertices
$b_2$
abutting to vertices
 $v_1$
,
$v_1$
,
 $v_2$
, respectively,
$v_2$
, respectively,
 $\phi _e\colon ((b_1)_*)^*S_{v_1} \stackrel {\sim }{\rightarrow } ((b_2)_*)^*S_{v_2}$
is an isomorphism in the temperoid
$\phi _e\colon ((b_1)_*)^*S_{v_1} \stackrel {\sim }{\rightarrow } ((b_2)_*)^*S_{v_2}$
is an isomorphism in the temperoid
 $\mathcal {G}_e$
corresponding to e—and whose morphisms are given by morphisms (in the evident sense) between such collections of data. In particular, the category (i.e., connected anabelioid)
$\mathcal {G}_e$
corresponding to e—and whose morphisms are given by morphisms (in the evident sense) between such collections of data. In particular, the category (i.e., connected anabelioid)
 $\mathcal {B}(\mathcal {G})$
of (iv) may be regarded as a full subcategory of
$\mathcal {B}(\mathcal {G})$
of (iv) may be regarded as a full subcategory of $$\begin{align*}\mathcal{B}(\mathcal{G}) \subseteq \mathcal{B}^{\mathrm{tp}}(\mathcal{G}) \end{align*}$$
$$\begin{align*}\mathcal{B}(\mathcal{G}) \subseteq \mathcal{B}^{\mathrm{tp}}(\mathcal{G}) \end{align*}$$
 $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
. One verifies immediately that any object
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
. One verifies immediately that any object
 $G'$
of
$G'$
of
 $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
determines, in a natural way, a semi-graph of temperoids
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
determines, in a natural way, a semi-graph of temperoids
 $\mathcal {G}'$
, together with a morphism of semi-graphs of temperoids
$\mathcal {G}'$
, together with a morphism of semi-graphs of temperoids
 $\mathcal {G}' \rightarrow \mathcal {G}$
. We shall refer to this morphism
$\mathcal {G}' \rightarrow \mathcal {G}$
. We shall refer to this morphism
 $\mathcal {G}' \rightarrow \mathcal {G}$
as the covering of
$\mathcal {G}' \rightarrow \mathcal {G}$
as the covering of
 $\mathcal {G}$
associated with
$\mathcal {G}$
associated with
 $G'$
. We shall say that a morphism of semi-graphs of temperoids is a covering (resp. finite étale covering) of
$G'$
. We shall say that a morphism of semi-graphs of temperoids is a covering (resp. finite étale covering) of
 $\mathcal {G}$
if it factors as the post-composite of an isomorphism of semi-graphs of temperoids with the covering of
$\mathcal {G}$
if it factors as the post-composite of an isomorphism of semi-graphs of temperoids with the covering of
 $\mathcal {G}$
associated with some object of
$\mathcal {G}$
associated with some object of
 $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
(resp. of
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
(resp. of
 $\mathcal {B}(\mathcal {G}) (\subseteq \mathcal {B}^{\mathrm {tp}}(\mathcal {G}))$
). We shall say that a covering of
$\mathcal {B}(\mathcal {G}) (\subseteq \mathcal {B}^{\mathrm {tp}}(\mathcal {G}))$
). We shall say that a covering of
 $\mathcal {G}$
is connected if the underlying semi-graph of the domain of the covering is connected.
$\mathcal {G}$
is connected if the underlying semi-graph of the domain of the covering is connected.
Remark 2.1.1. Since every profinite group is tempered (cf. [Reference Mochizuki17, Def. 3.1(i)]; [Reference Mochizuki17, Rem. 3.1.1]), it follows immediately that a connected anabelioid (cf. [Reference Mochizuki16, Def. 1.1.1]) determines, in a natural way (i.e., by considering formal countable coproducts, as in the discussion entitled “Categories” in [Reference Mochizuki17, §0]), a connected temperoid (cf. [Reference Mochizuki17, Def. 3.1(ii)]). In particular, a semi-graph of anabelioids (cf. [Reference Mochizuki17, Def. 2.1]) determines, in a natural way, a semi-graph of temperoids (cf. Definition 2.1(i)). By abuse of notation, we shall often use the same notation for the connected temperoid (resp. semi-graph of temperoids) naturally associated with a connected anabelioid (resp. semi-graph of anabelioids).
Definition 2.2.
-
(i) Let T be a topological space. Then we shall say that a closed subspace of T (resp. a closed subspace of T; an open subspace of T) is a circle (resp. a closed disk; an open disk) on T if it is homeomorphic to the set
 $\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 = 1\,\}$
(resp.
$\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 = 1\,\}$
(resp.
 $\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 \leq 1\,\}$
;
$\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 \leq 1\,\}$
;
 $\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 < 1\,\}$
) equipped with the topology induced by the topology of
$\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 < 1\,\}$
) equipped with the topology induced by the topology of
 $\mathbb {R}^2$
. If
$\mathbb {R}^2$
. If
 $D \subseteq T$
is a closed disk on T, then we shall write
$D \subseteq T$
is a closed disk on T, then we shall write
 $\partial D \subseteq D$
for the circle on T determined by the boundary of D regarded as a two-dimensional topological manifold with boundary (i.e., the closed subspace of D corresponding to the closed subspace
$\partial D \subseteq D$
for the circle on T determined by the boundary of D regarded as a two-dimensional topological manifold with boundary (i.e., the closed subspace of D corresponding to the closed subspace
 $\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 = 1\,\} \subseteq \{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 \leq 1\,\}$
) and
$\{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 = 1\,\} \subseteq \{\, (s,t)\in \mathbb {R}^{2} \,| \, s^2 + t^2 \leq 1\,\}$
) and
 $D^{\circ } \stackrel {\mathrm {def}}{=} D \setminus \partial D \subseteq D$
for the open disk on T obtained by forming the complement of
$D^{\circ } \stackrel {\mathrm {def}}{=} D \setminus \partial D \subseteq D$
for the open disk on T obtained by forming the complement of
 $\partial D$
in D.
$\partial D$
in D. -
(ii) Let
 $(g,r)$
be a pair of nonnegative integers. Then we shall say that a pair
$(g,r)$
be a pair of nonnegative integers. Then we shall say that a pair
 $X = (\overline X, \{D_i\}_{i = 1}^r)$
consisting of a connected orientable compact topological surface
$X = (\overline X, \{D_i\}_{i = 1}^r)$
consisting of a connected orientable compact topological surface
 $\overline X$
of genus g and a collection of r disjoint closed disks
$\overline X$
of genus g and a collection of r disjoint closed disks
 $D_i\subseteq \overline X$
of
$D_i\subseteq \overline X$
of
 $\overline X$
(cf. (i)) is of HS-type (where the “HS” stands for “hyperbolic surface”) if
$\overline X$
(cf. (i)) is of HS-type (where the “HS” stands for “hyperbolic surface”) if
 $2g-2+r> 0$
.
$2g-2+r> 0$
. -
(iii) Let
 $X = (\overline X, \{D_i\}_{i = 1}^r)$
be a pair of HS-type (cf. (ii)). Then we shall write
$X = (\overline X, \{D_i\}_{i = 1}^r)$
be a pair of HS-type (cf. (ii)). Then we shall write  $$\begin{align*}U_X \, \, \stackrel{\mathrm{def}}{=} \, \, \overline X \setminus \big(\bigcup_{i=1}^{r}D_i^{\circ}\big) \end{align*}$$
$$\begin{align*}U_X \, \, \stackrel{\mathrm{def}}{=} \, \, \overline X \setminus \big(\bigcup_{i=1}^{r}D_i^{\circ}\big) \end{align*}$$
(cf. (i)) and refer to
 $U_X$
as the interior of X. We shall refer to a circle on
$U_X$
as the interior of X. We shall refer to a circle on
 $U_X$
determined by some
$U_X$
determined by some
 $\partial D_i \subseteq U_X$
(cf. (i)) as a cusp of
$\partial D_i \subseteq U_X$
(cf. (i)) as a cusp of
 $U_X$
, or alternatively, X. Write
$U_X$
, or alternatively, X. Write
 $\partial U_X \subseteq U_X$
for the union of the cusps of
$\partial U_X \subseteq U_X$
for the union of the cusps of
 $U_X$
;
$U_X$
;
 $I_X$
for the group of homeomorphisms
$I_X$
for the group of homeomorphisms
 $\phi \colon \overline X \stackrel {\sim }{\rightarrow } \overline X$
such that
$\phi \colon \overline X \stackrel {\sim }{\rightarrow } \overline X$
such that
 $\phi $
restricts to the identity on
$\phi $
restricts to the identity on
 $U_X$
. Suppose that
$U_X$
. Suppose that
 $Y=(\overline {Y}, \{E_i\}^s_{j=1})$
is also a pair of HS-type. Then we define an isomorphism
$Y=(\overline {Y}, \{E_i\}^s_{j=1})$
is also a pair of HS-type. Then we define an isomorphism
 $X \stackrel {\sim }{\rightarrow } Y$
of pairs of HS-type to be an
$X \stackrel {\sim }{\rightarrow } Y$
of pairs of HS-type to be an
 $I_X$
-orbit of homeomorphisms
$I_X$
-orbit of homeomorphisms
 $\overline X \stackrel {\sim }{\rightarrow } \overline {Y}$
such that each homeomorphism
$\overline X \stackrel {\sim }{\rightarrow } \overline {Y}$
such that each homeomorphism
 $\psi $
that belongs to the
$\psi $
that belongs to the
 $I_X$
-orbit induces a homeomorphism
$I_X$
-orbit induces a homeomorphism
 $U_X \stackrel {\sim }{\rightarrow } U_Y$
.
$U_X \stackrel {\sim }{\rightarrow } U_Y$
. -
(iv) Let
 $X = (\overline X, \{D_i\}_{i = 1}^r)$
be a pair of HS-type (cf. (ii)) and
$X = (\overline X, \{D_i\}_{i = 1}^r)$
be a pair of HS-type (cf. (ii)) and
 $\{Y_j\}_{j\in J}$
a finite collection of pairs of HS-type. For each
$\{Y_j\}_{j\in J}$
a finite collection of pairs of HS-type. For each
 $j\in J$
, let
$j\in J$
, let
 $\iota _j \colon U_{Y_{j}} \hookrightarrow U_X$
(cf. (iii)) be a local immersion (i.e., a map that restricts to a homeomorphism between some open neighborhood of each point of the domain and the image of the open neighborhood, equipped with the induced topology, in the codomain) of topological spaces. Then we shall say that a pair
$\iota _j \colon U_{Y_{j}} \hookrightarrow U_X$
(cf. (iii)) be a local immersion (i.e., a map that restricts to a homeomorphism between some open neighborhood of each point of the domain and the image of the open neighborhood, equipped with the induced topology, in the codomain) of topological spaces. Then we shall say that a pair
 $(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
is an HS-decomposition of X if the following conditions are satisfied:
$(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
is an HS-decomposition of X if the following conditions are satisfied:-
(1)
 $U_X = \bigcup _{j\in J} \iota _j(U_{Y_{j}})$
.
$U_X = \bigcup _{j\in J} \iota _j(U_{Y_{j}})$
. -
(2) For any
 $j\in J$
, the complement of the diagonal in
$j\in J$
, the complement of the diagonal in
 $U_{Y_{j}} \times _{U_X} U_{Y_{j}}$
is a disjoint union of circles, each of which maps homeomorphically, via the two projections to
$U_{Y_{j}} \times _{U_X} U_{Y_{j}}$
is a disjoint union of circles, each of which maps homeomorphically, via the two projections to
 $U_{Y_{j}}$
, to two distinct cusps of
$U_{Y_{j}}$
, to two distinct cusps of
 $U_{Y_{j}}$
(cf. (iii)). (Thus, by “Brouwer invariance of domain,” it follows that
$U_{Y_{j}}$
(cf. (iii)). (Thus, by “Brouwer invariance of domain,” it follows that
 $\iota _j$
restricts to an open immersion on the complement of the cusps of
$\iota _j$
restricts to an open immersion on the complement of the cusps of
 $U_{Y_{j}}$
.)
$U_{Y_{j}}$
.) -
(3) For any j,
 $j' \in J$
such that
$j' \in J$
such that
 $j\neq j'$
, every connected component of
$j\neq j'$
, every connected component of
 $U_{Y_{j}} \times _{U_X} U_{Y_{j'}} $
projects homeomorphically onto cusps of
$U_{Y_{j}} \times _{U_X} U_{Y_{j'}} $
projects homeomorphically onto cusps of
 $U_{Y_{j}}$
and
$U_{Y_{j}}$
and
 $U_{Y_{j'}}$
.
$U_{Y_{j'}}$
. -
(4) For any (i.e., possibly equal) j,
 $j'\in J$
, we shall refer to a circle of
$j'\in J$
, we shall refer to a circle of
 $U_{Y_{j}}\times _{U_X} U_{Y_{j'}}$
that forms a connected component of
$U_{Y_{j}}\times _{U_X} U_{Y_{j'}}$
that forms a connected component of
 $U_{Y_{j}}\times _{U_X} U_{Y_{j'}}$
as a pre-node (of the HS-decomposition
$U_{Y_{j}}\times _{U_X} U_{Y_{j'}}$
as a pre-node (of the HS-decomposition
 $(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
) and to the cusps of
$(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
) and to the cusps of
 $U_{Y_{j}}$
,
$U_{Y_{j}}$
,
 $U_{Y_{j'}}$
that arise as the images of such a pre-node via the projections to
$U_{Y_{j'}}$
that arise as the images of such a pre-node via the projections to
 $U_{Y_{j}}$
,
$U_{Y_{j}}$
,
 $U_{Y_{j'}}$
as the branch cusps of the pre-node. Then we suppose further that every pre-node maps injectively into
$U_{Y_{j'}}$
as the branch cusps of the pre-node. Then we suppose further that every pre-node maps injectively into
 $U_X$
, and that the image in
$U_X$
, and that the image in
 $U_X$
of the pre-node has empty intersection with
$U_X$
of the pre-node has empty intersection with
 $\partial U_X$
, as well as with the image via
$\partial U_X$
, as well as with the image via
 $\iota _{j"}$
, for
$\iota _{j"}$
, for
 $j"\in J$
, of any cusp of
$j"\in J$
, of any cusp of
 $U_{Y_{j"}}$
which is not a branch cusp of the pre-node. We shall refer to the image in
$U_{Y_{j"}}$
which is not a branch cusp of the pre-node. We shall refer to the image in
 $U_X$
of a pre-node as a node (of the HS-decomposition
$U_X$
of a pre-node as a node (of the HS-decomposition
 $(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
). Thus, (one verifies easily that) every node arises from a unique pre-node. We shall refer to the branch cusps of the pre-node that gives rise to a node as the branch cusps of the node. (Thus, by “Brouwer invariance of domain,” it follows that, for any pre-node of
$(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
). Thus, (one verifies easily that) every node arises from a unique pre-node. We shall refer to the branch cusps of the pre-node that gives rise to a node as the branch cusps of the node. (Thus, by “Brouwer invariance of domain,” it follows that, for any pre-node of
 $U_{Y_{j}}\times _{U_X} U_{Y_{j'}}$
, the maps
$U_{Y_{j}}\times _{U_X} U_{Y_{j'}}$
, the maps
 $\iota _j$
,
$\iota _j$
,
 $\iota _{j'}$
determine a homeomorphism of the topological space obtained by gluing, along the associated node, suitable open neighborhoods of the branch cusps of
$\iota _{j'}$
determine a homeomorphism of the topological space obtained by gluing, along the associated node, suitable open neighborhoods of the branch cusps of
 $U_{Y_{j}}$
,
$U_{Y_{j}}$
,
 $U_{Y_{j'}}$
onto the topological space constituted by a suitable open neighborhood of the associated node in
$U_{Y_{j'}}$
onto the topological space constituted by a suitable open neighborhood of the associated node in
 $U_X$
.)
$U_X$
.) -
(5) For any
 $j\in J$
, every cusp of
$j\in J$
, every cusp of
 $U_{Y_{j}}$
maps homeomorphically onto either a cusp of
$U_{Y_{j}}$
maps homeomorphically onto either a cusp of
 $U_X$
or a node of
$U_X$
or a node of
 $(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
(cf. (4)). Moreover, every cusp of
$(\{Y_j\}_{j\in J}, \{\iota _j\}_{j\in J})$
(cf. (4)). Moreover, every cusp of
 $U_X$
arises in this way from a cusp of
$U_X$
arises in this way from a cusp of
 $U_{Y_{j}}$
for some (necessarily uniquely determined)
$U_{Y_{j}}$
for some (necessarily uniquely determined)
 $j\in J$
. (Thus, by “Brouwer invariance of domain”—together with a suitable gluing argument as in (4)—it follows that every cusp of
$j\in J$
. (Thus, by “Brouwer invariance of domain”—together with a suitable gluing argument as in (4)—it follows that every cusp of
 $U_X$
admits an open neighborhood that arises, for some
$U_X$
admits an open neighborhood that arises, for some
 $j\in J$
, as the homeomorphic image, via
$j\in J$
, as the homeomorphic image, via
 $\iota _j$
, of a suitable open neighborhood of a cusp of
$\iota _j$
, of a suitable open neighborhood of a cusp of
 $U_{Y_{j}}$
.)
$U_{Y_{j}}$
.)
If
 $(\{Y_j\}, \{\iota _j\})$
is an HS-decomposition of X, then we shall refer to the triple
$(\{Y_j\}, \{\iota _j\})$
is an HS-decomposition of X, then we shall refer to the triple
 $(X, \{Y_j\}, \{\iota _j\})$
as a collection of HSD-data (where the “HSD” stands for “hyperbolic surface decomposition”). If
$(X, \{Y_j\}, \{\iota _j\})$
as a collection of HSD-data (where the “HSD” stands for “hyperbolic surface decomposition”). If
 $\mathbb {X} = (X, \{Y_j\}, \{\iota _j\})$
is a collection of HSD-data, then we shall refer to the topological space
$\mathbb {X} = (X, \{Y_j\}, \{\iota _j\})$
is a collection of HSD-data, then we shall refer to the topological space
 $U_X$
(resp. [the closed subspace of
$U_X$
(resp. [the closed subspace of
 $U_X$
corresponding to] an element of the [finite] set
$U_X$
corresponding to] an element of the [finite] set
 $\{Y_j\}$
; a cusp of
$\{Y_j\}$
; a cusp of
 $U_X$
; a node of
$U_X$
; a node of
 $(\{Y_j\}, \{\iota _j\})$
[cf. (4)]) as the underlying surface (resp. a vertex; a cusp; a node) of
$(\{Y_j\}, \{\iota _j\})$
[cf. (4)]) as the underlying surface (resp. a vertex; a cusp; a node) of
 $\mathbb {X}$
. Also, we shall refer to a cusp or node of
$\mathbb {X}$
. Also, we shall refer to a cusp or node of
 $\mathbb {X}$
as an edge of
$\mathbb {X}$
as an edge of
 $\mathbb {X}$
.
$\mathbb {X}$
. -
Definition 2.3. Let
![]() $\mathbb {X} = (X, \{Y_j\}, \{\iota _j\})$
be a collection of HSD-data (cf. Definition 2.2(iv)).
$\mathbb {X} = (X, \{Y_j\}, \{\iota _j\})$
be a collection of HSD-data (cf. Definition 2.2(iv)).
-
(i) We shall refer to the semi-graph
defined as follows as the dual semi-graph of $$ \begin{align*}\mathbb{G}_{\mathbb{X}}\end{align*} $$
$$ \begin{align*}\mathbb{G}_{\mathbb{X}}\end{align*} $$
 $\mathbb {X}$
: we take the set of vertices (resp. open edges; closed edges) of
$\mathbb {X}$
: we take the set of vertices (resp. open edges; closed edges) of
 $\mathbb {G}_{\mathbb {X}}$
is the (finite) set of vertices (resp. cusps; nodes) of
$\mathbb {G}_{\mathbb {X}}$
is the (finite) set of vertices (resp. cusps; nodes) of
 $\mathbb {X}$
(cf. Definition 2.2(iv)). For a vertex v and an edge e of
$\mathbb {X}$
(cf. Definition 2.2(iv)). For a vertex v and an edge e of
 $\mathbb {X}$
, we take the set of branches of e that abut to v to be the set of natural inclusions (i.e., that arise from
$\mathbb {X}$
, we take the set of branches of e that abut to v to be the set of natural inclusions (i.e., that arise from
 $\mathbb {X}$
—cf. Definition 2.2(iv)) from the edge of
$\mathbb {X}$
—cf. Definition 2.2(iv)) from the edge of
 $\mathbb {X}$
corresponding to e into the topological space
$\mathbb {X}$
corresponding to e into the topological space
 $U_{Y_{j}}$
associated with the
$U_{Y_{j}}$
associated with the
 $Y_j$
corresponding to the vertex v.
$Y_j$
corresponding to the vertex v.
-
(ii) We shall refer to the connected semi-graph
of temperoids (cf. Definition 2.1(i)) defined as follows as the semi-graph of temperoids associated with $$ \begin{align*}\mathcal{G}_{\mathbb{X}}\end{align*} $$
$$ \begin{align*}\mathcal{G}_{\mathbb{X}}\end{align*} $$
 $\mathbb {X}$
: we take the underlying semi-graph of
$\mathbb {X}$
: we take the underlying semi-graph of
 $\mathcal {G}_{\mathbb {X}}$
to be
$\mathcal {G}_{\mathbb {X}}$
to be
 $\mathbb {G}_{\mathbb {X}}$
(cf. (i)). For each vertex v of
$\mathbb {G}_{\mathbb {X}}$
(cf. (i)). For each vertex v of
 $\mathbb {G}_{\mathbb {X}}$
, we take the connected temperoid of
$\mathbb {G}_{\mathbb {X}}$
, we take the connected temperoid of
 $\mathcal {G}_{\mathbb {X}}$
corresponding to v to be the connected temperoid determined by the category of topological coverings with countably many connected components of the topological space
$\mathcal {G}_{\mathbb {X}}$
corresponding to v to be the connected temperoid determined by the category of topological coverings with countably many connected components of the topological space
 $U_{Y_{j}}$
(cf. Definition 2.2(iii)) associated with the
$U_{Y_{j}}$
(cf. Definition 2.2(iii)) associated with the
 $Y_j$
corresponding to the vertex v. For each edge e of
$Y_j$
corresponding to the vertex v. For each edge e of
 $\mathbb {G}_{\mathbb {X}}$
, we take the connected temperoid of
$\mathbb {G}_{\mathbb {X}}$
, we take the connected temperoid of
 $\mathcal {G}_{\mathbb {X}}$
corresponding to e to be the connected temperoid determined by the category of topological coverings with countably many connected components of the circle (cf. Definition 2.2(i)) on
$\mathcal {G}_{\mathbb {X}}$
corresponding to e to be the connected temperoid determined by the category of topological coverings with countably many connected components of the circle (cf. Definition 2.2(i)) on
 $U_X$
corresponding to the edge e. For each branch b of
$U_X$
corresponding to the edge e. For each branch b of
 $\mathbb {G}_{\mathbb {X}}$
, we take the morphism of temperoids corresponding to b to be the morphism obtained by pulling back topological coverings of the topological spaces under consideration.
$\mathbb {G}_{\mathbb {X}}$
, we take the morphism of temperoids corresponding to b to be the morphism obtained by pulling back topological coverings of the topological spaces under consideration.
-
(iii) We shall say that a semi-graph of temperoids is of HSD-type if it is isomorphic to the semi-graph of temperoids associated with some collection of HSD-data (cf. (ii)).
Example 2.4 (Semi-graphs of temperoids of HSD-type associated with stable log curves).
Let
![]() $(g,r)$
be a pair of nonnegative integers such that
$(g,r)$
be a pair of nonnegative integers such that
![]() $2g-2+r>0$
. Write
$2g-2+r>0$
. Write
![]() $S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(\mathbb {C})$
. In the following, we shall apply the notation and terminology of the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0].
$S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(\mathbb {C})$
. In the following, we shall apply the notation and terminology of the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0].
-
(i) Let
 $S \rightarrow (\overline {\mathcal {M}}_{g,r})_{\mathbb {C}}$
be a
$S \rightarrow (\overline {\mathcal {M}}_{g,r})_{\mathbb {C}}$
be a
 $\mathbb {C}$
-valued point of
$\mathbb {C}$
-valued point of
 $(\overline {\mathcal {M}}_{g,r})_{\mathbb {C}}$
. Write
$(\overline {\mathcal {M}}_{g,r})_{\mathbb {C}}$
. Write
 $S^{\mathrm {log}}$
for the fs log scheme obtained by equipping S with the log structure induced by the log structure of
$S^{\mathrm {log}}$
for the fs log scheme obtained by equipping S with the log structure induced by the log structure of
 $(\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
;
$(\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
;
 $X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
for the stable log curve over
$X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
for the stable log curve over
 $S^{\mathrm {log}}$
corresponding to the resulting strict (
$S^{\mathrm {log}}$
corresponding to the resulting strict (
 $1$
-)morphism
$1$
-)morphism
 $S^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
; d for the rank of the group-characteristic of
$S^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
; d for the rank of the group-characteristic of
 $S^{\mathrm {log}}$
(cf. [Reference Mochizuki and Tamagawa23, Def. 5.1(i)]), that is, the number of nodes of
$S^{\mathrm {log}}$
(cf. [Reference Mochizuki and Tamagawa23, Def. 5.1(i)]), that is, the number of nodes of
 $X^{\mathrm {log}}$
;
$X^{\mathrm {log}}$
;
 $X^{\mathrm {log}}_{\mathrm {an}} \rightarrow S^{\mathrm {log}}_{\mathrm {an}}$
for the morphism of fs log analytic spaces determined by the morphism
$X^{\mathrm {log}}_{\mathrm {an}} \rightarrow S^{\mathrm {log}}_{\mathrm {an}}$
for the morphism of fs log analytic spaces determined by the morphism
 $X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
;
$X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
;
 $X_{\mathrm {an}} \rightarrow S_{\mathrm {an}}$
for the underlying morphism of analytic spaces of
$X_{\mathrm {an}} \rightarrow S_{\mathrm {an}}$
for the underlying morphism of analytic spaces of
 $X^{\mathrm {log}}_{\mathrm {an}} \rightarrow S^{\mathrm {log}}_{\mathrm {an}}$
;
$X^{\mathrm {log}}_{\mathrm {an}} \rightarrow S^{\mathrm {log}}_{\mathrm {an}}$
;
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
,
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
,
 $S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
for the respective topological spaces “
$S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
for the respective topological spaces “
 $X^{\mathrm {log}}$
” defined in [Reference Kato and Nakayama12, (1.2)] in the case where we take the “X” of [Reference Kato and Nakayama12, (1.2)] to be
$X^{\mathrm {log}}$
” defined in [Reference Kato and Nakayama12, (1.2)] in the case where we take the “X” of [Reference Kato and Nakayama12, (1.2)] to be
 $X_{\mathrm {an}}^{\mathrm {log}}$
,
$X_{\mathrm {an}}^{\mathrm {log}}$
,
 $S_{\mathrm {an}}^{\mathrm {log}}$
, that is, for
$S_{\mathrm {an}}^{\mathrm {log}}$
, that is, for
 $T \in \{X,S\}$
,
$T \in \{X,S\}$
,  $$\begin{align*}T_{\mathrm{an}}^{\mathrm{log}}(\mathbb{C}) \, \, \stackrel{\mathrm{def}}{=} \, \, \{\,(t,h) \,| \, t \in T_{\mathrm{an}}, \, h\in\mathrm{Hom}_{\mathrm{gp}}(M_{T_{\mathrm{an}},t}^{\mathrm{gp}},\mathbb{S}^1) \text{ such that } \end{align*}$$
—where we write
$$\begin{align*}T_{\mathrm{an}}^{\mathrm{log}}(\mathbb{C}) \, \, \stackrel{\mathrm{def}}{=} \, \, \{\,(t,h) \,| \, t \in T_{\mathrm{an}}, \, h\in\mathrm{Hom}_{\mathrm{gp}}(M_{T_{\mathrm{an}},t}^{\mathrm{gp}},\mathbb{S}^1) \text{ such that } \end{align*}$$
—where we write $$\begin{align*}h(f) = f(t) / |f(t)| \text{ for every } f \in \mathcal{O}_{T_{\mathrm{an}},t}^{\times} \subseteq M_{T_{\mathrm{an}},t}^{\mathrm{gp}} \,\} \end{align*}$$
$$\begin{align*}h(f) = f(t) / |f(t)| \text{ for every } f \in \mathcal{O}_{T_{\mathrm{an}},t}^{\times} \subseteq M_{T_{\mathrm{an}},t}^{\mathrm{gp}} \,\} \end{align*}$$
 $\mathbb {S}^1 \stackrel {\mathrm {def}}{=} \{ \, u \in \mathbb {C} \,| \, |u| = 1\,\}$
and
$\mathbb {S}^1 \stackrel {\mathrm {def}}{=} \{ \, u \in \mathbb {C} \,| \, |u| = 1\,\}$
and
 $M_{T_{\mathrm {an}}}$
for the sheaf of monoids on
$M_{T_{\mathrm {an}}}$
for the sheaf of monoids on
 $T_{\mathrm {an}}$
that defines the log structure of
$T_{\mathrm {an}}$
that defines the log structure of
 $T_{\mathrm {an}}^{\mathrm {log}}$
. Then, by considering the functoriality discussed in [Reference Kato and Nakayama12, (1.2.5)] and the respective maps
$T_{\mathrm {an}}^{\mathrm {log}}$
. Then, by considering the functoriality discussed in [Reference Kato and Nakayama12, (1.2.5)] and the respective maps
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow X_{\mathrm {an}}$
,
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow X_{\mathrm {an}}$
,
 $S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow S_{\mathrm {an}}$
induced by the first projections, we obtain a commutative diagram of topological spaces and continuous maps Now one verifies immediately from the various definitions involved that
$S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow S_{\mathrm {an}}$
induced by the first projections, we obtain a commutative diagram of topological spaces and continuous maps Now one verifies immediately from the various definitions involved that
 $S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
is homeomorphic to a product
$S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
is homeomorphic to a product
 $(\mathbb {S}^1)^{\times d}$
of d copies of
$(\mathbb {S}^1)^{\times d}$
of d copies of
 $\mathbb {S}^1$
; moreover, it follows from [Reference Nakayama and Ogus24, Th. 5.1] that the left-hand vertical arrow of the above diagram is a topological fiber bundle. Let
$\mathbb {S}^1$
; moreover, it follows from [Reference Nakayama and Ogus24, Th. 5.1] that the left-hand vertical arrow of the above diagram is a topological fiber bundle. Let
 $s \in S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
. Thus, since (one verifies easily that)
$s \in S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
. Thus, since (one verifies easily that)
 $(\mathbb {S}^1)^{\times d}$
is an Eilenberg–Maclane space (i.e., its universal covering space is contractible), the left-hand vertical arrow of the above diagram determines an exact sequence where we write
$(\mathbb {S}^1)^{\times d}$
is an Eilenberg–Maclane space (i.e., its universal covering space is contractible), the left-hand vertical arrow of the above diagram determines an exact sequence where we write $$\begin{align*}1 \longrightarrow \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) \longrightarrow \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \longrightarrow \pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}^{\oplus d}) \, \, \longrightarrow 1, \end{align*}$$
$$\begin{align*}1 \longrightarrow \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) \longrightarrow \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \longrightarrow \pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}^{\oplus d}) \, \, \longrightarrow 1, \end{align*}$$
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
for the fiber of the left-hand vertical arrow
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
for the fiber of the left-hand vertical arrow
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
of the above diagram at s, which thus determines an outer action Write
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
of the above diagram at s, which thus determines an outer action Write $$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}^{\oplus d}) \longrightarrow \mathrm{Out}( \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) ). \end{align*}$$
$$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}^{\oplus d}) \longrightarrow \mathrm{Out}( \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) ). \end{align*}$$
 $N \subseteq X_{\mathrm {an}}$
for the finite subset consisting of the nodes of
$N \subseteq X_{\mathrm {an}}$
for the finite subset consisting of the nodes of
 $X_{\mathrm {an}}^{\mathrm {log}}$
,
$X_{\mathrm {an}}^{\mathrm {log}}$
,
 $C \subseteq X_{\mathrm {an}}$
for the finite subset consisting of the cusps of
$C \subseteq X_{\mathrm {an}}$
for the finite subset consisting of the cusps of
 $X_{\mathrm {an}}^{\mathrm {log}}$
,
$X_{\mathrm {an}}^{\mathrm {log}}$
,
 $U \stackrel {\mathrm {def}}{=} X_{\mathrm {an}}\setminus (N\cup C) \subseteq X_{\mathrm {an}}$
, and
$U \stackrel {\mathrm {def}}{=} X_{\mathrm {an}}\setminus (N\cup C) \subseteq X_{\mathrm {an}}$
, and
 $\pi _0(U)$
for the finite set of connected components of U. For each node
$\pi _0(U)$
for the finite set of connected components of U. For each node
 $x \in N$
(resp. cusp
$x \in N$
(resp. cusp
 $y \in C$
; connected component
$y \in C$
; connected component
 $F \in \pi _0(U)$
of U), write
$F \in \pi _0(U)$
of U), write
 $C_{x}$
(resp.
$C_{x}$
(resp.
 $C_{y}$
;
$C_{y}$
;
 $Y_{F}$
)
$Y_{F}$
)
 $\subseteq X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
for the closure of the inverse image of
$\subseteq X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
for the closure of the inverse image of
 $\{x\}$
(resp.
$\{x\}$
(resp.
 $\{y\}$
; F)
$\{y\}$
; F)
 $\subseteq X_{\mathrm {an}}$
via the composite
$\subseteq X_{\mathrm {an}}$
via the composite
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s \stackrel {\mathrm {pr}_{1}}{\rightarrow } X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow X_{\mathrm {an}}$
—where the second arrow is the upper horizontal arrow of the above diagram. Then one verifies immediately from the various definitions involved that there exists a uniquely determined, up to unique isomorphism (in the evident sense), collection of data as follows:
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s \stackrel {\mathrm {pr}_{1}}{\rightarrow } X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}) \rightarrow X_{\mathrm {an}}$
—where the second arrow is the upper horizontal arrow of the above diagram. Then one verifies immediately from the various definitions involved that there exists a uniquely determined, up to unique isomorphism (in the evident sense), collection of data as follows:
-
• a pair of HS-type
 $Z = (\overline Z, \{D_i\}_{i=1}^r)$
of type
$Z = (\overline Z, \{D_i\}_{i=1}^r)$
of type
 $(g,r)$
(cf. Definition 2.2(ii));
$(g,r)$
(cf. Definition 2.2(ii)); -
• a homeomorphism
 $\phi \colon X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s \stackrel {\sim }{\rightarrow } U_Z$
of
$\phi \colon X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s \stackrel {\sim }{\rightarrow } U_Z$
of
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
with the interior
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
with the interior
 $U_Z$
of Z (cf. Definition 2.2(iii)) such that
$U_Z$
of Z (cf. Definition 2.2(iii)) such that
 $\phi $
restricts to a homeomorphism of
$\phi $
restricts to a homeomorphism of
 $\bigsqcup _{y\in C} \, C_{y} \subseteq X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
with
$\bigsqcup _{y\in C} \, C_{y} \subseteq X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
with
 $ \partial U_Z = \bigsqcup _{i=1}^r \, \partial D_i \subseteq U_Z$
(cf. Definition 2.2(iii)).
$ \partial U_Z = \bigsqcup _{i=1}^r \, \partial D_i \subseteq U_Z$
(cf. Definition 2.2(iii)).
Moreover, there exists a uniquely determined, up to unique isomorphism (in the evident sense), HS-decomposition of Z (cf. Definition 2.2(iv)) such that the set of vertices (resp. nodes; cusps) (cf. Definition 2.2(iv)) of the resulting collection of HSD-data (cf. Definition 2.2(iv)) is
 $\{\phi (Y_{F})\}_{F \in \pi _0(U)}$
(resp.
$\{\phi (Y_{F})\}_{F \in \pi _0(U)}$
(resp.
 $\{\phi (C_{x})\}_{x\in N}$
;
$\{\phi (C_{x})\}_{x\in N}$
;
 $\{\phi (C_{y})\}_{y\in C} $
). We shall write for the semi-graph of temperoids of HSD-type associated with this collection of HSD-data (cf. Definition 2.3(ii)) and refer to
$\{\phi (C_{y})\}_{y\in C} $
). We shall write for the semi-graph of temperoids of HSD-type associated with this collection of HSD-data (cf. Definition 2.3(ii)) and refer to $$ \begin{align*}\mathcal{G}_{X^{\mathrm{log}}}\end{align*} $$
$$ \begin{align*}\mathcal{G}_{X^{\mathrm{log}}}\end{align*} $$
 $\mathcal {G}_{X^{\mathrm {log}}}$
as the semi-graph of temperoids of HSD-type associated with
$\mathcal {G}_{X^{\mathrm {log}}}$
as the semi-graph of temperoids of HSD-type associated with
 $X^{\mathrm {log}}$
. Then one verifies immediately from the functoriality discussed in [Reference Kato and Nakayama12, (1.2.5)] applied to the vertices, nodes, and cusps of the data under consideration, that the locally trivial fibration
$X^{\mathrm {log}}$
. Then one verifies immediately from the functoriality discussed in [Reference Kato and Nakayama12, (1.2.5)] applied to the vertices, nodes, and cusps of the data under consideration, that the locally trivial fibration
 $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})\rightarrow S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
determines an action which is compatible, in the evident sense, with the outer action
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})\rightarrow S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
determines an action which is compatible, in the evident sense, with the outer action $$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}^{\oplus d}) \longrightarrow \mathrm{Aut}( \mathcal{G}_{X^{\mathrm{log}}} ) , \end{align*}$$
discussed above.
$$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}^{\oplus d}) \longrightarrow \mathrm{Aut}( \mathcal{G}_{X^{\mathrm{log}}} ) , \end{align*}$$
discussed above. $$ \begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \longrightarrow \mathrm{Out}( \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) )\end{align*} $$
$$ \begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \longrightarrow \mathrm{Out}( \pi_1(X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) )\end{align*} $$
-
-
(ii) Let
 $S^{\mathrm {log}}$
be the fs log scheme obtained by equipping S with the log structure given by the fs chart
$S^{\mathrm {log}}$
be the fs log scheme obtained by equipping S with the log structure given by the fs chart
 $\mathbb {N} \ni 1 \mapsto 0\in \mathbb {C}$
and
$\mathbb {N} \ni 1 \mapsto 0\in \mathbb {C}$
and
 $X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
a stable log curve of type
$X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
a stable log curve of type
 $(g,r)$
over
$(g,r)$
over
 $S^{\mathrm {log}}$
(cf. [Reference Mochizuki18, Exam. 2.5] in the case where
$S^{\mathrm {log}}$
(cf. [Reference Mochizuki18, Exam. 2.5] in the case where
 $ k = \mathbb {C}$
). Then one verifies easily that the classifying (
$ k = \mathbb {C}$
). Then one verifies easily that the classifying (
 $1$
-)morphism
$1$
-)morphism
 $S^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
of
$S^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
of
 $X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
factors as a composite
$X^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
factors as a composite
 $S^{\mathrm {log}} \rightarrow T^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
—where the first arrow is a morphism that induces an isomorphism between the underlying schemes, and the second arrow is strict—and, moreover, if we write
$S^{\mathrm {log}} \rightarrow T^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
—where the first arrow is a morphism that induces an isomorphism between the underlying schemes, and the second arrow is strict—and, moreover, if we write
 $Y^{\mathrm {log}} \rightarrow T^{\mathrm {log}}$
for the stable log curve determined by the strict (
$Y^{\mathrm {log}} \rightarrow T^{\mathrm {log}}$
for the stable log curve determined by the strict (
 $1$
-)morphism
$1$
-)morphism
 $T^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
, then we have a natural isomorphism over
$T^{\mathrm {log}} \rightarrow (\overline {\mathcal {M}}_{g,r}^{\mathrm {log}})_{\mathbb {C}}$
, then we have a natural isomorphism over
 $S^{\mathrm {log}}$
We shall write
$S^{\mathrm {log}}$
We shall write $$\begin{align*}X^{\mathrm{log}} \stackrel{\sim}{\longrightarrow} Y^{\mathrm{log}}\times_{T^{\mathrm{log}}} S^{\mathrm{log}}. \end{align*}$$
(cf. (i)) and refer to
$$\begin{align*}X^{\mathrm{log}} \stackrel{\sim}{\longrightarrow} Y^{\mathrm{log}}\times_{T^{\mathrm{log}}} S^{\mathrm{log}}. \end{align*}$$
(cf. (i)) and refer to $$ \begin{align*}\mathcal{G}_{X^{\mathrm{log}}} \, \, \stackrel{\mathrm{def}}{=} \, \, \mathcal{G}_{Y^{\mathrm{log}}}\end{align*} $$
$$ \begin{align*}\mathcal{G}_{X^{\mathrm{log}}} \, \, \stackrel{\mathrm{def}}{=} \, \, \mathcal{G}_{Y^{\mathrm{log}}}\end{align*} $$
 $\mathcal {G}_{X^{\mathrm {log}}}$
as the semi-graph of temperoids of HSD-type associated with
$\mathcal {G}_{X^{\mathrm {log}}}$
as the semi-graph of temperoids of HSD-type associated with
 $X^{\mathrm {log}}$
. Then, by pulling back the action of the second to last display of (i) via the homomorphism
$X^{\mathrm {log}}$
. Then, by pulling back the action of the second to last display of (i) via the homomorphism
 $\pi _1(S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}))\rightarrow \pi _1(T^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}))$
induced by the morphism
$\pi _1(S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}))\rightarrow \pi _1(T^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C}))$
induced by the morphism
 $S^{\mathrm {log}} \rightarrow T^{\mathrm {log}}$
, we obtain an action together with a compatible outer action
$S^{\mathrm {log}} \rightarrow T^{\mathrm {log}}$
, we obtain an action together with a compatible outer action $$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}) \longrightarrow \mathrm{Aut}( \mathcal{G}_{X^{\mathrm{log}}} ) , \end{align*}$$
$$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \, \, (\cong \mathbb{Z}) \longrightarrow \mathrm{Aut}( \mathcal{G}_{X^{\mathrm{log}}} ) , \end{align*}$$
 $$ \begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \longrightarrow \mathrm{Out}( \pi_1( X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) ). \end{align*} $$
$$ \begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \longrightarrow \mathrm{Out}( \pi_1( X^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})|_s) ). \end{align*} $$
Remark 2.4.1. One verifies easily that the discussion of Example 2.4(ii) generalizes immediately to the case of arbitrary fs log schemes
![]() $S^{\mathrm {log}}$
with underlying scheme
$S^{\mathrm {log}}$
with underlying scheme
![]() $S=\mathrm {Spec}(\mathbb {C})$
.
$S=\mathrm {Spec}(\mathbb {C})$
.
Proposition 2.5 (Fundamental groups of semi-graphs of temperoids of HSD-type).
Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type associated (cf. Definition 2.3(ii) and (iii)) to a collection of HSD-data
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type associated (cf. Definition 2.3(ii) and (iii)) to a collection of HSD-data
![]() $\mathbb {X}$
(cf. Definition 2.2(iv)). Write
$\mathbb {X}$
(cf. Definition 2.2(iv)). Write
![]() $U_{\mathbb {X}}$
for the underlying surface of
$U_{\mathbb {X}}$
for the underlying surface of
![]() $\mathbb {X}$
(cf. Definition 2.2(iv)) and
$\mathbb {X}$
(cf. Definition 2.2(iv)) and
for the connected temperoid (cf. [Reference Mochizuki17, Def. 3.1(ii)]) determined by the category of topological coverings with countably many connected components of the topological space
![]() $U_{\mathbb {X}}$
. Then the following hold:
$U_{\mathbb {X}}$
. Then the following hold:
-
(i) We have a natural equivalence of categories
(cf. Definition 2.1(vi)). In particular, $$\begin{align*}\mathcal{B}^{\mathrm{tp}}(U_{\mathbb{X}}) \stackrel{\sim}{\longrightarrow} \mathcal{B}^{\mathrm{tp}}(\mathcal{G}) \end{align*}$$
$$\begin{align*}\mathcal{B}^{\mathrm{tp}}(U_{\mathbb{X}}) \stackrel{\sim}{\longrightarrow} \mathcal{B}^{\mathrm{tp}}(\mathcal{G}) \end{align*}$$
 $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
is a connected temperoid. Write for the tempered fundamental group (which is well-defined, up to inner automorphism) of the connected temperoid
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
is a connected temperoid. Write for the tempered fundamental group (which is well-defined, up to inner automorphism) of the connected temperoid $$ \begin{align*}\Pi_{\mathcal{G}}\end{align*} $$
$$ \begin{align*}\Pi_{\mathcal{G}}\end{align*} $$
 $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
(cf. [Reference Mochizuki17, Rem. 3.2.1]; the discussion of “Galois-countable temperoids” in [Reference Mochizuki22, Rem. 2.5.3(i)]). (Thus, the tempered group
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
(cf. [Reference Mochizuki17, Rem. 3.2.1]; the discussion of “Galois-countable temperoids” in [Reference Mochizuki22, Rem. 2.5.3(i)]). (Thus, the tempered group
 $\Pi _{\mathcal {G}}$
admits a natural outer isomorphism with the topological fundamental group, equipped with the discrete topology, of the topological space
$\Pi _{\mathcal {G}}$
admits a natural outer isomorphism with the topological fundamental group, equipped with the discrete topology, of the topological space
 $U_{\mathbb {X}}$
.) We shall refer to this tempered group
$U_{\mathbb {X}}$
.) We shall refer to this tempered group
 $\Pi _{\mathcal {G}}$
as the fundamental group of
$\Pi _{\mathcal {G}}$
as the fundamental group of
 $\mathcal {G}$
.
$\mathcal {G}$
.
-
(ii) Every connected finite étale covering
 $\mathcal {H} \rightarrow \mathcal {G}$
(cf. Definition 2.1(vi)) admits a natural structure of semi-graph of temperoids of HSD-type.
$\mathcal {H} \rightarrow \mathcal {G}$
(cf. Definition 2.1(vi)) admits a natural structure of semi-graph of temperoids of HSD-type. -
(iii) The connected semi-graph of anabelioids
 $\mathcal {G}^{\Sigma }$
(cf. Definition 2.1(iii)) is of pro-
$\mathcal {G}^{\Sigma }$
(cf. Definition 2.1(iii)) is of pro-
 $\Sigma $
PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). Write
$\Sigma $
PSC-type (cf. [Reference Mochizuki18, Def. 1.1(i)]). Write
 $\Pi _{\mathcal {G}^{\Sigma }}$
for the (pro-
$\Pi _{\mathcal {G}^{\Sigma }}$
for the (pro-
 $\Sigma $
) fundamental group of
$\Sigma $
) fundamental group of
 $\mathcal {G}^{\Sigma }$
. Then the natural morphism
$\mathcal {G}^{\Sigma }$
. Then the natural morphism
 $\mathcal {G} \rightarrow \mathcal {G}^{\Sigma }$
of semi-graphs of temperoids of Definition 2.1(iii) induces a natural outer injection (cf. (i)). Moreover, this natural outer injection determines an outer isomorphism
$\mathcal {G} \rightarrow \mathcal {G}^{\Sigma }$
of semi-graphs of temperoids of Definition 2.1(iii) induces a natural outer injection (cf. (i)). Moreover, this natural outer injection determines an outer isomorphism $$\begin{align*}\Pi_{\mathcal{G}} \hookrightarrow \Pi_{\mathcal{G}^{\Sigma}} \end{align*}$$
where we write
$$\begin{align*}\Pi_{\mathcal{G}} \hookrightarrow \Pi_{\mathcal{G}^{\Sigma}} \end{align*}$$
where we write $$\begin{align*}\Pi_{\mathcal{G}}^{\Sigma} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}^{\Sigma}}, \end{align*}$$
$$\begin{align*}\Pi_{\mathcal{G}}^{\Sigma} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}^{\Sigma}}, \end{align*}$$
 $\Pi _{\mathcal {G}}^{\Sigma }$
for the pro-
$\Pi _{\mathcal {G}}^{\Sigma }$
for the pro-
 $\Sigma $
completion of
$\Sigma $
completion of
 $\Pi _{\mathcal {G}}$
.
$\Pi _{\mathcal {G}}$
.
-
(iv) Let
 $z \in \mathrm {VCN}(\mathcal {G})$
(cf. Definition 2.1(v)). Write
$z \in \mathrm {VCN}(\mathcal {G})$
(cf. Definition 2.1(v)). Write
 $\Pi _{\mathcal {G}_z}$
for the tempered fundamental group (cf. [Reference Mochizuki17, Rem. 3.2.1]) of the connected temperoid
$\Pi _{\mathcal {G}_z}$
for the tempered fundamental group (cf. [Reference Mochizuki17, Rem. 3.2.1]) of the connected temperoid
 $\mathcal {G}_z$
of
$\mathcal {G}_z$
of
 $\mathcal {G}$
corresponding to z. Then the natural outer homomorphism is a
$\mathcal {G}$
corresponding to z. Then the natural outer homomorphism is a $$\begin{align*}\Pi_{\mathcal{G}_z} \longrightarrow \Pi_{\mathcal{G}} \end{align*}$$
$$\begin{align*}\Pi_{\mathcal{G}_z} \longrightarrow \Pi_{\mathcal{G}} \end{align*}$$
 $\Sigma $
-compatible injection (cf. the discussion entitled “Groups” in §0).
$\Sigma $
-compatible injection (cf. the discussion entitled “Groups” in §0).
-
(v) In the notation of (iii) and (iv), the closure of the image of the composite
of the outer injections of (iii) and (iv) is a VCN-subgroup of $$\begin{align*}\Pi_{\mathcal{G}_z} \hookrightarrow \Pi_{\mathcal{G}} \hookrightarrow \Pi_{\mathcal{G}^{\Sigma}} \end{align*}$$
$$\begin{align*}\Pi_{\mathcal{G}_z} \hookrightarrow \Pi_{\mathcal{G}} \hookrightarrow \Pi_{\mathcal{G}^{\Sigma}} \end{align*}$$
 $\Pi _{\mathcal {G}^{\Sigma }}$
(cf. (iii); [Reference Hoshi and Mochizuki9, Def. 2.1(i)]) associated with
$\Pi _{\mathcal {G}^{\Sigma }}$
(cf. (iii); [Reference Hoshi and Mochizuki9, Def. 2.1(i)]) associated with
 $z \in \mathrm {VCN}(\mathcal {G}) = \mathrm {VCN}(\mathcal {G}^{\Sigma })$
.
$z \in \mathrm {VCN}(\mathcal {G}) = \mathrm {VCN}(\mathcal {G}^{\Sigma })$
.
Proof. A natural equivalence of categories as in assertion (i) may be obtained by observing that, after sorting through the various definitions involved, an object of
![]() $\mathcal {B}^{\mathrm {tp}}(U_{\mathbb {X}})$
(i.e., a topological covering of
$\mathcal {B}^{\mathrm {tp}}(U_{\mathbb {X}})$
(i.e., a topological covering of
![]() $U_{\mathbb {X}}$
) amounts to the same data as an object of
$U_{\mathbb {X}}$
) amounts to the same data as an object of
![]() $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
. Assertion (ii) follows immediately from the various definitions involved.
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
. Assertion (ii) follows immediately from the various definitions involved.
Next, we verify assertion (iii). The assertion that
![]() $\mathcal {G}^{\Sigma }$
is of pro-
$\mathcal {G}^{\Sigma }$
is of pro-
![]() $\Sigma $
PSC-type, as well as the assertion that the morphism
$\Sigma $
PSC-type, as well as the assertion that the morphism
![]() $\mathcal {G} \rightarrow \mathcal {G}^{\Sigma }$
determines an outer isomorphism
$\mathcal {G} \rightarrow \mathcal {G}^{\Sigma }$
determines an outer isomorphism
![]() $\Pi _{\mathcal {G}}^{\Sigma } \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\Sigma }}$
, follows immediately from the various definitions involved. Thus, the assertion that the morphism
$\Pi _{\mathcal {G}}^{\Sigma } \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\Sigma }}$
, follows immediately from the various definitions involved. Thus, the assertion that the morphism
![]() $\mathcal {G} \rightarrow \mathcal {G}^{\Sigma }$
determines an outer injection
$\mathcal {G} \rightarrow \mathcal {G}^{\Sigma }$
determines an outer injection
![]() $\Pi _{\mathcal {G}} \hookrightarrow \Pi _{\mathcal {G}^{\Sigma }}$
follows from the well-known fact that the discrete group
$\Pi _{\mathcal {G}} \hookrightarrow \Pi _{\mathcal {G}^{\Sigma }}$
follows from the well-known fact that the discrete group
![]() $\Pi _{\mathcal {G}}$
injects into its pro-l completion for any
$\Pi _{\mathcal {G}}$
injects into its pro-l completion for any
![]() $l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf., e.g., [Reference Ribes and Zalesskii27, Prop. 3.3.15]; [Reference Mochizuki17, Th. 1.7]).
$l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf., e.g., [Reference Ribes and Zalesskii27, Prop. 3.3.15]; [Reference Mochizuki17, Th. 1.7]).
Next, we verify the injectivity portion of assertion (iv). Let us first observe that it follows immediately from the various definitions involved that the composite
(cf. Definition 2.1(iii)) of the outer homomorphism under consideration and the outer injection of assertion (iii) (in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) factors as the composite
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) factors as the composite
of the outer homomorphism
![]() $\Pi _{\mathcal {G}_z} \rightarrow \Pi _{\widehat {\mathcal {G}}_z}$
induced by the morphism
$\Pi _{\mathcal {G}_z} \rightarrow \Pi _{\widehat {\mathcal {G}}_z}$
induced by the morphism
![]() $\mathcal {G}_z \rightarrow \widehat {\mathcal {G}}_z$
of Definition 2.1(ii) and the natural outer inclusion
$\mathcal {G}_z \rightarrow \widehat {\mathcal {G}}_z$
of Definition 2.1(ii) and the natural outer inclusion
![]() $\Pi _{\widehat {\mathcal {G}}_z} \hookrightarrow \Pi _{\widehat {\mathcal {G}}}$
(cf. [Reference Mochizuki17, Prop. 2.5(i)]). Thus, to complete the verification of the injectivity portion of assertion (iv), it suffices to verify that the outer homomorphism
$\Pi _{\widehat {\mathcal {G}}_z} \hookrightarrow \Pi _{\widehat {\mathcal {G}}}$
(cf. [Reference Mochizuki17, Prop. 2.5(i)]). Thus, to complete the verification of the injectivity portion of assertion (iv), it suffices to verify that the outer homomorphism
![]() $\Pi _{\mathcal {G}_z} \rightarrow \Pi _{\widehat {\mathcal {G}}_z}$
is injective. On the other hand, this follows from the well-known fact that
$\Pi _{\mathcal {G}_z} \rightarrow \Pi _{\widehat {\mathcal {G}}_z}$
is injective. On the other hand, this follows from the well-known fact that
![]() $\Pi _{\mathcal {G}_z}$
injects into its pro-l completion for any
$\Pi _{\mathcal {G}_z}$
injects into its pro-l completion for any
![]() $l\in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf., e.g., [Reference Ribes and Zalesskii27, Prop. 3.3.15]; [Reference Mochizuki17, Th. 1.7]). This completes the proof of the injectivity portion of assertion (iv). Assertion (v) follows immediately from the various definitions involved. Finally, it follows immediately from assertions (iii) and (v), together with the evident pro-
$l\in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf., e.g., [Reference Ribes and Zalesskii27, Prop. 3.3.15]; [Reference Mochizuki17, Th. 1.7]). This completes the proof of the injectivity portion of assertion (iv). Assertion (v) follows immediately from the various definitions involved. Finally, it follows immediately from assertions (iii) and (v), together with the evident pro-
![]() $\Sigma $
analogue of [Reference Mochizuki17, Prop. 2.5(i)], that the natural outer injection of assertion (iv) is
$\Sigma $
analogue of [Reference Mochizuki17, Prop. 2.5(i)], that the natural outer injection of assertion (iv) is
![]() $\Sigma $
-compatible. This completes the proof of assertion (iv), hence also of Proposition 2.5.
$\Sigma $
-compatible. This completes the proof of assertion (iv), hence also of Proposition 2.5.
Remark 2.5.1. In the notation of Proposition 2.5, as is discussed in Proposition 2.5(i), the fundamental group
![]() $\Pi _{\mathcal {G}}$
of the semi-graph of temperoids of HSD-type
$\Pi _{\mathcal {G}}$
of the semi-graph of temperoids of HSD-type
![]() $\mathcal {G}$
is naturally isomorphic, up to inner automorphism, to the topological fundamental group, equipped with the discrete topology, of the compact orientable hyperbolic topological surface with compact boundary
$\mathcal {G}$
is naturally isomorphic, up to inner automorphism, to the topological fundamental group, equipped with the discrete topology, of the compact orientable hyperbolic topological surface with compact boundary
![]() $U_{\mathbb {X}}$
. In particular,
$U_{\mathbb {X}}$
. In particular,
![]() $\Pi _{\mathcal {G}}$
is finitely generated, torsion-free, and center-free and injects into its pro-l completion for any
$\Pi _{\mathcal {G}}$
is finitely generated, torsion-free, and center-free and injects into its pro-l completion for any
![]() $l\in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Proposition 2.5(iii)). Moreover, it holds that
$l\in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Proposition 2.5(iii)). Moreover, it holds that
![]() $\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
(cf. Definition 2.1(v)) if and only if
$\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
(cf. Definition 2.1(v)) if and only if
![]() $\Pi _{\mathcal {G}}$
is free.
$\Pi _{\mathcal {G}}$
is free.
Remark 2.5.2. In the situation of Example 2.4(ii), write
![]() $\mathcal {G}_{X^{\mathrm {log}}}$
for the semi-graph of temperoids of HSD-type associated with
$\mathcal {G}_{X^{\mathrm {log}}}$
for the semi-graph of temperoids of HSD-type associated with
![]() $X^{\mathrm {log}}$
;
$X^{\mathrm {log}}$
;
![]() $\mathcal {G}^{\Sigma }_{X^{\mathrm {log}}}$
for the semi-graph of anabelioids of pro-
$\mathcal {G}^{\Sigma }_{X^{\mathrm {log}}}$
for the semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type of Proposition 2.5(iii) in the case where we take the “
$\Sigma $
PSC-type of Proposition 2.5(iii) in the case where we take the “
![]() $\mathcal {G}$
” of Proposition 2.5(iii), to be
$\mathcal {G}$
” of Proposition 2.5(iii), to be
![]() $\mathcal {G}_{X^{\mathrm {log}}}$
;
$\mathcal {G}_{X^{\mathrm {log}}}$
;
![]() $\mathcal {G}_{X^{\mathrm {log}}}^{\mathrm {PSC}\text {-}\Sigma }$
for the semi-graph of anabelioids of pro-
$\mathcal {G}_{X^{\mathrm {log}}}^{\mathrm {PSC}\text {-}\Sigma }$
for the semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type associated with
$\Sigma $
PSC-type associated with
![]() $X^{\mathrm {log}}$
(cf. [Reference Mochizuki18, Exam. 2.5]). Then it follows from Proposition 2.5(iii) that we have a natural outer isomorphism
$X^{\mathrm {log}}$
(cf. [Reference Mochizuki18, Exam. 2.5]). Then it follows from Proposition 2.5(iii) that we have a natural outer isomorphism
![]() $\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}^{\Sigma } \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\Sigma }_{X^{\mathrm {log}}}}$
. On the other hand, by associating finite étale coverings of
$\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}^{\Sigma } \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\Sigma }_{X^{\mathrm {log}}}}$
. On the other hand, by associating finite étale coverings of
![]() $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
to log étale coverings of Kummer type of
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
to log étale coverings of Kummer type of
![]() $X^{\mathrm {log}}$
(cf. [Reference Kato and Nakayama12, Lem. 2.2]) and then restricting such finite étale coverings to
$X^{\mathrm {log}}$
(cf. [Reference Kato and Nakayama12, Lem. 2.2]) and then restricting such finite étale coverings to
![]() $X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
(cf. Example 2.4(i)), we obtain an outer homomorphism
$X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s$
(cf. Example 2.4(i)), we obtain an outer homomorphism
![]() $ \Pi _{\mathcal {G}_{X^{\mathrm {log}}}}^{\Sigma } \rightarrow \Pi _{\mathcal {G}_{X^{\mathrm {log}}}^{\mathrm {PSC}\text {-}\Sigma }} $
. Then one verifies immediately from the various definitions involved that the composite of the two outer homomorphisms
$ \Pi _{\mathcal {G}_{X^{\mathrm {log}}}}^{\Sigma } \rightarrow \Pi _{\mathcal {G}_{X^{\mathrm {log}}}^{\mathrm {PSC}\text {-}\Sigma }} $
. Then one verifies immediately from the various definitions involved that the composite of the two outer homomorphisms
is a graphic outer isomorphism (cf. [Reference Mochizuki18, Def. 1.4(i)]), that is, arises from a uniquely determined isomorphism of semi-graphs of anabelioids
Finally, one verifies easily that the above discussion generalizes immediately to the case of arbitrary fs log schemes
![]() $S^{\mathrm {log}}$
with underlying scheme
$S^{\mathrm {log}}$
with underlying scheme
![]() $S=\mathrm {Spec}(\mathbb {C})$
(cf. Remark 2.4.1).
$S=\mathrm {Spec}(\mathbb {C})$
(cf. Remark 2.4.1).
Definition 2.6. Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Write
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Write
![]() $\Pi _{\mathcal {G}}$
for the fundamental group of
$\Pi _{\mathcal {G}}$
for the fundamental group of
![]() $\mathcal {G}$
.
$\mathcal {G}$
.
-
(i) Let
 $z \in \mathrm {VCN}(\mathcal {G})$
(cf. Definition 2.1(v)). Then we shall refer to a closed subgroup of
$z \in \mathrm {VCN}(\mathcal {G})$
(cf. Definition 2.1(v)). Then we shall refer to a closed subgroup of
 $\Pi _{\mathcal {G}}$
that belongs to the
$\Pi _{\mathcal {G}}$
that belongs to the
 $\Pi _{\mathcal {G}}$
-conjugacy class of closed subgroups determined by the image of the outer injection of the display of Proposition 2.5(iv) as a VCN-subgroup of
$\Pi _{\mathcal {G}}$
-conjugacy class of closed subgroups determined by the image of the outer injection of the display of Proposition 2.5(iv) as a VCN-subgroup of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $z \in \mathrm {VCN}(\mathcal {G})$
. If, moreover,
$z \in \mathrm {VCN}(\mathcal {G})$
. If, moreover,
 $z \in \mathrm {Vert}(\mathcal {G})$
(resp.
$z \in \mathrm {Vert}(\mathcal {G})$
(resp.
 $\in \mathrm {Cusp}(\mathcal {G})$
;
$\in \mathrm {Cusp}(\mathcal {G})$
;
 $\in \mathrm {Node}(\mathcal {G})$
;
$\in \mathrm {Node}(\mathcal {G})$
;
 $\in \mathrm {Edge}(\mathcal {G})$
) (cf. Definition 2.1(v)), then we shall refer to a VCN-subgroup of
$\in \mathrm {Edge}(\mathcal {G})$
) (cf. Definition 2.1(v)), then we shall refer to a VCN-subgroup of
 $\Pi _{\mathcal {G}}$
associated with z as a verticial (resp. a cuspidal; a nodal; an edge-like) subgroup of
$\Pi _{\mathcal {G}}$
associated with z as a verticial (resp. a cuspidal; a nodal; an edge-like) subgroup of
 $\Pi _{\mathcal {G}}$
associated with z.
$\Pi _{\mathcal {G}}$
associated with z. -
(ii) Write
 $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
for the universal covering of
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
for the universal covering of
 $\mathcal {G}$
corresponding to
$\mathcal {G}$
corresponding to
 $\Pi _{\mathcal {G}}$
. Let
$\Pi _{\mathcal {G}}$
. Let
 $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
(cf. Definition 2.1(v)). Then we shall refer to the VCN-subgroup
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
(cf. Definition 2.1(v)). Then we shall refer to the VCN-subgroup
 $\Pi _{\widetilde z} \subseteq \Pi _{\mathcal {G}}$
(cf. (i)) determined by
$\Pi _{\widetilde z} \subseteq \Pi _{\mathcal {G}}$
(cf. (i)) determined by
 $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
as the VCN-subgroup of
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
as the VCN-subgroup of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
. If, moreover,
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
. If, moreover,
 $\widetilde z \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
(resp.
$\widetilde z \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
(resp.
 $\in \mathrm {Cusp}(\widetilde {\mathcal {G}})$
;
$\in \mathrm {Cusp}(\widetilde {\mathcal {G}})$
;
 $\in \mathrm {Node}(\widetilde {\mathcal {G}})$
;
$\in \mathrm {Node}(\widetilde {\mathcal {G}})$
;
 $\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
) (cf. Definition 2.1(v)), then we shall refer to the VCN-subgroup of
$\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
) (cf. Definition 2.1(v)), then we shall refer to the VCN-subgroup of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde z$
as the verticial (resp. cuspidal; nodal; edge-like) subgroup of
$\widetilde z$
as the verticial (resp. cuspidal; nodal; edge-like) subgroup of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde z$
.
$\widetilde z$
. -
(iii) Let
 $(g,r)$
be a pair of nonnegative integers such that
$(g,r)$
be a pair of nonnegative integers such that
 $2g-2+r>0$
and
$2g-2+r>0$
and
 $v\in \mathrm {Vert}(\mathcal {G})$
. Then we shall say that v is of type
$v\in \mathrm {Vert}(\mathcal {G})$
. Then we shall say that v is of type
 $(g,r)$
if the “
$(g,r)$
if the “
 $(g,r)$
” appearing in Definition 2.2(ii) for the pair of HS-type corresponding to v coincides with
$(g,r)$
” appearing in Definition 2.2(ii) for the pair of HS-type corresponding to v coincides with
 $(g,r)$
. Thus, one verifies easily that v is of type
$(g,r)$
. Thus, one verifies easily that v is of type
 $(g,r)$
if and only if the number of the branches of edges of
$(g,r)$
if and only if the number of the branches of edges of
 $\mathcal {G}$
that abut to v is equal to r, and, moreover, where we use the notation
$\mathcal {G}$
that abut to v is equal to r, and, moreover, where we use the notation $$\begin{align*}\mathrm{rank}_{\mathbb{Z}}(\Pi_{v}^{\mathrm{ab}}) = 2g + \max\{0, r-1\}, \end{align*}$$
$$\begin{align*}\mathrm{rank}_{\mathbb{Z}}(\Pi_{v}^{\mathrm{ab}}) = 2g + \max\{0, r-1\}, \end{align*}$$
 $\Pi _{v}$
to denote a verticial subgroup associated with v.
$\Pi _{v}$
to denote a verticial subgroup associated with v.
Remark 2.6.1. In the notation of Definition 2.6, it follows from Proposition 2.5(iv) that every verticial subgroup of
![]() $\Pi _{\mathcal {G}}$
is naturally isomorphic, up to inner automorphism, to the topological fundamental group, equipped with the discrete topology, of a compact orientable hyperbolic topological surface with compact boundary. In particular, every verticial subgroup of
$\Pi _{\mathcal {G}}$
is naturally isomorphic, up to inner automorphism, to the topological fundamental group, equipped with the discrete topology, of a compact orientable hyperbolic topological surface with compact boundary. In particular, every verticial subgroup of
![]() $\Pi _{\mathcal {G}}$
is finitely generated, torsion-free, and center-free and injects into its pro-l completion for any
$\Pi _{\mathcal {G}}$
is finitely generated, torsion-free, and center-free and injects into its pro-l completion for any
![]() $l\in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Proposition 2.5(iii)). Moreover, it follows from Proposition 2.5(iv) that every edge-like subgroup of
$l\in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Proposition 2.5(iii)). Moreover, it follows from Proposition 2.5(iv) that every edge-like subgroup of
![]() $\Pi _{\mathcal {G}}$
is naturally isomorphic, up to inner automorphism, to the topological fundamental group, equipped with the discrete topology, of a unit circle (hence isomorphic to
$\Pi _{\mathcal {G}}$
is naturally isomorphic, up to inner automorphism, to the topological fundamental group, equipped with the discrete topology, of a unit circle (hence isomorphic to
![]() $\mathbb {Z}$
).
$\mathbb {Z}$
).
Definition 2.7. Let
![]() $\mathcal {G}$
and
$\mathcal {G}$
and
![]() $\mathcal {H}$
be semi-graphs of temperoids of HSD-type. Write
$\mathcal {H}$
be semi-graphs of temperoids of HSD-type. Write
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {H}}$
for the fundamental groups of
$\Pi _{\mathcal {H}}$
for the fundamental groups of
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
, respectively.
$\mathcal {H}$
, respectively.
-
(i) We shall say that an isomorphism of groups
 $\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal) if the isomorphism induces a bijection between the set of the verticial (resp. cuspidal; nodal) subgroups (cf. Definition 2.6(i)) of
$\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal) if the isomorphism induces a bijection between the set of the verticial (resp. cuspidal; nodal) subgroups (cf. Definition 2.6(i)) of
 $\Pi _{\mathcal {G}}$
and the set of the verticial (resp. cuspidal; nodal) subgroups of
$\Pi _{\mathcal {G}}$
and the set of the verticial (resp. cuspidal; nodal) subgroups of
 $\Pi _{\mathcal {H}}$
. We shall say that an outer isomorphism
$\Pi _{\mathcal {H}}$
. We shall say that an outer isomorphism
 $\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal) if it arises from an isomorphism
$\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal) if it arises from an isomorphism
 $\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
that is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal).
$\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
that is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal). -
(ii) We shall say that an outer isomorphism
 $\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is graphic if it arises from an isomorphism
$\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is graphic if it arises from an isomorphism
 $\mathcal {G} \stackrel {\sim }{\rightarrow } \mathcal {H}$
. We shall say that an isomorphism
$\mathcal {G} \stackrel {\sim }{\rightarrow } \mathcal {H}$
. We shall say that an isomorphism
 $\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is graphic if the outer isomorphism
$\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
is graphic if the outer isomorphism
 $\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
determined by it is graphic.
$\Pi _{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {H}}$
determined by it is graphic.
Definition 2.8. Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Write
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Write
![]() $\mathbb {G}$
for the underlying semi-graph of
$\mathbb {G}$
for the underlying semi-graph of
![]() $\mathcal {G}$
. Also, for each
$\mathcal {G}$
. Also, for each
![]() $z \in \mathrm {VCN}(\mathcal {G})$
, write
$z \in \mathrm {VCN}(\mathcal {G})$
, write
![]() $\mathcal {G}_z$
for the connected temperoid of
$\mathcal {G}_z$
for the connected temperoid of
![]() $\mathcal {G}$
corresponding to z.
$\mathcal {G}$
corresponding to z.
-
(i) Let
 $\mathbb {H}$
be a sub-semi-graph of PSC-type (cf. [Reference Hoshi and Mochizuki9, Def. 2.2(i)]) of
$\mathbb {H}$
be a sub-semi-graph of PSC-type (cf. [Reference Hoshi and Mochizuki9, Def. 2.2(i)]) of
 $\mathbb {G}$
. Then one may define a semi-graph of temperoids of HSD-type as follows (cf. Figure 2 of [Reference Hoshi and Mochizuki9]): we take the underlying semi-graph of
$\mathbb {G}$
. Then one may define a semi-graph of temperoids of HSD-type as follows (cf. Figure 2 of [Reference Hoshi and Mochizuki9]): we take the underlying semi-graph of $$ \begin{align*}\mathcal{G}|_{\mathbb{H}}\end{align*} $$
$$ \begin{align*}\mathcal{G}|_{\mathbb{H}}\end{align*} $$
 $\mathcal {G}|_{\mathbb {H}}$
to be
$\mathcal {G}|_{\mathbb {H}}$
to be
 $\mathbb {H}$
; for each vertex v (resp. edge e) of
$\mathbb {H}$
; for each vertex v (resp. edge e) of
 $\mathbb {H}$
, we take the temperoid corresponding to v (resp. e) to be
$\mathbb {H}$
, we take the temperoid corresponding to v (resp. e) to be
 $\mathcal {G}_v$
(resp.
$\mathcal {G}_v$
(resp.
 $\mathcal {G}_e$
); for each branch b of an edge e of
$\mathcal {G}_e$
); for each branch b of an edge e of
 $\mathbb {H}$
that abuts to a vertex v of
$\mathbb {H}$
that abuts to a vertex v of
 $\mathbb {H}$
, we take the morphism associated with b to be the morphism
$\mathbb {H}$
, we take the morphism associated with b to be the morphism
 $\mathcal {G}_e \rightarrow \mathcal {G}_v$
associated with the branch of
$\mathcal {G}_e \rightarrow \mathcal {G}_v$
associated with the branch of
 $\mathcal {G}$
corresponding to b. We shall refer to
$\mathcal {G}$
corresponding to b. We shall refer to
 $\mathcal {G}|_{\mathbb {H}}$
as the semi-graph of temperoids of HSD-type obtained by restricting
$\mathcal {G}|_{\mathbb {H}}$
as the semi-graph of temperoids of HSD-type obtained by restricting
 $\mathcal {G}$
to
$\mathcal {G}$
to
 $\mathbb {H}$
. Thus, one has a natural morphism of semi-graphs of temperoids.
$\mathbb {H}$
. Thus, one has a natural morphism of semi-graphs of temperoids. $$\begin{align*}\mathcal{G}|_{\mathbb{H}} \longrightarrow \mathcal{G} \end{align*}$$
$$\begin{align*}\mathcal{G}|_{\mathbb{H}} \longrightarrow \mathcal{G} \end{align*}$$
-
(ii) Let
 $S \subseteq \mathrm {Cusp}(\mathcal {G})$
be a subset of
$S \subseteq \mathrm {Cusp}(\mathcal {G})$
be a subset of
 $\mathrm {Cusp}(\mathcal {G})$
(cf. Definition 2.1(v)) which is omittable (cf. [Reference Hoshi and Mochizuki9, Def. 2.4(i)]) as a subset of the set of cusps
$\mathrm {Cusp}(\mathcal {G})$
(cf. Definition 2.1(v)) which is omittable (cf. [Reference Hoshi and Mochizuki9, Def. 2.4(i)]) as a subset of the set of cusps
 $\mathrm {Cusp}(\widehat {\mathcal {G}})$
of the semi-graph of anabelioids of pro-
$\mathrm {Cusp}(\widehat {\mathcal {G}})$
of the semi-graph of anabelioids of pro-
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
 $\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
$\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
 $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) relative to the natural identification
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) relative to the natural identification
 $\mathrm {Cusp}(\mathcal {G}) = \mathrm {Cusp}(\widehat {\mathcal {G}})$
. Then, by eliminating the cusps contained in S, and, for each vertex v of
$\mathrm {Cusp}(\mathcal {G}) = \mathrm {Cusp}(\widehat {\mathcal {G}})$
. Then, by eliminating the cusps contained in S, and, for each vertex v of
 $\mathcal {G}$
, replacing the temperoid
$\mathcal {G}$
, replacing the temperoid
 $\mathcal {G}_v$
by the temperoid of coverings of
$\mathcal {G}_v$
by the temperoid of coverings of
 $\mathcal {G}_v$
that restrict to a trivial covering over the cusps contained in S that abut to v, we obtain a semi-graph of temperoids of HSD-type
$\mathcal {G}_v$
that restrict to a trivial covering over the cusps contained in S that abut to v, we obtain a semi-graph of temperoids of HSD-type  $$ \begin{align*}\mathcal{G}_{\bullet S}\end{align*} $$
$$ \begin{align*}\mathcal{G}_{\bullet S}\end{align*} $$
(cf. Figure 3 of [Reference Hoshi and Mochizuki9]). We shall refer to
 $\mathcal {G}_{\bullet S}$
as the partial compactification of
$\mathcal {G}_{\bullet S}$
as the partial compactification of
 $\mathcal {G}$
with respect to S.
$\mathcal {G}$
with respect to S. -
(iii) Let
 $S\subseteq \mathrm {Node}(\mathcal {G})$
be a subset of
$S\subseteq \mathrm {Node}(\mathcal {G})$
be a subset of
 $\mathrm {Node}(\mathcal {G})$
(cf. Definition 2.1(v)) such that the semi-graph obtained by removing the closed edges corresponding to the elements of
$\mathrm {Node}(\mathcal {G})$
(cf. Definition 2.1(v)) such that the semi-graph obtained by removing the closed edges corresponding to the elements of
 $S$
from the underlying semi-graph of
$S$
from the underlying semi-graph of
 $\mathcal {G}$
is connected, that is, in the terminology of [Reference Hoshi and Mochizuki9, Def. 2.5(i)] that is not of separating type as a subset of the set of nodes
$\mathcal {G}$
is connected, that is, in the terminology of [Reference Hoshi and Mochizuki9, Def. 2.5(i)] that is not of separating type as a subset of the set of nodes
 $\mathrm {Node}(\widehat {\mathcal {G}})$
of the semi-graph of anabelioids of pro-
$\mathrm {Node}(\widehat {\mathcal {G}})$
of the semi-graph of anabelioids of pro-
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
 $\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
$\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
 $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) relative to the natural identification
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) relative to the natural identification
 $\mathrm {Node}(\mathcal {G}) = \mathrm {Node}(\widehat {\mathcal {G}})$
. Then one may define a semi-graph of temperoids of HSD-type as follows (cf. Figure 4 of [Reference Hoshi and Mochizuki9]): we take the underlying semi-graph of
$\mathrm {Node}(\mathcal {G}) = \mathrm {Node}(\widehat {\mathcal {G}})$
. Then one may define a semi-graph of temperoids of HSD-type as follows (cf. Figure 4 of [Reference Hoshi and Mochizuki9]): we take the underlying semi-graph of $$ \begin{align*}\mathcal{G}_{\succ S}\end{align*} $$
$$ \begin{align*}\mathcal{G}_{\succ S}\end{align*} $$
 $\mathcal {G}_{\succ S}$
to be the semi-graph obtained by replacing each node e of
$\mathcal {G}_{\succ S}$
to be the semi-graph obtained by replacing each node e of
 $\mathbb {G}$
contained in S such that
$\mathbb {G}$
contained in S such that
 $\mathcal {V}(e) = \{v_1, v_2\} \subseteq \mathrm {Vert}(\mathcal {G})$
(cf. Definition 2.1(v))—where
$\mathcal {V}(e) = \{v_1, v_2\} \subseteq \mathrm {Vert}(\mathcal {G})$
(cf. Definition 2.1(v))—where
 $v_1$
,
$v_1$
,
 $v_2$
are not necessarily distinct—by two cusps that abut to
$v_2$
are not necessarily distinct—by two cusps that abut to
 $v_1$
,
$v_1$
,
 $v_2 \in \mathrm {Vert}(\mathcal {G})$
, respectively, which we think as corresponding to the two branches of e. We take the temperoid corresponding to a vertex v (resp. node e) of
$v_2 \in \mathrm {Vert}(\mathcal {G})$
, respectively, which we think as corresponding to the two branches of e. We take the temperoid corresponding to a vertex v (resp. node e) of
 $\mathcal {G}_{\succ S}$
to be
$\mathcal {G}_{\succ S}$
to be
 $\mathcal {G}_v$
(resp.
$\mathcal {G}_v$
(resp.
 $\mathcal {G}_e$
). (Note that the set of vertices (resp. nodes) of
$\mathcal {G}_e$
). (Note that the set of vertices (resp. nodes) of
 $\mathcal {G}_{\succ S}$
may be naturally identified with
$\mathcal {G}_{\succ S}$
may be naturally identified with
 $\mathrm {Vert}(\mathcal {G})$
(resp.
$\mathrm {Vert}(\mathcal {G})$
(resp.
 $\mathrm {Node}(\mathcal {G}) \setminus S$
).) We take the temperoid corresponding to a cusp of
$\mathrm {Node}(\mathcal {G}) \setminus S$
).) We take the temperoid corresponding to a cusp of
 $\mathcal {G}_{\succ S}$
arising from a cusp e of
$\mathcal {G}_{\succ S}$
arising from a cusp e of
 $\mathcal {G}$
to be
$\mathcal {G}$
to be
 $\mathcal {G}_e$
. We take the temperoid corresponding to a cusp of
$\mathcal {G}_e$
. We take the temperoid corresponding to a cusp of
 $\mathcal {G}_{\succ S}$
arising from a node e of
$\mathcal {G}_{\succ S}$
arising from a node e of
 $\mathcal {G}$
to be
$\mathcal {G}$
to be
 $\mathcal {G}_{e}$
. For each branch b of
$\mathcal {G}_{e}$
. For each branch b of
 $\mathcal {G}_{\succ S}$
that abuts to a vertex v of a node e (resp. of a cusp e that does not arise from a node of
$\mathcal {G}_{\succ S}$
that abuts to a vertex v of a node e (resp. of a cusp e that does not arise from a node of
 $\mathcal {G}$
), we take the morphism associated with b to be the morphism
$\mathcal {G}$
), we take the morphism associated with b to be the morphism
 $\mathcal {G}_e \rightarrow \mathcal {G}_v$
associated with the branch of
$\mathcal {G}_e \rightarrow \mathcal {G}_v$
associated with the branch of
 $\mathcal {G}$
corresponding to b. For each branch b of
$\mathcal {G}$
corresponding to b. For each branch b of
 $\mathcal {G}_{\succ S}$
that abuts to a vertex v of a cusp of
$\mathcal {G}_{\succ S}$
that abuts to a vertex v of a cusp of
 $\mathcal {G}_{\succ S}$
that arises from a node e of
$\mathcal {G}_{\succ S}$
that arises from a node e of
 $\mathcal {G}$
, we take the morphism associated with b to be the morphism
$\mathcal {G}$
, we take the morphism associated with b to be the morphism
 $\mathcal {G}_{e} \rightarrow \mathcal {G}_v$
associated with the branch of
$\mathcal {G}_{e} \rightarrow \mathcal {G}_v$
associated with the branch of
 $\mathcal {G}$
corresponding to b. We shall refer to
$\mathcal {G}$
corresponding to b. We shall refer to
 $\mathcal {G}_{\succ S}$
as the semi-graph of temperoids of HSD-type obtained from
$\mathcal {G}_{\succ S}$
as the semi-graph of temperoids of HSD-type obtained from
 $\mathcal {G}$
by resolving S. Thus, one has a natural morphism of semi-graphs of temperoids.
$\mathcal {G}$
by resolving S. Thus, one has a natural morphism of semi-graphs of temperoids. $$\begin{align*}\mathcal{G}_{\succ S} \longrightarrow \mathcal{G} \end{align*}$$
$$\begin{align*}\mathcal{G}_{\succ S} \longrightarrow \mathcal{G} \end{align*}$$
Remark 2.8.1. One verifies immediately that the operations of restriction, partial compactification, and resolution discussed in Definition 2.8(i)–(iii) are compatible (in the evident sense) with the corresponding pro-
![]() $\Sigma $
operations—that is, as discussed in [Reference Hoshi and Mochizuki9, Defs. 2.2(ii), 2.4(ii), and 2.5(ii)]—relative to the operation of passing to the associated semi-graph of anabelioids of pro-
$\Sigma $
operations—that is, as discussed in [Reference Hoshi and Mochizuki9, Defs. 2.2(ii), 2.4(ii), and 2.5(ii)]—relative to the operation of passing to the associated semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type (cf. Proposition 2.5(iii)).
$\Sigma $
PSC-type (cf. Proposition 2.5(iii)).
Remark 2.8.2. We take this opportunity to correct an unfortunate misprint in [Reference Hoshi and Mochizuki9, Def. 2.5(ii)]: the phrase “by two cusps that abut to
![]() $v_1$
,
$v_1$
,
![]() $v_2 \in \mathrm {Vert}(\mathcal {G})$
, respectively” of [Reference Hoshi and Mochizuki9, Def. 2.5(ii)] should read “by two cusps that abut to
$v_2 \in \mathrm {Vert}(\mathcal {G})$
, respectively” of [Reference Hoshi and Mochizuki9, Def. 2.5(ii)] should read “by two cusps that abut to
![]() $v_1$
,
$v_1$
,
![]() $v_2 \in \mathrm {Vert}(\mathcal {G})$
, respectively, which we think as corresponding to the two branches of e.”
$v_2 \in \mathrm {Vert}(\mathcal {G})$
, respectively, which we think as corresponding to the two branches of e.”
Definition 2.9. In the notation of Definition 2.8, let
![]() $S\subseteq \mathrm {Node}(\mathcal {G})$
be a subset of
$S\subseteq \mathrm {Node}(\mathcal {G})$
be a subset of
![]() $\mathrm {Node}(\mathcal {G})$
(cf. Definition 2.1(v)). Then we define the semi-graph of temperoids of HSD-type
$\mathrm {Node}(\mathcal {G})$
(cf. Definition 2.1(v)). Then we define the semi-graph of temperoids of HSD-type
as follows (cf. Figure 5 of [Reference Hoshi and Mochizuki9]):
-
(i) We take
 $\mathrm {Cusp}(\mathcal {G}_{\leadsto {S}}) \stackrel {\mathrm {def}}{=} \mathrm {Cusp}(\mathcal {G})$
(cf. Definition 2.1(v)).
$\mathrm {Cusp}(\mathcal {G}_{\leadsto {S}}) \stackrel {\mathrm {def}}{=} \mathrm {Cusp}(\mathcal {G})$
(cf. Definition 2.1(v)). -
(ii) We take
 $\mathrm {Node}(\mathcal {G}_{\leadsto {S}}) \stackrel {\mathrm {def}}{=} \mathrm {Node}(\mathcal {G})\setminus S$
(cf. Definition 2.1(v)).
$\mathrm {Node}(\mathcal {G}_{\leadsto {S}}) \stackrel {\mathrm {def}}{=} \mathrm {Node}(\mathcal {G})\setminus S$
(cf. Definition 2.1(v)). -
(iii) We take
 $\mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
(cf. Definition 2.1(v)) to be the set of connected components of the semi-graph obtained from
$\mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
(cf. Definition 2.1(v)) to be the set of connected components of the semi-graph obtained from
 $\mathbb {G}$
by omitting the edges
$\mathbb {G}$
by omitting the edges
 $e\in \mathrm {Edge}(\mathcal {G})\setminus S$
(cf. Definition 2.1(v)). Alternatively, one may take
$e\in \mathrm {Edge}(\mathcal {G})\setminus S$
(cf. Definition 2.1(v)). Alternatively, one may take
 $\mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
to be the set of equivalence classes of elements of
$\mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
to be the set of equivalence classes of elements of
 $\mathrm {Vert}(\mathcal {G})$
with respect to the equivalence relation “
$\mathrm {Vert}(\mathcal {G})$
with respect to the equivalence relation “
 $\sim $
” defined as follows: for v,
$\sim $
” defined as follows: for v,
 $w \in \mathrm {Vert}(\mathcal {G})$
,
$w \in \mathrm {Vert}(\mathcal {G})$
,
 $v\sim w$
if either
$v\sim w$
if either
 $v = w$
or there exist n elements
$v = w$
or there exist n elements
 $e_1 , \dots , e_{n} \in S$
of S and
$e_1 , \dots , e_{n} \in S$
of S and
 $n+1$
vertices
$n+1$
vertices
 $v_0 , v_1 , \dots , v_n \in \mathrm {Vert}(\mathcal {G})$
of
$v_0 , v_1 , \dots , v_n \in \mathrm {Vert}(\mathcal {G})$
of
 $\mathcal {G}$
such that
$\mathcal {G}$
such that
 $v_0 \stackrel {\mathrm {def}}{=} v$
,
$v_0 \stackrel {\mathrm {def}}{=} v$
,
 $v_n \stackrel {\mathrm {def}}{=} w$
, and, for
$v_n \stackrel {\mathrm {def}}{=} w$
, and, for
 $1 \leq i \leq n$
, it holds that
$1 \leq i \leq n$
, it holds that
 $\mathcal {V}(e_i) = \{v_{i-1}, v_{i}\}$
(cf. Definition 2.1(v)).
$\mathcal {V}(e_i) = \{v_{i-1}, v_{i}\}$
(cf. Definition 2.1(v)). -
(iv) For each branch b of an edge
 $e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}})$
$e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}})$
 $(= \mathrm {Edge}(\mathcal {G})\setminus S$
—cf. (i) and (ii)) and each vertex
$(= \mathrm {Edge}(\mathcal {G})\setminus S$
—cf. (i) and (ii)) and each vertex
 $v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
of
$v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
of
 $\mathcal {G}_{\leadsto {S}}$
, b abuts, relative to
$\mathcal {G}_{\leadsto {S}}$
, b abuts, relative to
 $\mathcal {G}_{\leadsto {S}}$
, to v if b abuts, relative to
$\mathcal {G}_{\leadsto {S}}$
, to v if b abuts, relative to
 $\mathcal {G}$
, to an element of the equivalence class v (cf. (iii)).
$\mathcal {G}$
, to an element of the equivalence class v (cf. (iii)). -
(v) For each edge
 $e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}}) (= \mathrm {Edge}(\mathcal {G})\setminus S$
—cf. (i) and (ii)) of
$e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}}) (= \mathrm {Edge}(\mathcal {G})\setminus S$
—cf. (i) and (ii)) of
 $\mathcal {G}_{\leadsto {S}}$
, we take the temperoid of
$\mathcal {G}_{\leadsto {S}}$
, we take the temperoid of
 $\mathcal {G}_{\leadsto {S}}$
corresponding to
$\mathcal {G}_{\leadsto {S}}$
corresponding to
 $e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}})$
to be the temperoid
$e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}})$
to be the temperoid
 $\mathcal {G}_e$
.
$\mathcal {G}_e$
. -
(vi) Let
 $v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
be a vertex of
$v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
be a vertex of
 $\mathcal {G}_{\leadsto {S}}$
. Then one verifies easily that there exists a unique sub-semi-graph of PSC-type (cf. [Reference Hoshi and Mochizuki9, Def. 2.2(i)])
$\mathcal {G}_{\leadsto {S}}$
. Then one verifies easily that there exists a unique sub-semi-graph of PSC-type (cf. [Reference Hoshi and Mochizuki9, Def. 2.2(i)])
 $\mathbb {H}_v$
of the underlying semi-graph of
$\mathbb {H}_v$
of the underlying semi-graph of
 $\mathcal {G}$
whose set of vertices consists of the elements of the equivalence class v (cf. (iii)). Write
$\mathcal {G}$
whose set of vertices consists of the elements of the equivalence class v (cf. (iii)). Write  $$\begin{align*}T_v \, \, \stackrel{\mathrm{def}}{=} \, \, \mathrm{Node}(\mathcal{G}|_{\mathbb{H}_v}) \setminus (S\cap\mathrm{Node}(\mathcal{G}|_{\mathbb{H}_v})) \end{align*}$$
$$\begin{align*}T_v \, \, \stackrel{\mathrm{def}}{=} \, \, \mathrm{Node}(\mathcal{G}|_{\mathbb{H}_v}) \setminus (S\cap\mathrm{Node}(\mathcal{G}|_{\mathbb{H}_v})) \end{align*}$$
(cf. Definition 2.8(i)). Then we take the temperoid of
 $\mathcal {G}_{\leadsto {S}}$
corresponding to
$\mathcal {G}_{\leadsto {S}}$
corresponding to
 $v \in \mathrm {Vert} (\mathcal {G}_{\leadsto {S}})$
to be the temperoid
$v \in \mathrm {Vert} (\mathcal {G}_{\leadsto {S}})$
to be the temperoid
 $\mathcal {B}^{\mathrm {tp}}((\mathcal {G}|_{\mathbb {H}_v})_{\succ T_v})$
(cf. Definition 2.1(vi); Proposition 2.5(i); Definition 2.8(iii)).
$\mathcal {B}^{\mathrm {tp}}((\mathcal {G}|_{\mathbb {H}_v})_{\succ T_v})$
(cf. Definition 2.1(vi); Proposition 2.5(i); Definition 2.8(iii)). -
(vii) Let b be a branch of an edge
 $e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}}) (= \mathrm {Edge}(\mathcal {G})\setminus S$
—cf. (i) and (ii)) that abuts to a vertex
$e \in \mathrm {Edge}(\mathcal {G}_{\leadsto {S}}) (= \mathrm {Edge}(\mathcal {G})\setminus S$
—cf. (i) and (ii)) that abuts to a vertex
 $v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
. Then since b abuts to v, one verifies easily that there exists a unique vertex w of
$v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
. Then since b abuts to v, one verifies easily that there exists a unique vertex w of
 $\mathcal {G}$
which belongs to the equivalence class v (cf. (iii)) such that b abuts to w relative to
$\mathcal {G}$
which belongs to the equivalence class v (cf. (iii)) such that b abuts to w relative to
 $\mathcal {G}$
. We take the morphism of temperoids associated with b, relative to
$\mathcal {G}$
. We take the morphism of temperoids associated with b, relative to
 $\mathcal {G}_{\leadsto {S}}$
, to be the morphism naturally determined by post-composing the morphism of temperoids
$\mathcal {G}_{\leadsto {S}}$
, to be the morphism naturally determined by post-composing the morphism of temperoids
 $ \mathcal {G}_e \rightarrow \mathcal {G}_w $
corresponding to the branch b relative to
$ \mathcal {G}_e \rightarrow \mathcal {G}_w $
corresponding to the branch b relative to
 $\mathcal {G}$
with the natural morphism of temperoids
$\mathcal {G}$
with the natural morphism of temperoids
 $ \mathcal {G}_w \rightarrow \mathcal {B}^{\mathrm {tp}}((\mathcal {G}|_{\mathbb {H}_v})_{\succ T_v}) $
(cf. (vi)).
$ \mathcal {G}_w \rightarrow \mathcal {B}^{\mathrm {tp}}((\mathcal {G}|_{\mathbb {H}_v})_{\succ T_v}) $
(cf. (vi)).
We shall refer to this semi-graph of temperoids of HSD-type
![]() $\mathcal {G}_{\leadsto {S}}$
as the generization of
$\mathcal {G}_{\leadsto {S}}$
as the generization of
![]() $\mathcal {G}$
with respect to S.
$\mathcal {G}$
with respect to S.
Remark 2.9.1. One verifies immediately that the operation of generization discussed in Definition 2.9 is compatible (in the evident sense) with the corresponding pro-
![]() $\Sigma $
operation—that is, as discussed in [Reference Hoshi and Mochizuki9, Def. 2.8]—relative to the operation of passing to the associated semi-graph of anabelioids of pro-
$\Sigma $
operation—that is, as discussed in [Reference Hoshi and Mochizuki9, Def. 2.8]—relative to the operation of passing to the associated semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type (cf. Proposition 2.5(iii)).
$\Sigma $
PSC-type (cf. Proposition 2.5(iii)).
Remark 2.9.2. We take this opportunity to correct an unfortunate misprint in [Reference Hoshi and Mochizuki9, Def. 2.8(vii)]: the phrase “equivalent class” should read “equivalence class.”
Proposition 2.10 (Specialization outer isomorphisms).
Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type, and let
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type, and let
![]() $S \subseteq \mathrm {Node}(\mathcal {G})$
be a subset of
$S \subseteq \mathrm {Node}(\mathcal {G})$
be a subset of
![]() $\mathrm {Node}(\mathcal {G})$
. Write
$\mathrm {Node}(\mathcal {G})$
. Write
![]() $\Pi _{\mathcal {G}}$
for the fundamental group of
$\Pi _{\mathcal {G}}$
for the fundamental group of
![]() $\mathcal {G}$
and
$\mathcal {G}$
and
![]() $\Pi _{\mathcal {G}_{\leadsto {S}}}$
for the fundamental group of the generization
$\Pi _{\mathcal {G}_{\leadsto {S}}}$
for the fundamental group of the generization
![]() $\mathcal {G}_{\leadsto {S}}$
of
$\mathcal {G}_{\leadsto {S}}$
of
![]() $\mathcal {G}$
with respect to S (cf. Definition 2.9). Then there exists a natural outer isomorphism
$\mathcal {G}$
with respect to S (cf. Definition 2.9). Then there exists a natural outer isomorphism
which is functorial, in the evident sense, with respect to isomorphisms of the pair
![]() $(\mathcal {G}, S)$
and satisfies the following three conditions:
$(\mathcal {G}, S)$
and satisfies the following three conditions:
-
(a)
 $\Phi _{\mathcal {G}_{\leadsto {S}}}$
induces a bijection between the set of cuspidal subgroups (cf. Definition 2.6(i)) of
$\Phi _{\mathcal {G}_{\leadsto {S}}}$
induces a bijection between the set of cuspidal subgroups (cf. Definition 2.6(i)) of
 $\Pi _{\mathcal {G}_{\leadsto {S}}}$
and the set of cuspidal subgroups of
$\Pi _{\mathcal {G}_{\leadsto {S}}}$
and the set of cuspidal subgroups of
 $\Pi _{\mathcal {G}}$
.
$\Pi _{\mathcal {G}}$
. -
(b)
 $\Phi _{\mathcal {G}_{\leadsto {S}}}$
induces a bijection between the set of nodal subgroups (cf. Definition 2.6(i)) of
$\Phi _{\mathcal {G}_{\leadsto {S}}}$
induces a bijection between the set of nodal subgroups (cf. Definition 2.6(i)) of
 $\Pi _{\mathcal {G}_{\leadsto {S}}}$
and the set of nodal subgroups of
$\Pi _{\mathcal {G}_{\leadsto {S}}}$
and the set of nodal subgroups of
 $\Pi _{\mathcal {G}}$
associated with the elements of
$\Pi _{\mathcal {G}}$
associated with the elements of
 $\mathrm {Node}(\mathcal {G})\setminus S$
.
$\mathrm {Node}(\mathcal {G})\setminus S$
. -
(c) Let
 $v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
be a vertex of
$v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
be a vertex of
 $\mathcal {G}_{\leadsto {S}}$
;
$\mathcal {G}_{\leadsto {S}}$
;
 $\mathbb {H}_v$
,
$\mathbb {H}_v$
,
 $T_v$
as in Definition 2.9(vi). Then
$T_v$
as in Definition 2.9(vi). Then
 $\Phi _{\mathcal {G}_{\leadsto {S}}}$
induces a bijection between the
$\Phi _{\mathcal {G}_{\leadsto {S}}}$
induces a bijection between the
 $\Pi _{\mathcal {G}_{\leadsto {S}}}$
-conjugacy class of any verticial subgroup (cf. Definition 2.6(i))
$\Pi _{\mathcal {G}_{\leadsto {S}}}$
-conjugacy class of any verticial subgroup (cf. Definition 2.6(i))
 $\Pi _{v} \subseteq \Pi _{\mathcal {G}_{\leadsto {S}}}$
of
$\Pi _{v} \subseteq \Pi _{\mathcal {G}_{\leadsto {S}}}$
of
 $\Pi _{\mathcal {G}_{\leadsto {S}}}$
associated with
$\Pi _{\mathcal {G}_{\leadsto {S}}}$
associated with
 $v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
and the
$v \in \mathrm {Vert}(\mathcal {G}_{\leadsto {S}})$
and the
 $\Pi _{\mathcal {G}}$
-conjugacy class of subgroups obtained by forming the image of the outer homomorphism induced by the natural morphism
$\Pi _{\mathcal {G}}$
-conjugacy class of subgroups obtained by forming the image of the outer homomorphism induced by the natural morphism $$\begin{align*}\Pi_{(\mathcal{G}|_{\mathbb{H}_v})_{\succ T_v}} \longrightarrow \Pi_{\mathcal{G}} \end{align*}$$
$$\begin{align*}\Pi_{(\mathcal{G}|_{\mathbb{H}_v})_{\succ T_v}} \longrightarrow \Pi_{\mathcal{G}} \end{align*}$$
 $(\mathcal {G}|_{\mathbb {H}_v})_{\succ T_v} \rightarrow \mathcal {G}$
(cf. Definition 2.8(i) and (iii)) of semi-graphs of temperoids.
$(\mathcal {G}|_{\mathbb {H}_v})_{\succ T_v} \rightarrow \mathcal {G}$
(cf. Definition 2.8(i) and (iii)) of semi-graphs of temperoids.
We shall refer to this natural outer isomorphism
![]() $\Phi _{\mathcal {G}_{\leadsto {S}}}$
as the specialization outer isomorphism with respect to S.
$\Phi _{\mathcal {G}_{\leadsto {S}}}$
as the specialization outer isomorphism with respect to S.
Proof. An outer isomorphism that satisfies the three conditions in the statement of Proposition 2.10 may be obtained by observing that, after sorting through the various definitions involved, an object of
![]() $\mathcal {B}^{\mathrm {tp}}(\mathcal {G}_{\leadsto {S}})$
amounts to the same data as an object of
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G}_{\leadsto {S}})$
amounts to the same data as an object of
![]() $\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
. This completes the proof of Proposition 2.10.
$\mathcal {B}^{\mathrm {tp}}(\mathcal {G})$
. This completes the proof of Proposition 2.10.
Remark 2.10.1. One verifies immediately that the specialization outer isomorphism discussed in Proposition 2.10 is compatible (in the evident sense) with the corresponding pro-
![]() $\Sigma $
outer isomorphism—that is, as discussed in [Reference Hoshi and Mochizuki9, Prop. 2.9]—relative to the operation of passing to the associated semi-graph of anabelioids of pro-
$\Sigma $
outer isomorphism—that is, as discussed in [Reference Hoshi and Mochizuki9, Prop. 2.9]—relative to the operation of passing to the associated semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type (cf. Proposition 2.5(iii)).
$\Sigma $
PSC-type (cf. Proposition 2.5(iii)).
Lemma 2.11 (Infinite cyclic coverings).
Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Suppose that
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Suppose that
![]() $(\mathrm {Vert}(\mathcal {G})^\sharp , \mathrm {Node}(\mathcal {G})^\sharp ) = (1,1)$
, that is, the semi-graph of anabelioids of pro-
$(\mathrm {Vert}(\mathcal {G})^\sharp , \mathrm {Node}(\mathcal {G})^\sharp ) = (1,1)$
, that is, the semi-graph of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
![]() $\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
$\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) is cyclically primitive (cf. [Reference Hoshi and Mochizuki9, Def. 4.1]). Write
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) is cyclically primitive (cf. [Reference Hoshi and Mochizuki9, Def. 4.1]). Write
![]() $\Pi _{\mathcal {G}}$
for the fundamental group of
$\Pi _{\mathcal {G}}$
for the fundamental group of
![]() $\mathcal {G}$
;
$\mathcal {G}$
;
![]() $\mathbb {G}$
for the underlying semi-graph of
$\mathbb {G}$
for the underlying semi-graph of
![]() $\mathcal {G}$
;
$\mathcal {G}$
;
![]() $\Pi _{\mathbb {G}} (\cong \mathbb {Z})$
for the discrete topological fundamental group of
$\Pi _{\mathbb {G}} (\cong \mathbb {Z})$
for the discrete topological fundamental group of
![]() $\mathbb {G}$
;
$\mathbb {G}$
;
![]() $\mathcal {G}_\infty \rightarrow \mathcal {G}$
for the connected covering of
$\mathcal {G}_\infty \rightarrow \mathcal {G}$
for the connected covering of
![]() $\mathcal {G}$
(cf. Definition 2.1(vi)) corresponding to the natural surjection
$\mathcal {G}$
(cf. Definition 2.1(vi)) corresponding to the natural surjection
![]() $\Pi _{\mathcal {G}} \twoheadrightarrow \Pi _{\mathbb {G}}$
;
$\Pi _{\mathcal {G}} \twoheadrightarrow \Pi _{\mathbb {G}}$
;
![]() $\Pi _{\mathcal {G}_\infty } \stackrel {\mathrm {def}}{=} \mathrm {Ker}(\Pi _{\mathcal {G}} \twoheadrightarrow \Pi _{\mathbb {G}})$
. Then the following hold:
$\Pi _{\mathcal {G}_\infty } \stackrel {\mathrm {def}}{=} \mathrm {Ker}(\Pi _{\mathcal {G}} \twoheadrightarrow \Pi _{\mathbb {G}})$
. Then the following hold:
-
(i) Fix an isomorphism
 $\Pi _{\mathbb {G}}\stackrel {\sim }{\rightarrow } \mathbb {Z}$
. Then there exists a triple of bijections
$\Pi _{\mathbb {G}}\stackrel {\sim }{\rightarrow } \mathbb {Z}$
. Then there exists a triple of bijections  $$\begin{align*}V: \mathbb{Z} \stackrel{\sim}{\longrightarrow} \mathrm{Vert}(\mathcal{G}_\infty) , \, \, \, N: \mathbb{Z}\stackrel{\sim}{\longrightarrow} \mathrm{Node}(\mathcal{G}_\infty) ,\end{align*}$$
$$\begin{align*}V: \mathbb{Z} \stackrel{\sim}{\longrightarrow} \mathrm{Vert}(\mathcal{G}_\infty) , \, \, \, N: \mathbb{Z}\stackrel{\sim}{\longrightarrow} \mathrm{Node}(\mathcal{G}_\infty) ,\end{align*}$$
 $$\begin{align*}C:\mathbb{Z}\times\mathrm{Cusp}(\mathcal{G})\stackrel{\sim}{\longrightarrow}\mathrm{Cusp}(\mathcal{G}_\infty)\end{align*}$$
$$\begin{align*}C:\mathbb{Z}\times\mathrm{Cusp}(\mathcal{G})\stackrel{\sim}{\longrightarrow}\mathrm{Cusp}(\mathcal{G}_\infty)\end{align*}$$
(cf. Definition 2.1(v)) that satisfies the following properties:
-
• The bijections are equivariant with respect to the action of
 $\Pi _{\mathbb {G}}\stackrel {\sim }{\rightarrow } \mathbb {Z}$
on
$\Pi _{\mathbb {G}}\stackrel {\sim }{\rightarrow } \mathbb {Z}$
on
 $\mathbb {Z}$
by translations and the natural action of
$\mathbb {Z}$
by translations and the natural action of
 $\Pi _{\mathbb {G}}$
on “
$\Pi _{\mathbb {G}}$
on “
 $\mathrm {Vert}(-)$
,” “
$\mathrm {Vert}(-)$
,” “
 $\mathrm {Node}(-)$
,” “
$\mathrm {Node}(-)$
,” “
 $\mathrm {Cusp}(-)$
.”
$\mathrm {Cusp}(-)$
.” -
• The post-composite of C with the natural map
 $\mathrm {Cusp}(\mathcal {G}_\infty ) \rightarrow \mathrm {Cusp}(\mathcal {G})$
coincides with the projection
$\mathrm {Cusp}(\mathcal {G}_\infty ) \rightarrow \mathrm {Cusp}(\mathcal {G})$
coincides with the projection
 $\mathbb {Z}\times \mathrm {Cusp}(\mathcal {G}) \rightarrow \mathrm {Cusp}(\mathcal {G})$
to the second factor.
$\mathbb {Z}\times \mathrm {Cusp}(\mathcal {G}) \rightarrow \mathrm {Cusp}(\mathcal {G})$
to the second factor. -
• For each
 $a\in \mathbb {Z}$
, it holds that
$a\in \mathbb {Z}$
, it holds that
 $\mathcal {E}(V(a)) = \{ N(a), N(a+1)\}\sqcup \{ \, C(a, z) \, | \, z \in \, \mathrm {Cusp}(\mathcal {G}) \, \}$
(cf. Definition 2.1(v)).
$\mathcal {E}(V(a)) = \{ N(a), N(a+1)\}\sqcup \{ \, C(a, z) \, | \, z \in \, \mathrm {Cusp}(\mathcal {G}) \, \}$
(cf. Definition 2.1(v)).
Moreover, such a triple of bijections is unique, up to post-composition with the automorphisms of “
 $\mathrm {Vert}(-)$
,” “
$\mathrm {Vert}(-)$
,” “
 $\mathrm {Node}(-)$
,” “
$\mathrm {Node}(-)$
,” “
 $\mathrm {Cusp}(-)$
” determined by the action of a (single!) element of
$\mathrm {Cusp}(-)$
” determined by the action of a (single!) element of
 $\Pi _{\mathbb {G}}$
.
$\Pi _{\mathbb {G}}$
. -
-
(ii) Let
 $a\leq b$
be integers. Write
$a\leq b$
be integers. Write
 $\mathbb {G}_{[a,b]}$
for the (uniquely determined) sub-semi-graph of PSC-type (cf. [Reference Hoshi and Mochizuki9, Def. 2.2(i)]) of the underlying semi-graph of
$\mathbb {G}_{[a,b]}$
for the (uniquely determined) sub-semi-graph of PSC-type (cf. [Reference Hoshi and Mochizuki9, Def. 2.2(i)]) of the underlying semi-graph of
 $\mathcal {G}_\infty $
whose set of vertices is equal to
$\mathcal {G}_\infty $
whose set of vertices is equal to
 $\{V(a), V(a+1) , \dots , V(b)\}$
(cf. (i)). Also, write
$\{V(a), V(a+1) , \dots , V(b)\}$
(cf. (i)). Also, write
 $\mathcal {G}_{[a,b]}$
for the semi-graph of temperoids obtained by restricting
$\mathcal {G}_{[a,b]}$
for the semi-graph of temperoids obtained by restricting
 $\mathcal {G}_\infty $
to
$\mathcal {G}_\infty $
to
 $\mathbb {G}_{[a,b]}$
(in the evident sense—cf. also the procedure discussed in Definition 2.8(i)). Then
$\mathbb {G}_{[a,b]}$
(in the evident sense—cf. also the procedure discussed in Definition 2.8(i)). Then
 $\mathcal {G}_{[a,b]}$
is a semi-graph of temperoids of HSD-type.
$\mathcal {G}_{[a,b]}$
is a semi-graph of temperoids of HSD-type. -
(iii) Let
 $a\leq b$
be integers. For an integer c such that
$a\leq b$
be integers. For an integer c such that
 $a\leq c\leq b$
(resp.
$a\leq c\leq b$
(resp.
 $a+1\leq c\leq b$
), let
$a+1\leq c\leq b$
), let
 $\Pi _{V(c)} \subseteq \Pi _{\mathcal {G}_{[a,b]}}$
(resp.
$\Pi _{V(c)} \subseteq \Pi _{\mathcal {G}_{[a,b]}}$
(resp.
 $\Pi _{N(c)} \subseteq \Pi _{\mathcal {G}_{[a,b]}}$
) be a verticial (resp. nodal) subgroup of
$\Pi _{N(c)} \subseteq \Pi _{\mathcal {G}_{[a,b]}}$
) be a verticial (resp. nodal) subgroup of
 $\Pi _{\mathcal {G}_{[a,b]}}$
associated with
$\Pi _{\mathcal {G}_{[a,b]}}$
associated with
 $V(c) \in \mathrm {Vert}(\mathcal {G}_{[a,b]})$
(resp.
$V(c) \in \mathrm {Vert}(\mathcal {G}_{[a,b]})$
(resp.
 $N(c) \in \mathrm {Node}(\mathcal {G}_{[a,b]})$
) (cf. (i) and (ii)) such that, for
$N(c) \in \mathrm {Node}(\mathcal {G}_{[a,b]})$
) (cf. (i) and (ii)) such that, for
 $a+1\leq c\leq b$
, it holds that
$a+1\leq c\leq b$
, it holds that
 $\Pi _{N(c)} \subseteq \Pi _{V(c-1)} \cap \Pi _{V(c)}$
. Then the inclusions
$\Pi _{N(c)} \subseteq \Pi _{V(c-1)} \cap \Pi _{V(c)}$
. Then the inclusions
 $\Pi _{V(c)}$
,
$\Pi _{V(c)}$
,
 $\Pi _{N(c)} \hookrightarrow \Pi _{\mathcal {G}_{[a,b]}}$
determine an isomorphism
$\Pi _{N(c)} \hookrightarrow \Pi _{\mathcal {G}_{[a,b]}}$
determine an isomorphism  $$\begin{align*}\varinjlim \, \bigl( \Pi_{V(a)} \hookleftarrow \Pi_{N(a+1)} \hookrightarrow \Pi_{V(a+1)} \hookleftarrow \cdots \hookrightarrow \Pi_{V(b-1)} \hookleftarrow \Pi_{N(b)} \hookrightarrow \Pi_{V(b)} \bigr) \end{align*}$$
where
$$\begin{align*}\varinjlim \, \bigl( \Pi_{V(a)} \hookleftarrow \Pi_{N(a+1)} \hookrightarrow \Pi_{V(a+1)} \hookleftarrow \cdots \hookrightarrow \Pi_{V(b-1)} \hookleftarrow \Pi_{N(b)} \hookrightarrow \Pi_{V(b)} \bigr) \end{align*}$$
where $$\begin{align*}\stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}_{[a,b]}}, \end{align*}$$
$$\begin{align*}\stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}_{[a,b]}}, \end{align*}$$
 $\varinjlim $
denotes the inductive limit in the category of groups.
$\varinjlim $
denotes the inductive limit in the category of groups.
-
(iv) Let
 $a\leq b$
be integers. Then the composite
$a\leq b$
be integers. Then the composite
 $\mathcal {G}_{[a,b]} \rightarrow \mathcal {G}_\infty \rightarrow \mathcal {G}$
determines an outer injection
$\mathcal {G}_{[a,b]} \rightarrow \mathcal {G}_\infty \rightarrow \mathcal {G}$
determines an outer injection
 $\Pi _{\mathcal {G}_{[a,b]}} \hookrightarrow \Pi _{\mathcal {G}}$
. Moreover, the image of this outer injection is contained in the normal subgroup
$\Pi _{\mathcal {G}_{[a,b]}} \hookrightarrow \Pi _{\mathcal {G}}$
. Moreover, the image of this outer injection is contained in the normal subgroup
 $\Pi _{\mathcal {G}_\infty } \subseteq \Pi _{\mathcal {G}}$
.
$\Pi _{\mathcal {G}_\infty } \subseteq \Pi _{\mathcal {G}}$
. -
(v) There exists a collection
of subgroups $$\begin{align*}\{ D_{[-a, a]} \}_{1\le a \in \mathbb{Z}} \end{align*}$$
$$\begin{align*}\{ D_{[-a, a]} \}_{1\le a \in \mathbb{Z}} \end{align*}$$
 $D_{[-a, a]}\subseteq \Pi _{\mathcal {G}_\infty }$
indexed by the positive integers which satisfy the following properties:
$D_{[-a, a]}\subseteq \Pi _{\mathcal {G}_\infty }$
indexed by the positive integers which satisfy the following properties:
-
•
 $D_{[-a, a]}\subseteq \Pi _{\mathcal {G}_\infty }$
belongs to the
$D_{[-a, a]}\subseteq \Pi _{\mathcal {G}_\infty }$
belongs to the
 $\Pi _{\mathcal {G}}$
-conjugacy class (of subgroups of
$\Pi _{\mathcal {G}}$
-conjugacy class (of subgroups of
 $\Pi _{\mathcal {G}}$
) obtained by forming the image of the outer injection
$\Pi _{\mathcal {G}}$
) obtained by forming the image of the outer injection
 $\Pi _{\mathcal {G}_{[-a,a]}} \hookrightarrow \Pi _{\mathcal {G}}$
of (iv).
$\Pi _{\mathcal {G}_{[-a,a]}} \hookrightarrow \Pi _{\mathcal {G}}$
of (iv). -
•
 $D_{[-a, a]} \subseteq D_{[-a-1, a+1]}$
.
$D_{[-a, a]} \subseteq D_{[-a-1, a+1]}$
. -
• The inclusions
 $D_{[-a, a]} \hookrightarrow \Pi _{\mathcal {G}}$
(where a ranges over the positive integers) determine an isomorphism where
$D_{[-a, a]} \hookrightarrow \Pi _{\mathcal {G}}$
(where a ranges over the positive integers) determine an isomorphism where $$\begin{align*}\varinjlim \, \bigl( D_{[-1, 1]} \hookrightarrow D_{[-2, 2]} \hookrightarrow D_{[-3, 3]} \hookrightarrow \cdots \bigr) \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}_{\infty}}, \end{align*}$$
$$\begin{align*}\varinjlim \, \bigl( D_{[-1, 1]} \hookrightarrow D_{[-2, 2]} \hookrightarrow D_{[-3, 3]} \hookrightarrow \cdots \bigr) \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}_{\infty}}, \end{align*}$$
 $\varinjlim $
denotes the inductive limit in the category of groups.
$\varinjlim $
denotes the inductive limit in the category of groups.
-
-
(vi) In the situation of (v), since
 $\Pi _{\mathcal {G}}$
injects into its pro-l completion for any
$\Pi _{\mathcal {G}}$
injects into its pro-l completion for any
 $l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Remark 2.5.1), let us regard subgroups of
$l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf. Remark 2.5.1), let us regard subgroups of
 $\Pi _{\mathcal {G}}$
as subgroups of the pro-
$\Pi _{\mathcal {G}}$
as subgroups of the pro-
 $\Sigma $
completion
$\Sigma $
completion
 $\Pi _{\mathcal {G}}^{\Sigma }$
of
$\Pi _{\mathcal {G}}^{\Sigma }$
of
 $\Pi _{\mathcal {G}}$
. Let a be a positive integer. Write
$\Pi _{\mathcal {G}}$
. Let a be a positive integer. Write
 $\overline {D}_{[-a, a]} \subseteq \Pi _{\mathcal {G}}^{\Sigma }$
for the closure of
$\overline {D}_{[-a, a]} \subseteq \Pi _{\mathcal {G}}^{\Sigma }$
for the closure of
 $D_{[-a, a]}$
in
$D_{[-a, a]}$
in
 $\Pi _{\mathcal {G}}^{\Sigma }$
. Let
$\Pi _{\mathcal {G}}^{\Sigma }$
. Let
 $\widehat {\gamma } \in \Pi _{\mathcal {G}}^{\Sigma }$
. Suppose that
$\widehat {\gamma } \in \Pi _{\mathcal {G}}^{\Sigma }$
. Suppose that
 $\overline {D}_{[a, -a]} \cap \widehat {\gamma } \cdot \overline {D}_{[a, -a]} \cdot \widehat {\gamma }^{-1} \neq \{1\}$
. Then the image of
$\overline {D}_{[a, -a]} \cap \widehat {\gamma } \cdot \overline {D}_{[a, -a]} \cdot \widehat {\gamma }^{-1} \neq \{1\}$
. Then the image of
 $\widehat {\gamma } \in \Pi _{\mathcal {G}}^{\Sigma }$
in the pro-
$\widehat {\gamma } \in \Pi _{\mathcal {G}}^{\Sigma }$
in the pro-
 $\Sigma $
completion
$\Sigma $
completion
 $\Pi _{\mathbb {G}}^{\Sigma }$
of
$\Pi _{\mathbb {G}}^{\Sigma }$
of
 $\Pi _{\mathbb {G}}$
is contained in
$\Pi _{\mathbb {G}}$
is contained in
 $\Pi _{\mathbb {G}}\subseteq \Pi _{\mathbb {G}}^{\Sigma }$
.
$\Pi _{\mathbb {G}}\subseteq \Pi _{\mathbb {G}}^{\Sigma }$
. -
(vii) In the situation of (vi), suppose, moreover, that
 $\widehat {\gamma }$
is contained in the closure
$\widehat {\gamma }$
is contained in the closure
 $\overline {\Pi }_{\mathcal {G}_\infty } \subseteq \Pi _{\mathcal {G}}^{\Sigma }$
of
$\overline {\Pi }_{\mathcal {G}_\infty } \subseteq \Pi _{\mathcal {G}}^{\Sigma }$
of
 $\Pi _{\mathcal {G}_\infty }$
in
$\Pi _{\mathcal {G}_\infty }$
in
 $\Pi _{\mathcal {G}}^{\Sigma }$
. Then
$\Pi _{\mathcal {G}}^{\Sigma }$
. Then
 $\widehat {\gamma } \in \overline {D}_{[a, -a]}$
.
$\widehat {\gamma } \in \overline {D}_{[a, -a]}$
.
Proof. Assertions (i) and (ii) follow immediately from the various definitions involved. Assertion (iii) follows immediately from a similar argument to the argument applied in the proof of [Reference Mochizuki20, Prop. 1.5(iii)]. Next, we verify assertion (iv). The injectivity portion of assertion (iv) follows immediately—by considering a suitable finite étale subcovering of
![]() $\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—from Proposition 2.5(iv). The remainder of assertion (iv) follows immediately from the various definitions involved. This completes the proof of assertion (iv). Assertion (v) follows immediately from assertion (iii).
$\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—from Proposition 2.5(iv). The remainder of assertion (iv) follows immediately from the various definitions involved. This completes the proof of assertion (iv). Assertion (v) follows immediately from assertion (iii).
Next, we verify assertion (vi). Write
![]() $\mathcal {G}^{\Sigma }$
for the semi-graph of anabelioids of pro-
$\mathcal {G}^{\Sigma }$
for the semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type determined by
$\Sigma $
PSC-type determined by
![]() $\mathcal {G}$
(cf. Proposition 2.5(iii)),
$\mathcal {G}$
(cf. Proposition 2.5(iii)),
![]() $\widetilde {\mathcal {G}}^{\Sigma } \rightarrow \mathcal {G}^{\Sigma }$
for the universal covering of the semi-graph of anabelioids of pro-
$\widetilde {\mathcal {G}}^{\Sigma } \rightarrow \mathcal {G}^{\Sigma }$
for the universal covering of the semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type
$\Sigma $
PSC-type
![]() $\mathcal {G}^{\Sigma }$
corresponding to (the torsion-free group)
$\mathcal {G}^{\Sigma }$
corresponding to (the torsion-free group)
![]() $\Pi _{\mathcal {G}}^{\Sigma }$
(cf. Proposition 2.5(iii); [Reference Mochizuki and Tamagawa23, Rem. 1.2.2]), and
$\Pi _{\mathcal {G}}^{\Sigma }$
(cf. Proposition 2.5(iii); [Reference Mochizuki and Tamagawa23, Rem. 1.2.2]), and
![]() $\widetilde {\mathbb {G}} ^{\Sigma }$
for the underlying pro-semi-graph of
$\widetilde {\mathbb {G}} ^{\Sigma }$
for the underlying pro-semi-graph of
![]() $\widetilde {\mathcal {G}}^{\Sigma }$
. Then it follows immediately—that is, by considering a suitable finite étale subcovering of
$\widetilde {\mathcal {G}}^{\Sigma }$
. Then it follows immediately—that is, by considering a suitable finite étale subcovering of
![]() $\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—from [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)] that our assumption that
$\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—from [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)] that our assumption that
![]() $\overline {D}_{[a, -a]} \cap \widehat {\gamma } \cdot \overline {D}_{[a, -a]} \cdot \widehat {\gamma }^{-1} \neq \{1\}$
implies that the respective sub-pro-semi-graphs of
$\overline {D}_{[a, -a]} \cap \widehat {\gamma } \cdot \overline {D}_{[a, -a]} \cdot \widehat {\gamma }^{-1} \neq \{1\}$
implies that the respective sub-pro-semi-graphs of
![]() $\widetilde {\mathbb {G}}^{\Sigma }$
determined by
$\widetilde {\mathbb {G}}^{\Sigma }$
determined by
![]() $\overline {D}_{[a, -a]}$
,
$\overline {D}_{[a, -a]}$
,
![]() $\widehat {\gamma } \cdot \overline {D}_{[a, -a]} \cdot \widehat {\gamma }^{-1} \subseteq \Pi _{\mathcal {G}}^{\Sigma }$
(cf. Proposition 2.5(v)) either contain a common pro-vertex or may be joined to one another by a single pro-edge. But this implies that
$\widehat {\gamma } \cdot \overline {D}_{[a, -a]} \cdot \widehat {\gamma }^{-1} \subseteq \Pi _{\mathcal {G}}^{\Sigma }$
(cf. Proposition 2.5(v)) either contain a common pro-vertex or may be joined to one another by a single pro-edge. But this implies that
![]() $\widehat {\gamma }$
maps
$\widehat {\gamma }$
maps
![]() $\mathbb {G}_{[-a,a]}$
to some
$\mathbb {G}_{[-a,a]}$
to some
![]() $\Pi _{\mathbb {G}}$
-translate of
$\Pi _{\mathbb {G}}$
-translate of
![]() $\mathbb {G}_{[-a,a]}$
, hence, in particular, that the image of
$\mathbb {G}_{[-a,a]}$
, hence, in particular, that the image of
![]() $\widehat {\gamma } \in \Pi _{\mathcal {G}}^{\Sigma }$
in
$\widehat {\gamma } \in \Pi _{\mathcal {G}}^{\Sigma }$
in
![]() $\Pi _{\mathbb {G}}^{\Sigma }$
is contained in
$\Pi _{\mathbb {G}}^{\Sigma }$
is contained in
![]() $\Pi _{\mathbb {G}}\subseteq \Pi _{\mathbb {G}}^{\Sigma }$
, as desired. This completes the proof of assertion (vi). Assertion (vii) follows immediately—that is, by considering a suitable finite étale subcovering of
$\Pi _{\mathbb {G}}\subseteq \Pi _{\mathbb {G}}^{\Sigma }$
, as desired. This completes the proof of assertion (vi). Assertion (vii) follows immediately—that is, by considering a suitable finite étale subcovering of
![]() $\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—from the commensurable terminality (cf. [Reference Mochizuki18, Prop. 1.2(ii)]) of
$\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—from the commensurable terminality (cf. [Reference Mochizuki18, Prop. 1.2(ii)]) of
![]() $\overline {D}_{[a, -a]}$
in a suitable open subgroup of
$\overline {D}_{[a, -a]}$
in a suitable open subgroup of
![]() $\Pi _{\mathcal {G}}^{\Sigma }$
containing
$\Pi _{\mathcal {G}}^{\Sigma }$
containing
![]() $\overline {\Pi }_{\mathcal {G}_\infty }$
(cf. also [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)]). This completes the proof of Lemma 2.11.
$\overline {\Pi }_{\mathcal {G}_\infty }$
(cf. also [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)]). This completes the proof of Lemma 2.11.
The content of the following lemma is entirely elementary and well-known.
Lemma 2.12 (Action of the symplectic group).
Let g be a positive integer. For each positive integer n and
![]() $v = (v_1 , \dots , v_n) \in \mathbb {Z}^{\oplus n}$
, write
$v = (v_1 , \dots , v_n) \in \mathbb {Z}^{\oplus n}$
, write
![]() $\mathrm {vol}(v) \in \mathbb {Z}$
for the (uniquely determined) nonnegative integer that generates the ideal
$\mathrm {vol}(v) \in \mathbb {Z}$
for the (uniquely determined) nonnegative integer that generates the ideal
![]() $ \mathbb {Z}\cdot v_1 + \cdots + \mathbb {Z}\cdot v_n \subseteq \mathbb {Z}$
;
$ \mathbb {Z}\cdot v_1 + \cdots + \mathbb {Z}\cdot v_n \subseteq \mathbb {Z}$
;
![]() $M_n(\mathbb {Z})$
for the set of n by n matrices with coefficients in
$M_n(\mathbb {Z})$
for the set of n by n matrices with coefficients in
![]() $\mathbb {Z}$
;
$\mathbb {Z}$
;
![]() $\mathrm {GL}_n(\mathbb {Z}) \subseteq M_n(\mathbb {Z})$
for the group of matrices
$\mathrm {GL}_n(\mathbb {Z}) \subseteq M_n(\mathbb {Z})$
for the group of matrices
![]() $A \in M_n(\mathbb {Z})$
such that
$A \in M_n(\mathbb {Z})$
such that
![]() $\det (A) \in \{1, -1\}$
;
$\det (A) \in \{1, -1\}$
;
![]() $\mathrm {Sp}_{2g}(\mathbb {Z}) \subseteq \mathrm {GL}_{2g}(\mathbb {Z})$
for the subgroup of
$\mathrm {Sp}_{2g}(\mathbb {Z}) \subseteq \mathrm {GL}_{2g}(\mathbb {Z})$
for the subgroup of
![]() $2g$
by
$2g$
by
![]() $2g$
symplectic matrices, that is,
$2g$
symplectic matrices, that is,
![]() $B \in \mathrm {GL}_{2g}(\mathbb {Z})$
such that
$B \in \mathrm {GL}_{2g}(\mathbb {Z})$
such that
 $$ \begin{align*}B\cdot \begin{pmatrix} 0 & 1 \\ -1 & 0 \end{pmatrix} \cdot {}^t \! B = \begin{pmatrix} 0 & 1 \\ -1 & 0 \end{pmatrix}. \end{align*} $$
$$ \begin{align*}B\cdot \begin{pmatrix} 0 & 1 \\ -1 & 0 \end{pmatrix} \cdot {}^t \! B = \begin{pmatrix} 0 & 1 \\ -1 & 0 \end{pmatrix}. \end{align*} $$
(Note that one verifies immediately that, for every
![]() $A \in \mathrm {GL}_n(\mathbb {Z})$
, it holds that
$A \in \mathrm {GL}_n(\mathbb {Z})$
, it holds that
![]() $\mathrm {vol}(v) = \mathrm {vol}(vA)$
.) Then the following hold:
$\mathrm {vol}(v) = \mathrm {vol}(vA)$
.) Then the following hold:
-
(i) Let
 $v= (v_1 , \dots , v_{g}) \in \mathbb {Z}^{\oplus g}$
. Then there exists an invertible matrix
$v= (v_1 , \dots , v_{g}) \in \mathbb {Z}^{\oplus g}$
. Then there exists an invertible matrix
 $A\in \mathrm {GL}_{g}(\mathbb {Z})$
such that
$A\in \mathrm {GL}_{g}(\mathbb {Z})$
such that
 $vA = (\mathrm {vol}(v), \overbrace {0 , \dots , 0}^{g-1})$
.
$vA = (\mathrm {vol}(v), \overbrace {0 , \dots , 0}^{g-1})$
. -
(ii) Let
 $v= (v_1 , \dots , v_{2g}) \in \mathbb {Z}^{\oplus 2g}$
. Then there exists a symplectic matrix
$v= (v_1 , \dots , v_{2g}) \in \mathbb {Z}^{\oplus 2g}$
. Then there exists a symplectic matrix
 $B\in \mathrm {Sp}_{2g}(\mathbb {Z})$
such that
$B\in \mathrm {Sp}_{2g}(\mathbb {Z})$
such that
 $vB = (\mathrm {vol}(v), \overbrace {0 , \dots , 0}^{2g-1})$
.
$vB = (\mathrm {vol}(v), \overbrace {0 , \dots , 0}^{2g-1})$
. -
(iii) Let
 $N\subseteq \mathbb {Z}^{\oplus 2g}$
be a submodule of
$N\subseteq \mathbb {Z}^{\oplus 2g}$
be a submodule of
 $\mathbb {Z}^{\oplus 2g}$
and
$\mathbb {Z}^{\oplus 2g}$
and
 $v \in \mathbb {Z}^{\oplus 2g}$
. Suppose that
$v \in \mathbb {Z}^{\oplus 2g}$
. Suppose that
 $N\neq \{0\}$
. Then there exist a nonzero integer
$N\neq \{0\}$
. Then there exist a nonzero integer
 $n\in \mathbb {Z}\setminus \{0\}$
and a symplectic matrix
$n\in \mathbb {Z}\setminus \{0\}$
and a symplectic matrix
 $B \in \mathrm {Sp}_{2g}(\mathbb {Z})$
such that
$B \in \mathrm {Sp}_{2g}(\mathbb {Z})$
such that
 $n\cdot vB \in N$
.
$n\cdot vB \in N$
. -
(iv) Let
 $N\subseteq \mathbb {Z}^{\oplus 2g}$
be a submodule of
$N\subseteq \mathbb {Z}^{\oplus 2g}$
be a submodule of
 $\mathbb {Z}^{\oplus 2g}$
and
$\mathbb {Z}^{\oplus 2g}$
and
 $\pi \colon \mathbb {Z}^{\oplus 2g} \twoheadrightarrow \mathbb {Z}$
a surjection. Suppose that N is of infinite index in
$\pi \colon \mathbb {Z}^{\oplus 2g} \twoheadrightarrow \mathbb {Z}$
a surjection. Suppose that N is of infinite index in
 $\mathbb {Z}^{\oplus 2g}$
. Then there exists a symplectic matrix
$\mathbb {Z}^{\oplus 2g}$
. Then there exists a symplectic matrix
 $B \in \mathrm {Sp}_{2g}(\mathbb {Z})$
such that
$B \in \mathrm {Sp}_{2g}(\mathbb {Z})$
such that
 $N\cdot B \subseteq \mathrm {Ker}(\pi )$
.
$N\cdot B \subseteq \mathrm {Ker}(\pi )$
.
Proof. First, we verify assertion (i). Let us first observe that if
![]() $v = 0$
(i.e.,
$v = 0$
(i.e.,
![]() $\mathrm {vol}(v) = 0$
), then assertion (i) is immediate. Thus, to verify assertion (i), we may assume without loss of generality that
$\mathrm {vol}(v) = 0$
), then assertion (i) is immediate. Thus, to verify assertion (i), we may assume without loss of generality that
![]() $v\neq 0$
. In particular, to verify assertion (i), by replacing v by
$v\neq 0$
. In particular, to verify assertion (i), by replacing v by
![]() $\mathrm {vol}(v)^{-1}\cdot v \in \mathbb {Z}^{\oplus g}$
, we may assume without loss of generality that
$\mathrm {vol}(v)^{-1}\cdot v \in \mathbb {Z}^{\oplus g}$
, we may assume without loss of generality that
![]() $\mathrm {vol}(v) = 1$
. On the other hand, since
$\mathrm {vol}(v) = 1$
. On the other hand, since
![]() $\mathrm {vol}(v) = 1$
, one verifies immediately that
$\mathrm {vol}(v) = 1$
, one verifies immediately that
![]() $\mathbb {Z}^{\oplus g} / (\mathbb {Z}\cdot v)$
is a free
$\mathbb {Z}^{\oplus g} / (\mathbb {Z}\cdot v)$
is a free
![]() $\mathbb {Z}$
-module of rank
$\mathbb {Z}$
-module of rank
![]() $g-1$
, hence that there exists an injection
$g-1$
, hence that there exists an injection
![]() $\mathbb {Z}^{\oplus g-1} \hookrightarrow \mathbb {Z}^{\oplus g}$
that induces an isomorphism
$\mathbb {Z}^{\oplus g-1} \hookrightarrow \mathbb {Z}^{\oplus g}$
that induces an isomorphism
![]() $(\mathbb {Z}\cdot v) \oplus \mathbb {Z}^{\oplus g-1} \stackrel {\sim }{\rightarrow } \mathbb {Z}^{\oplus g}$
. This completes the proof of assertion (i).
$(\mathbb {Z}\cdot v) \oplus \mathbb {Z}^{\oplus g-1} \stackrel {\sim }{\rightarrow } \mathbb {Z}^{\oplus g}$
. This completes the proof of assertion (i).
Next, we verify assertion (ii). Since (one verifies easily that)
![]() $\mathrm {Sp}_2(\mathbb {Z}) = \mathrm {SL}_2(\mathbb {Z}) = \{ \,B \in \mathrm {GL}_2(\mathbb {Z}) \,| \,\det (B) = 1 \, \}$
, assertion (ii) in the case where
$\mathrm {Sp}_2(\mathbb {Z}) = \mathrm {SL}_2(\mathbb {Z}) = \{ \,B \in \mathrm {GL}_2(\mathbb {Z}) \,| \,\det (B) = 1 \, \}$
, assertion (ii) in the case where
![]() $g = 1$
follows immediately from assertion (i) (in the case where we take “g” in assertion (i) to be
$g = 1$
follows immediately from assertion (i) (in the case where we take “g” in assertion (i) to be
![]() $2$
), together with the (easily verified) fact that
$2$
), together with the (easily verified) fact that
 $$\begin{align*}\bigl\{ \det \begin{pmatrix} a & b \\ c & d \end{pmatrix}, \, \, \det \begin{pmatrix} a & -b \\ c & -d \end{pmatrix} \bigr\} = \{1,-1\} \, \, \text{for every} \, \, \begin{pmatrix} a & b \\ c & d \end{pmatrix} \in \mathrm{GL}_2(\mathbb{Z}). \end{align*}$$
$$\begin{align*}\bigl\{ \det \begin{pmatrix} a & b \\ c & d \end{pmatrix}, \, \, \det \begin{pmatrix} a & -b \\ c & -d \end{pmatrix} \bigr\} = \{1,-1\} \, \, \text{for every} \, \, \begin{pmatrix} a & b \\ c & d \end{pmatrix} \in \mathrm{GL}_2(\mathbb{Z}). \end{align*}$$
For
![]() $i\in \{1, \dots , g\}$
, write
$i\in \{1, \dots , g\}$
, write
![]() $M_i$
for the submodule of
$M_i$
for the submodule of
![]() $\mathbb {Z}^{\oplus 2g}$
generated by
$\mathbb {Z}^{\oplus 2g}$
generated by
where the “
![]() $1$
’s” lie, respectively, in the ith and
$1$
’s” lie, respectively, in the ith and
![]() $(g+i)$
th components. Then, by applying assertion (ii) in the case where
$(g+i)$
th components. Then, by applying assertion (ii) in the case where
![]() $g = 1$
(already verified above) to the
$g = 1$
(already verified above) to the
![]() $M_i$
’s, we conclude that, to complete the verification of assertion (ii), we may assume without loss of generality that
$M_i$
’s, we conclude that, to complete the verification of assertion (ii), we may assume without loss of generality that
![]() $v_{i} = 0$
for every
$v_{i} = 0$
for every
![]() $g+1 \leq i \leq 2g$
. Write
$g+1 \leq i \leq 2g$
. Write
![]() $v_{\leq g} \stackrel {\mathrm {def}}{=} (v_1 , \dots , v_g) \in \mathbb {Z}^{\oplus g}$
. Then let us observe that it follows from assertion (i) that there exists an invertible matrix
$v_{\leq g} \stackrel {\mathrm {def}}{=} (v_1 , \dots , v_g) \in \mathbb {Z}^{\oplus g}$
. Then let us observe that it follows from assertion (i) that there exists an invertible matrix
![]() $A \in \mathrm {GL}_g(\mathbb {Z})$
such that
$A \in \mathrm {GL}_g(\mathbb {Z})$
such that
![]() $v_{\leq g} A = (\mathrm {vol}(v_{\leq g}), 0 , \dots , 0) =(\mathrm {vol}(v), 0 , \dots , 0)$
. Thus, assertion (ii) follows immediately from the (easily verified) fact that
$v_{\leq g} A = (\mathrm {vol}(v_{\leq g}), 0 , \dots , 0) =(\mathrm {vol}(v), 0 , \dots , 0)$
. Thus, assertion (ii) follows immediately from the (easily verified) fact that
 $$ \begin{align*}\begin{pmatrix} A & 0 \\ 0 & {}^t\! A ^{-1} \end{pmatrix} \in \mathrm{Sp}_{2g}(\mathbb{Z}). \end{align*} $$
$$ \begin{align*}\begin{pmatrix} A & 0 \\ 0 & {}^t\! A ^{-1} \end{pmatrix} \in \mathrm{Sp}_{2g}(\mathbb{Z}). \end{align*} $$
This completes the proof of assertion (ii).
Assertion (iii) follows immediately from assertion (ii). Assertion (iv) follows immediately—by applying the self-duality of
![]() $\mathbb {Z}^{\oplus 2g}$
with respect to the symplectic form determined by
$\mathbb {Z}^{\oplus 2g}$
with respect to the symplectic form determined by
 $ \begin {pmatrix} 0 & 1 \\ -1 & 0 \end {pmatrix} $
—from assertion (iii). This completes the proof of Lemma 2.12.
$ \begin {pmatrix} 0 & 1 \\ -1 & 0 \end {pmatrix} $
—from assertion (iii). This completes the proof of Lemma 2.12.
Lemma 2.13 (Automorphisms of surface groups).
Let g be a positive integer,
![]() $\Pi _{}$
the topological fundamental group of a connected orientable compact topological surface of genus g,
$\Pi _{}$
the topological fundamental group of a connected orientable compact topological surface of genus g,
![]() $\pi \colon \Pi _{} \twoheadrightarrow \mathbb {Z}$
a surjection, and
$\pi \colon \Pi _{} \twoheadrightarrow \mathbb {Z}$
a surjection, and
![]() $J\subseteq \Pi _{}$
a subgroup of
$J\subseteq \Pi _{}$
a subgroup of
![]() $\Pi _{}$
such that the image of J in
$\Pi _{}$
such that the image of J in
![]() $\Pi _{}^{\mathrm {ab}}$
is of infinite index in
$\Pi _{}^{\mathrm {ab}}$
is of infinite index in
![]() $\Pi _{}^{\mathrm {ab}}$
. (For example, this will be the case if J is generated by
$\Pi _{}^{\mathrm {ab}}$
. (For example, this will be the case if J is generated by
![]() $2g-1$
elements.) Then there exists an automorphism
$2g-1$
elements.) Then there exists an automorphism
![]() $\sigma $
of
$\sigma $
of
![]() $\Pi _{}$
such that
$\Pi _{}$
such that
![]() $\sigma (J) \subseteq \mathrm {Ker}(\pi )$
.
$\sigma (J) \subseteq \mathrm {Ker}(\pi )$
.
Proof. Write
![]() $H \stackrel {\mathrm {def}}{=} \mathrm {Hom}(\Pi ,\mathbb {Z}) = \mathrm {Hom}_{\mathbb {Z}}(\Pi ^{\mathrm {ab}},\mathbb {Z})$
. Let us fix isomorphisms
$H \stackrel {\mathrm {def}}{=} \mathrm {Hom}(\Pi ,\mathbb {Z}) = \mathrm {Hom}_{\mathbb {Z}}(\Pi ^{\mathrm {ab}},\mathbb {Z})$
. Let us fix isomorphisms
![]() $H\stackrel {\sim }{\rightarrow } \mathbb {Z}^{\oplus 2g}$
and
$H\stackrel {\sim }{\rightarrow } \mathbb {Z}^{\oplus 2g}$
and
![]() $H^2(\Pi ,\mathbb {Z}) \stackrel {\sim }{\rightarrow } \mathbb {Z}$
. Then it follows from the well-known theory of Poincaré duality that the cup product in group cohomology
$H^2(\Pi ,\mathbb {Z}) \stackrel {\sim }{\rightarrow } \mathbb {Z}$
. Then it follows from the well-known theory of Poincaré duality that the cup product in group cohomology
determines a perfect pairing on H; moreover, if we write
![]() $\mathrm {Aut}_{\mathrm {PD}}(H)\subseteq \mathrm {Aut}(H)$
(
$\mathrm {Aut}_{\mathrm {PD}}(H)\subseteq \mathrm {Aut}(H)$
(
![]() $\stackrel {\sim }{\rightarrow } \mathrm {GL}_{2g}(\mathbb {Z})$
—cf. the notation of Lemma 2.12) for the subgroup of automorphisms of H that are compatible with this perfect pairing, then—by replacing the isomorphism
$\stackrel {\sim }{\rightarrow } \mathrm {GL}_{2g}(\mathbb {Z})$
—cf. the notation of Lemma 2.12) for the subgroup of automorphisms of H that are compatible with this perfect pairing, then—by replacing the isomorphism
![]() $H\stackrel {\sim }{\rightarrow } \mathbb {Z}^{\oplus 2g}$
by a suitable isomorphism if necessary—the isomorphism
$H\stackrel {\sim }{\rightarrow } \mathbb {Z}^{\oplus 2g}$
by a suitable isomorphism if necessary—the isomorphism
![]() $\mathrm {Aut}(H)\stackrel {\sim }{\rightarrow } \mathrm {GL}_{2g}(\mathbb {Z})$
determines an isomorphism
$\mathrm {Aut}(H)\stackrel {\sim }{\rightarrow } \mathrm {GL}_{2g}(\mathbb {Z})$
determines an isomorphism
![]() $\mathrm {Aut}_{\mathrm {PD}}(H)\stackrel {\sim }{\rightarrow }\mathrm {Sp}_{2g}(\mathbb {Z})$
(cf. the notation of Lemma 2.12). On the other hand, recall (cf., e.g., the discussion preceding [Reference Deligne and Mumford7, Th. 5.13]) that the natural homomorphism
$\mathrm {Aut}_{\mathrm {PD}}(H)\stackrel {\sim }{\rightarrow }\mathrm {Sp}_{2g}(\mathbb {Z})$
(cf. the notation of Lemma 2.12). On the other hand, recall (cf., e.g., the discussion preceding [Reference Deligne and Mumford7, Th. 5.13]) that the natural homomorphism
![]() $\mathrm {Aut}(\Pi _{}) \rightarrow \mathrm {Aut}(H)$
determines a surjection
$\mathrm {Aut}(\Pi _{}) \rightarrow \mathrm {Aut}(H)$
determines a surjection
![]() $\mathrm {Aut}(\Pi _{}) \twoheadrightarrow \mathrm {Aut}_{\mathrm {PD}}(H) (\subseteq \mathrm {Aut}(H))$
. Thus, Lemma 2.13 follows immediately from Lemma 2.12(iv). This completes the proof of Lemma 2.13.
$\mathrm {Aut}(\Pi _{}) \twoheadrightarrow \mathrm {Aut}_{\mathrm {PD}}(H) (\subseteq \mathrm {Aut}(H))$
. Thus, Lemma 2.13 follows immediately from Lemma 2.12(iv). This completes the proof of Lemma 2.13.
Lemma 2.14 (Finitely generated subgroups of surface groups).
Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type and
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type and
![]() $J \subseteq \Pi _{\mathcal {G}}$
a finitely generated subgroup of the fundamental group
$J \subseteq \Pi _{\mathcal {G}}$
a finitely generated subgroup of the fundamental group
![]() $\Pi _{\mathcal {G}}$
of
$\Pi _{\mathcal {G}}$
of
![]() $\mathcal {G}$
. Then the following hold:
$\mathcal {G}$
. Then the following hold:
-
(i) Suppose that
 $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
. Then there exist a subgroup
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
. Then there exist a subgroup
 $F\subseteq \Pi _{\mathcal {G}}$
of finite index and a surjection
$F\subseteq \Pi _{\mathcal {G}}$
of finite index and a surjection
 $F \twoheadrightarrow J$
such that
$F \twoheadrightarrow J$
such that
 $J\subseteq F$
, and, moreover, the restriction of the surjection
$J\subseteq F$
, and, moreover, the restriction of the surjection
 $F \twoheadrightarrow J$
to
$F \twoheadrightarrow J$
to
 $J\subseteq F$
is the identity automorphism of J.
$J\subseteq F$
is the identity automorphism of J. -
(ii) Suppose that
 $(\mathrm {Vert}(\mathcal {G})^\sharp , \mathrm {Cusp}(\mathcal {G})^\sharp , \mathrm {Node}(\mathcal {G})^\sharp ) = (1,0,1)$
. Thus, since we are in the situation of Lemma 2.11, we shall apply the notational conventions established in Lemma 2.11. Suppose that the image of J in
$(\mathrm {Vert}(\mathcal {G})^\sharp , \mathrm {Cusp}(\mathcal {G})^\sharp , \mathrm {Node}(\mathcal {G})^\sharp ) = (1,0,1)$
. Thus, since we are in the situation of Lemma 2.11, we shall apply the notational conventions established in Lemma 2.11. Suppose that the image of J in
 $\Pi _{\mathcal {G}}^{\mathrm {ab}}$
is of infinite index in
$\Pi _{\mathcal {G}}^{\mathrm {ab}}$
is of infinite index in
 $\Pi _{\mathcal {G}}^{\mathrm {ab}}$
. (For example, this will be the case if J is generated by
$\Pi _{\mathcal {G}}^{\mathrm {ab}}$
. (For example, this will be the case if J is generated by
 $\mathrm {rank}_{\mathbb {Z}}(\Pi _{\mathcal {G}}^{\mathrm {ab}}) -1$
elements.) Then there exists an automorphism
$\mathrm {rank}_{\mathbb {Z}}(\Pi _{\mathcal {G}}^{\mathrm {ab}}) -1$
elements.) Then there exists an automorphism
 $\sigma \in \mathrm {Aut}(\Pi _{\mathcal {G}})$
of
$\sigma \in \mathrm {Aut}(\Pi _{\mathcal {G}})$
of
 $\Pi _{\mathcal {G}}$
such that
$\Pi _{\mathcal {G}}$
such that
 $\sigma (J) \subseteq \Pi _{\mathcal {G}_\infty }$
.
$\sigma (J) \subseteq \Pi _{\mathcal {G}_\infty }$
. -
(iii) In the situation of (ii), suppose, moreover, that
 $J \subseteq \Pi _{\mathcal {G}_\infty }$
. Then there exists a positive integer
$J \subseteq \Pi _{\mathcal {G}_\infty }$
. Then there exists a positive integer
 $a \in \mathbb {Z}$
such that
$a \in \mathbb {Z}$
such that
 $J\subseteq D_{[-a, a]}$
(cf. Lemma 2.11(v)).
$J\subseteq D_{[-a, a]}$
(cf. Lemma 2.11(v)).
Proof. Assertion (i) follows from [Reference Mochizuki17, Cor. 1.6(ii)] together with the fact that
![]() $\Pi _{\mathcal {G}}$
is a finitely generated free group (cf. Remark 2.5.1). Assertion (ii) follows from Lemma 2.13. Assertion (iii) follows from Lemma 2.11(v) together with our assumption that J is finitely generated. This completes the proof of Lemma 2.14.
$\Pi _{\mathcal {G}}$
is a finitely generated free group (cf. Remark 2.5.1). Assertion (ii) follows from Lemma 2.13. Assertion (iii) follows from Lemma 2.11(v) together with our assumption that J is finitely generated. This completes the proof of Lemma 2.14.
Theorem 2.15 (Profinite conjugates of finitely generated
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible subgroups).
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible subgroups).
Let
![]() $\Pi _{}$
be the topological fundamental group of a compact orientable hyperbolic topological surface with compact boundary (cf. Remark 2.5.1) and H,
$\Pi _{}$
be the topological fundamental group of a compact orientable hyperbolic topological surface with compact boundary (cf. Remark 2.5.1) and H,
![]() $J \subseteq \Pi _{}$
subgroups. Since
$J \subseteq \Pi _{}$
subgroups. Since
![]() $\Pi _{}$
injects into its pro-l completion for any
$\Pi _{}$
injects into its pro-l completion for any
![]() $l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf., e.g., [Reference Ribes and Zalesskii27, Prop. 3.3.15]; [Reference Paris25, Th. 1.7]), let us regard subgroups of
$l \in \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
(cf., e.g., [Reference Ribes and Zalesskii27, Prop. 3.3.15]; [Reference Paris25, Th. 1.7]), let us regard subgroups of
![]() $\Pi _{}$
as subgroups of the profinite completion
$\Pi _{}$
as subgroups of the profinite completion
![]() $\widehat {\Pi }_{}$
of
$\widehat {\Pi }_{}$
of
![]() $\Pi _{}$
. Write
$\Pi _{}$
. Write
![]() $\overline {H}$
,
$\overline {H}$
,
![]() $\overline {J} \subseteq \widehat {\Pi }_{}$
for the closures of H, J in
$\overline {J} \subseteq \widehat {\Pi }_{}$
for the closures of H, J in
![]() $\widehat {\Pi }_{}$
, respectively. Suppose that the following conditions are satisfied:
$\widehat {\Pi }_{}$
, respectively. Suppose that the following conditions are satisfied:
-
(a) The subgroups H and J are finitely generated.
-
(b) If J is of infinite index in
 $\Pi _{}$
, then
$\Pi _{}$
, then
 $\overline J$
is of infinite index in
$\overline J$
is of infinite index in
 $\widehat {\Pi }_{}$
.
$\widehat {\Pi }_{}$
.
(Here, we note that condition (b) is automatically satisfied whenever
![]() $\Pi _{}$
is free—cf. [Reference Mochizuki17, Cor. 1.6(ii)].) Then the following hold:
$\Pi _{}$
is free—cf. [Reference Mochizuki17, Cor. 1.6(ii)].) Then the following hold:
-
(i) It holds that
 $J = \overline {J} \cap \Pi _{}$
.
$J = \overline {J} \cap \Pi _{}$
. -
(ii) Suppose that there exists an element
 $\widehat {\gamma } \in \widehat {\Pi }_{}$
such that
$\widehat {\gamma } \in \widehat {\Pi }_{}$
such that  $$\begin{align*}H \subseteq \widehat{\gamma} \cdot \overline{J} \cdot \widehat{\gamma}^{-1}. \end{align*}$$
$$\begin{align*}H \subseteq \widehat{\gamma} \cdot \overline{J} \cdot \widehat{\gamma}^{-1}. \end{align*}$$
Then there exists an element
 $\delta \in \Pi _{}$
such that
$\delta \in \Pi _{}$
such that  $$\begin{align*}H \subseteq \delta \cdot J \cdot \delta^{-1}. \end{align*}$$
$$\begin{align*}H \subseteq \delta \cdot J \cdot \delta^{-1}. \end{align*}$$
Proof. Let us first observe that, to verify Theorem 2.15, we may assume without loss of generality that
![]() $\Pi _{}$
is the fundamental group
$\Pi _{}$
is the fundamental group
![]() $\Pi _{\mathcal {G}}$
of a semi-graph of temperoids of HSD-type
$\Pi _{\mathcal {G}}$
of a semi-graph of temperoids of HSD-type
![]() $\mathcal {G}$
(cf. Definition 2.3).
$\mathcal {G}$
(cf. Definition 2.3).
Next, we claim that the following assertion holds:
Claim 2.15.A: Theorem 2.15 holds in the case where J is of finite index in
![]() $\Pi _{\mathcal {G}}$
.
$\Pi _{\mathcal {G}}$
.
Indeed, write
![]() $N \subseteq \Pi _{\mathcal {G}}$
for the normal subgroup of
$N \subseteq \Pi _{\mathcal {G}}$
for the normal subgroup of
![]() $\Pi _{\mathcal {G}}$
obtained by forming the intersection of all
$\Pi _{\mathcal {G}}$
obtained by forming the intersection of all
![]() $\Pi _{\mathcal {G}}$
-conjugates of J. Then since J is of finite index in
$\Pi _{\mathcal {G}}$
-conjugates of J. Then since J is of finite index in
![]() $\Pi _{\mathcal {G}}$
, it is immediate that N is of finite index in
$\Pi _{\mathcal {G}}$
, it is immediate that N is of finite index in
![]() $\Pi _{\mathcal {G}}$
. Thus, by considering the images in
$\Pi _{\mathcal {G}}$
. Thus, by considering the images in
![]() $\Pi _{\mathcal {G}} / N$
of the various groups involved, one verifies immediately that Theorem 2.15 holds in the case where J is of finite index in
$\Pi _{\mathcal {G}} / N$
of the various groups involved, one verifies immediately that Theorem 2.15 holds in the case where J is of finite index in
![]() $\Pi _{\mathcal {G}}$
. This completes the proof of Claim 2.15.A. Thus, in the remainder of the proof of Theorem 2.15, we may assume without loss of generality that J is of infinite index in
$\Pi _{\mathcal {G}}$
. This completes the proof of Claim 2.15.A. Thus, in the remainder of the proof of Theorem 2.15, we may assume without loss of generality that J is of infinite index in
![]() $\Pi _{\mathcal {G}}$
, which implies that
$\Pi _{\mathcal {G}}$
, which implies that
![]() $\overline {J}$
is of infinite index in
$\overline {J}$
is of infinite index in
![]() $\widehat {\Pi }_{\mathcal {G}}$
(cf. condition (b)).
$\widehat {\Pi }_{\mathcal {G}}$
(cf. condition (b)).
Next, we claim that the following assertion holds:
Claim 2.15.B: Let
![]() $F\subseteq \Pi _{\mathcal {G}}$
be a subgroup of finite index such that
$F\subseteq \Pi _{\mathcal {G}}$
be a subgroup of finite index such that
![]() $J\subseteq F$
. Suppose that the assertion obtained by replacing
$J\subseteq F$
. Suppose that the assertion obtained by replacing
![]() $\Pi _{\mathcal {G}}$
in assertion (i) by F holds. Then assertion (i) holds, and, in the situation of assertion (ii), there exists a
$\Pi _{\mathcal {G}}$
in assertion (i) by F holds. Then assertion (i) holds, and, in the situation of assertion (ii), there exists a
![]() $\Pi _{\mathcal {G}}$
-conjugate of H that is contained in F. If, moreover, the assertion obtained by replacing
$\Pi _{\mathcal {G}}$
-conjugate of H that is contained in F. If, moreover, the assertion obtained by replacing
![]() $\Pi _{\mathcal {G}}$
in assertion (ii) by F holds, then assertion (ii) holds.
$\Pi _{\mathcal {G}}$
in assertion (ii) by F holds, then assertion (ii) holds.
Indeed, let us first observe that since the natural inclusion
![]() $F \hookrightarrow \Pi _{\mathcal {G}}$
is
$F \hookrightarrow \Pi _{\mathcal {G}}$
is
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible (cf. the discussion entitled “Groups” in §0), the profinite completion
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible (cf. the discussion entitled “Groups” in §0), the profinite completion
![]() $\widehat {F}$
of F may be identified with the closure
$\widehat {F}$
of F may be identified with the closure
![]() $\overline {F}$
of F in
$\overline {F}$
of F in
![]() $\widehat {\Pi }_{\mathcal {G}}$
. In particular, the closure of J in
$\widehat {\Pi }_{\mathcal {G}}$
. In particular, the closure of J in
![]() $\widehat {F}$
is naturally isomorphic to the closure
$\widehat {F}$
is naturally isomorphic to the closure
![]() $\overline {J}$
of J in
$\overline {J}$
of J in
![]() $\widehat {\Pi }_{\mathcal {G}}$
. Thus, it follows from Claim 2.15.A applied to F that the assertion obtained by replacing
$\widehat {\Pi }_{\mathcal {G}}$
. Thus, it follows from Claim 2.15.A applied to F that the assertion obtained by replacing
![]() $\Pi _{\mathcal {G}}$
in assertion (i) by F implies assertion (i). Next, let us observe that in the situation of assertion (ii), since (one verifies immediately that)
$\Pi _{\mathcal {G}}$
in assertion (i) by F implies assertion (i). Next, let us observe that in the situation of assertion (ii), since (one verifies immediately that)
![]() $\Pi _{\mathcal {G}}\cdot \overline {F} = \widehat {\Pi }_{\mathcal {G}}$
, by replacing H by a suitable
$\Pi _{\mathcal {G}}\cdot \overline {F} = \widehat {\Pi }_{\mathcal {G}}$
, by replacing H by a suitable
![]() $\Pi _{\mathcal {G}}$
-conjugate of H, we may assume without loss of generality that
$\Pi _{\mathcal {G}}$
-conjugate of H, we may assume without loss of generality that
![]() $\widehat {\gamma } \in \overline {F}$
. In particular, since
$\widehat {\gamma } \in \overline {F}$
. In particular, since
![]() $H \subseteq \widehat {\gamma } \cdot \overline {J} \cdot \widehat {\gamma }^{-1} \subseteq \widehat {\gamma } \cdot \overline {F} \cdot \widehat {\gamma }^{-1}=\overline {F}$
, it follows that
$H \subseteq \widehat {\gamma } \cdot \overline {J} \cdot \widehat {\gamma }^{-1} \subseteq \widehat {\gamma } \cdot \overline {F} \cdot \widehat {\gamma }^{-1}=\overline {F}$
, it follows that
![]() $H \subseteq \overline {F} \cap \Pi _{\mathcal {G}} = F$
(cf. Claim 2.15.A). Thus, one verifies easily that the assertion obtained by replacing
$H \subseteq \overline {F} \cap \Pi _{\mathcal {G}} = F$
(cf. Claim 2.15.A). Thus, one verifies easily that the assertion obtained by replacing
![]() $\Pi _{\mathcal {G}}$
in assertion (ii) by F implies assertion (ii). This completes the proof of Claim 2.15.B.
$\Pi _{\mathcal {G}}$
in assertion (ii) by F implies assertion (ii). This completes the proof of Claim 2.15.B.
Next, we verify Theorem 2.15 in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
. Suppose that
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
. Suppose that
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
. Then it follows from Lemma 2.14(i) that there exist a subgroup
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
. Then it follows from Lemma 2.14(i) that there exist a subgroup
![]() $F\subseteq \Pi _{\mathcal {G}}$
of finite index and a surjection
$F\subseteq \Pi _{\mathcal {G}}$
of finite index and a surjection
![]() $\pi \colon F \twoheadrightarrow J$
such that
$\pi \colon F \twoheadrightarrow J$
such that
![]() $J\subseteq F$
, and, moreover, the restriction of
$J\subseteq F$
, and, moreover, the restriction of
![]() $\pi $
to
$\pi $
to
![]() $J\subseteq F$
is the identity automorphism of J. Now it follows immediately from Claim 2.15.B that, by replacing
$J\subseteq F$
is the identity automorphism of J. Now it follows immediately from Claim 2.15.B that, by replacing
![]() $\Pi _{\mathcal {G}}$
by F, we may assume without loss of generality that
$\Pi _{\mathcal {G}}$
by F, we may assume without loss of generality that
![]() $\Pi _{\mathcal {G}} = F$
. Next, let us observe that since (it is immediate that)
$\Pi _{\mathcal {G}} = F$
. Next, let us observe that since (it is immediate that)
![]() $J\subseteq \overline {J} \cap \Pi _{\mathcal {G}}$
, to complete the verification of assertion (i) in the case where
$J\subseteq \overline {J} \cap \Pi _{\mathcal {G}}$
, to complete the verification of assertion (i) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
, it suffices to verify that
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
, it suffices to verify that
![]() $\overline {J} \cap \Pi _{\mathcal {G}} \subseteq J$
. Moreover, since
$\overline {J} \cap \Pi _{\mathcal {G}} \subseteq J$
. Moreover, since
![]() $J\subseteq \overline {J} \cap \Pi _{\mathcal {G}} (\subseteq \overline {J})$
, it follows immediately from the equality
$J\subseteq \overline {J} \cap \Pi _{\mathcal {G}} (\subseteq \overline {J})$
, it follows immediately from the equality
![]() $\widehat {\pi }|_{\overline {J}} = \mathrm {id}_{\overline {J}}$
(where we write
$\widehat {\pi }|_{\overline {J}} = \mathrm {id}_{\overline {J}}$
(where we write
![]() $\widehat {\pi } \colon \widehat {\Pi }_{\mathcal {G}} \twoheadrightarrow \overline {J}$
for the surjection induced by
$\widehat {\pi } \colon \widehat {\Pi }_{\mathcal {G}} \twoheadrightarrow \overline {J}$
for the surjection induced by
![]() $\pi $
) that, to verify the inclusion
$\pi $
) that, to verify the inclusion
![]() $\overline {J} \cap \Pi _{\mathcal {G}} \subseteq J$
, it suffices to verify that
$\overline {J} \cap \Pi _{\mathcal {G}} \subseteq J$
, it suffices to verify that
![]() $ \widehat {\pi }(\overline {J} \cap \Pi _{\mathcal {G}}) \subseteq \widehat {\pi }(J)$
. On the other hand, one verifies easily that
$ \widehat {\pi }(\overline {J} \cap \Pi _{\mathcal {G}}) \subseteq \widehat {\pi }(J)$
. On the other hand, one verifies easily that
as desired. This completes the proof of assertion (i) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
.
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
.
Next, to verify assertion (ii) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
, let us observe that, by replacing
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
, let us observe that, by replacing
![]() $\widehat {\gamma }$
by
$\widehat {\gamma }$
by
![]() $\widehat {\gamma }\cdot \widehat {\pi }(\widehat {\gamma }^{-1})$
, we may assume without loss of generality that
$\widehat {\gamma }\cdot \widehat {\pi }(\widehat {\gamma }^{-1})$
, we may assume without loss of generality that
![]() $\widehat {\gamma } \in \mathrm {Ker}(\widehat {\pi })$
. Now we claim that the following assertion holds:
$\widehat {\gamma } \in \mathrm {Ker}(\widehat {\pi })$
. Now we claim that the following assertion holds:
Claim 2.15.C: It holds that
![]() $H\subseteq \widehat {\gamma } \cdot J \cdot \widehat {\gamma }^{-1}$
.
$H\subseteq \widehat {\gamma } \cdot J \cdot \widehat {\gamma }^{-1}$
.
Indeed, since (one verifies easily that)
![]() $\widehat {\gamma }^{-1} \cdot H \cdot \widehat {\gamma }$
,
$\widehat {\gamma }^{-1} \cdot H \cdot \widehat {\gamma }$
,
![]() $J \subseteq \overline {J}$
, it follows immediately from the equality
$J \subseteq \overline {J}$
, it follows immediately from the equality
![]() $\widehat {\pi }|_{\overline {J}} = \mathrm {id}_{\overline {J}}$
that, to verify Claim 2.15.C, it suffices to verify that
$\widehat {\pi }|_{\overline {J}} = \mathrm {id}_{\overline {J}}$
that, to verify Claim 2.15.C, it suffices to verify that
![]() $ \widehat {\pi }(\widehat {\gamma }^{-1} \cdot H \cdot \widehat {\gamma }) \subseteq \widehat {\pi }(J)$
. On the other hand, since
$ \widehat {\pi }(\widehat {\gamma }^{-1} \cdot H \cdot \widehat {\gamma }) \subseteq \widehat {\pi }(J)$
. On the other hand, since
![]() $\widehat {\gamma } \in \mathrm {Ker}(\widehat {\pi })$
, it holds that
$\widehat {\gamma } \in \mathrm {Ker}(\widehat {\pi })$
, it holds that
as desired. This completes the proof of Claim 2.15.C. In particular, it follows immediately from [Reference Mochizuki22, Th. 2.6] (i.e., in essence, the argument given in the proof of [Reference André1, Lem. 3.2.1]), that there exists an element
![]() $\delta \in \Pi _{\mathcal {G}}$
such that
$\delta \in \Pi _{\mathcal {G}}$
such that
![]() $\delta ^{-1} \cdot H \cdot \delta = \widehat {\gamma }^{-1} \cdot H \cdot \widehat {\gamma } \subseteq J$
. This completes the proof of assertion (ii) in the case where
$\delta ^{-1} \cdot H \cdot \delta = \widehat {\gamma }^{-1} \cdot H \cdot \widehat {\gamma } \subseteq J$
. This completes the proof of assertion (ii) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
, hence also of Theorem 2.15 in the case where
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
, hence also of Theorem 2.15 in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
.
$\mathrm {Cusp}(\mathcal {G}) \neq \emptyset $
.
Next, we verify Theorem 2.15 in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) = \emptyset $
. Suppose that
$\mathrm {Cusp}(\mathcal {G}) = \emptyset $
. Suppose that
![]() $\mathrm {Cusp}(\mathcal {G}) = \emptyset $
. First, we observe that since
$\mathrm {Cusp}(\mathcal {G}) = \emptyset $
. First, we observe that since
![]() $\overline J$
is of infinite index in
$\overline J$
is of infinite index in
![]() $\widehat {\Pi }_{\mathcal {G}}$
, it follows immediately that
$\widehat {\Pi }_{\mathcal {G}}$
, it follows immediately that
![]() $[\Pi _{\mathcal {G}}:J\cdot N] \rightarrow + \infty $
as N ranges over the normal subgroups of
$[\Pi _{\mathcal {G}}:J\cdot N] \rightarrow + \infty $
as N ranges over the normal subgroups of
![]() $\Pi _{\mathcal {G}}$
of finite index, hence (cf. Claim 2.15.B; the fact that J is finitely generated) that, by replacing
$\Pi _{\mathcal {G}}$
of finite index, hence (cf. Claim 2.15.B; the fact that J is finitely generated) that, by replacing
![]() $\Pi _{\mathcal {G}}$
by a suitable subgroup of finite index in
$\Pi _{\mathcal {G}}$
by a suitable subgroup of finite index in
![]() $\Pi _{\mathcal {G}}$
that contains J, we may assume without loss of generality that the image of J in
$\Pi _{\mathcal {G}}$
that contains J, we may assume without loss of generality that the image of J in
![]() $\Pi _{\mathcal {G}}^{\mathrm {ab}}$
is of infinite index in
$\Pi _{\mathcal {G}}^{\mathrm {ab}}$
is of infinite index in
![]() $\Pi _{\mathcal {G}}^{\mathrm {ab}}$
(cf. Remark 2.5.1). Moreover, by considering suitable specialization outer isomorphisms (cf. Proposition 2.10), we may assume without loss of generality that the equality
$\Pi _{\mathcal {G}}^{\mathrm {ab}}$
(cf. Remark 2.5.1). Moreover, by considering suitable specialization outer isomorphisms (cf. Proposition 2.10), we may assume without loss of generality that the equality
![]() $(\mathrm {Vert}(\mathcal {G})^\sharp , \mathrm {Cusp}(\mathcal {G})^\sharp , \mathrm {Node}(\mathcal {G})^\sharp ) = (1,0,1)$
holds. Thus, since we are in the situation of Lemma 2.11, we shall apply the notational conventions established in Lemma 2.11. Moreover, it follows from Lemma 2.14(ii) that, by considering a suitable automorphism of
$(\mathrm {Vert}(\mathcal {G})^\sharp , \mathrm {Cusp}(\mathcal {G})^\sharp , \mathrm {Node}(\mathcal {G})^\sharp ) = (1,0,1)$
holds. Thus, since we are in the situation of Lemma 2.11, we shall apply the notational conventions established in Lemma 2.11. Moreover, it follows from Lemma 2.14(ii) that, by considering a suitable automorphism of
![]() $\Pi _{\mathcal {G}}$
, we may assume without loss of generality that
$\Pi _{\mathcal {G}}$
, we may assume without loss of generality that
![]() $J\subseteq \Pi _{\mathcal {G}_\infty }$
. Thus, it follows from Lemma 2.14(iii) that there exists a positive integer
$J\subseteq \Pi _{\mathcal {G}_\infty }$
. Thus, it follows from Lemma 2.14(iii) that there exists a positive integer
![]() $a \in \mathbb {Z}$
such that
$a \in \mathbb {Z}$
such that
![]() $J\subseteq D_{[-a, a]}\subseteq \Pi _{\mathcal {G}_\infty }$
.
$J\subseteq D_{[-a, a]}\subseteq \Pi _{\mathcal {G}_\infty }$
.
Next, let us observe that since
![]() $\Pi _{\mathcal {G}} / \Pi _{\mathcal {G}_\infty } \stackrel {\sim }{\rightarrow } \Pi _{\mathbb {G}} (\cong \mathbb {Z})$
injects into its profinite completion, it follows that
$\Pi _{\mathcal {G}} / \Pi _{\mathcal {G}_\infty } \stackrel {\sim }{\rightarrow } \Pi _{\mathbb {G}} (\cong \mathbb {Z})$
injects into its profinite completion, it follows that
![]() $\overline {J} \cap \Pi _{\mathcal {G}}\subseteq \Pi _{\mathcal {G}_\infty }$
. In particular, by applying Lemma 2.14(iii), we conclude that, for any given fixed element
$\overline {J} \cap \Pi _{\mathcal {G}}\subseteq \Pi _{\mathcal {G}_\infty }$
. In particular, by applying Lemma 2.14(iii), we conclude that, for any given fixed element
![]() $\alpha \in \overline {J} \cap \Pi _{\mathcal {G}}$
, we may assume, by possibly enlarging a, that
$\alpha \in \overline {J} \cap \Pi _{\mathcal {G}}$
, we may assume, by possibly enlarging a, that
![]() $\alpha \in D_{[-a, a]}$
. Next, let us observe—that is, by considering a suitable finite étale subcovering of
$\alpha \in D_{[-a, a]}$
. Next, let us observe—that is, by considering a suitable finite étale subcovering of
![]() $\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—that the natural inclusion
$\mathcal {G}_\infty \rightarrow \mathcal {G}$
and applying a suitable specialization outer isomorphism (cf. Proposition 2.10)—that the natural inclusion
![]() $D_{[-a, a]} \hookrightarrow \Pi _{\mathcal {G}}$
is
$D_{[-a, a]} \hookrightarrow \Pi _{\mathcal {G}}$
is
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible (cf. Proposition 2.5(iv)). In particular, by replacing
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible (cf. Proposition 2.5(iv)). In particular, by replacing
![]() $\mathcal {G}$
by
$\mathcal {G}$
by
![]() $\mathcal {G}_{[-a,a]}$
(cf. Lemma 2.11(ii)), we conclude that assertion (i) in the case where
$\mathcal {G}_{[-a,a]}$
(cf. Lemma 2.11(ii)), we conclude that assertion (i) in the case where
![]() $\mathrm {Cusp}(\mathcal {G})=\emptyset $
follows from assertion (i) in the case where
$\mathrm {Cusp}(\mathcal {G})=\emptyset $
follows from assertion (i) in the case where
![]() $\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
(already verified above). This completes the proof of assertion (i) in the case where
$\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
(already verified above). This completes the proof of assertion (i) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) = \emptyset $
.
$\mathrm {Cusp}(\mathcal {G}) = \emptyset $
.
Finally, to verify assertion (ii) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) = \emptyset $
, let us observe that if
$\mathrm {Cusp}(\mathcal {G}) = \emptyset $
, let us observe that if
![]() $H=\{1\}$
, then assertion (ii) is immediate. Thus, we may assume without loss of generality that
$H=\{1\}$
, then assertion (ii) is immediate. Thus, we may assume without loss of generality that
![]() $H\neq \{1\}$
. Next, let us observe that since
$H\neq \{1\}$
. Next, let us observe that since
![]() $J\subseteq D_{[-a, a]} \subseteq \Pi _{\mathcal {G}_\infty }$
, and
$J\subseteq D_{[-a, a]} \subseteq \Pi _{\mathcal {G}_\infty }$
, and
![]() $\Pi _{\mathcal {G}} / \Pi _{\mathcal {G}_\infty } \stackrel {\sim }{\rightarrow } \Pi _{\mathbb {G}}$
$\Pi _{\mathcal {G}} / \Pi _{\mathcal {G}_\infty } \stackrel {\sim }{\rightarrow } \Pi _{\mathbb {G}}$
![]() $(\cong \mathbb {Z})$
injects into its profinite completion, one verifies immediately that
$(\cong \mathbb {Z})$
injects into its profinite completion, one verifies immediately that
![]() $H\subseteq \Pi _{\mathcal {G}_{\infty }}$
. Thus, since
$H\subseteq \Pi _{\mathcal {G}_{\infty }}$
. Thus, since
![]() $H \subseteq \Pi _{\mathcal {G}_ \infty }$
is finitely generated, it follows from Lemma 2.14(iii) that, by possibly enlarging a, we may assume without loss of generality that
$H \subseteq \Pi _{\mathcal {G}_ \infty }$
is finitely generated, it follows from Lemma 2.14(iii) that, by possibly enlarging a, we may assume without loss of generality that
![]() $H\subseteq D_{[-a, a]}$
. Since, moreover,
$H\subseteq D_{[-a, a]}$
. Since, moreover,
![]() $\{1\}\neq H\subseteq \overline {D}_{[-a, a]} \cap \widehat {\gamma } \cdot \overline {J} \cdot \widehat {\gamma }^{-1} \subseteq \overline {D}_{[-a, a]} \cap \widehat {\gamma } \cdot \overline {D}_{[-a, a]} \cdot \widehat {\gamma }^{-1}$
, it follows from Lemma 2.11(vi) that the image of
$\{1\}\neq H\subseteq \overline {D}_{[-a, a]} \cap \widehat {\gamma } \cdot \overline {J} \cdot \widehat {\gamma }^{-1} \subseteq \overline {D}_{[-a, a]} \cap \widehat {\gamma } \cdot \overline {D}_{[-a, a]} \cdot \widehat {\gamma }^{-1}$
, it follows from Lemma 2.11(vi) that the image of
![]() $\widehat {\gamma } \in \widehat {\Pi }_{\mathcal {G}}$
in the profinite completion
$\widehat {\gamma } \in \widehat {\Pi }_{\mathcal {G}}$
in the profinite completion
![]() $\widehat {\Pi }_{\mathbb {G}}$
of
$\widehat {\Pi }_{\mathbb {G}}$
of
![]() $\Pi _{\mathbb {G}}$
is contained in
$\Pi _{\mathbb {G}}$
is contained in
![]() $\Pi _{\mathbb {G}}\subseteq \widehat {\Pi }_{\mathbb {G}}$
, which thus implies that there exists an element
$\Pi _{\mathbb {G}}\subseteq \widehat {\Pi }_{\mathbb {G}}$
, which thus implies that there exists an element
![]() $\gamma ' \in \Pi _{\mathcal {G}}$
such that
$\gamma ' \in \Pi _{\mathcal {G}}$
such that
![]() $\gamma ' \widehat {\gamma } \in \overline {\Pi }_{\mathcal {G}_\infty }$
. In particular, by replacing H by
$\gamma ' \widehat {\gamma } \in \overline {\Pi }_{\mathcal {G}_\infty }$
. In particular, by replacing H by
![]() $\gamma ' \cdot H\cdot (\gamma ')^{-1}$
and possibly enlarging a, we may assume without loss of generality that
$\gamma ' \cdot H\cdot (\gamma ')^{-1}$
and possibly enlarging a, we may assume without loss of generality that
![]() $\widehat {\gamma } \in \overline {\Pi }_{\mathcal {G}_\infty }$
. Thus, again by applying the fact that
$\widehat {\gamma } \in \overline {\Pi }_{\mathcal {G}_\infty }$
. Thus, again by applying the fact that
![]() $\{1\}\neq \overline {D}_{[-a, a]} \cap \widehat {\gamma } \cdot \overline {D}_{[-a, a]} \cdot \widehat {\gamma }^{-1}$
, we conclude from Lemma 2.11(vii) that
$\{1\}\neq \overline {D}_{[-a, a]} \cap \widehat {\gamma } \cdot \overline {D}_{[-a, a]} \cdot \widehat {\gamma }^{-1}$
, we conclude from Lemma 2.11(vii) that
![]() $\widehat {\gamma } \in \overline {D}_{[-a, a]}$
. In particular, since, as discussed above (cf. the discussion immediately preceding the proof of assertion (i) in the case where
$\widehat {\gamma } \in \overline {D}_{[-a, a]}$
. In particular, since, as discussed above (cf. the discussion immediately preceding the proof of assertion (i) in the case where
![]() $\mathrm {Cusp}(\mathcal {G})=\emptyset $
), the natural inclusion
$\mathrm {Cusp}(\mathcal {G})=\emptyset $
), the natural inclusion
![]() $D_{[-a, a]} \hookrightarrow \Pi _{\mathcal {G}}$
is
$D_{[-a, a]} \hookrightarrow \Pi _{\mathcal {G}}$
is
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible, by replacing
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
-compatible, by replacing
![]() $\mathcal {G}$
by
$\mathcal {G}$
by
![]() $\mathcal {G}_{[-a, a]}$
, we conclude that assertion (ii) in the case where
$\mathcal {G}_{[-a, a]}$
, we conclude that assertion (ii) in the case where
![]() $\mathrm {Cusp}(\mathcal {G})=\emptyset $
follows from assertion (ii) in the case where
$\mathrm {Cusp}(\mathcal {G})=\emptyset $
follows from assertion (ii) in the case where
![]() $\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
(already verified above). This completes the proof of assertion (ii) in the case where
$\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
(already verified above). This completes the proof of assertion (ii) in the case where
![]() $\mathrm {Cusp}(\mathcal {G}) = \emptyset $
, hence also of Theorem 2.15.
$\mathrm {Cusp}(\mathcal {G}) = \emptyset $
, hence also of Theorem 2.15.
Remark 2.15.1. In passing, we observe that the analogue of Theorem 2.15 for arbitrary
![]() $\Sigma \neq \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
is false. Indeed, if, in the statement of Theorem 2.15, one replaces “
$\Sigma \neq \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
is false. Indeed, if, in the statement of Theorem 2.15, one replaces “
![]() $\Pi _{}$
” by the group
$\Pi _{}$
” by the group
![]() $\mathbb {Z}$
, then it is easy to construct counterexamples to assertions (i) and (ii). One may then obtain counterexamples in the case of the original “
$\mathbb {Z}$
, then it is easy to construct counterexamples to assertions (i) and (ii). One may then obtain counterexamples in the case of the original “
![]() $\Pi _{}$
” by considering the case where the original “
$\Pi _{}$
” by considering the case where the original “
![]() $\Pi _{}$
” is the fundamental group
$\Pi _{}$
” is the fundamental group
![]() $\Pi _{\mathcal {G}}$
of a semi-graph of temperoids of HSD-type
$\Pi _{\mathcal {G}}$
of a semi-graph of temperoids of HSD-type
![]() $\mathcal {G}$
such that
$\mathcal {G}$
such that
![]() $\mathrm {Edge}(\mathcal {G}) \neq \emptyset $
and considering suitable edge-like subgroups (i.e., isomorphic to
$\mathrm {Edge}(\mathcal {G}) \neq \emptyset $
and considering suitable edge-like subgroups (i.e., isomorphic to
![]() $\mathbb {Z}$
!) of
$\mathbb {Z}$
!) of
![]() $\Pi _{\mathcal {G}}$
.
$\Pi _{\mathcal {G}}$
.
Lemma 2.16 (VCN-subgroups of infinite index).
Let
![]() $\mathcal {G}$
be a semi-graph of anabelioids of pro-
$\mathcal {G}$
be a semi-graph of anabelioids of pro-
![]() $\Sigma $
PSC-type (resp. of temperoids of HSD-type). Write
$\Sigma $
PSC-type (resp. of temperoids of HSD-type). Write
![]() $J\stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^\Sigma $
(resp.
$J\stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}^\Sigma $
(resp.
![]() $J\stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}$
) for the (pro-
$J\stackrel {\mathrm {def}}{=} \Pi _{\mathcal {G}}$
) for the (pro-
![]() $\Sigma $
[resp. discrete]) fundamental group of
$\Sigma $
[resp. discrete]) fundamental group of
![]() $\mathcal {G}$
. Let
$\mathcal {G}$
. Let
![]() $H\subseteq J$
be a VCN-subgroup of J. Consider the following two (mutually exclusive) conditions:
$H\subseteq J$
be a VCN-subgroup of J. Consider the following two (mutually exclusive) conditions:
-
(1)
 $H = J$
.
$H = J$
. -
(2) H is of infinite index in J.
Then we have equivalences
with the following two conditions:
-
(1′) H is verticial, and
 $\mathrm {Node}(\mathcal {G}) = \emptyset $
.
$\mathrm {Node}(\mathcal {G}) = \emptyset $
. -
(2′) Either H is edge-like, or
 $\mathrm {Node}(\mathcal {G}) \neq \emptyset $
.
$\mathrm {Node}(\mathcal {G}) \neq \emptyset $
.
Proof. The implication (1
![]() $'$
)
$'$
)
![]() $\Rightarrow $
(1) follows immediately from the various definitions involved. Thus, one verifies immediately (by considering suitable contrapositive versions of the various implications involved) that, to complete the verification of Lemma 2.16, it suffices to verify the implication (2
$\Rightarrow $
(1) follows immediately from the various definitions involved. Thus, one verifies immediately (by considering suitable contrapositive versions of the various implications involved) that, to complete the verification of Lemma 2.16, it suffices to verify the implication (2
![]() $'$
)
$'$
)
![]() $\Rightarrow $
(2). To this end, let us observe that if H is edge-like, then since H is abelian, and every closed subgroup of J of finite index is center-free (cf., e.g., Remark 2.5.1; [Reference Mochizuki18, Rem. 1.1.3]), we conclude that H is of infinite index in J. Thus, we may assume without loss of generality that H is verticial and
$\Rightarrow $
(2). To this end, let us observe that if H is edge-like, then since H is abelian, and every closed subgroup of J of finite index is center-free (cf., e.g., Remark 2.5.1; [Reference Mochizuki18, Rem. 1.1.3]), we conclude that H is of infinite index in J. Thus, we may assume without loss of generality that H is verticial and
![]() $\mathrm {Node}(\mathcal {G}) \neq \emptyset $
. Now since
$\mathrm {Node}(\mathcal {G}) \neq \emptyset $
. Now since
![]() $\mathrm {Node}(\mathcal {G}) \neq \emptyset $
, it follows from a similar argument to the argument in the discussion entitled “Curves” in [Reference Mochizuki21, §0] that, by replacing
$\mathrm {Node}(\mathcal {G}) \neq \emptyset $
, it follows from a similar argument to the argument in the discussion entitled “Curves” in [Reference Mochizuki21, §0] that, by replacing
![]() $\mathcal {G}$
by a suitable connected finite étale covering of
$\mathcal {G}$
by a suitable connected finite étale covering of
![]() $\mathcal {G}$
, we may assume without loss of generality that the underlying semi-graph of
$\mathcal {G}$
, we may assume without loss of generality that the underlying semi-graph of
![]() $\mathcal {G}$
is loop-ample (cf. the discussion entitled “Semi-graphs” in [Reference Mochizuki21, §0]). In particular, since (one verifies easily that) the abelianization of the (pro-
$\mathcal {G}$
is loop-ample (cf. the discussion entitled “Semi-graphs” in [Reference Mochizuki21, §0]). In particular, since (one verifies easily that) the abelianization of the (pro-
![]() $\Sigma $
completion of the) topological fundamental group of a noncontractible semi-graph is infinite, the image of H in the abelianization of J is of infinite index, which thus implies that H is of infinite index in J, as desired. This completes the proof of Lemma 2.16.
$\Sigma $
completion of the) topological fundamental group of a noncontractible semi-graph is infinite, the image of H in the abelianization of J is of infinite index, which thus implies that H is of infinite index in J, as desired. This completes the proof of Lemma 2.16.
Corollary 2.17 (Profinite conjugates of VCN-subgroups).
Let
![]() $\mathcal {G}$
and
$\mathcal {G}$
and
![]() $\mathcal {H}$
be semi-graphs of temperoids of HSD-type. Write
$\mathcal {H}$
be semi-graphs of temperoids of HSD-type. Write
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {H}}$
for the respective fundamental groups of
$\Pi _{\mathcal {H}}$
for the respective fundamental groups of
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
. Thus, we obtain a semi-graph of anabelioids of pro-
$\mathcal {H}$
. Thus, we obtain a semi-graph of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
![]() $\widehat {\mathcal {H}}$
(cf. Proposition 2.5(iii) in the case where
$\widehat {\mathcal {H}}$
(cf. Proposition 2.5(iii) in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
). Let
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
). Let
![]() $z_{\mathcal {G}} \in \mathrm {VCN}(\mathcal {G})$
,
$z_{\mathcal {G}} \in \mathrm {VCN}(\mathcal {G})$
,
![]() $z_{\mathcal {H}} \in \mathrm {VCN}(\mathcal {H})$
,
$z_{\mathcal {H}} \in \mathrm {VCN}(\mathcal {H})$
,
![]() $\Pi _{z_{\mathcal {G}}} \subseteq \Pi _{\mathcal {G}}$
a VCN-subgroup of
$\Pi _{z_{\mathcal {G}}} \subseteq \Pi _{\mathcal {G}}$
a VCN-subgroup of
![]() $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
![]() $z_{\mathcal {G}} \in \mathrm {VCN}(\mathcal {G})$
,
$z_{\mathcal {G}} \in \mathrm {VCN}(\mathcal {G})$
,
![]() $\Pi _{z_{\mathcal {H}}} \subseteq \Pi _{\mathcal {H}}$
a VCN-subgroup of
$\Pi _{z_{\mathcal {H}}} \subseteq \Pi _{\mathcal {H}}$
a VCN-subgroup of
![]() $\Pi _{\mathcal {H}}$
associated with
$\Pi _{\mathcal {H}}$
associated with
![]() $z_{\mathcal {H}} \in \mathrm {VCN}(\mathcal {H})$
,
$z_{\mathcal {H}} \in \mathrm {VCN}(\mathcal {H})$
,
an isomorphism of groups, and
![]() $\widehat {\gamma } \in \Pi _{\widehat {\mathcal {H}}}$
an element of the (profinite) fundamental group
$\widehat {\gamma } \in \Pi _{\widehat {\mathcal {H}}}$
an element of the (profinite) fundamental group
![]() $\Pi _{\widehat {\mathcal {H}}}$
of
$\Pi _{\widehat {\mathcal {H}}}$
of
![]() $\widehat {\mathcal {H}}$
. Let us fix an injection
$\widehat {\mathcal {H}}$
. Let us fix an injection
![]() $\Pi _{\mathcal {H}} \hookrightarrow \Pi _{\widehat {\mathcal {H}}}$
such that the induced outer injection is the outer injection of Proposition 2.5(iii) and regard subgroups of
$\Pi _{\mathcal {H}} \hookrightarrow \Pi _{\widehat {\mathcal {H}}}$
such that the induced outer injection is the outer injection of Proposition 2.5(iii) and regard subgroups of
![]() $\Pi _{\mathcal {H}}$
as subgroups of
$\Pi _{\mathcal {H}}$
as subgroups of
![]() $\Pi _{\widehat {\mathcal {H}}}$
by means of this fixed injection. Write
$\Pi _{\widehat {\mathcal {H}}}$
by means of this fixed injection. Write
![]() $\overline {\Pi }_{z_{\mathcal {H}}} \subseteq \Pi _{\widehat {\mathcal {H}}}$
for the closure of
$\overline {\Pi }_{z_{\mathcal {H}}} \subseteq \Pi _{\widehat {\mathcal {H}}}$
for the closure of
![]() $\Pi _{z_{\mathcal {H}}}$
in
$\Pi _{z_{\mathcal {H}}}$
in
![]() $\Pi _{\widehat {\mathcal {H}}}$
. (Thus,
$\Pi _{\widehat {\mathcal {H}}}$
. (Thus,
![]() $\overline {\Pi }_{z_{\mathcal {H}}} \subseteq \Pi _{\widehat {\mathcal {H}}}$
is a VCN-subgroup of
$\overline {\Pi }_{z_{\mathcal {H}}} \subseteq \Pi _{\widehat {\mathcal {H}}}$
is a VCN-subgroup of
![]() $\Pi _{\widehat {\mathcal {H}}}$
associated with
$\Pi _{\widehat {\mathcal {H}}}$
associated with
![]() $z_{\mathcal {H}} \in \mathrm {VCN}(\widehat {\mathcal {H}}) = \mathrm {VCN}(\mathcal {H})$
—cf. Proposition 2.5(v).) Then the following hold:
$z_{\mathcal {H}} \in \mathrm {VCN}(\widehat {\mathcal {H}}) = \mathrm {VCN}(\mathcal {H})$
—cf. Proposition 2.5(v).) Then the following hold:
-
(i) It holds that
 $\Pi _{z_{\mathcal {H}}} = \overline {\Pi }_{z_{\mathcal {H}}} \cap \Pi _{\mathcal {H}}$
.
$\Pi _{z_{\mathcal {H}}} = \overline {\Pi }_{z_{\mathcal {H}}} \cap \Pi _{\mathcal {H}}$
. -
(ii) Suppose that
Then there exists an element $$\begin{align*}\widetilde\alpha(\Pi_{z_{\mathcal{G}}}) \subseteq \widehat{\gamma} \cdot \overline{\Pi}_{z_{\mathcal{H}}} \cdot \widehat{\gamma}^{-1}. \end{align*}$$
$$\begin{align*}\widetilde\alpha(\Pi_{z_{\mathcal{G}}}) \subseteq \widehat{\gamma} \cdot \overline{\Pi}_{z_{\mathcal{H}}} \cdot \widehat{\gamma}^{-1}. \end{align*}$$
 $\delta \in \Pi _{\mathcal {H}}$
such that
$\delta \in \Pi _{\mathcal {H}}$
such that  $$\begin{align*}\widetilde\alpha(\Pi_{z_{\mathcal{G}}}) \subseteq \delta \cdot \Pi_{z_{\mathcal{H}}} \cdot \delta^{-1}. \end{align*}$$
$$\begin{align*}\widetilde\alpha(\Pi_{z_{\mathcal{G}}}) \subseteq \delta \cdot \Pi_{z_{\mathcal{H}}} \cdot \delta^{-1}. \end{align*}$$
Proof. First, let us observe that it follows immediately from Definition 2.3(ii), together with the well-known structure of topological fundamental groups of topological surfaces, that
![]() $\Pi _{z_{\mathcal {G}}}$
and
$\Pi _{z_{\mathcal {G}}}$
and
![]() $\Pi _{z_{\mathcal {H}}}$
are finitely generated. Thus, it follows immediately from Theorem 2.15 that, to complete the verification of Corollary 2.17, it suffices to verify that the following assertion holds:
$\Pi _{z_{\mathcal {H}}}$
are finitely generated. Thus, it follows immediately from Theorem 2.15 that, to complete the verification of Corollary 2.17, it suffices to verify that the following assertion holds:
If
![]() $\Pi _{z_{\mathcal {H}}} \neq \Pi _{\mathcal {H}}$
, then
$\Pi _{z_{\mathcal {H}}} \neq \Pi _{\mathcal {H}}$
, then
![]() $\overline {\Pi }_{z_{\mathcal {H}}}$
is of infinite index in
$\overline {\Pi }_{z_{\mathcal {H}}}$
is of infinite index in
![]() $\Pi _{\widehat {\mathcal {H}}}$
.
$\Pi _{\widehat {\mathcal {H}}}$
.
To this end, let us observe that since
![]() $\Pi _{z_{\mathcal {H}}} \neq \Pi _{\mathcal {H}}$
, it follows from Lemma 2.16 (in the case where “
$\Pi _{z_{\mathcal {H}}} \neq \Pi _{\mathcal {H}}$
, it follows from Lemma 2.16 (in the case where “
![]() $\mathcal {G}$
” is a semi-graph of temperoids of HSD-type) that either
$\mathcal {G}$
” is a semi-graph of temperoids of HSD-type) that either
![]() $z_{\mathcal {H}}$
is an edge, or
$z_{\mathcal {H}}$
is an edge, or
![]() $\mathrm {Node}(\mathcal {H}) \neq \emptyset $
. On the other hand, in either of these two cases, it follows immediately from Lemma 2.16 (in the case where “
$\mathrm {Node}(\mathcal {H}) \neq \emptyset $
. On the other hand, in either of these two cases, it follows immediately from Lemma 2.16 (in the case where “
![]() $\mathcal {G}$
” is a semi-graph of anabelioids of PSC-type), together with Proposition 2.5(v), that
$\mathcal {G}$
” is a semi-graph of anabelioids of PSC-type), together with Proposition 2.5(v), that
![]() $\overline {\Pi }_{z_{\mathcal {H}}}$
is of infinite index in
$\overline {\Pi }_{z_{\mathcal {H}}}$
is of infinite index in
![]() $\Pi _{\widehat {\mathcal {H}}}$
. This completes the proof of Corollary 2.17.
$\Pi _{\widehat {\mathcal {H}}}$
. This completes the proof of Corollary 2.17.
Corollary 2.18 (Properties of VCN-subgroups).
Let
![]() $\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Write
$\mathcal {G}$
be a semi-graph of temperoids of HSD-type. Write
![]() $\Pi _{\mathcal {G}}$
for the fundamental group of
$\Pi _{\mathcal {G}}$
for the fundamental group of
![]() $\mathcal {G}$
. Also, write
$\mathcal {G}$
. Also, write
![]() $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
for the universal covering of
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
for the universal covering of
![]() $\mathcal {G}$
corresponding to
$\mathcal {G}$
corresponding to
![]() $\Pi _{\mathcal {G}}$
. Then the following hold:
$\Pi _{\mathcal {G}}$
. Then the following hold:
-
(i) For
 $i = 1$
,
$i = 1$
,
 $2$
, let
$2$
, let
 $\widetilde v_i\in \mathrm {Vert}(\widetilde {\mathcal {G}})$
(cf. Definition 2.1(v)). Write
$\widetilde v_i\in \mathrm {Vert}(\widetilde {\mathcal {G}})$
(cf. Definition 2.1(v)). Write
 $\Pi _{\widetilde v_i} \subseteq \Pi _{\mathcal {G}}$
for the verticial subgroup of
$\Pi _{\widetilde v_i} \subseteq \Pi _{\mathcal {G}}$
for the verticial subgroup of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde v_i$
(cf. Definition 2.6(ii)). Consider the following three (mutually exclusive) conditions (cf. Definition 2.1(v)):
$\widetilde v_i$
(cf. Definition 2.6(ii)). Consider the following three (mutually exclusive) conditions (cf. Definition 2.1(v)):-
(1)
 $\delta (\widetilde v_1, \widetilde v_2)=0$
.
$\delta (\widetilde v_1, \widetilde v_2)=0$
. -
(2)
 $\delta (\widetilde v_1, \widetilde v_2)=1$
.
$\delta (\widetilde v_1, \widetilde v_2)=1$
. -
(3)
 $\delta (\widetilde v_1, \widetilde v_2)\geq 2$
.
$\delta (\widetilde v_1, \widetilde v_2)\geq 2$
.
Then we have equivalences
with the following three conditions: $$\begin{align*}(1) \Longleftrightarrow (1'); \, \, \, (2) \Longleftrightarrow (2'); \, \, \, (3) \Longleftrightarrow (3') \end{align*}$$
$$\begin{align*}(1) \Longleftrightarrow (1'); \, \, \, (2) \Longleftrightarrow (2'); \, \, \, (3) \Longleftrightarrow (3') \end{align*}$$
-
(1′)
 $\Pi _{\widetilde v_1} = \Pi _{\widetilde v_2}$
.
$\Pi _{\widetilde v_1} = \Pi _{\widetilde v_2}$
. -
(2′)
 $\Pi _{\widetilde v_1} \cap \Pi _{\widetilde v_2} \neq \{1\}$
, but
$\Pi _{\widetilde v_1} \cap \Pi _{\widetilde v_2} \neq \{1\}$
, but
 $\Pi _{\widetilde v_1} \neq \Pi _{\widetilde v_2}$
.
$\Pi _{\widetilde v_1} \neq \Pi _{\widetilde v_2}$
. -
(3′)
 $\Pi _{\widetilde v_1} \cap \Pi _{\widetilde v_2} = \{1\}$
.
$\Pi _{\widetilde v_1} \cap \Pi _{\widetilde v_2} = \{1\}$
.
-
-
(ii) In the situation of (i), suppose that condition (2), hence also condition (2
 $'$
), holds. Then it holds that
$'$
), holds. Then it holds that
 $(\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2))^\sharp = 1$
(cf. Definition 2.1(v)), and, moreover, if we write
$(\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2))^\sharp = 1$
(cf. Definition 2.1(v)), and, moreover, if we write
 $\widetilde e \in \mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2)$
for the unique element of
$\widetilde e \in \mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2)$
for the unique element of
 $\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2)$
, then
$\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2)$
, then
 $\Pi _{\widetilde v_1}\cap \Pi _{\widetilde v_2}=\Pi _{\widetilde e}$
;
$\Pi _{\widetilde v_1}\cap \Pi _{\widetilde v_2}=\Pi _{\widetilde e}$
;
 $\Pi _{\widetilde e}\neq \Pi _{\widetilde v_1}$
;
$\Pi _{\widetilde e}\neq \Pi _{\widetilde v_1}$
;
 $\Pi _{\widetilde e}\neq \Pi _{\widetilde v_2}$
.
$\Pi _{\widetilde e}\neq \Pi _{\widetilde v_2}$
. -
(iii) For
 $i = 1$
,
$i = 1$
,
 $2$
, let
$2$
, let
 $\widetilde e_i\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
(cf. Definition 2.1(v)). Write
$\widetilde e_i\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
(cf. Definition 2.1(v)). Write
 $\Pi _{\widetilde e_i} \subseteq \Pi _{\mathcal {G}}$
for the edge-like subgroup of
$\Pi _{\widetilde e_i} \subseteq \Pi _{\mathcal {G}}$
for the edge-like subgroup of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde e_i$
(cf. Definition 2.6(ii)). Then
$\widetilde e_i$
(cf. Definition 2.6(ii)). Then
 $\Pi _{\widetilde e_1}\cap \Pi _{\widetilde e_2} \neq \{1\}$
if and only if
$\Pi _{\widetilde e_1}\cap \Pi _{\widetilde e_2} \neq \{1\}$
if and only if
 $\widetilde e_1 = \widetilde e_2$
. In particular,
$\widetilde e_1 = \widetilde e_2$
. In particular,
 $ \Pi _{\widetilde e_1} \cap \Pi _{\widetilde e_2} \neq \{1\}$
if and only if
$ \Pi _{\widetilde e_1} \cap \Pi _{\widetilde e_2} \neq \{1\}$
if and only if
 $\Pi _{\widetilde e_1} = \Pi _{\widetilde e_2}$
(cf. Remark 2.6.1).
$\Pi _{\widetilde e_1} = \Pi _{\widetilde e_2}$
(cf. Remark 2.6.1). -
(iv) Let
 $\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
,
$\widetilde v \in \mathrm {Vert}(\widetilde {\mathcal {G}})$
,
 $\widetilde e\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
. Write
$\widetilde e\in \mathrm {Edge}(\widetilde {\mathcal {G}})$
. Write
 $\Pi _{\widetilde v}$
,
$\Pi _{\widetilde v}$
,
 $\Pi _{\widetilde e} \subseteq \Pi _{\mathcal {G}}$
for the VCN-subgroups of
$\Pi _{\widetilde e} \subseteq \Pi _{\mathcal {G}}$
for the VCN-subgroups of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde v$
,
$\widetilde v$
,
 $\widetilde e$
, respectively. Then
$\widetilde e$
, respectively. Then
 $\Pi _{\widetilde e}\cap \Pi _{\widetilde v} \neq \{1\}$
if and only if
$\Pi _{\widetilde e}\cap \Pi _{\widetilde v} \neq \{1\}$
if and only if
 $\widetilde e\in \mathcal {E}(\widetilde v)$
. In particular,
$\widetilde e\in \mathcal {E}(\widetilde v)$
. In particular,
 $\Pi _{\widetilde e}\cap \Pi _{\widetilde v} \neq \{1\}$
if and only if
$\Pi _{\widetilde e}\cap \Pi _{\widetilde v} \neq \{1\}$
if and only if
 $\Pi _{\widetilde e}\subseteq \Pi _{\widetilde v}$
(cf. Remark 2.6.1).
$\Pi _{\widetilde e}\subseteq \Pi _{\widetilde v}$
(cf. Remark 2.6.1). -
(v) Every VCN-subgroup of
 $\Pi _{\mathcal {G}}$
is commensurably terminal in
$\Pi _{\mathcal {G}}$
is commensurably terminal in
 $\Pi _{\mathcal {G}}$
.
$\Pi _{\mathcal {G}}$
.
Proof. Write
![]() $\widetilde {\mathcal {G}}^\wedge \rightarrow \widehat {\mathcal {G}}$
for the universal profinite étale covering of the semi-graph of anabelioids of pro-
$\widetilde {\mathcal {G}}^\wedge \rightarrow \widehat {\mathcal {G}}$
for the universal profinite étale covering of the semi-graph of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
![]() $\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
$\widehat {\mathcal {G}}$
(cf. Proposition 2.5(iii) in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) determined by
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
) determined by
![]() $\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
and
$\widetilde {\mathcal {G}} \rightarrow \mathcal {G}$
and
![]() $\Pi _{\widehat {\mathcal {G}}}$
for the (profinite) fundamental group of
$\Pi _{\widehat {\mathcal {G}}}$
for the (profinite) fundamental group of
![]() $\widehat {\mathcal {G}}$
determined by the universal covering
$\widehat {\mathcal {G}}$
determined by the universal covering
![]() $\widetilde {\mathcal {G}}^\wedge \rightarrow \widehat {\mathcal {G}}$
. Thus, one verifies easily that one obtains a natural morphism of (pro-)semi-graphs of temperoids (cf. Remark 2.1.1)
$\widetilde {\mathcal {G}}^\wedge \rightarrow \widehat {\mathcal {G}}$
. Thus, one verifies easily that one obtains a natural morphism of (pro-)semi-graphs of temperoids (cf. Remark 2.1.1)
![]() $\widetilde {\mathcal {G}} \rightarrow \widetilde {\mathcal {G}}^\wedge $
that induces injections
$\widetilde {\mathcal {G}} \rightarrow \widetilde {\mathcal {G}}^\wedge $
that induces injections
![]() $\Pi _{\mathcal {G}} \hookrightarrow \Pi _{\widehat {\mathcal {G}}}$
(cf. Proposition 2.5(iii)) and
$\Pi _{\mathcal {G}} \hookrightarrow \Pi _{\widehat {\mathcal {G}}}$
(cf. Proposition 2.5(iii)) and
![]() $\mathrm {VCN}(\widetilde {\mathcal {G}}) \hookrightarrow \mathrm {VCN}(\widetilde {\mathcal {G}}^\wedge )$
(cf. [Reference Hoshi and Mochizuki8, Def. 1.1(iii)]) such that
$\mathrm {VCN}(\widetilde {\mathcal {G}}) \hookrightarrow \mathrm {VCN}(\widetilde {\mathcal {G}}^\wedge )$
(cf. [Reference Hoshi and Mochizuki8, Def. 1.1(iii)]) such that
-
• the injection
 $\mathrm {VCN}(\widetilde {\mathcal {G}}) \hookrightarrow \mathrm {VCN}(\widetilde {\mathcal {G}}^\wedge )$
is compatible with the respective “
$\mathrm {VCN}(\widetilde {\mathcal {G}}) \hookrightarrow \mathrm {VCN}(\widetilde {\mathcal {G}}^\wedge )$
is compatible with the respective “
 $\delta $
’s” (cf. Definition 2.1(v); [Reference Hoshi and Mochizuki8, Def. 1.1(viii)]), and, moreover,
$\delta $
’s” (cf. Definition 2.1(v); [Reference Hoshi and Mochizuki8, Def. 1.1(viii)]), and, moreover, -
• for each
 $\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, the closure
$\widetilde z \in \mathrm {VCN}(\widetilde {\mathcal {G}})$
, the closure
 $\overline {\Pi }_{\widetilde z} \subseteq \Pi _{\widehat {\mathcal {G}}}$
of the image of the VCN-subgroup
$\overline {\Pi }_{\widetilde z} \subseteq \Pi _{\widehat {\mathcal {G}}}$
of the image of the VCN-subgroup
 $\Pi _{\widetilde z} \subseteq \Pi _{\mathcal {G}}$
of
$\Pi _{\widetilde z} \subseteq \Pi _{\mathcal {G}}$
of
 $\Pi _{\mathcal {G}}$
associated with
$\Pi _{\mathcal {G}}$
associated with
 $\widetilde z$
via the injection
$\widetilde z$
via the injection
 $\Pi _{\mathcal {G}} \hookrightarrow \Pi _{\widehat {\mathcal {G}}}$
coincides with the VCN-subgroup of
$\Pi _{\mathcal {G}} \hookrightarrow \Pi _{\widehat {\mathcal {G}}}$
coincides with the VCN-subgroup of
 $\Pi _{\widehat {\mathcal {G}}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.1(i)]) associated with the image of
$\Pi _{\widehat {\mathcal {G}}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.1(i)]) associated with the image of
 $\widetilde z$
via the injection
$\widetilde z$
via the injection
 $\mathrm {VCN}(\widetilde {\mathcal {G}}) \hookrightarrow \mathrm {VCN}(\widetilde {\mathcal {G}}^\wedge )$
(cf. also Proposition 2.5(v)).
$\mathrm {VCN}(\widetilde {\mathcal {G}}) \hookrightarrow \mathrm {VCN}(\widetilde {\mathcal {G}}^\wedge )$
(cf. also Proposition 2.5(v)).
First, we verify assertion (i). The equivalence (1)
![]() $\Leftrightarrow $
(1
$\Leftrightarrow $
(1
![]() $'$
) follows immediately from the equivalence (1)
$'$
) follows immediately from the equivalence (1)
![]() $\Leftrightarrow $
(1
$\Leftrightarrow $
(1
![]() $'$
) of [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)], together with the discussion at the beginning of the present proof. Next, let us observe that, by considering the edge-like subgroup associated with an element of
$'$
) of [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)], together with the discussion at the beginning of the present proof. Next, let us observe that, by considering the edge-like subgroup associated with an element of
![]() $\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2)$
, we conclude that condition (2) implies the condition that
$\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2)$
, we conclude that condition (2) implies the condition that
![]() $\Pi _{\widetilde v_1}\cap \Pi _{\widetilde v_2} \neq \{1\}$
. Thus, the implication (2)
$\Pi _{\widetilde v_1}\cap \Pi _{\widetilde v_2} \neq \{1\}$
. Thus, the implication (2)
![]() $\Rightarrow $
(2
$\Rightarrow $
(2
![]() $'$
) follows immediately from the equivalence (1)
$'$
) follows immediately from the equivalence (1)
![]() $\Leftrightarrow $
(1
$\Leftrightarrow $
(1
![]() $'$
). The implication (2
$'$
). The implication (2
![]() $'$
)
$'$
)
![]() $\Rightarrow $
(2) follows immediately from Corollary 2.17(i) and the implication (2
$\Rightarrow $
(2) follows immediately from Corollary 2.17(i) and the implication (2
![]() $'$
)
$'$
)
![]() $\Rightarrow $
(2) of [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)], together with the discussion at the beginning of the present proof. The equivalence (3)
$\Rightarrow $
(2) of [Reference Hoshi and Mochizuki8, Lem. 1.9(ii)], together with the discussion at the beginning of the present proof. The equivalence (3)
![]() $\Leftrightarrow $
(3
$\Leftrightarrow $
(3
![]() $'$
) follows immediately from the equivalences (1)
$'$
) follows immediately from the equivalences (1)
![]() $\Leftrightarrow $
(1
$\Leftrightarrow $
(1
![]() $'$
) and (2)
$'$
) and (2)
![]() $\Leftrightarrow $
(2
$\Leftrightarrow $
(2
![]() $'$
). This completes the proof of assertion (i).
$'$
). This completes the proof of assertion (i).
Assertion (iii) (resp. (iv)) follows immediately from [Reference Hoshi and Mochizuki8, Lem. 1.5] (resp. [Reference Hoshi and Mochizuki8, Lem. 1.7]), together with the discussion at the beginning of the present proof. Assertion (v) follows formally from assertions (i) and (iii) (cf. also the proof of [Reference Mochizuki18, Prop. 1.2(ii)]).
Finally, we verify assertion (ii). Suppose that condition (2) (in the statement of assertion (i)), hence also condition (2
![]() $'$
) (in the statement of assertion (i)), holds. Then the assertion that
$'$
) (in the statement of assertion (i)), holds. Then the assertion that
![]() $(\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2))^\sharp = 1$
follows immediately from the fact that the underlying semi-graph of
$(\mathcal {E}(\widetilde v_1)\cap \mathcal {E}(\widetilde v_2))^\sharp = 1$
follows immediately from the fact that the underlying semi-graph of
![]() $\widetilde {\mathcal {G}}$
is a tree. The remainder of assertion (ii) follows immediately—in light of assertion (iii)—from Corollary 2.17(i) and [Reference Hoshi and Mochizuki8, Lem. 1.9(i)] (cf. also Remark 2.6.1), together with the discussion at the beginning of the present proof. This completes the proof of assertion (ii), hence also of Corollary 2.18.
$\widetilde {\mathcal {G}}$
is a tree. The remainder of assertion (ii) follows immediately—in light of assertion (iii)—from Corollary 2.17(i) and [Reference Hoshi and Mochizuki8, Lem. 1.9(i)] (cf. also Remark 2.6.1), together with the discussion at the beginning of the present proof. This completes the proof of assertion (ii), hence also of Corollary 2.18.
Corollary 2.19 (Graphicity of outer isomorphisms).
Let
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
be semi-graphs of temperoids of HSD-type. Write
$\mathcal {H}$
be semi-graphs of temperoids of HSD-type. Write
![]() $\widehat {\mathcal {G}}$
,
$\widehat {\mathcal {G}}$
,
![]() $\widehat {\mathcal {H}}$
for the semi-graphs of anabelioids of pro-
$\widehat {\mathcal {H}}$
for the semi-graphs of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type determined by
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type determined by
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
(cf. Proposition 2.5(iii) in the case where
$\mathcal {H}$
(cf. Proposition 2.5(iii) in the case where
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
), respectively;
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
), respectively;
![]() $\Pi _{\mathcal {G}}$
,
$\Pi _{\mathcal {G}}$
,
![]() $\Pi _{\mathcal {H}}$
for the respective fundamental groups of
$\Pi _{\mathcal {H}}$
for the respective fundamental groups of
![]() $\mathcal {G}$
,
$\mathcal {G}$
,
![]() $\mathcal {H}$
;
$\mathcal {H}$
;
![]() $\Pi _{\widehat {\mathcal {G}}}$
,
$\Pi _{\widehat {\mathcal {G}}}$
,
![]() $\Pi _{\widehat {\mathcal {H}}}$
for the respective (profinite) fundamental groups of
$\Pi _{\widehat {\mathcal {H}}}$
for the respective (profinite) fundamental groups of
![]() $\widehat {\mathcal {G}}$
,
$\widehat {\mathcal {G}}$
,
![]() $\widehat {\mathcal {H}}$
. Let
$\widehat {\mathcal {H}}$
. Let
be an outer isomorphism. Write
![]() $\widehat {\alpha }\colon \Pi _{\widehat {\mathcal {G}}}\stackrel {\sim }{\rightarrow }\Pi _{\widehat {\mathcal {H}}}$
for the outer isomorphism determined by the outer isomorphism
$\widehat {\alpha }\colon \Pi _{\widehat {\mathcal {G}}}\stackrel {\sim }{\rightarrow }\Pi _{\widehat {\mathcal {H}}}$
for the outer isomorphism determined by the outer isomorphism
![]() $\alpha $
and the natural outer isomorphisms
$\alpha $
and the natural outer isomorphisms
![]() $\widehat {\Pi }_{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {G}}}$
,
$\widehat {\Pi }_{\mathcal {G}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {G}}}$
,
![]() $\widehat {\Pi }_{\mathcal {H}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {H}}}$
of Proposition 2.5(iii). Then the following hold:
$\widehat {\Pi }_{\mathcal {H}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {H}}}$
of Proposition 2.5(iii). Then the following hold:
-
(i) The outer isomorphism
 $\alpha $
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal; graphic) (cf. Definition 2.7(i) and (ii)) if and only if
$\alpha $
is group-theoretically verticial (resp. group-theoretically cuspidal; group-theoretically nodal; graphic) (cf. Definition 2.7(i) and (ii)) if and only if
 $\widehat {\alpha }$
is group-theoretically verticial (cf. [Reference Mochizuki18, Def. 1.4(iv)]) (resp. group-theoretically cuspidal [cf. [Reference Mochizuki18, Def. 1.4(iv)]]; group-theoretically nodal [cf. [Reference Hoshi and Mochizuki8, Def. 1.12]]; graphic [cf. [Reference Mochizuki18, Def. 1.4(i)]]).
$\widehat {\alpha }$
is group-theoretically verticial (cf. [Reference Mochizuki18, Def. 1.4(iv)]) (resp. group-theoretically cuspidal [cf. [Reference Mochizuki18, Def. 1.4(iv)]]; group-theoretically nodal [cf. [Reference Hoshi and Mochizuki8, Def. 1.12]]; graphic [cf. [Reference Mochizuki18, Def. 1.4(i)]]). -
(ii) The outer isomorphism
 $\alpha $
is graphic if and only if
$\alpha $
is graphic if and only if
 $\alpha $
is group-theoretically verticial, group-theoretically cuspidal, and group-theoretically nodal.
$\alpha $
is group-theoretically verticial, group-theoretically cuspidal, and group-theoretically nodal.
Proof. Assertion (ii) follows immediately, in light of Corollary 2.18, from a similar argument to the argument applied in the proof of [Reference Mochizuki18, Prop. 1.5(ii)]. Thus, it remains to verify assertion (i). The necessity portion of assertion (i) follows immediately from Proposition 2.5(v). Next, let us observe that inclusions of verticial subgroups of the fundamental group of a semi-graph of temperoids of HSD-type are necessarily equalities (cf. Corollary 2.18(i) and (ii)); a similar statement holds concerning inclusions of edge-like subgroups (cf. Corollary 2.18(iii)). Thus, the sufficiency portion of assertion (i) follows immediately—in light of assertion (ii) and [Reference Mochizuki18, Prop. 1.5(ii)]—from Corollary 2.17(ii). This completes the proof of Corollary 2.19.
Corollary 2.20 (Discrete combinatorial cuspidalization).
Let
![]() $\Sigma \subseteq \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
be a subset which is either equal to
$\Sigma \subseteq \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
be a subset which is either equal to
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
or of cardinality one,
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
or of cardinality one,
![]() $(g,r)$
a pair of nonnegative numbers such that
$(g,r)$
a pair of nonnegative numbers such that
![]() $2g - 2 + r> 0$
, n a positive integer, and
$2g - 2 + r> 0$
, n a positive integer, and
![]() $\mathcal {X}$
a topological surface of type
$\mathcal {X}$
a topological surface of type
![]() $(g,r)$
(i.e., the complement of r distinct points in an orientable compact topological surface of genus g). For each positive integer i, write
$(g,r)$
(i.e., the complement of r distinct points in an orientable compact topological surface of genus g). For each positive integer i, write
![]() $\mathcal {X}_{i}$
for the ith configuration space of
$\mathcal {X}_{i}$
for the ith configuration space of
![]() $\mathcal {X}$
(i.e., the topological space obtained by forming the complement of the various diagonals in the direct product of i copies of
$\mathcal {X}$
(i.e., the topological space obtained by forming the complement of the various diagonals in the direct product of i copies of
![]() $\mathcal {X}$
);
$\mathcal {X}$
);
![]() $\Pi _{i}$
for the topological fundamental group of
$\Pi _{i}$
for the topological fundamental group of
![]() $\mathcal {X}_i$
;
$\mathcal {X}_i$
;
![]() $\Pi _{i}^{\Sigma }$
for the pro-
$\Pi _{i}^{\Sigma }$
for the pro-
![]() $\Sigma $
completion of
$\Sigma $
completion of
![]() $\Pi _{i}$
;
$\Pi _{i}$
;
![]() $\widehat {\Pi }_{i}$
for the profinite completion of
$\widehat {\Pi }_{i}$
for the profinite completion of
![]() $\Pi _{i}$
;
$\Pi _{i}$
;
for the subgroups of the group
![]() $\mathrm {Out}(\Pi _{i})$
of outomorphisms of
$\mathrm {Out}(\Pi _{i})$
of outomorphisms of
![]() $\Pi _{i}$
defined in the statement of [Reference Mochizuki20, Cor. 5.1] (cf. also the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]);
$\Pi _{i}$
defined in the statement of [Reference Mochizuki20, Cor. 5.1] (cf. also the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]);
for the subgroups of the group
![]() $\mathrm {Out}(\Pi _{i}^{\Sigma })$
of outomorphisms of
$\mathrm {Out}(\Pi _{i}^{\Sigma })$
of outomorphisms of
![]() $\Pi _{i}^{\Sigma }$
consisting of FC-admissible, F-admissible (cf. [Reference Mochizuki20, Def. 1.1(ii)]; the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]) outomorphisms, respectively. Then the following hold:
$\Pi _{i}^{\Sigma }$
consisting of FC-admissible, F-admissible (cf. [Reference Mochizuki20, Def. 1.1(ii)]; the discussion entitled “Topological groups” in [Reference Hoshi and Mochizuki9, §0]) outomorphisms, respectively. Then the following hold:
-
(i) The group
 $\Pi _{n}$
is normally terminal in
$\Pi _{n}$
is normally terminal in
 $\Pi _{n}^{\Sigma }$
(cf. Proposition 2.5(iii)). In particular, the natural homomorphism is injective. In the following, we shall regard subgroups of
$\Pi _{n}^{\Sigma }$
(cf. Proposition 2.5(iii)). In particular, the natural homomorphism is injective. In the following, we shall regard subgroups of $$ \begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n}) \longrightarrow \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\Sigma})\end{align*} $$
$$ \begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n}) \longrightarrow \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\Sigma})\end{align*} $$
 $\mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
as subgroups of
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
as subgroups of
 $\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\Sigma })$
.
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\Sigma })$
.
-
(ii) It holds that
 $\mathrm {Out}^{\mathrm {F}}(\Pi _{n}) \cap \mathrm {Out}^{\mathrm {FC}}(\widehat {\Pi }_{n}) = \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
.
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n}) \cap \mathrm {Out}^{\mathrm {FC}}(\widehat {\Pi }_{n}) = \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
. -
(iii) Consider the commutative diagram
—where the horizontal arrows are the injections of (i), and the vertical arrows are the homomorphisms induced by the projection
 $\mathcal {X}_{n+1} \rightarrow \mathcal {X}_n$
obtained by forgetting the
$\mathcal {X}_{n+1} \rightarrow \mathcal {X}_n$
obtained by forgetting the
 $(n+1)$
st factor. Suppose that the right-hand vertical arrow of the diagram is injective (cf. Remark 2.20.1 below). Then the commutative diagram of the above display is Cartesian. In particular, the left-hand vertical arrow of the diagram is injective.
$(n+1)$
st factor. Suppose that the right-hand vertical arrow of the diagram is injective (cf. Remark 2.20.1 below). Then the commutative diagram of the above display is Cartesian. In particular, the left-hand vertical arrow of the diagram is injective.
-
(iv) The image of the left-hand vertical arrow of the commutative diagram of (iii) (where we do not impose the assumption that the right-hand vertical arrow be injective) is contained in
 $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})\subseteq \mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
.
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})\subseteq \mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
. -
(v) Consider the commutative diagram
—where the horizontal arrows are the injections induced by the injections of (i), and the vertical arrows are the homomorphisms induced by the projection
 $\mathcal {X}_{n+1} \rightarrow \mathcal {X}_n$
obtained by forgetting the
$\mathcal {X}_{n+1} \rightarrow \mathcal {X}_n$
obtained by forgetting the
 $(n+1)$
st factor. This diagram is Cartesian, its right-hand vertical arrow is injective, and its left-hand vertical arrow is bijective.
$(n+1)$
st factor. This diagram is Cartesian, its right-hand vertical arrow is injective, and its left-hand vertical arrow is bijective.
-
(vi) Write
Suppose that $$\begin{align*}n_{\mathrm{FC}} \, \, \stackrel{\mathrm{def}}{=} \, \, \left\{ \begin{array}{ccl} 2, & & \text{if} \, \, (g,r) = (0,3) , \\ 3, & &\text{if} \, \, (g,r)\neq (0,3) \text{ and } r \neq 0 , \\ 4, & &\text{if} \, \, r = 0. \end{array} \right. \end{align*}$$
$$\begin{align*}n_{\mathrm{FC}} \, \, \stackrel{\mathrm{def}}{=} \, \, \left\{ \begin{array}{ccl} 2, & & \text{if} \, \, (g,r) = (0,3) , \\ 3, & &\text{if} \, \, (g,r)\neq (0,3) \text{ and } r \neq 0 , \\ 4, & &\text{if} \, \, r = 0. \end{array} \right. \end{align*}$$
 $n \geq n_{\mathrm {FC}}$
. Then it holds that the left-hand vertical arrow
$n \geq n_{\mathrm {FC}}$
. Then it holds that the left-hand vertical arrow $$ \begin{align*}\mathrm{Out}^{\mathrm{FC}}(\Pi_{n}) = \mathrm{Out}^{\mathrm{F}}(\Pi_{n}) ;\end{align*} $$
of the commutative diagram of (iii) is bijective.
$$ \begin{align*}\mathrm{Out}^{\mathrm{FC}}(\Pi_{n}) = \mathrm{Out}^{\mathrm{F}}(\Pi_{n}) ;\end{align*} $$
of the commutative diagram of (iii) is bijective. $$ \begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n+1}) \longrightarrow \mathrm{Out}^{\mathrm{F}}(\Pi_{n})\end{align*} $$
$$ \begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n+1}) \longrightarrow \mathrm{Out}^{\mathrm{F}}(\Pi_{n})\end{align*} $$
Proof. Let us first observe that, to verify assertion (i), it suffices to verify that
![]() $\Pi _{n}$
is normally terminal in
$\Pi _{n}$
is normally terminal in
![]() $\Pi _{n}^{\Sigma }$
. Moreover, once one proves the desired normal terminality in the case where
$\Pi _{n}^{\Sigma }$
. Moreover, once one proves the desired normal terminality in the case where
![]() $n = 1$
, the desired normal terminality in the case where
$n = 1$
, the desired normal terminality in the case where
![]() $n \geq 2$
follows immediately by induction (cf. the proof of [Reference Mochizuki20, Cor. 5.1(i)]). Thus, we conclude that, to verify assertion (i), it suffices to verify the normal terminality of
$n \geq 2$
follows immediately by induction (cf. the proof of [Reference Mochizuki20, Cor. 5.1(i)]). Thus, we conclude that, to verify assertion (i), it suffices to verify the normal terminality of
![]() $\Pi _{1}$
in
$\Pi _{1}$
in
![]() $\Pi _{1}^{\Sigma }$
.
$\Pi _{1}^{\Sigma }$
.
Next, we claim that the following assertion holds:
Claim 2.20.A: Let F be a free nonabelian group. Then F is normally terminal in the pro-
![]() $\Sigma $
completion of F.
$\Sigma $
completion of F.
Indeed, since F is conjugacy l-separable (cf. [Reference Paris25, Th. 3.2]) for every
![]() $l\in \Sigma $
, Claim 2.20.A follows from a similar argument to the argument applied in the proof of [Reference André1, Lem. 3.2.1]. This completes the proof of Claim 2.20.A.
$l\in \Sigma $
, Claim 2.20.A follows from a similar argument to the argument applied in the proof of [Reference André1, Lem. 3.2.1]. This completes the proof of Claim 2.20.A.
Next, let us observe that one verifies easily that there exist a semi-graph of temperoids of HSD-type
![]() $\mathcal {G}$
and an isomorphism of
$\mathcal {G}$
and an isomorphism of
![]() $\Pi _{1}$
with the fundamental group
$\Pi _{1}$
with the fundamental group
![]() $\Pi _{\mathcal {G}}$
of
$\Pi _{\mathcal {G}}$
of
![]() $\mathcal {G}$
. In the following, we shall identify
$\mathcal {G}$
. In the following, we shall identify
![]() $\Pi _{\mathcal {G}}$
with
$\Pi _{\mathcal {G}}$
with
![]() $\Pi _{1}$
by means of such an isomorphism. If
$\Pi _{1}$
by means of such an isomorphism. If
![]() $\mathcal {G}$
has a cusp, then it follows from Remark 2.5.1 that
$\mathcal {G}$
has a cusp, then it follows from Remark 2.5.1 that
![]() $\Pi _{1}$
is a free nonabelian group. Thus, the desired normal terminality follows from Claim 2.20.A. In the remainder of the proof of assertion (i), suppose that
$\Pi _{1}$
is a free nonabelian group. Thus, the desired normal terminality follows from Claim 2.20.A. In the remainder of the proof of assertion (i), suppose that
![]() $\mathcal {G}$
has no cusp. In particular, we may assume without loss of generality, by applying a suitable specialization outer isomorphism (cf. Proposition 2.10), that
$\mathcal {G}$
has no cusp. In particular, we may assume without loss of generality, by applying a suitable specialization outer isomorphism (cf. Proposition 2.10), that
![]() $\mathcal {G}$
has a node. Let
$\mathcal {G}$
has a node. Let
![]() $\widehat {\gamma } \in N_{\Pi _{1}^{\Sigma }}(\Pi _{1})$
be an element of the normalizer of
$\widehat {\gamma } \in N_{\Pi _{1}^{\Sigma }}(\Pi _{1})$
be an element of the normalizer of
![]() $\Pi _{1}$
in
$\Pi _{1}$
in
![]() $\Pi _{1}^{\Sigma }$
and
$\Pi _{1}^{\Sigma }$
and
![]() $\Pi _{v} \subseteq \Pi _{\mathcal {G}}$
a verticial subgroup of
$\Pi _{v} \subseteq \Pi _{\mathcal {G}}$
a verticial subgroup of
![]() $\Pi _{\mathcal {G}}$
. Then, by applying Corollary 2.17, (ii) (i.e., in the case where we take the “
$\Pi _{\mathcal {G}}$
. Then, by applying Corollary 2.17, (ii) (i.e., in the case where we take the “
![]() $(\mathcal {G}, \mathcal {H}, \Pi _{z_{\mathcal {H}}}, \Pi _{z_{\mathcal {G}}}, \widehat {\gamma })$
” of Corollary 2.17 to be
$(\mathcal {G}, \mathcal {H}, \Pi _{z_{\mathcal {H}}}, \Pi _{z_{\mathcal {G}}}, \widehat {\gamma })$
” of Corollary 2.17 to be
![]() $(\mathcal {G}, \mathcal {G}, \Pi _{v}, \Pi _{v}, \widehat {\gamma })$
and the “
$(\mathcal {G}, \mathcal {G}, \Pi _{v}, \Pi _{v}, \widehat {\gamma })$
and the “
![]() $\widetilde {\alpha }$
” of Corollary 2.17 to be the automorphism of
$\widetilde {\alpha }$
” of Corollary 2.17 to be the automorphism of
![]() $\Pi _{\mathcal {G}}$
obtained by conjugation by
$\Pi _{\mathcal {G}}$
obtained by conjugation by
![]() $\widehat {\gamma }$
), we conclude immediately (cf. also Corollary 2.18(i) and (ii)) that we may assume without loss of generality, by multiplying
$\widehat {\gamma }$
), we conclude immediately (cf. also Corollary 2.18(i) and (ii)) that we may assume without loss of generality, by multiplying
![]() $\widehat {\gamma }$
by a suitable element of
$\widehat {\gamma }$
by a suitable element of
![]() $\Pi _{\mathcal {G}}$
, that the element
$\Pi _{\mathcal {G}}$
, that the element
![]() $\widehat {\gamma } \in \Pi _{1}^{\Sigma }$
normalizes
$\widehat {\gamma } \in \Pi _{1}^{\Sigma }$
normalizes
![]() $\Pi _{v}$
, hence also the closure
$\Pi _{v}$
, hence also the closure
![]() $\overline {\Pi }_{v}$
of
$\overline {\Pi }_{v}$
of
![]() $\Pi _{v}$
in
$\Pi _{v}$
in
![]() $\Pi _{1}^{\Sigma }$
. In particular, it follows from Proposition 2.5(v) and [Reference Mochizuki18, Prop. 1.2(ii)] that
$\Pi _{1}^{\Sigma }$
. In particular, it follows from Proposition 2.5(v) and [Reference Mochizuki18, Prop. 1.2(ii)] that
![]() $\widehat {\gamma } \in \overline {\Pi }_{v}$
. On the other hand, since
$\widehat {\gamma } \in \overline {\Pi }_{v}$
. On the other hand, since
![]() $\mathcal {G}$
has a node, it follows from Proposition 2.5(iv) and Remark 2.6.1 that
$\mathcal {G}$
has a node, it follows from Proposition 2.5(iv) and Remark 2.6.1 that
![]() $\Pi _{v}$
is a free nonabelian group, and
$\Pi _{v}$
is a free nonabelian group, and
![]() $\overline {\Pi }_{v}$
may be identified with the pro-
$\overline {\Pi }_{v}$
may be identified with the pro-
![]() $\Sigma $
completion of
$\Sigma $
completion of
![]() $\Pi _{v}$
. Thus, it follows from Claim 2.20.A that
$\Pi _{v}$
. Thus, it follows from Claim 2.20.A that
![]() $\widehat {\gamma } \in \Pi _{v} \subseteq \Pi _{\mathcal {G}}$
, as desired. This completes the proof of assertion (i).
$\widehat {\gamma } \in \Pi _{v} \subseteq \Pi _{\mathcal {G}}$
, as desired. This completes the proof of assertion (i).
Assertion (ii) follows immediately from Corollary 2.19(i). Next, we verify assertion (iii). Let us first observe that since (we have assumed that) the right-hand vertical arrow of the diagram of assertion (iii) is injective, it follows immediately from assertion (i) that all arrows of the diagram of assertion (iii) are injective. Let
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
be such that the image of
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
be such that the image of
![]() $\alpha $
in
$\alpha $
in
![]() $\mathrm {Out}^{\mathrm {F}}(\widehat {\Pi }_{n})$
lies in the image of the right-hand vertical arrow of the diagram of assertion (iii). Then it follows from [Reference Hoshi and Mochizuki9, Th. A(ii)] that the image of
$\mathrm {Out}^{\mathrm {F}}(\widehat {\Pi }_{n})$
lies in the image of the right-hand vertical arrow of the diagram of assertion (iii). Then it follows from [Reference Hoshi and Mochizuki9, Th. A(ii)] that the image of
![]() $\alpha $
in
$\alpha $
in
![]() $\mathrm {Out}^{\mathrm {F}}(\widehat {\Pi }_{n})$
is FC-admissible. Thus, it follows from assertion (ii) that
$\mathrm {Out}^{\mathrm {F}}(\widehat {\Pi }_{n})$
is FC-admissible. Thus, it follows from assertion (ii) that
![]() $\alpha \in \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
. In particular, it follows from [Reference Hoshi and Mochizuki8, Cor. 6.6] that there exists a uniquely determined element of
$\alpha \in \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
. In particular, it follows from [Reference Hoshi and Mochizuki8, Cor. 6.6] that there exists a uniquely determined element of
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n+1})$
whose image in
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n+1})$
whose image in
![]() $\mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
coincides with
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
coincides with
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
. Thus, since all arrows of the diagram of assertion (iii) are injective (as verified above), we conclude that the diagram of assertion (iii) is Cartesian. This completes the proof of assertion (iii). Assertion (iv) follows immediately from [Reference Hoshi and Mochizuki9, Th. A(ii)], together with assertion (ii). Assertion (v) follows immediately from a similar argument to the argument applied in the proof of assertion (iii), together with the injectivity portion of [Reference Hoshi and Mochizuki8, Th. B]. Assertion (vi) follows immediately from [Reference Hoshi and Mochizuki10, Th. A(ii)] together with assertions (i), (ii), and (v). This completes the proof of Corollary 2.20.
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n})$
. Thus, since all arrows of the diagram of assertion (iii) are injective (as verified above), we conclude that the diagram of assertion (iii) is Cartesian. This completes the proof of assertion (iii). Assertion (iv) follows immediately from [Reference Hoshi and Mochizuki9, Th. A(ii)], together with assertion (ii). Assertion (v) follows immediately from a similar argument to the argument applied in the proof of assertion (iii), together with the injectivity portion of [Reference Hoshi and Mochizuki8, Th. B]. Assertion (vi) follows immediately from [Reference Hoshi and Mochizuki10, Th. A(ii)] together with assertions (i), (ii), and (v). This completes the proof of Corollary 2.20.
Remark 2.20.1. It follows from [Reference Hoshi and Mochizuki10, Th. A(i)] that if either
![]() $n\neq 1$
or
$n\neq 1$
or
![]() $r\neq 0$
, then the right-hand vertical arrow of the diagram of Corollary 2.20(iii) is injective.
$r\neq 0$
, then the right-hand vertical arrow of the diagram of Corollary 2.20(iii) is injective.
Remark 2.20.2. In the notation of Corollary 2.20, the bijectivity of the left-hand vertical arrow
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n+1}) \rightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
of the diagram of Corollary 2.20(v) is proven in [Reference Hoshi and Mochizuki8, Cor. 6.6] by applying, in essence, a well-known result concerning topological surfaces due to Dehn–Nielsen–Baer (cf. the proof of [Reference Mochizuki20, Cor. 5.1(ii)]). On the other hand, the equivalences of Corollary 2.19(i) (cf. also the injection of Corollary 2.20(i)), together with a similar argument to the argument applied in the proof of the bijectivity portion of [Reference Hoshi and Mochizuki8, Th. B]—that is, in essence, the argument applied in the proof of [Reference Mochizuki20, Cor. 3.3]—allow one to give a purely algebraic alternative proof of this bijectivity result in the case where
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n+1}) \rightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
of the diagram of Corollary 2.20(v) is proven in [Reference Hoshi and Mochizuki8, Cor. 6.6] by applying, in essence, a well-known result concerning topological surfaces due to Dehn–Nielsen–Baer (cf. the proof of [Reference Mochizuki20, Cor. 5.1(ii)]). On the other hand, the equivalences of Corollary 2.19(i) (cf. also the injection of Corollary 2.20(i)), together with a similar argument to the argument applied in the proof of the bijectivity portion of [Reference Hoshi and Mochizuki8, Th. B]—that is, in essence, the argument applied in the proof of [Reference Mochizuki20, Cor. 3.3]—allow one to give a purely algebraic alternative proof of this bijectivity result in the case where
![]() $n \geq \max \{3, n_{\mathrm {FC}}\}$
(cf. Corollary 2.20(vi)).
$n \geq \max \{3, n_{\mathrm {FC}}\}$
(cf. Corollary 2.20(vi)).
Corollary 2.21 (Discrete/profinite Dehn multi-twists).
In the situation of Example 2.4(i), write
![]() $\widehat {\mathcal {G}}_{X^{\mathrm {log}}}$
for the semi-graph of anabelioids of pro-
$\widehat {\mathcal {G}}_{X^{\mathrm {log}}}$
for the semi-graph of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of Proposition 2.5(iii) in the case where we take “
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of Proposition 2.5(iii) in the case where we take “
![]() $(\mathcal {G}, \Sigma )$
” to be
$(\mathcal {G}, \Sigma )$
” to be
![]() $(\mathcal {G}_{X^{\mathrm {log}}}, \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s})$
;
$(\mathcal {G}_{X^{\mathrm {log}}}, \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s})$
;
![]() $\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
,
$\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
,
![]() $\Pi _{\widehat {\mathcal {G}}_{X^{\mathrm {log}}}}$
for the respective fundamental groups of
$\Pi _{\widehat {\mathcal {G}}_{X^{\mathrm {log}}}}$
for the respective fundamental groups of
![]() $\mathcal {G}_{X^{\mathrm {log}}}$
,
$\mathcal {G}_{X^{\mathrm {log}}}$
,
![]() $\widehat {\mathcal {G}}_{X^{\mathrm {log}}}$
;
$\widehat {\mathcal {G}}_{X^{\mathrm {log}}}$
;
![]() $\widehat {\Pi }_{\mathcal {G}_{X^{\mathrm {log}}}}$
for the profinite completion of
$\widehat {\Pi }_{\mathcal {G}_{X^{\mathrm {log}}}}$
for the profinite completion of
![]() $\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
(so we have a natural outer isomorphism
$\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
(so we have a natural outer isomorphism
![]() $\widehat {\Pi }_{\mathcal {G}_{X^{\mathrm {log}}}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {G}}_{X^{\mathrm {log}}}}$
—cf. Proposition 2.5(iii));
$\widehat {\Pi }_{\mathcal {G}_{X^{\mathrm {log}}}} \stackrel {\sim }{\rightarrow } \Pi _{\widehat {\mathcal {G}}_{X^{\mathrm {log}}}}$
—cf. Proposition 2.5(iii));
for the subgroup consisting of the Dehn multi-twists of
![]() $\mathcal {G}_{X^{\mathrm {log}}}$
, that is, of
$\mathcal {G}_{X^{\mathrm {log}}}$
, that is, of
![]() $\alpha \in \mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
such that the following conditions are satisfied:
$\alpha \in \mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
such that the following conditions are satisfied:
-
(a)
 $\alpha $
is graphic (cf. Definition 2.7(ii)) and induces the identity automorphism on the underlying semi-graph of
$\alpha $
is graphic (cf. Definition 2.7(ii)) and induces the identity automorphism on the underlying semi-graph of
 $\mathcal {G}_{X^{\mathrm {log}}}$
.
$\mathcal {G}_{X^{\mathrm {log}}}$
. -
(b) Let
 $\Pi _{v} \subseteq \Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
be a verticial subgroup of
$\Pi _{v} \subseteq \Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
be a verticial subgroup of
 $\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
. Then the outomorphism of
$\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
. Then the outomorphism of
 $\Pi _{v}$
induced by restricting
$\Pi _{v}$
induced by restricting
 $\alpha $
(cf. (a); Corollary 2.18(v); the evident discrete analogue of [Reference Hoshi and Mochizuki10, Lem. 3.10]) is trivial.
$\alpha $
(cf. (a); Corollary 2.18(v); the evident discrete analogue of [Reference Hoshi and Mochizuki10, Lem. 3.10]) is trivial.
Then the following hold:
-
(i) The composite of natural outer homomorphisms
determines an injection $$\begin{align*}\Pi_{\mathcal{G}_{X^{\mathrm{log}}}} \longrightarrow \widehat{\Pi}_{\mathcal{G}_{X^{\mathrm{log}}}} \stackrel{\sim}{\longrightarrow} \Pi_{\widehat{\mathcal{G}}_{X^{\mathrm{log}}}} \end{align*}$$
$$\begin{align*}\Pi_{\mathcal{G}_{X^{\mathrm{log}}}} \longrightarrow \widehat{\Pi}_{\mathcal{G}_{X^{\mathrm{log}}}} \stackrel{\sim}{\longrightarrow} \Pi_{\widehat{\mathcal{G}}_{X^{\mathrm{log}}}} \end{align*}$$
 $$\begin{align*}\mathrm{Out}(\Pi_{\mathcal{G}_{X^{\mathrm{log}}}}) \hookrightarrow \mathrm{Out}(\Pi_{\widehat{\mathcal{G}}_{X^{\mathrm{log}}}}). \end{align*}$$
$$\begin{align*}\mathrm{Out}(\Pi_{\mathcal{G}_{X^{\mathrm{log}}}}) \hookrightarrow \mathrm{Out}(\Pi_{\widehat{\mathcal{G}}_{X^{\mathrm{log}}}}). \end{align*}$$
-
(ii) If one regards subgroups of
 $\mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
as subgroups of
$\mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
as subgroups of
 $\mathrm {Out}(\Pi _{\widehat {\mathcal {G}}_{X^{\mathrm {log}}}})$
by means of the injection of (i), then the equality
$\mathrm {Out}(\Pi _{\widehat {\mathcal {G}}_{X^{\mathrm {log}}}})$
by means of the injection of (i), then the equality  $$\begin{align*}\mathrm{Dehn}(\mathcal{G}_{X^{\mathrm{log}}}) = \mathrm{Dehn}(\widehat{\mathcal{G}}_{X^{\mathrm{log}}}) \cap \mathrm{Out}(\Pi_{\mathcal{G}_{X^{\mathrm{log}}}}) \end{align*}$$
$$\begin{align*}\mathrm{Dehn}(\mathcal{G}_{X^{\mathrm{log}}}) = \mathrm{Dehn}(\widehat{\mathcal{G}}_{X^{\mathrm{log}}}) \cap \mathrm{Out}(\Pi_{\mathcal{G}_{X^{\mathrm{log}}}}) \end{align*}$$
(cf. [Reference Hoshi and Mochizuki9, Def. 4.4]) holds.
-
(iii) The homomorphism of the final display of Example 2.4(i) determines, relative to the natural outer isomorphism
 $\pi _1(X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s) \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
, an isomorphism of free
$\pi _1(X^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})|_s) \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
, an isomorphism of free $$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \stackrel{\sim}{\longrightarrow} \mathrm{Dehn}(\mathcal{G}_{X^{\mathrm{log}}}) \end{align*}$$
$$\begin{align*}\pi_1(S^{\mathrm{log}}_{\mathrm{an}}(\mathbb{C})) \stackrel{\sim}{\longrightarrow} \mathrm{Dehn}(\mathcal{G}_{X^{\mathrm{log}}}) \end{align*}$$
 $\mathbb {Z}$
-modules of rank
$\mathbb {Z}$
-modules of rank
 $\mathrm {Node}(\mathcal {G}_{X^{\mathrm {log}}})^\sharp $
. Moreover, the image of this isomorphism is dense, relative to the profinite topology, in
$\mathrm {Node}(\mathcal {G}_{X^{\mathrm {log}}})^\sharp $
. Moreover, the image of this isomorphism is dense, relative to the profinite topology, in
 $\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
.
$\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
.
Proof. Assertion (i) follows from Corollary 2.20(i). Next, we verify assertion (ii). The inclusion
![]() $ \mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}}) \subseteq \mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}}) \cap \mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
follows immediately from the various definitions involved. To verify the reverse inclusion, let
$ \mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}}) \subseteq \mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}}) \cap \mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
follows immediately from the various definitions involved. To verify the reverse inclusion, let
![]() $\alpha \in \mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}}) \cap \mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
. Then it follows immediately from Corollary 2.19(i) together with the definition of
$\alpha \in \mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}}) \cap \mathrm {Out}(\Pi _{\mathcal {G}_{X^{\mathrm {log}}}})$
. Then it follows immediately from Corollary 2.19(i) together with the definition of
![]() $\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
, that the outomorphism
$\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
, that the outomorphism
![]() $\alpha $
of
$\alpha $
of
![]() $\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
satisfies the condition (a) in the statement of Corollary 2.21. Moreover, it follows immediately from Proposition 2.5(v) and Corollary 2.20(i), together with the definition of
$\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
satisfies the condition (a) in the statement of Corollary 2.21. Moreover, it follows immediately from Proposition 2.5(v) and Corollary 2.20(i), together with the definition of
![]() $\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
, that the outomorphism
$\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
, that the outomorphism
![]() $\alpha $
of
$\alpha $
of
![]() $\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
satisfies the condition (b) in the statement of Corollary 2.21. This completes the proof of assertion (ii).
$\Pi _{\mathcal {G}_{X^{\mathrm {log}}}}$
satisfies the condition (b) in the statement of Corollary 2.21. This completes the proof of assertion (ii).
Finally, we verify assertion (iii). First, let us observe that it follows immediately from the various definitions involved that the homomorphism of the final display of Example 2.4(i) factors through
![]() $\mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}})$
and has dense image (i.e., relative to the profinite topology) in
$\mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}})$
and has dense image (i.e., relative to the profinite topology) in
![]() $\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
(cf. [Reference Hoshi and Mochizuki9, Prop. 5.6(ii)]). Next, let us recall from [Reference Hoshi and Mochizuki9, Th. 4.8(ii) and (iv)] that if, for
$\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
(cf. [Reference Hoshi and Mochizuki9, Prop. 5.6(ii)]). Next, let us recall from [Reference Hoshi and Mochizuki9, Th. 4.8(ii) and (iv)] that if, for
![]() $e \in \mathrm {Node}(\mathcal {G}_{X^{\mathrm {log}}}) = \mathrm {Node}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
, we write
$e \in \mathrm {Node}(\mathcal {G}_{X^{\mathrm {log}}}) = \mathrm {Node}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
, we write
![]() $S_e \stackrel {\mathrm {def}}{=} \mathrm {Node}(\mathcal {G}_{X^{\mathrm {log}}}) \setminus \{e\}$
and
$S_e \stackrel {\mathrm {def}}{=} \mathrm {Node}(\mathcal {G}_{X^{\mathrm {log}}}) \setminus \{e\}$
and
![]() $(\mathcal {G}_{X^{\mathrm {log}}})_{\leadsto {S_e}}^\wedge $
for the semi-graph of anabelioids of pro-
$(\mathcal {G}_{X^{\mathrm {log}}})_{\leadsto {S_e}}^\wedge $
for the semi-graph of anabelioids of pro-
![]() $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of Proposition 2.5(iii), in the case where we take “
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of Proposition 2.5(iii), in the case where we take “
![]() $(\mathcal {G}, \Sigma )$
” to be
$(\mathcal {G}, \Sigma )$
” to be
![]() $((\mathcal {G}_{X^{\mathrm {log}}})_{\leadsto {S_e}}, \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s})$
(cf. Definition 2.9) and regard
$((\mathcal {G}_{X^{\mathrm {log}}})_{\leadsto {S_e}}, \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s})$
(cf. Definition 2.9) and regard
![]() $\mathrm {Dehn}((\mathcal {G}_{X^{\mathrm {log}}})_{\leadsto {S_e}}^\wedge )$
as a closed subgroup of
$\mathrm {Dehn}((\mathcal {G}_{X^{\mathrm {log}}})_{\leadsto {S_e}}^\wedge )$
as a closed subgroup of
![]() $\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
via the specialization outer isomorphism of [Reference Hoshi and Mochizuki9, Def. 2.10] (cf. also Remark 2.9.1 and Proposition 2.10 of the present paper), then we have an equality
$\mathrm {Dehn}(\widehat {\mathcal {G}}_{X^{\mathrm {log}}})$
via the specialization outer isomorphism of [Reference Hoshi and Mochizuki9, Def. 2.10] (cf. also Remark 2.9.1 and Proposition 2.10 of the present paper), then we have an equality
 $$\begin{align*}\mathrm{Dehn}(\widehat{\mathcal{G}}_{X^{\mathrm{log}}}) \, \, = \, \, \bigoplus_{e \in \mathrm{Node}(\mathcal{G}_{X^{\mathrm{log}}})} \, \mathrm{Dehn}((\mathcal{G}_{X^{\mathrm{log}}})_{\leadsto {S_e}}^\wedge), \end{align*}$$
$$\begin{align*}\mathrm{Dehn}(\widehat{\mathcal{G}}_{X^{\mathrm{log}}}) \, \, = \, \, \bigoplus_{e \in \mathrm{Node}(\mathcal{G}_{X^{\mathrm{log}}})} \, \mathrm{Dehn}((\mathcal{G}_{X^{\mathrm{log}}})_{\leadsto {S_e}}^\wedge), \end{align*}$$
where each direct summand is (noncanonically) isomorphic to
![]() $\widehat {\mathbb {Z}}$
. Here, we note that these specialization outer isomorphisms are compatible (cf. [Reference Hoshi and Mochizuki9, Prop. 5.6(ii)–(iv)]) with the corresponding homomorphisms of the final display of Example 2.4(i). Thus, in light of the density assertion that has already been verified, one verifies immediately that, to complete the verification of assertion (iii), it suffices to verify that the image of
$\widehat {\mathbb {Z}}$
. Here, we note that these specialization outer isomorphisms are compatible (cf. [Reference Hoshi and Mochizuki9, Prop. 5.6(ii)–(iv)]) with the corresponding homomorphisms of the final display of Example 2.4(i). Thus, in light of the density assertion that has already been verified, one verifies immediately that, to complete the verification of assertion (iii), it suffices to verify that the image of
![]() $\mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}})$
via the projection to any direct summand of the direct sum decomposition of the above display is contained in some submodule of the direct summand that is isomorphic to
$\mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}})$
via the projection to any direct summand of the direct sum decomposition of the above display is contained in some submodule of the direct summand that is isomorphic to
![]() $\mathbb {Z}$
. To this end, let us recall from [Reference Hoshi and Mochizuki9, Th. 4.8(iv)] that such an image via a projection to a direct summand may be computed by considering the homomorphism of the first display of [Reference Hoshi and Mochizuki9, Lem. 4.6(ii)], that is, which determines an isomorphism between the direct summand under consideration and any profinite nodal subgroup
$\mathbb {Z}$
. To this end, let us recall from [Reference Hoshi and Mochizuki9, Th. 4.8(iv)] that such an image via a projection to a direct summand may be computed by considering the homomorphism of the first display of [Reference Hoshi and Mochizuki9, Lem. 4.6(ii)], that is, which determines an isomorphism between the direct summand under consideration and any profinite nodal subgroup
![]() $\widehat {\Pi }_e$
associated with the node e corresponding to the direct summand. On the other hand, it follows immediately—in light of the definition of this isomorphism—from Proposition 2.5(v) and Corollary 2.17(i) that the image of
$\widehat {\Pi }_e$
associated with the node e corresponding to the direct summand. On the other hand, it follows immediately—in light of the definition of this isomorphism—from Proposition 2.5(v) and Corollary 2.17(i) that the image of
![]() $\mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}})$
under consideration is contained in a suitable discrete nodal subgroup
$\mathrm {Dehn}(\mathcal {G}_{X^{\mathrm {log}}})$
under consideration is contained in a suitable discrete nodal subgroup
![]() $\Pi _e (\cong \mathbb {Z})$
associated with e (cf. Remark 2.6.1). This completes the proof of assertion (iii).
$\Pi _e (\cong \mathbb {Z})$
associated with e (cf. Remark 2.6.1). This completes the proof of assertion (iii).
Definition 2.22. Suppose that
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
. Let
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
. Let
![]() $(g,r)$
be a pair of nonnegative integers such that
$(g,r)$
be a pair of nonnegative integers such that
![]() $2g-2+r> 0$
; n a positive integer;
$2g-2+r> 0$
; n a positive integer;
![]() $k \stackrel {\mathrm {def}}{=} \mathbb {C}$
;
$k \stackrel {\mathrm {def}}{=} \mathbb {C}$
;
![]() $S^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)^{\mathrm {log}}$
the log scheme obtained by equipping
$S^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)^{\mathrm {log}}$
the log scheme obtained by equipping
![]() $S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)$
with the log structure determined by the fs chart
$S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)$
with the log structure determined by the fs chart
![]() $\mathbb {N} \rightarrow k$
that maps
$\mathbb {N} \rightarrow k$
that maps
![]() $1 \mapsto 0$
;
$1 \mapsto 0$
;
![]() $X^{\mathrm {log}} = X_1^{\mathrm {log}}$
a stable log curve of type
$X^{\mathrm {log}} = X_1^{\mathrm {log}}$
a stable log curve of type
![]() $(g,r)$
over
$(g,r)$
over
![]() $S^{\mathrm {log}}$
. For each (possibly empty) subset
$S^{\mathrm {log}}$
. For each (possibly empty) subset
![]() $E\subseteq \{1, \dots , n\}$
, write
$E\subseteq \{1, \dots , n\}$
, write
for the
![]() $E^\sharp $
-th log configuration space of the stable log curve
$E^\sharp $
-th log configuration space of the stable log curve
![]() $X^{\mathrm {log}}$
(cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]), where we think of the factors as being labeled by the elements of
$X^{\mathrm {log}}$
(cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]), where we think of the factors as being labeled by the elements of
![]() $E \subseteq \{1, \dots , n\}$
(cf. the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3] in the case where
$E \subseteq \{1, \dots , n\}$
(cf. the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3] in the case where
![]() $(\Sigma , k) = (\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s},\mathbb {C})$
). For each nonnegative integer n and each (possibly empty) subset
$(\Sigma , k) = (\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s},\mathbb {C})$
). For each nonnegative integer n and each (possibly empty) subset
![]() $E\subseteq \{1 , \dots , n\}$
, write
$E\subseteq \{1 , \dots , n\}$
, write
![]() $(X_E^{\mathrm {log}})_{\mathrm {an}} \rightarrow S^{\mathrm {log}}_{\mathrm {an}}$
for the morphism of fs log analytic spaces determined by the morphism
$(X_E^{\mathrm {log}})_{\mathrm {an}} \rightarrow S^{\mathrm {log}}_{\mathrm {an}}$
for the morphism of fs log analytic spaces determined by the morphism
![]() $X_E^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
;
$X_E^{\mathrm {log}} \rightarrow S^{\mathrm {log}}$
;
![]() $(X_E^{\mathrm {log}})_{\mathrm {an}}(\mathbb {C})$
,
$(X_E^{\mathrm {log}})_{\mathrm {an}}(\mathbb {C})$
,
![]() $S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
for the respective topological spaces “
$S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
for the respective topological spaces “
![]() $X^{\mathrm {log}}$
” defined in [Reference Kato and Nakayama12, (1.2)] in the case where we take the “X” of [Reference Kato and Nakayama12, (1.2)] to be
$X^{\mathrm {log}}$
” defined in [Reference Kato and Nakayama12, (1.2)] in the case where we take the “X” of [Reference Kato and Nakayama12, (1.2)] to be
![]() $(X_E^{\mathrm {log}})_{\mathrm {an}}$
,
$(X_E^{\mathrm {log}})_{\mathrm {an}}$
,
![]() $S_{\mathrm {an}}^{\mathrm {log}}$
(cf. the notation established in Example 2.4(i)). Let
$S_{\mathrm {an}}^{\mathrm {log}}$
(cf. the notation established in Example 2.4(i)). Let
![]() $s \in S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
. Write
$s \in S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
. Write
for the fiber of the natural morphism
![]() $(X_E^{\mathrm {log}})_{\mathrm {an}}(\mathbb {C}) \rightarrow S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
at s;
$(X_E^{\mathrm {log}})_{\mathrm {an}}(\mathbb {C}) \rightarrow S^{\mathrm {log}}_{\mathrm {an}}(\mathbb {C})$
at s;
for the discrete topological fundamental group of
![]() $\mathfrak {X}_E$
;
$\mathfrak {X}_E$
;
Thus, for sets
![]() $E' \subseteq E \subseteq \{1, \dots , n\}$
, we have a projection
$E' \subseteq E \subseteq \{1, \dots , n\}$
, we have a projection
obtained by forgetting the factors that belong to
![]() $E\setminus E'$
. For sets
$E\setminus E'$
. For sets
![]() $E' \subseteq E \subseteq \{1, \dots , n\}$
and nonnegative integers
$E' \subseteq E \subseteq \{1, \dots , n\}$
and nonnegative integers
![]() $m \leq n$
, write
$m \leq n$
, write
for some fixed surjection (that belongs to the collection of surjections that constitutes the outer surjection) induced by
![]() $p_{E/E'}^{\mathrm {an}}$
;
$p_{E/E'}^{\mathrm {an}}$
;
Finally, we shall write “
![]() $\widehat {\Pi }^{\mathrm {disc}}_{(-)}$
” for the profinite completion of “
$\widehat {\Pi }^{\mathrm {disc}}_{(-)}$
” for the profinite completion of “
![]() $\Pi _{(-)}^{\mathrm {disc}}$
.” Thus, we have a natural outer isomorphism
$\Pi _{(-)}^{\mathrm {disc}}$
.” Thus, we have a natural outer isomorphism
where
![]() $\Pi _{E}$
is as in the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3]. In the following, we shall also write
$\Pi _{E}$
is as in the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3]. In the following, we shall also write
![]() $X^{\mathrm {log}}_n \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_{\{1 , \dots , n\}}$
;
$X^{\mathrm {log}}_n \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_{\{1 , \dots , n\}}$
;
![]() $\Pi _n \stackrel {\mathrm {def}}{=} \Pi _{\{1 , \dots , n \}}$
.
$\Pi _n \stackrel {\mathrm {def}}{=} \Pi _{\{1 , \dots , n \}}$
.
Definition 2.23. In the notation of Definition 2.22, let
![]() $i\in E \subseteq \{1 , \dots , n\}$
;
$i\in E \subseteq \{1 , \dots , n\}$
;
![]() $x \in X_n(\mathbb {C})$
a
$x \in X_n(\mathbb {C})$
a
![]() $\mathbb {C}$
-valued geometric point of the underlying scheme
$\mathbb {C}$
-valued geometric point of the underlying scheme
![]() $X_n$
of
$X_n$
of
![]() $X_n^{\mathrm {log}}$
.
$X_n^{\mathrm {log}}$
.
-
(i) We shall write
for the semi-graph of temperoids of HSD-type associated with $$\begin{align*}\mathcal{G}^{\mathrm{disc}} \end{align*}$$
$$\begin{align*}\mathcal{G}^{\mathrm{disc}} \end{align*}$$
 $X^{\mathrm {log}}$
(cf. Example 2.4(ii)); for the semi-graph of temperoids of HSD-type associated with the geometric fiber (cf. Example 2.4(ii); Remark 2.4.1) of the projection
$X^{\mathrm {log}}$
(cf. Example 2.4(ii)); for the semi-graph of temperoids of HSD-type associated with the geometric fiber (cf. Example 2.4(ii); Remark 2.4.1) of the projection $$\begin{align*}\mathcal{G}^{\mathrm{disc}}_{i\in E, x} \end{align*}$$
$$\begin{align*}\mathcal{G}^{\mathrm{disc}}_{i\in E, x} \end{align*}$$
 $p^{\mathrm {log}}_{E / (E\setminus \{i\})} \colon X_E^{\mathrm {log}} \rightarrow X_{E\setminus \{i\}}^{\mathrm {log}}$
over
$p^{\mathrm {log}}_{E / (E\setminus \{i\})} \colon X_E^{\mathrm {log}} \rightarrow X_{E\setminus \{i\}}^{\mathrm {log}}$
over
 $x_{E\setminus \{i\}}^{\mathrm {log}} \rightarrow X_{E\setminus \{i\}} ^{\mathrm {log}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(i)]); for the respective fundamental groups of
$x_{E\setminus \{i\}}^{\mathrm {log}} \rightarrow X_{E\setminus \{i\}} ^{\mathrm {log}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(i)]); for the respective fundamental groups of $$ \begin{align*}\Pi_{\mathcal{G}^{\mathrm{disc}}} , \, \, \, \Pi_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}}\end{align*} $$
$$ \begin{align*}\Pi_{\mathcal{G}^{\mathrm{disc}}} , \, \, \, \Pi_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}}\end{align*} $$
 $\mathcal {G}^{\mathrm {disc}}$
,
$\mathcal {G}^{\mathrm {disc}}$
,
 $\mathcal {G}^{\mathrm {disc}}_{i\in E, x}$
(cf. Proposition 2.5(i)); for the profinite completion of
$\mathcal {G}^{\mathrm {disc}}_{i\in E, x}$
(cf. Proposition 2.5(i)); for the profinite completion of $$ \begin{align*}\widehat{\Pi}_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}}\end{align*} $$
$$ \begin{align*}\widehat{\Pi}_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}}\end{align*} $$
 $ \Pi _{\mathcal {G}^{\mathrm {disc}}_{i\in E, x}} $
. Thus, it follows from the discussion of Remark 2.5.2 that we have a natural graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]) outer isomorphism where
$ \Pi _{\mathcal {G}^{\mathrm {disc}}_{i\in E, x}} $
. Thus, it follows from the discussion of Remark 2.5.2 that we have a natural graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]) outer isomorphism where $$ \begin{align*}\widehat{\Pi}_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}} \, \, \stackrel{\sim}{\longrightarrow} \, \, \Pi_{\mathcal{G}_{i\in E, x}}, \end{align*} $$
$$ \begin{align*}\widehat{\Pi}_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}} \, \, \stackrel{\sim}{\longrightarrow} \, \, \Pi_{\mathcal{G}_{i\in E, x}}, \end{align*} $$
 $\mathcal {G}_{i\in E, x}$
is the semi-graph of anabelioids of pro-
$\mathcal {G}_{i\in E, x}$
is the semi-graph of anabelioids of pro-
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of [Reference Hoshi and Mochizuki10, Def. 3.1(iii)], and hence a natural isomorphism of semi-graphs of anabelioids where we write
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of [Reference Hoshi and Mochizuki10, Def. 3.1(iii)], and hence a natural isomorphism of semi-graphs of anabelioids where we write $$\begin{align*}\widehat{\mathcal{G}}^{\mathrm{disc}}_{i\in E, x} \, \, \stackrel{\sim}{\longrightarrow} \, \, \mathcal{G}_{i\in E, x}, \end{align*}$$
$$\begin{align*}\widehat{\mathcal{G}}^{\mathrm{disc}}_{i\in E, x} \, \, \stackrel{\sim}{\longrightarrow} \, \, \mathcal{G}_{i\in E, x}, \end{align*}$$
 $\widehat {\mathcal {G}}^{\mathrm {disc}}_{i\in E, x}$
for the semi-graph of anabelioids of pro-
$\widehat {\mathcal {G}}^{\mathrm {disc}}_{i\in E, x}$
for the semi-graph of anabelioids of pro-
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of Proposition 2.5(iii) in the case where we take “
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type of Proposition 2.5(iii) in the case where we take “
 $(\mathcal {G}, \Sigma )$
” to be
$(\mathcal {G}, \Sigma )$
” to be
 $(\mathcal {G}^{\mathrm {disc}}_{i\in E, x}, \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s})$
. Moreover, it follows immediately from the discussion of Example 2.4 that we have a natural
$(\mathcal {G}^{\mathrm {disc}}_{i\in E, x}, \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s})$
. Moreover, it follows immediately from the discussion of Example 2.4 that we have a natural
 $\Pi _{E}^{\mathrm {disc}}$
-orbit (i.e., relative to composition with automorphisms induced by conjugation by elements of
$\Pi _{E}^{\mathrm {disc}}$
-orbit (i.e., relative to composition with automorphisms induced by conjugation by elements of
 $\Pi _{E}^{\mathrm {disc}}$
) of isomorphisms
$\Pi _{E}^{\mathrm {disc}}$
) of isomorphisms  $$\begin{align*}( \Pi_{E}^{\mathrm{disc}} \supseteq) \, \, \Pi_{{E / (E\setminus\{i\})}}^{\mathrm{disc}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}}. \end{align*}$$
$$\begin{align*}( \Pi_{E}^{\mathrm{disc}} \supseteq) \, \, \Pi_{{E / (E\setminus\{i\})}}^{\mathrm{disc}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}^{\mathrm{disc}}_{i\in E, x}}. \end{align*}$$
One verifies immediately from the various definitions involved that the diagram

—where the upper horizontal arrow is an element of the
 $\widehat {\Pi }^{\mathrm {disc}}_{E}$
-orbit of isomorphisms induced by the
$\widehat {\Pi }^{\mathrm {disc}}_{E}$
-orbit of isomorphisms induced by the
 $\Pi _{E}^{\mathrm {disc}}$
-orbit of isomorphisms of the above discussion; the lower horizontal arrow is an element of the
$\Pi _{E}^{\mathrm {disc}}$
-orbit of isomorphisms of the above discussion; the lower horizontal arrow is an element of the
 $\Pi _{E}$
-orbit of isomorphisms of [Reference Hoshi and Mochizuki10, Def. 3.1(iii)]; the left-hand vertical arrow is the isomorphism obtained by forming the restriction of an isomorphism
$\Pi _{E}$
-orbit of isomorphisms of [Reference Hoshi and Mochizuki10, Def. 3.1(iii)]; the left-hand vertical arrow is the isomorphism obtained by forming the restriction of an isomorphism
 $\widehat {\Pi }^{\mathrm {disc}}_{E} \stackrel {\sim }{\rightarrow } \Pi _{E}$
that belongs to the outer isomorphism of the final display of Definition 2.22; the right-hand vertical arrow is an isomorphism that belongs to the outer isomorphism of the above discussion—commutes up to composition with automorphisms induced by conjugation by elements of
$\widehat {\Pi }^{\mathrm {disc}}_{E} \stackrel {\sim }{\rightarrow } \Pi _{E}$
that belongs to the outer isomorphism of the final display of Definition 2.22; the right-hand vertical arrow is an isomorphism that belongs to the outer isomorphism of the above discussion—commutes up to composition with automorphisms induced by conjugation by elements of
 $\Pi _{E}$
.
$\Pi _{E}$
. -
(ii) We shall say that a vertex
 $v \in \mathrm {Vert}(\mathcal {G}^{\mathrm {disc}}_{i\in E, x})$
is a(n) (E-)tripod of
$v \in \mathrm {Vert}(\mathcal {G}^{\mathrm {disc}}_{i\in E, x})$
is a(n) (E-)tripod of
 $\mathfrak {X}_n$
if v is of type
$\mathfrak {X}_n$
if v is of type
 $(0,3)$
(cf. Definition 2.6(iii)). Thus, one verifies easily that
$(0,3)$
(cf. Definition 2.6(iii)). Thus, one verifies easily that
 $v \in \mathrm {Vert}(\mathcal {G}^{\mathrm {disc}}_{i\in E, x})$
is a(n) (E-)tripod if and only if the corresponding vertex of
$v \in \mathrm {Vert}(\mathcal {G}^{\mathrm {disc}}_{i\in E, x})$
is a(n) (E-)tripod if and only if the corresponding vertex of
 $\mathcal {G}_{i\in E, x}$
via the graphic outer isomorphism
$\mathcal {G}_{i\in E, x}$
via the graphic outer isomorphism
 $\widehat {\Pi }_{\mathcal {G}^{\mathrm {disc}}_{i\in E, x}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}_{i\in E, x}}$
of (i) is a(n) (E-)tripod of
$\widehat {\Pi }_{\mathcal {G}^{\mathrm {disc}}_{i\in E, x}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}_{i\in E, x}}$
of (i) is a(n) (E-)tripod of
 $X^{\mathrm {log}}_n$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(v)]). We shall refer to a verticial subgroup of
$X^{\mathrm {log}}_n$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(v)]). We shall refer to a verticial subgroup of
 $\Pi _{\mathcal {G}^{\mathrm {disc}}_{i\in E, x}}$
associated with a(n) (E-)tripod of
$\Pi _{\mathcal {G}^{\mathrm {disc}}_{i\in E, x}}$
associated with a(n) (E-)tripod of
 $\mathfrak {X}_n$
as a(n) (E-)tripod of
$\mathfrak {X}_n$
as a(n) (E-)tripod of
 $\Pi _{n}^{\mathrm {disc}}$
.
$\Pi _{n}^{\mathrm {disc}}$
. -
(iii) Let
 $\mathbb {P}$
be a property of (E-)tripods of
$\mathbb {P}$
be a property of (E-)tripods of
 $\Pi _{n}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.3(i)]) or
$\Pi _{n}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.3(i)]) or
 $X^{\mathrm {log}}_n$
(e.g., the property of being strict—cf. [Reference Hoshi and Mochizuki10, Def. 3.3(iii)]; the property of arising from an edge—cf. [Reference Hoshi and Mochizuki10, Def. 3.7(i)]; the property of being central—cf. [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]). Then we shall say that a(n) (E-)tripod of
$X^{\mathrm {log}}_n$
(e.g., the property of being strict—cf. [Reference Hoshi and Mochizuki10, Def. 3.3(iii)]; the property of arising from an edge—cf. [Reference Hoshi and Mochizuki10, Def. 3.7(i)]; the property of being central—cf. [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]). Then we shall say that a(n) (E-)tripod of
 $\Pi _{n}^{\mathrm {disc}}$
or
$\Pi _{n}^{\mathrm {disc}}$
or
 $\mathfrak {X}_n$
(cf. (ii)) satisfies
$\mathfrak {X}_n$
(cf. (ii)) satisfies
 $\mathbb {P}$
if the corresponding (E-)tripod of
$\mathbb {P}$
if the corresponding (E-)tripod of
 $\Pi _{n}$
or
$\Pi _{n}$
or
 $X^{\mathrm {log}}_n$
satisfies
$X^{\mathrm {log}}_n$
satisfies
 $\mathbb {P}$
.
$\mathbb {P}$
. -
(iv) Let
 $T \subseteq \Pi _{E}^{\mathrm {disc}}$
be an E-tripod of
$T \subseteq \Pi _{E}^{\mathrm {disc}}$
be an E-tripod of
 $\Pi _{n}^{\mathrm {disc}}$
(cf. (ii)). Then one may define the subgroups of
$\Pi _{n}^{\mathrm {disc}}$
(cf. (ii)). Then one may define the subgroups of $$ \begin{align*} \mathrm{Out}^{\mathrm{C}}(T) , \, \, \, \mathrm{Out}^{\mathrm{C}}(T)^{\mathrm{cusp}} , \, \, \, \mathrm{Out}^{\mathrm{C}}(T)^\Delta , \, \, \, \mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} \, \, \subseteq \, \, \mathrm{Out}(T)\end{align*} $$
$$ \begin{align*} \mathrm{Out}^{\mathrm{C}}(T) , \, \, \, \mathrm{Out}^{\mathrm{C}}(T)^{\mathrm{cusp}} , \, \, \, \mathrm{Out}^{\mathrm{C}}(T)^\Delta , \, \, \, \mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} \, \, \subseteq \, \, \mathrm{Out}(T)\end{align*} $$
 $\mathrm {Out}(T)$
in an entirely analogous fashion to the definition of the closed subgroups “
$\mathrm {Out}(T)$
in an entirely analogous fashion to the definition of the closed subgroups “
 $\mathrm {Out}^{\mathrm {C}}(T)$
,” “
$\mathrm {Out}^{\mathrm {C}}(T)$
,” “
 $\mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}}$
,” “
$\mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}}$
,” “
 $\mathrm {Out}^{\mathrm {C}}(T)^\Delta $
,” “
$\mathrm {Out}^{\mathrm {C}}(T)^\Delta $
,” “
 $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +}$
” of “
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +}$
” of “
 $\mathrm {Out}(T)$
” given in [Reference Hoshi and Mochizuki10, Def. 3.4(i)]. We leave the routine details to the reader.
$\mathrm {Out}(T)$
” given in [Reference Hoshi and Mochizuki10, Def. 3.4(i)]. We leave the routine details to the reader.
Theorem 2.24 (Outomorphisms preserving tripods).
In the notation of Definition 2.22, let
![]() $E \subseteq \{1 , \dots , n\}$
be a subset and
$E \subseteq \{1 , \dots , n\}$
be a subset and
![]() $T \subseteq \Pi _{E}^{\mathrm {disc}}$
an E-tripod of
$T \subseteq \Pi _{E}^{\mathrm {disc}}$
an E-tripod of
![]() $\Pi _{n}^{\mathrm {disc}}$
(cf. Definition 2.23(ii)). Let us write
$\Pi _{n}^{\mathrm {disc}}$
(cf. Definition 2.23(ii)). Let us write
for the subgroup of
![]() $\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
(cf. the notational conventions introduced in the statement of Corollary 2.20) consisting of
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
(cf. the notational conventions introduced in the statement of Corollary 2.20) consisting of
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
such that the outomorphism of
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
such that the outomorphism of
![]() $\Pi _{E}^{\mathrm {disc}}$
determined by
$\Pi _{E}^{\mathrm {disc}}$
determined by
![]() $\alpha $
preserves the
$\alpha $
preserves the
![]() $\Pi _{E}^{\mathrm {disc}}$
-conjugacy class of
$\Pi _{E}^{\mathrm {disc}}$
-conjugacy class of
![]() $T \subseteq \Pi _{E}^{\mathrm {disc}}$
;
$T \subseteq \Pi _{E}^{\mathrm {disc}}$
;
(cf. the notational conventions introduced in the statement of Corollary 2.20);
![]() $\Pi _{} \stackrel {\mathrm {def}}{=} \Pi _{1}$
;
$\Pi _{} \stackrel {\mathrm {def}}{=} \Pi _{1}$
;
![]() $\Pi _{}^{\mathrm {disc}} \stackrel {\mathrm {def}}{=} \Pi _{1}^{\mathrm {disc}}$
;
$\Pi _{}^{\mathrm {disc}} \stackrel {\mathrm {def}}{=} \Pi _{1}^{\mathrm {disc}}$
;
![]() $\mathrm {Out}^{\mathrm {C}}(\Pi _{}^{\mathrm {disc}}) \stackrel {\mathrm {def}}{=} \mathrm {Out}^{\mathrm {FC}}(\Pi _{}^{\mathrm {disc}})$
;
$\mathrm {Out}^{\mathrm {C}}(\Pi _{}^{\mathrm {disc}}) \stackrel {\mathrm {def}}{=} \mathrm {Out}^{\mathrm {FC}}(\Pi _{}^{\mathrm {disc}})$
;
![]() $\mathrm {Out}^{\mathrm {C}}(\Pi ) \stackrel {\mathrm {def}}{=} \mathrm {Out}^{\mathrm {FC}}(\Pi _{})$
. Then the following hold:
$\mathrm {Out}^{\mathrm {C}}(\Pi ) \stackrel {\mathrm {def}}{=} \mathrm {Out}^{\mathrm {FC}}(\Pi _{})$
. Then the following hold:
-
(i) Write
 $\widehat {T}$
for the profinite completion of T. Then the natural homomorphism is injective. If, moreover, one regards subgroups of
$\widehat {T}$
for the profinite completion of T. Then the natural homomorphism is injective. If, moreover, one regards subgroups of $$ \begin{align*}\mathrm{Out}(T) \longrightarrow \mathrm{Out}(\widehat{T}) \end{align*} $$
$$ \begin{align*}\mathrm{Out}(T) \longrightarrow \mathrm{Out}(\widehat{T}) \end{align*} $$
 $\mathrm {Out}(T)$
as subgroups of
$\mathrm {Out}(T)$
as subgroups of
 $\mathrm {Out}(\widehat {T})$
via this injection, then it holds that
$\mathrm {Out}(\widehat {T})$
via this injection, then it holds that  $$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T) = \mathrm{Out}^{\mathrm{C}}(\widehat{T}) \cap \mathrm{Out}(T) , \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T) = \mathrm{Out}^{\mathrm{C}}(\widehat{T}) \cap \mathrm{Out}(T) , \end{align*}$$
 $$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\mathrm{cusp}} = \mathrm{Out}^{\mathrm{C}}(\widehat{T})^{\mathrm{cusp}} \cap \mathrm{Out}(T) , \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\mathrm{cusp}} = \mathrm{Out}^{\mathrm{C}}(\widehat{T})^{\mathrm{cusp}} \cap \mathrm{Out}(T) , \end{align*}$$
 $$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\Delta} = \mathrm{Out}^{\mathrm{C}}(\widehat{T})^{\Delta} \cap \mathrm{Out}(T) , \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\Delta} = \mathrm{Out}^{\mathrm{C}}(\widehat{T})^{\Delta} \cap \mathrm{Out}(T) , \end{align*}$$
 $$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} = \mathrm{Out}^{\mathrm{C}}(\widehat{T})^{\Delta +} \cap \mathrm{Out}(T) \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} = \mathrm{Out}^{\mathrm{C}}(\widehat{T})^{\Delta +} \cap \mathrm{Out}(T) \end{align*}$$
(cf. Definition 2.23(iv); [Reference Hoshi and Mochizuki10, Def. 3.4(i)]).
-
(ii) It holds that
 $$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\mathrm{cusp}} = \mathrm{Out}^{\mathrm{C}}(T)^{\Delta} = \mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} \cong \mathbb{Z} / 2\mathbb{Z} , \end{align*}$$
where we write
$$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T)^{\mathrm{cusp}} = \mathrm{Out}^{\mathrm{C}}(T)^{\Delta} = \mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} \cong \mathbb{Z} / 2\mathbb{Z} , \end{align*}$$
where we write $$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T) \cong \mathbb{Z} / 2\mathbb{Z} \times \mathfrak{S}_3, \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{C}}(T) \cong \mathbb{Z} / 2\mathbb{Z} \times \mathfrak{S}_3, \end{align*}$$
 $\mathfrak {S}_3$
for the symmetric group on
$\mathfrak {S}_3$
for the symmetric group on
 $3$
letters.
$3$
letters.
-
(iii) The commensurator and centralizer of
 $T \in \Pi _{E}^{\mathrm {disc}}$
satisfy the equality Thus, by applying the evident discrete analogue of [Reference Hoshi and Mochizuki10, Lem. 3.10] to outomorphisms of
$T \in \Pi _{E}^{\mathrm {disc}}$
satisfy the equality Thus, by applying the evident discrete analogue of [Reference Hoshi and Mochizuki10, Lem. 3.10] to outomorphisms of $$\begin{align*}C_{\Pi_{E}^{\mathrm{disc}}}(T) = T\times Z_{\Pi_{E}^{\mathrm{disc}}}(T). \end{align*}$$
$$\begin{align*}C_{\Pi_{E}^{\mathrm{disc}}}(T) = T\times Z_{\Pi_{E}^{\mathrm{disc}}}(T). \end{align*}$$
 $\Pi _{E}^{\mathrm {disc}}$
determined by elements of
$\Pi _{E}^{\mathrm {disc}}$
determined by elements of
 $\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})[T]$
, one obtains a natural homomorphism
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})[T]$
, one obtains a natural homomorphism  $$\begin{align*}\mathfrak{T}_T \colon \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T] \longrightarrow \mathrm{Out}(T). \end{align*}$$
$$\begin{align*}\mathfrak{T}_T \colon \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T] \longrightarrow \mathrm{Out}(T). \end{align*}$$
-
(iv) Suppose that
 $n\geq 3$
, and that T is central (cf. Definition 2.23(iii)). Then it holds that Moreover, the homomorphism
$n\geq 3$
, and that T is central (cf. Definition 2.23(iii)). Then it holds that Moreover, the homomorphism $$\begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}}) = \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T]. \end{align*}$$
of (iii) determines a surjection
$$\begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}}) = \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T]. \end{align*}$$
of (iii) determines a surjection $$\begin{align*}\mathfrak{T}_T \colon \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}}) = \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T] \longrightarrow \mathrm{Out}(T) \end{align*}$$
We shall refer to this homomorphism as the tripod homomorphism associated with
$$\begin{align*}\mathfrak{T}_T \colon \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}}) = \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T] \longrightarrow \mathrm{Out}(T) \end{align*}$$
We shall refer to this homomorphism as the tripod homomorphism associated with $$\begin{align*}\mathrm{Out}^{\mathrm{FC}}(\Pi_{n}^{\mathrm{disc}}) \twoheadrightarrow \mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} \, \, (\cong \mathbb{Z} / 2\mathbb{Z}). \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{FC}}(\Pi_{n}^{\mathrm{disc}}) \twoheadrightarrow \mathrm{Out}^{\mathrm{C}}(T)^{\Delta +} \, \, (\cong \mathbb{Z} / 2\mathbb{Z}). \end{align*}$$
 $\Pi _{n}^{\mathrm {disc}}$
.
$\Pi _{n}^{\mathrm {disc}}$
.
-
(v) The profinite completion
 $\widehat T$
determines an E-tripod of
$\widehat T$
determines an E-tripod of
 $\Pi _{n}$
, which, by abuse of notation, we denote by
$\Pi _{n}$
, which, by abuse of notation, we denote by
 $\widehat T$
. Now suppose that T is E-strict (cf. Definition 2.23(iii)). Then it holds that
$\widehat T$
. Now suppose that T is E-strict (cf. Definition 2.23(iii)). Then it holds that  $$\begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T] \, \, = \, \, \mathrm{Out}^{\mathrm{F}}(\Pi_{n})[\widehat T] \cap \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}}) \end{align*}$$
$$\begin{align*}\mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}})[T] \, \, = \, \, \mathrm{Out}^{\mathrm{F}}(\Pi_{n})[\widehat T] \cap \mathrm{Out}^{\mathrm{F}}(\Pi_{n}^{\mathrm{disc}}) \end{align*}$$
(cf. [Reference Hoshi and Mochizuki10, Th. 3.16]).
-
(vi) Suppose that the semi-graph of anabelioids of pro-
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type
 $\mathcal {G}$
associated with
$\mathcal {G}$
associated with
 $X^{\mathrm {log}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(ii)]) is totally degenerate (cf. [Reference Hoshi and Mochizuki9, Def. 2.3(iv)]). Recall that
$X^{\mathrm {log}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(ii)]) is totally degenerate (cf. [Reference Hoshi and Mochizuki9, Def. 2.3(iv)]). Recall that
 $\mathcal {G}$
may be naturally identified with the semi-graph of anabelioids of pro-
$\mathcal {G}$
may be naturally identified with the semi-graph of anabelioids of pro-
 $\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type determined by
$\mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
PSC-type determined by
 $\mathcal {G}^{\mathrm {disc}}$
(cf. Proposition 2.5(iii); the discussion of Definition 2.23(i)). Then one has an equality —where the superscript “
$\mathcal {G}^{\mathrm {disc}}$
(cf. Proposition 2.5(iii); the discussion of Definition 2.23(i)). Then one has an equality —where the superscript “ $$\begin{align*}\mathrm{Aut}(\mathcal{G}^{\mathrm{disc}})^{-} \, \, = \, \, \mathrm{Aut}(\mathcal{G}) \, \cap \, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}})^{-} \, \, \, \, (\subseteq \, \mathrm{Out}^{\mathrm{C}}(\Pi_{})) \end{align*}$$
$$\begin{align*}\mathrm{Aut}(\mathcal{G}^{\mathrm{disc}})^{-} \, \, = \, \, \mathrm{Aut}(\mathcal{G}) \, \cap \, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}})^{-} \, \, \, \, (\subseteq \, \mathrm{Out}^{\mathrm{C}}(\Pi_{})) \end{align*}$$
 ${}^{-}$
’s” denote the closure in the profinite topology—of subgroups of
${}^{-}$
’s” denote the closure in the profinite topology—of subgroups of
 $\mathrm {Out}^{\mathrm {C}}(\Pi _{})$
(cf. Corollary 2.20(i)).
$\mathrm {Out}^{\mathrm {C}}(\Pi _{})$
(cf. Corollary 2.20(i)).
Proof. First, we verify assertion (i). The injectivity portion of assertion (i) follows from Corollary 2.20(i). The first equality follows from Corollary 2.20(ii). Thus, the second and third equalities follow immediately from the various definitions involved; the fourth equality follows from Corollary 2.20(v). This completes the proof of assertion (i).
Next, we verify assertion (ii). The inclusions
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +} \subseteq \mathrm {Out}^{\mathrm {C}}(T)^{\Delta }$
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +} \subseteq \mathrm {Out}^{\mathrm {C}}(T)^{\Delta }$
![]() $\subseteq \mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}}$
follow from assertion (i), together with [Reference Hoshi and Mochizuki10, Lem. 3.5]. The inclusion
$\subseteq \mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}}$
follow from assertion (i), together with [Reference Hoshi and Mochizuki10, Lem. 3.5]. The inclusion
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}} \subseteq \mathrm {Out}^{\mathrm {C}}(T)^{\Delta +}$
and the assertion that
$\mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}} \subseteq \mathrm {Out}^{\mathrm {C}}(T)^{\Delta +}$
and the assertion that
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}} \cong \mathbb {Z}/2\mathbb {Z}$
follow immediately from [Reference Mochizuki20, Cor. 5.3(i)], together with a classical result of Nielsen (cf. [Reference Mochizuki20, Rem. 5.3.1]). This completes the proof of the first line of the display of assertion (ii). Now since
$\mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}} \cong \mathbb {Z}/2\mathbb {Z}$
follow immediately from [Reference Mochizuki20, Cor. 5.3(i)], together with a classical result of Nielsen (cf. [Reference Mochizuki20, Rem. 5.3.1]). This completes the proof of the first line of the display of assertion (ii). Now since
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta } = \mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}}$
, by considering the action of
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta } = \mathrm {Out}^{\mathrm {C}}(T)^{\mathrm {cusp}}$
, by considering the action of
![]() $\mathrm {Out}^{\mathrm {C}}(T)$
on the set of the T-conjugacy classes of cuspidal inertia subgroups of T, we obtain an exact sequence
$\mathrm {Out}^{\mathrm {C}}(T)$
on the set of the T-conjugacy classes of cuspidal inertia subgroups of T, we obtain an exact sequence
By considering outomorphisms of T arising from automorphisms of analytic spaces, one obtains a section of this sequence; moreover, it follows from the definition of
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta }$
that this section determines an isomorphism
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta }$
that this section determines an isomorphism
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta } \times \mathfrak {S}_3 \stackrel {\sim }{\rightarrow } \mathrm {Out}^{\mathrm {C}}(T)$
. This completes the proof of assertion (ii).
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta } \times \mathfrak {S}_3 \stackrel {\sim }{\rightarrow } \mathrm {Out}^{\mathrm {C}}(T)$
. This completes the proof of assertion (ii).
Next, we verify assertion (iii). Recall that every finite index subgroup of T is normally terminal in its profinite completion (cf. Corollary 2.20(i)) and center-free (cf. Remark 2.6.1). Thus, assertion (iii) follows immediately from [Reference Hoshi and Mochizuki10, Th. 3.16(i)]. This completes the proof of assertion (iii).
Next, we verify assertion (iv). First, let us observe that it follows immediately from the definition of the notion of a central tripod (cf. Definition 2.23(iii); [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]) that we may assume without loss of generality that
![]() $n=3$
. To verify the equality of the first display of assertion (iv), we mimick the argument in the profinite case given in the proof of [Reference Mochizuki20, Cor. 1.10(i)]: let
$n=3$
. To verify the equality of the first display of assertion (iv), we mimick the argument in the profinite case given in the proof of [Reference Mochizuki20, Cor. 1.10(i)]: let
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
,
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
,
![]() $\widetilde \alpha \in \mathrm {Aut}(\Pi _{n}^{\mathrm {disc}})$
a lifting of
$\widetilde \alpha \in \mathrm {Aut}(\Pi _{n}^{\mathrm {disc}})$
a lifting of
![]() $\alpha $
. Write
$\alpha $
. Write
![]() $\widetilde \alpha _2 \in \mathrm {Aut}(\Pi _{2}^{\mathrm {disc}})$
for the automorphism induced by
$\widetilde \alpha _2 \in \mathrm {Aut}(\Pi _{2}^{\mathrm {disc}})$
for the automorphism induced by
![]() $\widetilde \alpha $
. Now observe that since
$\widetilde \alpha $
. Now observe that since
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
, it follows immediately from Corollary 2.20(iv) that
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})$
, it follows immediately from Corollary 2.20(iv) that
![]() $\widetilde \alpha _2$
determines an element of
$\widetilde \alpha _2$
determines an element of
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
, hence that
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
, hence that
![]() $\widetilde \alpha _2$
preserves the
$\widetilde \alpha _2$
preserves the
![]() $\Pi _{2}^{\mathrm {disc}}$
-conjugacy class of inertia groups associated with the diagonal cusp of any of the fibers of
$\Pi _{2}^{\mathrm {disc}}$
-conjugacy class of inertia groups associated with the diagonal cusp of any of the fibers of
![]() $p_{2/1}^{\mathrm {an}}$
(cf. Definition 2.22; the discussion of [Reference Mochizuki20, Rem. 1.1.5]). Thus, by replacing
$p_{2/1}^{\mathrm {an}}$
(cf. Definition 2.22; the discussion of [Reference Mochizuki20, Rem. 1.1.5]). Thus, by replacing
![]() $\widetilde \alpha $
by the composite of
$\widetilde \alpha $
by the composite of
![]() $\widetilde \alpha $
with a suitable inner automorphism, we may assume without loss of generality that
$\widetilde \alpha $
with a suitable inner automorphism, we may assume without loss of generality that
![]() $\widetilde \alpha _2$
preserves the inertia group associated with some diagonal cusp of a fiber of
$\widetilde \alpha _2$
preserves the inertia group associated with some diagonal cusp of a fiber of
![]() $p_{2/1}^{\mathrm {an}}$
. Now the fact that
$p_{2/1}^{\mathrm {an}}$
. Now the fact that
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})[T]$
follows immediately from Corollary 2.17(ii); [Reference Hoshi and Mochizuki10, Th. 1.9(ii)] (cf. the application of [Reference Mochizuki20, Prop. 1.3(iv)] in the proof of [Reference Mochizuki20, Cor. 1.10(i)]). The assertion that the restriction to
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}})[T]$
follows immediately from Corollary 2.17(ii); [Reference Hoshi and Mochizuki10, Th. 1.9(ii)] (cf. the application of [Reference Mochizuki20, Prop. 1.3(iv)] in the proof of [Reference Mochizuki20, Cor. 1.10(i)]). The assertion that the restriction to
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n}^{\mathrm {disc}})$
of the homomorphism
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n}^{\mathrm {disc}})$
of the homomorphism
![]() $\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}}) \rightarrow \mathrm {Out}(T)$
of assertion (iii) factors through
$\mathrm {Out}^{\mathrm {F}}(\Pi _{n}^{\mathrm {disc}}) \rightarrow \mathrm {Out}(T)$
of assertion (iii) factors through
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +} \subseteq \mathrm {Out}(T)$
follows immediately from from assertions (i) and (ii), together with [Reference Hoshi and Mochizuki10, Th. 3.16(v)]. The assertion that the resulting homomorphism is surjective follows immediately from the fact that the (unique) nontrivial element of
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +} \subseteq \mathrm {Out}(T)$
follows immediately from from assertions (i) and (ii), together with [Reference Hoshi and Mochizuki10, Th. 3.16(v)]. The assertion that the resulting homomorphism is surjective follows immediately from the fact that the (unique) nontrivial element of
![]() $\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +}$
is the outomorphism induced by complex conjugation (cf. [Reference Mochizuki20, Rem. 5.3.1]), together with the (easily verified) fact that the pointed stable curve over
$\mathrm {Out}^{\mathrm {C}}(T)^{\Delta +}$
is the outomorphism induced by complex conjugation (cf. [Reference Mochizuki20, Rem. 5.3.1]), together with the (easily verified) fact that the pointed stable curve over
![]() $\mathbb {C}$
corresponding to the given stable log curve
$\mathbb {C}$
corresponding to the given stable log curve
![]() $X^{\mathrm {log}}$
may be assumed, without loss of generality—that is, by applying a suitable specialization isomorphism (cf. the discussion preceding [Reference Mochizuki20, Def. 2.1] as well as [Reference Hoshi and Mochizuki9, Rem. 5.6.1]) and observing that such specialization isomorphisms are compatible with the various discrete fundamental groups involved (cf. Remarks 2.9.1 and 2.10.1)—to be defined over
$X^{\mathrm {log}}$
may be assumed, without loss of generality—that is, by applying a suitable specialization isomorphism (cf. the discussion preceding [Reference Mochizuki20, Def. 2.1] as well as [Reference Hoshi and Mochizuki9, Rem. 5.6.1]) and observing that such specialization isomorphisms are compatible with the various discrete fundamental groups involved (cf. Remarks 2.9.1 and 2.10.1)—to be defined over
![]() $\mathbb {R}$
. This completes the proof of assertion (iv).
$\mathbb {R}$
. This completes the proof of assertion (iv).
Next, we verify assertion (v). It follows immediately from the classification of E-strict tripods given in [Reference Hoshi and Mochizuki10, Lem. 3.8(ii)] that we may assume without loss of generality that
![]() $E^\sharp =n\leq 3$
. When
$E^\sharp =n\leq 3$
. When
![]() $n=3$
, assertion (v) follows formally from assertion (iv). When
$n=3$
, assertion (v) follows formally from assertion (iv). When
![]() $n=1$
, assertion (v) follows immediately from Corollary 2.17(ii). Thus, it remains to consider the case where
$n=1$
, assertion (v) follows immediately from Corollary 2.17(ii). Thus, it remains to consider the case where
![]() $n=2$
, that is, where the tripod T arises from an edge. In this case, assertion (v) follows from a similar argument to the argument applied in the proof of assertion (iv). That is to say, let
$n=2$
, that is, where the tripod T arises from an edge. In this case, assertion (v) follows from a similar argument to the argument applied in the proof of assertion (iv). That is to say, let
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2}^{\mathrm {disc}})$
,
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2}^{\mathrm {disc}})$
,
![]() $\widetilde \alpha \in \mathrm {Aut}(\Pi _{2}^{\mathrm {disc}})$
a lifting of
$\widetilde \alpha \in \mathrm {Aut}(\Pi _{2}^{\mathrm {disc}})$
a lifting of
![]() $\alpha $
. Write
$\alpha $
. Write
![]() $\widetilde \alpha _1\in \mathrm {Aut}(\Pi _{1}^{\mathrm {disc}})$
for the automorphism induced by
$\widetilde \alpha _1\in \mathrm {Aut}(\Pi _{1}^{\mathrm {disc}})$
for the automorphism induced by
![]() $\widetilde \alpha $
;
$\widetilde \alpha $
;
![]() $\widetilde \beta _1 \in \mathrm {Aut}(\Pi _{1})$
,
$\widetilde \beta _1 \in \mathrm {Aut}(\Pi _{1})$
,
![]() $\widetilde \beta \in \mathrm {Aut}(\Pi _{2})$
for the automorphisms determined by
$\widetilde \beta \in \mathrm {Aut}(\Pi _{2})$
for the automorphisms determined by
![]() $\widetilde \alpha $
. Then we must verify that
$\widetilde \alpha $
. Then we must verify that
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2}^{\mathrm {disc}})[T]$
under the assumption that
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2}^{\mathrm {disc}})[T]$
under the assumption that
![]() $\widetilde \beta $
determines an element
$\widetilde \beta $
determines an element
![]() $\beta \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2})[\widehat T]$
. Now observe that it follows immediately from the computation of the centralizer given in [Reference Hoshi and Mochizuki10, Lem. 3.11(vii)] that
$\beta \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2})[\widehat T]$
. Now observe that it follows immediately from the computation of the centralizer given in [Reference Hoshi and Mochizuki10, Lem. 3.11(vii)] that
![]() $\widetilde \beta _1$
preserves the
$\widetilde \beta _1$
preserves the
![]() $\Pi _{1}$
-conjugacy class of edge-like subgroups of
$\Pi _{1}$
-conjugacy class of edge-like subgroups of
![]() $\Pi _{1}$
determined by the edge that gives rise to the tripod T. Thus, we conclude from Corollary 2.17(ii) that, by replacing
$\Pi _{1}$
determined by the edge that gives rise to the tripod T. Thus, we conclude from Corollary 2.17(ii) that, by replacing
![]() $\widetilde \alpha $
by the composite of
$\widetilde \alpha $
by the composite of
![]() $\widetilde \alpha $
with a suitable inner automorphism, we may assume that
$\widetilde \alpha $
with a suitable inner automorphism, we may assume that
![]() $\widetilde \alpha _1$
preserves a specific edge-like subgroup of
$\widetilde \alpha _1$
preserves a specific edge-like subgroup of
![]() $\Pi _{1}^{\mathrm {disc}}$
corresponding to the edge that gives rise to the tripod T. Note that this assumption implies, in light of the commensurably terminality of edge-like subgroups (cf. [Reference Mochizuki18, Prop. 1.2(ii)]), that
$\Pi _{1}^{\mathrm {disc}}$
corresponding to the edge that gives rise to the tripod T. Note that this assumption implies, in light of the commensurably terminality of edge-like subgroups (cf. [Reference Mochizuki18, Prop. 1.2(ii)]), that
![]() $\widetilde \beta $
preserves the
$\widetilde \beta $
preserves the
![]() $\Pi _{2/1}$
-conjugacy class of the tripod
$\Pi _{2/1}$
-conjugacy class of the tripod
![]() $\widehat T$
. In particular, we conclude, as in the proof of assertion (iv), that is, by applying Corollary 2.17(ii), that
$\widehat T$
. In particular, we conclude, as in the proof of assertion (iv), that is, by applying Corollary 2.17(ii), that
![]() $\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2}^{\mathrm {disc}})[T]$
, as desired. This completes the proof of assertion (v).
$\alpha \in \mathrm {Out}^{\mathrm {F}}(\Pi _{2}^{\mathrm {disc}})[T]$
, as desired. This completes the proof of assertion (v).
Finally, we verify assertion (vi). First, let us observe that it follows immediately from Corollary 2.20(v) that both sides of the equality in question are
![]() $\subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{3}^{\mathrm {disc}})^{-} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
$\subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{3}^{\mathrm {disc}})^{-} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
![]() $(\subseteq \mathrm {Out}^{\mathrm {C}}(\Pi _{}))$
. Also, we observe that, by considering the case where
$(\subseteq \mathrm {Out}^{\mathrm {C}}(\Pi _{}))$
. Also, we observe that, by considering the case where
![]() $X^{\mathrm {log}}$
is defined over
$X^{\mathrm {log}}$
is defined over
![]() $\mathbb {R}$
(cf. the proof of assertion (iv)), it follows immediately that both sides of the equality in question surject, via the tripod homomorphism of assertion (iv), onto the finite group of order two that appears as the image of this tripod homomorphism (cf. also the fact that the topological group
$\mathbb {R}$
(cf. the proof of assertion (iv)), it follows immediately that both sides of the equality in question surject, via the tripod homomorphism of assertion (iv), onto the finite group of order two that appears as the image of this tripod homomorphism (cf. also the fact that the topological group
![]() $\mathrm {Out}(\widehat {T})$
is profinite, hence, in particular, Hausdorff). In particular, to complete the proof of assertion (v), it suffices to verify that the evident inclusion
$\mathrm {Out}(\widehat {T})$
is profinite, hence, in particular, Hausdorff). In particular, to complete the proof of assertion (v), it suffices to verify that the evident inclusion
—where we write
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
for the kernel of the tripod homomorphism on
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
for the kernel of the tripod homomorphism on
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19])—of subgroups of
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19])—of subgroups of
![]() $\mathrm {Out}^{\mathrm {C}}(\Pi _{})$
is, in fact, an equality. On the other hand, since
$\mathrm {Out}^{\mathrm {C}}(\Pi _{})$
is, in fact, an equality. On the other hand, since
![]() $\mathrm {Dehn}(\mathcal {G})$
is a normal open subgroup of both
$\mathrm {Dehn}(\mathcal {G})$
is a normal open subgroup of both
![]() $\mathrm {Aut}(\mathcal {G}^{\mathrm {disc}})^{-} \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
and
$\mathrm {Aut}(\mathcal {G}^{\mathrm {disc}})^{-} \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
and
![]() $\mathrm {Aut}(\mathcal {G}) \cap \mathrm {Out}^{\mathrm {C}}(\Pi _{}^{\mathrm {disc}})^{-} \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
(cf. Corollary 2.21(iii); [Reference Hoshi and Mochizuki9, Th. 4.8(i)]; the commutative diagram of [Reference Hoshi and Mochizuki10, Cor. 3.27(ii)]), and
$\mathrm {Aut}(\mathcal {G}) \cap \mathrm {Out}^{\mathrm {C}}(\Pi _{}^{\mathrm {disc}})^{-} \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
(cf. Corollary 2.21(iii); [Reference Hoshi and Mochizuki9, Th. 4.8(i)]; the commutative diagram of [Reference Hoshi and Mochizuki10, Cor. 3.27(ii)]), and
![]() $\mathrm {Aut}(\mathcal {G}^{\mathrm {disc}})^{-} \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
clearly surjects onto the finite group of automorphisms of the underlying semi-graph of
$\mathrm {Aut}(\mathcal {G}^{\mathrm {disc}})^{-} \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
clearly surjects onto the finite group of automorphisms of the underlying semi-graph of
![]() $\mathcal {G}^{\mathrm {disc}}$
, the desired equality follows immediately from [Reference Hoshi and Mochizuki10, Cor. 3.27(ii)]. This completes the proof of assertion (vi).
$\mathcal {G}^{\mathrm {disc}}$
, the desired equality follows immediately from [Reference Hoshi and Mochizuki10, Cor. 3.27(ii)]. This completes the proof of assertion (vi).
Remark 2.24.1. It is not clear to the authors at the time of writing whether or not one can remove the strictness assumption imposed in Theorem 2.24(v). Indeed, from the point of view of induction on n, the essential difficulty in removing this assumption may already be seen in the case of a non-E-strict tripod when
![]() $E^\sharp =n=2$
. From another point of view, this difficulty may be thought of as arising from the lack of an analogue for discrete topological fundamental groups of nth configuration spaces, when
$E^\sharp =n=2$
. From another point of view, this difficulty may be thought of as arising from the lack of an analogue for discrete topological fundamental groups of nth configuration spaces, when
![]() $n\geq 2$
, of Corollary 2.17.
$n\geq 2$
, of Corollary 2.17.
Remark 2.24.2.
-
(i) In the notation of Theorem 2.24, let us observe that it follows from Corollary 2.19(i) that we have an equality
of subgroups of $$ \begin{align*}\mathrm{Aut}(\mathcal{G}^{\mathrm{disc}}) \, = \, \mathrm{Aut}(\mathcal{G}) \, \cap \, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}}) \, \, \, \, (\subseteq \mathrm{Out}^{\mathrm{C}}(\Pi)) \end{align*} $$
$$ \begin{align*}\mathrm{Aut}(\mathcal{G}^{\mathrm{disc}}) \, = \, \mathrm{Aut}(\mathcal{G}) \, \cap \, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}}) \, \, \, \, (\subseteq \mathrm{Out}^{\mathrm{C}}(\Pi)) \end{align*} $$
 $\mathrm {Out}^{\mathrm {C}}(\Pi )$
(cf. Corollary 2.20(i)). On the other hand, it is by no means clear whether or not the evident inclusion (*)—where the superscript “
$\mathrm {Out}^{\mathrm {C}}(\Pi )$
(cf. Corollary 2.20(i)). On the other hand, it is by no means clear whether or not the evident inclusion (*)—where the superscript “ $$ \begin{align*}\hspace{24mm} \mathrm{Aut}(\mathcal{G}^{\mathrm{disc}})^{-} \, \subseteq \, \mathrm{Aut}(\mathcal{G})\, \cap\, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}})^{-} \, \, \, \, (\subseteq \mathrm{Out}^{\mathrm{C}}(\Pi))\end{align*} $$
$$ \begin{align*}\hspace{24mm} \mathrm{Aut}(\mathcal{G}^{\mathrm{disc}})^{-} \, \subseteq \, \mathrm{Aut}(\mathcal{G})\, \cap\, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}})^{-} \, \, \, \, (\subseteq \mathrm{Out}^{\mathrm{C}}(\Pi))\end{align*} $$
 ${}^{-}$
’s” denote the closure in the profinite topology—is an equality in general. On the other hand, when
${}^{-}$
’s” denote the closure in the profinite topology—is an equality in general. On the other hand, when
 $X^{\mathrm {log}}$
is totally degenerate, this equality is the content of Theorem 2.24(vi).
$X^{\mathrm {log}}$
is totally degenerate, this equality is the content of Theorem 2.24(vi).
-
(ii) We continue to use the notation of (i). Write
 $\mathcal {M}_{\mathbb {Q}}$
for the moduli stack of hyperbolic curves of type
$\mathcal {M}_{\mathbb {Q}}$
for the moduli stack of hyperbolic curves of type
 $(g,r)$
over
$(g,r)$
over
 $\mathbb {Q}$
and
$\mathbb {Q}$
and
 $\mathcal {C}_{\mathbb {Q}} \rightarrow \mathcal {M}_{\mathbb {Q}}$
for the tautological hyperbolic curve over
$\mathcal {C}_{\mathbb {Q}} \rightarrow \mathcal {M}_{\mathbb {Q}}$
for the tautological hyperbolic curve over
 $\mathcal {M}_{\mathbb {Q}}$
. Thus, for appropriate choices of basepoints, if we write
$\mathcal {M}_{\mathbb {Q}}$
. Thus, for appropriate choices of basepoints, if we write
 $\Pi _{\mathcal {C}} \stackrel {\mathrm {def}}{=} \pi _1(\mathcal {C}_{\mathbb {Q}})$
,
$\Pi _{\mathcal {C}} \stackrel {\mathrm {def}}{=} \pi _1(\mathcal {C}_{\mathbb {Q}})$
,
 $\Pi _{\mathcal {M}}\stackrel {\mathrm {def}}{=} \pi _1(\mathcal {M}_{\mathbb {Q}})$
for the respective étale fundamental groups, then we obtain an exact sequence of profinite groups
$\Pi _{\mathcal {M}}\stackrel {\mathrm {def}}{=} \pi _1(\mathcal {M}_{\mathbb {Q}})$
for the respective étale fundamental groups, then we obtain an exact sequence of profinite groups  $$\begin{align*}1 \longrightarrow \Delta_{\mathcal{C}/\mathcal{M}} \longrightarrow \Pi_{\mathcal{C}} \longrightarrow \Pi_{\mathcal{M}} \longrightarrow 1 \end{align*}$$
$$\begin{align*}1 \longrightarrow \Delta_{\mathcal{C}/\mathcal{M}} \longrightarrow \Pi_{\mathcal{C}} \longrightarrow \Pi_{\mathcal{M}} \longrightarrow 1 \end{align*}$$
—where
 $\Delta _{\mathcal {C}/\mathcal {M}}$
is defined so as to render the sequence exact—as well as a natural outer representation
$\Delta _{\mathcal {C}/\mathcal {M}}$
is defined so as to render the sequence exact—as well as a natural outer representation  $$ \begin{align*}\rho_{\mathcal{M}}\colon \Pi_{\mathcal{M}} \longrightarrow \mathrm{Out}^{\mathrm{C}}(\Pi) \end{align*} $$
$$ \begin{align*}\rho_{\mathcal{M}}\colon \Pi_{\mathcal{M}} \longrightarrow \mathrm{Out}^{\mathrm{C}}(\Pi) \end{align*} $$
—where, by choosing appropriate basepoints, we identify
 $\Pi $
with
$\Pi $
with
 $\Delta _{\mathcal {C}/\mathcal {M}}$
—and a natural outer surjection onto the absolute Galois group
$\Delta _{\mathcal {C}/\mathcal {M}}$
—and a natural outer surjection onto the absolute Galois group $$\begin{align*}\Pi_{\mathcal{M}} \twoheadrightarrow G_{\mathbb{Q}} \end{align*}$$
$$\begin{align*}\Pi_{\mathcal{M}} \twoheadrightarrow G_{\mathbb{Q}} \end{align*}$$
 $G_{\mathbb {Q}}$
of
$G_{\mathbb {Q}}$
of
 $\mathbb {Q}$
(cf. the discussion of [Reference Hoshi and Mochizuki10, Rem. 3.19.1]). Write
$\mathbb {Q}$
(cf. the discussion of [Reference Hoshi and Mochizuki10, Rem. 3.19.1]). Write
 $G_{\mathbb {R}} \subseteq G_{\mathbb {Q}}$
for the decomposition group (which is well-defined up to
$G_{\mathbb {R}} \subseteq G_{\mathbb {Q}}$
for the decomposition group (which is well-defined up to
 $G_{\mathbb {Q}}$
-conjugation) of the unique archimedean prime of
$G_{\mathbb {Q}}$
-conjugation) of the unique archimedean prime of
 $\mathbb {Q}$
. In the spirit of [Reference Boggi4]–[Reference Boggi6], let us write
$\mathbb {Q}$
. In the spirit of [Reference Boggi4]–[Reference Boggi6], let us write  $$ \begin{align*}\Gamma \, \, \stackrel{\mathrm{def}}{=} \, \, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}}) \, \, \, \, (\subseteq \mathrm{Out}^{\mathrm{C}}(\Pi)); \, \, \, \, \, {\check \Gamma} \, \, \stackrel{\mathrm{def}}{=} \, \, \rho_{\mathcal{M}} (\Pi_{\mathcal{M}}\times_{G_{\mathbb{Q}}} G_{\mathbb{R}}) \end{align*} $$
$$ \begin{align*}\Gamma \, \, \stackrel{\mathrm{def}}{=} \, \, \mathrm{Out}^{\mathrm{C}}(\Pi_{}^{\mathrm{disc}}) \, \, \, \, (\subseteq \mathrm{Out}^{\mathrm{C}}(\Pi)); \, \, \, \, \, {\check \Gamma} \, \, \stackrel{\mathrm{def}}{=} \, \, \rho_{\mathcal{M}} (\Pi_{\mathcal{M}}\times_{G_{\mathbb{Q}}} G_{\mathbb{R}}) \end{align*} $$
(cf. Corollary 2.20(i)). Thus, for appropriate choices of basepoints,
 ${\check \Gamma }$
is equal to the closure of
${\check \Gamma }$
is equal to the closure of
 $\Gamma $
in
$\Gamma $
in
 $\mathrm {Out}^{\mathrm {C}}(\Pi )$
. If
$\mathrm {Out}^{\mathrm {C}}(\Pi )$
. If
 $\sigma $
is a simplex of the complex of profinite curves
$\sigma $
is a simplex of the complex of profinite curves
 $L(\Pi )$
studied in [Reference Boggi4]–[Reference Boggi6] that arises from
$L(\Pi )$
studied in [Reference Boggi4]–[Reference Boggi6] that arises from
 $\Pi _{}^{\mathrm {disc}}$
, then the stabilizer in
$\Pi _{}^{\mathrm {disc}}$
, then the stabilizer in
 $\Gamma $
of
$\Gamma $
of
 $\sigma $
is denoted
$\sigma $
is denoted
 $\Gamma _\sigma $
, while the stabilizer in
$\Gamma _\sigma $
, while the stabilizer in
 ${\check \Gamma }$
of the image of
${\check \Gamma }$
of the image of
 $\sigma $
in the profinite curve complex corresponding to
$\sigma $
in the profinite curve complex corresponding to
 ${\check \Gamma }$
is denoted
${\check \Gamma }$
is denoted
 ${\check \Gamma }_\sigma $
. Then [Reference Boggi6, Th. 4.2] (cf. also [Reference Boggi4, Prop. 6.5]) asserts thatTranslated into the language of the present paper, this assertion corresponds precisely to the assertion that the inclusion
${\check \Gamma }_\sigma $
. Then [Reference Boggi6, Th. 4.2] (cf. also [Reference Boggi4, Prop. 6.5]) asserts thatTranslated into the language of the present paper, this assertion corresponds precisely to the assertion that the inclusionThe natural inclusion
 $\Gamma _\sigma ^{-} \subseteq {\check \Gamma }_\sigma $
is, in fact, an equality.
$\Gamma _\sigma ^{-} \subseteq {\check \Gamma }_\sigma $
is, in fact, an equality. $(*)$
considered in (i) is, in fact, an equality. In particular, Theorem 2.24(vi) corresponds, essentially, to a special case (i.e., the totally degenerate case) of [Reference Boggi6, Th. 4.2]. At a more concrete level, when
$(*)$
considered in (i) is, in fact, an equality. In particular, Theorem 2.24(vi) corresponds, essentially, to a special case (i.e., the totally degenerate case) of [Reference Boggi6, Th. 4.2]. At a more concrete level, when
 $\mathrm {Node}(\mathcal {G})^{\sharp }=1$
, and
$\mathrm {Node}(\mathcal {G})^{\sharp }=1$
, and
 $\sigma $
arises from a single simple closed curve that corresponds to the unique node e of
$\sigma $
arises from a single simple closed curve that corresponds to the unique node e of
 $\mathcal {G}$
, this assertion corresponds precisely to the assertion that—cf. Theorem 3.3, Remark 3.3.1, and Corollary 3.4 in §3 below. As discussed in (i), this sort of assertion is highly nontrivial. That is to say, this sort of coincidence between a profinite stabilizer and the closure of a corresponding discrete stabilizer is, in fact, false in general, as the example given in (iv) below demonstrates. In particular, this sort of coincidence is by no means a consequence of superficial “general nonsense”-type considerations, but rather, when true (cf., e.g., the case treated in Theorem 2.24(vi)), a consequence of deep properties of the specific groups and specific spaces (on which these groups act) under consideration.
$\mathcal {G}$
, this assertion corresponds precisely to the assertion that—cf. Theorem 3.3, Remark 3.3.1, and Corollary 3.4 in §3 below. As discussed in (i), this sort of assertion is highly nontrivial. That is to say, this sort of coincidence between a profinite stabilizer and the closure of a corresponding discrete stabilizer is, in fact, false in general, as the example given in (iv) below demonstrates. In particular, this sort of coincidence is by no means a consequence of superficial “general nonsense”-type considerations, but rather, when true (cf., e.g., the case treated in Theorem 2.24(vi)), a consequence of deep properties of the specific groups and specific spaces (on which these groups act) under consideration.the profinite stabilizer in
 ${\check \Gamma }$
of the
${\check \Gamma }$
of the
 $\Pi $
-conjugacy class of nodal subgroups of
$\Pi $
-conjugacy class of nodal subgroups of
 $\Pi $
determined by e coincides with the closure in
$\Pi $
determined by e coincides with the closure in
 ${\check \Gamma }$
of the discrete stabilizer in
${\check \Gamma }$
of the discrete stabilizer in
 $\Gamma $
of the
$\Gamma $
of the
 $\Pi _{}^{\mathrm {disc}}$
-conjugacy class of nodal subgroups of
$\Pi _{}^{\mathrm {disc}}$
-conjugacy class of nodal subgroups of
 $\Pi _{}^{\mathrm {disc}}$
determined by e
$\Pi _{}^{\mathrm {disc}}$
determined by e -
(iii) In closing, we observe that many of the results derived in [Reference Boggi6] as a consequence of the assertion discussed in (ii) were, in fact, already obtained in earlier papers by the authors. Indeed, the faithfulness asserted in [Reference Boggi6, Th. 7.7]—that is, the injectivity of the restriction of
 $\rho _{\mathcal {M}}$
to a section
$\rho _{\mathcal {M}}$
to a section
 $G_F\hookrightarrow \Pi _{\mathcal {M}}$
arising from a hyperbolic curve of type
$G_F\hookrightarrow \Pi _{\mathcal {M}}$
arising from a hyperbolic curve of type
 $(g,r)$
defined over a number field F—is a special case of [Reference Hoshi and Mochizuki8, Th. C]. On the other hand, in [Reference Hoshi and Mochizuki9, Th. D], a computation is given of the centralizer in
$(g,r)$
defined over a number field F—is a special case of [Reference Hoshi and Mochizuki8, Th. C]. On the other hand, in [Reference Hoshi and Mochizuki9, Th. D], a computation is given of the centralizer in
 $\mathrm {Out}^{\mathrm {C}}(\Pi )$
of an open subgroup of
$\mathrm {Out}^{\mathrm {C}}(\Pi )$
of an open subgroup of
 ${\check \Gamma }$
. Thus, the computation of centers given in [Reference Boggi6, Cor. 6.2] amounts to a special case of [Reference Hoshi and Mochizuki9, Th. D]. Finally, [Reference Boggi6, Cor. 7.6]—which may be regarded as the assertion that the inverse image via
${\check \Gamma }$
. Thus, the computation of centers given in [Reference Boggi6, Cor. 6.2] amounts to a special case of [Reference Hoshi and Mochizuki9, Th. D]. Finally, [Reference Boggi6, Cor. 7.6]—which may be regarded as the assertion that the inverse image via
 $\rho _{\mathcal {M}}$
of the centralizer of
$\rho _{\mathcal {M}}$
of the centralizer of
 ${\check \Gamma }$
in
${\check \Gamma }$
in
 $\mathrm {Out}^{\mathrm {C}}(\Pi )$
maps trivially to
$\mathrm {Out}^{\mathrm {C}}(\Pi )$
maps trivially to
 $G_{\mathbb {Q}}$
—amounts to a concatenation of
$G_{\mathbb {Q}}$
—amounts to a concatenation of-
• the computation of the centralizer given in [Reference Hoshi and Mochizuki9, Th. D] with
-
• the fact, stated in [Reference Hoshi and Mochizuki8, Cor. 6.4] that
 $\rho _{\mathcal {M}}^{-1}({\check \Gamma })$
maps trivially to
$\rho _{\mathcal {M}}^{-1}({\check \Gamma })$
maps trivially to
 $G_{\mathbb {Q}}$
.
$G_{\mathbb {Q}}$
.
-
-
(iv) Let
 $n\geq 3$
be an integer. Consider the natural conjugation action of the special linear group
$n\geq 3$
be an integer. Consider the natural conjugation action of the special linear group
 $\mathrm {SL}_n(\mathbb {Z})$
with coefficients
$\mathrm {SL}_n(\mathbb {Z})$
with coefficients
 $\in \mathbb {Z}$
on the module
$\in \mathbb {Z}$
on the module
 $\mathrm {M}_n(\mathbb {Z})$
of n by n matrices with coefficients
$\mathrm {M}_n(\mathbb {Z})$
of n by n matrices with coefficients
 $\in \mathbb {Z}$
. Write
$\in \mathbb {Z}$
. Write
 $A\in \mathrm {M}_n(\mathbb {Z})$
for the diagonal matrix whose entries are given by the integers
$A\in \mathrm {M}_n(\mathbb {Z})$
for the diagonal matrix whose entries are given by the integers
 $1 , \dots , n$
. Then one verifies immediately that the stabilizer of A, relative to the conjugacy action of
$1 , \dots , n$
. Then one verifies immediately that the stabilizer of A, relative to the conjugacy action of $$\begin{align*}\mathrm{SL}_n(\mathbb{Z})_A \end{align*}$$
$$\begin{align*}\mathrm{SL}_n(\mathbb{Z})_A \end{align*}$$
 $\mathrm {SL}_n(\mathbb {Z})$
, is equal to the subgroup of diagonal matrices of
$\mathrm {SL}_n(\mathbb {Z})$
, is equal to the subgroup of diagonal matrices of
 $\mathrm {SL}_n(\mathbb {Z})$
, hence isomorphic to the finite group given by a product of
$\mathrm {SL}_n(\mathbb {Z})$
, hence isomorphic to the finite group given by a product of
 $n-1$
copies of the finite group of order two
$n-1$
copies of the finite group of order two
 $\{\pm 1\}$
. On the other hand, if one considers the action of the special linear group
$\{\pm 1\}$
. On the other hand, if one considers the action of the special linear group
 $\mathrm {SL}_n(\widehat {\mathbb {Z}})$
with coefficients
$\mathrm {SL}_n(\widehat {\mathbb {Z}})$
with coefficients
 $\in \widehat {\mathbb {Z}}$
on the module
$\in \widehat {\mathbb {Z}}$
on the module
 $\mathrm {M}_n(\widehat {\mathbb {Z}})$
of n by n matrices with coefficients
$\mathrm {M}_n(\widehat {\mathbb {Z}})$
of n by n matrices with coefficients
 $\in \widehat {\mathbb {Z}}$
, then one verifies immediately that the stabilizer of A, relative to the conjugacy action of
$\in \widehat {\mathbb {Z}}$
, then one verifies immediately that the stabilizer of A, relative to the conjugacy action of $$\begin{align*}\mathrm{SL}_n(\widehat{\mathbb{Z}})_A \end{align*}$$
$$\begin{align*}\mathrm{SL}_n(\widehat{\mathbb{Z}})_A \end{align*}$$
 $\mathrm {SL}_n(\widehat {\mathbb {Z}})$
, is equal to the subgroup of diagonal matrices of
$\mathrm {SL}_n(\widehat {\mathbb {Z}})$
, is equal to the subgroup of diagonal matrices of
 $\mathrm {SL}_n(\widehat {\mathbb {Z}})$
, hence isomorphic to a product of
$\mathrm {SL}_n(\widehat {\mathbb {Z}})$
, hence isomorphic to a product of
 $n-1$
copies of
$n-1$
copies of
 $\widehat {\mathbb {Z}}^\times $
, a group of uncountable cardinality. That is to say,Here, we recall that since, as is well-known, the congruence subgroup problem has been resolved in the affirmative, in the case of
$\widehat {\mathbb {Z}}^\times $
, a group of uncountable cardinality. That is to say,Here, we recall that since, as is well-known, the congruence subgroup problem has been resolved in the affirmative, in the case ofThe profinite stabilizer
 $\mathrm {SL}_n(\widehat {\mathbb {Z}})_A$
is much larger than the profinite completion of the discrete stabilizer
$\mathrm {SL}_n(\widehat {\mathbb {Z}})_A$
is much larger than the profinite completion of the discrete stabilizer
 $\mathrm {SL}_n(\mathbb {Z})_A$
.
$\mathrm {SL}_n(\mathbb {Z})_A$
. $n\geq 3$
, the topological group
$n\geq 3$
, the topological group
 $\mathrm {SL}_n(\widehat {\mathbb {Z}})$
may be identified with the profinite completion of the group
$\mathrm {SL}_n(\widehat {\mathbb {Z}})$
may be identified with the profinite completion of the group
 $\mathrm {SL}_n(\mathbb {Z})$
. A similar example may be given in the case of the symplectic group
$\mathrm {SL}_n(\mathbb {Z})$
. A similar example may be given in the case of the symplectic group
 $\mathrm {Sp}_{2n}(\mathbb {Z})$
.
$\mathrm {Sp}_{2n}(\mathbb {Z})$
.
Corollary 2.25 (Characterization of the archimedean local Galois groups in the global Galois image associated with a hyperbolic curve).
Let F be a number field (i.e., a finite extension of the field of rational numbers);
![]() $\mathfrak {p}$
an archimedean prime of F;
$\mathfrak {p}$
an archimedean prime of F;
![]() $\overline F_{\mathfrak {p}}$
an algebraic closure of the
$\overline F_{\mathfrak {p}}$
an algebraic closure of the
![]() $\mathfrak {p}$
-adic completion
$\mathfrak {p}$
-adic completion
![]() $F_{\mathfrak {p}}$
of F (so
$F_{\mathfrak {p}}$
of F (so
![]() $\overline F_{\mathfrak {p}}$
is isomorphic to
$\overline F_{\mathfrak {p}}$
is isomorphic to
![]() $\mathbb {C}$
);
$\mathbb {C}$
);
![]() $\overline F \subseteq \overline F_{\mathfrak {p}}$
the algebraic closure of F in
$\overline F \subseteq \overline F_{\mathfrak {p}}$
the algebraic closure of F in
![]() $\overline F_{\mathfrak {p}}$
;
$\overline F_{\mathfrak {p}}$
;
![]() $X_F^{\mathrm {log}}$
a smooth log curve over F. Write
$X_F^{\mathrm {log}}$
a smooth log curve over F. Write
![]() $G_{\mathfrak {p}} \stackrel {\mathrm {def}}{=} \mathrm {Gal}(\overline F_{\mathfrak {p}} / F_{\mathfrak {p}}) \subseteq G_F \stackrel {\mathrm {def}}{=} \mathrm {Gal}(\overline F / F)$
;
$G_{\mathfrak {p}} \stackrel {\mathrm {def}}{=} \mathrm {Gal}(\overline F_{\mathfrak {p}} / F_{\mathfrak {p}}) \subseteq G_F \stackrel {\mathrm {def}}{=} \mathrm {Gal}(\overline F / F)$
;
![]() $X^{\mathrm {log}}_{\overline F} \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_F \times _F \overline F$
;
$X^{\mathrm {log}}_{\overline F} \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_F \times _F \overline F$
;
![]() $X^{\mathrm {log}}_{F_{\mathfrak {p}}} \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_F \times _F F_{\mathfrak {p}}$
;
$X^{\mathrm {log}}_{F_{\mathfrak {p}}} \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_F \times _F F_{\mathfrak {p}}$
;
![]() $X^{\mathrm {log}}_{\overline F_{\mathfrak {p}}} \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_F \times _F \overline F_{\mathfrak {p}}$
;
$X^{\mathrm {log}}_{\overline F_{\mathfrak {p}}} \stackrel {\mathrm {def}}{=} X^{\mathrm {log}}_F \times _F \overline F_{\mathfrak {p}}$
;
for the log fundamental group of
![]() $X^{\mathrm {log}}_{\overline F}$
;
$X^{\mathrm {log}}_{\overline F}$
;
for the (discrete) topological fundamental group of the analytic space associated with the interior of the log scheme
![]() $X^{\mathrm {log}}_{\overline F_{\mathfrak {p}}} $
;
$X^{\mathrm {log}}_{\overline F_{\mathfrak {p}}} $
;
for the profinite completion of
![]() $\pi _1^{\mathrm {disc}} ( X^{\mathrm {log}}_{\overline F_{\mathfrak {p}}} )$
;
$\pi _1^{\mathrm {disc}} ( X^{\mathrm {log}}_{\overline F_{\mathfrak {p}}} )$
;
for the natural outer Galois action associated with
![]() $X^{\mathrm {log}}_{F}$
;
$X^{\mathrm {log}}_{F}$
;
for the natural outer Galois action associated with
![]() $X_{F_{\mathfrak {p}}}^{\mathrm {log}}$
. Thus, we have a natural outer isomorphism
$X_{F_{\mathfrak {p}}}^{\mathrm {log}}$
. Thus, we have a natural outer isomorphism
which determines a natural injection
(cf. Corollary 2.20(i)). Then the following hold:
-
(i) We have a natural commutative diagram
—where the vertical arrows are the natural inclusions, and all arrows are injective.
-
(ii) The diagram of (i) is Cartesian, that is, if we regard the various groups involved as subgroups of
 $ \mathrm {Out} ( \pi _1 ( X^{\mathrm {log}}_{\overline F} ) )$
, then we have an equality
$ \mathrm {Out} ( \pi _1 ( X^{\mathrm {log}}_{\overline F} ) )$
, then we have an equality  $$\begin{align*}G_{\mathfrak{p}} = G_F \cap \mathrm{Out} ( \pi_1^{\mathrm{disc}} ( X^{\mathrm{log}}_{\overline F_{\mathfrak{p}}} ) ). \end{align*}$$
$$\begin{align*}G_{\mathfrak{p}} = G_F \cap \mathrm{Out} ( \pi_1^{\mathrm{disc}} ( X^{\mathrm{log}}_{\overline F_{\mathfrak{p}}} ) ). \end{align*}$$
Proof. Assertion (i) follows immediately from the injectivity of the lower horizontal arrow
![]() $\rho _{X_F^{\mathrm {log}}}$
(cf. [Reference Hoshi and Mochizuki8, Th. C]), together with the various definitions involved.
$\rho _{X_F^{\mathrm {log}}}$
(cf. [Reference Hoshi and Mochizuki8, Th. C]), together with the various definitions involved.
Finally, we verify assertion (ii). Write
![]() $(X_{\overline F})_3^{\mathrm {log}}$
for the
$(X_{\overline F})_3^{\mathrm {log}}$
for the
![]() $3$
-rd log configuration space of
$3$
-rd log configuration space of
![]() $X_{\overline F}^{\mathrm {log}}$
. Then it follows immediately from [Reference Hoshi and Mochizuki8, Th. B] that the group
$X_{\overline F}^{\mathrm {log}}$
. Then it follows immediately from [Reference Hoshi and Mochizuki8, Th. B] that the group
![]() $\mathrm {Out} ^{\mathrm {FC}} ( \pi _1 ( (X_{\overline F})_3^{\mathrm {log}} ) )$
of FC-admissible outomorphisms of the log fundamental group
$\mathrm {Out} ^{\mathrm {FC}} ( \pi _1 ( (X_{\overline F})_3^{\mathrm {log}} ) )$
of FC-admissible outomorphisms of the log fundamental group
![]() $\pi _1 ( (X_{\overline F})_3^{\mathrm {log}} )$
of
$\pi _1 ( (X_{\overline F})_3^{\mathrm {log}} )$
of
![]() $(X_{\overline F})_3^{\mathrm {log}}$
may be regarded as a closed subgroup of
$(X_{\overline F})_3^{\mathrm {log}}$
may be regarded as a closed subgroup of
![]() $ \mathrm {Out} ( \pi _1 ( X^{\mathrm {log}}_{\overline F} ) )$
. Moreover, it follows immediately from the various definitions involved that the respective images
$ \mathrm {Out} ( \pi _1 ( X^{\mathrm {log}}_{\overline F} ) )$
. Moreover, it follows immediately from the various definitions involved that the respective images
![]() $\mathrm {Im} ( \rho _{X^{\mathrm {log}}_F} )$
,
$\mathrm {Im} ( \rho _{X^{\mathrm {log}}_F} )$
,
![]() $\mathrm {Im} (\rho ^{\mathrm {disc}} _{X_F^{\mathrm {log}}, \mathfrak {p}}) $
of the natural outer Galois actions
$\mathrm {Im} (\rho ^{\mathrm {disc}} _{X_F^{\mathrm {log}}, \mathfrak {p}}) $
of the natural outer Galois actions
![]() $\rho _{X^{\mathrm {log}}_F}$
,
$\rho _{X^{\mathrm {log}}_F}$
,
![]() $\rho ^{\mathrm {disc}} _{X_F^{\mathrm {log}}, \mathfrak {p}}$
associated with
$\rho ^{\mathrm {disc}} _{X_F^{\mathrm {log}}, \mathfrak {p}}$
associated with
![]() $ X^{\mathrm {log}}_{F} $
,
$ X^{\mathrm {log}}_{F} $
,
![]() $X_{F_{\mathfrak {p}}}^{\mathrm {log}} $
are contained in this closed subgroup
$X_{F_{\mathfrak {p}}}^{\mathrm {log}} $
are contained in this closed subgroup
![]() $\mathrm {Out} ^{\mathrm {FC}} ( \pi _1 ( (X_{\overline F})_3^{\mathrm {log}} ) ) \subseteq \mathrm {Out} ( \pi _1 ( X^{\mathrm {log}}_{\overline F} ) )$
. Thus, to verify assertion (ii), one verifies immediately from Corollary 2.20(v) that it suffices to verify the equality
$\mathrm {Out} ^{\mathrm {FC}} ( \pi _1 ( (X_{\overline F})_3^{\mathrm {log}} ) ) \subseteq \mathrm {Out} ( \pi _1 ( X^{\mathrm {log}}_{\overline F} ) )$
. Thus, to verify assertion (ii), one verifies immediately from Corollary 2.20(v) that it suffices to verify the equality
—where we write
![]() $ (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} \stackrel {\mathrm {def}}{=} (X_{\overline F})_3^{\mathrm {log}} \times _{\overline F} \overline F_{\mathfrak {p}}$
and
$ (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} \stackrel {\mathrm {def}}{=} (X_{\overline F})_3^{\mathrm {log}} \times _{\overline F} \overline F_{\mathfrak {p}}$
and
![]() $\pi _1^{\mathrm {disc}} ( (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} )$
for the (discrete) topological fundamental group of the analytic space associated with the interior of the log scheme
$\pi _1^{\mathrm {disc}} ( (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} )$
for the (discrete) topological fundamental group of the analytic space associated with the interior of the log scheme
![]() $(X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}}$
. On the other hand, since the “
$(X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}}$
. On the other hand, since the “
![]() $\rho _{X^{\mathrm {log}}_F}$
” that occurs in the case where we take “
$\rho _{X^{\mathrm {log}}_F}$
” that occurs in the case where we take “
![]() $X_F^{\mathrm {log}}$
” to be the smooth log curve associated with
$X_F^{\mathrm {log}}$
” to be the smooth log curve associated with
![]() $\mathbb {P}_F^1\setminus \{0,1,\infty \}$
is injective (cf. assertion (i)), this equality follows immediately—by considering the images of the subgroups
$\mathbb {P}_F^1\setminus \{0,1,\infty \}$
is injective (cf. assertion (i)), this equality follows immediately—by considering the images of the subgroups
of
![]() $\mathrm {Out} ( \pi _1^{\mathrm {disc}} ( (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} ) ) $
via the (manifestly compatible!) tripod homomorphisms associated with
$\mathrm {Out} ( \pi _1^{\mathrm {disc}} ( (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} ) ) $
via the (manifestly compatible!) tripod homomorphisms associated with
![]() $\pi _1^{\mathrm {disc}} ( (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} )$
(cf. Theorem 2.24(iv)) and
$\pi _1^{\mathrm {disc}} ( (X_{\overline F_{\mathfrak {p}}})_3^{\mathrm {log}} )$
(cf. Theorem 2.24(iv)) and
![]() $\pi _1((X_{\overline F})_3^{\mathrm {log}})$
(cf. [Reference Hoshi and Mochizuki10, Th. 3.16(i) and (v)])—from [Reference André1, Th. 3.3.1]. This completes the proof of assertion (ii), hence also of Corollary 2.25.
$\pi _1((X_{\overline F})_3^{\mathrm {log}})$
(cf. [Reference Hoshi and Mochizuki10, Th. 3.16(i) and (v)])—from [Reference André1, Th. 3.3.1]. This completes the proof of assertion (ii), hence also of Corollary 2.25.
Remark 2.25.1. Corollary 2.25 is a generalization of [Reference André1, Th. 3.3.2] (cf. also the footnote of [Reference André1] following [Reference André1, Th. 3.3.2]). Although the proof given here of Corollary 2.25 is by no means the first proof of this result (cf. the discussion of this footnote of [Reference André1] following [Reference André1, Th. 3.3.2]; [Reference Hoshi and Mochizuki8, Cor. 6.4]), it is of interest to note that this result may also be derived in the context of the theory of the present paper, that is, via an argument that parallels the proof given in [Reference Hoshi and Mochizuki11] of [Reference Hoshi and Mochizuki11, Th. B] in the p-adic case (for which no alternative proofs are known!).
3 Canonical liftings of cycles
In the present section, we discuss certain canonical liftings of cycles (cf. Theorems 3.10 and 3.14 below). These canonical liftings are constructed in a fashion illustrated in Figure 1. This approach to constructing such canonical liftings was motivated (cf. Remark 3.10.1 below) by the arguments of [Reference Boggi5], where these canonical liftings were applied, in the context of the congruence subgroup problem for hyperelliptic modular groups, to derive certain injectivity results (cf. [Reference Boggi5, §2]), which may be regarded as special cases of [Reference Hoshi and Mochizuki8, Th. B]. Unfortunately, however, the authors of the present paper were unable to follow in detail these arguments of [Reference Boggi5], which appear to be based to a substantial extent on geometric intuition concerning the geometry of topological surfaces. Although, in the development of the present series of papers on combinatorial anabelian geometry, the authors were motivated by similar geometric intuition, the proofs of the results given in the present series of papers proceed by means of purely combinatorial and algebraic arguments concerning combinatorial (e.g., graphs) and group-theoretic (e.g., profinite fundamental groups) data that arise from a pointed stable curve. From the point of view of arithmetic geometry, the geometric intuition which underlies the topological arguments given in [Reference Boggi5] involving objects such as topological Dehn twists is of an essentially archimedean nature, hence, in particular, is fundamentally incompatible, at least from the point of view of establishing a rigorous mathematical formulation, with the highly nonarchimedean properties of profinite fundamental groups, as studied in the present series of papers—cf. the discussion of [Reference Mochizuki17, Rem. 1.5.1]. It was this state of affairs that motivated the authors to give, in the present section, a formulation of the constructions of [Reference Boggi5, §2] in terms of the purely combinatorial and algebraic techniques developed in the present series of papers.
In the present section, let
![]() $(g,r)$
be a pair of nonnegative integers such that
$(g,r)$
be a pair of nonnegative integers such that
![]() $2g-2+r> 0$
; n a positive integer;
$2g-2+r> 0$
; n a positive integer;
![]() $\Sigma $
a set of prime numbers which is either equal to the entire set of prime numbers or of cardinality one; k an algebraically closed field of characteristic
$\Sigma $
a set of prime numbers which is either equal to the entire set of prime numbers or of cardinality one; k an algebraically closed field of characteristic
![]() $\not \in \Sigma $
;
$\not \in \Sigma $
;
![]() $S^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)^{\mathrm {log}}$
the log scheme obtained by equipping
$S^{\mathrm {log}} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)^{\mathrm {log}}$
the log scheme obtained by equipping
![]() $S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)$
with the log structure determined by the fs chart
$S \stackrel {\mathrm {def}}{=} \mathrm {Spec}(k)$
with the log structure determined by the fs chart
![]() $\mathbb {N} \rightarrow k$
that maps
$\mathbb {N} \rightarrow k$
that maps
![]() $1 \mapsto 0$
;
$1 \mapsto 0$
;
![]() $X^{\mathrm {log}} = X_1^{\mathrm {log}}$
a stable log curve of type
$X^{\mathrm {log}} = X_1^{\mathrm {log}}$
a stable log curve of type
![]() $(g,r)$
over
$(g,r)$
over
![]() $S^{\mathrm {log}}$
. For each (possibly empty) subset
$S^{\mathrm {log}}$
. For each (possibly empty) subset
![]() $E\subseteq \{1 , \dots , n\}$
, write
$E\subseteq \{1 , \dots , n\}$
, write
for the
![]() $E^\sharp $
-th log configuration space of the stable log curve
$E^\sharp $
-th log configuration space of the stable log curve
![]() $X^{\mathrm {log}}$
(cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]), where we think of the factors as being labeled by the elements of
$X^{\mathrm {log}}$
(cf. the discussion entitled “Curves” in [Reference Hoshi and Mochizuki9, §0]), where we think of the factors as being labeled by the elements of
![]() $E \subseteq \{1 , \dots , n\}$
;
$E \subseteq \{1 , \dots , n\}$
;
for the maximal pro-
![]() $\Sigma $
quotient of the kernel of the natural surjection
$\Sigma $
quotient of the kernel of the natural surjection
![]() $\pi _1(X_E^{\mathrm {log}})\twoheadrightarrow \pi _1(S^{\mathrm {log}})$
;
$\pi _1(X_E^{\mathrm {log}})\twoheadrightarrow \pi _1(S^{\mathrm {log}})$
;
for the objects defined in the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3]; [Reference Hoshi and Mochizuki10, Def. 3.1]. In addition, we suppose that we have been given a pair of nonnegative integers
![]() $({}^{Y}\!g,{}^{Y}\!r)$
such that
$({}^{Y}\!g,{}^{Y}\!r)$
such that
![]() $2{}^{Y}\!g-2+{}^{Y}\!r> 0$
and a stable log curve
$2{}^{Y}\!g-2+{}^{Y}\!r> 0$
and a stable log curve
![]() $Y^{\mathrm {log}} = Y_1^{\mathrm {log}}$
of type
$Y^{\mathrm {log}} = Y_1^{\mathrm {log}}$
of type
![]() $({}^{Y}\!g,{}^{Y}\!r)$
over
$({}^{Y}\!g,{}^{Y}\!r)$
over
![]() $S^{\mathrm {log}}$
. We shall use similar notation
$S^{\mathrm {log}}$
. We shall use similar notation
for objects associated with the stable log curve
![]() $Y^{\mathrm {log}} = Y_1^{\mathrm {log}}$
to the notation introduced above for
$Y^{\mathrm {log}} = Y_1^{\mathrm {log}}$
to the notation introduced above for
![]() $X^{\mathrm {log}}$
(cf. the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3]]; [Reference Hoshi and Mochizuki10, Def. 3.1]).
$X^{\mathrm {log}}$
(cf. the discussion at the beginning of [Reference Hoshi and Mochizuki10, §3]]; [Reference Hoshi and Mochizuki10, Def. 3.1]).
Lemma 3.1 (Graphicity in the case of a single node).
In the notation of the discussion at the beginning of the present §3, suppose that
![]() $\mathrm {Node}(\mathcal {G})^\sharp =\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
. Write
$\mathrm {Node}(\mathcal {G})^\sharp =\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
. Write
for the unique node of
![]() $\mathcal {G}$
(resp.
$\mathcal {G}$
(resp.
![]() ${}^{Y}\!\mathcal {G}$
). Let
${}^{Y}\!\mathcal {G}$
). Let
![]() $\Pi _{e} \subseteq \Pi _{ 1}$
(resp.
$\Pi _{e} \subseteq \Pi _{ 1}$
(resp.
![]() $\Pi _{{}^{Y}\!e} \subseteq {}^{Y}\!\Pi _{ 1}$
) be a nodal subgroup of
$\Pi _{{}^{Y}\!e} \subseteq {}^{Y}\!\Pi _{ 1}$
) be a nodal subgroup of
![]() $\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
(resp.
$\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
(resp.
![]() $ {}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!\mathcal {G}}$
) associated with
$ {}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!\mathcal {G}}$
) associated with
![]() $e \in \mathrm {Node}(\mathcal {G})$
(resp.
$e \in \mathrm {Node}(\mathcal {G})$
(resp.
![]() ${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
);
${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
);
![]() $e_2\in X_2(k)$
(resp.
$e_2\in X_2(k)$
(resp.
![]() ${}^{Y}\!e_2\in Y_2(k)$
) a k-valued point of the underlying scheme
${}^{Y}\!e_2\in Y_2(k)$
) a k-valued point of the underlying scheme
![]() $X_2$
(resp.
$X_2$
(resp.
![]() $Y_2$
) of the log scheme
$Y_2$
) of the log scheme
![]() $X_2^{\mathrm {log}}$
(resp.
$X_2^{\mathrm {log}}$
(resp.
![]() $Y_2^{\mathrm {log}}$
) that lies, relative to
$Y_2^{\mathrm {log}}$
) that lies, relative to
![]() $p_{2/1}^{\mathrm {log}}$
(resp.
$p_{2/1}^{\mathrm {log}}$
(resp.
![]() ${}^{Y}\!p_{2/1}^{\mathrm {log}}$
), over the k-valued point of X (resp. Y) determined by the node
${}^{Y}\!p_{2/1}^{\mathrm {log}}$
), over the k-valued point of X (resp. Y) determined by the node
![]() $e \in \mathrm {Node}(\mathcal {G})$
(resp.
$e \in \mathrm {Node}(\mathcal {G})$
(resp.
![]() ${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
). Thus, we obtain an outer isomorphism
${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
). Thus, we obtain an outer isomorphism
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(iii)]) that may be characterized, up to composition with elements of
![]() $\mathrm {Aut}^{|\mathrm {grph}|}(\mathcal {G}_{2\in \{1,2\}, e_2}) \subseteq \mathrm {Out}(\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}})$
(resp.
$\mathrm {Aut}^{|\mathrm {grph}|}(\mathcal {G}_{2\in \{1,2\}, e_2}) \subseteq \mathrm {Out}(\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}})$
(resp.
![]() $\mathrm {Aut}^{|\mathrm {grph}|}({}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}) \subseteq \mathrm {Out}(\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}})$
) (cf. [Reference Hoshi and Mochizuki9, Def. 2.6(i)]; [Reference Hoshi and Mochizuki10, Rem. 4.1.2]), as the group-theoretically cuspidal (cf. [Reference Mochizuki18, Def. 1.4(iv)]) outer isomorphism such that the semi-graph of anabelioids structure on
$\mathrm {Aut}^{|\mathrm {grph}|}({}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}) \subseteq \mathrm {Out}(\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}})$
) (cf. [Reference Hoshi and Mochizuki9, Def. 2.6(i)]; [Reference Hoshi and Mochizuki10, Rem. 4.1.2]), as the group-theoretically cuspidal (cf. [Reference Mochizuki18, Def. 1.4(iv)]) outer isomorphism such that the semi-graph of anabelioids structure on
![]() $\mathcal {G}_{2\in \{1,2\}, e_2}$
(resp.
$\mathcal {G}_{2\in \{1,2\}, e_2}$
(resp.
![]() ${}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}$
) is the semi-graph of anabelioids structure determined (cf. [Reference Hoshi and Mochizuki8, Th. A) by the resulting composite outer representation
${}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}$
) is the semi-graph of anabelioids structure determined (cf. [Reference Hoshi and Mochizuki8, Th. A) by the resulting composite outer representation
—where the second arrow is the outer action determined by the exact sequence
![]() $1\rightarrow \Pi _{2/1} \rightarrow \Pi _{2} \rightarrow \Pi _{1} \rightarrow 1$
(resp.
$1\rightarrow \Pi _{2/1} \rightarrow \Pi _{2} \rightarrow \Pi _{1} \rightarrow 1$
(resp.
![]() $1\rightarrow {}^{Y}\!\Pi _{2/1} \rightarrow {}^{Y}\!\Pi _{2} \rightarrow {}^{Y}\!\Pi _{1} \rightarrow 1$
)—in a fashion compatible with the restriction
$1\rightarrow {}^{Y}\!\Pi _{2/1} \rightarrow {}^{Y}\!\Pi _{2} \rightarrow {}^{Y}\!\Pi _{1} \rightarrow 1$
)—in a fashion compatible with the restriction
![]() $\Pi _{2/1} \twoheadrightarrow \Pi _{\{2\}}$
(resp.
$\Pi _{2/1} \twoheadrightarrow \Pi _{\{2\}}$
(resp.
![]() ${}^{Y}\!\Pi _{2/1} \twoheadrightarrow {}^{Y}\!\Pi _{\{2\}}$
) of
${}^{Y}\!\Pi _{2/1} \twoheadrightarrow {}^{Y}\!\Pi _{\{2\}}$
) of
![]() $p_{\{1,2\}/\{2\}}^\Pi $
(resp.
$p_{\{1,2\}/\{2\}}^\Pi $
(resp.
![]() ${}^{Y}\!p_{\{1,2\}/\{2\}}^\Pi $
) to
${}^{Y}\!p_{\{1,2\}/\{2\}}^\Pi $
) to
![]() $\Pi _{2/1} \subseteq \Pi _{2}$
(resp.
$\Pi _{2/1} \subseteq \Pi _{2}$
(resp.
![]() ${}^{Y}\!\Pi _{2/1} \subseteq {}^{Y}\!\Pi _{2}$
) and the given outer isomorphisms
${}^{Y}\!\Pi _{2/1} \subseteq {}^{Y}\!\Pi _{2}$
) and the given outer isomorphisms
![]() $\Pi _{\{2\}} \stackrel {\sim }{\rightarrow } \Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
(resp.
$\Pi _{\{2\}} \stackrel {\sim }{\rightarrow } \Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
(resp.
![]() ${}^{Y}\!\Pi _{\{2\}} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{\mathcal {G}}$
). Let
${}^{Y}\!\Pi _{\{2\}} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{\mathcal {G}}$
). Let
be the
![]() $\{1, 2\}$
-tripod (cf. [Reference Hoshi and Mochizuki10, Def. 3.1(v)]) that arises from
$\{1, 2\}$
-tripod (cf. [Reference Hoshi and Mochizuki10, Def. 3.1(v)]) that arises from
![]() $e \in \mathrm {Node}(\mathcal {G})$
(resp.
$e \in \mathrm {Node}(\mathcal {G})$
(resp.
![]() ${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
) (cf. [Reference Hoshi and Mochizuki10, Def. 3.7(i)]);
${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
) (cf. [Reference Hoshi and Mochizuki10, Def. 3.7(i)]);
![]() $\Pi _v \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}\stackrel {\sim }{\leftarrow } \Pi _{2/1}$
(resp.
$\Pi _v \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}\stackrel {\sim }{\leftarrow } \Pi _{2/1}$
(resp.
![]() $\Pi _{{}^{Y}\!v} \subseteq \Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
$\Pi _{{}^{Y}\!v} \subseteq \Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
![]() $\stackrel {\sim }{\leftarrow } {}^{Y}\!\Pi _{2/1}$
) a
$\stackrel {\sim }{\leftarrow } {}^{Y}\!\Pi _{2/1}$
) a
![]() $\{1,2\}$
-tripod in
$\{1,2\}$
-tripod in
![]() $\Pi _{2}$
(resp.
$\Pi _{2}$
(resp.
![]() ${}^{Y}\!\Pi _{2}$
) associated with the tripod v (resp.
${}^{Y}\!\Pi _{2}$
) associated with the tripod v (resp.
![]() ${}^{Y}\!v$
) (cf. [Reference Hoshi and Mochizuki10, Def. 3.3(i)]);
${}^{Y}\!v$
) (cf. [Reference Hoshi and Mochizuki10, Def. 3.3(i)]);
an outer isomorphism of profinite groups. Suppose that the following conditions are satisfied:
-
(a) The outer isomorphism
 $\alpha $
is group-theoretically nodal (cf. [Reference Hoshi and Mochizuki8, Def. 1.12]), that is, determines a bijection of the set of
$\alpha $
is group-theoretically nodal (cf. [Reference Hoshi and Mochizuki8, Def. 1.12]), that is, determines a bijection of the set of
 $\Pi _{\mathcal {G}}$
-conjugates of
$\Pi _{\mathcal {G}}$
-conjugates of
 $\Pi _{e} \subseteq \Pi _{\mathcal {G}}$
and the set of
$\Pi _{e} \subseteq \Pi _{\mathcal {G}}$
and the set of
 $\Pi _{{}^{Y}\!\mathcal {G}}$
-conjugates of
$\Pi _{{}^{Y}\!\mathcal {G}}$
-conjugates of
 $\Pi _{{}^{Y}\!e} \subseteq \Pi _{{}^{Y}\!\mathcal {G}}$
.
$\Pi _{{}^{Y}\!e} \subseteq \Pi _{{}^{Y}\!\mathcal {G}}$
. -
(b) The outer isomorphism
 $\alpha $
is
$\alpha $
is
 $2$
-cuspidalizable (cf. [Reference Hoshi and Mochizuki10, Def. 3.20]), that is, the outer isomorphism arises from a (uniquely determined, up to permutation of the
$2$
-cuspidalizable (cf. [Reference Hoshi and Mochizuki10, Def. 3.20]), that is, the outer isomorphism arises from a (uniquely determined, up to permutation of the $$\begin{align*}\Pi_{1}\stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}} \stackrel{\alpha}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\sim}{\longleftarrow} {}^{Y}\!\Pi_{1} \end{align*}$$
$$\begin{align*}\Pi_{1}\stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}} \stackrel{\alpha}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\sim}{\longleftarrow} {}^{Y}\!\Pi_{1} \end{align*}$$
 $2$
factors—cf. [Reference Hoshi and Mochizuki8, Th. B) PFC-admissible (cf. [Reference Hoshi and Mochizuki9, Def. 1.4(iii)]) outer isomorphism
$2$
factors—cf. [Reference Hoshi and Mochizuki8, Th. B) PFC-admissible (cf. [Reference Hoshi and Mochizuki9, Def. 1.4(iii)]) outer isomorphism
 $\Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
. (In particular, the outer isomorphism
$\Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
. (In particular, the outer isomorphism
 $\alpha $
is group-theoretically cuspidal.)
$\alpha $
is group-theoretically cuspidal.)
Then the following hold:
-
(i) There exists a PFC-admissible isomorphism
 $\widetilde \alpha _2\colon \Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
that lifts
$\widetilde \alpha _2\colon \Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
that lifts
 $\alpha $
such that the composite
$\alpha $
such that the composite  $$ \begin{align*}\Pi_{\mathcal{G}_{2\in\{1,2\}, e_2}} \stackrel{\sim}{\longleftarrow} \Pi_{2/1} \stackrel{\sim}{\longrightarrow} {}^{Y}\!\Pi_{2/1} \stackrel{\sim}{\longrightarrow} \Pi_{{}^{Y}\!\mathcal{G}_{2\in\{1,2\}, {}^{Y}\!e_2}}\end{align*} $$
$$ \begin{align*}\Pi_{\mathcal{G}_{2\in\{1,2\}, e_2}} \stackrel{\sim}{\longleftarrow} \Pi_{2/1} \stackrel{\sim}{\longrightarrow} {}^{Y}\!\Pi_{2/1} \stackrel{\sim}{\longrightarrow} \Pi_{{}^{Y}\!\mathcal{G}_{2\in\{1,2\}, {}^{Y}\!e_2}}\end{align*} $$
—where the second arrow is the restriction of
 $\widetilde \alpha _2$
—is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
$\widetilde \alpha _2$
—is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]). -
(ii) The outer isomorphism
 $\alpha _2\colon \Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
determined by the isomorphism
$\alpha _2\colon \Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
determined by the isomorphism
 $\widetilde \alpha _2$
of (i) induces a bijection between the set of
$\widetilde \alpha _2$
of (i) induces a bijection between the set of
 $\Pi _{2}$
-conjugates of
$\Pi _{2}$
-conjugates of
 $\Pi _v \subseteq \Pi _{2}$
and the set of
$\Pi _v \subseteq \Pi _{2}$
and the set of
 ${}^{Y}\!\Pi _{2}$
-conjugates of
${}^{Y}\!\Pi _{2}$
-conjugates of
 $\Pi _{{}^{Y}\!v} \subseteq {}^{Y}\!\Pi _{2}$
. Moreover, if we think of
$\Pi _{{}^{Y}\!v} \subseteq {}^{Y}\!\Pi _{2}$
. Moreover, if we think of
 $\Pi _{v}$
,
$\Pi _{v}$
,
 $\Pi _{{}^{Y}\!v}$
as the respective (pro-
$\Pi _{{}^{Y}\!v}$
as the respective (pro-
 $\Sigma $
) fundamental groups of
$\Sigma $
) fundamental groups of
 $\mathcal {G}_{2\in \{1,2\}, e_2}|_v$
,
$\mathcal {G}_{2\in \{1,2\}, e_2}|_v$
,
 ${}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}|_{{}^{Y}\!v}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.1(iii)]; [Reference Hoshi and Mochizuki9, Rem. 2.1.1]), then the induced outer isomorphism
${}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}|_{{}^{Y}\!v}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.1(iii)]; [Reference Hoshi and Mochizuki9, Rem. 2.1.1]), then the induced outer isomorphism
 $\Pi _v \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!v}$
(cf. [Reference Hoshi and Mochizuki10, Th. 3.16(i)]) is group-theoretically cuspidal.
$\Pi _v \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!v}$
(cf. [Reference Hoshi and Mochizuki10, Th. 3.16(i)]) is group-theoretically cuspidal. -
(iii) The outer isomorphism
 $\alpha $
is graphic.
$\alpha $
is graphic.
Proof. In light of conditions (a) and (b), assertion (i) follows immediately from [Reference Hoshi and Mochizuki8, Th. A] (cf. also our assumption that
![]() $\mathrm {Node} (\mathcal {G})^\sharp $
$\mathrm {Node} (\mathcal {G})^\sharp $
![]() $ = \mathrm {Node} ({}^{Y}\!\mathcal {G})^\sharp = 1$
, which implies that the outer representation
$ = \mathrm {Node} ({}^{Y}\!\mathcal {G})^\sharp = 1$
, which implies that the outer representation
![]() $\Pi _{e}\rightarrow \mathrm {Out}(\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}})$
[resp.
$\Pi _{e}\rightarrow \mathrm {Out}(\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}})$
[resp.
![]() $\Pi _{{}^{Y}\!e} \rightarrow \mathrm {Out}(\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}})$
] is nodally nondegenerate!). Next, let us observe that the
$\Pi _{{}^{Y}\!e} \rightarrow \mathrm {Out}(\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}})$
] is nodally nondegenerate!). Next, let us observe that the
![]() $\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
- (resp.
$\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
- (resp.
![]() $\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
-) conjugacy class of
$\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
-) conjugacy class of
![]() $\Pi _v \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
(resp.
$\Pi _v \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
(resp.
![]() $\Pi _{{}^{Y}\!v} \subseteq \Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
) may be characterized as the unique
$\Pi _{{}^{Y}\!v} \subseteq \Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
) may be characterized as the unique
![]() $\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
- (resp.
$\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
- (resp.
![]() $\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
-) conjugacy class of verticial subgroups that fails to map injectively via the surjection
$\Pi _{{}^{Y}\!\mathcal {G}_{2\in \{1,2\}, {}^{Y}\!e_2}}$
-) conjugacy class of verticial subgroups that fails to map injectively via the surjection
![]() $\Pi _{2/1} \twoheadrightarrow \Pi _{\{2\}}$
(resp.
$\Pi _{2/1} \twoheadrightarrow \Pi _{\{2\}}$
(resp.
![]() ${}^{Y}\!\Pi _{2/1} \twoheadrightarrow {}^{Y}\!\Pi _{\{2\}}$
). Now assertion (ii) follows immediately from assertion (i). Assertion (iii) follows immediately—in light of [Reference Mochizuki20, Prop. 1.2(iii)]—from assertions (i) and (ii), together with the various definitions involved. This completes the proof of Lemma 3.1.
${}^{Y}\!\Pi _{2/1} \twoheadrightarrow {}^{Y}\!\Pi _{\{2\}}$
). Now assertion (ii) follows immediately from assertion (i). Assertion (iii) follows immediately—in light of [Reference Mochizuki20, Prop. 1.2(iii)]—from assertions (i) and (ii), together with the various definitions involved. This completes the proof of Lemma 3.1.
Before proceeding, we pause to observe that Lemma 3.1 may be applied to obtain an alternative proof of a slightly weaker version of Theorem 3.3 below, as follows.
Proposition 3.2 (Graphicity of group-theoretically nodal 2-cuspidalizable outer isomorphisms).
In the notation of the discussion at the beginning of the present §3, let
be an outer isomorphism of profinite groups. Suppose that the following conditions are satisfied:
-
(a) The outer isomorphism
 $\alpha $
is group-theoretically nodal (cf. [Reference Hoshi and Mochizuki8, Def. 1.12]).
$\alpha $
is group-theoretically nodal (cf. [Reference Hoshi and Mochizuki8, Def. 1.12]). -
(b) The outer isomorphism
 $\alpha $
is
$\alpha $
is
 $2$
-cuspidalizable (cf. [Reference Hoshi and Mochizuki10, Def. 3.20]), that is, the outer isomorphism arises from a (uniquely determined, up to permutation of the
$2$
-cuspidalizable (cf. [Reference Hoshi and Mochizuki10, Def. 3.20]), that is, the outer isomorphism arises from a (uniquely determined, up to permutation of the $$\begin{align*}\Pi_{1}\stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}} \stackrel{\alpha}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\sim}{\longleftarrow} {}^{Y}\!\Pi_{1} \end{align*}$$
$$\begin{align*}\Pi_{1}\stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}} \stackrel{\alpha}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\sim}{\longleftarrow} {}^{Y}\!\Pi_{1} \end{align*}$$
 $2$
factors—cf. [Reference Hoshi and Mochizuki8, Th. B]) PFC-admissible (cf. [Reference Hoshi and Mochizuki9, Def. 1.4(iii)]) outer isomorphism
$2$
factors—cf. [Reference Hoshi and Mochizuki8, Th. B]) PFC-admissible (cf. [Reference Hoshi and Mochizuki9, Def. 1.4(iii)]) outer isomorphism
 $\Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
. (In particular, the outer isomorphism
$\Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
. (In particular, the outer isomorphism
 $\alpha $
is group-theoretically cuspidal—cf. [Reference Mochizuki18, Def. 1.4(iv)].)
$\alpha $
is group-theoretically cuspidal—cf. [Reference Mochizuki18, Def. 1.4(iv)].)
Then the outer isomorphism
![]() $\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
$\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
Proof. Let us first observe that it follows from condition (a), together with [Reference Mochizuki18, Prop. 1.2(i)] that
![]() $\alpha $
determines a bijection
$\alpha $
determines a bijection
![]() $\mathrm {Node}(\mathcal {G})\stackrel {\sim }{\rightarrow }\mathrm {Node}({}^{Y}\!\mathcal {G})$
, so
$\mathrm {Node}(\mathcal {G})\stackrel {\sim }{\rightarrow }\mathrm {Node}({}^{Y}\!\mathcal {G})$
, so
![]() $\mathrm {Node}(\mathcal {G})^\sharp = \mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp $
. We verify Proposition 3.2 by induction on
$\mathrm {Node}(\mathcal {G})^\sharp = \mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp $
. We verify Proposition 3.2 by induction on
![]() $\mathrm {Node}(\mathcal {G})^\sharp = \mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp $
. If
$\mathrm {Node}(\mathcal {G})^\sharp = \mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp $
. If
![]() $\mathrm {Node}(\mathcal {G}) = \mathrm {Node}({}^{Y}\!\mathcal {G}) = \emptyset $
, then Proposition 3.2 is immediate. Thus, we may assume without loss of generality that
$\mathrm {Node}(\mathcal {G}) = \mathrm {Node}({}^{Y}\!\mathcal {G}) = \emptyset $
, then Proposition 3.2 is immediate. Thus, we may assume without loss of generality that
![]() $\mathrm {Node}(\mathcal {G})$
,
$\mathrm {Node}(\mathcal {G})$
,
![]() $\mathrm {Node}({}^{Y}\!\mathcal {G})\neq \emptyset $
. Let
$\mathrm {Node}({}^{Y}\!\mathcal {G})\neq \emptyset $
. Let
![]() $e \in \mathrm {Node}(\mathcal {G})$
. Write
$e \in \mathrm {Node}(\mathcal {G})$
. Write
![]() ${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
for the node of
${}^{Y}\!e \in \mathrm {Node}({}^{Y}\!\mathcal {G})$
for the node of
![]() ${}^{Y}\!\mathcal {G}$
that corresponds, via
${}^{Y}\!\mathcal {G}$
that corresponds, via
![]() $\alpha $
, to e. Write
$\alpha $
, to e. Write
![]() $\mathcal {G}_{\leadsto {\{e\}}}$
(resp.
$\mathcal {G}_{\leadsto {\{e\}}}$
(resp.
![]() ${}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}$
) for the generization of
${}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}$
) for the generization of
![]() $\mathcal {G}$
(resp.
$\mathcal {G}$
(resp.
![]() ${}^{Y}\!\mathcal {G}$
) with respect to
${}^{Y}\!\mathcal {G}$
) with respect to
![]() $\{e\}\subseteq \mathrm {Node}(\mathcal {G})$
(resp.
$\{e\}\subseteq \mathrm {Node}(\mathcal {G})$
(resp.
![]() $\{{}^{Y}\!e\}\subseteq \mathrm {Node}({}^{Y}\!\mathcal {G})$
) (cf. [Reference Hoshi and Mochizuki9, Def. 2.8]);
$\{{}^{Y}\!e\}\subseteq \mathrm {Node}({}^{Y}\!\mathcal {G})$
) (cf. [Reference Hoshi and Mochizuki9, Def. 2.8]);
![]() $\beta $
for the composite outer isomorphism
$\beta $
for the composite outer isomorphism
 $$\begin{align*}\Pi_{\mathcal{G}_{\leadsto {\{e\}}}} \stackrel{\Phi_{\mathcal{G}_{\leadsto {\{e\}}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{\mathcal{G}} \stackrel{\alpha}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\Phi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}}^{-1} }{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}} \end{align*}$$
$$\begin{align*}\Pi_{\mathcal{G}_{\leadsto {\{e\}}}} \stackrel{\Phi_{\mathcal{G}_{\leadsto {\{e\}}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{\mathcal{G}} \stackrel{\alpha}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\Phi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}}^{-1} }{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}} \end{align*}$$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]);
![]() $v_{0} \in \mathrm {Vert}(\mathcal {G}_{\leadsto {\{e\}}})$
(resp.
$v_{0} \in \mathrm {Vert}(\mathcal {G}_{\leadsto {\{e\}}})$
(resp.
![]() ${}^{Y}\!v_{0} \in \mathrm {Vert}({}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}})$
) for the (uniquely determined) vertex of the generization
${}^{Y}\!v_{0} \in \mathrm {Vert}({}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}})$
) for the (uniquely determined) vertex of the generization
![]() $\mathcal {G}_{\leadsto {\{e\}}}$
(resp.
$\mathcal {G}_{\leadsto {\{e\}}}$
(resp.
![]() ${}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}$
) that does not arise from a vertex of
${}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}$
) that does not arise from a vertex of
![]() $\mathrm {Vert}(\mathcal {G})$
(resp.
$\mathrm {Vert}(\mathcal {G})$
(resp.
![]() $\mathrm {Vert}({}^{Y}\!\mathcal {G})$
). Let
$\mathrm {Vert}({}^{Y}\!\mathcal {G})$
). Let
![]() $\Pi _{v_{0}} \subseteq \Pi _{\mathcal {G}_{\leadsto {\{e\}}}}$
(resp.
$\Pi _{v_{0}} \subseteq \Pi _{\mathcal {G}_{\leadsto {\{e\}}}}$
(resp.
![]() $\Pi _{{}^{Y}\!v_{0}} \subseteq \Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}}$
) be a verticial subgroup associated with
$\Pi _{{}^{Y}\!v_{0}} \subseteq \Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}}$
) be a verticial subgroup associated with
![]() $v_{0} \in \mathrm {Vert}(\mathcal {G}_{\leadsto {\{e\}}})$
(resp.
$v_{0} \in \mathrm {Vert}(\mathcal {G}_{\leadsto {\{e\}}})$
(resp.
![]() ${}^{Y}\!v_{0} \in \mathrm {Vert}({}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}})$
);
${}^{Y}\!v_{0} \in \mathrm {Vert}({}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}})$
);
![]() $\Pi _{e}\subseteq \Pi _{v_{0}}$
(resp.
$\Pi _{e}\subseteq \Pi _{v_{0}}$
(resp.
![]() $ \Pi _{{}^{Y}\!e}\subseteq \Pi _{{}^{Y}\!v_{0}} $
) a subgroup that maps to a nodal subgroup associated with e in
$ \Pi _{{}^{Y}\!e}\subseteq \Pi _{{}^{Y}\!v_{0}} $
) a subgroup that maps to a nodal subgroup associated with e in
![]() $\Pi _{\mathcal {G}}$
(resp. to
$\Pi _{\mathcal {G}}$
(resp. to
![]() ${}^{Y}\!e$
in
${}^{Y}\!e$
in
![]() $\Pi _{{}^{Y}\!\mathcal {G}}$
). Thus, it follows immediately from [Reference Hoshi and Mochizuki8, Lem. 1.9(i) and (ii)] (cf. also [Reference Hoshi and Mochizuki8, Lem. 1.5]; condition (2) of [Reference Hoshi and Mochizuki9, Prop. 2.9(i)]) that
$\Pi _{{}^{Y}\!\mathcal {G}}$
). Thus, it follows immediately from [Reference Hoshi and Mochizuki8, Lem. 1.9(i) and (ii)] (cf. also [Reference Hoshi and Mochizuki8, Lem. 1.5]; condition (2) of [Reference Hoshi and Mochizuki9, Prop. 2.9(i)]) that
![]() $\Pi _{v_{0}}$
(resp.
$\Pi _{v_{0}}$
(resp.
![]() $ \Pi _{{}^{Y}\!v_{0}} $
) may be characterized as the unique verticial subgroup of
$ \Pi _{{}^{Y}\!v_{0}} $
) may be characterized as the unique verticial subgroup of
![]() $\Pi _{\mathcal {G}_{\leadsto {\{e\}}}}$
(resp.
$\Pi _{\mathcal {G}_{\leadsto {\{e\}}}}$
(resp.
![]() $\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}}$
) that contains
$\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}}$
) that contains
![]() $\Pi _{e}$
(resp.
$\Pi _{e}$
(resp.
![]() $\Pi _{{}^{Y}\!e}$
).
$\Pi _{{}^{Y}\!e}$
).
Next, let us observe that, by applying the induction hypothesis to
![]() $\beta $
, we conclude that
$\beta $
, we conclude that
![]() $\beta $
is graphic. Thus, it follows immediately—in light of [Reference Mochizuki18, Prop. 1.5(ii)]—from the definition of the generizations under consideration (cf. condition (3) of [Reference Hoshi and Mochizuki9, Prop. 2.9(i)]) that, to complete the verification of Proposition 3.2, it suffices to verify that the following assertion holds:
$\beta $
is graphic. Thus, it follows immediately—in light of [Reference Mochizuki18, Prop. 1.5(ii)]—from the definition of the generizations under consideration (cf. condition (3) of [Reference Hoshi and Mochizuki9, Prop. 2.9(i)]) that, to complete the verification of Proposition 3.2, it suffices to verify that the following assertion holds:
Claim 3.2.A: Let
![]() $H\subseteq \Pi _{v_{0}} \subseteq \Pi _{\mathcal {G}_{\leadsto {\{e\}}}}$
be a closed subgroup of
$H\subseteq \Pi _{v_{0}} \subseteq \Pi _{\mathcal {G}_{\leadsto {\{e\}}}}$
be a closed subgroup of
![]() $\Pi _{v_{0}}$
whose image in
$\Pi _{v_{0}}$
whose image in
![]() $\Pi _{\mathcal {G}}$
is a verticial subgroup. Then the image of H via the composite
$\Pi _{\mathcal {G}}$
is a verticial subgroup. Then the image of H via the composite
 $$ \begin{align*}\Pi_{\mathcal{G}_{\leadsto {\{e\}}}} \stackrel{\beta}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}} \stackrel{\Phi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}}\end{align*} $$
$$ \begin{align*}\Pi_{\mathcal{G}_{\leadsto {\{e\}}}} \stackrel{\beta}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}} \stackrel{\Phi_{{}^{Y}\!\mathcal{G}_{\leadsto {\{{}^{Y}\!e\}}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}}\end{align*} $$
is a verticial subgroup.
To verify Claim 3.2.A, let us observe that since
![]() $\beta $
is graphic, it follows immediately from the above characterization of
$\beta $
is graphic, it follows immediately from the above characterization of
![]() $\Pi _{v_{0}}$
,
$\Pi _{v_{0}}$
,
![]() $\Pi _{{}^{Y}\!v_{0}}$
that
$\Pi _{{}^{Y}\!v_{0}}$
that
![]() $\beta $
maps
$\beta $
maps
![]() $\Pi _{v_{0}}$
bijectively onto a
$\Pi _{v_{0}}$
bijectively onto a
![]() $\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}}$
-conjugate of
$\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {\{{}^{Y}\!e\}}}}$
-conjugate of
![]() $\Pi _{{}^{Y}\!v_{0}}$
. Thus, it follows immediately from condition (b), together with the evident isomorphism (i.e., as opposed to outomorphism—cf. [Reference Hoshi and Mochizuki10, Rem. 4.14.1]) version of [Reference Hoshi and Mochizuki10, Lem. 4.8(i) and (ii)] that, in the notation of [Reference Hoshi and Mochizuki10, Def. 4.3], the outer isomorphism
$\Pi _{{}^{Y}\!v_{0}}$
. Thus, it follows immediately from condition (b), together with the evident isomorphism (i.e., as opposed to outomorphism—cf. [Reference Hoshi and Mochizuki10, Rem. 4.14.1]) version of [Reference Hoshi and Mochizuki10, Lem. 4.8(i) and (ii)] that, in the notation of [Reference Hoshi and Mochizuki10, Def. 4.3], the outer isomorphism
![]() $\Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
of condition (b) induces compatible outer isomorphisms
$\Pi _{2} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{2}$
of condition (b) induces compatible outer isomorphisms
![]() $(\Pi _{v_{0}})_2 \stackrel {\sim }{\rightarrow } (\Pi _{{}^{Y}\!v_{0}})_2$
,
$(\Pi _{v_{0}})_2 \stackrel {\sim }{\rightarrow } (\Pi _{{}^{Y}\!v_{0}})_2$
,
![]() $\Pi _{v_{0}} \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!v_{0}}$
. In particular, by applying Lemma 3.1(iii) to these outer isomorphisms, one concludes that Claim 3.2.A holds, as desired. This completes the proof of Proposition 3.2.
$\Pi _{v_{0}} \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!v_{0}}$
. In particular, by applying Lemma 3.1(iii) to these outer isomorphisms, one concludes that Claim 3.2.A holds, as desired. This completes the proof of Proposition 3.2.
Theorem 3.3 (Graphicity of profinite outer isomorphisms).
Let
![]() $\Sigma _0$
be a nonempty set of prime numbers;
$\Sigma _0$
be a nonempty set of prime numbers;
![]() $\mathcal {H}$
,
$\mathcal {H}$
,
![]() $\mathcal {J}$
semi-graphs of anabelioids of pro-
$\mathcal {J}$
semi-graphs of anabelioids of pro-
![]() $\Sigma _0$
PSC-type;
$\Sigma _0$
PSC-type;
![]() $\Pi _{\mathcal {H}}$
,
$\Pi _{\mathcal {H}}$
,
![]() $\Pi _{\mathcal {J}}$
the (pro-
$\Pi _{\mathcal {J}}$
the (pro-
![]() $\Sigma _0$
) fundamental groups of
$\Sigma _0$
) fundamental groups of
![]() $\mathcal {H}$
,
$\mathcal {H}$
,
![]() $\mathcal {J}$
, respectively;
$\mathcal {J}$
, respectively;
an outer isomorphism of profinite groups. Then the following conditions are equivalent:
-
(i) The outer isomorphism
 $\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]).
$\alpha $
is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]). -
(ii) The outer isomorphism
 $\alpha $
is group-theoretically verticial and group-theoretically cuspidal (cf. [Reference Mochizuki18, Def. 1.4(iv)]).
$\alpha $
is group-theoretically verticial and group-theoretically cuspidal (cf. [Reference Mochizuki18, Def. 1.4(iv)]). -
(iii) The outer isomorphism
 $\alpha $
is group-theoretically nodal (cf. [Reference Hoshi and Mochizuki8, Def. 1.12]) and group-theoretically cuspidal.
$\alpha $
is group-theoretically nodal (cf. [Reference Hoshi and Mochizuki8, Def. 1.12]) and group-theoretically cuspidal.
Proof. The implication (i)
![]() $\Rightarrow $
(ii) (resp. (ii)
$\Rightarrow $
(ii) (resp. (ii)
![]() $\Rightarrow $
(iii)) follows from the various definitions involved (resp. [Reference Hoshi and Mochizuki8, Lem. 1.9(i)]). Thus, it suffices to verify the implication (iii)
$\Rightarrow $
(iii)) follows from the various definitions involved (resp. [Reference Hoshi and Mochizuki8, Lem. 1.9(i)]). Thus, it suffices to verify the implication (iii)
![]() $\Rightarrow $
(i). Suppose that condition (iii) holds. Then, to verify the graphicity of
$\Rightarrow $
(i). Suppose that condition (iii) holds. Then, to verify the graphicity of
![]() $\alpha $
, it follows from [Reference Mochizuki18, Th. 1.6(ii)] that it suffices to verify that
$\alpha $
, it follows from [Reference Mochizuki18, Th. 1.6(ii)] that it suffices to verify that
![]() $\alpha $
is graphically filtration-preserving (cf. [Reference Mochizuki18, Def. 1.4(iii)]). In particular, by replacing
$\alpha $
is graphically filtration-preserving (cf. [Reference Mochizuki18, Def. 1.4(iii)]). In particular, by replacing
![]() $\Pi _{\mathcal {H}}$
,
$\Pi _{\mathcal {H}}$
,
![]() $\Pi _{\mathcal {J}}$
by suitable open subgroups of
$\Pi _{\mathcal {J}}$
by suitable open subgroups of
![]() $\Pi _{\mathcal {H}}$
,
$\Pi _{\mathcal {H}}$
,
![]() $\Pi _{\mathcal {J}}$
, it suffices to verify that
$\Pi _{\mathcal {J}}$
, it suffices to verify that
![]() $\alpha $
determines isomorphisms
$\alpha $
determines isomorphisms
where we write “
![]() $\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {edge}}$
,” “
$\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {edge}}$
,” “
![]() $\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {vert}}$
” for the closed subgroups of the abelianization “
$\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {vert}}$
” for the closed subgroups of the abelianization “
![]() $\Pi _{(-)}^{\mathrm {ab}}$
” of “
$\Pi _{(-)}^{\mathrm {ab}}$
” of “
![]() $\Pi _{(-)}$
” topologically generated by the images of the edge-like, verticial subgroups of “
$\Pi _{(-)}$
” topologically generated by the images of the edge-like, verticial subgroups of “
![]() $\Pi _{(-)}$
.” Here, we may assume without loss of generality that
$\Pi _{(-)}$
.” Here, we may assume without loss of generality that
![]() $\mathcal {H}$
and
$\mathcal {H}$
and
![]() $\mathcal {J}$
are sturdy, hence admit compactifications (cf. [Reference Mochizuki18, Rems. 1.1.5 and 1.1.6]). Now the assertion concerning “
$\mathcal {J}$
are sturdy, hence admit compactifications (cf. [Reference Mochizuki18, Rems. 1.1.5 and 1.1.6]). Now the assertion concerning “
![]() $\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {edge}}$
” follows immediately from condition (iii). On the other hand, the assertion concerning “
$\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {edge}}$
” follows immediately from condition (iii). On the other hand, the assertion concerning “
![]() $\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {vert}}$
” follows immediately from the duality discussed in [Reference Mochizuki18, Prop. 1.3] applied to the compactifications of
$\Pi _{(-)}^{\mathrm {ab}\text {-}\mathrm {vert}}$
” follows immediately from the duality discussed in [Reference Mochizuki18, Prop. 1.3] applied to the compactifications of
![]() $\mathcal {H}$
,
$\mathcal {H}$
,
![]() $\mathcal {J}$
, together with condition (iii). This completes the proof of Theorem 3.3.
$\mathcal {J}$
, together with condition (iii). This completes the proof of Theorem 3.3.
Remark 3.3.1. Here, we observe that results such as [Reference Boggi6, Cor. 6.1]; [Reference Boggi6, Cor. 6.4(ii)]; [Reference Boggi6, Th. 6.6] amount, when translated into the language of the present paper, to a special case of the result obtained by concatenating the equivalence (i)
![]() $\Leftrightarrow $
(iii) of Theorem 3.3, with the computation of the normalizer given in [Reference Hoshi and Mochizuki9, Th. 5.14(iii)] (i.e., in essence, [Reference Mochizuki18, Cor. 2.7(iii) and (iv)]). Moreover, the proof given above of this equivalence (i)
$\Leftrightarrow $
(iii) of Theorem 3.3, with the computation of the normalizer given in [Reference Hoshi and Mochizuki9, Th. 5.14(iii)] (i.e., in essence, [Reference Mochizuki18, Cor. 2.7(iii) and (iv)]). Moreover, the proof given above of this equivalence (i)
![]() $\Leftrightarrow $
(iii) of Theorem 3.3 is, essentially, a restatement of various results from the theory of [Reference Mochizuki18]. That is to say, although the statements of these results that occur in the present series of papers and in [Reference Boggi6] are formulated and arranged in a somewhat different way, the essential mathematical content that underlies these results is, in fact, entirely identical; moreover, this state of affairs is by no means a coincidence. Indeed, this mathematical content is given in [Reference Mochizuki18] as [Reference Mochizuki18, Prop. 1.3]; [Reference Mochizuki18, Prop. 2.6]. In [Reference Boggi6], this mathematical content is given as [Reference Boggi6, Lem. 5.11] (and the surrounding discussion), which, in fact, was related to the author of [Reference Boggi6] by the senior author of the present paper in the context of an explanation of the theory of [Reference Mochizuki18].
$\Leftrightarrow $
(iii) of Theorem 3.3 is, essentially, a restatement of various results from the theory of [Reference Mochizuki18]. That is to say, although the statements of these results that occur in the present series of papers and in [Reference Boggi6] are formulated and arranged in a somewhat different way, the essential mathematical content that underlies these results is, in fact, entirely identical; moreover, this state of affairs is by no means a coincidence. Indeed, this mathematical content is given in [Reference Mochizuki18] as [Reference Mochizuki18, Prop. 1.3]; [Reference Mochizuki18, Prop. 2.6]. In [Reference Boggi6], this mathematical content is given as [Reference Boggi6, Lem. 5.11] (and the surrounding discussion), which, in fact, was related to the author of [Reference Boggi6] by the senior author of the present paper in the context of an explanation of the theory of [Reference Mochizuki18].
Corollary 3.4 (Graphicity of discrete outer isomorphisms).
Let
![]() $\mathcal {H}$
,
$\mathcal {H}$
,
![]() $\mathcal {J}$
be semi-graphs of temperoids of HSD-type (cf. Definition 2.3(iii));
$\mathcal {J}$
be semi-graphs of temperoids of HSD-type (cf. Definition 2.3(iii));
![]() $\Pi _{\mathcal {H}}$
,
$\Pi _{\mathcal {H}}$
,
![]() $\Pi _{\mathcal {J}}$
the fundamental groups of
$\Pi _{\mathcal {J}}$
the fundamental groups of
![]() $\mathcal {H}$
,
$\mathcal {H}$
,
![]() $\mathcal {J}$
, respectively (cf. Proposition 2.5(i));
$\mathcal {J}$
, respectively (cf. Proposition 2.5(i));
an outer isomorphism. Then the following conditions are equivalent:
-
(i) The outer isomorphism
 $\alpha $
is graphic (cf. Definition 2.7(ii)).
$\alpha $
is graphic (cf. Definition 2.7(ii)). -
(ii) The outer isomorphism
 $\alpha $
is group-theoretically verticial and group-theoretically cuspidal (cf. Definition 2.7(i)).
$\alpha $
is group-theoretically verticial and group-theoretically cuspidal (cf. Definition 2.7(i)). -
(iii) The outer isomorphism
 $\alpha $
is group-theoretically nodal and group-theoretically cuspidal (cf. Definition 2.7(i)).
$\alpha $
is group-theoretically nodal and group-theoretically cuspidal (cf. Definition 2.7(i)).
Definition 3.5. Let
![]() $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
be a degeneration structure on
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
be a degeneration structure on
![]() $\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(i)]) and
$\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(i)]) and
![]() $e \in S$
.
$e \in S$
.
-
(i) We shall say that a closed subgroup
 $J\subseteq \Pi _{1}$
of
$J\subseteq \Pi _{1}$
of
 $\Pi _{1}$
is a cycle-subgroup of
$\Pi _{1}$
is a cycle-subgroup of
 $\Pi _{1}$
(with respect to
$\Pi _{1}$
(with respect to
 $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
, associated with
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
, associated with
 $e \in S$
) if J is contained in the
$e \in S$
) if J is contained in the
 $\Pi _{1}$
-conjugacy class of closed subgroups of
$\Pi _{1}$
-conjugacy class of closed subgroups of
 $\Pi _{1}$
obtained by forming the image of a nodal subgroup of
$\Pi _{1}$
obtained by forming the image of a nodal subgroup of
 $\Pi _{{}^{Y}\!\mathcal {G}}$
associated with e via the composite of outer isomorphisms
$\Pi _{{}^{Y}\!\mathcal {G}}$
associated with e via the composite of outer isomorphisms  $$\begin{align*}\Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\Phi^{-1}_{{}^{Y}\!\mathcal{G}_{\leadsto {S}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}_{\leadsto {S}}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}} \stackrel{\sim}{\longrightarrow} \Pi_{1} \end{align*}$$
$$\begin{align*}\Pi_{{}^{Y}\!\mathcal{G}} \stackrel{\Phi^{-1}_{{}^{Y}\!\mathcal{G}_{\leadsto {S}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}_{\leadsto {S}}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}} \stackrel{\sim}{\longrightarrow} \Pi_{1} \end{align*}$$
—where the first arrow is the inverse of the specialization outer isomorphism
 $\Phi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]), the second arrow is the graphic outer isomorphism
$\Phi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]), the second arrow is the graphic outer isomorphism
 $\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
induced by
$\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
induced by
 $\phi $
, and the third arrow is the natural outer isomorphism
$\phi $
, and the third arrow is the natural outer isomorphism
 $\Pi _{\mathcal {G}}\stackrel {\sim }{\rightarrow }\Pi _{1}$
of [Reference Hoshi and Mochizuki10, Def. 3.1(ii)] (cf. the left-hand portion of Figure 1).
$\Pi _{\mathcal {G}}\stackrel {\sim }{\rightarrow }\Pi _{1}$
of [Reference Hoshi and Mochizuki10, Def. 3.1(ii)] (cf. the left-hand portion of Figure 1). -
(ii) Let n be a positive integer. Then we shall say that a cycle-subgroup of
 $\Pi _{1}$
is n-cuspidalizable if it is a cycle-subgroup of
$\Pi _{1}$
is n-cuspidalizable if it is a cycle-subgroup of
 $\Pi _{1}$
with respect to some n-cuspidalizable degeneration structure on
$\Pi _{1}$
with respect to some n-cuspidalizable degeneration structure on
 $\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(v)]).
$\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(v)]).
Remark 3.5.1. Let
![]() $J\subseteq \Pi _{1}$
be a cycle-subgroup of
$J\subseteq \Pi _{1}$
be a cycle-subgroup of
![]() $\Pi _{1}$
with respect to a degeneration structure
$\Pi _{1}$
with respect to a degeneration structure
![]() $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
, associated with a node
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
, associated with a node
![]() $e\in S$
. Then it follows immediately from [Reference Mochizuki18, Prop. 1.2(i)] that the node e of
$e\in S$
. Then it follows immediately from [Reference Mochizuki18, Prop. 1.2(i)] that the node e of
![]() ${}^{Y}\!\mathcal {G}$
is uniquely determined by the subgroup
${}^{Y}\!\mathcal {G}$
is uniquely determined by the subgroup
![]() $J\subseteq \Pi _{1}$
and the degeneration structure
$J\subseteq \Pi _{1}$
and the degeneration structure
![]() $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
.
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
.
Definition 3.6. Let
![]() $J \subseteq \Pi _{1}$
be a
$J \subseteq \Pi _{1}$
be a
![]() $2$
-cuspidalizable cycle-subgroup of
$2$
-cuspidalizable cycle-subgroup of
![]() $\Pi _{1}$
(cf. Definition 3.5(i) and (ii)).
$\Pi _{1}$
(cf. Definition 3.5(i) and (ii)).
-
(i) It follows immediately from the various definitions involved that we have data as follows:
-
(a) a
 $2$
-cuspidalizable degeneration structure
$2$
-cuspidalizable degeneration structure
 $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}),$
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}),$
 $\phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
on
$\phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
on
 $\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(i) and (v)]),
$\mathcal {G}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(i) and (v)]), -
(b) an isomorphism
 ${}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{1}$
that is compatible with the composite of the display of Definition 3.5(i) (cf. also [Reference Hoshi and Mochizuki10, Def. 3.1(ii)]) in the case where we take the “
${}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{1}$
that is compatible with the composite of the display of Definition 3.5(i) (cf. also [Reference Hoshi and Mochizuki10, Def. 3.1(ii)]) in the case where we take the “
 $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
” of Definition 3.5 to be the degeneration structure of (a),
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
” of Definition 3.5 to be the degeneration structure of (a), -
(c) a PFC-admissible isomorphism
 ${}^{Y}\!\Pi _{2} \stackrel {\sim }{\rightarrow } \Pi _{2}$
that lifts the isomorphism of (b), and
${}^{Y}\!\Pi _{2} \stackrel {\sim }{\rightarrow } \Pi _{2}$
that lifts the isomorphism of (b), and -
(d) a nodal subgroup
 $\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}$
of
$\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}$
of
 $ {}^{Y}\!\Pi _{1}$
associated with a (uniquely determined—cf. Remark 3.5.1) node e of
$ {}^{Y}\!\Pi _{1}$
associated with a (uniquely determined—cf. Remark 3.5.1) node e of
 ${}^{Y}\!\mathcal {G}$
${}^{Y}\!\mathcal {G}$
such that the image of the nodal subgroup
 $\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}$
of (d) via the isomorphism
$\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}$
of (d) via the isomorphism
 ${}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{1}$
of (b) coincides with
${}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{1}$
of (b) coincides with
 $J \subseteq \Pi _{1}$
. We shall say that a closed subgroup
$J \subseteq \Pi _{1}$
. We shall say that a closed subgroup
 $T \subseteq \Pi _{2/1}$
of
$T \subseteq \Pi _{2/1}$
of
 $\Pi _{2/1}$
is a tripodal subgroup associated with J if T coincides—relative to some choice of data (a), (b), (c), (d) as above (but cf. also Remark 3.6.1!)—with the image, via the lifting
$\Pi _{2/1}$
is a tripodal subgroup associated with J if T coincides—relative to some choice of data (a), (b), (c), (d) as above (but cf. also Remark 3.6.1!)—with the image, via the lifting
 ${}^{Y}\!\Pi _{2} \stackrel {\sim }{\rightarrow } \Pi _{2}$
of (c), of some
${}^{Y}\!\Pi _{2} \stackrel {\sim }{\rightarrow } \Pi _{2}$
of (c), of some
 $\{1,2\}$
-tripod in
$\{1,2\}$
-tripod in
 ${}^{Y}\!\Pi _{2/1} \subseteq {}^{Y}\!\Pi _{2}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.3(i)]) arising from e (cf. [Reference Hoshi and Mochizuki10, Def. 3.7(i)]), and, moreover, the centralizer
${}^{Y}\!\Pi _{2/1} \subseteq {}^{Y}\!\Pi _{2}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.3(i)]) arising from e (cf. [Reference Hoshi and Mochizuki10, Def. 3.7(i)]), and, moreover, the centralizer
 $Z_{\Pi _{2}}(T)$
maps bijectively, via
$Z_{\Pi _{2}}(T)$
maps bijectively, via
 $p_{2/1}^\Pi \colon \Pi _{2} \twoheadrightarrow \Pi _{1}$
, onto
$p_{2/1}^\Pi \colon \Pi _{2} \twoheadrightarrow \Pi _{1}$
, onto
 $J\subseteq \Pi _{1}$
(cf. [Reference Hoshi and Mochizuki10, Lem. 3.11(iv) and (vii)]).
$J\subseteq \Pi _{1}$
(cf. [Reference Hoshi and Mochizuki10, Lem. 3.11(iv) and (vii)]). -
-
(ii) Let
 $T \subseteq \Pi _{2/1}$
be a tripodal subgroup associated with J (cf. (i)). Then we shall refer to a closed subgroup of T that arises from a nodal (resp. cuspidal) subgroup contained in the
$T \subseteq \Pi _{2/1}$
be a tripodal subgroup associated with J (cf. (i)). Then we shall refer to a closed subgroup of T that arises from a nodal (resp. cuspidal) subgroup contained in the
 $\{1,2\}$
-tripod in
$\{1,2\}$
-tripod in
 ${}^{Y}\!\Pi _{2/1} \subseteq {}^{Y}\!\Pi _{2}$
of (i) as a lifting cycle-subgroup (resp. distinguished cuspidal subgroup) of T (cf. the right-hand portion of Figure 1).
${}^{Y}\!\Pi _{2/1} \subseteq {}^{Y}\!\Pi _{2}$
of (i) as a lifting cycle-subgroup (resp. distinguished cuspidal subgroup) of T (cf. the right-hand portion of Figure 1).
Remark 3.6.1. Note that, in the situation of Definition 3.6, (i), it follows immediately from Lemma 3.1(ii) (i.e., by considering the generization of
![]() ${}^{Y}\!\mathcal {G}$
with respect to
${}^{Y}\!\mathcal {G}$
with respect to
![]() $\mathrm {Node}({}^{Y}\!\mathcal {G}) \setminus \{e\}$
—cf. [Reference Hoshi and Mochizuki9, Def. 2.8]), together with the computation of the centralizer given in [Reference Hoshi and Mochizuki10, Lem. 3.11(vii)], and the commensurable terminality of
$\mathrm {Node}({}^{Y}\!\mathcal {G}) \setminus \{e\}$
—cf. [Reference Hoshi and Mochizuki9, Def. 2.8]), together with the computation of the centralizer given in [Reference Hoshi and Mochizuki10, Lem. 3.11(vii)], and the commensurable terminality of
![]() $J\subseteq \Pi _{1}$
(cf. [Reference Mochizuki18, Prop. 1.2(ii)]), that the
$J\subseteq \Pi _{1}$
(cf. [Reference Mochizuki18, Prop. 1.2(ii)]), that the
![]() $\Pi _{2/1}$
-conjugacy class of a tripodal subgroup T is completely determined by the cycle-subgroup
$\Pi _{2/1}$
-conjugacy class of a tripodal subgroup T is completely determined by the cycle-subgroup
![]() $J\subseteq \Pi _{1}$
.
$J\subseteq \Pi _{1}$
.
Remark 3.6.2.
-
(i) Suppose that we are in the situation of Definition 3.5(i). Recall the module
 $\Lambda _{\mathcal {G}}$
, that is, the cyclotome associated with
$\Lambda _{\mathcal {G}}$
, that is, the cyclotome associated with
 $\mathcal {G}$
, defined in [Reference Hoshi and Mochizuki9, Def. 3.8(i)]. Thus, as an abstract module,
$\mathcal {G}$
, defined in [Reference Hoshi and Mochizuki9, Def. 3.8(i)]. Thus, as an abstract module,
 $\Lambda _{\mathcal {G}}$
is isomorphic to the pro-
$\Lambda _{\mathcal {G}}$
is isomorphic to the pro-
 $\Sigma $
completion
$\Sigma $
completion
 $\widehat {\mathbb {Z}}^{\Sigma }$
of
$\widehat {\mathbb {Z}}^{\Sigma }$
of
 $\mathbb {Z}$
. Recall, furthermore, from [Reference Hoshi and Mochizuki9, Cor. 3.9(v) and (vi)] that one may construct a natural, functorial
$\mathbb {Z}$
. Recall, furthermore, from [Reference Hoshi and Mochizuki9, Cor. 3.9(v) and (vi)] that one may construct a natural, functorial
 $\{ \pm \}$
-orbit of isomorphisms
$\{ \pm \}$
-orbit of isomorphisms  $$ \begin{align*}\Pi_e \stackrel{\sim}{\longrightarrow} \Lambda_{{}^{Y}\!\mathcal{G}} \end{align*} $$
$$ \begin{align*}\Pi_e \stackrel{\sim}{\longrightarrow} \Lambda_{{}^{Y}\!\mathcal{G}} \end{align*} $$
—where
 $\Pi _e \subseteq {}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!\mathcal {G}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(ii)]) denotes a nodal subgroup associated with e. Thus, by applying the natural, functorial (outer) isomorphisms
$\Pi _e \subseteq {}^{Y}\!\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{{}^{Y}\!\mathcal {G}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.1(ii)]) denotes a nodal subgroup associated with e. Thus, by applying the natural, functorial (outer) isomorphisms
 $\Lambda _{{}^{Y}\!\mathcal {G}} \stackrel {\sim }{\rightarrow } \Lambda _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}$
(cf. [Reference Hoshi and Mochizuki9, Cor. 3.9(i)]) and
$\Lambda _{{}^{Y}\!\mathcal {G}} \stackrel {\sim }{\rightarrow } \Lambda _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}$
(cf. [Reference Hoshi and Mochizuki9, Cor. 3.9(i)]) and
 $\Phi ^{-1}_{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}\colon \Pi _{{}^{Y}\!\mathcal {G}} \stackrel {\sim }{\rightarrow }\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]), together with the (outer) isomorphisms
$\Phi ^{-1}_{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}\colon \Pi _{{}^{Y}\!\mathcal {G}} \stackrel {\sim }{\rightarrow }\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}$
(cf. [Reference Hoshi and Mochizuki9, Def. 2.10]), together with the (outer) isomorphisms
 $\Lambda _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
and
$\Lambda _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
and
 $\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
induced by
$\Pi _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
induced by
 $\phi $
, we obtain a natural
$\phi $
, we obtain a natural
 $\{ \pm \}$
-orbit of isomorphisms associated with the cycle-subgroup
$\{ \pm \}$
-orbit of isomorphisms associated with the cycle-subgroup $$ \begin{align*}J\stackrel{\sim}{\longrightarrow} \Lambda_{\mathcal{G}} \end{align*} $$
$$ \begin{align*}J\stackrel{\sim}{\longrightarrow} \Lambda_{\mathcal{G}} \end{align*} $$
 $J\subseteq \Pi _1$
. Note that this
$J\subseteq \Pi _1$
. Note that this
 $\{ \pm \}$
-orbit of isomorphisms is functorial with respect to automorphisms
$\{ \pm \}$
-orbit of isomorphisms is functorial with respect to automorphisms
 $\alpha $
of
$\alpha $
of
 $\Pi _1$
such that
$\Pi _1$
such that
 $\alpha (J) = J$
, and, moreover, the outer automorphism of
$\alpha (J) = J$
, and, moreover, the outer automorphism of
 $\Pi _{\mathcal {G}}$
obtained by forming the conjugate of
$\Pi _{\mathcal {G}}$
obtained by forming the conjugate of
 $\alpha $
by the natural outer isomorphism
$\alpha $
by the natural outer isomorphism
 $\Pi _1 \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
is graphic (cf. the equivalence (i)
$\Pi _1 \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
is graphic (cf. the equivalence (i)
 $\Leftrightarrow $
(iii) of Theorem 3.3). In this context, it is natural to refer to either of the two isomorphisms in this
$\Leftrightarrow $
(iii) of Theorem 3.3). In this context, it is natural to refer to either of the two isomorphisms in this
 $\{ \pm \}$
-orbit as an orientation on the cycle-subgroup J.
$\{ \pm \}$
-orbit as an orientation on the cycle-subgroup J.
-
(ii) Now suppose that we are in the situation of Definition 3.6(i) and (ii). Then let us observe that the natural outer surjection
 ${}^{Y}\!\Pi _{2/1} \twoheadrightarrow {}^{Y}\!\Pi _{\{2\}} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{1}$
determined by
${}^{Y}\!\Pi _{2/1} \twoheadrightarrow {}^{Y}\!\Pi _{\{2\}} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{1}$
determined by
 ${}^{Y}\!p_{\{1,2\}/\{2\}}^\Pi $
induces a natural isomorphism
${}^{Y}\!p_{\{1,2\}/\{2\}}^\Pi $
induces a natural isomorphism  $$ \begin{align*}\Lambda_{{}^{Y}\!\mathcal{G}_{2\in \{ 1,2\}, e_2}}\stackrel{\sim}{\longrightarrow} \Lambda_{{}^{Y}\!\mathcal{G}} \end{align*} $$
$$ \begin{align*}\Lambda_{{}^{Y}\!\mathcal{G}_{2\in \{ 1,2\}, e_2}}\stackrel{\sim}{\longrightarrow} \Lambda_{{}^{Y}\!\mathcal{G}} \end{align*} $$
(cf. [Reference Hoshi and Mochizuki9, Cor. 3.9(ii)]), where we write
 $e_2 \in Y_2(k)$
for a k-valued point of
$e_2 \in Y_2(k)$
for a k-valued point of
 $Y_2$
that lies, relative to
$Y_2$
that lies, relative to
 ${}^{Y}\!p_{2/1}^{\mathrm {log}}$
, over the k-valued point of Y determined by the node e. Write v for the vertex of
${}^{Y}\!p_{2/1}^{\mathrm {log}}$
, over the k-valued point of Y determined by the node e. Write v for the vertex of
 ${}^{Y}\!\mathcal {G}_{2\in \{1,2\}, e_2}$
that gives rise to the tripodal subgroup
${}^{Y}\!\mathcal {G}_{2\in \{1,2\}, e_2}$
that gives rise to the tripodal subgroup
 $T\subseteq \Pi _{2/1}$
. Thus, we have a natural isomorphism
$T\subseteq \Pi _{2/1}$
. Thus, we have a natural isomorphism  $$ \begin{align*}\Lambda_v\stackrel{\sim}{\longrightarrow} \Lambda_{{}^{Y}\!\mathcal{G}_{2\in \{1,2\}, e_2}} \end{align*} $$
$$ \begin{align*}\Lambda_v\stackrel{\sim}{\longrightarrow} \Lambda_{{}^{Y}\!\mathcal{G}_{2\in \{1,2\}, e_2}} \end{align*} $$
(cf. [Reference Hoshi and Mochizuki9, Cor. 3.9(ii)]). Now suppose that
 $e^*$
is a node of
$e^*$
is a node of
 ${}^{Y}\!\mathcal {G}_{2\in \{ 1,2\}, e_2}$
that abuts to v and, moreover, gives rise to a lifting cycle-subgroup
${}^{Y}\!\mathcal {G}_{2\in \{ 1,2\}, e_2}$
that abuts to v and, moreover, gives rise to a lifting cycle-subgroup
 $J^*\subseteq T$
of the tripodal subgroup T. Thus, one verifies immediately that the natural outer surjection
$J^*\subseteq T$
of the tripodal subgroup T. Thus, one verifies immediately that the natural outer surjection
 $\Pi _{2/1} \twoheadrightarrow \Pi _{\{2\}} \stackrel {\sim }{\rightarrow } \Pi _1$
determined by
$\Pi _{2/1} \twoheadrightarrow \Pi _{\{2\}} \stackrel {\sim }{\rightarrow } \Pi _1$
determined by
 $p^\Pi _{ \{ 1,2\}/\{ 2\}}$
induces a natural isomorphism
$p^\Pi _{ \{ 1,2\}/\{ 2\}}$
induces a natural isomorphism
 $J^*\stackrel {\sim }{\rightarrow } J$
(cf. [Reference Hoshi and Mochizuki10, Lem. 3.6(iv)]). Let
$J^*\stackrel {\sim }{\rightarrow } J$
(cf. [Reference Hoshi and Mochizuki10, Lem. 3.6(iv)]). Let
 $\Pi _{e^*}\subseteq \Pi _{{}^{Y}\!\mathcal {G}_{2\in \{ 1,2\}, e_2}}$
be a nodal subgroup associated with
$\Pi _{e^*}\subseteq \Pi _{{}^{Y}\!\mathcal {G}_{2\in \{ 1,2\}, e_2}}$
be a nodal subgroup associated with
 $e^*$
. Then the (unique!) branch of
$e^*$
. Then the (unique!) branch of
 $e^*$
that abuts to v determines a natural isomorphism
$e^*$
that abuts to v determines a natural isomorphism  $$ \begin{align*}\Pi_{e^*}\stackrel{\sim}{\longrightarrow} \Lambda_v \end{align*} $$
$$ \begin{align*}\Pi_{e^*}\stackrel{\sim}{\longrightarrow} \Lambda_v \end{align*} $$
(cf. [Reference Hoshi and Mochizuki9, Cor. 3.9(v)]). Thus, by composing the isomorphisms of the last three displays with the isomorphism
 $\Lambda _{{}^{Y}\!\mathcal {G}} \stackrel {\sim }{\rightarrow } \Lambda _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}\stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
discussed in (i) and the inverse of the tautological isomorphism
$\Lambda _{{}^{Y}\!\mathcal {G}} \stackrel {\sim }{\rightarrow } \Lambda _{{}^{Y}\!\mathcal {G}_{\leadsto {S}}}\stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
discussed in (i) and the inverse of the tautological isomorphism
 $\Pi _{e^*}\stackrel {\sim }{\rightarrow } J^*$
, we obtain a natural isomorphism associated with the lifting cycle-subgroup
$\Pi _{e^*}\stackrel {\sim }{\rightarrow } J^*$
, we obtain a natural isomorphism associated with the lifting cycle-subgroup $$ \begin{align*}J^*\stackrel{\sim}{\longrightarrow} \Lambda_{\mathcal{G}} \end{align*} $$
$$ \begin{align*}J^*\stackrel{\sim}{\longrightarrow} \Lambda_{\mathcal{G}} \end{align*} $$
 $J^*\subseteq T$
. Note that this natural isomorphism is functorial with respect to FC-admissible automorphisms
$J^*\subseteq T$
. Note that this natural isomorphism is functorial with respect to FC-admissible automorphisms
 $\alpha _2$
of
$\alpha _2$
of
 $\Pi _2$
such that
$\Pi _2$
such that
 $\alpha _2(J^*) = J^*$
,
$\alpha _2(J^*) = J^*$
,
 $\alpha _2(T) = T$
, and, moreover, the outer automorphism of
$\alpha _2(T) = T$
, and, moreover, the outer automorphism of
 $\Pi _{\mathcal {G}}$
obtained by forming the conjugate, by the natural outer isomorphism
$\Pi _{\mathcal {G}}$
obtained by forming the conjugate, by the natural outer isomorphism
 $\Pi _1 \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
, of the outer automorphism of
$\Pi _1 \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
, of the outer automorphism of
 $\Pi _1$
determined by
$\Pi _1$
determined by
 $\alpha _2$
is graphic (cf. the equivalence (i)
$\alpha _2$
is graphic (cf. the equivalence (i)
 $\Leftrightarrow $
(iii) of Theorem 3.3; [Reference Hoshi and Mochizuki10, Lem. 3.11(vii)]). Finally, one verifies immediately from the construction of the isomorphisms of [Reference Hoshi and Mochizuki9, Cor. 3.9(v)] that if one composes this isomorphism
$\Leftrightarrow $
(iii) of Theorem 3.3; [Reference Hoshi and Mochizuki10, Lem. 3.11(vii)]). Finally, one verifies immediately from the construction of the isomorphisms of [Reference Hoshi and Mochizuki9, Cor. 3.9(v)] that if one composes this isomorphism
 $J^*\stackrel {\sim }{\rightarrow }\Lambda _{\mathcal {G}}$
with the inverse of the natural isomorphism
$J^*\stackrel {\sim }{\rightarrow }\Lambda _{\mathcal {G}}$
with the inverse of the natural isomorphism
 $J^*\stackrel {\sim }{\rightarrow } J$
discussed above, then the resulting isomorphism
$J^*\stackrel {\sim }{\rightarrow } J$
discussed above, then the resulting isomorphism
 $J\stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
is an orientation on the cycle-subgroup J, in the sense of the discussion of (i), and, moreover, that, if we define an orientation on the tripodal subgroup T to be a choice of a T-conjugacy class of lifting cycle-subgroups of T, then the resulting assignment
$J\stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
is an orientation on the cycle-subgroup J, in the sense of the discussion of (i), and, moreover, that, if we define an orientation on the tripodal subgroup T to be a choice of a T-conjugacy class of lifting cycle-subgroups of T, then the resulting assignment  $$ \begin{align*}\Big\{ \text{orientations on }T\Big\} \ \longrightarrow\ \Big\{ \text{orientations on }J\Big\} \end{align*} $$
$$ \begin{align*}\Big\{ \text{orientations on }T\Big\} \ \longrightarrow\ \Big\{ \text{orientations on }J\Big\} \end{align*} $$
is a bijection [between sets of cardinality
 $2$
].
$2$
].
Lemma 3.7. (Induced outomorphisms of tripods).
In the situation of Lemma 3.1, suppose that
![]() $X^{\mathrm {log}} = Y^{\mathrm {log}}$
. Write
$X^{\mathrm {log}} = Y^{\mathrm {log}}$
. Write
![]() $c \in \mathrm {Cusp}(\mathcal {G}_{2\in \{1,2\}, e_2})$
for the cusp arising from the diagonal divisor in
$c \in \mathrm {Cusp}(\mathcal {G}_{2\in \{1,2\}, e_2})$
for the cusp arising from the diagonal divisor in
![]() $X\times _k X$
. Let
$X\times _k X$
. Let
![]() $\Pi _{c} \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
be a cuspidal subgroup of
$\Pi _{c} \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
be a cuspidal subgroup of
![]() $\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
associated with c. Write
$\Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}$
associated with c. Write
(cf. Lemma 3.1(ii); [Reference Hoshi and Mochizuki10, Th. 3.16(i)]) for the result of applying the tripod homomorphism
![]() $\mathfrak {T}_{\Pi _{v}}$
to
$\mathfrak {T}_{\Pi _{v}}$
to
![]() $\alpha _2$
. (Thus, it follows immediately from Lemma 3.1(ii) that
$\alpha _2$
. (Thus, it follows immediately from Lemma 3.1(ii) that
![]() $\alpha _v\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})$
.) Suppose, moreover, that the following condition is satisfied:
$\alpha _v\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})$
.) Suppose, moreover, that the following condition is satisfied:
-
(c) The cuspidal subgroup
 $\Pi _{c} \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}\stackrel {\sim }{\leftarrow } \Pi _{2/1}$
is contained in
$\Pi _{c} \subseteq \Pi _{\mathcal {G}_{2\in \{1,2\}, e_2}}\stackrel {\sim }{\leftarrow } \Pi _{2/1}$
is contained in
 $\Pi _{v}$
.
$\Pi _{v}$
.
Then the following hold:
-
(i) Since
 $\Pi _{v}$
may be regarded as the “
$\Pi _{v}$
may be regarded as the “
 $\Pi _{1}$
” that occurs in the case where we take “
$\Pi _{1}$
” that occurs in the case where we take “
 $X^{\mathrm {log}}$
” to be the smooth log curve associated with
$X^{\mathrm {log}}$
” to be the smooth log curve associated with
 $\mathbb {P}_k^1\setminus \{0,1,\infty \}$
(cf. [Reference Hoshi and Mochizuki10, Rem. 3.3.1]), there exists a uniquely determined outomorphism of
$\mathbb {P}_k^1\setminus \{0,1,\infty \}$
(cf. [Reference Hoshi and Mochizuki10, Rem. 3.3.1]), there exists a uniquely determined outomorphism of $$ \begin{align*}\iota \in \mathrm{Out}(\Pi_{v})\end{align*} $$
$$ \begin{align*}\iota \in \mathrm{Out}(\Pi_{v})\end{align*} $$
 $\Pi _{v}$
that arises from an automorphism of
$\Pi _{v}$
that arises from an automorphism of
 $\mathbb {P}_k^1\setminus \{0,1,\infty \}$
over k and induces a nontrivial automorphism of the set
$\mathbb {P}_k^1\setminus \{0,1,\infty \}$
over k and induces a nontrivial automorphism of the set
 $\mathcal {N}(v)$
. Write if
$\mathcal {N}(v)$
. Write if $$ \begin{align*} |\alpha_v| \stackrel{\mathrm{def}}{=} \alpha_v\in \mathrm{Out}(\Pi_{v}) \quad (\mathrm{resp}. \, \, |\alpha_v| \stackrel{\mathrm{def}}{=} \iota\circ\alpha_v\in \mathrm{Out}(\Pi_{v})) \end{align*} $$
$$ \begin{align*} |\alpha_v| \stackrel{\mathrm{def}}{=} \alpha_v\in \mathrm{Out}(\Pi_{v}) \quad (\mathrm{resp}. \, \, |\alpha_v| \stackrel{\mathrm{def}}{=} \iota\circ\alpha_v\in \mathrm{Out}(\Pi_{v})) \end{align*} $$
 $\alpha _v\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
(resp.
$\alpha _v\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
(resp.
 $\not \in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
) (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(i)]). Then it holds that
$\not \in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
) (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(i)]). Then it holds that
 $|\alpha _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
.
$|\alpha _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
.
-
(ii) Let
 $\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}$
be a central
$\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}$
be a central
 $\{1,2,3\}$
-tripod of
$\{1,2,3\}$
-tripod of
 $\Pi _{3}$
(cf. [Reference Hoshi and Mochizuki10, Defs. 3.3(i) and 3.7(ii)]). Then every geometric (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(ii)]) outer isomorphism
$\Pi _{3}$
(cf. [Reference Hoshi and Mochizuki10, Defs. 3.3(i) and 3.7(ii)]). Then every geometric (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(ii)]) outer isomorphism
 $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
satisfies the following condition: let
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
satisfies the following condition: let
 $\beta \in \mathrm {Out}(\Pi _{1})\stackrel {\sim }{\rightarrow } \mathrm {Out}(\Pi _{\mathcal {G}})$
be an outomorphism of
$\beta \in \mathrm {Out}(\Pi _{1})\stackrel {\sim }{\rightarrow } \mathrm {Out}(\Pi _{\mathcal {G}})$
be an outomorphism of
 $\Pi _{1}\stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
that is group-theoretically nodal and 3-cuspidalizable, that is,
$\Pi _{1}\stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
that is group-theoretically nodal and 3-cuspidalizable, that is,
 $\beta \in \mathrm {Out}(\Pi _{1})$
arises from a(n) (uniquely determined—cf. [Reference Hoshi and Mochizuki8, Th. B]) FC-admissible outomorphism
$\beta \in \mathrm {Out}(\Pi _{1})$
arises from a(n) (uniquely determined—cf. [Reference Hoshi and Mochizuki8, Th. B]) FC-admissible outomorphism
 $\beta _3\in \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
. Then the image
$\beta _3\in \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})$
. Then the image  $$\begin{align*}\mathfrak{T}_{\Pi_{\mathrm{tpd}}}(\beta_3) \in \mathrm{Out}(\Pi_{\mathrm{tpd}})\end{align*}$$
$$\begin{align*}\mathfrak{T}_{\Pi_{\mathrm{tpd}}}(\beta_3) \in \mathrm{Out}(\Pi_{\mathrm{tpd}})\end{align*}$$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]) coincides—relative to the outer isomorphism
 $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
under consideration—with
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
under consideration—with  $$\begin{align*}|\beta_v|\in \mathrm{Out}(\Pi_{v})\end{align*}$$
$$\begin{align*}|\beta_v|\in \mathrm{Out}(\Pi_{v})\end{align*}$$
(cf. (i)), where we write
 $\beta _v\stackrel {\mathrm {def}}{=} \mathfrak {T}_{\Pi _{v}}(\beta _3) \in \mathrm {Out}(\Pi _{v})$
. In particular, it holds that
$\beta _v\stackrel {\mathrm {def}}{=} \mathfrak {T}_{\Pi _{v}}(\beta _3) \in \mathrm {Out}(\Pi _{v})$
. In particular, it holds that
 $|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v}) ^{\Delta +}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.4(i)]).
$|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v}) ^{\Delta +}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.4(i)]).
Proof. Assertion (i) follows immediately from the various definitions involved. Next, we verify assertion (ii). Let us first observe that the inclusion
![]() $|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^\Delta $
follows immediately from the coincidence of
$|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^\Delta $
follows immediately from the coincidence of
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
![]() $|\beta _v|$
, relative to some specific geometric outer isomorphism
$|\beta _v|$
, relative to some specific geometric outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
, together with the second displayed equality of [Reference Hoshi and Mochizuki10, Th. 3.16(v)]. The inclusion
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
, together with the second displayed equality of [Reference Hoshi and Mochizuki10, Th. 3.16(v)]. The inclusion
![]() $|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\Delta +}$
then follows from [Reference Hoshi and Mochizuki10, Lem. 3.5]; [Reference Hoshi and Mochizuki10, Th. 3.17(i)] (applied in the case where we take the “
$|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\Delta +}$
then follows from [Reference Hoshi and Mochizuki10, Lem. 3.5]; [Reference Hoshi and Mochizuki10, Th. 3.17(i)] (applied in the case where we take the “
![]() $(\Pi _2, T, T')$
” of [Reference Hoshi and Mochizuki10, Th. 3.17(i)] to be
$(\Pi _2, T, T')$
” of [Reference Hoshi and Mochizuki10, Th. 3.17(i)] to be
![]() $(\Pi _{3/1}, \Pi _v, \Pi _{\mathrm {tpd}})$
). Moreover, it follows immediately from the various definitions involved that the inclusion
$(\Pi _{3/1}, \Pi _v, \Pi _{\mathrm {tpd}})$
). Moreover, it follows immediately from the various definitions involved that the inclusion
![]() $|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^\Delta $
allows one to conclude that the coincidence of
$|\beta _v|\in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^\Delta $
allows one to conclude that the coincidence of
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
![]() $|\beta _v|$
, relative to some specific geometric outer isomorphism
$|\beta _v|$
, relative to some specific geometric outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
, implies the coincidence of
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
, implies the coincidence of
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
![]() $|\beta _v|$
, relative to an arbitrary geometric outer isomorphism
$|\beta _v|$
, relative to an arbitrary geometric outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
. Thus, to complete the verification of assertion (ii), it suffices to verify the coincidence of
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
. Thus, to complete the verification of assertion (ii), it suffices to verify the coincidence of
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3)$
with
![]() $|\beta _v|$
, relative to the specific geometric outer isomorphism
$|\beta _v|$
, relative to the specific geometric outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
whose existence is guaranteed by [Reference Hoshi and Mochizuki10, Th. 3.18(ii)]. In the following discussion, we fix this specific geometric outer isomorphism
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
whose existence is guaranteed by [Reference Hoshi and Mochizuki10, Th. 3.18(ii)]. In the following discussion, we fix this specific geometric outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
.
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \Pi _{v}$
.
Next, let us observe that if
![]() $\beta _v = |\beta _v|$
, that is,
$\beta _v = |\beta _v|$
, that is,
![]() $\beta _v \in \mathrm {Out}^{\mathrm {C}} (\Pi _{v})^{\mathrm {cusp}}$
, then it follows immediately from [Reference Hoshi and Mochizuki10, Ths. 3.16(v) and 3.18(ii)] that
$\beta _v \in \mathrm {Out}^{\mathrm {C}} (\Pi _{v})^{\mathrm {cusp}}$
, then it follows immediately from [Reference Hoshi and Mochizuki10, Ths. 3.16(v) and 3.18(ii)] that
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3) \in \mathrm {Out}(\Pi _{\mathrm {tpd}})$
coincides with
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta _3) \in \mathrm {Out}(\Pi _{\mathrm {tpd}})$
coincides with
![]() $|\beta _v|\in \mathrm {Out}(\Pi _{v})$
. Thus, to complete the verification of assertion (ii), we may assume without loss of generality that
$|\beta _v|\in \mathrm {Out}(\Pi _{v})$
. Thus, to complete the verification of assertion (ii), we may assume without loss of generality that
![]() $\beta _v \neq |\beta _v|$
, that is, that
$\beta _v \neq |\beta _v|$
, that is, that
![]() $\beta _v \not \in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
. Then let us observe that collections of data consisting of smooth log curves that (by gluing at prescribed cusps) give rise to a stable log curve whose associated semi-graph of anabelioids (of pro-
$\beta _v \not \in \mathrm {Out}^{\mathrm {C}}(\Pi _{v})^{\mathrm {cusp}}$
. Then let us observe that collections of data consisting of smooth log curves that (by gluing at prescribed cusps) give rise to a stable log curve whose associated semi-graph of anabelioids (of pro-
![]() $\Sigma $
PSC-type) is isomorphic to
$\Sigma $
PSC-type) is isomorphic to
![]() $\mathcal {G}$
may be parametrized by a smooth, connected moduli stack. Thus, one verifies easily that, by considering the étale fundamental groupoid of this moduli stack, together with a suitable scheme-theoretic automorphism of order
$\mathcal {G}$
may be parametrized by a smooth, connected moduli stack. Thus, one verifies easily that, by considering the étale fundamental groupoid of this moduli stack, together with a suitable scheme-theoretic automorphism of order
![]() $2$
of a collection of data parametrized by this moduli stack, one obtains a 3-cuspidalizable automorphism
$2$
of a collection of data parametrized by this moduli stack, one obtains a 3-cuspidalizable automorphism
![]() $\xi \in \mathrm {Aut}(\mathcal {G})$
(
$\xi \in \mathrm {Aut}(\mathcal {G})$
(
![]() $\hookrightarrow \mathrm {Out}(\Pi _{\mathcal {G}})$
) of
$\hookrightarrow \mathrm {Out}(\Pi _{\mathcal {G}})$
) of
![]() $\mathcal {G}$
such that
$\mathcal {G}$
such that
![]() $\xi _v$
(i.e., the “
$\xi _v$
(i.e., the “
![]() $\alpha _v$
” that occurs in the case where we take “
$\alpha _v$
” that occurs in the case where we take “
![]() $\alpha $
” to be
$\alpha $
” to be
![]() $\xi $
) coincides with
$\xi $
) coincides with
![]() $\iota $
. Thus, by applying the portion of assertion (ii) that has already been verified to
$\iota $
. Thus, by applying the portion of assertion (ii) that has already been verified to
![]() $\xi \circ \beta $
, we conclude that, to complete the verification of assertion (ii), it suffices to verify that
$\xi \circ \beta $
, we conclude that, to complete the verification of assertion (ii), it suffices to verify that
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\xi _3) = 1$
. On the other hand, this follows immediately from the fact that
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\xi _3) = 1$
. On the other hand, this follows immediately from the fact that
![]() $\xi $
was assumed to arise from a scheme-theoretic automorphism (cf. also [Reference Hoshi and Mochizuki10, Th. 3.16(v)]). This completes the proof of assertion (ii) and hence of Lemma 3.7.
$\xi $
was assumed to arise from a scheme-theoretic automorphism (cf. also [Reference Hoshi and Mochizuki10, Th. 3.16(v)]). This completes the proof of assertion (ii) and hence of Lemma 3.7.
Definition 3.8. Let
![]() $J\subseteq \Pi _{1}$
be a
$J\subseteq \Pi _{1}$
be a
![]() $2$
-cuspidalizable cycle-subgroup (cf. Definition 3.5(i) and (ii)); let us fix associated data as in Definition 3.6(i.a)–(i.d). Relative to these data, suppose that
$2$
-cuspidalizable cycle-subgroup (cf. Definition 3.5(i) and (ii)); let us fix associated data as in Definition 3.6(i.a)–(i.d). Relative to these data, suppose that
![]() $T \subseteq \Pi _{2/1}$
is a tripodal subgroup associated with
$T \subseteq \Pi _{2/1}$
is a tripodal subgroup associated with
![]() $J \subseteq \Pi _{1}$
(cf. Definition 3.6(i)), and that
$J \subseteq \Pi _{1}$
(cf. Definition 3.6(i)), and that
![]() $I\subseteq T$
is a distinguished cuspidal subgroup of T (cf. Definition 3.6(ii)). Note that these data, together with the log scheme structure of
$I\subseteq T$
is a distinguished cuspidal subgroup of T (cf. Definition 3.6(ii)). Note that these data, together with the log scheme structure of
![]() $Y^{\mathrm {log}}$
, allow one to speak of geometric (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(ii)]) outomorphisms of T. Then one verifies easily that there exists a uniquely determined nontrivial geometric outomorphism of T that preserves the T-conjugacy class of I. Thus, since I is commensurably terminal in T (cf. [Reference Mochizuki18, Prop. 1.2(ii)]), there exists a uniquely determined I-conjugacy class of automorphisms of T that lifts this outomorphism and preserves
$Y^{\mathrm {log}}$
, allow one to speak of geometric (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(ii)]) outomorphisms of T. Then one verifies easily that there exists a uniquely determined nontrivial geometric outomorphism of T that preserves the T-conjugacy class of I. Thus, since I is commensurably terminal in T (cf. [Reference Mochizuki18, Prop. 1.2(ii)]), there exists a uniquely determined I-conjugacy class of automorphisms of T that lifts this outomorphism and preserves
![]() $I\subseteq T$
. We shall refer to this I-conjugacy class of automorphisms of T as the cycle symmetry associated with I.
$I\subseteq T$
. We shall refer to this I-conjugacy class of automorphisms of T as the cycle symmetry associated with I.
Before proceeding, we pause to observe the following interesting “alternative formulation” of the essential content of Lemma 3.7(ii).
Lemma 3.9 (Geometricity of conjugates of geometric outer isomorphisms).
Suppose that we are in the situation of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)], that is,
![]() $n\geq 3$
, and T (resp.
$n\geq 3$
, and T (resp.
![]() $T'$
) is an E- (resp.
$T'$
) is an E- (resp.
![]() $E'$
-) tripod of
$E'$
-) tripod of
![]() $\Pi _{n}$
for some subset
$\Pi _{n}$
for some subset
![]() $E \subseteq \{1, \dots , n\}$
(resp.
$E \subseteq \{1, \dots , n\}$
(resp.
![]() $E'\subseteq \{1, \dots , n\}$
). Let
$E'\subseteq \{1, \dots , n\}$
). Let
![]() $\phi \colon T \stackrel {\sim }{\rightarrow } T'$
be a geometric (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(ii)]) outer isomorphism. Then, for every
$\phi \colon T \stackrel {\sim }{\rightarrow } T'$
be a geometric (cf. [Reference Hoshi and Mochizuki10, Def. 3.4(ii)]) outer isomorphism. Then, for every
![]() $\alpha \in \mathrm {Out}^{\mathrm {FC}}(\Pi _{n}) [T,T': \{|C|\}]$
, the composite of outer isomorphisms
$\alpha \in \mathrm {Out}^{\mathrm {FC}}(\Pi _{n}) [T,T': \{|C|\}]$
, the composite of outer isomorphisms
(cf. [Reference Hoshi and Mochizuki10, Th. 3.16(i)]) is equal to
![]() $\phi $
.
$\phi $
.
Proof. Let us first observe that the validity of Lemma 3.9 for some specific geometric outer isomorphism “
![]() $\phi $
” follows formally from the commutative diagram of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)]. Thus, the validity of Lemma 3.9 for an arbitrary geometric outer isomorphism “
$\phi $
” follows formally from the commutative diagram of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)]. Thus, the validity of Lemma 3.9 for an arbitrary geometric outer isomorphism “
![]() $\phi $
” follows immediately from the equality of the first display of [Reference Hoshi and Mochizuki10, Th. 3.18(i)], that is, the fact that
$\phi $
” follows immediately from the equality of the first display of [Reference Hoshi and Mochizuki10, Th. 3.18(i)], that is, the fact that
![]() $\mathfrak {T}_T(\alpha )$
commutes with arbitrary geometric outomorphisms of T. This completes the proof of Lemma 3.9.
$\mathfrak {T}_T(\alpha )$
commutes with arbitrary geometric outomorphisms of T. This completes the proof of Lemma 3.9.
Remark 3.9.1. One verifies immediately that a similar argument to the argument applied in the proof of Lemma 3.9 yields evident analogues of Lemma 3.9 in the respective situations of [Reference Hoshi and Mochizuki10, Th. 3.17(i) and (ii)].
Theorem 3.10 (Canonical liftings of cycles).
In the notation of the discussion at the beginning of the present §3, let
![]() $I \subseteq \Pi _{2/1} \subseteq \Pi _{2}$
be a cuspidal inertia group associated with the diagonal cusp of a fiber of
$I \subseteq \Pi _{2/1} \subseteq \Pi _{2}$
be a cuspidal inertia group associated with the diagonal cusp of a fiber of
![]() $p_{2/1}^{\mathrm {log}}$
;
$p_{2/1}^{\mathrm {log}}$
;
![]() $\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}$
a
$\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}$
a
![]() $3$
-central
$3$
-central
![]() $\{1,2,3\}$
-tripod of
$\{1,2,3\}$
-tripod of
![]() $\Pi _{3}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]);
$\Pi _{3}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.7(ii)]);
![]() $I_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
a cuspidal subgroup of
$I_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
a cuspidal subgroup of
![]() $\Pi _{\mathrm {tpd}}$
that does not arise from a cusp of a fiber of
$\Pi _{\mathrm {tpd}}$
that does not arise from a cusp of a fiber of
![]() $p_{3/2}^{\mathrm {log}}$
;
$p_{3/2}^{\mathrm {log}}$
;
![]() $J^{*}_{\mathrm {tpd}}$
,
$J^{*}_{\mathrm {tpd}}$
,
![]() $J^{**}_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
cuspidal subgroups of
$J^{**}_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
cuspidal subgroups of
![]() $\Pi _{\mathrm {tpd}}$
such that
$\Pi _{\mathrm {tpd}}$
such that
![]() $I_{\mathrm {tpd}}$
,
$I_{\mathrm {tpd}}$
,
![]() $J^{*}_{\mathrm {tpd}}$
, and
$J^{*}_{\mathrm {tpd}}$
, and
![]() $J^{**}_{\mathrm {tpd}}$
determine three distinct
$J^{**}_{\mathrm {tpd}}$
determine three distinct
![]() $\Pi _{\mathrm {tpd}}$
-conjugacy classes of closed subgroups of
$\Pi _{\mathrm {tpd}}$
-conjugacy classes of closed subgroups of
![]() $\Pi _{\mathrm {tpd}}$
. (Note that one verifies immediately from the various definitions involved that such cuspidal subgroups
$\Pi _{\mathrm {tpd}}$
. (Note that one verifies immediately from the various definitions involved that such cuspidal subgroups
![]() $I_{\mathrm {tpd}}$
,
$I_{\mathrm {tpd}}$
,
![]() $J^{*}_{\mathrm {tpd}}$
, and
$J^{*}_{\mathrm {tpd}}$
, and
![]() $J^{**}_{\mathrm {tpd}}$
always exist.) For positive integers
$J^{**}_{\mathrm {tpd}}$
always exist.) For positive integers
![]() $n\geq 2$
,
$n\geq 2$
,
![]() $m \leq n$
, and
$m \leq n$
, and
![]() $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
(cf. [Reference Mochizuki20, Def. 1.1(ii)]), write
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
(cf. [Reference Mochizuki20, Def. 1.1(ii)]), write
for the automorphism of
![]() $\Pi _{m}$
determined by
$\Pi _{m}$
determined by
![]() $\alpha $
;
$\alpha $
;
for the subgroup consisting of
![]() $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that
![]() $\beta _{2}(I) = I$
;
$\beta _{2}(I) = I$
;
for the subgroup consisting of
![]() $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that the image of
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n})$
such that the image of
![]() $\beta $
via the composite
$\beta $
via the composite
![]() $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}) \twoheadrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{n}) \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1}) \rightarrow \mathrm {Out}(\Pi _{\mathcal {G}})$
—where the second arrow is the natural injection of [Reference Hoshi and Mochizuki8, Th. B] and the third arrow is the homomorphism induced by the natural outer isomorphism
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}) \twoheadrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{n}) \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1}) \rightarrow \mathrm {Out}(\Pi _{\mathcal {G}})$
—where the second arrow is the natural injection of [Reference Hoshi and Mochizuki8, Th. B] and the third arrow is the homomorphism induced by the natural outer isomorphism
![]() $\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
—is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]);
$\Pi _{1} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}}$
—is graphic (cf. [Reference Mochizuki18, Def. 1.4(i)]);
for the set of n-cuspidalizable cycle-subgroups of
![]() $\Pi _{1}$
(cf. Definition 3.5(i) and (ii));
$\Pi _{1}$
(cf. Definition 3.5(i) and (ii));
for the set of closed subgroups
![]() $T \subseteq \Pi _{2/1}$
such that T is a tripodal subgroup associated with some
$T \subseteq \Pi _{2/1}$
such that T is a tripodal subgroup associated with some
![]() $2$
-cuspidalizable cycle-subgroup of
$2$
-cuspidalizable cycle-subgroup of
![]() $\Pi _{1}$
(cf. Definition 3.6(i)), and, moreover, I is a distinguished cuspidal subgroup (cf. Definition 3.6(ii)) of T. Then the following hold:
$\Pi _{1}$
(cf. Definition 3.6(i)), and, moreover, I is a distinguished cuspidal subgroup (cf. Definition 3.6(ii)) of T. Then the following hold:
-
(i) Let
 $n\geq 2$
be a positive integer,
$n\geq 2$
be a positive integer,
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
,
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
,
 $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
, and
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
, and
 $T \in \mathrm {Tpd}_I(\Pi _{2/1})$
. Then it holds that Thus,
$T \in \mathrm {Tpd}_I(\Pi _{2/1})$
. Then it holds that Thus, $$ \begin{align*}\alpha_1(J)\in \mathrm{Cycle}^{n}(\Pi_{1}) , \quad \alpha_2(T) \in \mathrm{Tpd}_I(\Pi_{2/1}) .\end{align*} $$
$$ \begin{align*}\alpha_1(J)\in \mathrm{Cycle}^{n}(\Pi_{1}) , \quad \alpha_2(T) \in \mathrm{Tpd}_I(\Pi_{2/1}) .\end{align*} $$
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
acts naturally on
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
acts naturally on
 $\mathrm {Cycle}^{n}(\Pi _{1})$
,
$\mathrm {Cycle}^{n}(\Pi _{1})$
,
 $\mathrm {Tpd}_I(\Pi _{2/1})$
.
$\mathrm {Tpd}_I(\Pi _{2/1})$
.
-
(ii) Let
 $n\geq 2$
be a positive integer. Then there exists a unique
$n\geq 2$
be a positive integer. Then there exists a unique
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariant (cf. (i)) map such that, for every
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
-equivariant (cf. (i)) map such that, for every $$ \begin{align*}\mathfrak{C}_I \colon \mathrm{Cycle}^{n}(\Pi_{1}) \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1}) \end{align*} $$
$$ \begin{align*}\mathfrak{C}_I \colon \mathrm{Cycle}^{n}(\Pi_{1}) \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1}) \end{align*} $$
 $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
,
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
,
 $\mathfrak {C}_I(J)$
is a tripodal subgroup associated with J. Moreover, for every
$\mathfrak {C}_I(J)$
is a tripodal subgroup associated with J. Moreover, for every
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
and
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
and
 $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
, the isomorphism
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
, the isomorphism
 $\mathfrak {C}_I(J) \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(\alpha _1(J))$
induced by
$\mathfrak {C}_I(J) \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(\alpha _1(J))$
induced by
 $\alpha _2$
maps every lifting cycle-subgroup (cf. Definition 3.6(ii)) of
$\alpha _2$
maps every lifting cycle-subgroup (cf. Definition 3.6(ii)) of
 $\mathfrak {C}_I(J)$
bijectively onto a lifting cycle-subgroup of
$\mathfrak {C}_I(J)$
bijectively onto a lifting cycle-subgroup of
 $\mathfrak {C}_I(\alpha _1(J))$
.
$\mathfrak {C}_I(\alpha _1(J))$
.
-
(iii) Let
 $n\geq 3$
be a positive integer. Then there exists an assignment
$n\geq 3$
be a positive integer. Then there exists an assignment  $$\begin{align*}\mathrm{Cycle}^{n}(\Pi_{1}) \ni J \, \, \mapsto \, \, \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
$$\begin{align*}\mathrm{Cycle}^{n}(\Pi_{1}) \ni J \, \, \mapsto \, \, \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
—where
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
denotes an I-conjugacy class of isomorphisms
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
denotes an I-conjugacy class of isomorphisms
 $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
—such that
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
—such that-
(a)
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps
 $I_{\mathrm {tpd}}$
bijectively onto I,
$I_{\mathrm {tpd}}$
bijectively onto I, -
(b)
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps the subgroups
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps the subgroups
 $J^{*}_{\mathrm {tpd}}$
,
$J^{*}_{\mathrm {tpd}}$
,
 $J^{**}_{\mathrm {tpd}}$
bijectively onto lifting cycle-subgroups of
$J^{**}_{\mathrm {tpd}}$
bijectively onto lifting cycle-subgroups of
 $\mathfrak {C}_I(J)$
, and
$\mathfrak {C}_I(J)$
, and -
(c) for
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, the diagram (of
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, the diagram (of
 $I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms)
$I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) 
—where the upper horizontal arrow is the (uniquely determined—cf. the commensurable terminality of
 $I_{\mathrm {tpd}}$
in
$I_{\mathrm {tpd}}$
in
 $\Pi _{\mathrm {tpd}}$
discussed in [Reference Mochizuki18, Prop. 1.2(ii)])
$\Pi _{\mathrm {tpd}}$
discussed in [Reference Mochizuki18, Prop. 1.2(ii)])
 $I_{\mathrm {tpd}}$
-conjugacy class of automorphisms of
$I_{\mathrm {tpd}}$
-conjugacy class of automorphisms of
 $\Pi _{\mathrm {tpd}}$
that lifts
$\Pi _{\mathrm {tpd}}$
that lifts
 $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]) and preserves
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]) and preserves
 $I_{\mathrm {tpd}}$
; the lower horizontal arrow is the I-conjugacy class of isomorphisms induced by
$I_{\mathrm {tpd}}$
; the lower horizontal arrow is the I-conjugacy class of isomorphisms induced by
 $\alpha _2$
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
$\alpha _2$
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
 $\mathfrak {C}_I(\alpha _1(J))$
associated with I (cf. Definition 3.8).
$\mathfrak {C}_I(\alpha _1(J))$
associated with I (cf. Definition 3.8).
Finally, the assignment
is uniquely determined, up to possible composition with cycle symmetries, by these conditions (a), (b), and (c). $$\begin{align*}J\mapsto \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
$$\begin{align*}J\mapsto \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
-
-
(iv) Let
 $n\geq 3$
be a positive integer,
$n\geq 3$
be a positive integer,
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, and
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
, and
 $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
. Suppose that one of the following conditions is satisfied:
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
. Suppose that one of the following conditions is satisfied:-
(a) The FC-admissible outomorphism of
 $\Pi _{3}$
determined by
$\Pi _{3}$
determined by
 $\alpha _3$
is
$\alpha _3$
is
 $ \in \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]).
$ \in \mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.19]). -
(b)
 $\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
.
$\mathrm {Cusp}(\mathcal {G})\neq \emptyset $
. -
(c)
 $n\geq 4$
.
$n\geq 4$
.
Then there exists an automorphism
 $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
such that the FC-admissible outomorphism of
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
such that the FC-admissible outomorphism of
 $\Pi _{3}$
determined by
$\Pi _{3}$
determined by
 $\beta _3$
is contained in
$\beta _3$
is contained in
 $\mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
, and, moreover,
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{3})^{\mathrm {geo}}$
, and, moreover,
 $\alpha _1(J) = \beta _1(J)$
. Finally, the diagram (of
$\alpha _1(J) = \beta _1(J)$
. Finally, the diagram (of
 $I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) —where the lower horizontal arrow is the isomorphism induced by
$I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) —where the lower horizontal arrow is the isomorphism induced by
 $\beta _2$
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
$\beta _2$
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
 $\mathfrak {C}_I(\alpha _1(J))= \mathfrak {C}_I(\beta _1(J))$
associated with I.
$\mathfrak {C}_I(\alpha _1(J))= \mathfrak {C}_I(\beta _1(J))$
associated with I.
-
Proof. Assertion (i) follows immediately from the various definitions involved. Next, we verify assertion (ii). The initial portion of assertion (ii) follows immediately from the discussion of Remark 3.6.1, together with the fact that T is uniquely determined among its
![]() $\Pi _{2/1}$
-conjugates by the condition
$\Pi _{2/1}$
-conjugates by the condition
![]() $I\subseteq T$
(cf. [Reference Mochizuki18, Prop. 1.5(i)]). The final portion of assertion (ii) follows immediately from Lemma 3.1(ii) (i.e., by considering a suitable generization operation, as in the discussion of Remark 3.6.1). This completes the proof of assertion (ii).
$I\subseteq T$
(cf. [Reference Mochizuki18, Prop. 1.5(i)]). The final portion of assertion (ii) follows immediately from Lemma 3.1(ii) (i.e., by considering a suitable generization operation, as in the discussion of Remark 3.6.1). This completes the proof of assertion (ii).
Next, we verify assertion (iii). Let us fix data
for
![]() $J \in \mathrm {Cycle}^{n}(\Pi _{1})$
as in Definition 3.6(i.a)–(i.d), and let
$J \in \mathrm {Cycle}^{n}(\Pi _{1})$
as in Definition 3.6(i.a)–(i.d), and let
![]() ${}^{Y}\!T \subseteq {}^{Y}\!\Pi _{2/1}$
be a
${}^{Y}\!T \subseteq {}^{Y}\!\Pi _{2/1}$
be a
![]() $\{1,2\}$
-tripod as in the discussion of Definition 3.6(i). Let
$\{1,2\}$
-tripod as in the discussion of Definition 3.6(i). Let
![]() ${}^{Y}\!\Pi _{\mathrm {tpd}} \subseteq {}^{Y}\!\Pi _{3}$
be a
${}^{Y}\!\Pi _{\mathrm {tpd}} \subseteq {}^{Y}\!\Pi _{3}$
be a
![]() $3$
-central tripod of
$3$
-central tripod of
![]() ${}^{Y}\!\Pi _{3}$
. Here, we note that since
${}^{Y}\!\Pi _{3}$
. Here, we note that since
![]() $J\in \mathrm {Cycle}^{n}(\Pi _{1})$
, and
$J\in \mathrm {Cycle}^{n}(\Pi _{1})$
, and
![]() $n\geq 3$
, it follows that the above isomorphism
$n\geq 3$
, it follows that the above isomorphism
![]() ${}^{Y}\!\Pi _{2} \stackrel {\sim }{\rightarrow } \Pi _{2}$
lifts to a PFC-admissible isomorphism
${}^{Y}\!\Pi _{2} \stackrel {\sim }{\rightarrow } \Pi _{2}$
lifts to a PFC-admissible isomorphism
![]() ${}^{Y}\!\Pi _{3} \stackrel {\sim }{\rightarrow } \Pi _{3}$
that maps
${}^{Y}\!\Pi _{3} \stackrel {\sim }{\rightarrow } \Pi _{3}$
that maps
![]() ${}^{Y}\!\Pi _{\mathrm {tpd}}$
to a
${}^{Y}\!\Pi _{\mathrm {tpd}}$
to a
![]() $\Pi _{3}$
-conjugate of
$\Pi _{3}$
-conjugate of
![]() $\Pi _{\mathrm {tpd}}$
(cf. [Reference Hoshi and Mochizuki8, Th. B]; [Reference Hoshi and Mochizuki10, Th. 3.16(v)]; [Reference Hoshi and Mochizuki10, Rem 4.14.1]).
$\Pi _{\mathrm {tpd}}$
(cf. [Reference Hoshi and Mochizuki8, Th. B]; [Reference Hoshi and Mochizuki10, Th. 3.16(v)]; [Reference Hoshi and Mochizuki10, Rem 4.14.1]).
Now one verifies immediately that, to verify the existence portion of assertion (iii), by applying a suitable generization operation as in the discussion of Remark 3.6.1, we may assume without loss of generality that
![]() $\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
(an assumption that will be invoked when we apply Lemma 3.7 in the argument to follow). Then, by considering the geometric (hence, in particular, C-admissible) outer isomorphism of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)] in the case where we take the “
$\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
(an assumption that will be invoked when we apply Lemma 3.7 in the argument to follow). Then, by considering the geometric (hence, in particular, C-admissible) outer isomorphism of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)] in the case where we take the “
![]() $(T, T')$
” of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)] to be
$(T, T')$
” of [Reference Hoshi and Mochizuki10, Th. 3.18(ii)] to be
![]() $({}^{Y}\!\Pi _{\mathrm {tpd}}, {}^{Y}\!T)$
, we obtain an outer isomorphism
$({}^{Y}\!\Pi _{\mathrm {tpd}}, {}^{Y}\!T)$
, we obtain an outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
. Moreover, by considering the composite of this outer isomorphism with a suitable geometric outomorphism of
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
. Moreover, by considering the composite of this outer isomorphism with a suitable geometric outomorphism of
![]() $\Pi _{\mathrm {tpd}}$
, we may assume without loss of generality that this outer isomorphism
$\Pi _{\mathrm {tpd}}$
, we may assume without loss of generality that this outer isomorphism
![]() $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
maps the
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
maps the
![]() $\Pi _{\mathrm {tpd}}$
-conjugacy class of
$\Pi _{\mathrm {tpd}}$
-conjugacy class of
![]() $I_{\mathrm {tpd}}$
to the
$I_{\mathrm {tpd}}$
to the
![]() $\mathfrak {C}_I(J)$
-conjugacy class of I. Thus, since I is commensurably terminal in
$\mathfrak {C}_I(J)$
-conjugacy class of I. Thus, since I is commensurably terminal in
![]() $\mathfrak {C}_I(J)$
(cf. [Reference Mochizuki18, Prop. 1.2(ii)]), we obtain a uniquely determined I-conjugacy class of isomorphisms
$\mathfrak {C}_I(J)$
(cf. [Reference Mochizuki18, Prop. 1.2(ii)]), we obtain a uniquely determined I-conjugacy class of isomorphisms
![]() $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J} \colon \Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
that lifts the outer isomorphism just discussed and satisfies condition (a). On the other hand, one verifies immediately from the various definitions involved that
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J} \colon \Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
that lifts the outer isomorphism just discussed and satisfies condition (a). On the other hand, one verifies immediately from the various definitions involved that
![]() $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
also satisfies condition (b).
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
also satisfies condition (b).
Next, we verify that
![]() $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
satisfies condition (c). To this end, let us observe that it follows immediately from the various definitions involved (cf. also our assumption that
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
satisfies condition (c). To this end, let us observe that it follows immediately from the various definitions involved (cf. also our assumption that
![]() $\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
), that
$\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
), that
![]() $\alpha _1(J)$
admits data as in Definition 3.6 (i.a)–(i.d) such that
$\alpha _1(J)$
admits data as in Definition 3.6 (i.a)–(i.d) such that
-
• the portion of these data that correspond to the data of Definition 3.6(i.a) and (i.d) is of the form
for some isomorphism $$ \begin{align*}({}^{Y}\!\mathcal{G}, S \subseteq \mathrm{Node}({}^{Y}\!\mathcal{G}), \psi \colon {}^{Y}\!\mathcal{G}_{\leadsto {S}} \stackrel{\sim}{\rightarrow} \mathcal{G}); \, \, \, \Pi_{e} \subseteq {}^{Y}\!\Pi_{1} \end{align*} $$
$$ \begin{align*}({}^{Y}\!\mathcal{G}, S \subseteq \mathrm{Node}({}^{Y}\!\mathcal{G}), \psi \colon {}^{Y}\!\mathcal{G}_{\leadsto {S}} \stackrel{\sim}{\rightarrow} \mathcal{G}); \, \, \, \Pi_{e} \subseteq {}^{Y}\!\Pi_{1} \end{align*} $$
 $\psi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}$
, and, moreover,
$\psi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}$
, and, moreover,
-
• the composite
—where the first (resp. third) arrow is the isomorphism arising from the data (cf. Definition 3.6(i.c)) for J (resp. $$ \begin{align*}{}^{Y}\!\Pi_{2} \stackrel{\sim}{\longrightarrow} \Pi_{2} \stackrel{\alpha_2}{\stackrel{\sim}{\longrightarrow}} \Pi_{2} \stackrel{\sim}{\longleftarrow} {}^{Y}\!\Pi_{2} \end{align*} $$
$$ \begin{align*}{}^{Y}\!\Pi_{2} \stackrel{\sim}{\longrightarrow} \Pi_{2} \stackrel{\alpha_2}{\stackrel{\sim}{\longrightarrow}} \Pi_{2} \stackrel{\sim}{\longleftarrow} {}^{Y}\!\Pi_{2} \end{align*} $$
 $\alpha _1(J)$
)
$\alpha _1(J)$
)
 $\in \mathrm {Cycle}^{n}(\Pi _{1})$
under consideration— is the identity automorphism.
$\in \mathrm {Cycle}^{n}(\Pi _{1})$
under consideration— is the identity automorphism.
Thus, to verify the assertion that
![]() $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
satisfies condition (c), it suffices to verify that the I-conjugacy class of isomorphisms “
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
satisfies condition (c), it suffices to verify that the I-conjugacy class of isomorphisms “
![]() $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}\colon \Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
” constructed above from a fixed choice of data as in Definition 3.6(i.a)–(i.d) does not depend on this choice of data. On the other hand, this follows immediately from Lemma 3.7(ii) (cf. our assumption that
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}\colon \Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
” constructed above from a fixed choice of data as in Definition 3.6(i.a)–(i.d) does not depend on this choice of data. On the other hand, this follows immediately from Lemma 3.7(ii) (cf. our assumption that
![]() $\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
).
$\mathrm {Node}({}^{Y}\!\mathcal {G})^\sharp = 1$
).
Finally, we consider the final portion of assertion (iii) concerning uniqueness. To this end, we observe that, by considering the case where
![]() ${}^{Y}\!\mathcal {G}$
, as well as each of the branches of the underlying semi-graph of
${}^{Y}\!\mathcal {G}$
, as well as each of the branches of the underlying semi-graph of
![]() ${}^{Y}\!\mathcal {G}$
, is defined over a number field F, it follows immediately, by considering automorphisms
${}^{Y}\!\mathcal {G}$
, is defined over a number field F, it follows immediately, by considering automorphisms
![]() $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
that arise from scheme theory, that given any element
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
that arise from scheme theory, that given any element
![]() $\gamma \in \mathrm {Out}(\Pi _{\mathrm {tpd}})$
that arises from an element of the absolute Galois group of F, there exists an
$\gamma \in \mathrm {Out}(\Pi _{\mathrm {tpd}})$
that arises from an element of the absolute Galois group of F, there exists an
![]() $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
such that
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{n}, I)^{\mathrm {G}}$
such that
![]() $\alpha (J)=J$
and
$\alpha (J)=J$
and
![]() $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )=\gamma $
. Thus, the uniqueness under consideration follows immediately from the geometricity of elements of
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )=\gamma $
. Thus, the uniqueness under consideration follows immediately from the geometricity of elements of
![]() $\mathrm {Out}(\Pi _{\mathrm {tpd}})$
that commute with the image of the absolute Galois group of F, that is, in other words, from the Grothendieck Conjecture for tripods over number fields (cf. [Reference Tamagawa28, Th. 0.3]; [Reference Mochizuki13, Th. A]). This completes the proof of assertion (iii).
$\mathrm {Out}(\Pi _{\mathrm {tpd}})$
that commute with the image of the absolute Galois group of F, that is, in other words, from the Grothendieck Conjecture for tripods over number fields (cf. [Reference Tamagawa28, Th. 0.3]; [Reference Mochizuki13, Th. A]). This completes the proof of assertion (iii).
Finally, we verify assertion (iv). If condition (a) is satisfied, then, by taking the “
![]() $\beta $
” of assertion (iv) to be
$\beta $
” of assertion (iv) to be
![]() $\alpha $
, we conclude that assertion (iv) follows immediately from assertion (iii), together with the definition of
$\alpha $
, we conclude that assertion (iv) follows immediately from assertion (iii), together with the definition of
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {geo}}$
. Next, let us observe that, by applying assertion (iv) in the case where condition (a) is satisfied, we conclude that, to verify assertion (iv) in the case where either (b) or (c) is satisfied, it suffices to verify that the following assertion holds:
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {geo}}$
. Next, let us observe that, by applying assertion (iv) in the case where condition (a) is satisfied, we conclude that, to verify assertion (iv) in the case where either (b) or (c) is satisfied, it suffices to verify that the following assertion holds:
Claim 3.10.A: Write
for the subgroup of
![]() $\mathrm {Out}(\Pi _{1})$
consisting of outomorphisms of
$\mathrm {Out}(\Pi _{1})$
consisting of outomorphisms of
![]() $\Pi _{1}$
that preserve the
$\Pi _{1}$
that preserve the
![]() $\Pi _{1}$
-conjugacy class of J and
$\Pi _{1}$
-conjugacy class of J and
Then every element of the image of the natural injection
(cf. [Reference Hoshi and Mochizuki8, Th. B]) may be written as a product of an element of the image of the natural injection
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {geo}} \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1})$
and an element of
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {geo}} \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1})$
and an element of
![]() $ \mathrm {Out}(\Pi _{1}\supseteq J) ^{\mathrm {G}} \stackrel {\mathrm {def}}{=} \mathrm {Out}(\Pi _{1}\supseteq J) \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{1})^{\mathrm {G}}$
.
$ \mathrm {Out}(\Pi _{1}\supseteq J) ^{\mathrm {G}} \stackrel {\mathrm {def}}{=} \mathrm {Out}(\Pi _{1}\supseteq J) \cap \mathrm {Out}^{\mathrm {FC}}(\Pi _{1})^{\mathrm {G}}$
.
To verify Claim 3.10.A, write
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n}, J)^{\mathrm {G}} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {G}}$
for the subgroup of
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n}, J)^{\mathrm {G}} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {G}}$
for the subgroup of
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {G}}$
obtained by forming the inverse image of the closed subgroup
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {G}}$
obtained by forming the inverse image of the closed subgroup
![]() $\mathrm {Out}(\Pi _{1}\supseteq J) \subseteq \mathrm {Out}(\Pi _{1})$
via the natural injection
$\mathrm {Out}(\Pi _{1}\supseteq J) \subseteq \mathrm {Out}(\Pi _{1})$
via the natural injection
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {G}} \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1})$
. Then one verifies immediately, by considering the exact sequence
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})^{\mathrm {G}} \hookrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{1})$
. Then one verifies immediately, by considering the exact sequence
(cf. conditions (b) and (c); [Reference Hoshi and Mochizuki10, Def. 3.19]; [Reference Hoshi and Mochizuki10, Cor. 4.15]), that, to verify Claim 3.10.A, it suffices to verify that the following assertion holds:
Claim 3.10.B: The composite
is surjective.
To verify Claim 3.10.B, let
![]() $({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
be an n-cuspidalizable degeneration structure on
$({}^{Y}\!\mathcal {G}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}), \phi \colon {}^{Y}\!\mathcal {G}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G})$
be an n-cuspidalizable degeneration structure on
![]() $\mathcal {G}$
with respect to which J is a cycle-subgroup such that
$\mathcal {G}$
with respect to which J is a cycle-subgroup such that
![]() ${}^{Y}\!\mathcal {G}$
is totally degenerate (cf. [Reference Hoshi and Mochizuki9, Def. 2.3(iv)]). (One verifies immediately that such a degeneration structure always exists.) Now let us identify
${}^{Y}\!\mathcal {G}$
is totally degenerate (cf. [Reference Hoshi and Mochizuki9, Def. 2.3(iv)]). (One verifies immediately that such a degeneration structure always exists.) Now let us identify
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
with
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
with
![]() $\mathrm {Out}^{\mathrm {FC}}({}^{Y}\!\Pi _{n})$
via a(n) (uniquely determined, up to permutation of the n factors—cf. [Reference Hoshi and Mochizuki8, Th. B]) PFC-admissible (cf. [Reference Hoshi and Mochizuki9, Def. 1.4(iii)]) outer isomorphism
$\mathrm {Out}^{\mathrm {FC}}({}^{Y}\!\Pi _{n})$
via a(n) (uniquely determined, up to permutation of the n factors—cf. [Reference Hoshi and Mochizuki8, Th. B]) PFC-admissible (cf. [Reference Hoshi and Mochizuki9, Def. 1.4(iii)]) outer isomorphism
![]() $\Pi _{n} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{n}$
that is compatible with the outomorphism of the display of Definition 3.5(i) (cf. [Reference Hoshi and Mochizuki10, Prop. 3.24(i)]). Then it follows immediately from the various definitions involved that the closed subgroup
$\Pi _{n} \stackrel {\sim }{\rightarrow } {}^{Y}\!\Pi _{n}$
that is compatible with the outomorphism of the display of Definition 3.5(i) (cf. [Reference Hoshi and Mochizuki10, Prop. 3.24(i)]). Then it follows immediately from the various definitions involved that the closed subgroup
![]() $\mathrm {Out}^{\mathrm {FC}}({}^{Y}\!\Pi _{n})^{\mathrm {brch}} \subseteq \mathrm {Out}^{\mathrm {FC}}({}^{Y}\!\Pi _{n})$
(cf. [Reference Hoshi and Mochizuki10, Def. 4.6(i)]) is contained in the closed subgroup
$\mathrm {Out}^{\mathrm {FC}}({}^{Y}\!\Pi _{n})^{\mathrm {brch}} \subseteq \mathrm {Out}^{\mathrm {FC}}({}^{Y}\!\Pi _{n})$
(cf. [Reference Hoshi and Mochizuki10, Def. 4.6(i)]) is contained in the closed subgroup
![]() $\mathrm {Out}^{\mathrm {FC}}(\Pi _{n}, J)^{\mathrm {G}} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
. On the other hand, it follows immediately from the proof of [Reference Hoshi and Mochizuki10, Cor. 4.15] that the composite
$\mathrm {Out}^{\mathrm {FC}}(\Pi _{n}, J)^{\mathrm {G}} \subseteq \mathrm {Out}^{\mathrm {FC}}(\Pi _{n})$
. On the other hand, it follows immediately from the proof of [Reference Hoshi and Mochizuki10, Cor. 4.15] that the composite
is surjective. This completes the proof of Claim 3.10.B, hence also of assertion (iv) in the case where either (b) or (c) is satisfied.
Remark 3.10.1.
-
(i) The content of Theorem 3.10(iv) may be regarded, that is, by considering the various lifting cycle-subgroups involved, as a formulation of the construction of the two sections discussed in [Reference Boggi5, Prop. 2.7] (which plays an essential role in the proof of [Reference Boggi5, Th. 2.4]), in terms of the purely combinatorial and algebraic techniques developed in the present series of papers.
-
(ii) In this context, we observe in passing that (one verifies immediately that) for arbitrary nonnegative integers g, r such that
-
•
 $3g-3+r>0$
, and, moreover,
$3g-3+r>0$
, and, moreover, -
• if
 $g = 0$
, then r is even,
$g = 0$
, then r is even,
there exists a stable log curve of type
 $(g,r)$
which admits an automorphism that is linear over the base scheme under consideration and fixes a node of the stable log curve, but switches the branches of this node. Thus, by considering the resulting automorphism of the associated semi-graph of anabelioids of pro-
$(g,r)$
which admits an automorphism that is linear over the base scheme under consideration and fixes a node of the stable log curve, but switches the branches of this node. Thus, by considering the resulting automorphism of the associated semi-graph of anabelioids of pro-
 $\Sigma $
PSC-type, one concludes that the diagrams of Theorem 3.10(iii) and (iv) fail to commute, in general, if one does not allow for the possibility of composition with a cycle symmetry. This situation contrasts with the situation discussed in [Reference Boggi5, Prop. 2.7], where two independent sections are obtained, by considering orientations on the various cycles involved.
$\Sigma $
PSC-type, one concludes that the diagrams of Theorem 3.10(iii) and (iv) fail to commute, in general, if one does not allow for the possibility of composition with a cycle symmetry. This situation contrasts with the situation discussed in [Reference Boggi5, Prop. 2.7], where two independent sections are obtained, by considering orientations on the various cycles involved. -
-
(iii) The orientation-theoretic portion of [Reference Boggi5, Prop. 2.7] referred to in (ii) above may be interpreted, from the point of view of the present paper, as a lifting “
 $\mathfrak {C}_I^\pm $
” of the map
$\mathfrak {C}_I^\pm $
” of the map
 $\mathfrak {C}_I$
of Theorem 3.10(ii) as follows. In the notation of Theorem 3.10, let us write
$\mathfrak {C}_I$
of Theorem 3.10(ii) as follows. In the notation of Theorem 3.10, let us write-
•
 $ \mathrm {Cycle}^{n}(\Pi _1)^\pm $
for the set of pairs consisting of a cycle-subgroup
$ \mathrm {Cycle}^{n}(\Pi _1)^\pm $
for the set of pairs consisting of a cycle-subgroup
 $J\in \mathrm {Cycle}^{n}(\Pi _1)$
and an orientation on J (cf. Remark 3.6.2(i));
$J\in \mathrm {Cycle}^{n}(\Pi _1)$
and an orientation on J (cf. Remark 3.6.2(i)); -
•
 $ \mathrm {Tpd}_I(\Pi _{2/1})^\pm $
for the set of pairs consisting of a tripodal subgroup
$ \mathrm {Tpd}_I(\Pi _{2/1})^\pm $
for the set of pairs consisting of a tripodal subgroup
 $T\in \mathrm {Tpd}_I(\Pi _{2/1})$
and an orientation on T (cf. Remark 3.6.2(ii)).
$T\in \mathrm {Tpd}_I(\Pi _{2/1})$
and an orientation on T (cf. Remark 3.6.2(ii)).
Thus, one has natural surjections
 $\mathrm {Cycle}^{n}(\Pi _1)^\pm \twoheadrightarrow \mathrm {Cycle}^{n}(\Pi _1)$
,
$\mathrm {Cycle}^{n}(\Pi _1)^\pm \twoheadrightarrow \mathrm {Cycle}^{n}(\Pi _1)$
,
 $\mathrm {Tpd}_I(\Pi _{2/1})^\pm \twoheadrightarrow \mathrm {Tpd}_I(\Pi _{2/1})$
, which may be regarded as torsors over the group
$\mathrm {Tpd}_I(\Pi _{2/1})^\pm \twoheadrightarrow \mathrm {Tpd}_I(\Pi _{2/1})$
, which may be regarded as torsors over the group
 $\{\pm 1\}$
. Moreover, one verifies immediately from the functoriality of the various isomorphisms that appeared in the constructions of Remark 3.6.2(i) and (ii) that the action (cf. Theorem 3.10(i)) of
$\{\pm 1\}$
. Moreover, one verifies immediately from the functoriality of the various isomorphisms that appeared in the constructions of Remark 3.6.2(i) and (ii) that the action (cf. Theorem 3.10(i)) of
 $\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
on the sets
$\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
on the sets
 $\mathrm {Cycle}^{n}(\Pi _1)$
,
$\mathrm {Cycle}^{n}(\Pi _1)$
,
 $\mathrm {Tpd}_I(\Pi _{2/1})$
lifts naturally to an action of
$\mathrm {Tpd}_I(\Pi _{2/1})$
lifts naturally to an action of
 $\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
on the sets
$\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
on the sets
 $\mathrm {Cycle}^{n}(\Pi _1)^\pm $
,
$\mathrm {Cycle}^{n}(\Pi _1)^\pm $
,
 $\mathrm {Tpd}_I(\Pi _{2/1})^\pm $
. Thus, the inverse of the bijective correspondence of the final display of Remark 3.6.2(ii) determines a natural
$\mathrm {Tpd}_I(\Pi _{2/1})^\pm $
. Thus, the inverse of the bijective correspondence of the final display of Remark 3.6.2(ii) determines a natural
 $\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
-equivariant lifting of the map
$\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
-equivariant lifting of the map $$ \begin{align*}\mathfrak{C}_I^\pm\colon \mathrm{Cycle}^{n}(\Pi_1)^\pm\ \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1})^\pm \end{align*} $$
$$ \begin{align*}\mathfrak{C}_I^\pm\colon \mathrm{Cycle}^{n}(\Pi_1)^\pm\ \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1})^\pm \end{align*} $$
 $\mathfrak {C}_I$
of Theorem 3.10(ii). (Thus, the
$\mathfrak {C}_I$
of Theorem 3.10(ii). (Thus, the
 $\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
-equivariance of
$\mathrm {Aut}^{\text {FC}}(\Pi _n,I)^{\text {G}}$
-equivariance of
 $\mathfrak {C}_I^\pm $
implies, in particular, that
$\mathfrak {C}_I^\pm $
implies, in particular, that
 $\mathfrak {C}_I^\pm $
does not factor through the natural surjection
$\mathfrak {C}_I^\pm $
does not factor through the natural surjection
 $\mathrm {Cycle}^{n}(\Pi _1)^\pm \twoheadrightarrow \mathrm {Cycle}^{n}(\Pi _1)$
.) Moreover, if
$\mathrm {Cycle}^{n}(\Pi _1)^\pm \twoheadrightarrow \mathrm {Cycle}^{n}(\Pi _1)$
.) Moreover, if
 $n\geq 3$
, and one regards the
$n\geq 3$
, and one regards the
 $\Pi _{\text {tpd}}$
-conjugacy class of cuspidal subgroups of
$\Pi _{\text {tpd}}$
-conjugacy class of cuspidal subgroups of
 $\Pi _{\text {tpd}}$
determined by
$\Pi _{\text {tpd}}$
determined by
 $J^*_{\text {tpd}}$
as being “positive,” then it follows immediately from the definition of
$J^*_{\text {tpd}}$
as being “positive,” then it follows immediately from the definition of
 $\mathrm {Tpd}_I(\Pi _{2/1})^\pm $
that this lifting
$\mathrm {Tpd}_I(\Pi _{2/1})^\pm $
that this lifting
 $\mathfrak {C}_I^\pm $
naturally determines an assignment —where
$\mathfrak {C}_I^\pm $
naturally determines an assignment —where $$ \begin{align*}\mathrm{Cycle}^{n}(\Pi_1)^\pm \ni J^\pm\ \mapsto\ \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J^{\pm}}^{\pm} \end{align*} $$
$$ \begin{align*}\mathrm{Cycle}^{n}(\Pi_1)^\pm \ni J^\pm\ \mapsto\ \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J^{\pm}}^{\pm} \end{align*} $$
 $J^\pm \mapsto J\in \mathrm {Cycle}^{n}(\Pi _1)$
, and
$J^\pm \mapsto J\in \mathrm {Cycle}^{n}(\Pi _1)$
, and
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J^{\pm }}^{\pm }$
denotes an I-conjugacy class of isomorphisms
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J^{\pm }}^{\pm }$
denotes an I-conjugacy class of isomorphisms
 $\Pi _{\text {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
that coincides, up to possible composition with a cycle symmetry, with the I-conjugacy class of isomorphisms
$\Pi _{\text {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
that coincides, up to possible composition with a cycle symmetry, with the I-conjugacy class of isomorphisms
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
of Theorem 3.10(iii)—such that if, in the diagram (of
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
of Theorem 3.10(iii)—such that if, in the diagram (of
 $I_{\text {tpd}}$
-, I-conjugacy classes of isomorphisms) in the display of Theorem 3.10(iii.c), one replaces “
$I_{\text {tpd}}$
-, I-conjugacy classes of isomorphisms) in the display of Theorem 3.10(iii.c), one replaces “
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}$
” by “
$\mathfrak {s}\mathfrak {y}\mathfrak {n}$
” by “
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}^{\pm }$
,” then the diagram commutes, that is, even if one does not allow for possible composition with cycle symmetries.
$\mathfrak {s}\mathfrak {y}\mathfrak {n}^{\pm }$
,” then the diagram commutes, that is, even if one does not allow for possible composition with cycle symmetries.
-
Definition 3.11. Suppose that
![]() $\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
, and that
$\Sigma = \mathfrak {P}\mathfrak {r}\mathfrak {i}\mathfrak {m}\mathfrak {e}\mathfrak {s}$
, and that
![]() $k=\mathbb {C}$
, that is, that we are in the situation of Definition 2.22. We shall apply the notational conventions established in Definition 2.22. Moreover, we shall use similar notation
$k=\mathbb {C}$
, that is, that we are in the situation of Definition 2.22. We shall apply the notational conventions established in Definition 2.22. Moreover, we shall use similar notation
for objects associated with the stable log curve
![]() $Y^{\mathrm {log}} = Y_1^{\mathrm {log}}$
to the notation introduced in Definitions 2.22 and 2.23.
$Y^{\mathrm {log}} = Y_1^{\mathrm {log}}$
to the notation introduced in Definitions 2.22 and 2.23.
Definition 3.12. Let
![]() $\mathcal {J}$
be a semi-graph of temperoids of HSD-type (cf. Definition 2.3(iii)). Then we shall refer to a triple
$\mathcal {J}$
be a semi-graph of temperoids of HSD-type (cf. Definition 2.3(iii)). Then we shall refer to a triple
(cf. Definition 2.9) consisting of a semi-graph of temperoids of HSD-type
![]() $\mathcal {H}$
, a subset
$\mathcal {H}$
, a subset
![]() ${S\subseteq \mathrm {Node}(\mathcal {H})}$
, and an isomorphism
${S\subseteq \mathrm {Node}(\mathcal {H})}$
, and an isomorphism
![]() $\phi \colon \mathcal {H}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {J}$
of semi-graphs of temperoids of HSD-type as a degeneration structure on
$\phi \colon \mathcal {H}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {J}$
of semi-graphs of temperoids of HSD-type as a degeneration structure on
![]() $\mathcal {J}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(i)]).
$\mathcal {J}$
(cf. [Reference Hoshi and Mochizuki10, Def. 3.23(i)]).
Definition 3.13. In the situation of Definition 3.11:
-
(i) Let
 $({}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}})$
be a degeneration structure on
$({}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}})$
be a degeneration structure on
 $\mathcal {G}^{\mathrm {disc}}$
(cf. Definition 3.12),
$\mathcal {G}^{\mathrm {disc}}$
(cf. Definition 3.12),
 $e \in S$
, and
$e \in S$
, and
 $J\subseteq \Pi _{1}^{\mathrm {disc}}$
a subgroup of
$J\subseteq \Pi _{1}^{\mathrm {disc}}$
a subgroup of
 $\Pi _{1}^{\mathrm {disc}}$
. Then we shall say that
$\Pi _{1}^{\mathrm {disc}}$
. Then we shall say that
 $J\subseteq \Pi _{1}^{\mathrm {disc}}$
is a cycle-subgroup of
$J\subseteq \Pi _{1}^{\mathrm {disc}}$
is a cycle-subgroup of
 $\Pi _{1}^{\mathrm {disc}}$
(with respect to
$\Pi _{1}^{\mathrm {disc}}$
(with respect to
 $[{}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}}]$
, associated with
$[{}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}}]$
, associated with
 $e \in S$
) if J is contained in the
$e \in S$
) if J is contained in the
 $\Pi _{1}^{\mathrm {disc}}$
-conjugacy class of subgroups of
$\Pi _{1}^{\mathrm {disc}}$
-conjugacy class of subgroups of
 $\Pi _{1}^{\mathrm {disc}}$
obtained by forming the image of a nodal subgroup of
$\Pi _{1}^{\mathrm {disc}}$
obtained by forming the image of a nodal subgroup of
 $\Pi _{{}^{Y}\!\mathcal {G}^{\mathrm {disc}}}$
associated with e via the composite of outer isomorphisms
$\Pi _{{}^{Y}\!\mathcal {G}^{\mathrm {disc}}}$
associated with e via the composite of outer isomorphisms  $$\begin{align*}\Pi_{{}^{Y}\!\mathcal{G}^{\mathrm{disc}}} \stackrel{\Phi^{-1}_{{}^{Y}\!\mathcal{G}^{\mathrm{disc}}_{\leadsto {S}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}^{\mathrm{disc}}_{\leadsto {S}}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}^{\mathrm{disc}}} \stackrel{\sim}{\longrightarrow} \Pi_{1}^{\mathrm{disc}} \end{align*}$$
$$\begin{align*}\Pi_{{}^{Y}\!\mathcal{G}^{\mathrm{disc}}} \stackrel{\Phi^{-1}_{{}^{Y}\!\mathcal{G}^{\mathrm{disc}}_{\leadsto {S}}}}{\stackrel{\sim}{\longrightarrow}} \Pi_{{}^{Y}\!\mathcal{G}^{\mathrm{disc}}_{\leadsto {S}}} \stackrel{\sim}{\longrightarrow} \Pi_{\mathcal{G}^{\mathrm{disc}}} \stackrel{\sim}{\longrightarrow} \Pi_{1}^{\mathrm{disc}} \end{align*}$$
—where the first arrow is the inverse of the specialization outer isomorphism
 $\Phi _{{}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}}}$
(cf. Proposition 2.10), the second arrow is the graphic (cf. Definition 2.7(ii)) outer isomorphism
$\Phi _{{}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}}}$
(cf. Proposition 2.10), the second arrow is the graphic (cf. Definition 2.7(ii)) outer isomorphism
 $\Pi _{{}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\mathrm {disc}}}$
induced by
$\Pi _{{}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\mathrm {disc}}}$
induced by
 $\phi $
, and the third arrow is the natural outer isomorphism
$\phi $
, and the third arrow is the natural outer isomorphism
 $\Pi _{\mathcal {G}^{\mathrm {disc}}}\stackrel {\sim }{\rightarrow }\Pi _{1}^{\mathrm {disc}}$
of (the second to last display of) Definition 2.23(i) (cf. the left-hand portion of Figure 1).
$\Pi _{\mathcal {G}^{\mathrm {disc}}}\stackrel {\sim }{\rightarrow }\Pi _{1}^{\mathrm {disc}}$
of (the second to last display of) Definition 2.23(i) (cf. the left-hand portion of Figure 1). -
(ii) Let
 $J\subseteq \Pi _{1}^{\mathrm {disc}}$
be a cycle-subgroup of
$J\subseteq \Pi _{1}^{\mathrm {disc}}$
be a cycle-subgroup of
 $\Pi _{1}^{\mathrm {disc}}$
(cf. (i)). Thus, we have
$\Pi _{1}^{\mathrm {disc}}$
(cf. (i)). Thus, we have-
(a) a degeneration structure
 $({}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}})$
on
$({}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}})$
on
 $\mathcal {G}^{\mathrm {disc}}$
(cf. Definition 3.12),
$\mathcal {G}^{\mathrm {disc}}$
(cf. Definition 3.12), -
(b) an isomorphism
 ${}^{Y}\!\Pi _{1}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{1}^{\mathrm {disc}}$
that is compatible with the composite of the display of (i) (cf. also [the second to last display of] Definition 2.23(i)) in the case where we take the “
${}^{Y}\!\Pi _{1}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{1}^{\mathrm {disc}}$
that is compatible with the composite of the display of (i) (cf. also [the second to last display of] Definition 2.23(i)) in the case where we take the “
 $({}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}})$
” of (i) to be the degeneration structure of (a),
$({}^{Y}\!\mathcal {G}^{\mathrm {disc}}, S \subseteq \mathrm {Node}({}^{Y}\!\mathcal {G}^{\mathrm {disc}}), \phi \colon {}^{Y}\!\mathcal {G}^{\mathrm {disc}}_{\leadsto {S}} \stackrel {\sim }{\rightarrow } \mathcal {G}^{\mathrm {disc}})$
” of (i) to be the degeneration structure of (a), -
(c) an isomorphism
 ${}^{Y}\!\Pi _{2}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{2}^{\mathrm {disc}}$
that lifts (cf. Corollary 2.20(v)) the isomorphism of (b) and, moreover, determines a PFC-admissible isomorphism between the respective profinite completions, and
${}^{Y}\!\Pi _{2}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{2}^{\mathrm {disc}}$
that lifts (cf. Corollary 2.20(v)) the isomorphism of (b) and, moreover, determines a PFC-admissible isomorphism between the respective profinite completions, and -
(d) a nodal subgroup
 $\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}^{\mathrm {disc}}$
of
$\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}^{\mathrm {disc}}$
of
 $ {}^{Y}\!\Pi _{1}^{\mathrm {disc}}$
associated with a (uniquely determined—cf. Corollary 2.18(iii)) node e of
$ {}^{Y}\!\Pi _{1}^{\mathrm {disc}}$
associated with a (uniquely determined—cf. Corollary 2.18(iii)) node e of
 ${}^{Y}\!\mathcal {G}^{\mathrm {disc}}$
${}^{Y}\!\mathcal {G}^{\mathrm {disc}}$
such that the image of the nodal subgroup
 $\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}^{\mathrm {disc}}$
of (d) via the isomorphism
$\Pi _{e} \subseteq {}^{Y}\!\Pi _{1}^{\mathrm {disc}}$
of (d) via the isomorphism
 ${}^{Y}\!\Pi _{1}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{1}^{\mathrm {disc}}$
of (b) coincides with
${}^{Y}\!\Pi _{1}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{1}^{\mathrm {disc}}$
of (b) coincides with
 $J \subseteq \Pi _{1}^{\mathrm {disc}}$
. We shall say that a subgroup
$J \subseteq \Pi _{1}^{\mathrm {disc}}$
. We shall say that a subgroup
 $T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
of
$T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
of
 $\Pi _{2/1}^{\mathrm {disc}}$
is a tripodal subgroup associated with J if T coincides—relative to some choice of data (a), (b), (c), (d) as above (but cf. also Remark 3.6.1 and Corollary 2.19(i)!)—with the image, via the lifting
$\Pi _{2/1}^{\mathrm {disc}}$
is a tripodal subgroup associated with J if T coincides—relative to some choice of data (a), (b), (c), (d) as above (but cf. also Remark 3.6.1 and Corollary 2.19(i)!)—with the image, via the lifting
 ${}^{Y}\!\Pi _{2}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{2}^{\mathrm {disc}}$
of (c), of some
${}^{Y}\!\Pi _{2}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{2}^{\mathrm {disc}}$
of (c), of some
 $\{1,2\}$
-tripod in
$\{1,2\}$
-tripod in
 ${}^{Y}\!\Pi _{2/1}^{\mathrm {disc}} \subseteq {}^{Y}\!\Pi _{2}^{\mathrm {disc}}$
(cf. Definition 2.23(ii)) arising from e (cf. Definition 2.23(iii); [Reference Hoshi and Mochizuki10, Def. 3.7(i)]), and, moreover, the centralizer
${}^{Y}\!\Pi _{2/1}^{\mathrm {disc}} \subseteq {}^{Y}\!\Pi _{2}^{\mathrm {disc}}$
(cf. Definition 2.23(ii)) arising from e (cf. Definition 2.23(iii); [Reference Hoshi and Mochizuki10, Def. 3.7(i)]), and, moreover, the centralizer
 $Z_{\Pi _{2}^{\mathrm {disc}}}(T)$
maps bijectively, via
$Z_{\Pi _{2}^{\mathrm {disc}}}(T)$
maps bijectively, via
 $p_{2/1}^{\Pi ^{\mathrm {disc}}}\colon \Pi _{2}^{\mathrm {disc}} \twoheadrightarrow \Pi _{1}^{\mathrm {disc}}$
, onto
$p_{2/1}^{\Pi ^{\mathrm {disc}}}\colon \Pi _{2}^{\mathrm {disc}} \twoheadrightarrow \Pi _{1}^{\mathrm {disc}}$
, onto
 $J\subseteq \Pi _{1}^{\mathrm {disc}}$
(cf. Corollary 2.17(i); [Reference Hoshi and Mochizuki10, Lem. 3.11(iv) and (vii)]).
$J\subseteq \Pi _{1}^{\mathrm {disc}}$
(cf. Corollary 2.17(i); [Reference Hoshi and Mochizuki10, Lem. 3.11(iv) and (vii)]). -
-
(iii) Let
 $J\subseteq \Pi _{1}^{\mathrm {disc}}$
be a cycle-subgroup of
$J\subseteq \Pi _{1}^{\mathrm {disc}}$
be a cycle-subgroup of
 $\Pi _{1}^{\mathrm {disc}}$
(cf. (i)) and
$\Pi _{1}^{\mathrm {disc}}$
(cf. (i)) and
 $T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
a tripodal subgroup associated with J (cf. (ii)). Then we shall refer to a subgroup of T that arises from a nodal (resp. cuspidal) subgroup contained in the
$T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
a tripodal subgroup associated with J (cf. (ii)). Then we shall refer to a subgroup of T that arises from a nodal (resp. cuspidal) subgroup contained in the
 $\{1,2\}$
-tripod in
$\{1,2\}$
-tripod in
 ${}^{Y}\!\Pi _{2/1}^{\mathrm {disc}} \subseteq {}^{Y}\!\Pi _{2}^{\mathrm {disc}}$
of (ii) as a lifting cycle-subgroup (resp. distinguished cuspidal subgroup) of T (cf. the right-hand portion of Figure 1).
${}^{Y}\!\Pi _{2/1}^{\mathrm {disc}} \subseteq {}^{Y}\!\Pi _{2}^{\mathrm {disc}}$
of (ii) as a lifting cycle-subgroup (resp. distinguished cuspidal subgroup) of T (cf. the right-hand portion of Figure 1). -
(iv) Let
 $J\subseteq \Pi _{1}^{\mathrm {disc}}$
be a cycle-subgroup (cf. (i));
$J\subseteq \Pi _{1}^{\mathrm {disc}}$
be a cycle-subgroup (cf. (i));
 $T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
a tripodal subgroup associated with J (cf. (ii));
$T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
a tripodal subgroup associated with J (cf. (ii));
 $I \subseteq T$
a distinguished cuspidal subgroup of T (cf. (iii)). Then it follows immediately from the various definitions involved, together with Theorem 2.24(i) that there exists a unique outomorphism
$I \subseteq T$
a distinguished cuspidal subgroup of T (cf. (iii)). Then it follows immediately from the various definitions involved, together with Theorem 2.24(i) that there exists a unique outomorphism
 $\iota $
of T such that the induced outomorphism of the profinite completion
$\iota $
of T such that the induced outomorphism of the profinite completion
 $\widehat T$
of T coincides with the outomorphism of
$\widehat T$
of T coincides with the outomorphism of
 $\widehat T$
determined by the cycle symmetry of
$\widehat T$
determined by the cycle symmetry of
 $\widehat T$
associated with the profinite completion
$\widehat T$
associated with the profinite completion
 $\widehat {I}$
of I (cf. Definition 3.8). Moreover, since I is commensurably terminal in T (cf. Corollary 2.18(v)), it follows immediately from Corollary 2.17(ii) that there exists a uniquely determined I-conjugacy class of automorphisms of T that lifts
$\widehat {I}$
of I (cf. Definition 3.8). Moreover, since I is commensurably terminal in T (cf. Corollary 2.18(v)), it follows immediately from Corollary 2.17(ii) that there exists a uniquely determined I-conjugacy class of automorphisms of T that lifts
 $\iota $
and preserves
$\iota $
and preserves
 $I\subseteq T$
. We shall refer to this I-conjugacy class of automorphisms of T as the cycle symmetry of T associated with I.
$I\subseteq T$
. We shall refer to this I-conjugacy class of automorphisms of T as the cycle symmetry of T associated with I.
Theorem 3.14 (Discrete version of canonical liftings of cycles).
In the notation of Definition 3.11, let
![]() $I \subseteq \Pi _{2/1}^{\mathrm {disc}}\subseteq \Pi _{2}^{\mathrm {disc}}$
be a cuspidal inertia group associated with the diagonal cusp of a fiber of
$I \subseteq \Pi _{2/1}^{\mathrm {disc}}\subseteq \Pi _{2}^{\mathrm {disc}}$
be a cuspidal inertia group associated with the diagonal cusp of a fiber of
![]() $p_{2/1}^{\mathrm {an}}$
;
$p_{2/1}^{\mathrm {an}}$
;
![]() $\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}^{\mathrm {disc}}$
a
$\Pi _{\mathrm {tpd}} \subseteq \Pi _{3}^{\mathrm {disc}}$
a
![]() $3$
-central
$3$
-central
![]() $\{1,2,3\}$
-tripod of
$\{1,2,3\}$
-tripod of
![]() $\Pi _{3}^{\mathrm {disc}}$
(cf. Definition 2.23(ii) and (iii));
$\Pi _{3}^{\mathrm {disc}}$
(cf. Definition 2.23(ii) and (iii));
![]() $I_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
a cuspidal subgroup of
$I_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
a cuspidal subgroup of
![]() $\Pi _{\mathrm {tpd}}$
that does not arise from a cusp of a fiber of
$\Pi _{\mathrm {tpd}}$
that does not arise from a cusp of a fiber of
![]() $p_{3/2}^{\mathrm {an}}$
;
$p_{3/2}^{\mathrm {an}}$
;
![]() $J^{*}_{\mathrm {tpd}}$
,
$J^{*}_{\mathrm {tpd}}$
,
![]() $J^{**}_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
cuspidal subgroups of
$J^{**}_{\mathrm {tpd}} \subseteq \Pi _{\mathrm {tpd}}$
cuspidal subgroups of
![]() $\Pi _{\mathrm {tpd}}$
such that
$\Pi _{\mathrm {tpd}}$
such that
![]() $I_{\mathrm {tpd}}$
,
$I_{\mathrm {tpd}}$
,
![]() $J^{*}_{\mathrm {tpd}}$
, and
$J^{*}_{\mathrm {tpd}}$
, and
![]() $J^{**}_{\mathrm {tpd}}$
determine three distinct
$J^{**}_{\mathrm {tpd}}$
determine three distinct
![]() $\Pi _{\mathrm {tpd}}$
-conjugacy classes of subgroups of
$\Pi _{\mathrm {tpd}}$
-conjugacy classes of subgroups of
![]() $\Pi _{\mathrm {tpd}}$
. (Note that one verifies immediately from the various definitions involved that such cuspidal subgroups
$\Pi _{\mathrm {tpd}}$
. (Note that one verifies immediately from the various definitions involved that such cuspidal subgroups
![]() $I_{\mathrm {tpd}}$
,
$I_{\mathrm {tpd}}$
,
![]() $J^{*}_{\mathrm {tpd}}$
, and
$J^{*}_{\mathrm {tpd}}$
, and
![]() $J^{**}_{\mathrm {tpd}}$
always exist.) For
$J^{**}_{\mathrm {tpd}}$
always exist.) For
![]() $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
(cf. the notational conventions introduced in the statement of Corollary 2.20), write
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
(cf. the notational conventions introduced in the statement of Corollary 2.20), write
for the automorphism of
![]() $\Pi _{1}^{\mathrm {disc}}$
determined by
$\Pi _{1}^{\mathrm {disc}}$
determined by
![]() $\alpha $
;
$\alpha $
;
for the subgroup consisting of
![]() $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
such that
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
such that
![]() $\beta (I) = I$
;
$\beta (I) = I$
;
for the subgroup consisting of
![]() $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
such that the image of
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}})$
such that the image of
![]() $\beta $
via the composite
$\beta $
via the composite
![]() $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}) \twoheadrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}) \stackrel {\sim }{\rightarrow } \mathrm {Out}^{\mathrm {FC}}(\Pi _{1}^{\mathrm {disc}}) \rightarrow \mathrm {Out}(\Pi _{\mathcal {G}^{\mathrm {disc}}})$
—where the second arrow is the natural bijection of Corollary 2.20(v), and the third arrow is the homomorphism induced by the natural outer isomorphism
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}) \twoheadrightarrow \mathrm {Out}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}) \stackrel {\sim }{\rightarrow } \mathrm {Out}^{\mathrm {FC}}(\Pi _{1}^{\mathrm {disc}}) \rightarrow \mathrm {Out}(\Pi _{\mathcal {G}^{\mathrm {disc}}})$
—where the second arrow is the natural bijection of Corollary 2.20(v), and the third arrow is the homomorphism induced by the natural outer isomorphism
![]() $\Pi _{1}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\mathrm {disc}}}$
—is graphic (cf. Definition 2.7(ii));
$\Pi _{1}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{\mathcal {G}^{\mathrm {disc}}}$
—is graphic (cf. Definition 2.7(ii));
for the set of cycle-subgroups of
![]() $\Pi _{1}^{\mathrm {disc}}$
(cf. Definition 3.13(i));
$\Pi _{1}^{\mathrm {disc}}$
(cf. Definition 3.13(i));
for the set of subgroups
![]() $T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
such that T is a tripodal subgroup associated with some cycle-subgroup of
$T \subseteq \Pi _{2/1}^{\mathrm {disc}}$
such that T is a tripodal subgroup associated with some cycle-subgroup of
![]() $\Pi _{1}^{\mathrm {disc}}$
(cf. Definition 3.13(ii)), and, moreover, I is a distinguished cuspidal subgroup (cf. Definition 3.13(iii)) of T. Then the following hold:
$\Pi _{1}^{\mathrm {disc}}$
(cf. Definition 3.13(ii)), and, moreover, I is a distinguished cuspidal subgroup (cf. Definition 3.13(iii)) of T. Then the following hold:
-
(i) Let
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
,
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
,
 $J\in \mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
, and
$J\in \mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
, and
 $T \in \mathrm {Tpd}_I(\Pi _{2/1}^{\mathrm {disc}})$
. Then it holds that Thus,
$T \in \mathrm {Tpd}_I(\Pi _{2/1}^{\mathrm {disc}})$
. Then it holds that Thus, $$ \begin{align*}\alpha_1(J)\in \mathrm{Cycle}^{}(\Pi_{1}^{\mathrm{disc}}), \quad \alpha(T) \in \mathrm{Tpd}_I(\Pi_{2/1}^{\mathrm{disc}}) .\end{align*} $$
$$ \begin{align*}\alpha_1(J)\in \mathrm{Cycle}^{}(\Pi_{1}^{\mathrm{disc}}), \quad \alpha(T) \in \mathrm{Tpd}_I(\Pi_{2/1}^{\mathrm{disc}}) .\end{align*} $$
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
acts naturally on
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
acts naturally on
 $\mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
,
$\mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
,
 $\mathrm {Tpd}_I(\Pi _{2/1}^{\mathrm {disc}})$
.
$\mathrm {Tpd}_I(\Pi _{2/1}^{\mathrm {disc}})$
.
-
(ii) There exists a unique
 $\mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
-equivariant (cf. (i)) map such that, for every
$\mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
-equivariant (cf. (i)) map such that, for every $$ \begin{align*}\mathfrak{C}_I \colon \mathrm{Cycle}^{}(\Pi_{1}^{\mathrm{disc}}) \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1}^{\mathrm{disc}}) \end{align*} $$
$$ \begin{align*}\mathfrak{C}_I \colon \mathrm{Cycle}^{}(\Pi_{1}^{\mathrm{disc}}) \longrightarrow \mathrm{Tpd}_I(\Pi_{2/1}^{\mathrm{disc}}) \end{align*} $$
 $J\in \mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
,
$J\in \mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
,
 $\mathfrak {C}_I(J)$
is a tripodal subgroup associated with J. Moreover, for every
$\mathfrak {C}_I(J)$
is a tripodal subgroup associated with J. Moreover, for every
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
and
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
and
 $J\in \mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
, the isomorphism
$J\in \mathrm {Cycle}^{}(\Pi _{1}^{\mathrm {disc}})$
, the isomorphism
 $\mathfrak {C}_I(J) \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(\alpha _1(J))$
induced by
$\mathfrak {C}_I(J) \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(\alpha _1(J))$
induced by
 $\alpha $
maps every lifting cycle-subgroup (cf. Definition 3.13(iii)) of
$\alpha $
maps every lifting cycle-subgroup (cf. Definition 3.13(iii)) of
 $\mathfrak {C}_I(J)$
bijectively onto a lifting cycle-subgroup of
$\mathfrak {C}_I(J)$
bijectively onto a lifting cycle-subgroup of
 $\mathfrak {C}_I(\alpha _1(J))$
.
$\mathfrak {C}_I(\alpha _1(J))$
.
-
(iii) There exists an assignment
 $$\begin{align*}\mathrm{Cycle}^{}(\Pi_{1}^{\mathrm{disc}}) \ni J \, \, \mapsto \, \, \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
$$\begin{align*}\mathrm{Cycle}^{}(\Pi_{1}^{\mathrm{disc}}) \ni J \, \, \mapsto \, \, \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
—where
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
denotes an I-conjugacy class of isomorphisms
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
denotes an I-conjugacy class of isomorphisms
 $\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
—such that
$\Pi _{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } \mathfrak {C}_I(J)$
—such that-
(a)
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps
 $I_{\mathrm {tpd}}$
bijectively onto I in a fashion that is compatible with the natural isomorphism
$I_{\mathrm {tpd}}$
bijectively onto I in a fashion that is compatible with the natural isomorphism
 $I_{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } I$
induced by the projection
$I_{\mathrm {tpd}} \stackrel {\sim }{\rightarrow } I$
induced by the projection
 $p_{\{1,2,3\}/\{1, 3\}}^{\Pi ^{\mathrm {disc}}}\colon \Pi _{3}^{\mathrm {disc}} \twoheadrightarrow \Pi _{\{1, 3\}}^{\mathrm {disc}}$
and the natural outer isomorphism
$p_{\{1,2,3\}/\{1, 3\}}^{\Pi ^{\mathrm {disc}}}\colon \Pi _{3}^{\mathrm {disc}} \twoheadrightarrow \Pi _{\{1, 3\}}^{\mathrm {disc}}$
and the natural outer isomorphism
 $\Pi _{\{1, 3\}}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{\{1, 2\}}^{\mathrm {disc}}$
obtained by switching the labels “
$\Pi _{\{1, 3\}}^{\mathrm {disc}} \stackrel {\sim }{\rightarrow } \Pi _{\{1, 2\}}^{\mathrm {disc}}$
obtained by switching the labels “
 $2$
” and “
$2$
” and “
 $3$
” (cf. Corollary 2.17(ii); Corollary 2.18(v); [Reference Hoshi and Mochizuki10, Lem. 3.6(iv)]),
$3$
” (cf. Corollary 2.17(ii); Corollary 2.18(v); [Reference Hoshi and Mochizuki10, Lem. 3.6(iv)]), -
(b)
 $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps the subgroups
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I, J}$
maps the subgroups
 $J^{*}_{\mathrm {tpd}}$
,
$J^{*}_{\mathrm {tpd}}$
,
 $J^{**}_{\mathrm {tpd}}$
bijectively onto lifting cycle-subgroups of
$J^{**}_{\mathrm {tpd}}$
bijectively onto lifting cycle-subgroups of
 $\mathfrak {C}_I(J)$
, and
$\mathfrak {C}_I(J)$
, and -
(c) for
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
, the diagram (of
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
, the diagram (of
 $I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms)
$I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) 
—where the upper horizontal arrow is the (uniquely determined—cf. the commensurable terminality of
 $I_{\mathrm {tpd}}$
of
$I_{\mathrm {tpd}}$
of
 $\Pi _{\mathrm {tpd}}$
discussed in Corollary 2.18(v))
$\Pi _{\mathrm {tpd}}$
discussed in Corollary 2.18(v))
 $I_{\mathrm {tpd}}$
-conjugacy class of automorphisms of
$I_{\mathrm {tpd}}$
-conjugacy class of automorphisms of
 $\Pi _{\mathrm {tpd}}$
that lifts
$\Pi _{\mathrm {tpd}}$
that lifts
 $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )$
(cf. Corollary 2.20(v); Theorem 2.24(iv)) and preserves
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\alpha )$
(cf. Corollary 2.20(v); Theorem 2.24(iv)) and preserves
 $I_{\mathrm {tpd}}$
; the lower horizontal arrow is the I-conjugacy class of isomorphisms induced by
$I_{\mathrm {tpd}}$
; the lower horizontal arrow is the I-conjugacy class of isomorphisms induced by
 $\alpha $
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
$\alpha $
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
 $\mathfrak {C}_I(\alpha _1(J))$
associated with I (cf. Definition 3.13(iv)).
$\mathfrak {C}_I(\alpha _1(J))$
associated with I (cf. Definition 3.13(iv)).
Finally, the assignment
is uniquely determined, up to possible composition with cycle symmetries, by these conditions (a), (b), and (c). $$\begin{align*}J\mapsto \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
$$\begin{align*}J\mapsto \mathfrak{s}\mathfrak{y}\mathfrak{n}_{I, J} \end{align*}$$
-
-
(iv) Let
 $\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
and
$\alpha \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
and
 $J\in \mathrm {Cycle}^{}(\Pi _{1})$
. Then there exists an automorphism
$J\in \mathrm {Cycle}^{}(\Pi _{1})$
. Then there exists an automorphism
 $\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
such that
$\beta \in \mathrm {Aut}^{\mathrm {FC}}(\Pi _{2}^{\mathrm {disc}}, I)^{\mathrm {G}}$
such that
 $\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta )$
(cf. Corollary 2.20(v); Theorem 2.24(iv)) is trivial, and, moreover,
$\mathfrak {T}_{\Pi _{\mathrm {tpd}}}(\beta )$
(cf. Corollary 2.20(v); Theorem 2.24(iv)) is trivial, and, moreover,
 $\alpha _1(J) = \beta _1(J)$
. Finally, the diagram (of
$\alpha _1(J) = \beta _1(J)$
. Finally, the diagram (of
 $I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms)
$I_{\mathrm {tpd}}$
-, I-conjugacy classes of isomorphisms) 
—where the lower horizontal arrow is the isomorphism induced by
 $\beta $
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
$\beta $
(cf. (ii))—commutes up to possible composition with the cycle symmetry of
 $\mathfrak {C}_I(\alpha _1(J))=\mathfrak {C}_I(\beta _1(J))$
associated with I.
$\mathfrak {C}_I(\alpha _1(J))=\mathfrak {C}_I(\beta _1(J))$
associated with I.
Proof. Assertion (i) follows from the various definitions involved. Assertion (ii) follows immediately from the evident discrete version (cf. Corollaries 2.17(ii); 2.19(i)) of the argument involving Remark 3.6.1 that was given in the proof of Theorem 3.10,(ii). The existence portion of assertion (iii) follows, in light of Corollaries 2.17(ii); 2.20(i) and (v), from a similar argument to the argument applied in the proof of the existence portion of Theorem 3.10(iii) (cf. also the fact that the “
![]() $\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
” of Theorem 3.10(iii) was constructed from a suitable geometric outer isomorphism). The uniqueness portion of assertion (iii) follows from the compatibility portion of condition (a), together with the computation of discrete outomorphism groups given in Theorem 2.24(ii). Assertion (iv) follows immediately from assertion (iii), together with a similar argument to the argument applied in the proof of the surjectivity portion of Theorem 2.24(iv) (cf. the argument given in the proof of Theorem 3.10(iv)). This completes the proof of Theorem 3.14.
$\mathfrak {s}\mathfrak {y}\mathfrak {n}_{I,J}$
” of Theorem 3.10(iii) was constructed from a suitable geometric outer isomorphism). The uniqueness portion of assertion (iii) follows from the compatibility portion of condition (a), together with the computation of discrete outomorphism groups given in Theorem 2.24(ii). Assertion (iv) follows immediately from assertion (iii), together with a similar argument to the argument applied in the proof of the surjectivity portion of Theorem 2.24(iv) (cf. the argument given in the proof of Theorem 3.10(iv)). This completes the proof of Theorem 3.14.
Remark 3.14.1. One verifies immediately that the discrete constructions of Theorem 3.14(i)–(iv) are compatible, in an evident sense, with the pro-
![]() $\Sigma $
constructions of Theorem 3.10(i)–(iv). We leave the routine details to the reader.
$\Sigma $
constructions of Theorem 3.10(i)–(iv). We leave the routine details to the reader.
Remark 3.14.2. One verifies immediately that remarks analogous to Remarks 3.6.2 and 3.10.1 in the profinite case may be made in the discrete situation treated in Theorem 3.14. In this context, we observe that the theory of the “modules of local orientations
![]() $\Lambda $
” developed in [Reference Hoshi and Mochizuki9, §3] admits a straightforward discrete analogue, which may be applied to conclude that the “orientation isomorphisms
$\Lambda $
” developed in [Reference Hoshi and Mochizuki9, §3] admits a straightforward discrete analogue, which may be applied to conclude that the “orientation isomorphisms
![]() $J \stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
” of Remark 3.6.2(i) are compatible with the natural discrete structures on the domain and codomain. Alternatively, in the discrete case, relative to the notation of Definition 2.2(iii), one may think of these modules “
$J \stackrel {\sim }{\rightarrow } \Lambda _{\mathcal {G}}$
” of Remark 3.6.2(i) are compatible with the natural discrete structures on the domain and codomain. Alternatively, in the discrete case, relative to the notation of Definition 2.2(iii), one may think of these modules “
![]() $\Lambda $
” as the
$\Lambda $
” as the
![]() $\mathbb {Z}$
-duals of the second relative singular cohomology modules (with
$\mathbb {Z}$
-duals of the second relative singular cohomology modules (with
![]() $\mathbb {Z}$
-coefficients)
$\mathbb {Z}$
-coefficients)
—cf. the discussion of orientations in [Reference Hoshi and Mochizuki9, Introduction]. Then the discrete version of the key isomorphisms (cf. the constructions of Remark 3.6.2) of [Reference Hoshi and Mochizuki9, Cor. 3.9(v) and (vi)] may be obtained by considering the connecting homomorphism (from first to second cohomology modules) in the long exact cohomology sequence associated with the pair
![]() $(U_X,\partial U_X)$
. We leave the routine details to the reader.
$(U_X,\partial U_X)$
. We leave the routine details to the reader.
Appendix. Explicit limit seminorms associated with sequences of toric surfaces
In the proof of Corollary 1.15(ii), we considered sequences of discrete valuations that arose from vertices or edges of the dual semi-graphs associated with the geometric special fibers of a tower of coverings of stable log curves and, in particular, observed that the convergence of a suitable subsequence of such a sequence follows immediately from the general theory of Berkovich spaces. In the present appendix, we reexamine this convergence phenomenon from a more elementary and explicit—albeit logically unnecessary, from the point of view of proving Corollary 1.15(ii)!—point of view that only requires a knowledge of elementary facts concerning log regular log schemes, that is, without applying the terminology and notions (e.g., of “Stone–Čech compactifications”) that frequently appear in the general theory of Berkovich spaces (cf. the proof of [Reference Berkovich2, Th. 1.2.1]). In particular, we discuss the notion of a “stratum” of a “toric surface” (cf. Definition A.1 below), which generalizes the notion of a vertex or edge of the dual graph of the special fiber of a stable curve over a complete discrete valuation ring. We observe that such a stratum determines a discrete valuation (cf. Definition A.4) and consider, at a quite explicit level, the limit of a suitable subsequence of a given sequence of such discrete valuations (cf. Theorem A.7 below). The material presented in this appendix is quite elementary and “well-known,” but we chose to include it in the present paper since we were unable to find a suitable reference that discusses this material from a similar point of view.
In the present appendix, let R be a complete discrete valuation ring. Write K for the field of fractions of R and
![]() $\mathcal {S}^{\mathrm {log}}$
for the log scheme obtained by equipping
$\mathcal {S}^{\mathrm {log}}$
for the log scheme obtained by equipping
![]() $\mathcal {S} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(R)$
with the log structure determined by the unique closed point of
$\mathcal {S} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(R)$
with the log structure determined by the unique closed point of
![]() $\mathcal {S}$
.
$\mathcal {S}$
.
Definition A.1.
-
(i) We shall refer to an fs log scheme
 $\mathcal {X}^{\mathrm {log}}$
over
$\mathcal {X}^{\mathrm {log}}$
over
 $\mathcal {S}^{\mathrm {log}}$
as a toric surface over
$\mathcal {S}^{\mathrm {log}}$
as a toric surface over
 $\mathcal {S}^{\mathrm {log}}$
if the following conditions are satisfied:
$\mathcal {S}^{\mathrm {log}}$
if the following conditions are satisfied:-
(a) The underlying scheme
 $\mathcal {X}$
of
$\mathcal {X}$
of
 $\mathcal {X}^{\mathrm {log}}$
is of finite type, flat, and of pure relative dimension one (i.e., every irreducible component of every fiber of the underlying morphism of schemes
$\mathcal {X}^{\mathrm {log}}$
is of finite type, flat, and of pure relative dimension one (i.e., every irreducible component of every fiber of the underlying morphism of schemes
 $\mathcal {X} \rightarrow \mathcal {S}$
is of dimension one) over
$\mathcal {X} \rightarrow \mathcal {S}$
is of dimension one) over
 $\mathcal {S}$
.
$\mathcal {S}$
. -
(b) The fs log scheme
 $\mathcal {X}^{\mathrm {log}}$
is log regular.
$\mathcal {X}^{\mathrm {log}}$
is log regular. -
(c) The interior (cf., e.g., [Reference Mochizuki and Tamagawa23, Def. 5.1(i)]) of the log scheme
 $\mathcal {X}^{\mathrm {log}}$
is equal to the open subscheme
$\mathcal {X}^{\mathrm {log}}$
is equal to the open subscheme
 $\mathcal {X}\times _R K\subseteq \mathcal {X}$
.
$\mathcal {X}\times _R K\subseteq \mathcal {X}$
.
Given two toric surface s over
 $\mathcal {S}^{\mathrm {log}}$
, there is an evident notion of isomorphism of toric surfaces over
$\mathcal {S}^{\mathrm {log}}$
, there is an evident notion of isomorphism of toric surfaces over
 $\mathcal {S}^{\mathrm {log}}$
.
$\mathcal {S}^{\mathrm {log}}$
. -
-
(ii) Let
 $\mathcal {X}^{\mathrm {log}} $
be a toric surface over
$\mathcal {X}^{\mathrm {log}} $
be a toric surface over
 $\mathcal {S}^{\mathrm {log}}$
(cf. (i)) and n a nonnegative integer. Write
$\mathcal {S}^{\mathrm {log}}$
(cf. (i)) and n a nonnegative integer. Write
 $\mathcal {X}^{[n]} \subseteq \mathcal {X}$
for the n-interior of
$\mathcal {X}^{[n]} \subseteq \mathcal {X}$
for the n-interior of
 $\mathcal {X}^{\mathrm {log}}$
(cf. [Reference Mochizuki and Tamagawa23, Def. 5.1(i)]) and
$\mathcal {X}^{\mathrm {log}}$
(cf. [Reference Mochizuki and Tamagawa23, Def. 5.1(i)]) and
 $\mathcal {X}^{[-1]} \subseteq \mathcal {X}$
for the empty subscheme. Then we shall refer to a connected component of
$\mathcal {X}^{[-1]} \subseteq \mathcal {X}$
for the empty subscheme. Then we shall refer to a connected component of
 $\mathcal {X}^{[n]} \setminus \mathcal {X}^{[n-1]}$
as an n-stratum of
$\mathcal {X}^{[n]} \setminus \mathcal {X}^{[n-1]}$
as an n-stratum of
 $\mathcal {X}^{\mathrm {log}}$
. We shall write for the set of n-strata of
$\mathcal {X}^{\mathrm {log}}$
. We shall write for the set of n-strata of $$\begin{align*}\mathrm{Str}^{n}(\mathcal{X}^{\mathrm{log}}) \end{align*}$$
$$\begin{align*}\mathrm{Str}^{n}(\mathcal{X}^{\mathrm{log}}) \end{align*}$$
 $\mathcal {X}^{\mathrm {log}}$
(so
$\mathcal {X}^{\mathrm {log}}$
(so
 $\mathrm {Str}^{n}(\mathcal {X}^{\mathrm {log}}) = \emptyset $
if
$\mathrm {Str}^{n}(\mathcal {X}^{\mathrm {log}}) = \emptyset $
if
 $n\geq 3$
) and
$n\geq 3$
) and  $$\begin{align*}\mathrm{Str}^{}(\mathcal{X}^{\mathrm{log}})\ \stackrel{\mathrm{def}}{=}\ \mathrm{Str}^{1}(\mathcal{X}^{\mathrm{log}})\ \sqcup\ \mathrm{Str}^{2}(\mathcal{X}^{\mathrm{log}}). \end{align*}$$
$$\begin{align*}\mathrm{Str}^{}(\mathcal{X}^{\mathrm{log}})\ \stackrel{\mathrm{def}}{=}\ \mathrm{Str}^{1}(\mathcal{X}^{\mathrm{log}})\ \sqcup\ \mathrm{Str}^{2}(\mathcal{X}^{\mathrm{log}}). \end{align*}$$
Definition A.2. Let I be a totally ordered set that is isomorphic to
![]() $\mathbb {N}$
(equipped with its usual ordering). In particular, it makes sense to speak of “limits
$\mathbb {N}$
(equipped with its usual ordering). In particular, it makes sense to speak of “limits
![]() $i\to \infty $
” of collections of objects indexed by
$i\to \infty $
” of collections of objects indexed by
![]() $i\in I$
, as well as to speak of the “next largest element”
$i\in I$
, as well as to speak of the “next largest element”
![]() $i+1 \in I$
associated with a given element
$i+1 \in I$
associated with a given element
![]() $i\in I$
. Then we shall refer to a sequence of fs log schemes
$i\in I$
. Then we shall refer to a sequence of fs log schemes
—where i ranges over the elements of I —over
![]() $\mathcal {S}^{\mathrm {log}}$
(indexed by I) as a sequence of toric surface s over
$\mathcal {S}^{\mathrm {log}}$
(indexed by I) as a sequence of toric surface s over
![]() $\mathcal {S}^{\mathrm {log}}$
if, for each
$\mathcal {S}^{\mathrm {log}}$
if, for each
![]() $i\in I$
,
$i\in I$
,
![]() $\mathcal {X}_i^{\mathrm {log}}$
is a toric surface over
$\mathcal {X}_i^{\mathrm {log}}$
is a toric surface over
![]() $\mathcal {S}^{\mathrm {log}}$
(cf. Definition A.1(i)), and, moreover, the morphism
$\mathcal {S}^{\mathrm {log}}$
(cf. Definition A.1(i)), and, moreover, the morphism
![]() $\mathcal {X}_{i+1}^{\mathrm {log}} \rightarrow \mathcal {X}_i^{\mathrm {log}}$
is dominant. Observe that the horizontal arrows of the above diagram determine (by considering the induced maps of generic points of strata) a sequence of maps of sets
$\mathcal {X}_{i+1}^{\mathrm {log}} \rightarrow \mathcal {X}_i^{\mathrm {log}}$
is dominant. Observe that the horizontal arrows of the above diagram determine (by considering the induced maps of generic points of strata) a sequence of maps of sets
Finally, given two sequences of toric surface s over
![]() $\mathcal {S}^{\mathrm {log}}$
, there is an evident notion of isomorphism of sequences of toric surface s over
$\mathcal {S}^{\mathrm {log}}$
, there is an evident notion of isomorphism of sequences of toric surface s over
![]() $\mathcal {S}^{\mathrm {log}}$
.
$\mathcal {S}^{\mathrm {log}}$
.
Definition A.3. Let
![]() $\mathcal {X}^{\mathrm {log}}$
be a toric surface over
$\mathcal {X}^{\mathrm {log}}$
be a toric surface over
![]() $\mathcal {S}^{\mathrm {log}}$
and A a strict henselization of
$\mathcal {S}^{\mathrm {log}}$
and A a strict henselization of
![]() $\mathcal {X}$
at (the closed point determined by)
$\mathcal {X}$
at (the closed point determined by)
![]() $z \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}})$
(cf. Definition A.1(i) and (ii)). Write F for the field of fractions of A; k for the residue field of A;
$z \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}})$
(cf. Definition A.1(i) and (ii)). Write F for the field of fractions of A; k for the residue field of A;
![]() $\mathfrak {m}_A$
for the maximal ideal of A;
$\mathfrak {m}_A$
for the maximal ideal of A;
![]() $\mathcal {X}_z \stackrel {\mathrm {def}}{=} \mathrm {Spec}(A)$
;
$\mathcal {X}_z \stackrel {\mathrm {def}}{=} \mathrm {Spec}(A)$
;
![]() $\mathcal {M}_{\mathcal {X}}$
for the sheaf of monoids on
$\mathcal {M}_{\mathcal {X}}$
for the sheaf of monoids on
![]() $\mathcal {X}$
that defines the log structure of
$\mathcal {X}$
that defines the log structure of
![]() $\mathcal {X}^{\mathrm {log}}$
; M for the fiber of
$\mathcal {X}^{\mathrm {log}}$
; M for the fiber of
![]() $\mathcal {M}_{\mathcal {X}}/\mathcal {O}^{\times }_{\mathcal {X}}$
at the maximal ideal of A;
$\mathcal {M}_{\mathcal {X}}/\mathcal {O}^{\times }_{\mathcal {X}}$
at the maximal ideal of A;
—where we write
![]() $\mathbb {Q}_{\geq 0}$
,
$\mathbb {Q}_{\geq 0}$
,
![]() $\mathbb {R}_{\geq 0}$
for the respective submonoids determined by the nonnegative elements of the (additive groups)
$\mathbb {R}_{\geq 0}$
for the respective submonoids determined by the nonnegative elements of the (additive groups)
![]() $\mathbb {Q}$
,
$\mathbb {Q}$
,
![]() $\mathbb {R}$
and “
$\mathbb {R}$
and “
![]() $\mathrm {Hom}(M, -)$
” for the monoid consisting of homomorphisms of monoids from M to “
$\mathrm {Hom}(M, -)$
” for the monoid consisting of homomorphisms of monoids from M to “
![]() $(-)$
.” Thus, one verifies easily that V is equipped with a natural structure of two-dimensional vector space over
$(-)$
.” Thus, one verifies easily that V is equipped with a natural structure of two-dimensional vector space over
![]() $\mathbb {R}$
. In the following, we shall use the superscript “gp” to denote the groupification of any of the monoids of the above discussion.
$\mathbb {R}$
. In the following, we shall use the superscript “gp” to denote the groupification of any of the monoids of the above discussion.
-
(i) We shall say that a submonoid
 $L \subseteq P$
of P is a P-ray if L is the
$L \subseteq P$
of P is a P-ray if L is the
 $\mathbb {R}_{\geq 0}$
-orbit of some nonzero element of P, relative to the natural (multiplicative) action of
$\mathbb {R}_{\geq 0}$
-orbit of some nonzero element of P, relative to the natural (multiplicative) action of
 $\mathbb {R}_{\geq 0}$
on P.
$\mathbb {R}_{\geq 0}$
on P. -
(ii) We shall say that a P-ray
 $L\subseteq P$
(cf. (i)) is rational (resp. irrational) if
$L\subseteq P$
(cf. (i)) is rational (resp. irrational) if
 $L \cap Q \neq \{0\}$
(resp.
$L \cap Q \neq \{0\}$
(resp.
 $L\cap Q = \{0\}$
).
$L\cap Q = \{0\}$
). -
(iii) Let
 $L\subseteq P$
be a rational P-ray (cf. (i) and (ii)). Then we shall write
$L\subseteq P$
be a rational P-ray (cf. (i) and (ii)). Then we shall write
 $v_L\colon F^{\times } \rightarrow \mathbb {Q} \subseteq \mathbb {R}$
for the discrete valuation associated with the irreducible component of the blowup of
$v_L\colon F^{\times } \rightarrow \mathbb {Q} \subseteq \mathbb {R}$
for the discrete valuation associated with the irreducible component of the blowup of
 $\mathcal {X}_z$
associated with
$\mathcal {X}_z$
associated with
 $L\subseteq P$
, normalized so as to map each prime element
$L\subseteq P$
, normalized so as to map each prime element
 $\pi _R$
of
$\pi _R$
of
 $R \subseteq F$
to
$R \subseteq F$
to
 $1\in \mathbb {Q}$
. That is to say, if
$1\in \mathbb {Q}$
. That is to say, if
 $\lambda \in L$
(which, by a slight abuse of notation, we regard as a homomorphism
$\lambda \in L$
(which, by a slight abuse of notation, we regard as a homomorphism
 $M^{\mathrm {gp}} \rightarrow \mathbb {R}$
) maps
$M^{\mathrm {gp}} \rightarrow \mathbb {R}$
) maps
 $\pi _R \mapsto 1\in \mathbb {Q}$
(so
$\pi _R \mapsto 1\in \mathbb {Q}$
(so
 $\lambda \in L \cap Q$
), and
$\lambda \in L \cap Q$
), and
 $f\in F$
lies in the
$f\in F$
lies in the
 $A^\times $
-orbit determined by
$A^\times $
-orbit determined by
 $m\in M^{\mathrm {gp}}$
, then Here, we observe that (one verifies easily that) the submonoid
$m\in M^{\mathrm {gp}}$
, then Here, we observe that (one verifies easily that) the submonoid $$\begin{align*}v_L(f)\ =\ \lambda(m)\ \in\ \mathbb{Q}. \end{align*}$$
$$\begin{align*}v_L(f)\ =\ \lambda(m)\ \in\ \mathbb{Q}. \end{align*}$$
 $M_L \stackrel {\mathrm {def}}{=}\lambda ^{-1}(\mathbb {Q}_{\geq 0}) \subseteq M^{\mathrm {gp}}$
is isomorphic to
$M_L \stackrel {\mathrm {def}}{=}\lambda ^{-1}(\mathbb {Q}_{\geq 0}) \subseteq M^{\mathrm {gp}}$
is isomorphic to
 $\mathbb {Z} \times \mathbb {N}$
. In particular, if we denote by
$\mathbb {Z} \times \mathbb {N}$
. In particular, if we denote by
 $F_L \subseteq F$
the set of
$F_L \subseteq F$
the set of
 $f\in F$
that lie in the
$f\in F$
that lie in the
 $A^\times $
-orbits determined by
$A^\times $
-orbits determined by
 $m \in M_L$
and write
$m \in M_L$
and write
 $A_L\subseteq F$
for the A-subalgebra generated by
$A_L\subseteq F$
for the A-subalgebra generated by
 $f\in F_L$
, then the “blowup of
$f\in F_L$
, then the “blowup of
 $\mathcal {X}_z$
associated with L” referred to above may be described explicitly as Indeed, if we write
$\mathcal {X}_z$
associated with L” referred to above may be described explicitly as Indeed, if we write $$\begin{align*}\mathcal{X}_L\ \stackrel{\mathrm{def}}{=}\ \mathrm{Spec}(A_L)\ \longrightarrow\ \mathcal{X}_z. \end{align*}$$
$$\begin{align*}\mathcal{X}_L\ \stackrel{\mathrm{def}}{=}\ \mathrm{Spec}(A_L)\ \longrightarrow\ \mathcal{X}_z. \end{align*}$$
 $\mathfrak {p}_L \subseteq A_L$
for the ideal generated by the set of
$\mathfrak {p}_L \subseteq A_L$
for the ideal generated by the set of
 $f \in F$
that lie in the
$f \in F$
that lie in the
 $A^\times $
-orbits determined by the noninvertible elements
$A^\times $
-orbits determined by the noninvertible elements
 $m\in M_L$
, then it follows immediately from the simple structure of the monoid
$m\in M_L$
, then it follows immediately from the simple structure of the monoid
 $\mathbb {Z} \times \mathbb {N}$
that
$\mathbb {Z} \times \mathbb {N}$
that
 $\mathfrak {p}_L$
is the prime ideal of height one in
$\mathfrak {p}_L$
is the prime ideal of height one in
 $A_L$
that corresponds to the discrete valuation
$A_L$
that corresponds to the discrete valuation
 $v_L$
, and that the k-algebra
$v_L$
, and that the k-algebra
 $A_L/\mathfrak {p}_L$
is isomorphic to
$A_L/\mathfrak {p}_L$
is isomorphic to
 $k[U,U^{-1}]$
, where U is an indeterminate.
$k[U,U^{-1}]$
, where U is an indeterminate.
-
(iv) Write
 $\mathcal {M}_{\mathcal {S}}$
for the sheaf of monoids on
$\mathcal {M}_{\mathcal {S}}$
for the sheaf of monoids on
 $\mathcal {S}$
that defines the log structure of
$\mathcal {S}$
that defines the log structure of
 $\mathcal {S}^{\mathrm {log}}$
;
$\mathcal {S}^{\mathrm {log}}$
;
 $M_R$
for the fiber of
$M_R$
for the fiber of
 $\mathcal {M}_{\mathcal {S}}/\mathcal {O}^{\times }_{\mathcal {S}}$
at the unique closed point of
$\mathcal {M}_{\mathcal {S}}/\mathcal {O}^{\times }_{\mathcal {S}}$
at the unique closed point of
 $\mathcal {S}$
;
$\mathcal {S}$
;
 $V_R \stackrel {\mathrm {def}}{=} \mathrm {Hom}(M_R, \mathbb {R})$
. Then one verifies easily that
$V_R \stackrel {\mathrm {def}}{=} \mathrm {Hom}(M_R, \mathbb {R})$
. Then one verifies easily that
 $V_R$
is a one-dimensional vector space over
$V_R$
is a one-dimensional vector space over
 $\mathbb {R}$
, and that the morphism
$\mathbb {R}$
, and that the morphism
 $\mathcal {X}^{\mathrm {log}} \rightarrow \mathcal {S}^{\mathrm {log}}$
determines an
$\mathcal {X}^{\mathrm {log}} \rightarrow \mathcal {S}^{\mathrm {log}}$
determines an
 $\mathbb {R}$
-linear surjection
$\mathbb {R}$
-linear surjection
 $V \twoheadrightarrow V_R$
. Let
$V \twoheadrightarrow V_R$
. Let
 $e_\alpha $
,
$e_\alpha $
,
 $e_\beta \in P$
be such that
$e_\beta \in P$
be such that
 $ \mathbb {R}_{\geq 0} \cdot e_\alpha + \mathbb {R}_{\geq 0} \cdot e_\beta = P$
, and, moreover, the images of
$ \mathbb {R}_{\geq 0} \cdot e_\alpha + \mathbb {R}_{\geq 0} \cdot e_\beta = P$
, and, moreover, the images of
 $e_\alpha $
,
$e_\alpha $
,
 $e_\beta $
in
$e_\beta $
in
 $V_R$
coincide. (Note that the existence of such elements
$V_R$
coincide. (Note that the existence of such elements
 $e_\alpha $
,
$e_\alpha $
,
 $e_\beta \in P$
follows, for example, from [Reference Mochizuki14, Prop. 1.7].) Then we shall refer to the (necessarily rational—cf. (ii)) P-ray
$e_\beta \in P$
follows, for example, from [Reference Mochizuki14, Prop. 1.7].) Then we shall refer to the (necessarily rational—cf. (ii)) P-ray
 $\mathbb {R}_{\geq 0} \cdot (e_\alpha + e_\beta ) \subseteq P$
(cf. (i)) as the midpoint P-ray at
$\mathbb {R}_{\geq 0} \cdot (e_\alpha + e_\beta ) \subseteq P$
(cf. (i)) as the midpoint P-ray at
 $z \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}})$
. Here, we note that one verifies easily that the P-ray
$z \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}})$
. Here, we note that one verifies easily that the P-ray
 $\mathbb {R}_{\geq 0} \cdot (e_\alpha + e_\beta )$
does not depend on the choice of the pair
$\mathbb {R}_{\geq 0} \cdot (e_\alpha + e_\beta )$
does not depend on the choice of the pair
 $(e_\alpha , e_\beta )$
.
$(e_\alpha , e_\beta )$
. -
(v) We shall refer to a valuation
 $w\colon F^\times \rightarrow \mathbb {R}$
as admissible if w dominates A and maps each prime element
$w\colon F^\times \rightarrow \mathbb {R}$
as admissible if w dominates A and maps each prime element
 $\pi _R$
of
$\pi _R$
of
 $R\subseteq F$
to
$R\subseteq F$
to
 $1\in \mathbb {R}$
. Let w be an admissible valuation. Then, by restricting w to the elements
$1\in \mathbb {R}$
. Let w be an admissible valuation. Then, by restricting w to the elements
 $f\in F$
that lie in the
$f\in F$
that lie in the
 $A^\times $
-orbits determined by
$A^\times $
-orbits determined by
 $m\in M$
, one obtains a nonzero homomorphism of monoids
$m\in M$
, one obtains a nonzero homomorphism of monoids
 $M \rightarrow \mathbb {R}_{\geq 0}$
, that is, an element of P. We shall refer to the P-ray
$M \rightarrow \mathbb {R}_{\geq 0}$
, that is, an element of P. We shall refer to the P-ray
 $L_w$
determined by this element of P as the P-ray associated with the admissible valuation w. Thus, if
$L_w$
determined by this element of P as the P-ray associated with the admissible valuation w. Thus, if
 $L_w$
is rational (cf. (ii)), then it follows immediately from the definitions that, in the notation of (iii), the valuation of A determined by w extends to a valuation of
$L_w$
is rational (cf. (ii)), then it follows immediately from the definitions that, in the notation of (iii), the valuation of A determined by w extends to a valuation of
 $A_{L_w} (\supseteq A)$
.
$A_{L_w} (\supseteq A)$
.
Remark A.3.1. In the notation of Definition A.3, the usual topology on the real vector space V naturally determines a topology on the subspace
![]() $P \subseteq V$
, as well as on the set of P-rays (i.e., which may be regarded as the complement of the “zero element” in the quotient space
$P \subseteq V$
, as well as on the set of P-rays (i.e., which may be regarded as the complement of the “zero element” in the quotient space
![]() $P / \mathbb {R}_{\geq 0}$
). Moreover, one verifies easily that, if
$P / \mathbb {R}_{\geq 0}$
). Moreover, one verifies easily that, if
![]() $e_\alpha $
and
$e_\alpha $
and
![]() $e_\beta $
are as in Definition A.3(iv), then the assignment
$e_\beta $
are as in Definition A.3(iv), then the assignment
determines a homeomorphism of the closed interval
![]() $[0,1] \subseteq \mathbb {R}$
onto the resulting topological space of P-rays, and that the subset of rational P-rays is dense in the space of P-rays. In particular, it makes sense to speak of non-extremal (resp. extremal) P-rays, that is, P-rays that lie (resp. do not lie) in the interior—that is, relative to the homeomorphism just discussed, the open interval
$[0,1] \subseteq \mathbb {R}$
onto the resulting topological space of P-rays, and that the subset of rational P-rays is dense in the space of P-rays. In particular, it makes sense to speak of non-extremal (resp. extremal) P-rays, that is, P-rays that lie (resp. do not lie) in the interior—that is, relative to the homeomorphism just discussed, the open interval
![]() $(0,1) \subseteq [0,1]$
(resp. the endpoints
$(0,1) \subseteq [0,1]$
(resp. the endpoints
![]() $\{0,1\} \subseteq [0,1]$
)—of the space of P-rays. Finally, we observe that the two extremal P-rays are rational, and that a rational P-ray is non-extremal if and only if its associated discrete valuation (cf. Definition A.3(iii)) is admissible (cf. Definition A.3(v)).
$\{0,1\} \subseteq [0,1]$
)—of the space of P-rays. Finally, we observe that the two extremal P-rays are rational, and that a rational P-ray is non-extremal if and only if its associated discrete valuation (cf. Definition A.3(iii)) is admissible (cf. Definition A.3(v)).
Definition A.4. Let
![]() $\mathcal {X}^{\mathrm {log}}$
be a toric surface over
$\mathcal {X}^{\mathrm {log}}$
be a toric surface over
![]() $\mathcal {S}^{\mathrm {log}}$
;
$\mathcal {S}^{\mathrm {log}}$
;
![]() $z \in \mathrm {Str}^{}(\mathcal {X}^{\mathrm {log}})$
(cf. Definition A.1 (i) and (ii)). Write F for the residue field of the generic point of the irreducible component of
$z \in \mathrm {Str}^{}(\mathcal {X}^{\mathrm {log}})$
(cf. Definition A.1 (i) and (ii)). Write F for the residue field of the generic point of the irreducible component of
![]() $\mathcal {X}$
on which (the subset of
$\mathcal {X}$
on which (the subset of
![]() $\mathcal {X}$
determined by)
$\mathcal {X}$
determined by)
![]() $z \in \mathrm {Str}^{}(\mathcal {X}^{\mathrm {log}})$
lies. Then one may associate with
$z \in \mathrm {Str}^{}(\mathcal {X}^{\mathrm {log}})$
lies. Then one may associate with
![]() $z\in \mathrm {Str}^{}(\mathcal {X}^{\mathrm {log}})$
a collection of distinguished valuations on F, as well as a uniquely determined canonical valuation on F, as follows:
$z\in \mathrm {Str}^{}(\mathcal {X}^{\mathrm {log}})$
a collection of distinguished valuations on F, as well as a uniquely determined canonical valuation on F, as follows:
-
(i) If z is a
 $1$
-stratum, then we take both the unique distinguished valuation and the canonical valuation associated with z to be the discrete valuation associated with the prime of height
$1$
-stratum, then we take both the unique distinguished valuation and the canonical valuation associated with z to be the discrete valuation associated with the prime of height $$ \begin{align*} F^{\times} \longrightarrow \mathbb{Q}\subseteq\mathbb{R}\end{align*} $$
$$ \begin{align*} F^{\times} \longrightarrow \mathbb{Q}\subseteq\mathbb{R}\end{align*} $$
 $1$
determined by z, normalized so as to map each prime element
$1$
determined by z, normalized so as to map each prime element
 $\pi _R$
of
$\pi _R$
of
 $R \subseteq F$
to
$R \subseteq F$
to
 $1\in \mathbb {Q}$
.
$1\in \mathbb {Q}$
.
-
(ii) If z is a
 $2$
-stratum, then we take the collection of distinguished valuations associated with z to be the discrete valuations determined by the restrictions of the discrete valuations associated with the rational P-rays (cf. Definition A.3(iii)). We take the canonical valuation associated with z to be the discrete valuation determined by the restriction of the discrete valuation associated with the midpoint P-ray at z (cf. Definition A.3(iii) and (iv)).
$2$
-stratum, then we take the collection of distinguished valuations associated with z to be the discrete valuations determined by the restrictions of the discrete valuations associated with the rational P-rays (cf. Definition A.3(iii)). We take the canonical valuation associated with z to be the discrete valuation determined by the restriction of the discrete valuation associated with the midpoint P-ray at z (cf. Definition A.3(iii) and (iv)). $$ \begin{align*} F^{\times} \longrightarrow \mathbb{Q}\subseteq\mathbb{R}\end{align*} $$
$$ \begin{align*} F^{\times} \longrightarrow \mathbb{Q}\subseteq\mathbb{R}\end{align*} $$
Here, we note that the construction from z of either the collection of distinguished valuations or the uniquely determined canonical valuation is functorial with respect to arbitrary isomorphisms of pairs
![]() $(\mathcal {X}^{\mathrm {log}},z)$
(i.e., pairs consisting of a toric surface over
$(\mathcal {X}^{\mathrm {log}},z)$
(i.e., pairs consisting of a toric surface over
![]() $\mathcal {S}^{\mathrm {log}}$
and an element of “
$\mathcal {S}^{\mathrm {log}}$
and an element of “
![]() $\mathrm {Str}^{}(-)$
” of the toric surface).
$\mathrm {Str}^{}(-)$
” of the toric surface).
Remark A.4.1. One verifies immediately that the (noncuspidal) valuations of the discussion preceding Corollary 1.15 correspond precisely to the canonical valuations of Definition A.4.
Lemma A.5 (Valuations associated with irrational rays).
In the notation of Definition A.3, let
![]() $L \subseteq P$
be an irrational P-ray (cf. Definition A.3(i) and (ii)),
$L \subseteq P$
be an irrational P-ray (cf. Definition A.3(i) and (ii)),
![]() $\{L_i\}_{i = 1}^\infty $
a sequence of P-rays such that
$\{L_i\}_{i = 1}^\infty $
a sequence of P-rays such that
![]() $L = \lim _{i \rightarrow \infty } L_i$
(cf. Remark A.3.1), and
$L = \lim _{i \rightarrow \infty } L_i$
(cf. Remark A.3.1), and
![]() $\{w_i\}_{i=1}^\infty $
a sequence of admissible valuations such that, for each positive integer i,
$\{w_i\}_{i=1}^\infty $
a sequence of admissible valuations such that, for each positive integer i,
![]() $L_i$
is the P-ray associated with
$L_i$
is the P-ray associated with
![]() $w_i$
(cf. Definition A.3(v)). Then there exists an admissible valuation (cf. Definition A.3(v))
$w_i$
(cf. Definition A.3(v)). Then there exists an admissible valuation (cf. Definition A.3(v))
which satisfies the following conditions:
-
(a) The P-ray associated with
 $v_L$
(cf. Definition A.3(v)) is equal to L.
$v_L$
(cf. Definition A.3(v)) is equal to L. -
(b) For each
 $f \in F^{\times }$
, it holds that
$f \in F^{\times }$
, it holds that  $$ \begin{align*}v_L(f)\ =\ \lim_{i \rightarrow \infty}\ w_{i}(f) .\end{align*} $$
$$ \begin{align*}v_L(f)\ =\ \lim_{i \rightarrow \infty}\ w_{i}(f) .\end{align*} $$
-
(c) If
 $\lambda \in L$
maps a prime element
$\lambda \in L$
maps a prime element
 $\pi _R$
of R to
$\pi _R$
of R to
 $1\in \mathbb {R}$
, J is a nonempty finite set,
$1\in \mathbb {R}$
, J is a nonempty finite set,
 $\{m_j\}_{j\in J}$
is a collection of distinct elements of
$\{m_j\}_{j\in J}$
is a collection of distinct elements of
 $M^{\mathrm {gp}}$
, and
$M^{\mathrm {gp}}$
, and
 $\{f_j\}_{j\in J}$
is a collection of elements of F such that
$\{f_j\}_{j\in J}$
is a collection of elements of F such that
 $f_j$
lies in the
$f_j$
lies in the
 $A^\times $
-orbit determined by
$A^\times $
-orbit determined by
 $m_j$
, then
$m_j$
, then  $$\begin{align*}v_L(\sum_{j\in J} f_j)\ =\ \min_{j\in J}\ \lambda(m_j)\ \in\ \mathbb{R}. \end{align*}$$
$$\begin{align*}v_L(\sum_{j\in J} f_j)\ =\ \min_{j\in J}\ \lambda(m_j)\ \in\ \mathbb{R}. \end{align*}$$
Moreover, this valuation
![]() $v_L$
is the unique admissible valuation (i.e., in the sense of Definition A.3(v)) that satisfies condition (a). In particular,
$v_L$
is the unique admissible valuation (i.e., in the sense of Definition A.3(v)) that satisfies condition (a). In particular,
![]() $v_L$
depends only on the P-ray
$v_L$
depends only on the P-ray
![]() $L\subseteq P$
, that is, is independent of the choice of the sequences
$L\subseteq P$
, that is, is independent of the choice of the sequences
![]() $\{L_i\}_{i = 1}^\infty $
and
$\{L_i\}_{i = 1}^\infty $
and
![]() $\{w_i\}_{i=1}^\infty $
.
$\{w_i\}_{i=1}^\infty $
.
Proof. One may define a map
![]() $v_L\colon F^\times \rightarrow \mathbb {R}$
by applying the formula in the display of condition (c) in the case where
$v_L\colon F^\times \rightarrow \mathbb {R}$
by applying the formula in the display of condition (c) in the case where
![]() $J^\sharp = 1$
. Then one verifies easily that this map
$J^\sharp = 1$
. Then one verifies easily that this map
![]() $v_L$
is a homomorphism (with respect to the multiplicative structure of
$v_L$
is a homomorphism (with respect to the multiplicative structure of
![]() $F^\times $
) and satisfies condition (b). Next, let us observe that since (we have assumed that) L is irrational, the map
$F^\times $
) and satisfies condition (b). Next, let us observe that since (we have assumed that) L is irrational, the map
![]() $M^{\mathrm {gp}} \rightarrow \mathbb {R}$
determined by
$M^{\mathrm {gp}} \rightarrow \mathbb {R}$
determined by
![]() $\lambda \in L$
is injective. Thus, it follows from condition (b), together with the fact that each of the
$\lambda \in L$
is injective. Thus, it follows from condition (b), together with the fact that each of the
![]() $w_i$
’s is a valuation, that the map
$w_i$
’s is a valuation, that the map
![]() $v_L$
satisfies condition (c), which implies that the map
$v_L$
satisfies condition (c), which implies that the map
![]() $v_L$
is a (necessarily admissible) valuation on F. Moreover, it follows immediately from the definition of
$v_L$
is a (necessarily admissible) valuation on F. Moreover, it follows immediately from the definition of
![]() $v_L$
that
$v_L$
that
![]() $v_L$
satisfies condition (a). This completes the proof of Lemma A.5.
$v_L$
satisfies condition (a). This completes the proof of Lemma A.5.
Lemma A.6 (Convergence of midpoints of closed intervals).
Let
—where i ranges over the nonnegative integers—be a sequence of inclusions of nonempty closed intervals in
![]() $[0,1]$
. For each i, write
$[0,1]$
. For each i, write
![]() $c_i$
for the midpoint of the closed interval
$c_i$
for the midpoint of the closed interval
![]() $[a_i,b_i]$
, that is,
$[a_i,b_i]$
, that is,
![]() $c_i \stackrel {\mathrm {def}}{=} (a_i + b_i) / 2 \in [a_i,b_i]$
. Then the sequence of midpoints
$c_i \stackrel {\mathrm {def}}{=} (a_i + b_i) / 2 \in [a_i,b_i]$
. Then the sequence of midpoints
![]() $\{c_i\}_{i=1}^\infty $
converges.
$\{c_i\}_{i=1}^\infty $
converges.
Proof. This follows immediately from the (easily verified) fact that the sequences
![]() $\{a_i\}_{i = 1}^\infty $
,
$\{a_i\}_{i = 1}^\infty $
,
![]() $\{b_i\}_{i = 1}^\infty $
converge.
$\{b_i\}_{i = 1}^\infty $
converge.
Theorem A.7 (Explicit limit seminorms associated with sequences of toric surfaces).
Let R be a complete discrete valuation ring and I a totally ordered set that is isomorphic to
![]() $\mathbb {N}$
(equipped with its usual ordering). Write K for the field of fractions of R and
$\mathbb {N}$
(equipped with its usual ordering). Write K for the field of fractions of R and
![]() $\mathcal {S}^{\mathrm {log}}$
for the log scheme obtained by equipping
$\mathcal {S}^{\mathrm {log}}$
for the log scheme obtained by equipping
![]() $\mathcal {S} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(R)$
with the log structure determined by the unique closed point of
$\mathcal {S} \stackrel {\mathrm {def}}{=} \mathrm {Spec}(R)$
with the log structure determined by the unique closed point of
![]() $\mathcal {S}$
. Let
$\mathcal {S}$
. Let
be a sequence of toric surface s over
![]() $\mathcal {S}^{\mathrm {log}}$
indexed by I (cf. Definition A.2) and
$\mathcal {S}^{\mathrm {log}}$
indexed by I (cf. Definition A.2) and
(cf. Definitions A.1 (ii) and A.2). Then, after possibly replacing I by a suitable cofinal subset of I, there exist sequences
—where, for each
![]() $i\in I$
,
$i\in I$
,
![]() $F_i$
denotes the residue field of some point
$F_i$
denotes the residue field of some point
![]() $x_i \in \mathcal {X}_i\times _R K$
;
$x_i \in \mathcal {X}_i\times _R K$
;
![]() $v_i\colon F_i^{\times } \rightarrow \mathbb {R}$
is a valuation;
$v_i\colon F_i^{\times } \rightarrow \mathbb {R}$
is a valuation;
![]() $v_{z_i}$
is a distinguished valuation associated with
$v_{z_i}$
is a distinguished valuation associated with
![]() $z_i$
(cf. Definition A.4)—such that
$z_i$
(cf. Definition A.4)—such that
-
(a)
 $v_i$
maps each prime element of
$v_i$
maps each prime element of
 $R \subseteq F_i$
to
$R \subseteq F_i$
to
 $1 \in \mathbb {R}$
(which thus implies that
$1 \in \mathbb {R}$
(which thus implies that
 $v_i$
dominates R);
$v_i$
dominates R); -
(b) the
 $x_i$
’s and
$x_i$
’s and
 $v_i$
’s are compatible (in the evident sense) with respect to the upper horizontal arrows
$v_i$
’s are compatible (in the evident sense) with respect to the upper horizontal arrows
 $\mathcal {X}_{i+1}^{\mathrm {log}} \rightarrow \mathcal {X}_i^{\mathrm {log}}$
of the above diagram;
$\mathcal {X}_{i+1}^{\mathrm {log}} \rightarrow \mathcal {X}_i^{\mathrm {log}}$
of the above diagram; -
(c) for every nonzero rational function f on the irreducible component of
 $\mathcal {X}_i$
containing
$\mathcal {X}_i$
containing
 $x_i$
that is regular at
$x_i$
that is regular at
 $x_i$
, hence determines an element
$x_i$
, hence determines an element
 $\overline {f} \in F_i$
(cf. Remark A.7.1 below), it holds that
$\overline {f} \in F_i$
(cf. Remark A.7.1 below), it holds that  $$\begin{align*}v_i(\overline{f})\ =\ \lim_{j\rightarrow \infty} v_{z_j}(f) \end{align*}$$
$$\begin{align*}v_i(\overline{f})\ =\ \lim_{j\rightarrow \infty} v_{z_j}(f) \end{align*}$$
(cf. Definition A.4)—where j ranges over the elements of I that are
 $\geq i$
, and we regard
$\geq i$
, and we regard
 $v_i$
as a map defined on
$v_i$
as a map defined on
 $F_i$
by sending
$F_i$
by sending
 $F_i \ni 0 \mapsto +\infty $
.
$F_i \ni 0 \mapsto +\infty $
.
Finally, these sequences of valuations
![]() $\{v_i\}_{i\in I}$
,
$\{v_i\}_{i\in I}$
,
![]() $\{v_{z_i}\}_{i\in I}$
may be constructed in a way that is functorial (in the evident sense) with respect to isomorphisms of pairs consisting of a sequence of toric surfaces over
$\{v_{z_i}\}_{i\in I}$
may be constructed in a way that is functorial (in the evident sense) with respect to isomorphisms of pairs consisting of a sequence of toric surfaces over
![]() $\mathcal {S}^{\mathrm {log}}$
and a compatible collection of strata (i.e., “
$\mathcal {S}^{\mathrm {log}}$
and a compatible collection of strata (i.e., “
![]() $\{z_i\}_{i\in I}$
”).
$\{z_i\}_{i\in I}$
”).
Proof. Until further notice, we take, for each
![]() $i\in I$
,
$i\in I$
,
![]() $v_{z_i}$
to be the canonical valuation associated with
$v_{z_i}$
to be the canonical valuation associated with
![]() $z_i$
(cf. Definition A.4). Next, let us observe that one verifies easily that we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that there exists an element
$z_i$
(cf. Definition A.4). Next, let us observe that one verifies easily that we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that there exists an element
![]() $n\in \{1,2\}$
such that every member of
$n\in \{1,2\}$
such that every member of
![]() $\{z_i\}$
is an n-stratum, that is, one of the following conditions is satisfied:
$\{z_i\}$
is an n-stratum, that is, one of the following conditions is satisfied:
-
(1) Every member of
 $\{z_i\}$
is a
$\{z_i\}$
is a
 $1$
-stratum.
$1$
-stratum. -
(2) Every member of
 $\{z_i\}$
is a
$\{z_i\}$
is a
 $2$
-stratum.
$2$
-stratum.
First, we consider Theorem A.7 in the case where condition (1) is satisfied. For each
![]() $i \in I$
, write
$i \in I$
, write
![]() $\mathcal {Z}_i \subseteq \mathcal {X}_i$
for the reduced closed subscheme of
$\mathcal {Z}_i \subseteq \mathcal {X}_i$
for the reduced closed subscheme of
![]() $\mathcal {X}_i$
whose underlying closed subset [
$\mathcal {X}_i$
whose underlying closed subset [
![]() $\subseteq \mathcal {X}_i$
] is the closure of the subset of
$\subseteq \mathcal {X}_i$
] is the closure of the subset of
![]() $\mathcal {X}$
determined by the
$\mathcal {X}$
determined by the
![]() $1$
-stratum
$1$
-stratum
![]() $z_i$
. Then let us observe that if, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
$z_i$
. Then let us observe that if, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
![]() $i \in I$
, the composite
$i \in I$
, the composite
![]() $\mathcal {Z}_{i+1} \hookrightarrow \mathcal {X}_{i+1} \rightarrow \mathcal {X}_i$
is quasi-finite, then the system consisting of the
$\mathcal {Z}_{i+1} \hookrightarrow \mathcal {X}_{i+1} \rightarrow \mathcal {X}_i$
is quasi-finite, then the system consisting of the
![]() $v_{z_i}$
’s (cf. Definition A.4(i)) already yields a system of valuations
$v_{z_i}$
’s (cf. Definition A.4(i)) already yields a system of valuations
![]() $\{v_i\}_{i\in I}$
as desired. Thus, we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
$\{v_i\}_{i\in I}$
as desired. Thus, we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
![]() $i \in I$
, the composite
$i \in I$
, the composite
![]() $\mathcal {Z}_{i+1} \hookrightarrow \mathcal {X}_{i+1} \rightarrow \mathcal {X}_i$
is not quasi-finite, that is, that the image of this composite is a closed point
$\mathcal {Z}_{i+1} \hookrightarrow \mathcal {X}_{i+1} \rightarrow \mathcal {X}_i$
is not quasi-finite, that is, that the image of this composite is a closed point
![]() $y_i \in \mathcal {X}_i$
of
$y_i \in \mathcal {X}_i$
of
![]() $\mathcal {X}_i$
. Here, we observe that since we are operating under the assumption that condition (1) is satisfied, it follows from the fact that
$\mathcal {X}_i$
. Here, we observe that since we are operating under the assumption that condition (1) is satisfied, it follows from the fact that
![]() $z_{i+1} \mapsto z_i$
that
$z_{i+1} \mapsto z_i$
that
![]() $y_i$
necessarily lies in the regular locus of
$y_i$
necessarily lies in the regular locus of
![]() $\mathcal {X}_i$
.
$\mathcal {X}_i$
.
For each
![]() $i \in I$
, write
$i \in I$
, write
![]() $B_i$
for the local ring of
$B_i$
for the local ring of
![]() $\mathcal {X}_i$
at
$\mathcal {X}_i$
at
![]() $y_i \in \mathcal {X}_i$
,
$y_i \in \mathcal {X}_i$
,
![]() $E_i$
for the field of fractions of
$E_i$
for the field of fractions of
![]() $B_i$
, and
$B_i$
, and
![]() $v_{z_i} \colon E_i^{\times } \rightarrow \mathbb {R}$
for the discrete valuation defined in Definition A.4(i). Thus, one verifies immediately that the morphisms
$v_{z_i} \colon E_i^{\times } \rightarrow \mathbb {R}$
for the discrete valuation defined in Definition A.4(i). Thus, one verifies immediately that the morphisms
induce compatible chains of injections
Moreover, if
![]() $\pi _R$
is a prime element of R, then the discrete valuation
$\pi _R$
is a prime element of R, then the discrete valuation
![]() $v_{z_i}$
may be interpreted as the discrete valuation of
$v_{z_i}$
may be interpreted as the discrete valuation of
![]() $B_i$
determined by the unique height one prime of
$B_i$
determined by the unique height one prime of
![]() $B_i$
that contains
$B_i$
that contains
![]() $\pi _R$
. In particular, since
$\pi _R$
. In particular, since
![]() $B_i$
is regular, hence a unique factorization domain, one verifies immediately—by considering the extent to which positive powers of an element
$B_i$
is regular, hence a unique factorization domain, one verifies immediately—by considering the extent to which positive powers of an element
![]() $f\in B_i$
are divisible, in
$f\in B_i$
are divisible, in
![]() $B_i$
or in
$B_i$
or in
![]() $B_{i+1}$
, by positive powers of
$B_{i+1}$
, by positive powers of
![]() $\pi _R$
—that, for each
$\pi _R$
—that, for each
![]() $i \in I$
and
$i \in I$
and
![]() $f \in B_i$
, it holds that
$f \in B_i$
, it holds that
For each
![]() $i \in I$
, write
$i \in I$
, write
Then since each
![]() $v_{z_j}$
is a (discrete) valuation, one verifies immediately that
$v_{z_j}$
is a (discrete) valuation, one verifies immediately that
![]() $\mathfrak {p}_i \subseteq B_i$
is a prime ideal of
$\mathfrak {p}_i \subseteq B_i$
is a prime ideal of
![]() $B_i$
. Moreover, since
$B_i$
. Moreover, since
![]() $\pi _R\not \in \mathfrak {p}_i$
, we conclude that the ideal
$\pi _R\not \in \mathfrak {p}_i$
, we conclude that the ideal
![]() $\mathfrak {p}_i$
is not maximal, that is, that the height of
$\mathfrak {p}_i$
is not maximal, that is, that the height of
![]() $\mathfrak {p}_i$
is
$\mathfrak {p}_i$
is
![]() $\in \{0,1\}$
. Next, let us observe that if, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
$\in \{0,1\}$
. Next, let us observe that if, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
![]() $i\in I$
, the prime ideal
$i\in I$
, the prime ideal
![]() $\mathfrak {p}_i$
is of height
$\mathfrak {p}_i$
is of height
![]() $1$
, then it follows immediately that
$1$
, then it follows immediately that
![]() $\mathfrak {p}_i$
determines a closed point
$\mathfrak {p}_i$
determines a closed point
![]() $x_i$
of the generic fiber of
$x_i$
of the generic fiber of
![]() $\mathcal {X}_i$
, and that, if we write
$\mathcal {X}_i$
, and that, if we write
![]() $F_i$
for the residue field of
$F_i$
for the residue field of
![]() $\mathcal {X}_i$
at
$\mathcal {X}_i$
at
![]() $x_i$
and
$x_i$
and
![]() $v_i\colon F^\times _i \rightarrow \mathbb {R}$
for the uniquely determined (since
$v_i\colon F^\times _i \rightarrow \mathbb {R}$
for the uniquely determined (since
![]() $F_i$
is a finite extension of K) discrete valuation on
$F_i$
is a finite extension of K) discrete valuation on
![]() $F_i$
that extends the given discrete valuation on K and maps
$F_i$
that extends the given discrete valuation on K and maps
![]() $\pi _R\mapsto 1\in \mathbb {R}$
, then the limit
$\pi _R\mapsto 1\in \mathbb {R}$
, then the limit
![]() $\lim _{j \rightarrow \infty } v_{z_j}(-)$
(cf.
$\lim _{j \rightarrow \infty } v_{z_j}(-)$
(cf.
![]() $(*)$
) determines a valuation on
$(*)$
) determines a valuation on
![]() $F_i = (B_i)_{\mathfrak {p}_i} / \mathfrak {p}_i(B_i)_{\mathfrak {p}_i}$
that necessarily coincides (since
$F_i = (B_i)_{\mathfrak {p}_i} / \mathfrak {p}_i(B_i)_{\mathfrak {p}_i}$
that necessarily coincides (since
![]() $F_i$
is a finite extension of K) with
$F_i$
is a finite extension of K) with
![]() $v_i$
; in particular, one obtains a system of valuations
$v_i$
; in particular, one obtains a system of valuations
![]() $\{v_i\}_{i\in I}$
as desired.
$\{v_i\}_{i\in I}$
as desired.
Thus, we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
![]() $i\in I$
, the prime ideal
$i\in I$
, the prime ideal
![]() $\mathfrak {p}_i$
is of height
$\mathfrak {p}_i$
is of height
![]() $0$
, that is,
$0$
, that is,
![]() $\mathfrak {p}_i = \{0\}$
, hence determines a generic point
$\mathfrak {p}_i = \{0\}$
, hence determines a generic point
![]() $x_i$
of some irreducible component of
$x_i$
of some irreducible component of
![]() $\mathcal {X}_i$
such that
$\mathcal {X}_i$
such that
![]() $E_i$
may be naturally identified with the residue field
$E_i$
may be naturally identified with the residue field
![]() $F_i$
of
$F_i$
of
![]() $\mathcal {X}_i$
at
$\mathcal {X}_i$
at
![]() $x_i$
. But this implies that, for
$x_i$
. But this implies that, for
![]() $f \in E_i^{\times }= F_i^{\times }$
, the quantity
$f \in E_i^{\times }= F_i^{\times }$
, the quantity
is well-defined (cf.
![]() $(*)$
). Moreover, one verifies immediately that this definition of
$(*)$
). Moreover, one verifies immediately that this definition of
![]() $v_{i}$
determines a valuation on
$v_{i}$
determines a valuation on
![]() $E_i=F_i$
. In particular, one obtains a system of valuations
$E_i=F_i$
. In particular, one obtains a system of valuations
![]() $\{v_{i}\}_{i\in I}$
as desired. This completes the proof of Theorem A.7 in the case where condition (1) is satisfied.
$\{v_{i}\}_{i\in I}$
as desired. This completes the proof of Theorem A.7 in the case where condition (1) is satisfied.
Next, we consider Theorem A.7 in the case where condition (2) is satisfied. For each
![]() $i \in I$
, write
$i \in I$
, write
![]() $Q_i$
,
$Q_i$
,
![]() $P_i$
,
$P_i$
,
![]() $V_i$
for the objects “Q,” “P,” “V” defined in Definition A.3 in the case where we take the data “
$V_i$
for the objects “Q,” “P,” “V” defined in Definition A.3 in the case where we take the data “
![]() $(\mathcal {X}^{\mathrm {log}}, z \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}}))$
” in Definition A.3 to be
$(\mathcal {X}^{\mathrm {log}}, z \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}}))$
” in Definition A.3 to be
![]() $(\mathcal {X}_i^{\mathrm {log}}, z_i \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}}_i))$
. Then one verifies easily that the morphism
$(\mathcal {X}_i^{\mathrm {log}}, z_i \in \mathrm {Str}^{2}(\mathcal {X}^{\mathrm {log}}_i))$
. Then one verifies easily that the morphism
![]() $\mathcal {X}_{i+1}^{\mathrm {log}} \rightarrow \mathcal {X}_i^{\mathrm {log}}$
determines a nontrivial
$\mathcal {X}_{i+1}^{\mathrm {log}} \rightarrow \mathcal {X}_i^{\mathrm {log}}$
determines a nontrivial
![]() $\mathbb {R}$
-linear map
$\mathbb {R}$
-linear map
![]() $V_{i+1}\rightarrow V_i$
that maps
$V_{i+1}\rightarrow V_i$
that maps
![]() $Q_{i+1}$
,
$Q_{i+1}$
,
![]() $P_{i+1} \subseteq V_{i+1}$
into
$P_{i+1} \subseteq V_{i+1}$
into
![]() $Q_{i}$
,
$Q_{i}$
,
![]() $P_{i} \subseteq V_{i}$
, respectively.
$P_{i} \subseteq V_{i}$
, respectively.
Next, let us observe that if, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
![]() $i\in I$
, the
$i\in I$
, the
![]() $\mathbb {R}$
-linear map
$\mathbb {R}$
-linear map
![]() $V_{i+1} \rightarrow V_i$
is of rank one, that is, the image of
$V_{i+1} \rightarrow V_i$
is of rank one, that is, the image of
![]() $P_{i+1} \subseteq V_{i+1}$
in
$P_{i+1} \subseteq V_{i+1}$
in
![]() $V_i$
is a rational
$V_i$
is a rational
![]() $P_i$
-ray
$P_i$
-ray
![]() $L_i$
(cf. Definition A.3(i) and (ii)), then we may assume without loss of generality, by taking
$L_i$
(cf. Definition A.3(i) and (ii)), then we may assume without loss of generality, by taking
![]() $v_{z_i}$
to be the distinguished valuation associated with the rational
$v_{z_i}$
to be the distinguished valuation associated with the rational
![]() $P_i$
-ray
$P_i$
-ray
![]() $L_{i}$
(cf. Definition A.4(ii); Remark A.7.2 below) and then replacing the pair
$L_{i}$
(cf. Definition A.4(ii); Remark A.7.2 below) and then replacing the pair
![]() $(\mathcal {X}_i, z_i)$
by the pair consisting of the blow-up of
$(\mathcal {X}_i, z_i)$
by the pair consisting of the blow-up of
![]() $\mathcal {X}_i$
and the
$\mathcal {X}_i$
and the
![]() $1$
-stratum of this blow-up determined by
$1$
-stratum of this blow-up determined by
![]() $L_{i}$
(cf. the discussion of Definition A.3(iii)), that condition (1) is satisfied. Thus, we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
$L_{i}$
(cf. the discussion of Definition A.3(iii)), that condition (1) is satisfied. Thus, we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
![]() $i \in I$
, the
$i \in I$
, the
![]() $\mathbb {R}$
-linear map
$\mathbb {R}$
-linear map
![]() $V_{i+1} \rightarrow V_i$
is of rank
$V_{i+1} \rightarrow V_i$
is of rank
![]() $\neq 1$
, hence (cf. the existence of the
$\neq 1$
, hence (cf. the existence of the
![]() $\mathbb {R}$
-linear surjection “
$\mathbb {R}$
-linear surjection “
![]() $V \twoheadrightarrow V_R$
” of Definition A.3(iv)) of rank two, that is, an isomorphism.
$V \twoheadrightarrow V_R$
” of Definition A.3(iv)) of rank two, that is, an isomorphism.
Since the
![]() $\mathbb {R}$
-linear map
$\mathbb {R}$
-linear map
![]() $V_{i+1} \rightarrow V_i$
is an isomorphism, it follows immediately from Lemma A.6, together with Remark A.3.1, that, for each
$V_{i+1} \rightarrow V_i$
is an isomorphism, it follows immediately from Lemma A.6, together with Remark A.3.1, that, for each
![]() $i \in I$
, the sequence consisting of the images in
$i \in I$
, the sequence consisting of the images in
![]() $P_i$
of the midpoint
$P_i$
of the midpoint
![]() $P_j$
-rays (cf. Definition A.3(iv)), where j ranges over the elements of I such that
$P_j$
-rays (cf. Definition A.3(iv)), where j ranges over the elements of I such that
![]() $j\geq i$
, converges to a (not necessarily rational)
$j\geq i$
, converges to a (not necessarily rational)
![]() $P_i$
-ray
$P_i$
-ray
![]() $L_{i,\infty } \subseteq P_i$
. If, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
$L_{i,\infty } \subseteq P_i$
. If, after possibly replacing I by a suitable cofinal subset of I, it holds that, for each
![]() $i\in I$
, the
$i\in I$
, the
![]() $P_i$
-ray
$P_i$
-ray
![]() $L_{i,\infty }$
is rational, then we may assume without loss of generality, by taking
$L_{i,\infty }$
is rational, then we may assume without loss of generality, by taking
![]() $v_{z_i}$
to be the distinguished valuation associated with the rational
$v_{z_i}$
to be the distinguished valuation associated with the rational
![]() $P_i$
-ray
$P_i$
-ray
![]() $L_{i,\infty }$
(cf. Definition A.4(ii); Remark A.7.2 below) and then replacing the pair
$L_{i,\infty }$
(cf. Definition A.4(ii); Remark A.7.2 below) and then replacing the pair
![]() $(\mathcal {X}_i,z_i)$
by the pair consisting of the blow-up of
$(\mathcal {X}_i,z_i)$
by the pair consisting of the blow-up of
![]() $\mathcal {X}_i$
and the
$\mathcal {X}_i$
and the
![]() $1$
-stratum of this blow-up determined by
$1$
-stratum of this blow-up determined by
![]() $L_{i,\infty }$
(cf. the discussion of Definition A.3(iii)), that condition (1) is satisfied. Thus, it remains to consider the case in which we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
$L_{i,\infty }$
(cf. the discussion of Definition A.3(iii)), that condition (1) is satisfied. Thus, it remains to consider the case in which we may assume without loss of generality, by replacing I by a suitable cofinal subset of I, that, for each
![]() $i\in I$
, the
$i\in I$
, the
![]() $P_i$
-ray
$P_i$
-ray
![]() $L_{i,\infty }$
is irrational. Then the system consisting of the valuations
$L_{i,\infty }$
is irrational. Then the system consisting of the valuations
![]() $v_{L_{i,\infty }}$
’s of Lemma A.5 yields a system of valuations
$v_{L_{i,\infty }}$
’s of Lemma A.5 yields a system of valuations
![]() $\{v_i\}_{i\in I}$
as desired. This completes the proof of Theorem A.7.
$\{v_i\}_{i\in I}$
as desired. This completes the proof of Theorem A.7.
Remark A.7.1. In the situation of Theorem A.7, for
![]() $I\ni j \geq i$
, write
$I\ni j \geq i$
, write
![]() $z^i_j$
for the irreducible locally closed subset of
$z^i_j$
for the irreducible locally closed subset of
![]() $\mathcal {X}_i$
determined by the image of the stratum
$\mathcal {X}_i$
determined by the image of the stratum
![]() $z_j$
in
$z_j$
in
![]() $\mathcal {X}_i$
. Thus,
$\mathcal {X}_i$
. Thus,
![]() $z^i_{j'} \subseteq z^i_j$
for all
$z^i_{j'} \subseteq z^i_j$
for all
![]() $j' \geq j$
, and one verifies immediately that the intersection
$j' \geq j$
, and one verifies immediately that the intersection
 $$\begin{align*}z^i_\infty\ \stackrel{\mathrm{def}}{=}\ \bigcap_{j\geq i}\ z^i_j \end{align*}$$
$$\begin{align*}z^i_\infty\ \stackrel{\mathrm{def}}{=}\ \bigcap_{j\geq i}\ z^i_j \end{align*}$$
is nonempty. Moreover, it follows immediately from the constructions discussed in the proof of Theorem A.7 that if
![]() $\xi _i \in z^i_\infty $
, then any element f of the local ring
$\xi _i \in z^i_\infty $
, then any element f of the local ring
![]() $\mathcal {O}_{\mathcal {X}_i, \xi _i}$
of
$\mathcal {O}_{\mathcal {X}_i, \xi _i}$
of
![]() $\mathcal {X}_i$
at
$\mathcal {X}_i$
at
![]() $\xi _i$
determines a rational function on the irreducible component of
$\xi _i$
determines a rational function on the irreducible component of
![]() $\mathcal {X}_i$
containing
$\mathcal {X}_i$
containing
![]() $x_i$
that is regular at
$x_i$
that is regular at
![]() $x_i$
(cf. Theorem A.7(c)).
$x_i$
(cf. Theorem A.7(c)).
Remark A.7.2. Although, in certain cases (cf. the final portion of the proof of Theorem A.7), the distinguished valuation
![]() $v_{z_i}$
in the statement of Theorem A.7 is not necessarily canonical, the system of valuations
$v_{z_i}$
in the statement of Theorem A.7 is not necessarily canonical, the system of valuations
![]() $\{v_i\}_{i\in I}$
obtained in Theorem A.7 is nevertheless sufficient (cf. the functoriality discussed in the final portion of Theorem A.7) to derive the conclusion of Corollary 1.15(ii), that is, without applying the theory of [Reference Berkovich2].
$\{v_i\}_{i\in I}$
obtained in Theorem A.7 is nevertheless sufficient (cf. the functoriality discussed in the final portion of Theorem A.7) to derive the conclusion of Corollary 1.15(ii), that is, without applying the theory of [Reference Berkovich2].
Acknowledgments
The authors would like to thank Yu Iijima and Yu Yang for pointing out minor errors in an earlier version of the present paper. The first author was supported by Grant-in-Aid for Scientific Research (C), No. 24540016, Japan Society for the Promotion of Science.