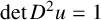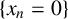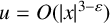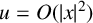1 Introduction
The existence and asymptotic behaviour at infinity of the convex viscosity solution of the Monge–Ampère equation:
 $$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{in the half space } \mathbb{R}^n_+,\\ u(x',x_n)=\frac{1}{2}|x'|^2 & \mbox{on the boundary }\{x_n=0\}, \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{in the half space } \mathbb{R}^n_+,\\ u(x',x_n)=\frac{1}{2}|x'|^2 & \mbox{on the boundary }\{x_n=0\}, \end{cases} \end{align} $$
is investigated in [Reference Jia, Li and Li5], when the space dimension
![]() $n\geq 2$
and
$n\geq 2$
and
![]() $f\in C^0(\overline {\mathbb {R}^n_+})$
satisfies
$f\in C^0(\overline {\mathbb {R}^n_+})$
satisfies
and
for some
![]() $\lambda $
with
$\lambda $
with
![]() $0<\lambda <1$
. The main result in [Reference Jia, Li and Li5] states that any viscosity solution of (1.1) must tend to a quadratic polynomial at infinity if u satisfies the quadratic growth condition at infinity and f satisfies (1.2) and (1.3). We say a function u satisfies the quadratic growth condition at infinity, if
$0<\lambda <1$
. The main result in [Reference Jia, Li and Li5] states that any viscosity solution of (1.1) must tend to a quadratic polynomial at infinity if u satisfies the quadratic growth condition at infinity and f satisfies (1.2) and (1.3). We say a function u satisfies the quadratic growth condition at infinity, if
at infinity for some constant
![]() $\tau \in (0,\tfrac 12]$
.
$\tau \in (0,\tfrac 12]$
.
For the whole space case, the Liouville type theorem and the asymptotic behaviour of solutions for Monge–Ampère equations over exterior domains have been widely studied (see, for example, [Reference Caffarelli and Li1, Reference Zhou, Gong and Bao11] and the references therein). For the half space case, the Liouville type theorem has been obtained by Mooney [Reference Mooney7]. He showed that if u is a convex viscosity solution of (1.1) and satisfies the quadratic growth condition at infinity, then u is a quadratic polynomial. The reason for assuming the quadratic growth condition is that the convex function
 $$ \begin{align*} u(x_1,\ldots,x_n)=\frac{x_1^2}{2(x_n+1)}+\frac{1}{2}(x_2^2+\cdots+x_{n-1}^2)+\frac{1}{6}(x_n^3+3x_n^2) \end{align*} $$
$$ \begin{align*} u(x_1,\ldots,x_n)=\frac{x_1^2}{2(x_n+1)}+\frac{1}{2}(x_2^2+\cdots+x_{n-1}^2)+\frac{1}{6}(x_n^3+3x_n^2) \end{align*} $$
solves the Monge–Ampère equation (1.1) with
![]() $f\equiv 1$
, but it is obviously not a quadratic polynomial. Recently, for the Liouville type theorem, the quadratic growth condition at infinity has been weakened. Savin [Reference Savin9] showed that if there exists
$f\equiv 1$
, but it is obviously not a quadratic polynomial. Recently, for the Liouville type theorem, the quadratic growth condition at infinity has been weakened. Savin [Reference Savin9] showed that if there exists
![]() $\epsilon>0$
such that
$\epsilon>0$
such that
![]() $u=O(|x|^{3-\varepsilon })$
at infinity, then u is a quadratic polynomial. In [Reference Savin9], the important tools are the rescaling method and a key lemma (Lemma 8.3), which holds if the right-hand term f in (1.1) is very close to some constant near the origin. Obviously, the example mentioned above does not satisfy
$u=O(|x|^{3-\varepsilon })$
at infinity, then u is a quadratic polynomial. In [Reference Savin9], the important tools are the rescaling method and a key lemma (Lemma 8.3), which holds if the right-hand term f in (1.1) is very close to some constant near the origin. Obviously, the example mentioned above does not satisfy
![]() $u=O(|x|^{3-\varepsilon })$
at infinity.
$u=O(|x|^{3-\varepsilon })$
at infinity.
This raises an interesting question for the general asymptotic result for the solutions of Monge–Ampère equations. Can the quadratic growth condition in [Reference Jia, Li and Li5] be weakened to
![]() $u=O(|x|^{3-\varepsilon })$
at infinity? The main difficulty comes from the fact that f may not be close to some constant near the origin and thus [Reference Savin9, Lemma 8.3] does not work directly.
$u=O(|x|^{3-\varepsilon })$
at infinity? The main difficulty comes from the fact that f may not be close to some constant near the origin and thus [Reference Savin9, Lemma 8.3] does not work directly.
We consider the convex viscosity solution of the Monge–Ampère equation:
 $$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{in } \mathbb{R}^n_+,\\ u(x',x_n)=\varphi(x') & \mbox{on }\{x_n=0\}, \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{in } \mathbb{R}^n_+,\\ u(x',x_n)=\varphi(x') & \mbox{on }\{x_n=0\}, \end{cases} \end{align} $$
where
![]() $n\geq 2$
,
$n\geq 2$
,
![]() $f\in C^0(\overline {\mathbb {R}^n_+})$
satisfies (1.3) and
$f\in C^0(\overline {\mathbb {R}^n_+})$
satisfies (1.3) and
for some
![]() $s>0$
, and
$s>0$
, and
![]() $\varphi \in C^2(\mathbb {R}^{n-1})$
satisfies
$\varphi \in C^2(\mathbb {R}^{n-1})$
satisfies
 $$ \begin{align} \mu I_{n-1}\leq D^2\varphi\leq \mu^{-1}I_{n-1}\quad \mbox{on }\{x_n=0\},\quad I_{n-1}=\mbox{diag}(\underbrace{1,1,\cdots,1}_{n-1}), \end{align} $$
$$ \begin{align} \mu I_{n-1}\leq D^2\varphi\leq \mu^{-1}I_{n-1}\quad \mbox{on }\{x_n=0\},\quad I_{n-1}=\mbox{diag}(\underbrace{1,1,\cdots,1}_{n-1}), \end{align} $$
for some
![]() $\mu \in (0,1]$
. We also assume that for some
$\mu \in (0,1]$
. We also assume that for some
![]() $\varepsilon>0$
,
$\varepsilon>0$
,
Our main result gives a positive answer to the question posed above. Throughout the paper, we use the following standard notation:
-
• for any
 $x\in \mathbb {R}^n$
,
$x\in \mathbb {R}^n$
,
 $x=(x_1,x_2,\ldots ,x_n)=(x',x_n)$
, with
$x=(x_1,x_2,\ldots ,x_n)=(x',x_n)$
, with
 $x'\in \mathbb {R}^{n-1}$
;
$x'\in \mathbb {R}^{n-1}$
; -
•
 $\mathbb {R}^n_+=\{x\in \mathbb {R}^n:x_n>0\}$
;
$\mathbb {R}^n_+=\{x\in \mathbb {R}^n:x_n>0\}$
;
 $\overline {\mathbb {R}^n_+}=\{x\in \mathbb {R}^n:x_n\geq 0\}$
;
$\overline {\mathbb {R}^n_+}=\{x\in \mathbb {R}^n:x_n\geq 0\}$
; -
• for any
 $x\in \mathbb {R}^n$
and any
$x\in \mathbb {R}^n$
and any
 $r>0$
, we write
$r>0$
, we write
 $B_r(x)=\{y\in \mathbb {R}^n:|y-x|<r\}$
and
$B_r(x)=\{y\in \mathbb {R}^n:|y-x|<r\}$
and
 $B_r^+(0)=B_r(0)\cap \{x_n>0\}$
; for simplicity, we set
$B_r^+(0)=B_r(0)\cap \{x_n>0\}$
; for simplicity, we set
 $B_r=B_r(0)$
and
$B_r=B_r(0)$
and
 $B_r^+=B_r^+(0)$
.
$B_r^+=B_r^+(0)$
.
Theorem 1.1. Let
![]() $n\geq 2$
,
$n\geq 2$
,
![]() $u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.4), where
$u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.4), where
![]() $\varphi $
satisfies (1.6) and f satisfies (1.3) and (1.5). If
$\varphi $
satisfies (1.6) and f satisfies (1.3) and (1.5). If
![]() $|u|\leq |x|^{3-\varepsilon }$
in
$|u|\leq |x|^{3-\varepsilon }$
in
![]() $\mathbb {R}^n_+\backslash B_1^+$
for some
$\mathbb {R}^n_+\backslash B_1^+$
for some
![]() $\varepsilon>0$
, then
$\varepsilon>0$
, then
where
![]() $\tau \in (0,\tfrac 12]$
is a constant depending only on
$\tau \in (0,\tfrac 12]$
is a constant depending only on
![]() $\mu $
,
$\mu $
,
![]() $\varepsilon $
,
$\varepsilon $
,
![]() $\lambda $
and n.
$\lambda $
and n.
Theorem 1.1 yields the following theorem. To obtain it, we will apply the extension theorem [Reference Yan10, Theorem 3.2], which holds for all
![]() $C^2$
uniformly convex functions over nonconvex domains.
$C^2$
uniformly convex functions over nonconvex domains.
Theorem 1.2. Let
![]() $n\geq 2$
,
$n\geq 2$
,
![]() $u\in C^2(\overline {\mathbb {R}^n_+\backslash {B_{R}^+}})$
be a convex viscosity solution of
$u\in C^2(\overline {\mathbb {R}^n_+\backslash {B_{R}^+}})$
be a convex viscosity solution of
 $$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{in } \mathbb{R}^n_+\backslash \overline{B_{R}^+},\\ u(x',x_n)=\varphi(x') & \mbox{on } \{x_n=0:|x'|\geq R\}, \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{in } \mathbb{R}^n_+\backslash \overline{B_{R}^+},\\ u(x',x_n)=\varphi(x') & \mbox{on } \{x_n=0:|x'|\geq R\}, \end{cases} \end{align} $$
where
![]() $R>0$
is a constant,
$R>0$
is a constant,
![]() $\varphi \in C^{2}$
satisfies (1.6) on
$\varphi \in C^{2}$
satisfies (1.6) on
![]() $\{x_n=0 : |x'|\geq R\}$
, and
$\{x_n=0 : |x'|\geq R\}$
, and
![]() $f\in C^0(\overline {\mathbb {R}^n_+\backslash {B_{R}^+}})$
satisfies (1.3) and (1.5). If (1.7) holds, then u satisfies the quadratic growth condition at infinity.
$f\in C^0(\overline {\mathbb {R}^n_+\backslash {B_{R}^+}})$
satisfies (1.3) and (1.5). If (1.7) holds, then u satisfies the quadratic growth condition at infinity.
Remark 1.3. In Theorem 1.2, the special domain
![]() $B_{R}^+$
can be replaced by any bounded domain in
$B_{R}^+$
can be replaced by any bounded domain in
![]() $\mathbb {R}^n_+$
. Indeed, for any bounded domain
$\mathbb {R}^n_+$
. Indeed, for any bounded domain
![]() $\Omega \subset \mathbb {R}^n_+$
, there exists some
$\Omega \subset \mathbb {R}^n_+$
, there exists some
![]() $R>0$
such that
$R>0$
such that
![]() $\Omega \subset B_R^+$
.
$\Omega \subset B_R^+$
.
This paper is organised as follows. In Section 2, we prove a basic and crucial lemma on the measure of cross sections of solutions and introduce some important lemmas which were obtained in [Reference Savin8, Reference Savin9]. In Section 3, we prove Theorems 1.1 and 1.2 and then we use Theorem 1.1 in Section 4 to obtain an asymptotic result for Monge–Ampère equations in half spaces.
2 Preliminaries
From now on, for any
![]() $u\in C^0(\overline {\mathbb {R}^n_+})$
in Theorem 1.1, we assume
$u\in C^0(\overline {\mathbb {R}^n_+})$
in Theorem 1.1, we assume
which means that
![]() $x_{n+1}=0$
is a support plane of u at the origin, but for any
$x_{n+1}=0$
is a support plane of u at the origin, but for any
![]() $\epsilon>0$
,
$\epsilon>0$
,
![]() $x_{n+1}=\epsilon x_n$
is not a support plane. We can reduce the general case to (2.1) by subtracting a linear function. Then we can denote by
$x_{n+1}=\epsilon x_n$
is not a support plane. We can reduce the general case to (2.1) by subtracting a linear function. Then we can denote by
the cross section of u at zero with height h (see [Reference Figalli2, Reference Gutiérrez4]). We write
![]() $S_h$
for convenience if there is no confusion. Equation (2.1) together with (1.6) shows that
$S_h$
for convenience if there is no confusion. Equation (2.1) together with (1.6) shows that
2.1 Estimate of cross sections
In this subsection, we first estimate the Lebesgue measure of cross sections as in [Reference Savin8].
Lemma 2.1. Assume that the convex function
![]() $u\in C^0(\overline {\mathbb {R}^n_{+}})$
solves
$u\in C^0(\overline {\mathbb {R}^n_{+}})$
solves
 $$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{ in } \mathbb{R}^n_+,\\ u(x',x_n)=\varphi(x') & \mbox{on } \{x_n=0\}, \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2u(x)=f(x) & \mbox{ in } \mathbb{R}^n_+,\\ u(x',x_n)=\varphi(x') & \mbox{on } \{x_n=0\}, \end{cases} \end{align} $$
where f satisfies (1.3),
![]() $\varphi $
satisfies (1.6) and u satisfies (2.1). Then for any
$\varphi $
satisfies (1.6) and u satisfies (2.1). Then for any
![]() $h>0$
,
$h>0$
,
where
![]() $C>0$
depends only on
$C>0$
depends only on
![]() $\lambda $
,
$\lambda $
,
![]() $\varphi (x')$
and n.
$\varphi (x')$
and n.
Proof. For all
![]() $h>0$
, let
$h>0$
, let
![]() $x_h^*=((x_h^*)',x_h^*\cdot e_n)$
be the centre of mass of
$x_h^*=((x_h^*)',x_h^*\cdot e_n)$
be the centre of mass of
![]() $S_h$
. We define
$S_h$
. We define
 $$ \begin{align*} A_hx = x-\nu x_n,\quad \nu=\bigg(\frac{(x_h^*)^{\prime}}{x_h^* \cdot e_n}, 0\bigg),\quad \widetilde{u}(A_hx)=u(x). \end{align*} $$
$$ \begin{align*} A_hx = x-\nu x_n,\quad \nu=\bigg(\frac{(x_h^*)^{\prime}}{x_h^* \cdot e_n}, 0\bigg),\quad \widetilde{u}(A_hx)=u(x). \end{align*} $$
Then the centre of mass of
![]() $\widetilde {S}_h:=\{x\in \mathbb {R}^n_+: \widetilde {u}(x)<h\}$
satisfies
$\widetilde {S}_h:=\{x\in \mathbb {R}^n_+: \widetilde {u}(x)<h\}$
satisfies
In view of the definition of
![]() $A_h$
:
$A_h$
:
-
•
 $\det |A_h|=1$
and then
$\det |A_h|=1$
and then  $$ \begin{align*}\det D^2\widetilde{u}(x)=\det((A_h^{-1})^T D^2u A_h^{-1})(A_h^{-1}x)=\det D^2u(A_h^{-1}x)=f(A_h^{-1}x);\end{align*} $$
$$ \begin{align*}\det D^2\widetilde{u}(x)=\det((A_h^{-1})^T D^2u A_h^{-1})(A_h^{-1}x)=\det D^2u(A_h^{-1}x)=f(A_h^{-1}x);\end{align*} $$
-
• the sliding transformation preserves the volume of the level set, that is,
 $$ \begin{align*} |\widetilde{S}_h|=\int_{\widetilde{S}_h} \,{d} y=\int_{S_h} \,{d} A_h x=\int_{S_h}|A_h| \,{d} x=|S_h|; \end{align*} $$
$$ \begin{align*} |\widetilde{S}_h|=\int_{\widetilde{S}_h} \,{d} y=\int_{S_h} \,{d} A_h x=\int_{S_h}|A_h| \,{d} x=|S_h|; \end{align*} $$
-
• the centre of mass
 $\widetilde {x}_h^*$
of
$\widetilde {x}_h^*$
of
 $\widetilde {S}_h=A_h S_h$
lies on the
$\widetilde {S}_h=A_h S_h$
lies on the
 $x_n$
-axis, since for
$x_n$
-axis, since for
 $i=1,2, \ldots , n-1$
,
$i=1,2, \ldots , n-1$
,  $$ \begin{align*} \begin{aligned} \widetilde{x}_h^* \cdot e_i & =\frac{1}{|\widetilde{S}_h|} \int_{\widetilde{S}_h}\bigg(x_i-\frac{x_h^* \cdot e_i}{x_h^* \cdot e_n}x_n\bigg) \cdot \operatorname{det} A_h \,{d} x =\frac{1}{|S_h|} \int_{S_h} x_i \,{d} x-x_h^* \cdot e_i =0. \end{aligned} \end{align*} $$
$$ \begin{align*} \begin{aligned} \widetilde{x}_h^* \cdot e_i & =\frac{1}{|\widetilde{S}_h|} \int_{\widetilde{S}_h}\bigg(x_i-\frac{x_h^* \cdot e_i}{x_h^* \cdot e_n}x_n\bigg) \cdot \operatorname{det} A_h \,{d} x =\frac{1}{|S_h|} \int_{S_h} x_i \,{d} x-x_h^* \cdot e_i =0. \end{aligned} \end{align*} $$
By using John’s lemma (relabelling the
![]() $x'$
coordinates if necessary),
$x'$
coordinates if necessary),
where
![]() $D_h=\mbox {diag}(d_1,d_2\ldots ,d_n)$
. We first claim that for all
$D_h=\mbox {diag}(d_1,d_2\ldots ,d_n)$
. We first claim that for all
![]() $h>0$
,
$h>0$
,
 $$ \begin{align} \prod_{i=1}^{n}d_i\geq c_0h^{{n}/{2}}, \end{align} $$
$$ \begin{align} \prod_{i=1}^{n}d_i\geq c_0h^{{n}/{2}}, \end{align} $$
where
![]() $c_0>0$
depends only on
$c_0>0$
depends only on
![]() $\lambda $
,
$\lambda $
,
![]() $\varphi (x')$
and n. It is clear that (2.6) immediately implies
$\varphi (x')$
and n. It is clear that (2.6) immediately implies
![]() $|\widetilde {S}_h|\geq ch^{{n}/{2}}$
for some constant c depending only on
$|\widetilde {S}_h|\geq ch^{{n}/{2}}$
for some constant c depending only on
![]() $\lambda $
,
$\lambda $
,
![]() $\varphi (x')$
and n. Now we show (2.6). Let
$\varphi (x')$
and n. Now we show (2.6). Let
 $$ \begin{align*} w=\varepsilon x_n+ \sum_{i=1}^{n}ch\bigg(\frac{x_i}{d_i}\bigg)^2, \end{align*} $$
$$ \begin{align*} w=\varepsilon x_n+ \sum_{i=1}^{n}ch\bigg(\frac{x_i}{d_i}\bigg)^2, \end{align*} $$
where
![]() $\varepsilon , c>0$
are small constants to be determined. The boundary condition implies that
$\varepsilon , c>0$
are small constants to be determined. The boundary condition implies that
![]() $ c_1|x'|^2\leq \widetilde { u}(x',0)\leq c_1^{-1}|x'|^2$
for some
$ c_1|x'|^2\leq \widetilde { u}(x',0)\leq c_1^{-1}|x'|^2$
for some
![]() $c_1$
depending only on
$c_1$
depending only on
![]() $\varphi (x')$
. Then for all
$\varphi (x')$
. Then for all
![]() $h>0$
,
$h>0$
,
 $$ \begin{align*} \widetilde{S}_h =\{x \in \overline{\mathbb{R}_{+}^n}: \widetilde{u}(x)<h\} & \supset\{(x^{\prime}, 0) \in \mathbb{R}^{n-1} \times\{x_n=0\}: c_1^{-1}|x^{\prime}|^2<h\} \\ & =B_{c_1^{1/2}h^{1/2}} \cap\{x_n=0\}. \end{align*} $$
$$ \begin{align*} \widetilde{S}_h =\{x \in \overline{\mathbb{R}_{+}^n}: \widetilde{u}(x)<h\} & \supset\{(x^{\prime}, 0) \in \mathbb{R}^{n-1} \times\{x_n=0\}: c_1^{-1}|x^{\prime}|^2<h\} \\ & =B_{c_1^{1/2}h^{1/2}} \cap\{x_n=0\}. \end{align*} $$
Consequently, since
![]() $\widetilde {x}_h^*$
lands on the
$\widetilde {x}_h^*$
lands on the
![]() $x_n$
-axis and
$x_n$
-axis and
![]() $C(n) D_h B_1$
is an ellipsoid,
$C(n) D_h B_1$
is an ellipsoid,
where
![]() $C=C(n)>0$
. Using (1.6) and (2.1), we can choose
$C=C(n)>0$
. Using (1.6) and (2.1), we can choose
![]() $\varepsilon >0$
depending only on
$\varepsilon >0$
depending only on
![]() $h$
, and
$h$
, and
![]() $c>0$
small depending only on
$c>0$
small depending only on
![]() $\lambda$
,
$\lambda$
,
![]() $\varphi(x')$
and
$\varphi(x')$
and
![]() $n$
, such that for all
$n$
, such that for all
![]() $h>0$
,
$h>0$
,
If (2.6) is false, then
 $$ \begin{align*} \det D^2w=(2ch)^n\bigg(\prod_{i=1}^{n}d_i\bigg)^{-2}>\Lambda. \end{align*} $$
$$ \begin{align*} \det D^2w=(2ch)^n\bigg(\prod_{i=1}^{n}d_i\bigg)^{-2}>\Lambda. \end{align*} $$
By the comparison principle, we have
![]() $w\leq \widetilde {u}$
in
$w\leq \widetilde {u}$
in
![]() $\widetilde {S}_h$
. By the definition of
$\widetilde {S}_h$
. By the definition of
![]() $\widetilde {u}$
,
$\widetilde {u}$
,
![]() $x_{n+1}=0$
is also the tangent plane of
$x_{n+1}=0$
is also the tangent plane of
![]() $\widetilde {u}$
at the origin. This gives a contradiction since
$\widetilde {u}$
at the origin. This gives a contradiction since
![]() $\widetilde {u}\geq w\geq \varepsilon x_n$
. Thus, (2.6) is proved.
$\widetilde {u}\geq w\geq \varepsilon x_n$
. Thus, (2.6) is proved.
Next, we only need to show that for all
![]() $h>0$
,
$h>0$
,
where C is a large constant depending only on
![]() $\lambda $
and n. In fact, for all
$\lambda $
and n. In fact, for all
![]() $h>0$
, there exists
$h>0$
, there exists
![]() $v\in C^0(\widetilde {S}_h)$
satisfying
$v\in C^0(\widetilde {S}_h)$
satisfying
 $$ \begin{align} \begin{cases} \det D^2v=\lambda & \mbox{in } \widetilde{S}_h,\\ v=h & \mbox{on } \partial\widetilde{S}_h. \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2v=\lambda & \mbox{in } \widetilde{S}_h,\\ v=h & \mbox{on } \partial\widetilde{S}_h. \end{cases} \end{align} $$
By the comparison principle,
![]() $v\geq u\geq 0$
in
$v\geq u\geq 0$
in
![]() $\widetilde {S}_h$
. There exists some constant
$\widetilde {S}_h$
. There exists some constant
![]() $c>0$
small depending only on
$c>0$
small depending only on
![]() $\lambda $
and n such that
$\lambda $
and n such that
(see [Reference Mooney6, Lemma 2.2] and [Reference Gutiérrez4]). This establishes (2.7).
2.2 Two kinds of function spaces
First, we define the classes
![]() $\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
and
$\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
and
![]() $\mathbb {D}_0^\mu (a_1,\ldots ,a_{n-1})$
(see [Reference Savin8, Reference Savin9]).
$\mathbb {D}_0^\mu (a_1,\ldots ,a_{n-1})$
(see [Reference Savin8, Reference Savin9]).
The class
![]() $\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
. Let
$\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
. Let
![]() $\mu $
and
$\mu $
and
![]() $\lambda $
be positive small fixed constants. For an increasing sequence
$\lambda $
be positive small fixed constants. For an increasing sequence
![]() $\{a_i\}_{i=1}^{n-1}$
with
$\{a_i\}_{i=1}^{n-1}$
with
![]() $\mu \leq a_1\leq a_2 \leq \cdots \leq a_{n-1}$
, we say that the convex function
$\mu \leq a_1\leq a_2 \leq \cdots \leq a_{n-1}$
, we say that the convex function
![]() $u:\overline {\Omega }\rightarrow \mathbb {R}$
belongs to the function space
$u:\overline {\Omega }\rightarrow \mathbb {R}$
belongs to the function space
![]() $\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
if u and
$\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
if u and
![]() $\Omega $
satisfy
$\Omega $
satisfy
the boundary
![]() $\partial \Omega $
has a closed subset
$\partial \Omega $
has a closed subset
![]() $G\subset \{x_n\leq \sigma \}\cap \partial \Omega $
, which is a graph in the
$G\subset \{x_n\leq \sigma \}\cap \partial \Omega $
, which is a graph in the
![]() $e_n$
direction with projection
$e_n$
direction with projection
![]() $\pi _n(G)\subset \mathbb {R}^{n-1}$
along
$\pi _n(G)\subset \mathbb {R}^{n-1}$
along
![]() $e_n$
, that is,
$e_n$
, that is,
 $$ \begin{align*} \bigg\{ \mu^{-1} \sum_{i=1}^{n-1}a_i^2x_i^2 \leq 1 \bigg\}\subset \pi_n(G)\subset \bigg\{ \mu \sum_{i=1}^{n-1}a_i^2x_i^2 \leq 1 \bigg\}, \end{align*} $$
$$ \begin{align*} \bigg\{ \mu^{-1} \sum_{i=1}^{n-1}a_i^2x_i^2 \leq 1 \bigg\}\subset \pi_n(G)\subset \bigg\{ \mu \sum_{i=1}^{n-1}a_i^2x_i^2 \leq 1 \bigg\}, \end{align*} $$
and
![]() $u=\varphi $
on
$u=\varphi $
on
![]() $\partial \Omega $
with
$\partial \Omega $
with
 $$ \begin{align*} \varphi=1 \quad \mbox{on }\partial\Omega\backslash G \quad\mbox{and}\quad \mu \sum_{i=1}^{n-1}a_i^2x_i^2 \leq \varphi \leq \min\bigg\{1, \mu^{-1} \sum_{i=1}^{n-1}a_i^2x_i^2 \bigg\} \quad \text {on } G. \end{align*} $$
$$ \begin{align*} \varphi=1 \quad \mbox{on }\partial\Omega\backslash G \quad\mbox{and}\quad \mu \sum_{i=1}^{n-1}a_i^2x_i^2 \leq \varphi \leq \min\bigg\{1, \mu^{-1} \sum_{i=1}^{n-1}a_i^2x_i^2 \bigg\} \quad \text {on } G. \end{align*} $$
Note that in [Reference Savin8, Reference Savin9],
![]() $\mathcal {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
denotes two different function spaces, both of which will be used in our paper.
$\mathcal {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
denotes two different function spaces, both of which will be used in our paper.
The class
![]() $\mathbb {D}_0^\mu (a_1,\ldots ,a_{n-1})$
. We introduce the limiting solutions of
$\mathbb {D}_0^\mu (a_1,\ldots ,a_{n-1})$
. We introduce the limiting solutions of
![]() $\mathbb {D}_{\sigma }^{\mu }(a_{1}, \ldots , a_{n-1})$
when
$\mathbb {D}_{\sigma }^{\mu }(a_{1}, \ldots , a_{n-1})$
when
![]() $a_{k+1} \rightarrow \infty $
and
$a_{k+1} \rightarrow \infty $
and
![]() $\sigma \rightarrow 0$
. If
$\sigma \rightarrow 0$
. If
![]() $\mu \leq a_{1} \leq \cdots \leq a_{k},$
we denote by
$\mu \leq a_{1} \leq \cdots \leq a_{k},$
we denote by
the class of functions u satisfying (2.9), (2.10), (2.11) with
![]() $ G \subset \{x_{i}=0: i>k\} \cap \partial \Omega , $
and, if we restrict to the space generated by the first k coordinates, then
$ G \subset \{x_{i}=0: i>k\} \cap \partial \Omega , $
and, if we restrict to the space generated by the first k coordinates, then
 $$ \begin{align*} \bigg\{\mu^{-1} \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2} \leq 1\bigg\} \subset G \subset\bigg\{\mu \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2} \leq 1\bigg\}, \end{align*} $$
$$ \begin{align*} \bigg\{\mu^{-1} \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2} \leq 1\bigg\} \subset G \subset\bigg\{\mu \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2} \leq 1\bigg\}, \end{align*} $$
and
![]() $u=\varphi $
on
$u=\varphi $
on
![]() $\partial \Omega $
with
$\partial \Omega $
with
 $$ \begin{align*} \varphi=1 \quad \text {on } \partial \Omega \backslash G \quad\mbox{and}\quad \mu \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2} \leq \varphi \leq \min \bigg\{1, \mu^{-1} \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2}\bigg\} \quad \text {on } G. \end{align*} $$
$$ \begin{align*} \varphi=1 \quad \text {on } \partial \Omega \backslash G \quad\mbox{and}\quad \mu \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2} \leq \varphi \leq \min \bigg\{1, \mu^{-1} \sum_{i=1}^{k} a_{i}^{2} x_{i}^{2}\bigg\} \quad \text {on } G. \end{align*} $$
Remark 2.2. By Lemma 2.1, there exists some
![]() $\mu $
, depending only on
$\mu $
, depending only on
![]() $\lambda , \varphi (x^{\prime })$
and n, such that (2.10) holds. Consequently, the solution u in Lemma 2.1 belongs to some space
$\lambda , \varphi (x^{\prime })$
and n, such that (2.10) holds. Consequently, the solution u in Lemma 2.1 belongs to some space
![]() $\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
.
$\mathbb {D}_\sigma ^\mu (a_1,\ldots ,a_{n-1})$
.
Lemma 2.3 [Reference Savin8, Theorem 2.7].
Assume
![]() $u_m\in \mathbb {D}_{\sigma _m}^\mu (a_1^m,\ldots ,a_{n-1}^m)$
is a sequence of functions with
$u_m\in \mathbb {D}_{\sigma _m}^\mu (a_1^m,\ldots ,a_{n-1}^m)$
is a sequence of functions with
![]() $\sigma _m\rightarrow 0, a_{k+1}\rightarrow \infty $
. Then we can extract a subsequence converging to a function u with
$\sigma _m\rightarrow 0, a_{k+1}\rightarrow \infty $
. Then we can extract a subsequence converging to a function u with
![]() $u\in \mathbb {D}_{0}^\mu (a_1,\ldots ,a_{l}, \infty ,\ldots ,\infty )$
for some
$u\in \mathbb {D}_{0}^\mu (a_1,\ldots ,a_{l}, \infty ,\ldots ,\infty )$
for some
![]() $l\in [0,k]$
.
$l\in [0,k]$
.
The class
![]() $\mathcal {D}^{{\mu }}_\sigma $
. Let
$\mathcal {D}^{{\mu }}_\sigma $
. Let
![]() ${\mu }, \sigma $
be small positive fixed constants and let
${\mu }, \sigma $
be small positive fixed constants and let
![]() ${\mu } \leq a_1 \leq \cdots \leq a_{n-1}$
be real numbers. We say that
${\mu } \leq a_1 \leq \cdots \leq a_{n-1}$
be real numbers. We say that
![]() $ u \in \mathcal {D}^{{\mu }}_\sigma (a_1, \ldots , a_{n-1}) $
if u is a continuous convex function defined on a convex set
$ u \in \mathcal {D}^{{\mu }}_\sigma (a_1, \ldots , a_{n-1}) $
if u is a continuous convex function defined on a convex set
![]() $\bar {\Omega }$
such that:
$\bar {\Omega }$
such that:
-
(1)
 $0 \in \partial \Omega $
,
$0 \in \partial \Omega $
,
 $B_{{\mu }}(x_0) \subset \Omega \subset B_{1 / {\mu }}^{+} (0)$
for some
$B_{{\mu }}(x_0) \subset \Omega \subset B_{1 / {\mu }}^{+} (0)$
for some
 $x_0$
,
$x_0$
,
 $1 \geq u \geq 0$
,
$1 \geq u \geq 0$
,
 $u(0)=0$
,
$u(0)=0$
,
 $\nabla u(0)=0$
;
$\nabla u(0)=0$
; -
(2) in the interior of
 $\Omega $
, the function u satisfies
$\Omega $
, the function u satisfies
 $1-\sigma \leq \operatorname {det} D^2 u \leq 1+\sigma $
;
$1-\sigma \leq \operatorname {det} D^2 u \leq 1+\sigma $
; -
(3) on
 $\partial \Omega $
, the function u satisfies the following condition: there is a closed set
$\partial \Omega $
, the function u satisfies the following condition: there is a closed set
 ${G \subset \partial \Omega }$
, which is a graph
${G \subset \partial \Omega }$
, which is a graph
 $(x^{\prime }, g(x^{\prime }))$
with
$(x^{\prime }, g(x^{\prime }))$
with
 $g(x^{\prime }) \leq \sigma |x^{\prime }|^2$
, such that
$g(x^{\prime }) \leq \sigma |x^{\prime }|^2$
, such that
 $u=1$
on
$u=1$
on
 $\partial \Omega \backslash G$
and
$\partial \Omega \backslash G$
and
 $(1-\sigma ) \varphi _u(x^{\prime }) \leq u \leq (1+\sigma ) \varphi _u(x^{\prime })$
on G for some function
$(1-\sigma ) \varphi _u(x^{\prime }) \leq u \leq (1+\sigma ) \varphi _u(x^{\prime })$
on G for some function
 $\varphi _u$
satisfying
$\varphi _u$
satisfying  $$ \begin{align*} {\mu}^{-1} \mathcal{N} \geq D_{x^{\prime}}^2 \varphi_u \geq {\mu} \mathcal{N}, \quad \text {with } \mathcal{N}=\operatorname{diag}(a_1^2, a_2^2, \ldots, a_{n-1}^2). \end{align*} $$
$$ \begin{align*} {\mu}^{-1} \mathcal{N} \geq D_{x^{\prime}}^2 \varphi_u \geq {\mu} \mathcal{N}, \quad \text {with } \mathcal{N}=\operatorname{diag}(a_1^2, a_2^2, \ldots, a_{n-1}^2). \end{align*} $$
The class
![]() $\mathcal {D}_{{0}}^\sigma $
. Let
$\mathcal {D}_{{0}}^\sigma $
. Let
![]() $\mu $
be a small positive fixed constant and
$\mu $
be a small positive fixed constant and
![]() $\mu \leq a_1\leq a_2\leq \cdots \leq a_k$
be k real numbers with
$\mu \leq a_1\leq a_2\leq \cdots \leq a_k$
be k real numbers with
![]() $0\leq k\leq n-1$
. We say
$0\leq k\leq n-1$
. We say
![]() $ u\in \mathcal {D}_0^\mu (a_1,\ldots ,a_{k},\infty ,\ldots ,\infty )$
if
$ u\in \mathcal {D}_0^\mu (a_1,\ldots ,a_{k},\infty ,\ldots ,\infty )$
if
![]() $u:\overline {\Omega }\rightarrow \mathbb {R}$
is a convex function defined on the convex domain
$u:\overline {\Omega }\rightarrow \mathbb {R}$
is a convex function defined on the convex domain
![]() $\overline \Omega $
such that
$\overline \Omega $
such that
 $$ \begin{align} u= \begin{cases} \varphi_u & \mbox{on }G\subset \partial\Omega, \\ 1 & \mbox{on }\partial\Omega\backslash G, \end{cases} \end{align} $$
$$ \begin{align} u= \begin{cases} \varphi_u & \mbox{on }G\subset \partial\Omega, \\ 1 & \mbox{on }\partial\Omega\backslash G, \end{cases} \end{align} $$
where
![]() $ \varphi _u(x_1,\ldots ,x_k)$
is a nonnegative convex function of k variables satisfying
$ \varphi _u(x_1,\ldots ,x_k)$
is a nonnegative convex function of k variables satisfying
and G represents the k dimensional set of
![]() $\mathbb {R}^n$
where
$\mathbb {R}^n$
where
![]() $\varphi _u\leq 1$
, that is,
$\varphi _u\leq 1$
, that is,
Remark 2.4. The difference between the definitions of
![]() $\mathbb {D}^\mu _\sigma $
and
$\mathbb {D}^\mu _\sigma $
and
![]() $\mathcal {D}^\mu _\sigma $
is that the functions u solve different equations. The condition on f in
$\mathcal {D}^\mu _\sigma $
is that the functions u solve different equations. The condition on f in
![]() $\mathcal {D}^\mu _0$
is stronger than that in
$\mathcal {D}^\mu _0$
is stronger than that in
![]() $\mathbb {D}^\mu _0$
.
$\mathbb {D}^\mu _0$
.
Savin obtained the following crucial result (see [Reference Savin9, Lemma 8.3] with
![]() $\alpha =0$
).
$\alpha =0$
).
Lemma 2.5. For any
![]() $\varepsilon '>0$
small, there exists some
$\varepsilon '>0$
small, there exists some
![]() $C_*$
depending only on
$C_*$
depending only on
![]() $\varepsilon '$
,
$\varepsilon '$
,
![]() $\mu $
,
$\mu $
,
![]() $\lambda $
,
$\lambda $
,
![]() $\Lambda $
and n such that if
$\Lambda $
and n such that if
![]() $ u\in \mathcal {D}_0^\mu (a_1, \ldots , a_{n-1})$
with
$ u\in \mathcal {D}_0^\mu (a_1, \ldots , a_{n-1})$
with
![]() $a_{n-1}\geq C_*$
, then there exists some
$a_{n-1}\geq C_*$
, then there exists some
![]() ${t\in [C_*^{-1},1]}$
such that
${t\in [C_*^{-1},1]}$
such that
 $$ \begin{align*} \sup_{S_t(u)}x_n\geq \frac{2}{\mu}t^{{1}/{(3-\varepsilon')}}. \end{align*} $$
$$ \begin{align*} \sup_{S_t(u)}x_n\geq \frac{2}{\mu}t^{{1}/{(3-\varepsilon')}}. \end{align*} $$
3 Proof of the main theorems
In this section, we prove Theorems 1.1 and 1.2.
Proof of Theorem 1.1.
For all
![]() $h>0$
, as in the proof of Lemma 2.1, we define
$h>0$
, as in the proof of Lemma 2.1, we define
 $$ \begin{align*} A_hx = x-\nu x_n,\quad \nu=\bigg(\frac{(x_h^*)^{\prime}}{x_h^* \cdot e_n}, 0\bigg), \quad \widetilde{u}(A_hx)=u(x), \end{align*} $$
$$ \begin{align*} A_hx = x-\nu x_n,\quad \nu=\bigg(\frac{(x_h^*)^{\prime}}{x_h^* \cdot e_n}, 0\bigg), \quad \widetilde{u}(A_hx)=u(x), \end{align*} $$
where
![]() $x_h^*=((x_h^*)',x_h^*\cdot e_n)$
is the centre of mass of
$x_h^*=((x_h^*)',x_h^*\cdot e_n)$
is the centre of mass of
![]() $S_h$
. By using John’s lemma (relabelling the
$S_h$
. By using John’s lemma (relabelling the
![]() $x'$
coordinates if necessary),
$x'$
coordinates if necessary),
where
![]() $D_h=\mbox {diag}(d_1,\ldots ,d_n)$
. Then,
$D_h=\mbox {diag}(d_1,\ldots ,d_n)$
. Then,
 $$ \begin{align*} \begin{cases} \det D^2\widetilde{u}(x)=\widetilde{f}(x) &\mbox{in } \widetilde{S}_h=A_hS_h,\\ \widetilde{u}(x)=\varphi(x') &\mbox{on }\partial\widetilde{S}_h\cap\{x_n=0\},\\ \widetilde{u}(x)=h & \mbox{on }\partial\widetilde{S}_h\cap\{x_n>0\}, \end{cases} \end{align*} $$
$$ \begin{align*} \begin{cases} \det D^2\widetilde{u}(x)=\widetilde{f}(x) &\mbox{in } \widetilde{S}_h=A_hS_h,\\ \widetilde{u}(x)=\varphi(x') &\mbox{on }\partial\widetilde{S}_h\cap\{x_n=0\},\\ \widetilde{u}(x)=h & \mbox{on }\partial\widetilde{S}_h\cap\{x_n>0\}, \end{cases} \end{align*} $$
where
![]() $\widetilde {f}(x)=f(A_h^{-1}x)$
. For any
$\widetilde {f}(x)=f(A_h^{-1}x)$
. For any
![]() $h>0$
, we denote
$h>0$
, we denote
 $$ \begin{align*} \varsigma_h = h^{-{n}/{2}}\prod\limits_{i=1}^{n}d_i. \end{align*} $$
$$ \begin{align*} \varsigma_h = h^{-{n}/{2}}\prod\limits_{i=1}^{n}d_i. \end{align*} $$
By Lemma 2.1, there exists
![]() $C>0$
depending only on
$C>0$
depending only on
![]() $\lambda $
,
$\lambda $
,
![]() $\varphi (x')$
and n such that
$\varphi (x')$
and n such that
Note that
![]() $\varsigma _h$
has uniformly positive lower and upper bounds and is constant for any fixed
$\varsigma _h$
has uniformly positive lower and upper bounds and is constant for any fixed
![]() $h>0$
. Now we divide this proof into two steps.
$h>0$
. Now we divide this proof into two steps.
Step 1. We claim that
will never happen. In fact, if it happens, then
![]() $ \widetilde {u}(0,x_n)=o(x^2_n)$
as
$ \widetilde {u}(0,x_n)=o(x^2_n)$
as
![]() $x_n\rightarrow \infty $
, which implies
$x_n\rightarrow \infty $
, which implies
![]() $ d_n \cdot h^{-{1}/{2}} \rightarrow \infty $
as
$ d_n \cdot h^{-{1}/{2}} \rightarrow \infty $
as
![]() $h\rightarrow \infty $
. By the boundary condition, the property of
$h\rightarrow \infty $
. By the boundary condition, the property of
![]() $A_h$
and the convexity of
$A_h$
and the convexity of
![]() $\widetilde {S}_h$
,
$\widetilde {S}_h$
,
However, then
![]() $\varsigma _h \rightarrow \infty $
as
$\varsigma _h \rightarrow \infty $
as
![]() $h\rightarrow \infty $
, which contradicts (3.1). This completes Step 1.
$h\rightarrow \infty $
, which contradicts (3.1). This completes Step 1.
Step 2. We show that
Indeed, if not, then
By Lemma 2.1, there exists at least one of
![]() $\{d_i\}_{i=1}^{n-1}$
(we denote it by
$\{d_i\}_{i=1}^{n-1}$
(we denote it by
![]() $d_{n-1}$
) such that
$d_{n-1}$
) such that
Let
 $$ \begin{align*} U_h(x)=\frac{\widetilde{u}(F_hx)}{h},\quad F_hx=\bigg(d_1 x_1,d_2 x_2,\ldots, { {\frac{1}{\varsigma_h}}}d_n x_n\bigg). \end{align*} $$
$$ \begin{align*} U_h(x)=\frac{\widetilde{u}(F_hx)}{h},\quad F_hx=\bigg(d_1 x_1,d_2 x_2,\ldots, { {\frac{1}{\varsigma_h}}}d_n x_n\bigg). \end{align*} $$
It is easy to show that
![]() $U_h(x)$
solves
$U_h(x)$
solves
 $$ \begin{align} \begin{cases} \det D^2U_h=\widetilde{f}(F_hx) &\mbox{in } \mathcal{O}_h,\\ U_h(x)=\varphi((F_hx)') &\mbox{on } \partial\mathcal{O}_h\cap\{x_n=0\},\\ U_h(x)=1 &\mbox{on } \partial\mathcal{O}_h\cap\{x_n>0\}, \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2U_h=\widetilde{f}(F_hx) &\mbox{in } \mathcal{O}_h,\\ U_h(x)=\varphi((F_hx)') &\mbox{on } \partial\mathcal{O}_h\cap\{x_n=0\},\\ U_h(x)=1 &\mbox{on } \partial\mathcal{O}_h\cap\{x_n>0\}, \end{cases} \end{align} $$
where
![]() $\mathcal {O}_h=F_h^{-1}\widetilde {S}_h$
satisfies
$\mathcal {O}_h=F_h^{-1}\widetilde {S}_h$
satisfies
with
![]() $D=\mbox {diag}(1,1,\ldots ,\varsigma _h)$
. By its definition,
$D=\mbox {diag}(1,1,\ldots ,\varsigma _h)$
. By its definition,
![]() $U_h\in \mathbb {D}_0^\mu (a_1,a_2,\ldots ,a_{n-1})$
with
$U_h\in \mathbb {D}_0^\mu (a_1,a_2,\ldots ,a_{n-1})$
with
By (3.4),
![]() $a_{n-1}\rightarrow \infty $
as
$a_{n-1}\rightarrow \infty $
as
![]() $h\rightarrow \infty $
. The equations
$h\rightarrow \infty $
. The equations
 $$ \begin{align} \begin{cases} \det D^2w_h=1 & \mbox{in } \mathcal{O}_h,\\ w_h =\varphi((F_hx)') & \mbox{on } \partial\mathcal{O}_h\cap\{x_n=0\},\\ w_h =1& \mbox{on } \partial\mathcal{O}_h\cap\{x_n>0\}, \end{cases} \end{align} $$
$$ \begin{align} \begin{cases} \det D^2w_h=1 & \mbox{in } \mathcal{O}_h,\\ w_h =\varphi((F_hx)') & \mbox{on } \partial\mathcal{O}_h\cap\{x_n=0\},\\ w_h =1& \mbox{on } \partial\mathcal{O}_h\cap\{x_n>0\}, \end{cases} \end{align} $$
determine a unique convex function
![]() $w_h(x)\in C^0(\overline {\mathcal {O}_h})$
(see [Reference Jia, Li and Li5]). We now divide this step into three parts.
$w_h(x)\in C^0(\overline {\mathcal {O}_h})$
(see [Reference Jia, Li and Li5]). We now divide this step into three parts.
Step 2.1. We claim that for all
![]() $h>0$
small, there exists a constant
$h>0$
small, there exists a constant
![]() $C>0$
depending only on f,
$C>0$
depending only on f,
![]() $\lambda $
,
$\lambda $
,
![]() $\varphi $
and n such that
$\varphi $
and n such that
where s is the constant in (1.5). In fact, by the Alexandrov estimate (see, [Reference Gilbarg and Trudinger3, Lemma 9.2]),
 $$ \begin{align*} -\min_{\mathcal{O}_h}(w_h-U_h)\leq C\bigg\{\int_{\mathcal{O}_{h,1}}\det D^2(w_h-U_h)\bigg\}^{1/n}, \end{align*} $$
$$ \begin{align*} -\min_{\mathcal{O}_h}(w_h-U_h)\leq C\bigg\{\int_{\mathcal{O}_{h,1}}\det D^2(w_h-U_h)\bigg\}^{1/n}, \end{align*} $$
where
![]() $\mathcal {O}_{h,1}:=\{x\in \mathcal {O}_{h}: D^2(w_h-U_h)>0\}$
and
$\mathcal {O}_{h,1}:=\{x\in \mathcal {O}_{h}: D^2(w_h-U_h)>0\}$
and
![]() $C>0$
depends only on n and the diameter of the domain
$C>0$
depends only on n and the diameter of the domain
![]() $\mathcal {O}_h$
. By virtue of (3.6) and (3.1), the diameter can be controlled uniformly. By the convexity of
$\mathcal {O}_h$
. By virtue of (3.6) and (3.1), the diameter can be controlled uniformly. By the convexity of
![]() $(\det \cdot )^{{1}/{n}}$
,
$(\det \cdot )^{{1}/{n}}$
,
This implies that
Together with (1.3) and (1.5), this yields
 $$ \begin{align*} -\min_{\mathcal{O}_h}(w_h-U_h)&\leq C\bigg\{\int_{\mathcal{O}_{h,1}} (1-{\widetilde{f}^{1/n}(F_hx)})^n\,dx\bigg\}^{1/n}\\ &\leq Ch^{-{1}/{2}}\bigg\{\bigg(\int_{S_h\backslash B_{h^{{1}/{2(1+s)}}}^+}+\int_{B_{h^{{1}/{2(1+s)}}}^+} \bigg) (1-{\widetilde{f}^{1/n}(x)})^n\,dx\bigg\}^{1/n}\\ &\leq Ch^{-{1}/{2}}\{ h^{{n}/{2(1+s)}}+h^{-{sn}/{2(1+s)}+{n}/{2}}\}^{1/n}\\ &\leq Ch^{-{s}/{2(1+s)}}. \end{align*} $$
$$ \begin{align*} -\min_{\mathcal{O}_h}(w_h-U_h)&\leq C\bigg\{\int_{\mathcal{O}_{h,1}} (1-{\widetilde{f}^{1/n}(F_hx)})^n\,dx\bigg\}^{1/n}\\ &\leq Ch^{-{1}/{2}}\bigg\{\bigg(\int_{S_h\backslash B_{h^{{1}/{2(1+s)}}}^+}+\int_{B_{h^{{1}/{2(1+s)}}}^+} \bigg) (1-{\widetilde{f}^{1/n}(x)})^n\,dx\bigg\}^{1/n}\\ &\leq Ch^{-{1}/{2}}\{ h^{{n}/{2(1+s)}}+h^{-{sn}/{2(1+s)}+{n}/{2}}\}^{1/n}\\ &\leq Ch^{-{s}/{2(1+s)}}. \end{align*} $$
Similarly,
 $$ \begin{align*} -\min_{\mathcal{O}_h}(U_h-w_h)\leq C\bigg\{\int_{\mathcal{O}_{h,2}} ({\widetilde{f}^{1/n}(F_hx)}-1)^n\bigg\}^{1/n}\leq Ch^{-{s}/{2(1+s)}}, \end{align*} $$
$$ \begin{align*} -\min_{\mathcal{O}_h}(U_h-w_h)\leq C\bigg\{\int_{\mathcal{O}_{h,2}} ({\widetilde{f}^{1/n}(F_hx)}-1)^n\bigg\}^{1/n}\leq Ch^{-{s}/{2(1+s)}}, \end{align*} $$
where
![]() $ \mathcal {O}_{h,2}:=\{x\in \mathcal {O}_{h}: D^2(U_h-w_h)>0\}. $
Thus,
$ \mathcal {O}_{h,2}:=\{x\in \mathcal {O}_{h}: D^2(U_h-w_h)>0\}. $
Thus,
![]() $|w_h-U_h|\leq Ch^{-{s}/{2(1+s)}}$
in
$|w_h-U_h|\leq Ch^{-{s}/{2(1+s)}}$
in
![]() $\mathcal {O}_h$
.
$\mathcal {O}_h$
.
Step 2.2. We claim that
In fact, if not, then for any small
![]() $\varepsilon>0$
, there exists a subsequence
$\varepsilon>0$
, there exists a subsequence
![]() ${w_{h_m}}$
such that
${w_{h_m}}$
such that
![]() $D_nw_{w_{h_m}}(0)\nrightarrow 0$
as
$D_nw_{w_{h_m}}(0)\nrightarrow 0$
as
![]() $m\rightarrow \infty $
. Then, by Lemma 2.3, we can extract a convergent subsequence
$m\rightarrow \infty $
. Then, by Lemma 2.3, we can extract a convergent subsequence
![]() $U_{h_{m_k}}$
to a function
$U_{h_{m_k}}$
to a function
![]() $U_\infty \in \mathbb {D}_0^\mu (a_1,a_2,\ldots ,a_{n-1})$
with
$U_\infty \in \mathbb {D}_0^\mu (a_1,a_2,\ldots ,a_{n-1})$
with
![]() $ U_{h_{m_{k}}}\rightarrow U_\infty $
as
$ U_{h_{m_{k}}}\rightarrow U_\infty $
as
![]() $k\rightarrow \infty $
. Combined with Step 2.1, we obtain
$k\rightarrow \infty $
. Combined with Step 2.1, we obtain
![]() $ w_{h_{m_{k}}}\rightarrow U_\infty $
as
$ w_{h_{m_{k}}}\rightarrow U_\infty $
as
![]() $k\rightarrow \infty $
.
$k\rightarrow \infty $
.
Since
![]() $\nabla U_{h_{m_{k}}}(0)=0$
, we have
$\nabla U_{h_{m_{k}}}(0)=0$
, we have
![]() $D_nU_\infty (0)=0$
(which means that the support plane of
$D_nU_\infty (0)=0$
(which means that the support plane of
![]() $U_{\infty }$
at the origin is
$U_{\infty }$
at the origin is
![]() $x_{n+1}=0$
, that is, for any small
$x_{n+1}=0$
, that is, for any small
![]() $\varepsilon>0$
, the plane
$\varepsilon>0$
, the plane
![]() $x_{n+1}=\varepsilon x_n$
is not a support plane of
$x_{n+1}=\varepsilon x_n$
is not a support plane of
![]() $U_{\infty }$
at the origin). However, this contradicts the previous convergence result. Therefore,
$U_{\infty }$
at the origin). However, this contradicts the previous convergence result. Therefore,
![]() $D_nw_h(0)\rightarrow 0$
as
$D_nw_h(0)\rightarrow 0$
as
![]() $h\rightarrow \infty $
.
$h\rightarrow \infty $
.
Step 2.3. Now we show (3.2). For h large enough, by Step 2.2, we can subtract a linear function
![]() $l_h=D_nw_h(0)x_n$
, which is very close to the plane
$l_h=D_nw_h(0)x_n$
, which is very close to the plane
![]() $x_{n+1}=0$
, such that
$x_{n+1}=0$
, such that
![]() $w_h-l_h$
belongs to
$w_h-l_h$
belongs to
![]() $\mathcal {D}_0^\mu (a_1,a_2,\ldots ,a_{n-1})$
. This together with Lemma 2.5 implies that for any
$\mathcal {D}_0^\mu (a_1,a_2,\ldots ,a_{n-1})$
. This together with Lemma 2.5 implies that for any
![]() $\varepsilon '>0$
small, there exists some
$\varepsilon '>0$
small, there exists some
![]() $t\in [C_*^{-1},1]$
such that
$t\in [C_*^{-1},1]$
such that
 $$ \begin{align} \sup_{S_t(w_h)}x_n\geq \frac{7}{4\mu}t^{{1}/{(3-\varepsilon')}} \end{align} $$
$$ \begin{align} \sup_{S_t(w_h)}x_n\geq \frac{7}{4\mu}t^{{1}/{(3-\varepsilon')}} \end{align} $$
for large h, where
![]() $t<1$
since
$t<1$
since
![]() $\sup _{S_1(w_h)}x_n\leq \mu ^{-1}$
and
$\sup _{S_1(w_h)}x_n\leq \mu ^{-1}$
and
![]() $a_{n-1}$
is large enough. Indeed, if
$a_{n-1}$
is large enough. Indeed, if
![]() $a_{n-1} \leq C_*^{-1}$
, one can deduce that
$a_{n-1} \leq C_*^{-1}$
, one can deduce that
![]() $d_n\cdot h^{-1/2}\rightarrow 0$
will never happen. This completes the proof of Step 2.3.
$d_n\cdot h^{-1/2}\rightarrow 0$
will never happen. This completes the proof of Step 2.3.
Equation (3.8) together with Step 2.1 yields
 $$ \begin{align*} \sup_{S_{t+\varrho}(U_{h})}x_n\geq \frac{7}{4\mu}t^{{1}/{(3-\varepsilon')}} \geq\frac{3}{2\mu}(t+\varrho)^{{1}/{(3-\varepsilon')}}, \end{align*} $$
$$ \begin{align*} \sup_{S_{t+\varrho}(U_{h})}x_n\geq \frac{7}{4\mu}t^{{1}/{(3-\varepsilon')}} \geq\frac{3}{2\mu}(t+\varrho)^{{1}/{(3-\varepsilon')}}, \end{align*} $$
for all
![]() $\varrho $
small enough and large h. Without loss of generality, we may assume that for some
$\varrho $
small enough and large h. Without loss of generality, we may assume that for some
![]() $t\in [C_*^{-1},1]$
and all
$t\in [C_*^{-1},1]$
and all
![]() ${h}\geq h_0$
,
${h}\geq h_0$
,
 $$ \begin{align*} b(t):=\sup_{S_t(U_{h})}x_n\geq \frac{3}{2\mu}t^{{1}/{(3-\varepsilon')}}. \end{align*} $$
$$ \begin{align*} b(t):=\sup_{S_t(U_{h})}x_n\geq \frac{3}{2\mu}t^{{1}/{(3-\varepsilon')}}. \end{align*} $$
Together with the definition of
![]() $U_h$
, this means that for some
$U_h$
, this means that for some
![]() $t\in [C_*^{-1},1]$
,
$t\in [C_*^{-1},1]$
,
 $$ \begin{align*} \frac{b_{\widetilde{u}}(t{h})}{b_{\widetilde{u}}(h)}=\frac{b(t)}{b(1)}\geq \frac{3}{2\mu}t^{{1}/{(3-\varepsilon')}}, \end{align*} $$
$$ \begin{align*} \frac{b_{\widetilde{u}}(t{h})}{b_{\widetilde{u}}(h)}=\frac{b(t)}{b(1)}\geq \frac{3}{2\mu}t^{{1}/{(3-\varepsilon')}}, \end{align*} $$
where
Note that
![]() $b_{\widetilde {u}}(t{h})$
is different from
$b_{\widetilde {u}}(t{h})$
is different from
![]() $\sup _{S_{t{h}}(\widetilde {u})} x_n$
. Then for any
$\sup _{S_{t{h}}(\widetilde {u})} x_n$
. Then for any
![]() ${h}$
large enough,
${h}$
large enough,
where
![]() $q({h})=b_{\widetilde {u}}({h}){h}^{-{1}/{(3-\varepsilon ')}}.$
This implies that
$q({h})=b_{\widetilde {u}}({h}){h}^{-{1}/{(3-\varepsilon ')}}.$
This implies that
![]() $q({h})\rightarrow 0$
as
$q({h})\rightarrow 0$
as
![]() ${h}\rightarrow \infty $
, contradicting
${h}\rightarrow \infty $
, contradicting
![]() $u=O(|x|^{3-\varepsilon })$
at infinity, when
$u=O(|x|^{3-\varepsilon })$
at infinity, when
![]() $\varepsilon '<\varepsilon $
. Thus, Step 2 is finished.
$\varepsilon '<\varepsilon $
. Thus, Step 2 is finished.
Combining Steps 1 and 2, we have shown that there is a large constant C such that
![]() $C^{-1}x_n^{2}\leq \widetilde {u}(0, x_n) \leq Cx_n^{2}$
at infinity. This yields
$C^{-1}x_n^{2}\leq \widetilde {u}(0, x_n) \leq Cx_n^{2}$
at infinity. This yields
![]() $C^{-1}h^{{1}/{2}}\leq d_n\leq Ch^{{1}/{2}}$
. Then by the boundary condition, the convexity of
$C^{-1}h^{{1}/{2}}\leq d_n\leq Ch^{{1}/{2}}$
. Then by the boundary condition, the convexity of
![]() $S_h$
and Lemma 2.1,
$S_h$
and Lemma 2.1,
which completes the proof of Theorem 1.1.
Proof of Theorem 1.2.
We deduce Theorem 1.2 from Theorem 1.1.
We claim that u is uniformly convex in
![]() $\overline {B_{10R}^+}\backslash B_{3R}^+$
. Otherwise,
$\overline {B_{10R}^+}\backslash B_{3R}^+$
. Otherwise,
![]() $D_{ee}u(x)=0$
at some interior point x for some unit vector e. This gives a contradiction because
$D_{ee}u(x)=0$
at some interior point x for some unit vector e. This gives a contradiction because
![]() $u\in C^2$
and u solves (1.8).
$u\in C^2$
and u solves (1.8).
Now we construct a solution of (1.4). Near
![]() $\{x_n=0\}$
, by the uniform convexity of u, we can extend u to
$\{x_n=0\}$
, by the uniform convexity of u, we can extend u to
![]() $\widetilde {u}$
defined in
$\widetilde {u}$
defined in
![]() $(B_{10R} \backslash \overline {B_{2R}})\cap \{x_n>-\varepsilon \}$
for
$(B_{10R} \backslash \overline {B_{2R}})\cap \{x_n>-\varepsilon \}$
for
![]() $\varepsilon $
small such that
$\varepsilon $
small such that
![]() $\widetilde {u}$
is uniformly convex and
$\widetilde {u}$
is uniformly convex and
![]() $\widetilde {u}=u$
in
$\widetilde {u}=u$
in
![]() $\overline {B_{10R}^+}\backslash B_{2R}^+$
. By the extension theorem for uniformly convex functions on nonconvex domains (see [Reference Yan10, Theorem 3.2]), we can obtain a uniformly convex
$\overline {B_{10R}^+}\backslash B_{2R}^+$
. By the extension theorem for uniformly convex functions on nonconvex domains (see [Reference Yan10, Theorem 3.2]), we can obtain a uniformly convex
![]() $\widetilde {\widetilde {u}}$
which is equal to u in
$\widetilde {\widetilde {u}}$
which is equal to u in
![]() $\overline {B_{10R}^+}\backslash B_{3R}^+$
. We extend
$\overline {B_{10R}^+}\backslash B_{3R}^+$
. We extend
![]() $\widetilde {\widetilde {u}}$
to the half space and equal to u in
$\widetilde {\widetilde {u}}$
to the half space and equal to u in
![]() $\overline {\mathbb {R}^n_+}\backslash B_{10R}^+$
. Then,
$\overline {\mathbb {R}^n_+}\backslash B_{10R}^+$
. Then,
![]() $\widetilde {\widetilde {u}}=O(|x|^{3-\varepsilon })$
at infinity for some
$\widetilde {\widetilde {u}}=O(|x|^{3-\varepsilon })$
at infinity for some
![]() $\varepsilon>0$
, and
$\varepsilon>0$
, and
![]() $\widetilde {\widetilde {u}}$
solves (1.4) with proper f and
$\widetilde {\widetilde {u}}$
solves (1.4) with proper f and
![]() $\varphi $
satisfying (1.3), (1.5) and (1.6), respectively, where
$\varphi $
satisfying (1.3), (1.5) and (1.6), respectively, where
![]() $\mu $
and
$\mu $
and
![]() $\lambda $
will be chosen smaller if necessary. Applying Theorem 1.1 to
$\lambda $
will be chosen smaller if necessary. Applying Theorem 1.1 to
![]() $\widetilde {\widetilde {u}}$
shows that
$\widetilde {\widetilde {u}}$
shows that
![]() $\widetilde {\widetilde {u}}$
enjoys the quadratic growth condition and so does u.
$\widetilde {\widetilde {u}}$
enjoys the quadratic growth condition and so does u.
4 An application
We first state a result on the asymptotic behaviour at infinity of convex viscosity solutions of Monge–Ampère equations in half spaces.
Theorem 4.1 [Reference Jia, Li and Li5].
Let
![]() $n\geq 2$
and
$n\geq 2$
and
![]() $u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.1) satisfying
$u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.1) satisfying
for some constants
![]() $0<\mu \leq \tfrac 12$
,
$0<\mu \leq \tfrac 12$
,
![]() $R_0>0$
, where
$R_0>0$
, where
![]() $f(x)$
satisfies (1.2) and (1.3). Then there exist some symmetric positive definite matrix A with
$f(x)$
satisfies (1.2) and (1.3). Then there exist some symmetric positive definite matrix A with
![]() $\det A=1$
, vector
$\det A=1$
, vector
![]() $b\in \mathbb {R}^n$
and constant
$b\in \mathbb {R}^n$
and constant
![]() $c\in \mathbb {R}$
such that
$c\in \mathbb {R}$
such that
where
![]() $x=(x',x_n)$
, and C and
$x=(x',x_n)$
, and C and
![]() $R\geq R_0$
depend only on n,
$R\geq R_0$
depend only on n,
![]() $\mu $
,
$\mu $
,
![]() $R_0$
and the diameter of the domain
$R_0$
and the diameter of the domain
![]() $\Omega _0$
. Moreover,
$\Omega _0$
. Moreover,
![]() $u\in C^{\infty } (\overline {\mathbb {R}^n_+}\backslash \Omega _0)$
and for any
$u\in C^{\infty } (\overline {\mathbb {R}^n_+}\backslash \Omega _0)$
and for any
![]() $k\geq 1$
,
$k\geq 1$
,
where C depends only on n,
![]() $\mu $
, k,
$\mu $
, k,
![]() $R_0$
and the diameter of the domain
$R_0$
and the diameter of the domain
![]() $\Omega _0$
.
$\Omega _0$
.
By Theorem 1.2, the quadratic growth condition (4.1) in Theorem 4.1 can be deduced from
![]() $u=O(|x|^{3-\varepsilon })$
at infinity for some
$u=O(|x|^{3-\varepsilon })$
at infinity for some
![]() $\varepsilon>0$
. Therefore, we can obtain an improved theorem as follows.
$\varepsilon>0$
. Therefore, we can obtain an improved theorem as follows.
Theorem 4.2. Let
![]() $n\geq 2$
,
$n\geq 2$
,
![]() $u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.1) with f satisfying (1.2) and (1.3). If
$u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.1) with f satisfying (1.2) and (1.3). If
![]() $u=O(|x|^{3-\varepsilon })$
at infinity for some
$u=O(|x|^{3-\varepsilon })$
at infinity for some
![]() $\varepsilon>0$
, then the conclusions of Theorem 4.1 hold.
$\varepsilon>0$
, then the conclusions of Theorem 4.1 hold.
Remark 4.3. Theorem 4.2 also holds over exterior domains in half spaces. In fact, the asymptotic behaviour at infinity of solutions of Monge–Ampère equations only depends on the datum at infinity, for example, the right-hand term of the equation, boundary values and the growth condition. Additionally, by Theorem 1.2, Theorem 4.2 also holds for
![]() $u\in C^2$
over exterior domains in half spaces if
$u\in C^2$
over exterior domains in half spaces if
![]() $u=O(|x|^{3-\varepsilon })$
at infinity with
$u=O(|x|^{3-\varepsilon })$
at infinity with
![]() $\varepsilon>0$
.
$\varepsilon>0$
.
As a corollary of Theorem 4.2, it is easy to deduce the following Liouville theorem, which improves the result in [Reference Mooney7].
Theorem 4.4. Let
![]() $n\geq 2$
and
$n\geq 2$
and
![]() $u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.1), where
$u\in C^0(\overline {\mathbb {R}^n_+})$
be a convex viscosity solution of (1.1), where
![]() $f(x)\equiv 1$
. If
$f(x)\equiv 1$
. If
![]() $u=O(|x|^{3-\varepsilon })$
at infinity for some
$u=O(|x|^{3-\varepsilon })$
at infinity for some
![]() $\varepsilon>0$
, then u must be a quadratic polynomial.
$\varepsilon>0$
, then u must be a quadratic polynomial.
Acknowledgement
We are grateful to the anonymous referee for valuable comments and suggestions.