No CrossRef data available.
Article contents
Periodic solutions of four-order degenerate differential equations with finite delay in vector-valued function spaces
Published online by Cambridge University Press: 14 September 2023
Abstract
In this paper, we mainly investigate the well-posedness of the four-order degenerate differential equation ($P_4$ ): $(Mu)''''(t) + \alpha (Lu)'''(t) + (Lu)''(t)$
): $(Mu)''''(t) + \alpha (Lu)'''(t) + (Lu)''(t)$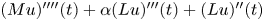 $=\beta Au(t) + \gamma Bu'(t) + Gu'_t + Fu_t + f(t),\,( t\in [0,\,2\pi ])$
$=\beta Au(t) + \gamma Bu'(t) + Gu'_t + Fu_t + f(t),\,( t\in [0,\,2\pi ])$ in periodic Lebesgue–Bochner spaces $L^p(\mathbb {T}; X)$
in periodic Lebesgue–Bochner spaces $L^p(\mathbb {T}; X)$ and periodic Besov spaces $B_{p,q}^s\;(\mathbb {T}; X)$
and periodic Besov spaces $B_{p,q}^s\;(\mathbb {T}; X)$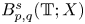 , where $A$
, where $A$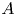 , $B$
, $B$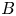 , $L$
, $L$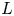 and $M$
and $M$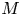 are closed linear operators on a Banach space $X$
are closed linear operators on a Banach space $X$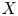 such that $D(A)\cap D(B)\subset D(M)\cap D(L)$
such that $D(A)\cap D(B)\subset D(M)\cap D(L)$ and $\alpha,\,\beta,\,\gamma \in \mathbb {C}$
and $\alpha,\,\beta,\,\gamma \in \mathbb {C}$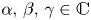 , $G$
, $G$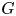 and $F$
and $F$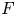 are bounded linear operators from $L^p([-2\pi,\,0];X)$
are bounded linear operators from $L^p([-2\pi,\,0];X)$ (respectively $B_{p,q}^s([-2\pi,\,0];X)$
(respectively $B_{p,q}^s([-2\pi,\,0];X)$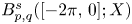 ) into $X$
) into $X$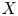 , $u_t(\cdot ) = u(t+\cdot )$
, $u_t(\cdot ) = u(t+\cdot )$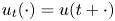 and $u'_t(\cdot ) = u'(t+\cdot )$
and $u'_t(\cdot ) = u'(t+\cdot )$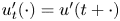 are defined on $[-2\pi,\,0]$
are defined on $[-2\pi,\,0]$ for $t\in [0,\, 2\pi ]$
for $t\in [0,\, 2\pi ]$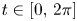 . We completely characterize the well-posedness of ($P_4$
. We completely characterize the well-posedness of ($P_4$ ) in the above two function spaces by using known operator-valued Fourier multiplier theorems.
) in the above two function spaces by using known operator-valued Fourier multiplier theorems.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh



