Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-20T07:40:16.306Z Has data issue: false hasContentIssue false
  • English
  • Français

TRACTARIAN LOGICISM: OPERATIONS, NUMBERS, INDUCTION

Published online by Cambridge University Press:  21 October 2020

GREGORY LANDINI
GREGORY LANDINI*
Affiliation:
UNIVERSITY OF IOWAIOWA CITY, IA52241, USAE-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In his Tractatus, Wittgenstein maintained that arithmetic consists of equations arrived at by the practice of calculating outcomes of operations 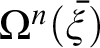$\Omega ^{n}(\bar {\xi })$ defined with the help of numeral exponents. Since 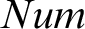$Num$ (x) and quantification over numbers seem ill-formed, Ramsey wrote that the approach is faced with “insuperable difficulties.” This paper takes Wittgenstein to have assumed that his audience would have an understanding of the implicit general rules governing his operations. By employing the Tractarian logicist interpretation that the N-operator 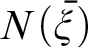$N(\bar {\xi })$ and recursively defined arithmetic operators 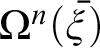$\Omega ^{n}(\bar {\xi })$ are not different in kind, we can address Ramsey’s problem. Moreover, we can take important steps toward better understanding how Wittgenstein might have imagined emulating proof by mathematical induction.

Keywords

WittgensteinRamseyRussellmathematical inductionN-operatorTractarian operations
Type
Research Article
Information
The Review of Symbolic Logic , Volume 14 , Issue 4 , December 2021 , pp. 973 - 1010
Check for updates
Copyright
© Association for Symbolic Logic 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Anscombe, G. E. M. (1959). An Introduction to Wittgensteins Tractatus. New York: Harper and Row.Google Scholar
Cuter, J. V. G. (2005). Operations and truth-operations in the tractatus. Philosophical Investigations, 28, 63–75.CrossRefGoogle Scholar
Fitch, F. (1974). Elements of Combinatory Logic. New Haven, CT: Yale University Press.Google Scholar
Floyd, J. (2001). Number and ascriptions of number in Wittgenstein’s tractatus. In Floyd, J., and Sheih, S., editors. Future Pasts: The Analytic Tradition in Twentieth Century Philosophy. Oxford: Oxford University Press.CrossRefGoogle Scholar
Fogelin, R. (1982). Wittgenstein’s operator N. Analysis, 42, 124–128.CrossRefGoogle Scholar
Fogelin, R. (1987). Wittgenstein, second edition. London: Routledge & Kegan Paul.Google Scholar
Frascolla, P. (1994). Wittgenstein’s Philosophy of Mathematics . New York: Routledge.Google Scholar
Frascolla, P. (1997). The tractarian system of arithmetic. Synthese , 112, 353–378.CrossRefGoogle Scholar
Geach, P. T. (1981). Wittgenstein’s operator N. Analysis , 41, 168–171.CrossRefGoogle Scholar
Geach, P. T. (1982). More on Wittgenstein’s operator N. Analysis, 42, 127–128.CrossRefGoogle Scholar
Landini, G. (1996). The definability of the set of natural numbers in the 1925 Principia Mathematica . Journal of Philosophical Logic, 25, 597–615.CrossRefGoogle Scholar
Landini, G. (2007). Wittgenstein’s Apprenticeship with Russell. Cambridge: Cambridge University Press.CrossRefGoogle Scholar
Landini, G. (2013). Review of The Evolution of Principia Mathematica, ed. by Linsky B. (Cambridge, 2011). History and Philosophy of Logic, 34, 79–97.CrossRefGoogle Scholar
Landini, G. (2018). On the curious calculi of Wittgenstein and spencer-brown. Journal of the History of Analytic Philosophy, 6, 1–28.Google Scholar
Lowe, V., & Schneewind, J. B. (1990). Alfred North Whitehead: The Man and His Work, Vol. II. Baltimore: Johns Hopkins Press, 1910–1947.Google Scholar
Marion, M., & Mitsuhiro, O. (2018). Wittgenstein, Goodstein, and the origin of the uniqueness rule for primitive recursive arithmetic. In Stern, D., editor. Wittgenstein in the 1930s: Between the Tractatus and the Investigations. Cambridge: Cambridge University Press, 253–271.CrossRefGoogle Scholar
Mendelson, E. (2010). Introduction to Mathematical Logic, fifth edition. London: Chapman & Hall.Google Scholar
Moore, G. E. (2016) In Stern, D. G., Rogers, B., Citron, G., editors. Wittgenstein: Lectures, Cambridge 1930-1933: From the Notes of G. E. Moore. Cambridge: Cambridge University Press.Google Scholar
Potter, M. (2000). Reason's Nearest Kin:Philosophies of Mathematics from Kant to Carnap, Oxford: Oxford University Press.Google Scholar
Ramsey, F. P. (1931). The foundations of mathematics, in Braithwaite, R. B., editor. The Foundations of Mathematics and Other Essays by Frank Plumpton Ramsey. New York: Harcourt Brace and Co., p. 1931.Google Scholar
Rodgers, B., & Wehmeier, K. (2012). Tractarian first order logic: Identity and the N-operator. Review of Symbolic Logic, 5, 538–573.CrossRefGoogle Scholar
Russell, B. (1968). The Autobiography of Bertrand Russell , Vol. II. Boston, MA: Little Brown & Co., pp. 1914–1916.Google Scholar
Russell, B. (1990). Unpublished Correspondence between Russell and Wittgenstein. Brian McGuinness and G. H. von Wright. Russell: The Journal of the Bertrand Russell Archives, 10, 107–108.Google Scholar
Soames, S. (1983). Generality, truth-functions, and experssive capacity in the tractatus. The Philosophical Review, 92, 573–589.CrossRefGoogle Scholar
Soames, S. (2017). The Analytic Tradition in Philosophy: Volume 2, A New Vision. Princeton, NJ: Princeton University Press.CrossRefGoogle Scholar
Waismann, F. (1931). In McGuinness, B., editor (1979). Wittgenstein and the Vienna Circle. Oxford: Blackwell.Google Scholar
Wehmeier, K. (2004). Wittgenstein’s predicate logic. Notre Dame Journal of Formal Logic, 45, 1–11.CrossRefGoogle Scholar
Whitehead, A. N. (1929). Process and Reality: An Essay in Cosmology. New York: Harper & Brothers.Google Scholar
Wittgenstein, L. (1971). In McGuinnesss, B. F., Nybert, T., and von Wright, G. H., with trans. by Pears, F. F., and McGuinness, B. M., Prototractatus: An Early Version of Tractatus Logio-Philosophicus. London: Routledge & Kegan Paul.Google Scholar
Wittgenstein, L. (1973). Letter of Sept. 1923. In von Wright, G. G., editor. Ludwig Wittgenstein: Letters to C. K. Ogden with and Appendix of Letters by Frank Plumpton Ramsey. Oxford: Basil Blackwell.Google Scholar
Wittgenstein, L. (PB). Philosophical Remarks. Oxford, UK: Basil Blackwell, 1975.Google Scholar
Wittgenstein, L. (1979). Notebooks 1914–1916, eds. von Wright, G. H. & Anscombe, G. E.M., Chicago: University of Chicago Press.Google Scholar