Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T17:07:58.342Z Has data issue: false hasContentIssue false
  • English
  • Français

The group of homotopy self-equivalences of non-simply-connected spaces using Postnikov decompositions*

Published online by Cambridge University Press:  14 November 2011

J. W. Rutter
J. W. Rutter
Affiliation:
Institut des Hautes Études Scientifiques, 91440 Bures sur Yvette, Franceand Department of Pure Mathematics, Liverpool University, Liverpool L69 3BX, England
Get access Rights & Permissions [Opens in a new window]

Synopsis

We give here a group extension sequence for calculating, for a non-simply-connected space X, the group of self-homotopy-equivalence classes which induce the identity automorphism of the fundamental group, that is the kernel of the representation 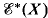→ aut (π1(X)). This group extension sequence gives 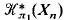in terms of 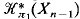, where Xn is the n-th stage of a Postnikov decomposition. As special cases, we calculate for non-simply-connected spaces having at most two non-trivial homotopy groups, in dimensions 1 and n, as the unit group of a semigroup structure on ; and we calculate up to extension for non-simply-connected spaces having at most three non-trivial homotopy groups. The group is, for nice spaces, isomorphic to the groups 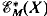and 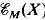of self-homotopy-equivalence classes of X in the categories top*M and top M, respectively, where X→M = K1(π1(X)) is a top fibration which determines an isomorphism of the fundamental group; and our results are obtained initially in topM.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 120 , Issue 1-2 , 1992 , pp. 47 - 60
Copyright
Copyright © Royal Society of Edinburgh 1992

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Alsoufl, A. A.. Some problems in the homotopy theory of spaces under A and B (Ph.D. Thesis, University of Liverpool, 1982).Google Scholar
2Baues, H. J.. Algebraic homotopy (Cambridge: Cambridge University Press, 1989).CrossRefGoogle Scholar
3Didierjean, G.. Homotopie de l'espace des equivalences fibrees. Ann. lnst. Fourier (Grenoble) 35 (1985), 33–47.CrossRefGoogle Scholar
4James, I. M. and Thomas, E.. An approach to the enumeration problem for non-stable vector bundles. Math. Mech. 14 (1965), 485–506.Google Scholar
5McClendon, J. F.. Obstruction theory in fibre spaces. Math. Z. 120 (1971), 1–17.CrossRefGoogle Scholar
6McClendon, J. F.. Relative principal fibrations. Bol. Soc. Mat. Mex. 19 (1974), 38–43.Google Scholar
7Nomura, Y.. Homotopy equivalences in a principal fibre space. Math. Z. 92 (1966), 380—388.CrossRefGoogle Scholar
8Robinson, C. A.. Moore-Postnikov systems for non-simple fibrations. Illinois J. Math. 16 (1972), 234–242.CrossRefGoogle Scholar
9Rutter, J. W.. A homotopy classification of maps into an induced fibre space. Topology 6 (1967), 379–403.CrossRefGoogle Scholar
10Rutter, J. W.. Self-equivalences and principal morphisms. Proc. London Math. Soc. 20 (1970), 644–658.CrossRefGoogle Scholar
11Rutter, J. W.. Groups of self-homotopy equivalences of induced spaces. Comment. Math. Helv. 45 (1970), 236–255.CrossRefGoogle Scholar
12Rutter, J. W.. Maps and equivalences into equalizing fibrations and from co-equalizing cofibrations. Math. Z. 122 (1971), 125–141.CrossRefGoogle Scholar
13Rutter, J. W.. The group of homotopy self-equivalence classes of CW complexes. Math. Proc. Cambridge Philos. Soc. 93 (1983), 275–293.CrossRefGoogle Scholar
14Rutter, J. W.. The group of homotopy self-equivalence classes using an homology decomposition. Math. Proc. Cambridge Philos. Soc. 103 (1988), 305–315.CrossRefGoogle Scholar
15Rutter, J. W.. Whitney-sums (fibre-joins) in over space theory and obstruction theory for cohomology with local coefficients. Proc. Roy. Soc. Edinburgh Sect. A 115 (1990), 359–365.CrossRefGoogle Scholar
16Schellenberg, B.. The group of homotopy self-equivalences of some compact CW-complexes. Math. Ann. 200 (1973), 253–266.CrossRefGoogle Scholar
17Shih, W.. On the group &[X] of homotopy equivalence maps. Bull. Amer. Math. Soc. 70 (1964), 361–365.CrossRefGoogle Scholar
18Tsukiyama, K.. Note on self-maps inducing the identity automorphism of homotopy groups. Hiroshima Math. J. 5 (1975), 215–222.CrossRefGoogle Scholar
19Tsukiyama, K.. Note on self-homotopy-equivalences of the twisted principal fibrations. Mem. Fac. Ed. Shimane Univ. Natur. Sci. 11 (1977), 1–8.Google Scholar
20Tsukiyama, K.. Self-homotopy equivalences of a space with two non-vanishing homotopy groups. Proc. Amer. Math. Soc. 79 (1980), 134–138.CrossRefGoogle Scholar