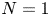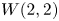1. Introduction
We denote by $\mathbb {Z}, \mathbb {N}, \mathbb {Z}_+, \mathbb {C}$![]() and $\mathbb {C}^*$
and $\mathbb {C}^*$![]() the sets of all integers, non-negative integers, positive integers, complex numbers and nonzero complex numbers, respectively. All vector spaces and algebras in this paper are over $\mathbb {C}$
the sets of all integers, non-negative integers, positive integers, complex numbers and nonzero complex numbers, respectively. All vector spaces and algebras in this paper are over $\mathbb {C}$![]() . Throughout the paper, we always assume that all vector superspaces (resp. superalgebras, supermodules) are defined over $\mathbb {C}$
. Throughout the paper, we always assume that all vector superspaces (resp. superalgebras, supermodules) are defined over $\mathbb {C}$![]() , and sometimes simply call them spaces (resp. algebras, modules). We use $U(L)$
, and sometimes simply call them spaces (resp. algebras, modules). We use $U(L)$![]() to denote the universal enveloping algebra for a Lie superalgebra $L$
to denote the universal enveloping algebra for a Lie superalgebra $L$![]() .
.
Superconformal algebras may be viewed as natural super-extensions of the Virasoro algebra and have been playing a fundamental role in string theory and conformal field theory. In [Reference Kac16], Kac classified all physical superconformal algebras: namely, the $N = 0$![]() (the Virasoro algebra Vir), $N = 1$
(the Virasoro algebra Vir), $N = 1$![]() (the super Virasoro algebras), $N = 2, 3, 4$
(the super Virasoro algebras), $N = 2, 3, 4$![]() superconformal algebras, the superalgebra of all vector fields on the $N=2$
superconformal algebras, the superalgebra of all vector fields on the $N=2$![]() supercircle, and a new superalgebra $CK(6)$
supercircle, and a new superalgebra $CK(6)$![]() . Representation theory for superconformal algebras has been intensively studied. It is a challenging problem to give complete classifications of simple Harish–Chandra modules for superconformal algebras. Based on the classification of simple jet modules introduced by Billig in [Reference Billig4] (see also [Reference Rao25]), Billig and Futorny gave a complete classification of simple Harish–Chandra modules for the Lie algebra of vector fields on a torus with the so-called $A$
. Representation theory for superconformal algebras has been intensively studied. It is a challenging problem to give complete classifications of simple Harish–Chandra modules for superconformal algebras. Based on the classification of simple jet modules introduced by Billig in [Reference Billig4] (see also [Reference Rao25]), Billig and Futorny gave a complete classification of simple Harish–Chandra modules for the Lie algebra of vector fields on a torus with the so-called $A$![]() cover theory in [Reference Billig and Futorny5]. Recently, with the study of jet modules, the classifications of such modules were completed for many Lie superalgebras: the $N=1$
cover theory in [Reference Billig and Futorny5]. Recently, with the study of jet modules, the classifications of such modules were completed for many Lie superalgebras: the $N=1$![]() Ramond algebra in [Reference Cai, Liu and Lü8], the Witt superalgebra in [Reference Billig, Futorny, Iohara and Kashuba6, Reference Xue and Lü30], the affine-Virasoro superalgebra in [Reference Cai, Lü and Wang10, Reference He, Liu and Wang15], etc. The above Lie superalgebras are all $\mathbb {Z}$
Ramond algebra in [Reference Cai, Liu and Lü8], the Witt superalgebra in [Reference Billig, Futorny, Iohara and Kashuba6, Reference Xue and Lü30], the affine-Virasoro superalgebra in [Reference Cai, Lü and Wang10, Reference He, Liu and Wang15], etc. The above Lie superalgebras are all $\mathbb {Z}$![]() -graded. Compared with $\mathbb {Z}$
-graded. Compared with $\mathbb {Z}$![]() -graded Lie superalgebras, it is more complicated to classify all simple jet modules for $1/2\mathbb {Z}$
-graded Lie superalgebras, it is more complicated to classify all simple jet modules for $1/2\mathbb {Z}$![]() -graded Lie superalgebras (see [Reference Cai and Lü9] for the case of $N=1$
-graded Lie superalgebras (see [Reference Cai and Lü9] for the case of $N=1$![]() Neveu–Schwarz algebra). Up to now, there are few papers to classify all simple Harish–Chandra modules over $1/2\mathbb {Z}$
Neveu–Schwarz algebra). Up to now, there are few papers to classify all simple Harish–Chandra modules over $1/2\mathbb {Z}$![]() -graded Lie superalgebras.
-graded Lie superalgebras.
The Ovsienko–Roger Lie algebra $\mathfrak L_\lambda :={\rm Vir}\ltimes \mathcal {F}_{\lambda }$![]() was introduced in [Reference Ovsienko and Roger24] to study the extensions of the Virasoro algebra by the density module $\mathcal {F}_{\lambda }$
was introduced in [Reference Ovsienko and Roger24] to study the extensions of the Virasoro algebra by the density module $\mathcal {F}_{\lambda }$![]() . In particular, the algebra $\mathfrak L_{0}$
. In particular, the algebra $\mathfrak L_{0}$![]() , which is called the twisted Heisenberg–Virasoro algebra, plays an important role in moduli spaces of curves [Reference Arbarello, De Concini, Kac and Procesi1]. The algebra $\mathfrak L_{-1}$
, which is called the twisted Heisenberg–Virasoro algebra, plays an important role in moduli spaces of curves [Reference Arbarello, De Concini, Kac and Procesi1]. The algebra $\mathfrak L_{-1}$![]() , named $W(2,2)$
, named $W(2,2)$![]() , is better known in the context of vertex operator algebras [Reference Zhang and Dong31] and BMS/GCA correspondence [Reference Bagchi2]. Moreover, with the study of the Ovsienko–Roger Lie algebra $\mathfrak L_\lambda$
, is better known in the context of vertex operator algebras [Reference Zhang and Dong31] and BMS/GCA correspondence [Reference Bagchi2]. Moreover, with the study of the Ovsienko–Roger Lie algebra $\mathfrak L_\lambda$![]() , Harish–Chandra modules for many Lie algebras related to the Virasoro algebra have been classified (see [Reference Liu18, Reference Liu, Pei and Xia20]).
, Harish–Chandra modules for many Lie algebras related to the Virasoro algebra have been classified (see [Reference Liu18, Reference Liu, Pei and Xia20]).
Motivated by the above researches, we introduce the Ovsienko–Roger Lie superalgebra $\mathfrak L(\lambda, \epsilon ):={\rm Vir}\ltimes \mathcal {F}_{\lambda }, \epsilon =0, 1/2$![]() , where $\mathcal {F}_{\lambda }$
, where $\mathcal {F}_{\lambda }$![]() is viewed as the odd part of ${\mathfrak L}(\lambda, \epsilon )$
is viewed as the odd part of ${\mathfrak L}(\lambda, \epsilon )$![]() . In the special case, ${\mathfrak L}(1/2, \epsilon )$
. In the special case, ${\mathfrak L}(1/2, \epsilon )$![]() , named Kuper algebra, is connected with the super Camassa–Holm-type systems (see [Reference Ge13]). Meanwhile, ${\mathfrak L}(-1/2,\epsilon )$
, named Kuper algebra, is connected with the super Camassa–Holm-type systems (see [Reference Ge13]). Meanwhile, ${\mathfrak L}(-1/2,\epsilon )$![]() is a subalgebra or quotient algebra of many Lie superalgebras (see § 5).
is a subalgebra or quotient algebra of many Lie superalgebras (see § 5).
It is well known that the annihilation operator for cuspidal modules over the Virasoro algebra in [Reference Billig and Futorny5] (lemma 2.2 below) plays an important role in the classification of simple cuspidal modules. With such annihilation operators for cuspidal modules over the super Virasoro algebras in [Reference Cai, Liu and Lü8, Reference Cai and Lü9] (lemma 3.1 below), we get an annihilation operator for cuspidal modules over the Ovsienko–Roger superalgebra (lemma 3.2 below) and give a new method to classify all simple Harish–Chandra modules for the $\mathbb {Z}$![]() -graded and $1/2\mathbb {Z}$
-graded and $1/2\mathbb {Z}$![]() -graded Ovsienko-Roger superalgebras (theorem 3.4 below). Moreover, this classification can be applied to studying various classes of Lie superalgebras uniformly (see § 5). Note that we just do our research for $\lambda =-1/2$
-graded Ovsienko-Roger superalgebras (theorem 3.4 below). Moreover, this classification can be applied to studying various classes of Lie superalgebras uniformly (see § 5). Note that we just do our research for $\lambda =-1/2$![]() in the whole paper although our calculations and proofs are all suitable for any $\lambda \in \mathbb {C}$
in the whole paper although our calculations and proofs are all suitable for any $\lambda \in \mathbb {C}$![]() .
.
The paper is organized as follows. In § 2, we collect some basic results for our study. Simple cuspidal modules are classified in § 3. In § 4, we classify all simple Harish–Chandra modules for the Ovsienko–Roger superalgebra. Finally, using this classification, we can classify all simple Harish–Chandra modules over some related Lie superalgebras, including the $N=1$![]() BMS$_3$
BMS$_3$![]() superalgebra, the super $W(2,2)$
superalgebra, the super $W(2,2)$![]() algebra, etc., in § 5.
algebra, etc., in § 5.
2. Preliminaries
In this section, we collect some basic definitions and results for our study.
By definition, as a vector space, the Virasoro algebra ${\rm Vir}$![]() has a basis $\{L_m, C \mid m\in \mathbb {Z}\}$
has a basis $\{L_m, C \mid m\in \mathbb {Z}\}$![]() , subject to the following relations:
, subject to the following relations:
For any ${\rm Vir}$![]() -module $V$
-module $V$![]() and $\lambda \in \mathbb {C}$
and $\lambda \in \mathbb {C}$![]() , set $V^{\lambda }:=\bigl \{v\in V\bigm |L_0v=\lambda v\bigr \}$
, set $V^{\lambda }:=\bigl \{v\in V\bigm |L_0v=\lambda v\bigr \}$![]() , which is generally called the weight space of $V$
, which is generally called the weight space of $V$![]() corresponding to the weight $\lambda$
corresponding to the weight $\lambda$![]() . A ${\rm Vir}$
. A ${\rm Vir}$![]() -module $V$
-module $V$![]() is called a weight module if $V$
is called a weight module if $V$![]() is the sum of all its weight spaces.
is the sum of all its weight spaces.
For a weight module $V$![]() , we define
, we define
Obviously, if $V$![]() is a weight ${\rm Vir}$
is a weight ${\rm Vir}$![]() -module, then there exists $\lambda \in \mathbb {C}$
-module, then there exists $\lambda \in \mathbb {C}$![]() such that ${\rm Supp}(V)\subset \lambda +\mathbb {Z}$
such that ${\rm Supp}(V)\subset \lambda +\mathbb {Z}$![]() . So $V=\sum _{i\in \mathbb {Z}}V_i$
. So $V=\sum _{i\in \mathbb {Z}}V_i$![]() is $\mathbb {Z}$
is $\mathbb {Z}$![]() -graded, where $V_i:=V^{\lambda +i}$
-graded, where $V_i:=V^{\lambda +i}$![]() . A weight ${\rm Vir}$
. A weight ${\rm Vir}$![]() -module $V=\sum V_i$
-module $V=\sum V_i$![]() is called Harish–Chandra if all $V_i$
is called Harish–Chandra if all $V_i$![]() are finite-dimensional. If, in addition, there exists a positive integer $p$
are finite-dimensional. If, in addition, there exists a positive integer $p$![]() such that
such that
the module $V$![]() is called cuspidal. A cuspidal module $V$
is called cuspidal. A cuspidal module $V$![]() with $p\le 1$
with $p\le 1$![]() is called a module of the intermediate series.
is called a module of the intermediate series.
A ${\rm Vir}$![]() -module $V$
-module $V$![]() is called a highest (resp. lowest) weight module, if there exists a nonzero $v \in V_{\lambda }$
is called a highest (resp. lowest) weight module, if there exists a nonzero $v \in V_{\lambda }$![]() such that
such that
(1) $V$
 is generated by $v$
is generated by $v$ as a ${\rm Vir}$
as a ${\rm Vir}$ -module with $L_0v=hv$
-module with $L_0v=hv$ and $Cv=cv$
and $Cv=cv$ for some $h,c\in \mathbb {C}$
for some $h,c\in \mathbb {C}$ ;
;(2) ${{\rm Vir}}_+v=0$
 (resp. ${{\rm Vir}}_- v=0$
(resp. ${{\rm Vir}}_- v=0$ ), where ${{\rm Vir}}_+=\sum _{i>0}{{\rm Vir}}_i$
), where ${{\rm Vir}}_+=\sum _{i>0}{{\rm Vir}}_i$ , ${{\rm Vir}}_-=\sum _{i<0}{{\rm Vir}}_i$
, ${{\rm Vir}}_-=\sum _{i<0}{{\rm Vir}}_i$ .
.
Clearly highest or lowest weight modules are Harish–Chandra modules. All simple Harish–Chandra modules over the Virasoro algebra were classified in [Reference Mathieu23].
Theorem 2.1 [Reference Mathieu23]
Let $V$![]() be a simple Harish–Chandra modules over the Virasoro algebra ${\rm Vir}$
be a simple Harish–Chandra modules over the Virasoro algebra ${\rm Vir}$![]() . Then $V$
. Then $V$![]() is a highest weight module, lowest weight module or a module of the intermediate series.
is a highest weight module, lowest weight module or a module of the intermediate series.
It is well known that the intermediate series ${\rm Vir}$![]() -module is
-module is
where $a, b\in \mathbb {C}$![]() . ${\mathcal {A}}_{a,\; b}$
. ${\mathcal {A}}_{a,\; b}$![]() is simple if and only if $a\not \in \mathbb {Z}$
is simple if and only if $a\not \in \mathbb {Z}$![]() or $b\ne 0, 1$
or $b\ne 0, 1$![]() . As usual, we use $\mathcal {A}_{a,\; b}'$
. As usual, we use $\mathcal {A}_{a,\; b}'$![]() to denote the irreducible sub-quotient module of ${\mathcal {A}}_{a,\; b}$
to denote the irreducible sub-quotient module of ${\mathcal {A}}_{a,\; b}$![]() (see [Reference Kaplansky and Santharoubane17]). If $a\in \mathbb {Z}$
(see [Reference Kaplansky and Santharoubane17]). If $a\in \mathbb {Z}$![]() , then ${\mathcal {A}}_{a,\; b}\cong {\mathcal {A}}_{0,\; b}$
, then ${\mathcal {A}}_{a,\; b}\cong {\mathcal {A}}_{0,\; b}$![]() . So we always suppose that $a\notin \mathbb {Z}$
. So we always suppose that $a\notin \mathbb {Z}$![]() or $a=0$
or $a=0$![]() in ${\mathcal {A}}_{a,\; b}$
in ${\mathcal {A}}_{a,\; b}$![]() . As in [Reference Fuks12, Reference Ovsienko and Roger24], we set ${\mathcal {F}}_\lambda ={\mathcal {A}}_{0,\; \lambda }, \lambda \in \mathbb {C}$
. As in [Reference Fuks12, Reference Ovsienko and Roger24], we set ${\mathcal {F}}_\lambda ={\mathcal {A}}_{0,\; \lambda }, \lambda \in \mathbb {C}$![]() , which is called density module of the Virasoro algebra.
, which is called density module of the Virasoro algebra.
Motivated by [Reference Ovsienko and Roger24], for $\epsilon =0, 1/2$![]() , we can define the Ovsienko–Roger superalgebra ${\mathfrak L}(\epsilon ):={\rm Vir}\ltimes \mathcal {F}_{-1/2}$
, we can define the Ovsienko–Roger superalgebra ${\mathfrak L}(\epsilon ):={\rm Vir}\ltimes \mathcal {F}_{-1/2}$![]() . More precisely, ${\mathfrak L}(\epsilon )$
. More precisely, ${\mathfrak L}(\epsilon )$![]() has a basis $\{L_n, G_r,C| n\in \mathbb {Z}, r\in \mathbb {Z}+\epsilon \}$
has a basis $\{L_n, G_r,C| n\in \mathbb {Z}, r\in \mathbb {Z}+\epsilon \}$![]() with the brackets
with the brackets

Here we shall note that the odd part of ${\mathfrak L}(\epsilon )$![]() is spanned by $\{G_{n}\mid n\in \mathbb {Z}+\epsilon \}$
is spanned by $\{G_{n}\mid n\in \mathbb {Z}+\epsilon \}$![]() .
.
In the case of $\epsilon =0$![]() , ${\mathfrak L}(0)$
, ${\mathfrak L}(0)$![]() can be realized as the affine-Virasoro superalgebra $\mathbb {C} x\otimes \mathbb {C}[t, t^{-1}]\rtimes {\rm Vir}$
can be realized as the affine-Virasoro superalgebra $\mathbb {C} x\otimes \mathbb {C}[t, t^{-1}]\rtimes {\rm Vir}$![]() , where $\mathbb {C} x$
, where $\mathbb {C} x$![]() is the one-dimensional abelian Lie superalgebra.
is the one-dimensional abelian Lie superalgebra.
For the Ovsienko–Roger superalgebra, all simple Harish–Chandra modules over ${\mathfrak L}(0)$![]() were classified in [Reference Cai, Lü and Wang10]. For the case of $\epsilon =1/2$
were classified in [Reference Cai, Lü and Wang10]. For the case of $\epsilon =1/2$![]() , it is more complicated to classify such modules as in [Reference Cai and Lü9]. This paper gives a uniform new method to consider both cases of $\epsilon =0$
, it is more complicated to classify such modules as in [Reference Cai and Lü9]. This paper gives a uniform new method to consider both cases of $\epsilon =0$![]() and $\epsilon =1/2$
and $\epsilon =1/2$![]() . For convenience, we just write our research for the case of $\epsilon =1/2$
. For convenience, we just write our research for the case of $\epsilon =1/2$![]() . So from now on we denote by ${\mathfrak L}={\mathfrak L}(1/2)$
. So from now on we denote by ${\mathfrak L}={\mathfrak L}(1/2)$![]() for short. Clearly, $\mathfrak L$
for short. Clearly, $\mathfrak L$![]() is a $\frac {1}{2}\mathbb {Z}$
is a $\frac {1}{2}\mathbb {Z}$![]() -graded Lie superalgebra with ${\mathfrak L}_i=\mathbb {C} L_i\oplus \delta _{0, i}\mathbb {C} C, \forall i\in \mathbb {Z}$
-graded Lie superalgebra with ${\mathfrak L}_i=\mathbb {C} L_i\oplus \delta _{0, i}\mathbb {C} C, \forall i\in \mathbb {Z}$![]() and ${\mathfrak L}_{i+1/2}=\mathbb {C} G_{i+\frac 12}, \forall i\in \mathbb {Z}$
and ${\mathfrak L}_{i+1/2}=\mathbb {C} G_{i+\frac 12}, \forall i\in \mathbb {Z}$![]() . The subalgebra of $\mathfrak L$
. The subalgebra of $\mathfrak L$![]() spanned by $\{L_i, C| i\in \mathbb {Z}\}$
spanned by $\{L_i, C| i\in \mathbb {Z}\}$![]() is isomorphic to the Virasoro algebra ${\rm Vir}$
is isomorphic to the Virasoro algebra ${\rm Vir}$![]() . The following annihilation operator $\Omega _{k, s}^{(m)}$
. The following annihilation operator $\Omega _{k, s}^{(m)}$![]() plays an important role in the classification of simple cuspidal modules over the Virasoro algebra.
plays an important role in the classification of simple cuspidal modules over the Virasoro algebra.
Lemma 2.2 [Reference Billig and Futorny5, Corollary 3.7]
Let $\Omega _{k, s}^{(m)}=\sum _{i=0}^m(-1)^i\binom {m}{i}L_{k-i}L_{s+i}$![]() . For every $\ell \in \mathbb {Z}_+$
. For every $\ell \in \mathbb {Z}_+$![]() there exists $m\in \mathbb {Z}_+$
there exists $m\in \mathbb {Z}_+$![]() such that for all $k, s\in \mathbb {Z}$
such that for all $k, s\in \mathbb {Z}$![]() , $\Omega _{k,s}^{(m)}$
, $\Omega _{k,s}^{(m)}$![]() annihilate every cuspidal ${\rm Vir}$
annihilate every cuspidal ${\rm Vir}$![]() -module with a composition series of length $\ell$
-module with a composition series of length $\ell$![]() .
.
3. Simple cuspidal $\mathfrak L$ -module
-module
In this section, we shall consider cuspidal $\mathfrak L$![]() -modules. Note that $C$
-modules. Note that $C$![]() acts trivially on any cuspidal module by [Reference Kaplansky and Santharoubane17]. The following annihilation operator $\overline {\Omega }_{r, s}^{(m)}$
acts trivially on any cuspidal module by [Reference Kaplansky and Santharoubane17]. The following annihilation operator $\overline {\Omega }_{r, s}^{(m)}$![]() was given in [Reference Cai and Lü9] (also see [Reference Cai, Liu and Lü8]).
was given in [Reference Cai and Lü9] (also see [Reference Cai, Liu and Lü8]).
Lemma 3.1 [Reference Cai and Lü9]
Let $V$![]() be a cuspidal ${\mathfrak L}$
be a cuspidal ${\mathfrak L}$![]() -module. Then there exists $m\in \mathbb {Z}_+$
-module. Then there exists $m\in \mathbb {Z}_+$![]() such that for all $r\in \mathbb {Z}+\frac 12, s\in \mathbb {Z}$
such that for all $r\in \mathbb {Z}+\frac 12, s\in \mathbb {Z}$![]() , $\overline {\Omega }_{r, s}^{(m)}$
, $\overline {\Omega }_{r, s}^{(m)}$![]() annihilate $V,$
annihilate $V,$![]() where $\overline {\Omega }_{r, s}^{(m)}=\sum _{i=0}^m(-1)^i\binom {m}{i}G_{r-i}L_{s+i}$
where $\overline {\Omega }_{r, s}^{(m)}=\sum _{i=0}^m(-1)^i\binom {m}{i}G_{r-i}L_{s+i}$![]() .
.
By lemma 3.1, we can easily get the following annihilation operator $\underline {\Omega }_{r, s}^{(m)}$![]() on cuspidal modules over $\mathfrak L$
on cuspidal modules over $\mathfrak L$![]() .
.
Lemma 3.2 Let $V$![]() be a cuspidal ${\mathfrak L}$
be a cuspidal ${\mathfrak L}$![]() -module. Then there exists $m\in \mathbb {Z}_+$
-module. Then there exists $m\in \mathbb {Z}_+$![]() such that
such that
where $\underline {\Omega }_{r, s}^{(m)}:=\sum _{i=0}^m(-1)^i\binom {m}{i}G_{r-i}G_{s+i}$![]() for any $r, s\in \mathbb {Z}+\frac 12$
for any $r, s\in \mathbb {Z}+\frac 12$![]() .
.
Proof. For the cuspidal module $V$![]() , by lemma 3.1, there exists $m\in \mathbb {Z}_+$
, by lemma 3.1, there exists $m\in \mathbb {Z}_+$![]() such that for all $r\in \mathbb {Z}+\frac 12, s\in \mathbb {Z}$
such that for all $r\in \mathbb {Z}+\frac 12, s\in \mathbb {Z}$![]() , $\overline {\Omega }_{r, s}^{(m)}V=0$
, $\overline {\Omega }_{r, s}^{(m)}V=0$![]() , that is
, that is

By the action of $G_t$![]() on (3.2), we get
on (3.2), we get

Choosing $t=t_1, t_2, t_1\ne t_2$![]() in (3.3), we get the lemma.
in (3.3), we get the lemma.
Now we use lemma 3.2 to classify all simple cuspidal modules over $\mathfrak L$![]() without complicated calculations.
without complicated calculations.
Lemma 3.3 Let $V$![]() be a simple cuspidal ${\mathfrak L}$
be a simple cuspidal ${\mathfrak L}$![]() -module. Then there exists $N\in \mathbb {Z}_+$
-module. Then there exists $N\in \mathbb {Z}_+$![]() such that $\mathfrak L_{\bar 1}^NV=0$
such that $\mathfrak L_{\bar 1}^NV=0$![]() .
.
Proof. By lemma 3.2, we can get (3.1). Multiply both sides of (3.1) by $G_{s+1} G_{s+2}\cdots G_{s+m}$![]() , $G_{r-j+1}\cdots G_{r-1}G_rG_{s+j+1}\cdots G_{s+m}, 1\le j\le m$
, $G_{r-j+1}\cdots G_{r-1}G_rG_{s+j+1}\cdots G_{s+m}, 1\le j\le m$![]() to get
to get
Fix some $s\in \mathbb {Z}+1/2$![]() and set ${\mathcal {O}}_n=\{s, s+1, s+2, \cdots, s+n\}$
and set ${\mathcal {O}}_n=\{s, s+1, s+2, \cdots, s+n\}$![]() . By (3.4) the following identity
. By (3.4) the following identity
holds for all $r_0, r_1, \cdots, r_{m+1}\in {\mathcal {O}}_{m+1}$![]() .
.
By (3.4) and (3.5) we see that (3.6) holds for all $r_0, r_1, \cdots, r_{m+1}\in {\mathcal {O}}_{m+2}$![]() .
.
We shall use the induction on $k$![]() to prove that
to prove that
for all $r_0, r_1,\cdots, r_{m+1}\in {\mathcal {O}}_{m+k}$![]() for all $k\ge 1$
for all $k\ge 1$![]() . Then, according to the arbitrariness of $s$
. Then, according to the arbitrariness of $s$![]() , we get the lemma by choosing $N=m+2$
, we get the lemma by choosing $N=m+2$![]() .
.
Suppose that (3.6) holds for all $r_0, r_1, \cdots, r_{m+1}\in {\mathcal {O}}_{n}$![]() and some $n>m+1$
and some $n>m+1$![]() . Now we shall prove that
. Now we shall prove that
holds for all $r_0< r_1<\cdots < r_{m}\in {\mathcal {O}}_{n}$![]() .
.
Case 1. $r_0=s+n-m$![]() .
.
In this case $r_i=s+n-m+i$![]() for any $i=1, 2, \ldots, m$
for any $i=1, 2, \ldots, m$![]() . So (3.7) follows from (3.4) directly.
. So (3.7) follows from (3.4) directly.
Case 2. $r_0=s+n-m-k$![]() for some $1\le k\le n-m$
for some $1\le k\le n-m$![]() .
.
Replacing $s, r$![]() by $s+n-m+1, s+n-k$
by $s+n-m+1, s+n-k$![]() in (3.1), respectively, we get
in (3.1), respectively, we get

So we get

In this case (3.7) follows by inductive hypothesis.
Theorem 3.4 Let $V$![]() be a simple cuspidal ${\mathfrak L}$
be a simple cuspidal ${\mathfrak L}$![]() -module. Then $V$
-module. Then $V$![]() is isomorphic to the Harish-Chandra module of the intermediate series: $V=\sum v_i\cong {\mathcal {A}}_{a, b}'$
is isomorphic to the Harish-Chandra module of the intermediate series: $V=\sum v_i\cong {\mathcal {A}}_{a, b}'$![]() for some $a, b\in \mathbb {C}$
for some $a, b\in \mathbb {C}$![]() with $L_mv_i=(a+i+bm)v_{m+i}, G_rv_i=0$
with $L_mv_i=(a+i+bm)v_{m+i}, G_rv_i=0$![]() for all $m,i\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$
for all $m,i\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$![]() .
.
Proof. Clearly $\dim V_i\le p$![]() for some positive integer $p$
for some positive integer $p$![]() holds for almost $i\in \mathbb {Z}$
holds for almost $i\in \mathbb {Z}$![]() and $C$
and $C$![]() acts on $V$
acts on $V$![]() as zero (see [Reference Kaplansky and Santharoubane17]). Now ${\mathfrak L}_{\bar 1}^iV$
as zero (see [Reference Kaplansky and Santharoubane17]). Now ${\mathfrak L}_{\bar 1}^iV$![]() is ${\mathfrak L}$
is ${\mathfrak L}$![]() -submodule since ${\mathfrak L}_{\bar 1}^{i+1}V\subset {\mathfrak L}_{\bar 1}^iV$
-submodule since ${\mathfrak L}_{\bar 1}^{i+1}V\subset {\mathfrak L}_{\bar 1}^iV$![]() for all $i\in \mathbb {N}$
for all $i\in \mathbb {N}$![]() . So ${\mathfrak L}_{\bar 1}V=V$
. So ${\mathfrak L}_{\bar 1}V=V$![]() or ${\mathfrak L}_{\bar 1}V=0$
or ${\mathfrak L}_{\bar 1}V=0$![]() .
.
By lemma 3.3, we get
If ${\mathfrak L}_{\bar 1}V=V$![]() then ${\mathfrak L}_{\bar 1}^NV=V=0$
then ${\mathfrak L}_{\bar 1}^NV=V=0$![]() , which is a contradiction. So ${\mathfrak L}_{\bar 1}V=0$
, which is a contradiction. So ${\mathfrak L}_{\bar 1}V=0$![]() and the proposition follows from theorem 2.1.
and the proposition follows from theorem 2.1.
4. Simple Harish–Chandra module
Now we can classify all simple Harish–Chandra modules over ${\mathfrak L}$![]() . The following result is well-known.
. The following result is well-known.
Lemma 4.1 Let $M$![]() be a Harish–Chandra module over the Virasoro algebra with $\mathrm {supp}(M)\subseteq \lambda +\mathbb {Z}$
be a Harish–Chandra module over the Virasoro algebra with $\mathrm {supp}(M)\subseteq \lambda +\mathbb {Z}$![]() . If for any $v\in M,$
. If for any $v\in M,$![]() there exists $N(v)\in \mathbb {N}$
there exists $N(v)\in \mathbb {N}$![]() such that $L_iv=0, \forall i\geq N(v)$
such that $L_iv=0, \forall i\geq N(v)$![]() , then $\mathrm {supp}(M)$
, then $\mathrm {supp}(M)$![]() is upper bounded.
is upper bounded.
Lemma 4.2 Suppose $M$![]() is a simple Harish–Chandra module which is not cuspidal over ${\mathfrak L}$
is a simple Harish–Chandra module which is not cuspidal over ${\mathfrak L}$![]() , then $M$
, then $M$![]() is a highest (or lowest) weight module.
is a highest (or lowest) weight module.
Proof. It is essentially the same as that of lemma 4.2 (1) in [Reference Cai and Lü9].
Fix $\lambda \in \mathrm {supp}(M)$![]() . Since $M$
. Since $M$![]() is not cuspidal, there exists $k\in \frac 12\mathbb {Z}$
is not cuspidal, there exists $k\in \frac 12\mathbb {Z}$![]() such that $\dim M_{-k+\lambda }>2(\dim M_\lambda +M_{\lambda +\frac 12}+\dim M_{\lambda +1})$
such that $\dim M_{-k+\lambda }>2(\dim M_\lambda +M_{\lambda +\frac 12}+\dim M_{\lambda +1})$![]() . Without loss of generality, we may assume that $k\in \mathbb {N}$
. Without loss of generality, we may assume that $k\in \mathbb {N}$![]() . Then there exists a nonzero element $w\in M_{-k+\lambda }$
. Then there exists a nonzero element $w\in M_{-k+\lambda }$![]() such that $L_kw=L_{k+1}w=G_{k+\frac 12}w=0$
such that $L_kw=L_{k+1}w=G_{k+\frac 12}w=0$![]() . Therefore, $L_iw=G_{i-\frac 12}w=0$
. Therefore, $L_iw=G_{i-\frac 12}w=0$![]() for all $i\geq k^2$
for all $i\geq k^2$![]() , since $[{\mathfrak L}_i,{\mathfrak L}_j]={\mathfrak L}_{i+j}$
, since $[{\mathfrak L}_i,{\mathfrak L}_j]={\mathfrak L}_{i+j}$![]() .
.
It is easy to see that $M'=\{v\in M|\dim {\mathfrak L}^+v<\infty \}$![]() is a nonzero submodule of $M$
is a nonzero submodule of $M$![]() , where ${\mathfrak L}^+=\sum _{n\in \mathbb {Z}_+}(\mathbb {C} L_n+\mathbb {C} G_{n-\frac 12})$
, where ${\mathfrak L}^+=\sum _{n\in \mathbb {Z}_+}(\mathbb {C} L_n+\mathbb {C} G_{n-\frac 12})$![]() . Hence $M=M'$
. Hence $M=M'$![]() . So, lemma 4.1 tells us that $\mathrm {supp}(M)$
. So, lemma 4.1 tells us that $\mathrm {supp}(M)$![]() is upper bounded, that is $M$
is upper bounded, that is $M$![]() is a highest weight module.
is a highest weight module.
Combining lemma 4.2 and theorem 3.4, we can get the following result.
Theorem 4.3 Any simple Harish–Chandra module over ${\mathfrak L}$![]() is a highest weight module, lowest weight module or is isomorphic to $\mathcal {A}_{a,b}'$
is a highest weight module, lowest weight module or is isomorphic to $\mathcal {A}_{a,b}'$![]() for some $a, b\in \mathbb {C}$
for some $a, b\in \mathbb {C}$![]() .
.
5. Applications
Some Lie superalgebras were constructed in [Reference Wang, Chen and Bai28] as an application of the classification of Balinsky–Novikov super-algebras with dimension $2|2$![]() . As applications of the above results, we can classify all Harish–Chandra modules over many Lie superalgebras listed in table 7 in [Reference Wang, Chen and Bai28].
. As applications of the above results, we can classify all Harish–Chandra modules over many Lie superalgebras listed in table 7 in [Reference Wang, Chen and Bai28].
5.1 The Lie superalgebra $\frak q$
By definition the Lie superalgebra $\frak q=\frak q_{\bar 0}+\frak q_{\bar 1}$![]() , where $\frak q_{\bar 0}:=\mathbb {C}\{L_m, H_m, C\mid m\in \mathbb {Z}\}$
, where $\frak q_{\bar 0}:=\mathbb {C}\{L_m, H_m, C\mid m\in \mathbb {Z}\}$![]() and $\frak q_{\bar 1}=\mathbb {C}\{G_p\mid p\in \mathbb {Z}+\frac 12\}$
and $\frak q_{\bar 1}=\mathbb {C}\{G_p\mid p\in \mathbb {Z}+\frac 12\}$![]() , is a subalgebra of the $N=2$
, is a subalgebra of the $N=2$![]() Neveu–Schwarz superconformal algebra, with the following relations:
Neveu–Schwarz superconformal algebra, with the following relations:

for $m,n\in \mathbb {Z},p, q\in \mathbb {Z}+\frac 12$![]() .
.
Clearly $\mathcal {A}_{a, b, c}=\sum _{i\in \mathbb {Z}}\mathbb {C} v_i$![]() is a $\frak q$
is a $\frak q$![]() -module with
-module with
Moreover ${\mathcal {A}}_{a, b, c}$![]() is simple if and only if $a\not \in \mathbb {Z}$
is simple if and only if $a\not \in \mathbb {Z}$![]() , or $b\ne 0, 1$
, or $b\ne 0, 1$![]() or $c\ne 0$
or $c\ne 0$![]() . We also use $\mathcal {A}_{a, b, c}'$
. We also use $\mathcal {A}_{a, b, c}'$![]() to denote the simple sub-quotient of $\mathcal {A}_{a, b, c}$
to denote the simple sub-quotient of $\mathcal {A}_{a, b, c}$![]() .
.
Proposition 5.1 Any simple cuspidal $\frak q$![]() -module $V$
-module $V$![]() is isomorphic to the module $\mathcal {A}_{a, b, c}'$
is isomorphic to the module $\mathcal {A}_{a, b, c}'$![]() of the intermediate series for some $a, b, c\in \mathbb {C}$
of the intermediate series for some $a, b, c\in \mathbb {C}$![]() .
.
Proof. Clearly, the subalgebra $\frak q':={\rm span}\{L_m, G_r, C\mid m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$![]() is isomorphic to ${\mathfrak L}$
is isomorphic to ${\mathfrak L}$![]() . By theorem 3.4, we can choose an irreducible $\frak q'$
. By theorem 3.4, we can choose an irreducible $\frak q'$![]() -module $V'$
-module $V'$![]() with $G_rV'=0$
with $G_rV'=0$![]() for all $r\in \mathbb {Z}+\frac 12$
for all $r\in \mathbb {Z}+\frac 12$![]() . In this case we have $V={\rm Ind}_{\frak q'}^{\frak q}V'$
. In this case we have $V={\rm Ind}_{\frak q'}^{\frak q}V'$![]() . Moreover we have $G_rV=0$
. Moreover we have $G_rV=0$![]() for all $r\in \mathbb {Z}+\frac 12$
for all $r\in \mathbb {Z}+\frac 12$![]() by (5.1). In this case the $\frak q$
by (5.1). In this case the $\frak q$![]() -module $V$
-module $V$![]() is simple if and only if $V$
is simple if and only if $V$![]() is a simple $\frak q_{\bar 0}$
is a simple $\frak q_{\bar 0}$![]() -module. So the proposition follows from the main theorem in [Reference Lü and Zhao22].
-module. So the proposition follows from the main theorem in [Reference Lü and Zhao22].
Remark 5.2 Proposition 5.1 palys a key role in the classification of all simple cuspidal weight modules for the $N=2$![]() Neveu–Schwarz superconformal algebra, see [Reference Liu, Pei and Xia21].
Neveu–Schwarz superconformal algebra, see [Reference Liu, Pei and Xia21].
5.2 The $N=1$ BMS$_3$
BMS$_3$ superalgebra
superalgebra
The Bondi–Metzner–Sachs (BMS$_3$![]() ) algebra is the symmetry algebra of asymptotically flat three-dimensional spacetimes [Reference Bondi, Van der Burg and Metzner7]. It is the semi-direct product of the Virasoro algebra with its adjoint module. The $N=1$
) algebra is the symmetry algebra of asymptotically flat three-dimensional spacetimes [Reference Bondi, Van der Burg and Metzner7]. It is the semi-direct product of the Virasoro algebra with its adjoint module. The $N=1$![]() super-BMS$_3$
super-BMS$_3$![]() is a minimal supersymmetric extension of the BMS$_3$
is a minimal supersymmetric extension of the BMS$_3$![]() algebra, which has been introduced to describe the asymptotic structure of the $N=1$
algebra, which has been introduced to describe the asymptotic structure of the $N=1$![]() supergravity in [Reference Barnich, Donnay, Matulich and Troncoso3, Reference Dilxat, Gao and Liu11].
supergravity in [Reference Barnich, Donnay, Matulich and Troncoso3, Reference Dilxat, Gao and Liu11].
Definition 5.3 The $N$![]() =1 BMS$_3$
=1 BMS$_3$![]() superalgebra $\mathcal {B}$
superalgebra $\mathcal {B}$![]() is a Lie superalgebra with a basis $\{L_m, I_m, Q_r, C_1, C_2\mid m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$
is a Lie superalgebra with a basis $\{L_m, I_m, Q_r, C_1, C_2\mid m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$![]() , with the following commutation relations:
, with the following commutation relations:

for any $m, n\in \mathbb {Z}, r, s\in \mathbb {Z}+\frac 12$![]() .
.
Note that $\mathcal {B}=\mathcal {B}_{\bar 0}+\mathcal {B}_{\bar 1}$![]() , where $\mathcal {B}_{\bar 0}:=\mathbb {C}\{L_m, I_m, C_1, C_2\mid m\in \mathbb {Z}\}$
, where $\mathcal {B}_{\bar 0}:=\mathbb {C}\{L_m, I_m, C_1, C_2\mid m\in \mathbb {Z}\}$![]() and $\mathcal {B}_{\bar 1}=\mathbb {C}\{Q_p\mid p\in \mathbb {Z}+\frac 12\}$
and $\mathcal {B}_{\bar 1}=\mathbb {C}\{Q_p\mid p\in \mathbb {Z}+\frac 12\}$![]() . The quotient algebra $\mathcal {B}/J$
. The quotient algebra $\mathcal {B}/J$![]() is isomorphic to ${\mathfrak L}$
is isomorphic to ${\mathfrak L}$![]() , where $J=\mathbb {C}\{I_m, C_2\mid m\in \mathbb {Z}\}$
, where $J=\mathbb {C}\{I_m, C_2\mid m\in \mathbb {Z}\}$![]() .
.
Clearly the Vir-module $\mathcal {A}_{a, b}$![]() can become a $\mathcal {B}$
can become a $\mathcal {B}$![]() -module with the trivial actions of $I_m, Q_r$
-module with the trivial actions of $I_m, Q_r$![]() for any $m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$
for any $m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$![]() .
.
Proposition 5.4 Any simple cuspidal $\mathcal {B}$![]() -module $V$
-module $V$![]() is isomorphic to the module $\mathcal {A}_{a, b}'$
is isomorphic to the module $\mathcal {A}_{a, b}'$![]() of the intermediate series for some $a, b\in \mathbb {C}$
of the intermediate series for some $a, b\in \mathbb {C}$![]() .
.
Proof. Clearly, the subalgebra $\mathcal {B}_{\bar 0}$![]() is isomorphic to $W(2, 2)$
is isomorphic to $W(2, 2)$![]() . By theorem 4.6 in [Reference Guo, Lü and Zhao14], we can choose an irreducible $\mathcal {B}_{\bar 0}$
. By theorem 4.6 in [Reference Guo, Lü and Zhao14], we can choose an irreducible $\mathcal {B}_{\bar 0}$![]() -module $V'$
-module $V'$![]() with $I_mV'=C_1V'=C_2V'=0$
with $I_mV'=C_1V'=C_2V'=0$![]() for all $m\in \mathbb {Z}$
for all $m\in \mathbb {Z}$![]() . In this case we have $V={\rm Ind}_{\mathcal {B}_{\bar 0}}^{\mathcal {B}}V'$
. In this case we have $V={\rm Ind}_{\mathcal {B}_{\bar 0}}^{\mathcal {B}}V'$![]() . Moreover we have $I_mV=0$
. Moreover we have $I_mV=0$![]() and $[G_r, G_s]V=0$
and $[G_r, G_s]V=0$![]() for all $m\in \mathbb {Z}, r, s\in \mathbb {Z}+\frac 12$
for all $m\in \mathbb {Z}, r, s\in \mathbb {Z}+\frac 12$![]() by definition 5.3. In this case the $\mathcal {B}$
by definition 5.3. In this case the $\mathcal {B}$![]() -module $V$
-module $V$![]() is simple if and only if $V$
is simple if and only if $V$![]() is a simple $\mathcal {B}/J$
is a simple $\mathcal {B}/J$![]() -module. So the proposition follows from theorem 3.4.
-module. So the proposition follows from theorem 3.4.
5.3 The super $W(2,2)$ algebra
algebra
By definition, the super $W(2,2)$![]() algebra is the Lie superalgebra $SW(2,2):= \mathbb {C}\{L_m, I_m, G_r, Q_r, C_1, C_2\mid m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$
algebra is the Lie superalgebra $SW(2,2):= \mathbb {C}\{L_m, I_m, G_r, Q_r, C_1, C_2\mid m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$![]() with the following relations:
with the following relations:

for any $m, n\in \mathbb {Z}, r, s\in \mathbb {Z}+\frac 12$![]() .
.
Note that $SW(2,2)=SW(2,2)_{\bar 0}+SW(2,2)_{\bar 1}$![]() , where $SW(2,2)_{\bar 0}:=\mathbb {C}\{L_m, I_m, C_1, C_2\mid m\in \mathbb {Z}\}$
, where $SW(2,2)_{\bar 0}:=\mathbb {C}\{L_m, I_m, C_1, C_2\mid m\in \mathbb {Z}\}$![]() and $SW(2,2)_{\bar 1}=\mathbb {C}\{G_p, Q_p\mid p\in \mathbb {Z}+\frac 12\}$
and $SW(2,2)_{\bar 1}=\mathbb {C}\{G_p, Q_p\mid p\in \mathbb {Z}+\frac 12\}$![]() .
.
Clearly the subalgebra generated by $\{L_m, G_r, C~|~ m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$![]() is isomorphic to the $N=1$
is isomorphic to the $N=1$![]() Neveu–Schwarz algebra $\frak S$
Neveu–Schwarz algebra $\frak S$![]() (see [Reference Liu, Pei and Xia19]). From [Reference Su26] we see that $S_{a, b}$
(see [Reference Liu, Pei and Xia19]). From [Reference Su26] we see that $S_{a, b}$![]() or $\Pi S_{a, b}$
or $\Pi S_{a, b}$![]() is the Harich–Chandra module of intermediate series over $\frak S$
is the Harich–Chandra module of intermediate series over $\frak S$![]() for some $a, b\in \mathbb {C}$
for some $a, b\in \mathbb {C}$![]() , where $S_{a, b}$
, where $S_{a, b}$![]() is defined as follows:
is defined as follows:

for all $n, i\in \mathbb {Z}, r, k\in \mathbb {Z}+\frac 12$![]() .
.
Moreover $S_{a, b}$![]() is simple if and only if $a\not \in \mathbb {Z}$
is simple if and only if $a\not \in \mathbb {Z}$![]() or $a\in \mathbb {Z}$
or $a\in \mathbb {Z}$![]() and $b\ne 0, \frac 12$
and $b\ne 0, \frac 12$![]() . We also use $S_{a, b}'$
. We also use $S_{a, b}'$![]() to denote the simple sub-quotient of $S_{a, b}$
to denote the simple sub-quotient of $S_{a, b}$![]() .
.
Clearly the $\frak S$![]() -modules $S_{a, b}$
-modules $S_{a, b}$![]() and $\Pi S_{a, b}$
and $\Pi S_{a, b}$![]() become $SW(2,2)$
become $SW(2,2)$![]() -modules with trivial actions of $I_m, Q_{m+\frac 12}$
-modules with trivial actions of $I_m, Q_{m+\frac 12}$![]() for any $m\in \mathbb {Z}$
for any $m\in \mathbb {Z}$![]() .
.
Proposition 5.5 Any simple cuspidal $SW(2, 2)$![]() -module $V$
-module $V$![]() is isomorphic to $S_{a, b}'$
is isomorphic to $S_{a, b}'$![]() or $\Pi S_{a, b}'$
or $\Pi S_{a, b}'$![]() for some $a, b\in \mathbb {C}$
for some $a, b\in \mathbb {C}$![]() .
.
Proof. Set $\frak p={\rm span}\{L_m, I_m, Q_r, C_1, C_2\mid m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12\}$![]() . By proposition 5.4 we can choose a simple $\frak p$
. By proposition 5.4 we can choose a simple $\frak p$![]() -module $V'$
-module $V'$![]() with $I_mV'=Q_rV'=C_2V'=C_2V'=0$
with $I_mV'=Q_rV'=C_2V'=C_2V'=0$![]() for all $m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$
for all $m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$![]() . In this case we have $V={\rm Ind}_{\frak p}^{SW(2,2)}V'$
. In this case we have $V={\rm Ind}_{\frak p}^{SW(2,2)}V'$![]() . Moreover we have $I_mV=Q_rV=0$
. Moreover we have $I_mV=Q_rV=0$![]() for all $m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$
for all $m\in \mathbb {Z}, r\in \mathbb {Z}+\frac 12$![]() by definition 5.2. In this case the $SW(2, 2)$
by definition 5.2. In this case the $SW(2, 2)$![]() -module $V$
-module $V$![]() is simple if and only if $V$
is simple if and only if $V$![]() is a simple $\frak S$
is a simple $\frak S$![]() -module. So the proposition follows from the main theorem in [Reference Su26] (also see theorem 4.5 in [Reference Cai and Lü9]).
-module. So the proposition follows from the main theorem in [Reference Su26] (also see theorem 4.5 in [Reference Cai and Lü9]).
Remark 5.6 We can easily prove that any simple Harish–Chandra module over the above Lie superalgebras is a cuspidal module, or a highest (or lowest) weight module as lemma 4.2. So all simple Harish–Chandra modules over the above Lie superalgebras are also classified.
Remark 5.7 All indecomposable modules of the intermediate series and some other representations were studied in [Reference Wang, Geng and Chen29] and [27].
Acknowledgements
This work is partially supported by the NNSF (Nos. 12071405, 12271085, 11971315, 11871249), and is partially supported by Xinjiang Uygur Autonomous Region graduate scientific research innovation project (No. XJ2021G021). The authors would like to thank Prof. Rencai Lü for helpful discussions.