No CrossRef data available.
Multiple positive solutions for an unbounded Dirichlet boundary problem involving sign-changing weight
Published online by Cambridge University Press: 04 November 2009
Abstract
We study the multiplicity of positive solutions for the following semilinear elliptic equation:

where 1 < q < 2 < p < 2* (2* = 2N/(N − 2) if N ≥ 3, 2* = ∞ if N = 2), the parameters λ, μ ≥ 0, 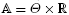 is an infinite strip in ℝN and Θ is a bounded domain in ℝN−1 We assume that fλ(x) = λf+(x) + f−(x) and gμ(x) = a(x) + μb(x), where the functions f±, a and b satisfy suitable conditions.
is an infinite strip in ℝN and Θ is a bounded domain in ℝN−1 We assume that fλ(x) = λf+(x) + f−(x) and gμ(x) = a(x) + μb(x), where the functions f±, a and b satisfy suitable conditions.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 139 , Issue 6 , December 2009 , pp. 1297 - 1325
- Copyright
- Copyright © Royal Society of Edinburgh 2009


