Article contents
Multipliers of invariant subspaces in the bidisc
Published online by Cambridge University Press: 20 January 2009
Abstract
For any nonzero invariant subspace M in H2(T2), set 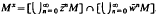 . Then Mx is also an invariant subspace of H2(T2) that contains M. If M is of finite codimension in H2(T2) then Mx = H2(T2) and if M = qH2(T2) for some inner function q then Mx = M. In this paper invariant subspaces with Mx = M are studied. If M = q1H2(T2) ∩ q2H2(T2) and q1, q2 are inner functions then Mx = M. However in general this invariant subspace may not be of the form: qH2(T2) for some inner function q. Put
. Then Mx is also an invariant subspace of H2(T2) that contains M. If M is of finite codimension in H2(T2) then Mx = H2(T2) and if M = qH2(T2) for some inner function q then Mx = M. In this paper invariant subspaces with Mx = M are studied. If M = q1H2(T2) ∩ q2H2(T2) and q1, q2 are inner functions then Mx = M. However in general this invariant subspace may not be of the form: qH2(T2) for some inner function q. Put 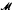 (M) = {ø ∈ L ∞: ø M ⊆ H2(T2)}; then
(M) = {ø ∈ L ∞: ø M ⊆ H2(T2)}; then 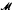 (M) is described and
(M) is described and 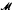 (M) =
(M) = 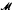 (Mx) is shown. This is the set of all multipliers of M in the title. A necessary and sufficient condition for
(Mx) is shown. This is the set of all multipliers of M in the title. A necessary and sufficient condition for 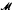 (M) = H∞(T2) is given. It is noted that the kernel of a Hankel operator is an invariant subspace M with Mx = M. The argument applies to the polydisc case.
(M) = H∞(T2) is given. It is noted that the kernel of a Hankel operator is an invariant subspace M with Mx = M. The argument applies to the polydisc case.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1994
References
REFERENCES
- 1
- Cited by


