No CrossRef data available.
Article contents
The Absolute Cesaro Summability of the Successively Derived Allied Series of a Fourier Series
Published online by Cambridge University Press: 20 January 2009
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We suppose that f(t) is integrable in the Lebesgue sense m (π, π) and is periodic with period 2π. We denote its Fourier series by
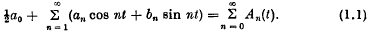
Then the allied series is
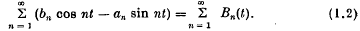
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 8 , Issue 4 , October 1950 , pp. 163 - 176
- Copyright
- Copyright © Edinburgh Mathematical Society 1950
References
REFERENCES
Bhatnagar, S. P., “On the Fourier coefficients of a discontinuous function,” Proe. Edinburgh Math. Soc. (2), 6 (1939–1941), 231–256.Google Scholar
Bosanquet, L. S., | FS 0 | , “Note on the absolute summability (C) of a Fourier series,” Journal London Math. Soc., 11 (1936), 11–15.Google Scholar
Bosanquet, L. S., | FS a | , “The absolute Cesaro summability of a Fourier series,” Proe. London Math. Soc. (2), 41 (1936), 517–528.Google Scholar
Bosanquet, L. S., DFS, “Note on differentiated Fourier series,” Quart. J. of Math. (Oxford Series), 10 (1939), 67–74.Google Scholar
Bosanquet, L. S., DrFS, “A solution of the Cesàro summability problem for successively derived Fourier series,” Proe. London Math. Soc. (2), 46 (1940), 270–289.CrossRefGoogle Scholar
Bosanquet, L. S., | DFS | , “The absolute Cesàro summability problem for differentiated Fourier series,” Quart J. of Math. (Oxford Series), 12 (1941), 15–25.Google Scholar
Bosanquet, L. S., CL, “Some properties of Cesàro-Lebesgue integrals,” Proe. London Math. Soc. (2), 49 (1945), 40–62.Google Scholar
Bosanquet, L. S., DrFS “The Cesàro summability of the successively derived allied series of a Fourier series,” Proe. London Math. Soc. (2), 49 (1945), 63–76.Google Scholar
Bosanquet, L. S. and Chow, H. C., “Some analogues of a theorem of Andersen,” Journal London Math. Soc, 16 (1941), 42–48.CrossRefGoogle Scholar
Bosanquet, L. S. and Hyslop, J. M., “On the absolute summability of the allied series of a Fourier series,” Math. Zeit., 42 (1937), 489–512.Google Scholar
Chow, H. C., “On the absolute summability (C) of power series,” Journal London Math. Soc., 14 (1939), 101–112.Google Scholar
Hardy, G. H., “Notes on some points in the integral calculus (LXVI): The arithmetic mean of a Fourier constant,” Messenger of Math., 58 (1928), 50–52.Google Scholar
Hardy, G. H. and Rogosinski, W. W., “Notes on Fourier series (IV):Summability (R2),” Proc. Cambridge Phil. Soc., 43 (1947), 10–25.Google Scholar
Obrechkoff, N., “Sur la sommation des séries trigonométriques de Fourier par les moyennes arithmétiques,” Bull, de la Soc. Math, de France, 62 (1934), 84–109 and 167–184.Google Scholar
Zygmund, A., “Sur un théorème de M. Gronwall,” Bull, de l'cad. polonaise (Cracovie), A (1925), 207–217.Google Scholar


