Hostname: page-component-745bb68f8f-s22k5 Total loading time: 0 Render date: 2025-02-11T16:45:53.648Z Has data issue: false hasContentIssue false
  • English
  • Français

LITTLEWOOD–PALEY CHARACTERIZATIONS OF ANISOTROPIC WEAK MUSIELAK–ORLICZ HARDY SPACES

Part of: Linear function spaces and their duals Harmonic analysis in several variables

Published online by Cambridge University Press:  16 March 2018

BO LI ,
RUIRUI SUN ,
MINFENG LIAO  and
BAODE LI
BO LI
Affiliation:
College of Mathematics and System Science, Xinjiang University, Urumqi 830046, PR China email [email protected]
RUIRUI SUN
Affiliation:
College of Mathematics and System Science, Xinjiang University, Urumqi 830046, PR China email [email protected]
MINFENG LIAO
Affiliation:
College of Mathematics and System Science, Xinjiang University, Urumqi 830046, PR China email [email protected]
BAODE LI*
Affiliation:
College of Mathematics and System Science, Xinjiang University, Urumqi 830046, PR China email [email protected]
*
*Corresponding author.
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 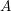$A$ be an expansive dilation on $\mathbb{R}^{n}$ and 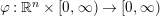$\unicode[STIX]{x1D711}:\mathbb{R}^{n}\times [0,\infty )\rightarrow [0,\infty )$ an anisotropic growth function. In this article, the authors introduce the anisotropic weak Musielak–Orlicz Hardy space 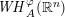$\mathit{WH}_{A}^{\unicode[STIX]{x1D711}}(\mathbb{R}^{n})$ via the nontangential grand maximal function and then obtain its Littlewood–Paley characterizations in terms of the anisotropic Lusin-area function, 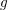$g$-function or 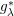$g_{\unicode[STIX]{x1D706}}^{\ast }$-function, respectively. All these characterizations for anisotropic weak Hardy spaces 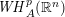$\mathit{WH}_{A}^{p}(\mathbb{R}^{n})$ (namely, $\unicode[STIX]{x1D711}(x,t):=t^{p}$ for all 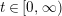$t\in [0,\infty )$ and 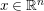$x\in \mathbb{R}^{n}$ with 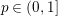$p\in (0,1]$) are new. Moreover, the range of 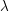$\unicode[STIX]{x1D706}$ in the anisotropic $g_{\unicode[STIX]{x1D706}}^{\ast }$-function characterization of 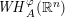$\mathit{WH}_{A}^{\unicode[STIX]{x1D711}}(\mathbb{R}^{n})$ coincides with the best known range of the $g_{\unicode[STIX]{x1D706}}^{\ast }$-function characterization of classical Hardy space 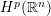$H^{p}(\mathbb{R}^{n})$ or its weighted variants, where $p\in (0,1]$.

MSC classification

Primary: 42B25: Maximal functions, Littlewood-Paley theory 42B30: $H^p$-spaces 42B35: Function spaces arising in harmonic analysis 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.)
Type
Article
Information
Nagoya Mathematical Journal , Volume 237 , March 2020 , pp. 39 - 78
Copyright
© 2018 Foundation Nagoya Mathematical Journal  

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This project is partially supported by the National Natural Science Foundation of China (Grant Nos. 11461065 and 11661075).

References

Aguilera, N. and Segovia, C., Weighted norm inequalities relating the g 𝜆 ∗ and the area functions , Studia Math. 61 (1977), 293–303.Google Scholar
Álvarez, J. and Milman, M., H p continuity properties of Calderón–Zygmund-type operators , J. Math. Anal. Appl. 118 (1986), 63–79.Google Scholar
Astala, K., Iwaniec, T., Koskela, P. and Martin, G., Mappings of BMO-bounded distortion , Math. Ann. 317 (2000), 703–726.Google Scholar
Birnbaum, Z. and Orlicz, W., Über die verallgemeinerung des begriffes der zueinander konjugierten potenzen , Studia Math. 3 (1931), 1–67.Google Scholar
Bonami, A., Feuto, J. and Grellier, S., Endpoint for the DIV-CURL lemma in Hardy spaces , Publ. Mat. 54 (2010), 341–358.Google Scholar
Bonami, A., Grellier, S. and Ky, L. D., Paraproducts and products of functions in BMO (ℝ n ) and H 1(ℝ n ) through wavelets , J. Math. Pures Appl. (9) 97 (2012), 230–241.Google Scholar
Bonami, A., Iwaniec, T., Jones, P. and Zinsmeister, M., On the product of functions in BMO and H 1 , Ann. Inst. Fourier (Grenoble) 57 (2007), 1405–1439.Google Scholar
Bownik, M., Anisotropic Hardy spaces and wavelets , Mem. Amer. Math. Soc. 164 (2003), vi + 122 pp.Google Scholar
Bownik, M., Boundedness of operators on Hardy spaces via atomic decompositions , Proc. Amer. Math. Soc. 133 (2005), 3535–3542.Google Scholar
Bownik, M., Anisotropic Triebel–Lizorkin spaces with doubling measures , J. Geom. Anal. 17 (2007), 387–424.Google Scholar
Bownik, M. and Ho, K.-P., Atomic and molecular decompositions of anisotropic Triebel–Lizorkin spaces , Trans. Amer. Math. Soc. 358 (2006), 1469–1510.Google Scholar
Bownik, M., Li, B., Yang, D. and Zhou, Y., Weighted anisotropic Hardy spaces and their applications in boundedness of sublinear operators , Indiana Univ. Math. J. 57 (2008), 3065–3100.Google Scholar
Bownik, M., Li, B., Yang, D. and Zhou, Y., Weighted anisotropic product Hardy spaces and boundedness of sublinear operators , Math. Nachr. 283 (2010), 392–442.Google Scholar
Bui, T. A., Cao, J., Ky, L. D., Yang, D. and Yang, S., Musielak–Orlicz Hardy spaces associated with operators satisfying reinforced off-diagonal estimates , Anal. Geom. Metr. Spaces 1 (2013), 69–129.Google Scholar
Chang, D.-C., Yang, D. and Yang, S., “ Real-variable theory of Orlicz-type function spaces associated with operators — a survey ”, in Some Topics in Harmonic Analysis and Applications, Adv. Lect. Math. 34 , Int. Press, Somerville, MA, 2016, 27–70.Google Scholar
Coifman, R. R. and Weiss, G., Analyse Harmonique Non-commutative sur Certains Espaces Homogènes, Lect. Notes Math. 242 , Springer, Berlin, New York, 1971, v + 160 pp.Google Scholar
Diening, L., Maximal function on Musielak–Orlicz spaces and generalized Lebesgue spaces , Bull. Sci. Math. 129 (2005), 657–700.Google Scholar
Ding, Y. and Lan, S., Anisotropic Hardy space estimates for multilinear operators , Adv. Math. (China) 38 (2009), 168–184.Google Scholar
Fefferman, R. and Soria, F., The space weak H 1 , Studia Math. 85 (1986), 1–16.Google Scholar
Folland, G. B. and Stein, E. M., Hardy Spaces on Homogeneous Groups, Math. Notes 28 , Princeton University Press, Princeton, NJ; University of Tokyo Press, Tokyo, 1982, xii + 285 pp.Google Scholar
Frazier, M., Jawerth, B. and Weiss, G., Littlewood–Paley Theory and the Study of Function Spaces, CBMS Regional Conference Series in Mathematics 79, Published for the Conference Board of the Mathematical Sciences, Washington, DC, American Mathematical Society, Providence, RI, 1991, viii + 132 pp.Google Scholar
García-Cuerva, J. and Rubio de Francia, J. L., Weighted Norm Inequalities and Related Topics, North-Holland Mathematics Studies 116 , North-Holland, Amsterdam, 1985, x + 604 pp.Google Scholar
Grafakos, L., Modern Fourier Analysis, 2nd ed., Graduate Texts in Mathematics 250 , Springer, New York, 2009, xvi + 504 pp.Google Scholar
Graversen, S. E., Pes̆kir, G. and Weber, M., The continuity principle in exponential type Orlicz spaces , Nagoya Math. J. 137 (1995), 55–75.Google Scholar
Hou, S., Yang, D. and Yang, S., Lusin area function and molecular characterizations of Musielak–Orlicz Hardy spaces and their applications , Commun. Contemp. Math. 15 (2013), 423–434.Google Scholar
Iwaniec, T. and Onninen, J., H 1 -estimates of Jacobians by subdeterminants , Math. Ann. 324 (2002), 341–358.Google Scholar
Janson, S., Generalizations of Lipschitz spaces and an application to Hardy spaces and bounded mean oscillation , Duke Math. J. 47 (1980), 959–982.Google Scholar
Jiang, R. and Yang, D., New Orlicz–Hardy spaces associated with divergence form elliptic operators , J. Funct. Anal. 258 (2010), 1167–1224.Google Scholar
Johnson, R. and Neugebauer, C. J., Homeomorphisms preserving A p , Rev. Mat. Iberoam. 3 (1987), 249–273.Google Scholar
Kalton, N. J., Linear operators on L p for 0 < p < 1 , Trans. Amer. Math. Soc. 259 (1980), 319–355.Google Scholar
Kilpeläinen, T., Koskela, P. and Masaoka, H., Harmonic Hardy–Orlicz spaces , Ann. Acad. Sci. Fenn. Math. 38 (2013), 309–325.Google Scholar
Ky, L. D., Bilinear decompositions and commutators of singular integral operators , Trans. Amer. Math. Soc. 365 (2013), 2931–2958.Google Scholar
Ky, L. D., New Hardy spaces of Musielak–Orlicz type and boundedness of sublinear operators , Integral Equations Operator Theory 78 (2014), 115–150.Google Scholar
Ky, L. D., Bilinear decompositions for the product space H L 1 ×BMO L , Math. Nachr. 287 (2014), 1288–1297.Google Scholar
Lerner, A. K., Sharp weighted norm inequalities for Littlewood–Paley operators and singular integrals , Adv. Math. 226 (2011), 3912–3926.Google Scholar
Li, B., Fan, X. and Yang, D., Littlewood–Paley characterizations of anisotropic Hardy spaces of Musielak–Orlicz type , Taiwanese J. Math. 19 (2015), 279–314.Google Scholar
Li, B., Yang, D. and Yuan, W., Anisotropic Hardy spaces of Musielak–Orlicz type with applications to boundedness of sublinear operators , The Scientific World Journal 2014 (2014), 19 pp.Google Scholar
Liang, Y., Huang, J. and Yang, D., New real-variable characterizations of Musielak–Orlicz Hardy spaces , J. Math. Anal. Appl. 395 (2012), 413–428.Google Scholar
Liang, Y. and Yang, D., Musielak–Orlicz Campanato spaces and applications , J. Math. Anal. Appl. 406 (2013), 307–322.Google Scholar
Liang, Y. and Yang, D., Intrinsic Littlewood–Paley function characterizations of Musielak–Orlicz Hardy spaces , Trans. Amer. Math. Soc. 367 (2015), 3225–3256.Google Scholar
Liang, Y., Yang, D. and Jiang, R., Weak Musielak–Orlicz Hardy spaces and applications , Math Nachr. 289 (2016), 637–677.Google Scholar
Liu, H., “ The weak H p spaces on homogenous groups ”, in Harmonic Analysis (Tianjin, 1988), Lecture Notes in Mathematics 1984 , Springer, Berlin, 1991, 113–118.Google Scholar
Martínez, S. and Wolanski, N., A minimum problem with free boundary in Orlicz spaces , Adv. Math. 218 (2008), 1914–1971.Google Scholar
Musielak, J., Orlicz Spaces and Modular Spaces, Lecture Notes in Mathematics 1034 , Springer, Berlin, 1983, iii + 222 pp.Google Scholar
Nakai, E. and Sawano, Y., Orlicz–Hardy spaces and their duals , Sci. China Math. 57 (2014), 903–962.Google Scholar
Orlicz, W., Über eine gewisse Klasse von Räumen vom Typus B , Bull. Int. Acad. Polon. Ser. A 8 (1932), 207–220.Google Scholar
Qi, C., Zhang, H. and Li, B., Boundedness of Littlewood–Paley functions on anisotropic weak Hardy spaces of Musielak–Orlicz type , J. Xinjiang Univ. Natur. Sci. 33 (2016), 287–295.Google Scholar
Quek, T. and Yang, D., Calderón–Zygmund-type operators on weighted weak Hardy spaces over ℝ n , Acta Math. Sin. (Engl. Ser.) 16 (2000), 141–160.Google Scholar
Stein, E. M., Taibleson, M. H. and Weiss, G., Weak type estimates for maximal operators on certain H p classes , Rend. Circ. Mat. Palermo 1 (1981), 81–97.Google Scholar
Strömberg, J.-O., Bounded mean oscillation with Orlicz norms and duality of Hardy spaces , Indiana Univ. Math. J. 28 (1979), 511–544.Google Scholar
Strömberg, J.-O. and Torchinsky, A., Weighted Hardy Spaces, Lecture Notes in Mathematics 1381 , Springer, Berlin, 1989, vi + 193 pp.Google Scholar
Tran, T. D., Musielak–Orlicz Hardy spaces associated with divergence form elliptic operators without weight assumptions , Nagoya Math. J. 216 (2014), 71–110.Google Scholar
Triebel, H., Theory of Function Spaces, Monographs in Mathematics 78 , Birkhäuser, Basel, 1983, 284 pp.Google Scholar
Yang, D., Liang, Y. and Ky, L. D., Real-Variable Theory of Musielak–Orlicz Hardy Spaces, Lecture Notes in Mathematics 2182 , Springer, Cham, 2017, xiii + 466 pp.Google Scholar
Zhang, H., Qi, C. and Li, B., Anisotropic weak Hardy spaces of Musielak–Orlicz type and their applications , Front. Math. China 12 (2017), 993–1022.Google Scholar