Article contents
CONCAVITY PROPERTY OF MINIMAL 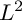 $L^{2}$ INTEGRALS WITH LEBESGUE MEASURABLE GAIN
$L^{2}$ INTEGRALS WITH LEBESGUE MEASURABLE GAIN
Published online by Cambridge University Press: 05 June 2023
Abstract
In this article, we present a concavity property of the minimal 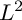 $L^{2}$ integrals related to multiplier ideal sheaves with Lebesgue measurable gain. As applications, we give necessary conditions for our concavity degenerating to linearity, characterizations for 1-dimensional case, and a characterization for the holding of the equality in optimal
$L^{2}$ integrals related to multiplier ideal sheaves with Lebesgue measurable gain. As applications, we give necessary conditions for our concavity degenerating to linearity, characterizations for 1-dimensional case, and a characterization for the holding of the equality in optimal 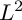 $L^2$ extension problem on open Riemann surfaces with weights may not be subharmonic.
$L^2$ extension problem on open Riemann surfaces with weights may not be subharmonic.
MSC classification
- Type
- Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of Foundation Nagoya Mathematical Journal
Footnotes
Qi’an Guan was supported by the National Key R&D Program of China (Grant No. 2021YFA1003100) and the National Natural Science Foundation of China (Grant No. NSFC-11825101, NSFC-11522101, and NSFC-11431013).
References
- 8
- Cited by



