Crystal structures of rhodium-containing erlichmanite–laurite solid solutions (Os_1–x–yRu_xRh_yS₂: x = 0.09–0.60, y = 0.07–0.10) with unique compositional dependence

Abstract

Rh-rich and Ir-poor erlichmanite–laurite OsS₂–RuS₂ solid solutions have been discovered at placers in Haraigawa, Misato-machi, Kumamoto, Japan. Microprobe analysis was performed to identify solid solutions containing few sub-components other than Rh. Approximately 10 at.% Rh was found to be present in the solid-solution samples. Structural refinement was performed using four natural samples: Os_0.32Ru_0.61Rh_0.07S₂, Os_0.49Ru_0.43Rh_0.08S₂, Os_0.58Ru_0.33Rh_0.08S₂ and Os_0.81Ru_0.09Rh_0.10S₂. The unit-cell parameters for the solid solutions containing Rh from Haraigawa varied from 5.61826(6) to 5.63142(8) Å. The (Os, Ru, Rh)–S distances in the Os_1–x–yRu_xRh_yS₂ system were almost constant with a small variation of 0.001 Å. Conversely, the S–S distances varied significantly, with variations approaching 0.1 Å. Rh substitution of Os rather than Ru had a larger impact on the crystal structure. The atomic displacement ellipsoid of both cations and anions was almost spherical, and no elongation along the M–S and S–S bond directions was observed. The bulk Debye temperatures were estimated from the Debye–Waller factor for the sulfide site. The bulk Debye temperatures of pure OsS₂ and RuS₂ were 688 K and 661 K, respectively, which suggests that the melting point of erlichmanite is higher than that of laurite. The high Debye temperature of OsS₂ is inconsistent with the crystallisation of laurite prior to erlichmanite from the primitive magma, which suggests that $f_{\rm S_2}$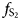, rather than temperature, is the main cause of the known crystallisation order. The presence of several percent Rh has a significant effect on the thermal stability of OsS₂ and lowers the melting point of the erlichmanite solid solution compared to that of the laurite solid solution.

Introduction

Laurite (RuS₂)–erlichmanite (OsS₂) solid-solution series

Laurite (ideal end-member: RuS₂) has long been known as a rare mineral and was described by Wöhler (1866). Conversely, erlichmanite (OsS₂) was discovered much more recently: erlichmanite present in a Pt–Fe alloy from placer deposits was first reported by Snetsinger (1971), and several other studies (Sutarno et al., 1967; Leonard et al., 1969; Harris, 1974; Begizov et al. 1976; Cabri, 2002) have detailed mineralogical descriptions of the laurite–erlichmanite solid-solution series compositions obtained from placer deposits. A complete solid solution can be formed between laurite and erlichmanite, with considerable substitution of Os and Ru by Ir, Rh, Pd and Pt (Leonard et al., 1969; Snetsinger, 1971; Harris, 1974; Begizov et al., 1976; Bowles et al., 1983; Cabri, 2002). In most cases, laurite and erlichmanite contain elements in the order Ir, Rh, Pd and Pt, though Ni and Fe have been detected as trace elements (Cabri, 2002). Bowles et al. (1983) reported a very high Ir content of 0.04–0.13 atoms per formula unit (apfu) in some laurite–erlichmanite solid solutions. In solid solutions with Ru >0.15 apfu [x > 0.15 in Os_1–x–yRu_x(Ir,Rh,Pd,Pt)_yS₂)], Ir is predominant over Rh. Thus, for the majority of the laurite–erlichmanite solid solutions, Ir is the predominant additional metal, causing a large fluctuation in the Ir/Rh atomic ratio. However, owing to significant amounts of transition metals such as Ir, Rh, Pd and Pt dissolved in naturally occurring laurite–erlichmanite solid-solution minerals (Bowles et al., 1983), no systematic study of the structure of this solid solution has been conducted.

Members of the laurite–erlichmanite solid-solution series are common platinum-group minerals (PGM) in podiform chromite ores hosted by mantle peridotites in ophiolite complexes. They occur mainly as small mineral inclusions in chromites associated with other PGM, base-metal sulfides, and silicates (Stockman and Hlava, 1984; Legendre and Augé, 1986; Augé and Johan, 1988; Corrivaux and Gilles Laflamme, 1990; Torres-Ruiz et al., 1996; Melcher et al., 1997; Garuti et al., 1999a, 1999b; Ahmed and Arai, 2003; Gervilla et al., 2005; González-Jiménez et al., 2007; Arai, 2012). Several studies have reported that some laurite–erlichmanite crystals form directly from sulfur-undersaturated mafic melts prior to, or along with, the crystallisation of chromite, and that they act as nuclei for chromite crystallisation (Stockman and Hlava, 1984; Augé, 1985; Ahmed and Arai, 2003). The laurite–erlichmanite solid solution in depleted ophiolites are usually Ir rich and somewhat Rh poor. Laurite–erlichmanite solid solutions from different types of chromitite have certain characteristics in terms of the amount of trace elements in their solid solutions.

Two different types of placers are known for PGM, one corresponding to the iridium-subgroup element (IPGE: Ru, Os and Ir) distribution, and the other to the palladium-subgroup element (PPGE: Rh, Pd and Pt) distribution (Mertie, 1969; Ohta and Nakagawa, 1990; Harris and Cabri, 1991; Nakagawa and Ohta, 1993; Arai et al., 1999). A bimodal distribution characterised by IPGE-rich and PPGE-rich chromitites is also known for the compositional tendency of the platinum-group elements. IPGE-rich chromitites are formed in the deeper mantle and PPGE-rich chromitites in the uppermost part of the mantle, near the Moho transition zone (Ferrario and Garuti, 1990; Leblanc, 1991; Garuti et al., 1995; 1999a, 1999b; Ahmed and Arai, 2003). PGM from the depleted ophiolites tend to be rich in IPGE whereas PPGE-rich PGM originate from the host rock by partial melting of the upper mantle. PPGE tend to concentrate in magma because of their incompatible behaviour with IPGE (Matsumoto, 1928; Leblanc, 1991; Nakagawa et al., 1991; Ahmed and Arai, 2003; Arai, 2012).

Platinum-group elements have a siderophile nature (Goldschmit, 1937), and tend to be strongly fractionated into sulfide phases. They are potentially useful indicators of the degree of partial melting in the mantle and the partial pressure of sulfur saturation in the melt (e.g. Arculus and Delano, 1981; Arai et al., 1999). Because the composition of a laurite–erlichmanite solid-solution series should be influenced strongly by temperature and sulfur fugacity, $f_{S_2}$ (Brenan and Andrews, 2001; Andrews and Brenan, 2002; Bockrath et al., 2004), each individual inclusion may record valuable information on the thermodynamic conditions prevailing during its crystallisation (Augé and Johan, 1988; Nakagawa and Franco, 1997; Garuti et al., 1999a). Detailed mineralogical investigations into the physical properties and structures of PGM solid solutions are required, in addition to petrological observations to understand this system.

Crystal structure of laurite (RuS₂) and erlichmanite (OsS₂)

Laurite and erlichmanite have the same pyrite-type structures. Both covalent and ionic bonds have been identified in pyrite-type compounds, and both high- and low-spin states have been observed in transition-metal pyrite-type compounds under ambient conditions (Elliot, 1960; Folmer et al., 1988; Tokuda et al., 2019). Many stable platinum-group chalcogenides have a pyrite-type structure (Furuseth et al., 1965; Sutarno et al., 1967; Stassen and Heyding, 1968), in addition Ru and Os belong to the same family as Fe in the periodic table. Pyrite (FeS₂) is a diamagnetic semiconductor in which Fe ions exist in a low-spin divalent state (Elliot, 1960; Folmer et al., 1988); cattierite (CoS₂) has a pyrite-type structure (Nowack et al., 1991); the existence of pyrite-type RhS₂ is unconfirmed, though data have been proposed for the unit-cell parameter of hypothetical RhS₂ (Thomassen, 1929; Hulliger, 1964); and pyrite-type IrS₂ has been synthesised under high pressures (Munson, 1968). Rhodium, a homologous element of Co and Ir, can have various valences and low- or high-spin states. The second and third transition metal cations in the complexes are usually in a low-spin state.

Lutz et al. (1990) and Stingl et al. (1992) refined the structures of synthetic RuS₂ and OsS₂ end-members, respectively, using single-crystal diffraction experiments. There are few detailed studies on the variation of crystal structure with composition in the laurite–erlichmanite system. In natural specimens, substitutions of various additional elements such as Ir, Rh, Pd and Pt occur simultaneously; therefore, no systematic study of the structure of the solid solution has been conducted.

Minerals with Rh-rich and Ir-poor laurite–erlichmanite solid-solution series compositions have recently been discovered as inclusions of isoferroplatinum (Pt₃Fe) from a mantle-derived ultramafic massif in PPGE-type placers in Kumamoto, Japan (Nishio-Hamane et al., 2019). Erlichmanite and laurite are the most abundant inclusions in isoferroplatinum (Pt₃Fe), they form a wide range of solid solutions between Os and Ru, and a certain amount of Rh is also substituted in most cases. The solid-solution samples from this area form suitable crystals for investigating the effect of only Rh on the RuS₂–OsS₂ crystal structures.

Debye–Waller factors can be used for determining the quantitative changes in the vibration characteristics of materials. The Debye temperature Θ_D for each atom at a crystallographically independent site can be estimated using the dynamic component of the Debye–Waller factor based on the Debye approximation (Willis and Pryor, 1975; Wood et al., 2002; Yoshiasa et al., 2016; Yoshiasa et al., 2021). The Debye temperatures are related to the mechanical and thermal properties of the materials; hence the Debye temperature is one of the physical quantities that can be compared for materials with different compositions. Occurrences such as the crystallisation order of mantle minerals can be discussed based on the Debye temperature.

Herein, the crystal structures of the pyrite-type Os_1–x–yRu_xRh_yS₂ solid solutions (x = 0.09–0.60 and y = 0.07–0.10) were refined using natural samples from Haraigawa, Misato-machi, Kumamoto, Japan. We found a unique compositional dependence of the unit-cell parameters and S–S distances in the pyrite-type solid solution. When Os (not Ru) is replaced by Rh, an interesting phenomenon occurs in the solid-solution crystals. The substitution of Os by Rh in erlichmanite (OsS₂) has a significant effect on cell size, bonding distances and vibrational properties. This seems to be controlled by the structural requirements. Under structurally restricted environments in solid solutions, the electronic state of Rh as a subcomponent in solid solutions is different from that of the end-member pure crystal.

One of the aims of this study is to elucidate the nature of the unique chemical bonding state observed in the pyrite-type structure. Even in the well-known pyrite-type platinum-group element compounds, there are many unsolved themes in terms of chemical bonding state and ionic radii. Another aim is to clarify the mineralogical significance of accessory minerals and the important roles of minor elements on crystals. Unique and diverse effects of minor and subcomponent ions on the structural and physical properties have been observed in the Rh-bearing erlichmanite–laurite solid-solution system.

Experiments

Specimens and chemical analyses

The compositional characteristics of PGM found in a small stream crossing the clinopyroxenite mass at Haraigawa, Kumamoto, Japan (32°34' 15''N, 130°47'25''E) were consistent with those of the PPGE-rich placer (Nishio-Hamane et al., 2019). The placer specimens were collected by panning the river sand from Haraigawa. Almost all grains were predominantly isoferroplatinum with diverse PPGE-based PGM. The grain size was generally <1 mm and rarely exceeded 2 mm. The PPGE-rich PGM in this region has been reported to be derived from the host rock owing to the partial melting of the upper mantle (Nishio-Hamane et al., 2019). Ultramafic rocks are exposed along the Kurosegawa belt in central Kyushu, and clinopyroxenite, in association with serpentinite, is distributed in the Tomochi area of Kumamoto (Kanmera, 1952; Saito et al., 2004, 2005). Clinopyroxenite is regarded as a cumulate that developed at the bottom of the magma chamber and is generated by partial melting of the upper mantle. Osanai et al. (2014) considered that clinopyroxenite in the serpentine mélange of the Kurosegawa belt was formed by the accumulation of crystals at the base of a magma chamber under the mid-ocean ridge.

The isoferroplatinum from Haraigawa has a composition close to the ideal Pt₃Fe composition and commonly contains a small amount of Pd. Isoferroplatinum rims often coexist with tulameenite (Pt₂CuFe) and tetraferroplatinum (PtFe). Isoferroplatinum-based grains are accompanied by the recently discovered mineral minakawaite (RhSb) and various PGM grains, such as laurite–erlichmanite solid-solution minerals, osmium (Os), bowieite (Rh₂S₃), kingstonite (Rh₃S₄), miassite (Rh₁₇S₁₅), cherepanovite (RhAs), hollingworthite (RhAsS), cuprorhodsite [(Cu,Fe)Rh₂S₄] and irarsite (IrAsS) (Nishio-Hamane et al., 2019). Grains with laurite–erlichmanite solid-solution compositions are the most abundant sulfide inclusions in isoferroplatinum-based grains and have subhedral or rounded forms, occasionally with a clear zonal texture. Bowieite (Rh₂S₃) is the second-most abundant sulfide inclusion in isoferroplatinum (Pt₃Fe) (Nishio-Hamane et al., 2019). A characteristic of the placer from Haraigawa is that it is rich in Rh and produces a variety of Rh minerals.

The major and minor elements of Ir-poor laurite–erlichmanite grains with sizes ranging from several tens to ~100 micrometres were analysed using a JEOL scanning electron microscope (SEM, JSM–7001F operated at 15 kV and 1.0 nA) equipped with an Oxford energy dispersive X-ray spectroscopy at Kumamoto University, Japan. Corrections were made using the Aztec Oxford software. Quantitative analyses have been confirmed for many laboratory-standard compounds including synthetic platinum-group compounds such as RuSe₂, Rh₂S₃, PdSb₂ and PtP₂. The deviation from 100 wt.% in total and from the ideal number of the chemical formula obtained by specifying the number of anions was <0.5%. Relative analytical errors (1σ) of standard compounds were generally better than ~1%. Elements containing >0.1 wt.% can be detected, but the quantification is poor for elements with content <1 wt.%.

A back-scattered electron image and element-distribution maps in Fig. 1 show a rounded subhedral laurite–erlichmanite solid-solution grain from Haraigawa surrounded by tulameenite (Pt₂CuFe) and isoferroplatinum (Pt₃Fe). The laurite–erlichmanite samples from Haraigawa contained very low amounts of Ir, Pd, or Pt, and we searched for areas containing no, or the lowest Ir or Pd compared with Rh as additional components. The variation in Ru and Rh components with respect to the change in Os content in eight solid-solution grains are shown in Fig. 2.

Figure. 1. Back-scattered electron image and element distribution maps of grain with an erlichmanite–laurite solid-solution composition surrounded by tulameenite and isoferroplatinum.

Figure. 2. Variation in Ru and Rh contents vs. Os (atoms per formula unit, apfu) in the erlichmanite–laurite Os_1–x–yRu_xRh_yS₂ solid-solution series. There is no solid solution in the area shown in grey because both Ru and Rh are substituted. Red circles represent the contents of Rh at both end-members estimated by the red dashed line.

The grains contain zoning and only small areas (several tens of μm) for each crystal were homogeneous. Single crystals were carefully selected for crystallography from homogeneous areas that contained almost no Ir. The variation in the atomic ratios of Ru, Os and Rh among the analysed points in each area was within ~1–4% (Table 1). Other elements such as Pd, Pt, As and Se were below the detection limit in each area.

Table 1. Chemical compositions of the erlichmanite–laurite solid-solution specimens used for single-crystal structure analyses.

n.d. – not detected

Single-crystal X-ray diffraction and structure refinement

Structural analyses were performed on four single crystals (Table 2). Crystallographic data were collected on an Rigaku XtaLAB Synergy diffractometer with a HyPix6000 area detector. Systematic absences were found to be consistent with the space group $Pa {\overline {3} }$, and no evidence of lower symmetry was detected in any of the four crystals. The intensity of the reflection was measured using MoKα radiation (0.71073 Å) focused by a mirror. The details of the data-correction method are described in the crystallographic information files, deposited with the Principal Editor of Mineralogical Magazine and are available as Supplementary material (see below). Independent reflections were used to refine the crystal structure using the full-matrix least-squares method in the SHELXL program (Sheldrick, 2015). The refinement was initiated with the positional parameters reported by Stingl et al. (1992). Because it is difficult to distinguish between Ru and Rh from each X-ray scattering factor, the Rh value was fixed using its average chemical analysis value, and the site occupancies of Ru and Os atoms were refined at the M site of each crystal. The ratio of Ru and Os at the M sites was refined by assuming that the rest of Rh is occupied by both Ru and Os. The following chemical formulas for the four crystals were determined from the site-occupancy refinements: Os_0.332(4)Ru_0.601(4)Rh_0.067(8)S₂; Os_0.457(3)Ru_0.467(3)Rh_0.076(7)S₂; Os_0.595(5)Ru_0.325(5)Rh_0.080(5)S₂; and Os_0.812(6)Ru_0.092(6)Rh_0.096(4)S₂. Each chemical formula obtained from site-occupancy refinement was in good agreement with the respective values obtained from chemical analysis (Table 1). The R ₁ indices (R ₁ = Σ||F _o| − |F _c||/Σ|F _o|) for the four crystals converged between 0.0071 and 0.0096, using anisotropic temperature factors. The structural refinement data and selected interatomic distances are listed in Tables 2 and 3, respectively.

Table 2. Crystallographic data, data collection parameters, and refinement parameters for Os_1–x–yRu_xRh_yS₂ pyrite-type erlichmanite–laurite solid solution from Haraigawa, Kumamoto.

Table 3. Selected bond distances and bond-angle variance for MS₂ pyrite-type Os_1–x–yRu_xRh_yS₂ erlichmanite–laurite solid solution.

Results and discussion

Rh substitution for Ru and Os in the erlichmanite–laurite solid solutions

The erlichmanite–laurite solid-solution samples from Haraigawa are characteristically Rh rich and Ir poor. The solid solutions from Haraigawa are unique in that trace amounts of Cu up to 0.85 wt.% are substituted in some crystals rich in Os (Table 1). No significant substitutions of Pd and Pt were observed in the examined grains, despite the coexistence of Pd and Pt minerals (Nishio-Hamane et al., 2019) contrary to reports by Bowles et al. (1983) that significant amounts of Pd and Pt are dissolved.

Rhodium is present in all erlichmanite–laurite samples from Haraigawa, and its content ranges from 0.07 to 0.10 apfu (y = 0.07–0.10 in Os_1–x–yRu_xRh_yS₂). For this reason, Os and Ru contents are limited in a range between Os_0.18Ru_0.75Rh_0.07S₂ with minimum Os and maximum Ru and Os_0.82Ru_0.08Rh_0.10S₂ with maximum Os and minimum Ru (grey shading in Fig. 2). Note that in erlichmanite component-rich solid solutions with Os > 0.80 apfu and Ru < 0.10 apfu, Rh predominates over Ru (red circle, Fig. 2). The results in the present study and the published data on the Rh-rich erlichmanite–laurite solid solution (e.g. Begizov et al., 1976) suggest that Rh tends to substitute for Os slightly more than Ru.

Compositional dependence of the unit-cell parameter

The unit-cell parameters and u parameters (u,u,u coordinate for the S atom) of the synthesised pure RuS₂ and OsS₂ are 5.6106(3)Å, 0.38831(4) (Lutz et al., 1990) and 5.6194(7) Å, 0.38616(6) (Stingl et al., 1992), respectively. Sutarno et al. (1967) also reported the unit-cell dimensions and u parameter (5.6095(5) Å, 0.3885(7) for RuS₂ and 5.6196(3) Å, 0.3864(13) for OsS₂) using the powder X-ray diffraction method. In the cases where Vegard's relationship holds, a linear composition dependence of the unit-cell parameter is observed. The unit-cell parameter of the Rh-free Os_1–xRu_xS₂ solid solution can be expressed as:

$$[ {a\;( { {\ring {\rm A} }} ) } ] ^{{\rm O}{\rm s}_{1\ndash x}{\rm R}{\rm u}_x{\rm S}_2} = x[ {a\;( {\ring {\rm A} ) } ] ^{{\rm Ru}{\rm S}_2} + ( {1\ndash x} ) \cdot [ {a\;( {\ring {\rm A} ) } ] ^{{\rm O}{\rm S}_2}$$

Osmium and Ru are assumed to differ in size and exhibit constant atomic/ionic radii (Denton and Ashcroft, 1991). Hence adding a constant amount of larger ions leads to an increase in the unit-cell parameter, approximately parallel to the straight line of Vegard's relationship.

The unit-cell parameters for the Rh-bearing erlichmanite–laurite solid solutions from Haraigawa change from 5.61826(6) Å to 5.63142(8) Å (Fig. 3a and b), which is very different from the change expected from Vegard's law using the data of these end-member components. On the RuS₂-rich side, (v = 0 or w = 1), although the Rh content was ~7 at.%, the projected data line shows no large change in the unit-cell parameter. On the OsS₂-rich side, a much larger increase in the unit-cell parameter was observed than expected by Vegard's law. Although the Rh substitution of Os has a significantly different effect on the crystal structure than that of Ru substitution, a linear relationship was observed in Fig. 3a and b.

Figure. 3. Compositional dependences of unit-cell parameters: (a) v in (Ru,Rh)_1–vOs_v and (b) w in (Os,Rh)_1–wRu_w in the erlichmanite–laurite Os_1–x–yRu_xRh_yS₂ solid solutions (x = 0.09–0.60 and y = 0.07–0.10), as orange diamonds. The end-members of both series (v = 0, RuS₂, v = 1, OsS₂; and w = 0, OsS₂, w = 1, RuS₂) are shown for comparison. Data from: White triangles – Sutarno et al. (1967); black square – Lutz et al. (1990); and red circle – Stingl et al. (1992). The red dashed line shows the change expected from Vegard's Law for Os_1–xRu_xS₂ solid solutions.

The composition without Os of (Ru_0.95Rh_0.05)S₂ can be estimated from extrapolation in Fig. 2 (the extrapolated values of Ru and Rh crossing the point Os = 0) and without Ru of (Os_0.90Rh_0.10)S₂ (the extrapolated value where the Rh line crosses the x axis, where Ru = 0). Then the effect of the Rh content on the unit-cell parameters in (Ru_0.95Rh_0.05)S₂ can be estimated by extrapolating the best fit line for the solid-solution samples (diamonds) in Fig. 3a to Os = 0.0 (v = 0). This gives the value of 5.609 Å which is thus due to 5 at.% Rh in RuS₂ i.e. (Ru_0.95Rh_0.05)S₂, which is 0.0016 Å smaller than that (5.6106(3) Å) for pure RuS₂. Applying Vegard's law (0.0016 Å is multiplied by 20.0, and subtract from that for RuS₂) gives a = 5.579 Å for the hypothetical RhS₂. This value is close to the value of 5.58 Å given previously for pyrite-type RhS₂ by Thomassen (1929).

The value of 5.633 Å due to (Os_0.90Rh_0.10)S₂, i.e. 10 at.% Rh in OsS₂ can be estimated by similar extrapolation of the compositional changes to Ru = 0.0 (w = 0) in Fig. 3b, and it differs by 0.0136 Å from that (5.6194(7) Å) for pure OsS₂. If Vegard's law is applied (0.0136 Å is multiplied by 10.0, and added to that for OsS₂), a = 5.755 Å is obtained as the unit-cell parameter of hypothetical pure RhS₂. This value is as large as the unit-cell parameter of 5.73 Å for the hypothetical pyrite-type RhS₂ phase derived by Hulliger (1964). Conflicting unit-cell parameters have been reported for RhS₂ (Thomassen, 1929; Hulliger, 1964). No single-crystal structure analysis of pyrite-type RhS₂ has been reported so far. Pyrite-type RhS₂ has not been observed in high-temperature regions under normal pressure (Parthé et al., 1967; Foise et al., 1983). Pyrite-type RhSe₂ (a = 5.9336(4); Geller and Cetlin, 1955) and CoS₂ (a = 5.5385(2); Nowack et al., 1991) exist, and pyrite-type IrS_1.9 has been synthesised (a = 5.68 Å) at 6 GPa and 1500°C by Munson (1968). Many stable platinum-group chalcogenides have pyrite-type structures (Furuseth et al., 1965; Sutarno et al., 1967; Stassen and Heyding, 1968; Tokuda et al., 2019).

Structure distortion and S–S distance in Os_1–x–yRu_xRh_yS₂ solid solution

Laurite and erlichmanite have S₂ sub-units ($\rm S_2^{2\ndash }$, disulfide ion). The pyrite-type structure MS₂ is closely related to the NaCl-type structure. The S₂ groups (connected by covalent bonds) and M cations in the pyrite-type structure are arranged in the anion and cation sites of the NaCl-type structure, respectively. M cations form a face-centred cubic sublattice (Fig. 4). The M cation is bound to six S atoms in the six S₂ groups. The S₂ groups are arranged along the three-fold rotoinversion axes of a lower class of cubic symmetry (the space group Pa $\bar{3}$), which run in the direction of the body diagonals of the cell and do not intersect each other. Thus, the pyrite-type structure of MS₂ is a three-dimensional assembly of corner-sharing MS₆ octahedra, wherein the M cation is bound to only one S ion in the S₂ group, and each S anion is common to three octahedra.

Figure. 4. (a) The crystal structure of Os_0.81Ru_0.09Rh_0.10S₂ erlichmanite, viewed parallel to the a-axis. The S–S bond is shown in the middle of the unit cell. (b) The (S₂)M ₆ octahedron around S₂ and M cations are located at the face-centred cubic sub-unit. Each S ion bonds with one S ion (S–S: 2.2613(4) Å) and three M cations (M–S: 2.3517(3) Å). Atomic displacement ellipsoids are drawn for the 99% probability level. The crystal structures were illustrated using VESTA (Momma and Izumi, 2011).

The polyhedron around the X₂ group forms an undistorted (X₂)M ₆ octahedron (Fig. 4b). Cation M is located at the origin of the face-centred cubic sub-unit and has no degrees of freedom with respect to its atomic coordinates. Interestingly, this octahedron has an unusually high symmetry as a local structure, and exhibits no structural relaxation owing to electrostatic repulsion. The S anion site is at the Wyckoff position 8c, and the atomic coordinates of the S anion are specified by a single u parameter (u, u, u coordinates). The S anions possess a degree of freedom only in the direction of the body diagonals, that is, in the (u, u, u) direction. In pyrite-type compounds, the arrangement of atoms for structural optimisation must be achieved using only two parameters, the unit-cell parameter a and the parameter u. The unit-cell parameter a increases whereas the u parameter decreases with increasing Os contents in the solid solutions (Table 1).

Owing to the symmetry of the S site, the freedom of atomic coordinates (u parameter) of the S atoms is limited to vary only along the three-fold rotoinversion axis. For the same unit-cell parameters, the M–S distance decreased, and the molecular distance S–S increased as the u parameter decreased. Hence, a decrease in the u parameter leads to a decrease in the (M–S)/(S–S) ratio. Decreasing the u parameter also leads to an increase in the long(S⋅⋅⋅S)/short(S⋅⋅⋅S) ratio. Similarly, compounds with smaller u parameters exhibit an increased angle variance for the MS₆ octahedron (Tables 2, 3). The differences between the long S⋅⋅⋅S distance and the short S⋅⋅⋅S or between the narrow S–M–S angle and the wide S–M–S angle increase with increasing Os content (Table 3). The FeS₆ octahedra in FeS₂ pyrite ((Fe–S)/(S–S) = 1.0475(5), long(S⋅⋅⋅S)/short(S⋅⋅⋅S) = 1.0791, S–Fe–S(1) = 85.64(4)° and S–Fe–S(2) = 94.36(4)°; Tokuda et al. (2019) had exactly the same distortion (Table 3) as that in the Os_0.60Ru_0.32Rh_0.08S₂ solid solution from Haraigawa samples.

Owing to the low degree of freedom for structural changes, crystal stabilisation involves a unique chemical bonding state with higher energy, which is rare in other structures. In many cases, unusual chemical bonding occurs to achieve a three-dimensional periodicity and acceptable M–S distances. In pyrite, all electrons in the low-spin state Fe²⁺ occupy t _2g orbitals (S = 0), which is a biased electronic arrangement. In terms of transition metal ions, the energy of the low-spin state is always higher than that of the high-spin state. The number of electrons in the d-orbital increases to d ⁶, d ⁷ and d ⁸ across the first transition metal period for Fe²⁺, Co²⁺ and Ni²⁺ respectively. The radii of Fe²⁺ and Co²⁺ in the low-spin state are 0.61 and 0.65 Å, respectively (Shannon, 1976). The size difference between Fe²⁺ and Co²⁺ is 0.04 Å. In sulfides, the bond between each cation and the sulfide ion has both ionic and covalent characteristics, and the spin state of the transition metal ion can change. For Rh, Rh and Os, ionic (Pauling, 1940; Shannon et al., 1981), covalent (Pauling, 1940; Cordero et al., 2008) and metallic (Pauling, 1940) radii have been proposed corresponding to their respective bonding states. Size differences of 0.006 to 0.04 Å are expected among these elements, even though their bonding characteristics are similar. Curiously, the M–S distances in the solid-solution minerals from Haraigawa showed little variation (Fig. 5; Table 3). The chemical bonding characteristics of the atoms cannot be interpreted from the published interatomic distances.

Figure. 5. Compositional dependences in M–S and S–S bonding distances with respect to Os content in the erlichmanite–laurite solid solutions (Os_1–x–yRu_xRh_yS₂) (y = 0.07–0.10). Both series end-members are shown with dark symbols.

The compositional dependence of the M–S and S–S distances in the Rh-bearing erlichmanite–laurite solid solution from Haraigawa, and those of pure RuS₂ (Lutz et al., 1990) and OsS₂ (Stingl et al., 1992) are shown in Fig. 5. A unique compositional dependence of the distances was observed as a structural change in the solid solution. The unit-cell parameters of pure RuS₂ and OsS₂ are different (Fig. 3). However, the M–S distances in the Os_1–x–yRu_xRh_yS₂ system, including the solid solutions containing Rh, changed by only 0.001 Å (Table 3). In contrast, the S–S distances change significantly, and the difference between them reaches 0.1 Å. These changes are essential phenomena that occur in this system, even when comparing the values of pure RuS₂ and OsS₂. Moreover, the unique feature of this solid solution containing ~10 at.% Rh is the peculiar increase in the S–S distance in the solid solution containing a substantial amount of Os (Fig. 5). The elongations of the S–S distance are not very large in Ru-rich solid solutions (Os_0.33Ru_0.60Rh_0.07S₂), although they contain 7 at.% Rh (Table 3). In Fig. 5, a linear relationship between the S–S distance and the change in Os content can be seen in the Os_1–x–yRu_xRh_yS₂ solid solution (y = 0.07–0.10). A negligible effect of Rh substitution in RuS₂ was observed with respect to the S–S distance, whereas the effect of Rh substitution on OsS₂ was extremely large. The Rh substitution of Ru in RuS₂ and the Rh substitution of Os in OsS₂ have very different effects on the S–S distance, that is, on the chemical bonding characteristics of the anions. The change in covalent character at the S–S bond seems to eliminate the size effect of the cation itself.

Because the M–S interaction is greater for low-spin compounds, S may be attracted strongly to low-spin metals. The longer S–S distance implies a decrease in covalent character at the S–S bond in pyrite-type MS₂ compounds. The delocalisation of the electrons in the S₂ group weakens the molecular S–S bond.

The S–S bond in NiS₂ (2.065 Å, Nowack et al., 1991), which is the minimum among pyrite-type sulfides, is almost equivalent to the S–S single bond (2.06 Å) in a disulfur molecule (S₂) and the S–S distance (2.055(2) Å) in elemental solid sulphur (Rettig and Trotter, 1987). The S–S distance in RuS₂ (2.1707(8) Å, Lutz, 1990) is even longer than the S–S distance in NiS₂, which demonstrates an increase in ionicity and a decrease in covalent bonding character in S–S bonds. The S–S distance in OsS₂ (2.2160(12) Å, Stingl et al., 1992) is significantly larger than that in RuS₂ (2.1707(8) Å), FeS₂ (2.1618(9) Å, Tokuda et al., 2019) and CoS₂ (2.124 Å, Nowack et al., 1991). In OsS₂, the ionicity of the S–S bond further increased compared to that of FeS₂ and RuS₂. The S–S distance was even longer in the Os_0.81Ru_0.09Rh_0.10S₂ erlichmanite than in OsS₂ (Fig. 5). The molecular S–S distances in the laurite–erlichmanite solid solutions were longer than those in other pyrite-type compounds (Tokuda et al., 2019). The M–S distances in OsS₂ (2.3510(5) Å) and RuS₂ (2.3520(3) Å) are the same, and the Fe–S distance in FeS₂ was 2.264 (1) Å. A unique change in the bonding distances occurs in pyrite-type sulfides of Fe, Ru and Os belonging to the same group in the periodic table. The (M–S)/(S–S) ratios decreased with increasing Os content, as listed in Table 3, suggesting a corresponding decrease in the bond strength of the S₂ groups. The increase in electron transfer from cations to S–S molecules in the Os_0.81Ru_0.09Rh_0.10S₂ erlichmanite is one of the factors responsible for the elongation of the S–S bond distances. Furthermore, the fact that the M–S distance does not change even when the neighbouring group is replaced by 10 at.% Rh indicates a change in the bonding characteristics of the Os–S, Ru–S, and Rh–S bonds from the standard states. Thus, we conclude that the factor that lowers the total energy in pyrite-type crystals is mainly controlled by anions.

Debye–Waller factor and static disorder in the solid solutions

The Debye–Waller factors obtained from the diffraction experiments include the effects of static and dynamic disorders. Static disorder is a configurational disorder, whereas dynamic disorder arises from the thermal vibrations of atoms. In the case of a solid solution, a static disorder component is always included. The compositional dependence of the Debye–Waller factor U ₁₁ in solid solutions from Haraigawa is shown in Fig. 6 as it increases with an increase in the Os component (Fig. 6; Table 2). It can be seen that the effect of Rh on the Debye–Waller factor is different for Os and Ru substitutions. A large increase in the Debye–Waller factor occurs at 10 at.% Rh in Os-rich solid solutions, whereas no large effect occurs even at ~7 at.% Rh in Ru-rich solid solutions (Table 2). In the Os_0.81Ru_0.09Rh_0.10S₂ erlichmanite, U ₁₁ (0.00319(4) Ų) for the M site increases by 0.0011 Ų and U ₁₁ (0.00480(9) Ų) for the S site increases by 0.0019 Ų compared to those for pure OsS₂ (0.00205(3) Ų and 0.00288(6) Ų, respectively, black symbols). The root-mean-square displacements (RMSD) for M and S sites (0.0565 Å and 0.0693 Å) in Os_0.81Ru_0.09Rh_0.10S₂ increase by 0.0112 Å and 0.0156 Å, respectively. The amplitude due to the thermal vibration is dominant in pure OsS₂. The value of 0.0156 Å is approximately a quarter of the RMSD (0.0537 Å) for the S atom in OsS_2. This value is approximately one-third of the increase in the S–S distance (0.045 Å) observed in the solid solution. We conclude that the increase in the S–S distance by the Rh replacement of Os with only several percent in the Rh-bearing erlichmanite–laurite solid solutions reflects the change in S–S bonding characteristics throughout the crystal. The substitution of some Os by Rh affects all (Os, Ru, Rh)–S bonds.

Figure. 6. Variation of Debye–Waller factor U ₁₁ against Os component in the erlichmanite–laurite Os_1–x–yRu_xRh_yS₂ solid solutions (y = 0.07–0.10). End-members RuS₂ and OsS₂ (0.00205(3) Ų M site and 0.00288(6) Ų S site) are shown with dark symbols.

Atomic displacement ellipsoids of the M (M: Os_0.81Ru_0.09Rh_0.10) and S sites in the Os_0.81Ru_0.09Rh_0.10S₂ erlichmanite are shown in Fig. 4. The atomic displacement ellipsoids of both M and S are effectively spherical, and no elongation along the M–S and S–S bond directions was observed. Based on the site-symmetry restrictions, U ₁₂, U ₁₃ and U ₂₃ are equivalent; therefore, changes to these parameters results in the distortion of the atomic displacement ellipsoid along [111], which is parallel to the S–S bond. The U ₁₂ parameters of the S sites in MS₂ have the same positive value of ~0.0002 Ų for all anions, and the degree of deformation from a sphere is slightly larger in Os_0.81Ru_0.09Rh_0.10S₂ erlichmanite. The atomic displacement ellipsoids for S are almost spherical (U ₁₂ = 0 indicates a sphere), implying that few static atomic displacements in specific directions are observed in the solid-solution system.

Debye temperature of erlichmanite–laurite solid solution and effect of Rh substitution

The Debye temperature Θ_D corresponding to the atom at each crystallographically equivalent site can be estimated using the dynamic part of the Debye–Waller factor based on the Debye approximation (Willis and Pryor, 1975; Wood et al., 2002; Yoshiasa et al., 2016; Yoshiasa et al., 2021). We estimated Θ_D for each atom in the site occupied solely by Ru, Os, or S using the values of U ₁₁ under the assumption of no configurational disorder of the atoms in pure RuS₂ and pure OsS₂. The values of Θ_D calculated for Ru and S in RuS₂ are 434 K and 661 K, respectively, using the data obtained by Lutz et al. (1990). The Θ_D values for Os and S in OsS₂ are 335 K and 688 K respectively, using data from Stingl et al. (1992). The Debye temperature for the site with the highest value in the crystal corresponds to the bulk Debye temperature of the compound (Tokuda et al., 2019; Yoshiasa et al., 2021). The bulk Debye temperatures Θ_D for RuS₂ and OsS₂ are estimated to be 661 and 688 K, respectively. These values are extremely high among the many known sulfides. The Debye temperature is a physical index that can be used to compare materials with different compositions. The Debye temperature is related to the melting temperature, formation temperature, thermal conductivity, atomic diffusion and hardness of the materials. Laurite (RuS₂) is the best-known hard sulfide mineral (hardness = 7.5). Bowles (1983) showed that the microindentation hardness of the laurite–erlichmanite solid-solution minerals are considerably higher than that of other sulfide minerals. González-Jiménez et al. (2009) attributed the preservation of laurite–erlichmanite zoning to the low diffusion coefficient of Ru and Os in pyrite-type structures. The high hardness and low diffusion coefficient of the laurite–erlichmanite series minerals are in good agreement with the calculated Debye temperatures.

The Debye temperatures of RuS₂ (661 K) and OsS₂ (688 K) are similar to those of the upper mantle constituent silicate end-member minerals, such as diopside (668 K; Levien et al., 1979), enstatite (732 K; Weidner et al., 1978), forsterite (758 K; Sumino et al., 1977) and fayalite (507 K; Sumino, 1979). Some laurite–erlichmanite grains occurring in sealed inclusions of chromite are of magmatic origin. The euhedral shape of the laurite crystals and their sporadic distribution even within the same pod strongly suggest that laurite represents a high-temperature phase on the liquidus of primitive magma (Ahmed and Arai, 2003; Arai, 2012). In particular, laurite acts as a nucleus for the early formation of chromian spinel and silicate crystals (Tredoux et al., 1995). Laurite–erlichmanite minerals form at a very high temperature (～1250°C; Brenan and Andrews, 2001). Our synthetic experiments without flux under vacuum conditions (Unoki et al., 2021; Yoshiasa et al., 2021) indicated that RuS₂ and RuSe₂ crystals did not melt nor grow, even at 1300°C.

Several researchers (Stockman and Hlava, 1984; Augé and Johan, 1988; Melcher et al., 1997; Garuti et al., 1999a; Brenan and Andrews, 2001) have reported that a progressive increase in the Os content of laurite on crystallisation (Os solubility in laurite) has the effect of both lowering temperature and increasing $f_{S_2}$. Laurite is composed of nearly pure RuS₂ with low concentrations of Os and Ir at high temperatures and low $f_{S_2}$ (Brenan and Andrews, 2001). When $f_{S_2}$ increases in the melt, laurite–erlichmanite solid solutions are formed by the reaction of Ru and Os components in magma, or previously formed Ru–Os alloys with sulfur. Bockrath et al. (2004) considered that the origin of zoning in the solid solution was interpreted mainly as a result of changes in $f_{S_2}$ and, to a lesser extent, in melt temperature. The bulk Debye temperature of OsS₂ is higher than that of RuS₂. This indicates that the melting temperature of erlichmanite is higher than that of laurite. The melting points of Ru, Os, Rh and Ir are 2310, 3045, 1965 and 2410°C, respectively. The high melting point of Os metal and the high Debye temperature of OsS₂ do not explain the crystallisation of Ru-rich solid solutions and RuS₂ before those of the Os-rich solid solutions and OsS₂. We therefore conclude that $f_{S_2}$ is the main cause of laurite crystallisation prior to erlichmanite.

Contrary to the expectations from the position of the elements in the periodic table, the substitution of Ir is preceded by Ru compared to Os in petrological observations. This also depends on the effect of $f_{S_2}$. Because Ru is more easily sulfurised than Os and crystallises faster, Ir can be incorporated into laurite. Various Rh-rich minerals, such as Rh₃S₄ and Rh₂S₃, which show the diversity of $f_{S_2}$, are observed along with the laurite–erlichmanite solid-solution minerals in samples from Haraigawa. It is very likely that there were fluctuations in $f_{S_2}$ in the magma chamber. Precipitation of PGE arsenide and PGE antimonide was also observed (Nishio-Hamane et al., 2019). The $f_{S_2}$ value in the magma increases as silicate crystals accumulate. The increase in $f_{S_2}$ appears to induce the precipitation of Rh-rich platinum-group sulfide minerals. In the Haraigawa samples bowieite (Rh₂S₃) is the second most abundant sulfide inclusion, and irarsite (IrAsS) is also commonly observed in isoferroplatinum (Pt₃Fe).

The estimated values of 523–580 K (Fig. 7; Table 2) for the solid solutions are sufficiently large Debye temperatures for sulfides. The true bulk Debye temperature for solid solutions should be higher than the estimated value because the static effect increases the Debye–Waller factor and reduces the Debye temperature value. The Debye–Waller factors U ₁₁ and Debye temperatures of the solid solutions (Figs 6 and 7) exhibit a trend including pure RuS₂, however extrapolation of each trend deviates significantly from its respective value of OsS₂ (dark symbols in Figs 6 and 7). Substitution of Ru by Os with 10 at.% Rh significantly lowered the Debye temperature by as much as 170 K. Our comparison of the Debye temperature of each end-member indicated that the melting temperature of erlichmanite (688 K) is higher than that of laurite (661 K). On the other hand, the results show that the presence of several percent Rh lowers the melting point of erlichmanite solid solution compared to that of the laurite solid solution. The present study revealed a significant effect of the minor component Rh on the thermal stability of OsS₂ and that these effects were significantly different between OsS₂ and RuS₂. It is shown that the effect of a minor element on physical properties is different even in the same structure with similar composition. In the erlichmanite–laurite solid-solution system, a small amount of Ir may also have some effect on physical properties.

Figure. 7. Debye temperatures vs. Os component of cation and sulfide ion sites in the erlichmanite–laurite Os_1–x–yRu_xRh_yS₂ solid solutions (y = 0.07–0.10). End-members are shown with dark symbols.