Given a sequence a(l), a(2), a(3), … of complex numbers such that a(n) ≤ 0(nc) for some c > 0, we define, for Im(z) > 0,

where q(λ) = exp (2πiz/λ), λ > 0 and a is a real number. Throughout this paper, for complex numbers x, w with x ≠ 0, xw = exp (w log x) and the principal branch is taken for the logarithm. Then it is easily verified that the infinite product converges absolutely and uniformly in every compact subset of the upper half plane H. Hence f(z) is holomorphic in H. The aim of this paper is to determine holomorphic functions in H defined by (1) which satisfy the special transformation formula

for some real number k under certain assumptions.

Let Φ : L → ℤ be a positive definite even unimodular quadratic form, L ≅ ℤn; we put f(x) = Φ(x)/2 and call (L, f) a lattice, for short. Let min f be the minimum of the numbers f(x) ≠ 0. Fixing n (a multiple of 8), one is interested in the largest possible minimum. It follows from the theory of modular forms (cf. Sloane [6]) that

Let an be a non-increasing real sequence such that 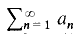converges; then clearly an ↓ 0. We shall ignore the trivial case where an = 0 for all large n, and so we assume that an > 0 for all n, from now onwards. In [1] J. B. Wilker introduced certain new sequences associated with the rate of convergence of 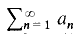, and obtained various relations between them, in order to investigate packing problems in convex geometry. Let us define

We write P, Q and T respectively for the inferior limits of pn, qn and tn, and P1, Q1 and T1 for the corresponding superior limits. Further, we put

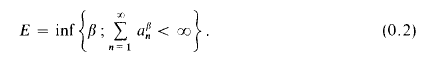
It is immediately clear from these definitions and our assumptions about an that

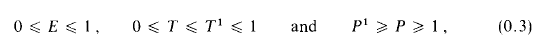
the latter since nan → 0 by Olivier's theorem [2, p. 124].

We define s-dimensional Hausdorff outer measure on subsets of ℝn by

Here | | denotes the diameter of a set. The Souslin sets, which include the Borel sets, are Hs-measurable for any s ≥ 0.

In [4] we initiated a study of K-Lusin sets. We characterized the K-Lusin sets in a Hausdorff space X as the sets that can be obtained as the image of some paracompact Čech complete space G, under a continuous injective map that maps discrete families in G to discretely σ-decomposable families in X [4, Theorem 2, p. 195]. Unfortunately, we cannot substantiate a second characterization of K-Lusin sets in completely regular spaces, given in the second part of Theorem 14 of [4].

This paper is concerned with the solution of the following interesting geometrical problem. For what set of n points on the sphere is the sum of all Euclidean distances between points maximal, and what is the maximum?

Our starting point is the following surprising “invariance principle” due to K. B. Stolarsky: The sum of the distances between points plus the quadratic average of a discrepancy type quantity is constant. Thus the sum of distances is maximized by a well distributed set of points. We now introduce some notation to make the statement more precise.

In July 1982, I was asked by Prof. Jorgen Hoffmann-Jorgensen to construct an uncountable compact set K in the line which was symmetric about 0 and had the property that, for all n, the set of sums of n-tuples from K has measure 0. There are two equivalent conditions: the set of such sums should never contain an interval, or K* ≠ ℝ, where K* is the subgroup of (ℝ, +) generated by K. I did so, and the set I constructed had entropy dimension 0 (and thus also Hausdorff dimension 0). Hoffmann-Jorgensen showed that every set of entropy dimension 0 would exhibit the same behaviour. However, I did not believe that the essence of the example lay in its dimension, and I here modify my construction so that the set K has dimension 1 (and thus also entropy dimension 1), while K* ≠ ℝ, as before. By contrast, the Cantor ternary set has dimension log3(2), but the set of differences is the interval [ –1, 1], so that it does generate ℝ. It follows that the property under consideration is arithmetical rather than dimensional.

It has been shown in [1, 2] that it is sometimes possible to justify the uncoupled and quasi-static approximations which are commonly invoked to simplify the solution of initial and boundary value problems in the linear theory of thermoelasticity. The justification involves showing, among other things, that the temperature predicted by the coupled dynamic theory is approximated by a solution of the classical heat equation.

A study is made of Stokes flows in which a line rotlet or stokeslet is in the presence of a circular cylinder in a viscous fluid. In contrast to the Stokes Paradox for flow past an isolated cylinder, it is shown that if either type of singularity, with suitably chosen strength and location, is present, there can exist a flow which is uniform at infinity. A similar phenomenon can occur when two equal cylinders rotate with equal and opposite angular velocities, and the flow pattern is then such that there is a closed streamline enclosing both cylinders.

It is shown that every compact convex set in with mean width equal to that of a line segment of length 2 and with Steiner point at the origin is contained in the unit ball. As a consequence, the diameter with respect to the Hausdorff metric of the space of all such sets is 1. There also results a sharp bound for the Hausdorff distance between any two compact convex sets.

We describe a toroidal polyhedral map which can be geometrically realized in R3 but not via a Schlegel diagram of a convex 4-polytope. Moreover, this map is not isomorphic to a subcomplex of the boundary complex of any convex polytope.

In this paper we investigate the p-periodicity of the S-arithmetic groups G = GL(n, Os(K)) and G1 = SL(n, Os(K)) where Os(K) is the ring of S-integers of a number field K (cf. [12, 13]; S is a finite set of places in K including the infinite places). These groups are known to be virtually of finite (cohomological) dimension, and thus the concept of p-periodicity is defined; it refers to a rational prime p and to the p-primary component Ĥi(G, A, p) of the Farrell-Tate cohomology Ĥi(G, A) with respect to an arbitrary G-module A. We recall that Ĥi coincides with the usual cohomology Hi for all i above the virtual dimension of G, and that in the case of a finite group (i.e., a group of virtual dimension zero) the Ĥi, i ∈ℤ, are the usual Tate cohomology groups. The group G is called p-periodic if Ĥi(G, A, p) is periodic in i, for all A, and the smallest corresponding period is then simply called the p-period of G. If G has no p-torsion, the p-primary component of all its Ĥi is 0, and thus G is trivially p-periodic.

In this paper we are concerned with upper bounds for the sums

where

and Δ2(n) is written simply as Δ(n). These functions were introduced by Hooley [4] and applied in a novel way to problems related to Waring's, and in Diophantine approximation. Thus Hooley deduced from his result about S2(x) that for any irrational θ, real γ, and fixed ε > 0, the inequality

holds for infinitely many n. His result for S3(x) led to a proof that

where r8(n) denotes the number of representations of n as the sum of eight positive cubes.

The following theorem is proved in [2[.

Let K be a finitely generated field over its prime field. Then for almost all e-tuples σ = (σ1, …, σe) of elements of the abstract Galois group G(K) of K and for all elliptic curves E defined over the following results hold.

Let denote the set of convex bodies of Ed, i.e. the set of all compact convex subsets of Ed. Let Bi be the unit i-ball, ωi its volume and Si-1 the unit sphere bd Bi. For an arbitrary denote the j-th quermassintegral (for definition and properties compare [7]). A different normalization of the functionals Wo, …, Wd leads to the intrinsic volumes Vj (which were introduced in [9]) defined by The intrinsic volumes are independent of the dimension of the space in which K is embedded. In particular, V0(K) = 1, Vd-1 (K) is half the surface area of K and Vd{K) = V(K) is its volume.

Given an integral lattice L and a hyperbolic decomposition of some quotient L/pL, there is a simple technique for obtaining other lattices of the same dimension and discriminant as L⊥ … ⊥L. When applied to the D4 and E8 root lattices, for example, this yields a new sphere packing in ℝ32, which is denser than those known up to now, and an extremal type II lattice in ℝ64.

Rather more than thirty years ago Erdős and Mirsky [2] asked whether there exist infinitely many integers n for which d(n) = d(n + 1). At one time it seemed that this might be as hard to resolve as the twin prime problem, see Vaughan [6] and Halberstam and Richert [3, pp. 268, 338]. The reasoning was roughly as follows. A natural way to arrange that d(n) = d(n + l) is to take n = 2p, where 2p + 1 = 3q, with p, q primes. However sieve methods yield only 2p + 1 = 3P2 (by the method of Chen [1]). To specify that P2 should be a prime q entails resolving the “parity problem” of sieve theory. Doing this would equally allow one to replace P2 by a prime in Chen's p + 2 = P2 result.

In a recent paper, Parson and Sheingorn [11[ gave some estimates for certain exponential sums associated with Ramanujan's τ-function and, in general, with the Fourier coefficients of the cusp forms for the full modular group which are eigenfunctions for the Hecke operators. The exponential sums considered in that paper are closely connected with the exponential sum

where α ∈ ℝ and e(θ) = e2πiθ, and the methods used in [11] go back essentially to Hardy-Littlewood [5], Hardy [4], Hecke [6], Wilton [19] and Walfisz [18].

Let

It is conjectured that for any k,

for some constant ck. It is well known that (2) holds for k = 0, 1 and 2 with c0 = 1, C1 = 1, and c2 = (2π2)-1, but there is not even a conjectural value of ck for any other k. However, it is known that the Riemann hypothesis implies

for all k ≥ 0 (see Ramachandra [2] and Heath-Brown [1]).