In this paper, we will denote by convex figure a compact convex subset of the n-dimensional Euclidean space ℝn, and by convex body a convex figure with non-empty interior. The principal kinematic formula in integral geometry gives the measure of the set of congruent convex bodies intersecting with a fixed convex body. Specifically, let K, L be two convex bodies in ℝn and G(n) the group of special motions in ℝn. Each element, g: ℝn → ℝn, of G(n) can be represented by

where b∈ℝn and e is an orthogonal matrix of determinant 1. Let μ be the Haar measure on G(n) normalized as follows: Let μ:ℝn × SO(n) → G(n) be defined by φ(t, e)x = ex + t, xεℝn, where SO(n) is the rotation group of ℝn. If v is the unique invariant probability measure on SO(n), η is the Lebesgue measure on ℝn, then μ is chosen as the pull back measure of η⊗v under φ−1. If Wi(K), Wi(L) are the quermassintegrals of K, L, i= 0, 1,…, n, the principal kinematic formula states that
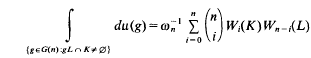
where ωn is the volume of the unit n–ball.