Research Article
The Hausdorff measure of the intersection of sets of positive Lebesgue measure
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 1-9
-
- Article
- Export citation
A problematic identity
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 10-12
-
- Article
- Export citation
A slow motion of viscous liquid caused by the rotation of a solid sphere
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 13-24
-
- Article
- Export citation
Plane elastostatic boundary value problems (I). Stresses in an elliptic plate
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 25-28
-
- Article
- Export citation
Plane elastostatic boundary value problems (II). The role of inversion
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 29-34
-
- Article
- Export citation
Plane elastostatic boundary value problems (III). Stresses in a parabolic mound
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 35-42
-
- Article
- Export citation
The Hilbert function of the tensor product of two multigraded modules
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 43-57
-
- Article
- Export citation
On a selection problem for a sequence of finite sets
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 58-63
-
- Article
- Export citation
Approximation of convex surfaces by algebraic surfaces
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 64-71
-
- Article
- Export citation
A note on the axisymmetric Stokes flow of viscous fluid past a spherical cap
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 72-78
-
- Article
- Export citation
On the embedding of complete graphs in orientable surfaces
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 79-83
-
- Article
- Export citation
Multiple packing of spherical caps
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 84-88
-
- Article
- Export citation
Front matter
MTK volume 10 issue 1 Front matter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. f1-f2
-
- Article
-
- You have access
- Export citation

 contains a perfect subset (and is therefore of power
contains a perfect subset (and is therefore of power 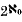 ). They asked for what Hausdorff measure functions
). They asked for what Hausdorff measure functions 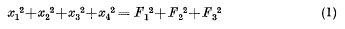
 be a set of points on a sphere, centre
be a set of points on a sphere, centre  . Let
. Let  provides a
provides a