Research Article
The determination of convex bodies from their mean radius of curvature functions
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 1-13
-
- Article
- Export citation
The large sieve
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 14-20
-
- Article
- Export citation
On the power free values of polynomials
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 21-26
-
- Article
- Export citation
Uniqueness theorems for linearized theories of interacting continua
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 27-42
-
- Article
- Export citation
Slow motion of an incompressible viscous liquid generated by the rotation of two spheres in contact
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 43-46
-
- Article
- Export citation
On the zeros of Epstein's zeta function
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 47-55
-
- Article
- Export citation
Nul solutions of the Kolmogorov differential equations
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 56-61
-
- Article
- Export citation
On the existence of non-atomic measures
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 62-67
-
- Article
- Export citation
Supersonic flow and wing-body interference
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 68-93
-
- Article
- Export citation
Classnumbers and unit signatures
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 94-98
-
- Article
- Export citation
A note on successive approximation
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 99-101
-
- Article
- Export citation
Linear forms in the logarithms of algebraic numbers (II)
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 102-107
-
- Article
- Export citation
On the Hankel determinants of univalent functions
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. 108-112
-
- Article
- Export citation
Front matter
MTK volume 14 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 26 February 2010, pp. f1-f4
-
- Article
-
- You have access
- Export citation

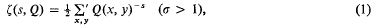
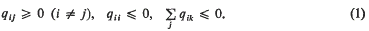
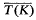 of
of 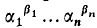 is transcendental.
is transcendental.