Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-27T04:56:37.905Z Has data issue: false hasContentIssue false
  • English
  • Français

WEIGHTED INEQUALITIES FOR MARTINGALE TRANSFORMS AND STOCHASTIC INTEGRALS

Part of: Stochastic processes Linear function spaces and their duals Harmonic analysis in several variables

Published online by Cambridge University Press:  20 February 2017

Adam Osȩkowski
Adam Osȩkowski*
Affiliation:
Department of Mathematics, Informatics and Mechanics, University of Warsaw, Banacha 2, 02-097 Warsaw, Poland email [email protected]
Get access

Abstract

The paper is devoted to the study of Fefferman–Stein inequalities for stochastic integrals. If 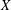$X$ is a martingale, 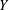$Y$ is the stochastic integral, with respect to 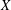$X$, of some predictable process taking values in $[-1,1]$, then for any weight 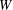$W$ belonging to the class 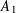$A_{1}$ we have the estimates 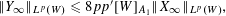$\Vert Y_{\infty }\Vert _{L^{p}(W)}\leqslant 8pp^{\prime }[W]_{A_{1}}\Vert X_{\infty }\Vert _{L^{p}(W)},$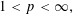$1<p<\infty ,$ and 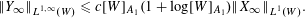$\Vert Y_{\infty }\Vert _{L^{1,\infty }(W)}\leqslant c[W]_{A_{1}}(1+\log [W]_{A_{1}})\Vert X_{\infty }\Vert _{L^{1}(W)}.$ The proofs rest on the Bellman function method: the inequalities are deduced from the existence of certain special functions, enjoying appropriate majorization and concavity. As an application, related statements for Haar multipliers are indicated. The above estimates can be regarded as probabilistic counterparts of the recent results of Lerner, Ombrosi and Pérez concerning singular integral operators.

MSC classification

Primary: 42B25: Maximal functions, Littlewood-Paley theory
Secondary: 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.) 60G44: Martingales with continuous parameter
Type
Research Article
Information
Mathematika , Volume 63 , Issue 2 , 2017 , pp. 433 - 450
Copyright
Copyright © University College London 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Buckley, S. M., Estimates for operator norms on weighted spaces and reverse Jensen inequalities. Trans. Amer. Math. Soc. 340(1) 1993, 253–272.CrossRefGoogle Scholar
Burkholder, D. L., Boundary value problems and sharp inequalities for martingale transforms. Ann. Probab. 12 1984, 647–702.Google Scholar
Dellacherie, C. and Meyer, P.-A., Probabilities and Potential B: Theory of Martingales, North-Holland (Amsterdam, 1982).Google Scholar
Fefferman, C. and Stein, E. M., Some maximal inequalities. Amer. J. Math. 93 1971, 107–115.Google Scholar
Izumisawa, M. and Kazamaki, N., Weighted norm inequalities for martingales. Tohoku Math. J. (2) 29 1977, 115–124.Google Scholar
Kazamaki, N., Continuous Exponential Martingales and BMO (Lecture Notes in Mathematics 1579 ), Springer (Berlin, 1994).CrossRefGoogle Scholar
Lerner, A. K., Ombrosi, S. and Pérez, C., Sharp A 1 bounds for Calderón–Zygmund operators and the relationship with a problem of Muckenhoupt and Wheeden. Int. Math. Res. Not. IMRN 6 2008, Art. ID rnm161, 11 p.Google Scholar
Lerner, A. K., Ombrosi, S. and Pérez, C., A 1 bounds for Calderón–Zygmund operators related to a problem of Muckenhoupt and Wheeden. Math. Res. Lett. 16 2009, 149–156.Google Scholar
Nazarov, F. L., Reznikov, A., Vasyunin, V. and Volberg, A., A Bellman function counterexample to the $A_{1}$ conjecture: the blow-up of the weak norm estimates of weighted singular operators. Preprint, 2015, arXiv:1506.04710.Google Scholar
Nazarov, F. L. and Treil, S. R., The hunt for a Bellman function: applications to estimates for singular integral operators and to other classical problems of harmonic analysis. St. Petersburg Math. J. 8 1997, 721–824.Google Scholar
Nazarov, F. L., Treil, S. R. and Volberg, A., The Bellman functions and two-weight inequalities for Haar multipliers. J. Amer. Math. Soc. 12 1999, 909–928.CrossRefGoogle Scholar
Obłój, J., The Skorokhod embedding problem and its offspring. Probab. Surv. 1 2004, 321–392.Google Scholar
Osȩkowski, A., Sharp Martingale and Semimartingale Inequalities (Monografie Matematyczne 72 ), Birkhäuser (Basel, 2012), 462 pp.Google Scholar
Petermichl, S. and Wittwer, J., A sharp estimate for the weighted Hilbert transform via Bellman functions. Michigan Math. J. 50 2002, 71–87.CrossRefGoogle Scholar
Reguera, M. C., On Muckenhoupt–Wheeden conjecture. Adv. Math. 227(4) 2011, 1436–1450.CrossRefGoogle Scholar
Reguera, M. C. and Thiele, C., The Hilbert transform does not map L 1(Mw) to L 1, ∞ (w). Math. Res. Lett. 19(1) 2012, 1–7.Google Scholar
Slavin, L. and Vasyunin, V., Sharp results in the integral-form John–Nirenberg inequality. Trans. Amer. Math. Soc. 363 2011, 4135–4169.CrossRefGoogle Scholar
Vasyunin, V. and Volberg, A., Monge–Ampére equation and Bellman optimization of Carleson Embedding Theorems. In Linear and Complex Analysis (American Mathematical Society Translations (2) 226 ), American Mathematical Society (Providence, RI, 2009), 195–238.Google Scholar