Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-27T06:40:18.863Z Has data issue: false hasContentIssue false
  • English
  • Français

MINIMIZING DIRICHLET EIGENVALUES ON CUBOIDS OF UNIT MEASURE

Part of: Elliptic equations and systems Spectral theory and eigenvalue problems

Published online by Cambridge University Press:  13 March 2017

M. van den Berg  and
K. Gittins
M. van den Berg
Affiliation:
School of Mathematics, University of Bristol, University Walk, Bristol BS8 1TW, U.K. email [email protected]
K. Gittins
Affiliation:
Institut de mathématiques, Rue Emile-Argand 11, 2000 Neuchâtel, Switzerland email [email protected]
Get access

Abstract

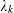We consider the minimization of Dirichlet eigenvalues $\unicode[STIX]{x1D706}_{k}$, 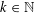$k\in \mathbb{N}$, of the Laplacian on cuboids of unit measure in $\mathbb{R}^{3}$. We prove that any sequence of optimal cuboids in 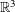$\mathbb{R}^{3}$ converges to a cube of unit measure in the sense of Hausdorff as 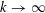$k\rightarrow \infty$. We also obtain an upper bound for that rate of convergence.

MSC classification

Primary: 35J20: Variational methods for second-order elliptic equations 35P99: None of the above, but in this section
Type
Research Article
Information
Mathematika , Volume 63 , Issue 2 , 2017 , pp. 469 - 482
Copyright
Copyright © University College London 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antunes, P. R. S. and Freitas, P., Numerical optimisation of low eigenvalues of the Dirichlet and Neumann Laplacians. J. Optim. Theory Appl. 154 2012, 235–257.Google Scholar
Antunes, P. R. S. and Freitas, P., Optimal spectral rectangles and lattice ellipses. Proc. R. Soc. Lond. A 469 2013, 20120492.Google Scholar
Antunes, P. R. S. and Freitas, P., Optimisation of eigenvalues of the Dirichlet Laplacian with a surface area restriction. Appl. Math. Optim. 73 2016, 313–328.Google Scholar
van den Berg, M., On the minimization of Dirichlet eigenvalues. Bull. Lond. Math. Soc. 47 2015, 143–155.Google Scholar
van den Berg, M. and Iversen, M., On the minimization of Dirichlet eigenvalues of the Laplace operator. J. Geom. Anal. 23 2013, 660–676.Google Scholar
Berger, A., The eigenvalues of the Laplacian with Dirichlet boundary condition in ℝ2 are almost never minimized by disks. Ann. Global Anal. Geom. 47 2015, 285–304.Google Scholar
Bucur, D., Minimization of the kth eigenvalue of the Dirichlet Laplacian. Arch. Ration. Mech. Anal. 206 2012, 1073–1083.Google Scholar
Bucur, D., Buttazzo, G. and Henrot, A., Minimization of 𝜆2(𝛺) with a perimeter constraint. Indiana Univ. Math. J. 58 2009, 2709–2728.CrossRefGoogle Scholar
Bucur, D. and Freitas, P., Asymptotic behaviour of optimal spectral planar domains with fixed perimeter. J. Math. Phys. 54 2013, 053504.Google Scholar
Chamizo, F. and Pastor, C., Lattice points in elliptic paraboloids, Preprint, 2016, arXiv:1611.04498v1.Google Scholar
Colbois, B. and El Soufi, A., Extremal eigenvalues of the Laplacian on Euclidean domains and closed surfaces. Math. Z. 278 2014, 529–546.Google Scholar
De Philippis, G. and Velichkov, B., Existence and regularity of minimizers for some spectral functionals with perimeter constraint. Appl. Math. Optim. 69 2014, 199–231.Google Scholar
Gradshteyn, I. S. and Ryzhik, I. M., Table of Integrals, Series and Products, Elsevier/Academic Press (Amsterdam, 2007).Google Scholar
Heath-Brown, D. R., Lattice points in the sphere. In Number Theory in Progress, Vol. 2 (Zakopane-Koscielisko, 1997), de Gruyter (Berlin, 1999), 883–892.Google Scholar
Henrot, A., Extremum problems for eigenvalues of elliptic operators. In Frontiers in Mathematics, Birkhäuser (Basel, 2006).Google Scholar
Huxley, M. N., Exponential sums and lattice points III. Proc. Lond. Math. Soc. (3) 87 2003, 591–609.Google Scholar
Mazzoleni, D. and Pratelli, A., Existence of minimizers for spectral problems. J. Math. Pures Appl. 100 2013, 433–453.Google Scholar
Niven, I., Zuckerman, H. S. and Montgomery, H. L., An Introduction to the Theory of Numbers, Wiley (New York, 1991).Google Scholar
Pólya, G., On the eigenvalues of vibrating membranes. Proc. Lond. Math. Soc. (3) 11 1961, 419–433.Google Scholar
Urakawa, H., Lower bounds for the eigenvalues of the fixed vibrating membrane problems. Tohoku Math. J. (2) 36 1984, 185–189.Google Scholar
Wolf, S. A. and Keller, J. B., Range of the first two eigenvalues of the Laplacian. Proc. R. Soc. Lond. A 447 1994, 397–412.Google Scholar