Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Matsumoto, Kohji
Nawashiro, Akihiko
and
Tsumura, Hirofumi
2021.
Double Dirichlet series associated with arithmetic functions.
Kodai Mathematical Journal,
Vol. 44,
Issue. 3,
Matsumoto, Kohji
2021.
A Survey on the Theory of Multiple Dirichlet Series with Arithmetical Coefficients as Numerators.
Lithuanian Mathematical Journal,
Vol. 61,
Issue. 3,
p.
391.
Cantarini, Marco
Gambini, Alessandro
and
Zaccagnini, Alessandro
2021.
Cesàro averages for Goldbach representations with summands in arithmetic progressions.
International Journal of Number Theory,
Vol. 17,
Issue. 10,
p.
2379.
Bhowmik, Gautami
and
Halupczok, Karin
2021.
Combinatorial and Additive Number Theory IV.
Vol. 347,
Issue. ,
p.
25.
Inoue, Shōta
2021.
Some explicit formulas for partial sums of Möbius functions.
Journal de Théorie des Nombres de Bordeaux,
Vol. 33,
Issue. 2,
p.
273.
Friedlander, J.B.
Goldston, D.A.
Iwaniec, H.
and
Suriajaya, A.I.
2022.
Exceptional zeros and the Goldbach problem.
Journal of Number Theory,
Vol. 233,
Issue. ,
p.
78.
Bauer, C.
2022.
A Montgomery–Hooley theorem for the number of Goldbach representations.
Acta Mathematica Hungarica,
Vol. 168,
Issue. 1,
p.
95.
Bauer, Claus
2022.
The Barban–Davenport–Halberstam theorem for a restricted set of arithmetic progressions.
International Journal of Number Theory,
Vol. 18,
Issue. 09,
p.
2075.
Martin, Kimball
2022.
Refined Goldbach Conjectures with Primes in Progressions.
Experimental Mathematics,
Vol. 31,
Issue. 1,
p.
226.
Jia, Chao Hua
2022.
On the Conditional Bounds for Siegel Zeros.
Acta Mathematica Sinica, English Series,
Vol. 38,
Issue. 5,
p.
869.
Friedlander, John B.
and
Iwaniec, Henryk
2022.
Exceptional zeros, sieve parity, Goldbach.
Essential Number Theory,
Vol. 1,
Issue. 1,
p.
13.
GOLDSTON, DANIEL A.
and
SURIAJAYA, ADE IRMA
2023.
ON AN AVERAGE GOLDBACH REPRESENTATION FORMULA OF FUJII.
Nagoya Mathematical Journal,
Vol. 250,
Issue. ,
p.
511.
Goldston, Daniel A.
and
Suriajaya, Ade Irma
2023.
Number Theory in Memory of Eduard Wirsing.
p.
145.
Matsumoto, Kohji
and
Tsumura, Hirofumi
2023.
Double Dirichlet series associated with arithmetic functions II.
Kodai Mathematical Journal,
Vol. 46,
Issue. 1,
Ikeda, Karin
and
Suriajaya, Ade Irma
2025.
The average number of Goldbach representations over multiples of $q$.
Functiones et Approximatio Commentarii Mathematici,
Vol. -1,
Issue. -1,
Billington, Keith
Cheng, Maddie
Schettler, Jordan
and
Irma Suriajaya, Ade
2025.
The average number of Goldbach representations and Zero-free regions of the Riemann zeta function.
International Journal of Number Theory,
Vol. 21,
Issue. 02,
p.
289.
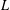 $L$-functions we get asymptotic results on the average number of representations of an integer as the sum of two primes in arithmetic progression. On the other hand the existence of good error terms gives information on the location of zeros of
$L$-functions we get asymptotic results on the average number of representations of an integer as the sum of two primes in arithmetic progression. On the other hand the existence of good error terms gives information on the location of zeros of 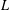 $L$-functions. Similar results are obtained for an integer in a congruence class expressed as the sum of two primes.
$L$-functions. Similar results are obtained for an integer in a congruence class expressed as the sum of two primes.

