No CrossRef data available.
Published online by Cambridge University Press: 26 February 2010
The main aim of this note is the proof of the following
Let −∞ ≤ a > b ≤ ∞ and let A ⊂ (a, b) be a measurable set such that λ((a, b)\A) = 0, where λ denotes Lebesgue measure on ℝ. Let f: A→ℝ be a measurable and midconvex function, i.e.

whenever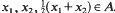 . Then there exists a convex function
. Then there exists a convex function such that
such that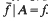 .
.