Published online by Cambridge University Press: 26 February 2010
Let 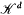 denote the set of convex bodies of Ed, i.e. the set of all compact convex subsets of Ed. Let Bi be the unit i-ball, ωi its volume and Si-1 the unit sphere bd Bi. For an arbitrary
denote the set of convex bodies of Ed, i.e. the set of all compact convex subsets of Ed. Let Bi be the unit i-ball, ωi its volume and Si-1 the unit sphere bd Bi. For an arbitrary  denote the j-th quermassintegral (for definition and properties compare [7]). A different normalization of the functionals Wo, …, Wd leads to the intrinsic volumes Vj (which were introduced in [9]) defined by
denote the j-th quermassintegral (for definition and properties compare [7]). A different normalization of the functionals Wo, …, Wd leads to the intrinsic volumes Vj (which were introduced in [9]) defined by 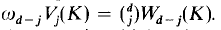 The intrinsic volumes are independent of the dimension of the space in which K is embedded. In particular, V0(K) = 1, Vd-1 (K) is half the surface area of K and Vd{K) = V(K) is its volume.
The intrinsic volumes are independent of the dimension of the space in which K is embedded. In particular, V0(K) = 1, Vd-1 (K) is half the surface area of K and Vd{K) = V(K) is its volume.