Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hickman, Jonathan
and
Wright, James
2023.
A Non-archimedean Variant of Littlewood–Paley Theory for Curves.
The Journal of Geometric Analysis,
Vol. 33,
Issue. 3,


 where
where 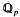 . Then
. Then 