The packing of certain sets of cubes

Extract

Let (X₁, …, X_n) be the coordinates of the centre of a unit cube C, in n-dimensional space, whose (n − 1)-dimensional faces are parallel to the axes of coordinates. Further let the X's be integers. Let ε_i = ± 1 for r (≤ n) values of i, 1 ≤ i ≤ n, and let ε_i = 0 for the remaining n − r values of i. Then (X₁ + ε₁, …, X_n + ε_n) gives the centre of a cube C′, which touches the cube C along an (n − r)-dimensional edge or face. The cube C and the cubes C′, for all possible arrangements of the ε's, which are subject to the above conditions, form a symmetrical arrangement of 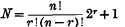cubes. This paper discusses the possibility of completely filling space by means of the packing together of such sets of cubes.