Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T01:36:04.426Z Has data issue: false hasContentIssue false
  • English
  • Français

Maximal one-sided ideals in Banach algebras

Published online by Cambridge University Press:  24 October 2008

Michael D. Green
Michael D. Green
Affiliation:
University of Newcastle-upon-Tyne
Get access Rights & Permissions [Opens in a new window]

Extract

In (1) Akemann and Rosenfeld introduced a property for Banach algebras which they called (*). A Banach algebra satisfies (*) if every maximal one-sided ideal in is closed. They proved that certain classes of Banach algebra with 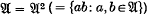satisfy (*), and they mentioned a conjecture that if is a Banach algebra with 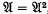, then satisfies (*). In this paper we show that, if is a Banach algebra with a bounded right (left) approximate identity, then maximal left (right) ideals in are closed, and we give a counter-example to the above conjecture. We also give an independent proof that C*-algebras satisfy (*).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 80 , Issue 1 , July 1976 , pp. 109 - 111
Copyright
Copyright © Cambridge Philosophical Society 1976

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Axemann, C. A. and Rosenfeld, M.Maximal one-sided ideals in operator algebras. Amer. J. Math. 94 (1972), 723–728.CrossRefGoogle Scholar
(2)Bonsall, F. F. and Duncan, J.Complete Wormed algebras (Springer-Verlag, Berlin, Heidelberg, New York, 1973).CrossRefGoogle Scholar
(3)Combes, F.Quelques propriétes des C*-algèbres. Bull. Sci. Math. 2e Série 94 (1970), 165–192.Google Scholar
(4)Dixmier, J.Les algèbres d'opérateurs dans l'espace hilbertien (algèbres de von Neumann) 2e edition (Gauthier-Villars, Paris, 1969).Google Scholar
(5)Hewitt, E. and Ross, K. A.Abstract harmonic analysis II (Springer-Verlag, Berlin, Heidelberg, New York, 1970).Google Scholar
(6)Rickart, C. E.General theory of Banach algebras (Van Nostrand, Princeton, 1960).Google Scholar