Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hartley, B.
1972.
Some examples of locally finite groups.
Archiv der Mathematik,
Vol. 23,
Issue. 1,
p.
225.
Tretkoff, Carol
1976.
Some remarks on just-inpinitb groups.
Communications in Algebra,
Vol. 4,
Issue. 5,
p.
483.
Wells, Charles
1976.
Some Applications of the Wreath Product Construction.
The American Mathematical Monthly,
Vol. 83,
Issue. 5,
p.
317.
Pride, Stephen J.
1979.
On groups of finite height.
Journal of the Australian Mathematical Society,
Vol. 28,
Issue. 1,
p.
87.
Pride, Stephen J.
1980.
WORD PROBLEMS II.
Vol. 95,
Issue. ,
p.
299.
Edjvet, M.
and
Pride, Stephen J.
1984.
Groups — Korea 1983.
Vol. 1098,
Issue. ,
p.
29.
Kurdachenko, L. A.
and
Pylaev, V. V.
1984.
Groups with noncyclic subgroups of finite index.
Ukrainian Mathematical Journal,
Vol. 35,
Issue. 4,
p.
372.
Kurdachenko, L. A.
and
Pylaev, V. V.
1990.
Groups with minimax factor groups.
Ukrainian Mathematical Journal,
Vol. 42,
Issue. 5,
p.
550.
Derrick, A.J.
1994.
Groups with no nontrivial linear representations.
Bulletin of the Australian Mathematical Society,
Vol. 50,
Issue. 1,
p.
1.
Kalashnikova, N. V.
1998.
Groups all proper quotient groups of which possess layer-Chernikov properties.
Ukrainian Mathematical Journal,
Vol. 50,
Issue. 11,
p.
1710.
Kurdachenko, L. A.
and
Subbotin, I. Y.
1998.
Groups whose proper quotients are hypercentral.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 65,
Issue. 2,
p.
224.
Otal, J.
and
Kurdachenko, L. A.
1999.
Some noetherian modules and non-monolithic just non-CC-groups.
Journal of Group Theory,
Vol. 2,
Issue. 1,
Kurdarhenko, L.A.
and
Subbotin, I.Ya
2000.
Groups with many periodic factor-groups.
Communications in Algebra,
Vol. 28,
Issue. 3,
p.
1593.
Wilson, John S.
2000.
New Horizons in pro-p Groups.
p.
181.
Grigorchuk, R. I.
2000.
New Horizons in pro-p Groups.
p.
121.
Григорчук, Ростислав Иванович
and
Grigorchuk, Rostislav Ivanovich
2000.
Ветвящиеся группы.
Математические заметки,
Vol. 67,
Issue. 6,
p.
852.
Kurdachenko, L. A.
and
Otal, J.
2000.
Groups all proper quotient groups of which have Chernikov conjugacy classes.
Ukrainian Mathematical Journal,
Vol. 52,
Issue. 3,
p.
400.
Grigorchuk, R. I.
2000.
Branch groups.
Mathematical Notes,
Vol. 67,
Issue. 6,
p.
718.
Abért, Miklós
and
Virág, Bálint
2004.
Dimension and randomness in groups acting on rooted trees.
Journal of the American Mathematical Society,
Vol. 18,
Issue. 1,
p.
157.
Rémy, Bertrand
2005.
Integrability of induction cocycles for Kac-Moody groups.
Mathematische Annalen,
Vol. 333,
Issue. 1,
p.
29.
 for the class of groups all of whose non-trivial normal subgroups have finite index. Thus, rather obviously, finite groups, simple groups, and the infinite cyclic and dihedral groups all lie in the class
for the class of groups all of whose non-trivial normal subgroups have finite index. Thus, rather obviously, finite groups, simple groups, and the infinite cyclic and dihedral groups all lie in the class  . Other examples of
. Other examples of  -groups are to be found in a variety of contexts. The main result of Mennicke (8) shows that, for n ≥ 3, the factor group of SL(n, Z) by its centre is a
-groups are to be found in a variety of contexts. The main result of Mennicke (8) shows that, for n ≥ 3, the factor group of SL(n, Z) by its centre is a  -group, where SL(n, Z) denotes the unimodular group of all n × n invertible matrices with integer coefficients and with determinant 1. In McLain(7), an example is given of an infinite, locally finite, locally soluble
-group, where SL(n, Z) denotes the unimodular group of all n × n invertible matrices with integer coefficients and with determinant 1. In McLain(7), an example is given of an infinite, locally finite, locally soluble  -group, whose only non-trivial normal subgroups are the terms of its derived series, and in (4), P. Hall discusses an infinite
-group, whose only non-trivial normal subgroups are the terms of its derived series, and in (4), P. Hall discusses an infinite 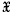 -group, all of whose proper quotients are finite p-groups. If
-group, all of whose proper quotients are finite p-groups. If  is a class of groups closed under taking homomorphic images, it is easily seen that the existence of an infinite
is a class of groups closed under taking homomorphic images, it is easily seen that the existence of an infinite 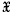 -group satisfying the maximal condition for normal subgroups implies the existence of an infinite
-group satisfying the maximal condition for normal subgroups implies the existence of an infinite 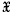 -group in the class
-group in the class  , so that certain questions concerning the finiteness of groups satisfying the maximal condition for normal subgroups can be interpreted as questions about
, so that certain questions concerning the finiteness of groups satisfying the maximal condition for normal subgroups can be interpreted as questions about  -groups.
-groups.